Detection Optimization of an Optically Trapped Microparticle in Vacuum with Kalman Filter
Abstract
:1. Introduction
2. Preliminaries
2.1. Counter-Propagating Dual-Beams Optical Trap
2.2. System Model
2.3. Kalman Filter
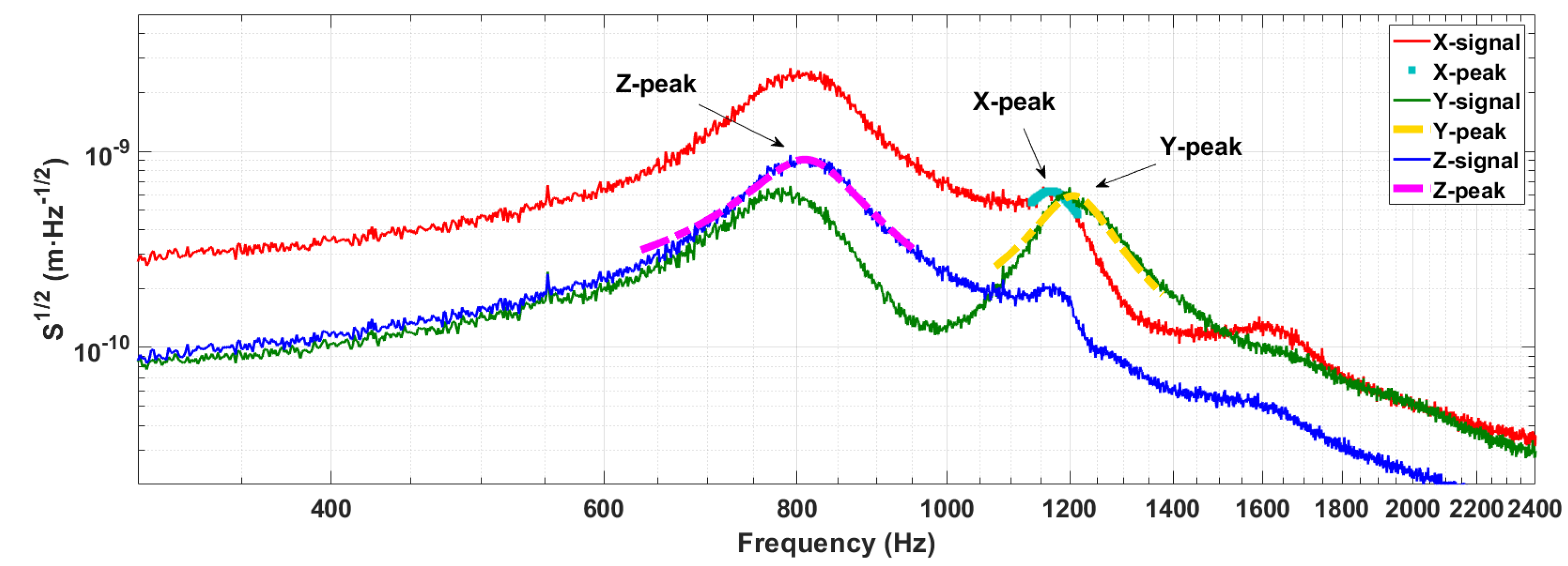
3. Simulation and Results
3.1. Simulink Setup
3.2. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| COM | Center of Mass |
| PID | Proportional Integral Derivative |
| AOM | Acoustic Optical Modulator |
| RMSE | Root Mean Square Error |
| FPGA | Field-programmable Gate Array |
References
- Ashkin, A. Acceleration and Trapping of Particles by Radiation Pressure. Phys. Rev. Lett. 1970, 24, 156–159. [Google Scholar] [CrossRef]
- Kotnala, A.; DePaoli, D.; Gordon, R. Sensing nanoparticles using a double nanohole optical trap. Lab Chip 2013, 13, 4142–4146. [Google Scholar] [CrossRef]
- Gordon, R. Future Prospects for Biomolecular Trapping with Nanostructured Metals. ACS Photonics 2022, 9, 1127–1135. [Google Scholar] [CrossRef]
- Brunetti, G.; Sasanelli, N.; Armenise, M.N.; Ciminelli, C. Nanoscale Optical Trapping by Means of Dielectric Bowtie. Photonics 2022, 9, 425. [Google Scholar] [CrossRef]
- Shen, K.; Duan, Y.; Ju, P.; Xu, Z.; Chen, X.; Zhang, L.; Ahn, J.; Ni, X.; Li, T. On-chip optical levitation with a metalens in vacuum. Optica 2021, 8, 1359–1362. [Google Scholar] [CrossRef]
- Donato, M.G.; Patti, F.; Saija, R.; Iatì, M.A.; Gucciardi, P.G.; Pedaci, F.; Strangi, G.; Maragò, O.M. Improved backscattering detection in photonic force microscopy near dielectric surfaces with cylindrical vector beams. J. Quant. Spectrosc. Radiat. Transf. 2021, 258, 107381. [Google Scholar] [CrossRef]
- Bustamante, C.J.; Chemla, Y.R.; Liu, S.; Wang, M.D. Optical tweezers in single-molecule biophysics. Nat. Rev. Methods Primers 2021, 1, 1–29. [Google Scholar] [CrossRef]
- Peng, P.W.; Yang, J.C.; Colley, M.M.; Yang, T.S. An Optical Tweezers-Based Single-Cell Manipulation and Detection Platform for Probing Real-Time Cancer Cell Chemotaxis and Response to Tyrosine Kinase Inhibitor PD153035. Photonics 2021, 8, 533. [Google Scholar] [CrossRef]
- Lin, S.; Crozier, K.B. Trapping-Assisted Sensing of Particles and Proteins Using On-Chip Optical Microcavities. ACS Nano 2013, 7, 1725–1730. [Google Scholar] [CrossRef]
- Li, T.; Kheifets, S.; Medellin, D.; Raizen, M.G. Measurement of the Instantaneous Velocity of a Brownian Particle. Science 2010, 328, 1673–1675. [Google Scholar] [CrossRef] [Green Version]
- Li, T.; Kheifets, S.; Raizen, M.G. Millikelvin cooling of an optically trapped microsphere in vacuum. Nat. Phys. 2011, 7, 527–530. [Google Scholar] [CrossRef]
- Monteiro, F.; Li, W.; Afek, G.; Li, C.L.; Mossman, M.; Moore, D.C. Force and acceleration sensing with optically levitated nanogram masses at microkelvin temperatures. Phys. Rev. A 2020, 101, 053835. [Google Scholar] [CrossRef]
- Ranjit, G.; Atherton, D.P.; Stutz, J.H.; Cunningham, M.; Geraci, A.A. Attonewton force detection using microspheres in a dual-beam optical trap in high vacuum. Phys. Rev. A 2015, 91, 051805. [Google Scholar] [CrossRef]
- Ranjit, G.; Cunningham, M.; Casey, K.; Geraci, A.A. Zeptonewton force sensing with nanospheres in an optical lattice. Phys. Rev. A 2016, 93, 053801. [Google Scholar] [CrossRef]
- Rider, A.D.; Blakemore, C.P.; Gratta, G.; Moore, D.C. Single-beam dielectric-microsphere trapping with optical heterodyne detection. Phys. Rev. A 2018, 97, 013842. [Google Scholar] [CrossRef]
- Gonzalez-Ballestero, C.; Aspelmeyer, M.; Novotny, L.; Quidant, R.; Romero-Isart, O. Levitodynamics: Levitation and control of microscopic objects in vacuum. Science 2021, 374, eabg3027. [Google Scholar] [CrossRef]
- Volpe, G.; Maragò, O.M.; Rubinzstein-Dunlop, H.; Pesce, G.; Stilgoe, A.B.; Volpe, G.; Tkachenko, G.; Truong, V.G.; Chormaic, S.N.; Kalantarifard, F.; et al. Roadmap for Optical Tweezers. arXiv 2022, arXiv:2206.13789. [Google Scholar]
- Blakemore, C.P.; Fieguth, A.; Kawasaki, A.; Priel, N.; Martin, D.; Rider, A.D.; Wang, Q.; Gratta, G. Search for non-Newtonian interactions at micrometer scale with a levitated test mass. Phys. Rev. D 2021, 104, L061101. [Google Scholar] [CrossRef]
- Kawasaki, A.; Fieguth, A.; Priel, N.; Blakemore, C.P.; Martin, D.; Gratta, G. High sensitivity, levitated microsphere apparatus for short-distance force measurements. Rev. Sci. Instrum. 2020, 91, 083201. [Google Scholar] [CrossRef]
- Moore, D.C.; Geraci, A.A. Searching for new physics using optically levitated sensors. Quantum Sci. Technol. 2021, 6, 014008. [Google Scholar] [CrossRef]
- Carney, D.; Krnjaic, G.; Moore, D.C.; Regal, C.A.; Afek, G.; Bhave, S.; Brubaker, B.; Corbitt, T.; Cripe, J.; Crisosto, N.; et al. Mechanical quantum sensing in the search for dark matter. Quantum Sci. Technol. 2021, 6, 024002. [Google Scholar] [CrossRef]
- Taylor, M.A.; Bowen, W.P. A computational tool to characterize particle tracking measurements in optical tweezers. J. Opt. 2013, 15, 085701. [Google Scholar] [CrossRef]
- Zhu, X.; Li, N.; Yang, J.; Chen, X.; Hu, H. Displacement Detection Decoupling in Counter-Propagating Dual-Beams Optical Tweezers with Large-Sized Particle. Sensors 2020, 20, 4916. [Google Scholar] [CrossRef] [PubMed]
- Kalman, R.E. Contributions to the theory of optimal control. Bol. Soc. Mat. Mex. 1960, 5, 102–119. [Google Scholar]
- Kalman, R.E. A New Approach to Linear Filtering and Prediction Problems. J. Basic Eng. 1960, 82, 35–45. [Google Scholar] [CrossRef]
- Sandhu, F.; Selamat, H.; Alavi, S.; Mahalleh, V.B.S. FPGA-based implementation of Kalman filter for real-time estimation of tire velocity and acceleration. IEEE Sens. J. 2017, 17, 5749–5758. [Google Scholar] [CrossRef]
- Arroyo-Marioli, F.; Bullano, F.; Kucinskas, S.; Rondón-Moreno, C. Tracking R of COVID-19: A new real-time estimation using the Kalman filter. PLoS ONE 2021, 16, e0244474. [Google Scholar] [CrossRef]
- Li, Y.; Yin, G.; Zhuang, W.; Zhang, N.; Wang, J.; Geng, K. Compensating Delays and Noises in Motion Control of Autonomous Electric Vehicles by Using Deep Learning and Unscented Kalman Predictor. IEEE Trans. Syst. Man Cybern. Syst. 2020, 50, 4326–4338. [Google Scholar] [CrossRef]
- Wieczorek, W.; Hofer, S.G.; Hoelscher-Obermaier, J.; Riedinger, R.; Hammerer, K.; Aspelmeyer, M. Optimal State Estimation for Cavity Optomechanical Systems. Phys. Rev. Lett. 2015, 114, 223601. [Google Scholar] [CrossRef]
- Setter, A.; Toroš, M.; Ralph, J.; Ulbricht, H. Real-Time Kalman Filter: Cooling of an Optically Levitated Nanoparticle. Phys. Rev. A 2017, 97, 033822. [Google Scholar] [CrossRef]
- Jost, M.; Schaffner, M.; Magno, M.; Korb, M.; Benini, L.; Reimann, R.; Jain, V.; Grossi, M.; Militara, A.; Frimmer, M.; et al. An accurate system for optimal state estimation of a levitated nanoparticle. In Proceedings of the 2018 IEEE Sensors Applications Symposium (SAS), Seoul, Korea, 12–14 March 2018; pp. 1–6. [Google Scholar]
- Liao, J.; Jost, M.; Schaffner, M.; Magno, M.; Korb, M.; Benini, L.; Tebbenjohanns, F.; Reimann, R.; Jain, V.; Gross, M.; et al. FPGA Implementation of a Kalman-Based Motion Estimator for Levitated Nanoparticles. IEEE Trans. Instrum. Meas. 2019, 68, 2374–2386. [Google Scholar] [CrossRef]
- Magrini, L.; Rosenzweig, P.; Bach, C.; Deutschmann-Olek, A.; Hofer, S.G.; Hong, S.; Kiesel, N.; Kugi, A.; Aspelmeyer, M. Real-time optimal quantum control of mechanical motion at room temperature. Nature 2021, 595, 373–377. [Google Scholar] [CrossRef] [PubMed]
- Li, T. Fundamental Tests of Physics with Optically Trapped Microspheres; Springer: New York, NY, USA, 2013. [Google Scholar] [CrossRef]
- Zeng, X.; Zhang, B.; Han, X.; Chen, Z.; Xiong, W.; Chen, X.; Xiao, G.; Luo, H. Time delay remaining in the displacement detection of the optically trapped particles using Kalman filter. In Proceedings of the Third International Conference on Optoelectronic Science and Materials (ICOSM 2021), Hefei, China, 10–12 September 2021; Chen, S., Wang, P., Eds.; SPIE: Hefei, China, 2021; p. 13. [Google Scholar]
- Ahn, J.; Xu, Z.; Bang, J.; Ju, P.; Gao, X.; Li, T. Ultrasensitive torque detection with an optically levitated nanorotor. Nat. Nanotechnol. 2020, 15, 89–93. [Google Scholar] [CrossRef] [PubMed] [Green Version]




| System Parameter | Value |
|---|---|
| Resonance frequency of z-axis () | Hz |
| Resonance frequency of x-axis () | Hz |
| Resonance frequency of y-axis () | Hz |
| Diameter (D) | 10 m |
| Density () | kg/m |
| Particle mass (M) | kg |
| Pressure (P) | 1 mBar |
| Damping () | Hz |
| Sampling frequency () | Hz |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, S.; Chen, M.; Yang, J.; Chen, X.; Li, N.; Hu, H. Detection Optimization of an Optically Trapped Microparticle in Vacuum with Kalman Filter. Photonics 2022, 9, 700. https://doi.org/10.3390/photonics9100700
Xu S, Chen M, Yang J, Chen X, Li N, Hu H. Detection Optimization of an Optically Trapped Microparticle in Vacuum with Kalman Filter. Photonics. 2022; 9(10):700. https://doi.org/10.3390/photonics9100700
Chicago/Turabian StyleXu, Shidong, Ming Chen, Jianyu Yang, Xingfan Chen, Nan Li, and Huizhu Hu. 2022. "Detection Optimization of an Optically Trapped Microparticle in Vacuum with Kalman Filter" Photonics 9, no. 10: 700. https://doi.org/10.3390/photonics9100700
APA StyleXu, S., Chen, M., Yang, J., Chen, X., Li, N., & Hu, H. (2022). Detection Optimization of an Optically Trapped Microparticle in Vacuum with Kalman Filter. Photonics, 9(10), 700. https://doi.org/10.3390/photonics9100700







