Generation of Elliptic-Symmetry Radially Polarized Optical Beam by Circle-Cassinian Optical Coordinate Transformation
Abstract
:1. Introduction
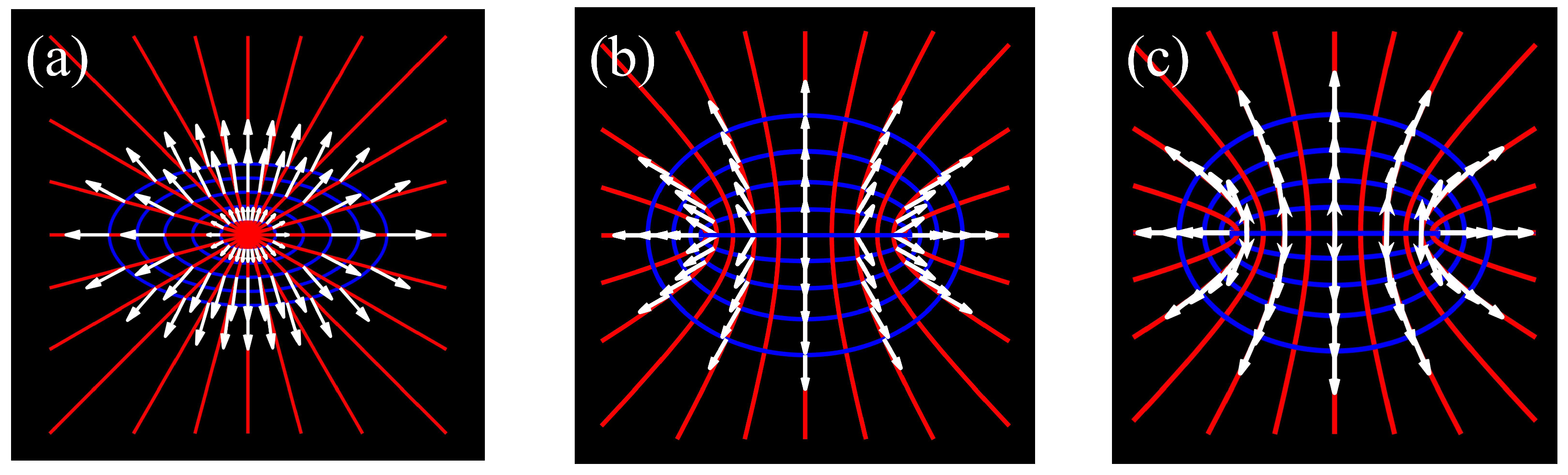
2. Polarization Structure of ESRP Beam
3. Principle of Using Circle-Cassinian Transformation to Obtain ESRP Beam
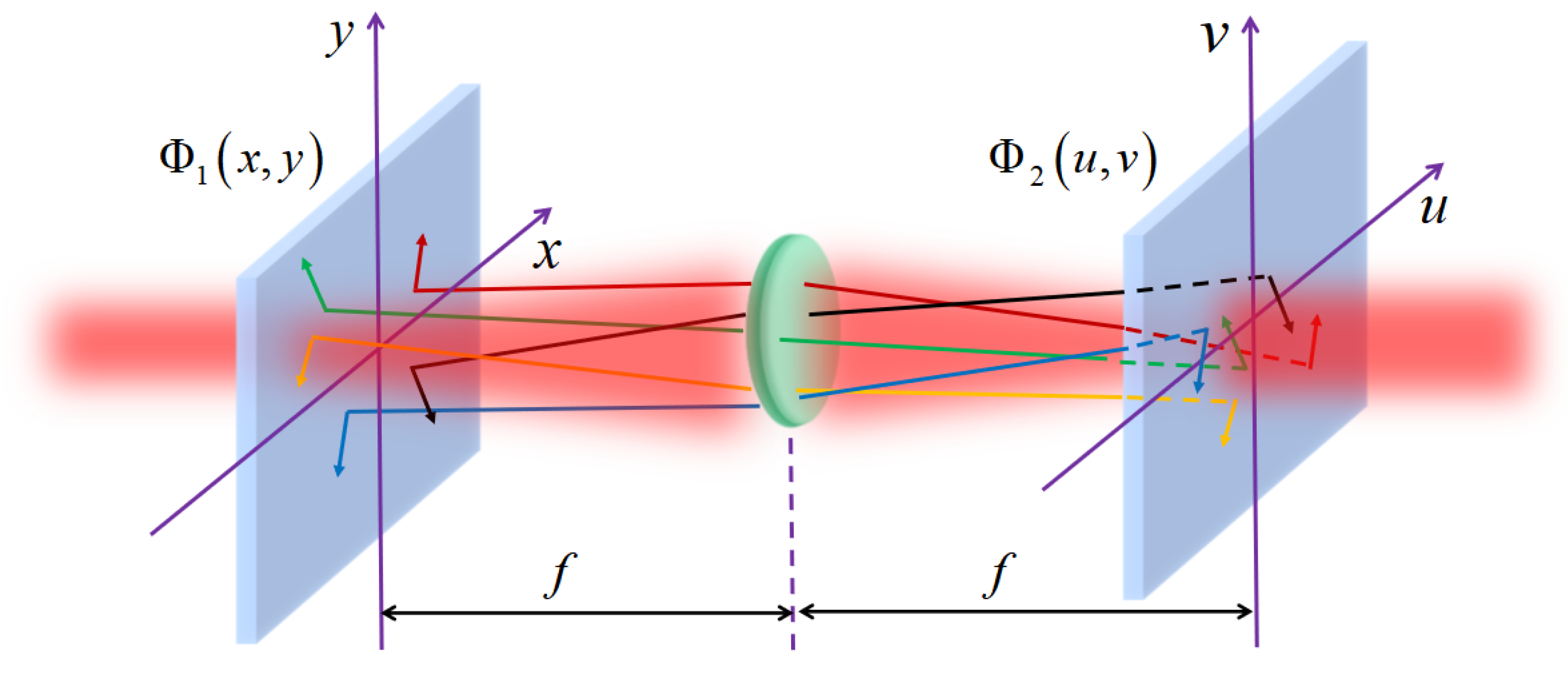
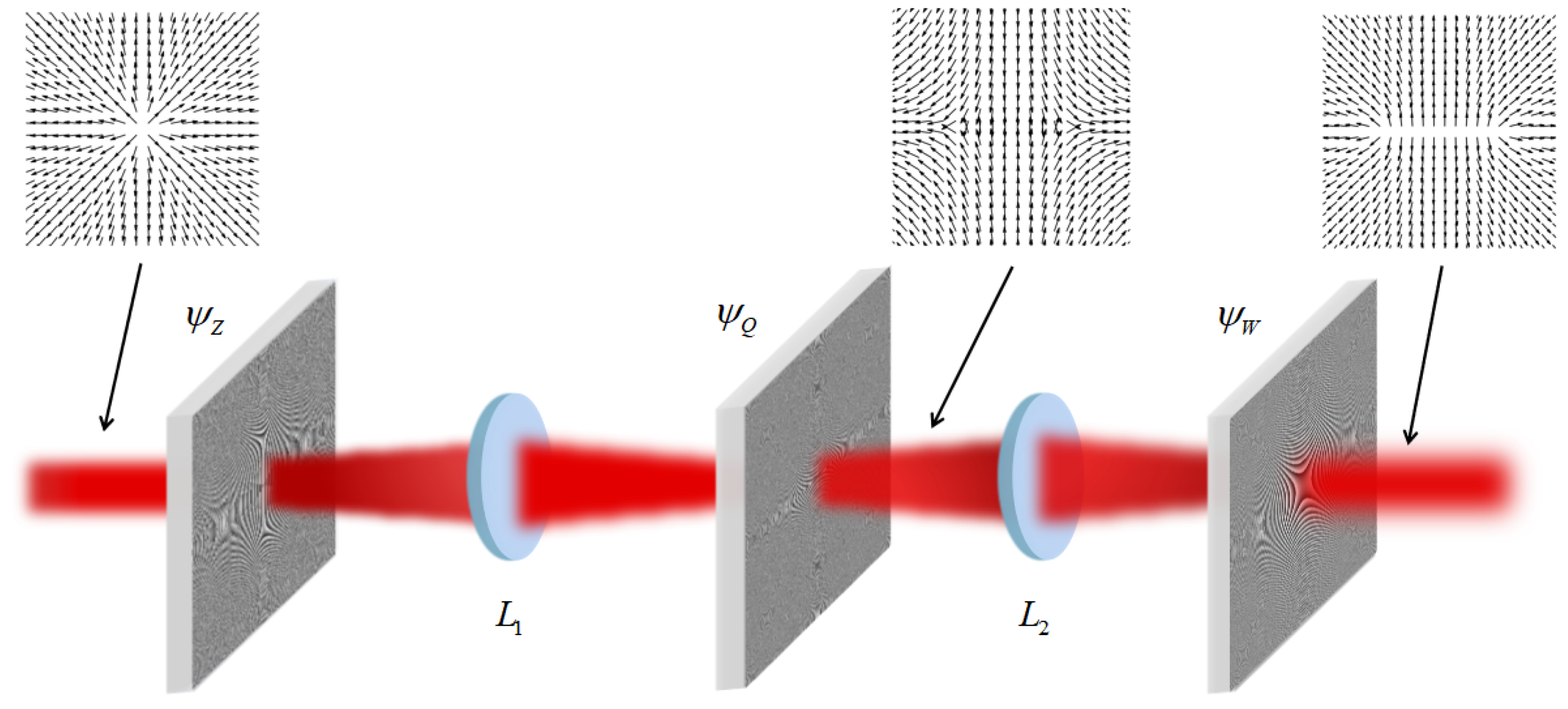
4. Phase Plate Design and Related Optical System
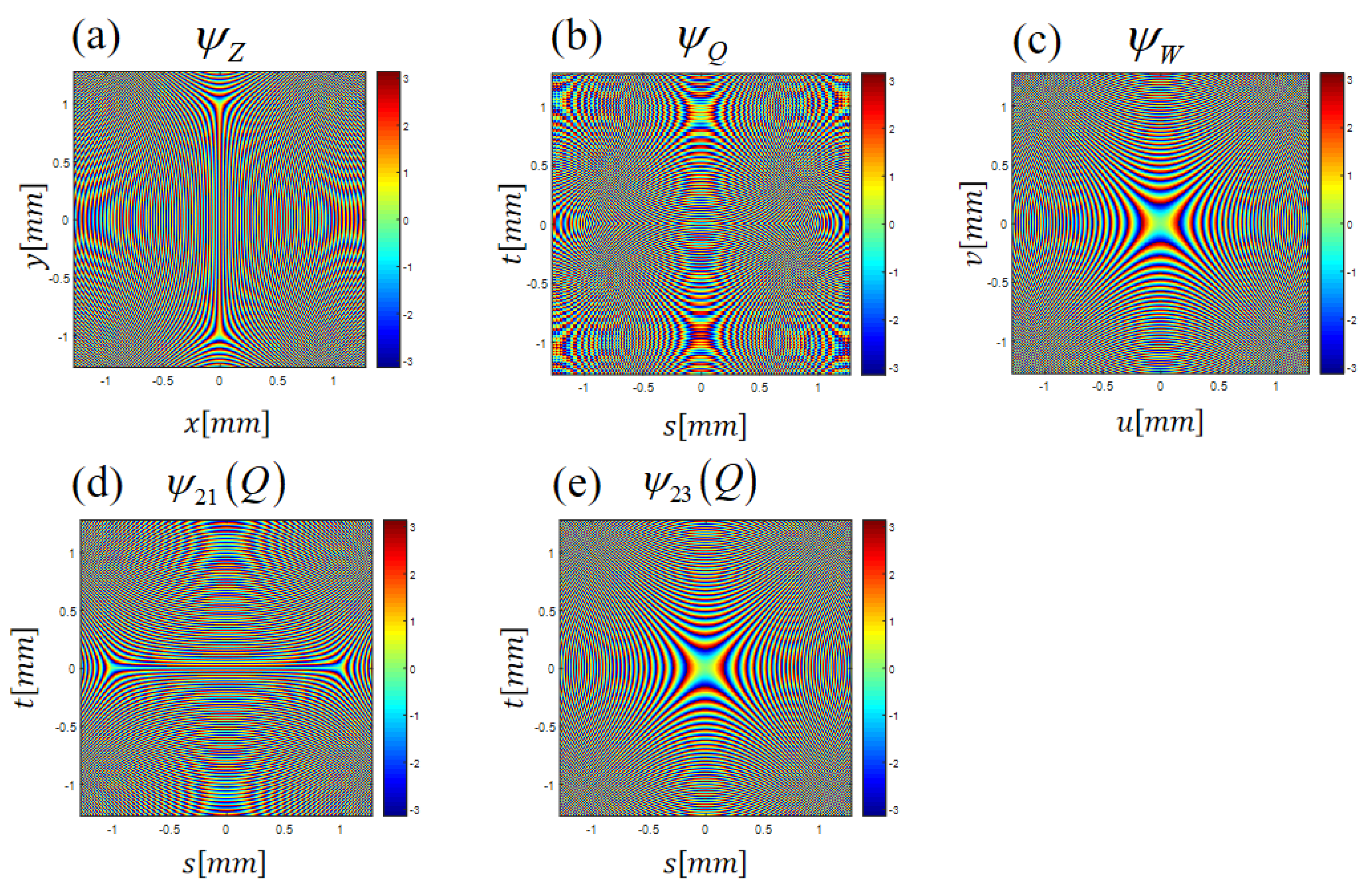
5. Numerical Simulation Results
6. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A. Circle-Cassinian Transformation and Its Properties
Appendix B. Geometric Proof of Vector Property of Circle-Cassinian Transformation
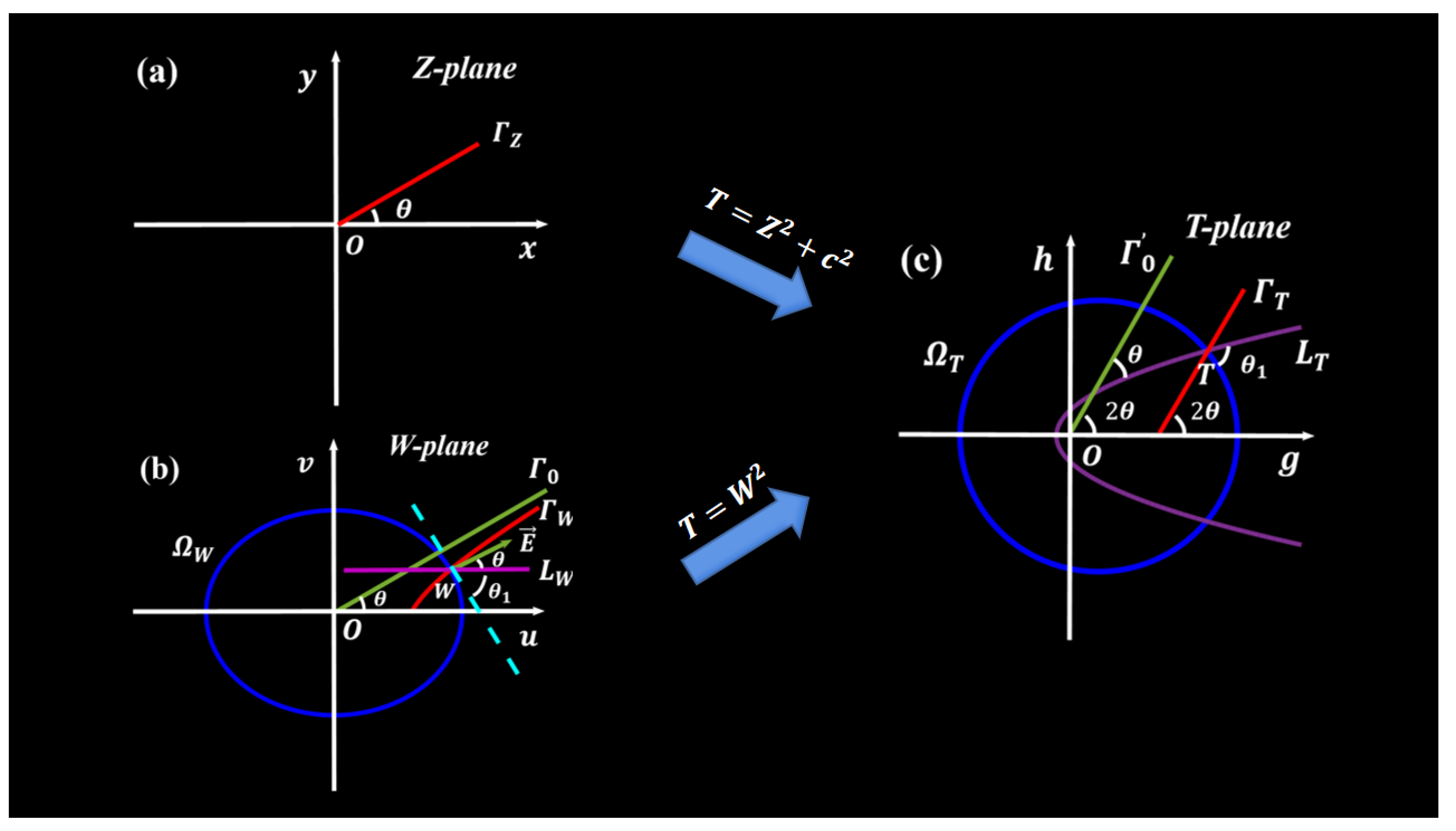
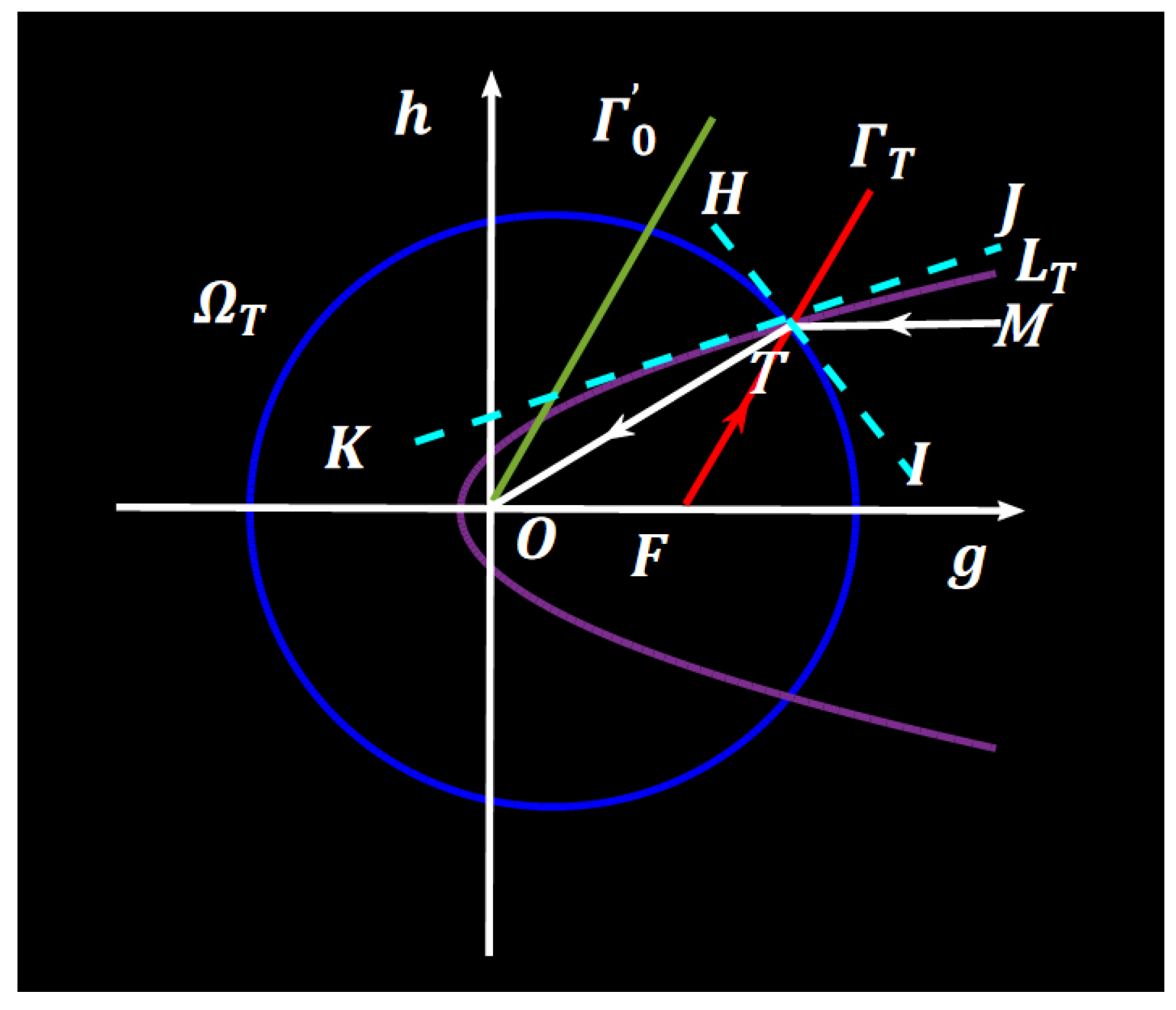
Appendix C. Calculation of Phase Profiles for Circle-Cassinian Transformation
Appendix D. Calculation of Amplitude Correction Factor
References
- Shen, Y.; Wang, X.; Xie, Z.; Min, C.; Fu, X.; Liu, Q.; Gong, M.; Yuan, X. Optical vortices 30 years on: OAM manipulation from topological charge to multiple singularities. Light Sci. Appl. 2019, 8, 90. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Forbes, A.; de Oliveira, M.; Dennis, M.R. Structured light. Nat. Photonics 2021, 15, 253–262. [Google Scholar] [CrossRef]
- Forbes, A. Structured light from lasers. Laser Photonics Rev. 2019, 13, 1900140. [Google Scholar] [CrossRef]
- Yang, Y.; Ren, Y.; Chen, M.; Arita, Y.; Rosales-Guzmán, C. Optical trapping with structured light: A review. Adv. Photonics 2021, 3, 034001. [Google Scholar] [CrossRef]
- Li, Y.; Hu, X.; Perez-Garcia, B.; Zhao, B.; Gao, W.; Zhu, Z.; Rosales-Guzmán, C. Classically entangled Ince–Gaussian modes. Appl. Phys. Lett. 2020, 116, 221105. [Google Scholar] [CrossRef]
- Rosales-Guzmán, C.; Hu, X.; Rodríguez-Fajardo, V.; Hernandez-Aranda, R.I.; Forbes, A.; Perez-Garcia, B. Experimental generation of helical Mathieu–Gauss vector modes. J. Opt. 2021, 23, 034004. [Google Scholar] [CrossRef]
- Pan, Y.; Li, Y.; Li, S.-M.; Ren, Z.-C.; Kong, L.-J.; Tu, C.; Wang, H.-T. Elliptic-symmetry vector optical fields. Opt. Express 2014, 22, 19302–19313. [Google Scholar] [CrossRef] [PubMed]
- Lerman, G.M.; Levy, U. Tight focusing of spatially variant vector optical fields with elliptical symmetry of linear polarization. Opt. Lett. 2007, 32, 2194–2196. [Google Scholar] [CrossRef]
- Lerman, G.M.; Lilach, Y.; Levy, U. Demonstration of spatially inhomogeneous vector beams with elliptical symmetry. Opt. Lett. 2009, 34, 1669–1671. [Google Scholar] [CrossRef]
- Lerman, G.M.; Yanai, A.; Ben-Yosef, N.; Levy, U. Demonstration of an elliptical plasmonic lens illuminated with radially–like polarized field. Opt. Express 2010, 18, 10871–10877. [Google Scholar] [CrossRef]
- Bryngdahl, O. Geometrical transformations in optics. J. Opt. Soc. Am. 1974, 64, 1092–1099. [Google Scholar] [CrossRef]
- Hossack, W.; Darling, A.; Dahdouh, A. Coordinate transformations with multiple computer-generated optical elements. J. Mod. Opt. 1987, 34, 1235–1250. [Google Scholar] [CrossRef]
- Berkhout, G.C.; Lavery, M.P.; Courtial, J.; Beijersbergen, M.W.; Padgett, M.J. Efficient sorting of orbital angular momentum states of light. Phys. Rev. Lett. 2010, 105, 153601. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lavery, M.P.; Robertson, D.J.; Berkhout, G.C.; Love, G.D.; Padgett, M.J.; Courtial, J. Refractive elements for the measurement of the orbital angular momentum of a single photon. Opt. Express 2012, 20, 2110–2115. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Mirhosseini, M.; Malik, M.; Shi, Z.; Boyd, R.W. Efficient separation of the orbital angular momentum eigenstates of light. Nat. Commun. 2013, 4, 2781. [Google Scholar] [CrossRef] [PubMed]
- Wan, C.; Chen, J.; Zhan, Q. Compact and high-resolution optical orbital angular momentum sorter. APL Photonics 2017, 2, 031302. [Google Scholar] [CrossRef] [Green Version]
- Ruffato, G.; Girardi, M.; Massari, M.; Mafakheri, E.; Sephton, B.; Capaldo, P.; Forbes, A.; Romanato, F. A compact diffractive sorter for high-resolution demultiplexing of orbital angular momentum beams. Sci. Rep. 2018, 8, 10248. [Google Scholar] [CrossRef] [Green Version]
- Yang, J.; Liu, Z.; Gao, S.; Huang, X.; Feng, Y.; Liu, W.; Li, Z. Two-dimension and high-resolution demultiplexing of coaxial multiple orbital angular momentum beams. Opt. Express 2019, 27, 4338–4345. [Google Scholar] [CrossRef]
- Malik, M.; Mirhosseini, M.; Lavery, M.P.; Leach, J.; Padgett, M.J.; Boyd, R.W. Direct measurement of a 27-dimensional orbital-angular-momentum state vector. Nat. Commun. 2014, 5, 3115. [Google Scholar] [CrossRef] [Green Version]
- Cheng, J.; Wan, C.; Zhan, Q. High-resolution optical orbital angular momentum sorter based on Archimedean spiral mapping. Opt. Express 2022, 30, 16330–16339. [Google Scholar] [CrossRef]
- Zhou, H.; Dong, J.; Wang, J.; Li, S.; Cai, X.; Yu, S.; Zhang, X. Orbital angular momentum divider of light. IEEE Photonics J. 2016, 9, 6500208. [Google Scholar] [CrossRef]
- Zhao, Z.; Ren, Y.; Xie, G.; Li, L.; Yan, Y.; Ahmed, N.; Wang, Z.; Liu, C.; Willner, A.J.; Ashrafi, S.; et al. Invited Article: Division and multiplication of the state order for data-carrying orbital angular momentum beams. APL Photonics 2016, 1, 090802. [Google Scholar] [CrossRef]
- Takashima, S.; Kobayashi, H.; Iwashita, K. Integer multiplier for the orbital angular momentum of light using a circular-sector transformation. Phys. Rev. A 2019, 100, 063822. [Google Scholar] [CrossRef] [Green Version]
- Ruffato, G.; Massari, M.; Romanato, F. Multiplication and division of the orbital angular momentum of light with diffractive transformation optics. Light Sci. Appl. 2019, 8, 113. [Google Scholar] [CrossRef] [Green Version]
- Wen, Y.; Chremmos, I.; Chen, Y.; Zhang, Y.; Yu, S. Arbitrary multiplication and Division of the Orbital Angular Momentum of Light. Phys. Rev. Lett. 2020, 124, 213901. [Google Scholar] [CrossRef]
- Needham, T. Visual Complex Analysis; Oxford University Press: New York, NY, USA, 1998; pp. 532–538. [Google Scholar]
- Qian, X. Digital Lab of Information Optics; Science Press: Beijing, China, 2015; pp. 35–39. [Google Scholar]
- Ruffato, G.; Kobayashi, H. Roulette caustics in transformation optics of structured light beams. Opt. Commun. 2021, 490, 126893. [Google Scholar] [CrossRef]
- Han, W.; Yang, Y.; Cheng, W.; Zhan, Q. Vectorial optical field generator for the creation of arbitrarily complex fields. Opt. Express 2013, 21, 20692–20706. [Google Scholar] [CrossRef]
- Mendoza-Yero, O.; Mínguez-Vega, G.; Lancis, J. Encoding complex fields by using a phase-only optical element. Opt. Lett. 2014, 39, 1740–1743. [Google Scholar] [CrossRef] [Green Version]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wei, H.; Fang, H. Generation of Elliptic-Symmetry Radially Polarized Optical Beam by Circle-Cassinian Optical Coordinate Transformation. Photonics 2022, 9, 563. https://doi.org/10.3390/photonics9080563
Wei H, Fang H. Generation of Elliptic-Symmetry Radially Polarized Optical Beam by Circle-Cassinian Optical Coordinate Transformation. Photonics. 2022; 9(8):563. https://doi.org/10.3390/photonics9080563
Chicago/Turabian StyleWei, Huan, and Hui Fang. 2022. "Generation of Elliptic-Symmetry Radially Polarized Optical Beam by Circle-Cassinian Optical Coordinate Transformation" Photonics 9, no. 8: 563. https://doi.org/10.3390/photonics9080563
APA StyleWei, H., & Fang, H. (2022). Generation of Elliptic-Symmetry Radially Polarized Optical Beam by Circle-Cassinian Optical Coordinate Transformation. Photonics, 9(8), 563. https://doi.org/10.3390/photonics9080563





