In-Fiber Interferometric-Based Sensors: Overview and Recent Advances
Abstract
:Featured Application
Abstract
1. Introduction
2. In-Fiber Interferometric-Based Sensors
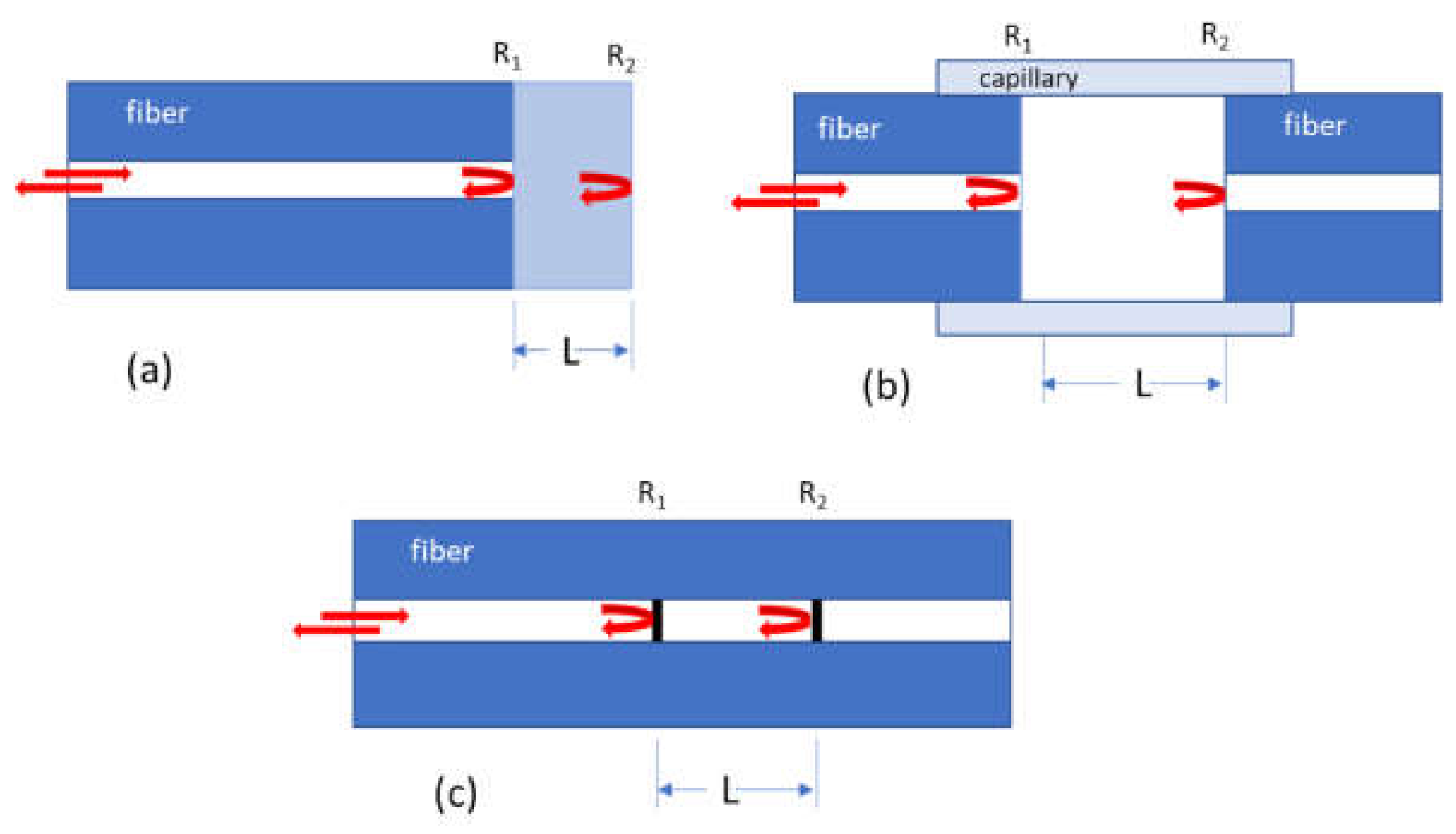
2.1. Fabry-Perot-Based Sensors
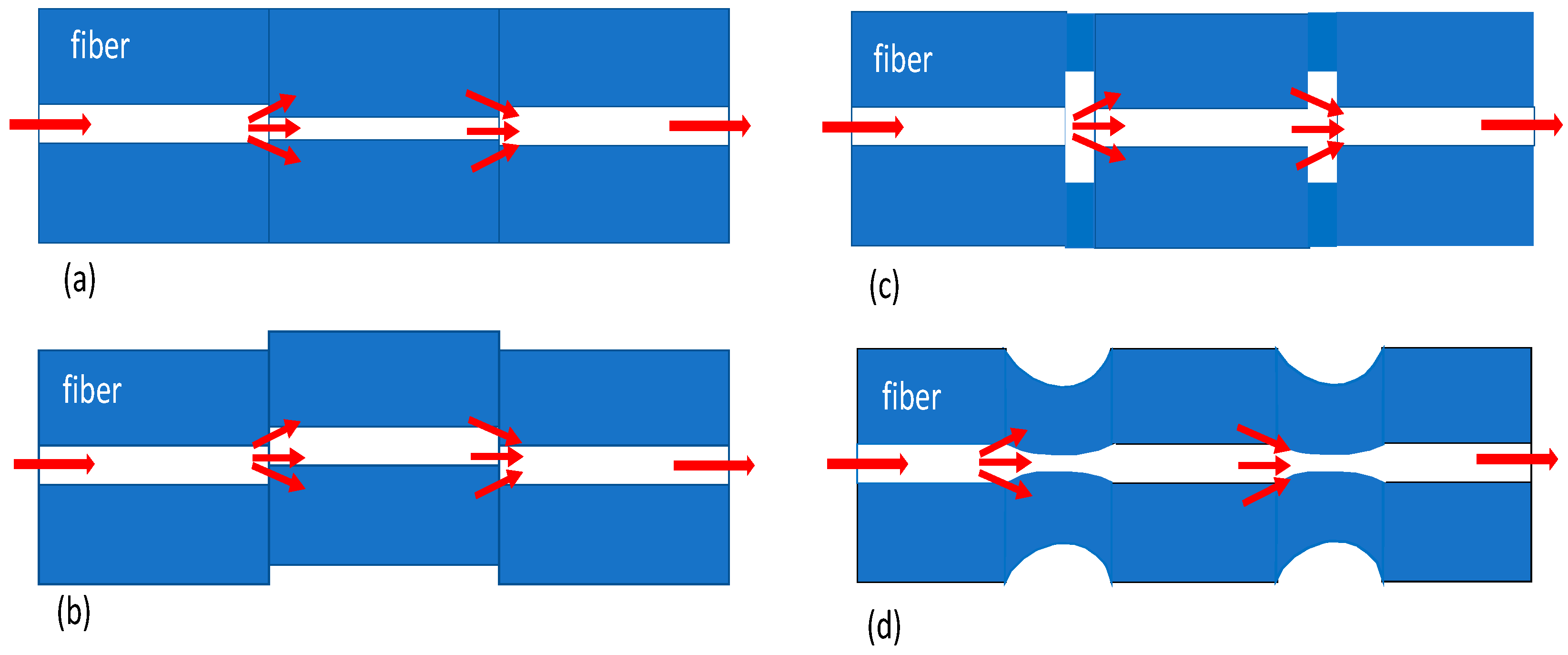
2.2. Mach-Zehnder-Based Sensors
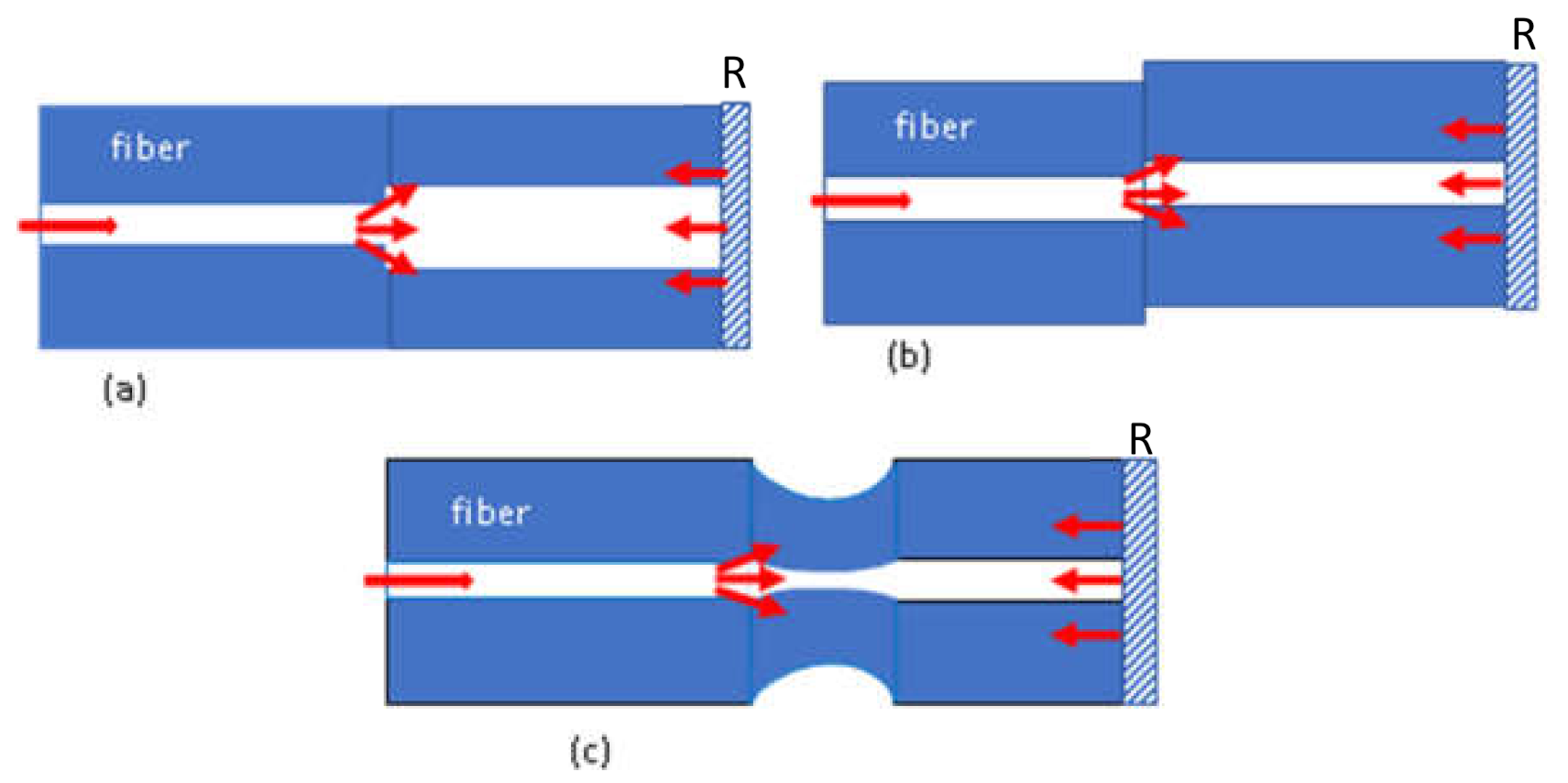
2.3. Michelson-Based Sensor
2.4. Sagnac-Based Sensors
2.5. Multiple In-Fiber Interferometric Sensors
2.6. Signal Processing Methods for Optical Path Difference Demodulation
3. Concluding Remarks and Discussion
4. Conclusions
Funding
Conflicts of Interest
References
- Lee, B.H.; Kim, Y.H.; Park, K.S.; Eom, J.B.; Kim, M.J.; Rho, B.S.; Choi, H.Y. Interferometric fiber optic sensors. Sensors 2012, 12, 2467–2486. [Google Scholar] [CrossRef] [Green Version]
- Kang, J.U. Fiber Optic Sensing and Imaging; Springer: New York, NY, USA, 2013; ISBN 9781461474821. [Google Scholar]
- Zhu, T.; Wu, D.; Liu, M.; Duan, D.W. In-line fiber optic interferometric sensors in single-mode fibers. Sensors 2012, 12, 10430–10449. [Google Scholar] [CrossRef] [Green Version]
- Sweeney, D.C.; Schrell, A.M.; Liu, Y.; Petrie, C.M. Metal-embedded fiber optic sensor packaging and signal demodulation scheme towards high-frequency dynamic measurements in harsh environments. Sensors Actuators A Phys. 2020, 312, 112075. [Google Scholar] [CrossRef]
- Lyu, D.; Peng, J.; Huang, Q.; Zheng, W.; Xiong, L.; Yang, M. Radiation-resistant optical fiber Fabry-Perot interferometer used for high-temperature sensing. IEEE Sens. J. 2020, 1748, 57–61. [Google Scholar] [CrossRef]
- Suematsu, M.; Takeda, M. Wavelength-shift interferometry for distance measurements using the Fourier transform technique for fringe analysis. Appl. Opt. 1991, 30, 4046. [Google Scholar] [CrossRef] [PubMed]
- Fischer, E.; Dalhoff, E.; Heim, S.; Hofbauer, U.; Tiziani, H.J. Absolute interferometric distance measurement using a FM-demodulation technique. Appl. Opt. 1995, 34, 5589. [Google Scholar] [CrossRef] [PubMed]
- Martinek, R.; Nedoma, J.; Fajkus, M.; Kahankova, R.; Konecny, J.; Janku, P.; Kepak, S.; Bilik, P.; Nazeran, H. A phonocardiographic-based fiber-optic sensor and adaptive filtering system for noninvasive continuous fetal heart rate monitoring. Sensors 2017, 17, 890. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Liu, G.; Hou, W.; Han, M. Unambiguous Peak Recognition for a Silicon Fabry-Pérot Interferometric Temperature Sensor. J. Light. Technol. 2018, 36, 1970–1978. [Google Scholar] [CrossRef]
- Han, M. Theoretical and Experimental Study of Low-Finesse Extrinsic Fabry-Perot Interferometric Fiber Optic Sensors. Ph.D. Thesis, Virginia Tech, Blacksburg, VA, USA, 2006. [Google Scholar]
- Liu, G.; Sheng, Q.; Hou, W.; Han, M. High-resolution, large dynamic range fiber-optic thermometer with cascaded Fabry–Perot cavities. Opt. Lett. 2016, 41, 5134. [Google Scholar] [CrossRef]
- Yi, J. High-resolution interrogation technique for fiber optic extrinsic Fabry-Perot interferometric sensors by the peak-to-peak method. Appl. Opt. 2008, 47, 925–932. [Google Scholar] [CrossRef]
- Han, M.; Zhang, Y.; Shen, F.; Pickrell, G.R.; Wang, A. Signal-processing algorithm for white-light optical fiber extrinsic Fabry–Perot interferometric sensors. Opt. Lett. 2004, 29, 1736. [Google Scholar] [CrossRef] [PubMed]
- Pevec, S.; Donlagić, D. Multiparameter fiber-optic sensors: A review. Opt. Eng. 2019, 58, 072009. [Google Scholar] [CrossRef] [Green Version]
- Liu, H.; Yang, H.Z.; Qiao, X.G.; Hu, M.L.; Feng, Z.Y.; Wang, R.; Rong, Q.; Gunawardena, D.S.; Lim, K.S.; Ahmad, H. Strain measurement at high temperature environment based on Fabry-Perot interferometer cascaded fiber regeneration grating. Sensors Actuators A Phys. 2016, 248, 199–205. [Google Scholar] [CrossRef]
- Sun, B.; Wang, Y.; Qu, J.; Liao, C.; Yin, G.; He, J.; Zhou, J.; Tang, J.; Liu, S.; Li, Z.; et al. Simultaneous measurement of pressure and temperature by employing Fabry-Perot interferometer based on pendant polymer droplet. Opt. Express 2015, 23, 1906. [Google Scholar] [CrossRef] [Green Version]
- Chen, K.; Yang, B.; Deng, H.; Guo, M.; Zhang, B.; Yang, Y.; Liu, S.; Zhao, Y.; Peng, W.; Yu, Q. Simultaneous measurement of acoustic pressure and temperature using a Fabry-Perot interferometric fiber-optic cantilever sensor. Opt. Express 2020, 28, 15050. [Google Scholar] [CrossRef] [PubMed]
- Marzejon, M.; Karpienko, K.; Mazikowski, A.; Jȩdrzejewska-Szczerska, M. Fibre-optic sensor for simultaneous measurement of thickness and refractive index of liquid layers. Metrol. Meas. Syst. 2019, 26, 561–568. [Google Scholar] [CrossRef]
- Sheng, Q.; Liu, G.; Uddin, N.; Reinke, M.L.; Han, M. Fiber-Optic Silicon Fabry-Perot Interferometric Bolometer: The Influence of Mechanical Vibration and Magnetic Field. J. Light. Technol. 2020, 38, 2547–2554. [Google Scholar] [CrossRef]
- Zhang, L.; Zhang, W.; Chen, L.; Yan, T.; Wang, L.; Wang, B.; Zhou, Q. A fiber bending vector sensor based on M-Z interferometer exploiting two hump-shaped tapers. IEEE Photon. Technol. Lett. 2015, 27, 1240–1243. [Google Scholar] [CrossRef]
- Li, C.; Ning, T.; Zhang, C.; Li, J.; Zhang, C.; Wen, X.; Lin, H.; Pei, L. All-fiber multipath Mach–Zehnder interferometer based on a four-core fiber for sensing applications. Sensors Actuators A Phys. 2016, 248, 148–154. [Google Scholar] [CrossRef]
- Sun, B.; Huang, Y.; Liu, S.; Wang, C.; He, J.; Liao, C.; Yin, G.; Zhao, J.; Liu, Y.; Tang, J.; et al. Asymmetrical in-fiber Mach-Zehnder interferometer for curvature measurement. Opt. Express 2015, 23, 14596. [Google Scholar] [CrossRef] [Green Version]
- Musa, S.M.A.; Baharin, N.F.; Azmi, A.I.; Ibrahim, R.K.R.; Abdullah, A.S.; Mohd Noor, M.Y.; Qi, H. Double-clad fiber Michelson interferometer for measurement of temperature and refractive index. Microw. Opt. Technol. Lett. 2018, 60, 822–827. [Google Scholar] [CrossRef]
- Zhao, Y.; Li, X.G.; Cai, L.; Zhang, Y.N. Measurement of RI and Temperature Using Composite Interferometer with Hollow-Core Fiber and Photonic Crystal Fiber. IEEE Trans. Instrum. Meas. 2016, 65, 2631–2636. [Google Scholar] [CrossRef]
- Dash, J.N.; Jha, R. Mach-Zehnder interferometer based on tapered PCF with an up-tapered joint for curvature, strain and temperature interrogation. J. Opt. 2016, 18. [Google Scholar] [CrossRef]
- Bian, J.; Lang, T.; Kong, W.; Chen, J. A polarization maintaining fiber sensor for simultaneous measurement of temperature and strain. Optik 2016, 127, 10090–10095. [Google Scholar] [CrossRef]
- Liu, D.; Sun, Q.; Lu, P.; Xia, L.; Sima, C. Research progress in the key device and technology for fiber optic sensor network. Photonic Sens. 2016, 6, 1–25. [Google Scholar] [CrossRef] [Green Version]
- Jones, J.D.C.; McBride, R. Multiplexing optical fiber sensors. Opt. Fiber Sens. Technol. 1998, 2, 117–166. [Google Scholar] [CrossRef]
- Li, W.; Yuan, Y.; Yang, J.; Yuan, L. Review of Optical Fiber Sensor Network Technology Based on White Light Interferometry. Photonic Sens. 2021, 11, 31–44. [Google Scholar] [CrossRef]
- Liu, T.G.; Yu, Z.; Jiang, J.F.; Liu, K.; Zhang, X.Z.; Ding, Z.Y.; Wang, S.; Hu, H.F.; Han, Q.; Zhang, H.X.; et al. Advances of some critical technologies in discrete and distributed optical fiber sensing research. Wuli Xuebao Acta Phys. Sin. 2017, 66. [Google Scholar] [CrossRef]
- Rajibul Islam, M.; Mahmood Ali, M.; Lai, M.H.; Lim, K.S.; Ahmad, H. Chronology of fabry-perot interferometer fiber-optic sensors and their applications: A review. Sensors 2014, 14, 7451–7488. [Google Scholar] [CrossRef] [Green Version]
- Li, S.; Yu, B.; Wu, X.; Shi, J.; Ge, Q.; Zhang, G.; Guo, M.; Zhang, Y.; Fang, S.S.; Zuo, C. Low-cost fiber optic extrinsic Fabry–Perot interferometer based on a polyethylene diaphragm for vibration detection. Opt. Commun. 2020, 457, 124332. [Google Scholar] [CrossRef]
- Maciak, E. Low-coherence interferometric fiber optic sensor for humidity monitoring based on nafion® thin film. Sensors 2019, 19, 629. [Google Scholar] [CrossRef] [Green Version]
- Zhou, X.; Ma, F.; Ling, H.; Yu, B.; Peng, W.; Yu, Q. A compact hydrogen sensor based on the fiber-optic Fabry-Perot interferometer. Opt. Laser Technol. 2020, 124, 105995. [Google Scholar] [CrossRef]
- Costa, G.K.B.; Gouvêa, P.M.P.; Soares, L.M.B.; Pereira, J.M.B.; Favero, F.; Braga, A.M.B.; Palffy-Muhoray, P.; Bruno, A.C.; Carvalho, I.C.S. In-fiber Fabry-Perot interferometer for strain and magnetic field sensing. Opt. Express 2016, 24, 14690. [Google Scholar] [CrossRef]
- Yang, Y.; Zhang, X.; Bai, H.; Shao, H.; Pan, H.; Pang, F.; Wang, T. Force sensors based on intrinsic fiber Fabry-Perot interferometer fabricated by the femtosecond laser. Opt. InfoBase Conf. Pap. 2014, 3–6. [Google Scholar] [CrossRef]
- Yuan, L.; Huang, J.; Lan, X.; Wang, H.; Jiang, L.; Xiao, H. All-in-fiber optofluidic sensor fabricated by femtosecond laser assisted chemical etching. Opt. Lett. 2014, 39, 2358. [Google Scholar] [CrossRef]
- Liao, C.R.; Hu, T.Y.; Wang, D.N. Optical fiber Fabry-Perot interferometer cavity fabricated by femtosecond laser micromachining and fusion splicing for refractive index sensing. Opt. Express 2012, 20, 22813. [Google Scholar] [CrossRef] [PubMed]
- Gutierrez-Rivera, M.; Jauregui-Vazquez, D.; Garcia-Mina, D.F.; Sierra-Hernandez, J.M.; Estudillo-Ayala, J.M.; Almanee, M.; Rojas-Laguna, R. Fiber Optic Fabry-Perot Micro-Displacement Sensor Based on Low-Cost Polymer Film. IEEE Sens. J. 2020, 20, 4719–4725. [Google Scholar] [CrossRef]
- Dash, J.N.; Jha, R.; Dass, S. Ultrasensitive displacement sensor based on photonic crystal fiber modal interferometer. Opt. Sensors, 2014 2014, 40, 467–470. [Google Scholar] [CrossRef]
- Monteiro, C.S.; Ferreira, M.S.; Silva, S.O.; Kobelke, J.; Schuster, K.; Bierlich, J.; Frazão, O. Fiber Fabry-Perot interferometer for curvature sensing. Photonic Sens. 2016, 6, 339–344. [Google Scholar] [CrossRef] [Green Version]
- Wang, S.; Lu, P.; Liu, L.; Liao, H.; Sun, Y.; Ni, W.; Fu, X.; Jiang, X.; Liu, D.; Zhang, J.; et al. An Infrasound Sensor Based on Extrinsic Fiber-Optic Fabry-Perot Interferometer Structure. IEEE Photon. Technol. Lett. 2016, 28, 1264–1267. [Google Scholar] [CrossRef]
- Ni, W.; Lu, P.; Fu, X.; Zhang, W.; Shum, P.P.; Sun, H.; Yang, C.; Liu, D.; Zhang, J. Ultrathin graphene diaphragm-based extrinsic Fabry-Perot interferometer for ultra-wideband fiber optic acoustic sensing. Opt. Express 2018, 26, 20758. [Google Scholar] [CrossRef] [PubMed]
- Qiu, H.; Min, F.; Yang, Y. Fiber optic sensing technologies potentially applicable for hypersonic wind tunnel harsh environments. Adv. Aerodyn. 2020, 2. [Google Scholar] [CrossRef]
- Xu, B.; Wang, C.; Wang, D.N.; Liu, Y.; Li, Y. Fiber-tip gas pressure sensor based on dual capillaries. Opt. Express 2015, 23, 23484. [Google Scholar] [CrossRef] [PubMed]
- Tang, J.; Yin, G.; Liao, C.; Liu, S.; Li, Z.; Zhong, X.; Wang, Q.; Zhao, J.; Yang, K.; Wang, Y. High-Sensitivity Gas Pressure Sensor Based on Fabry-Pérot Interferometer with a Side-Opened Channel in Hollow-Core Photonic Bandgap Fiber. IEEE Photon. J. 2015, 7, 1–7. [Google Scholar]
- Wei, H.; Chen, M.; Krishnaswamy, S. Three-dimensional-printed Fabry–Perot interferometer on an optical fiber tip for a gas pressure sensor. Appl. Opt. 2020, 59, 2173. [Google Scholar] [CrossRef]
- Kim, H.-T.; Hwang, W.; Liu, Y.; Yu, M. Ultracompact gas sensor with metal-organic-framework-based differential fiber-optic Fabry-Perot nanocavities. Opt. Express 2020, 28, 29937. [Google Scholar] [CrossRef] [PubMed]
- Shrivastav, A.M.; Gunawardena, D.S.; Liu, Z.; Tam, H.Y. Microstructured optical fiber based Fabry–Pérot interferometer as a humidity sensor utilizing chitosan polymeric matrix for breath monitoring. Sci. Rep. 2020, 10, 1–10. [Google Scholar] [CrossRef]
- Yang, R.; Xu, Z.; Zeng, S.; Jing, W.; Trontz, A.; Dong, J. A fiber optic interferometric sensor platform for determining gas diffusivity in zeolite films. Sensors 2018, 18, 90. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Chen, F.; Jiang, Y.; Gao, H.; Jiang, L. A high-finesse fiber optic Fabry-Perot interferometer based magnetic-field sensor. Opt. Lasers Eng. 2015, 71, 62–65. [Google Scholar] [CrossRef]
- Cano-Velázquez, M.S.; López-Marín, L.M.; Hernández-Cordero, J. Fiber optic interferometric immunosensor based on polydimethilsiloxane (PDMS) and bioactive lipids. Biomed. Opt. Express 2020, 11, 1316. [Google Scholar] [CrossRef]
- Hirsch, M.; Majchrowicz, D.; Wierzba, P.; Weber, M.; Bechelany, M.; Jędrzejewska-Szczerska, M. Low-coherence interferometric fiber-optic sensors with potential applications as biosensors. Sensors 2017, 17, 261. [Google Scholar] [CrossRef] [Green Version]
- Li, Z.; Zhang, Y.; Ren, C.; Sui, Z.; Li, J. A high sensitivity temperature sensing probe based on microfiber fabry-perot interference. Sensors 2019, 19, 1819. [Google Scholar] [CrossRef] [Green Version]
- Zhuang, Y. Embedded Fiber Optic Sensors for Temperature Monitoring of Continuous Casting Mold. Master’s Thesis, Missouri University of Science and Technology, Rolla, MO, USA, 2018. [Google Scholar]
- Ding, W.; Jiang, Y.; Gao, R.; Liu, Y. High-temperature fiber-optic Fabry-Perot interferometric sensors. Rev. Sci. Instrum. 2015, 86, 1–5. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wu, S.; Yan, G.; Zhou, B.; Lee, E.H.; He, S. Open-Cavity Fabry-Perot Interferometer Based on Etched Side-Hole Fiber for Microfluidic Sensing. IEEE Photon. Technol. Lett. 2015, 27, 1813–1816. [Google Scholar] [CrossRef]
- Huang, Y.W.; Tao, J.; Huang, X.G. Research progress on F-P interference-based fiber-optic sensors. Sensors 2016, 16, 1424. [Google Scholar] [CrossRef] [Green Version]
- Dash, J.N.; Jha, R. Inline microcavity-based PCF interferometer for refractive index and temperature sensing. IEEE Photon. Technol. Lett. 2015, 27, 1325–1328. [Google Scholar] [CrossRef]
- Wang, M.; Yang, Y.; Huang, S.; Wu, J.; Zhao, K.; Li, Y.; Peng, Z.; Zou, R.; Lan, H.; Ohodnicki, P.R.; et al. Multiplexable high-temperature stable and low-loss intrinsic Fabry-Perot in-fiber sensors through nanograting engineering. Opt. Express 2020, 28, 20225. [Google Scholar] [CrossRef]
- Ma, W.; Jiang, Y.; Hu, J.; Jiang, L.; Zhang, T. Microelectromechanical system-based, high-finesse, optical fiber Fabry–Perot interferometric pressure sensors. Sens. Actuators A Phys. 2020, 302, 111795. [Google Scholar] [CrossRef]
- Ma, W.; Jiang, Y.; Gao, H. Miniature all-fiber extrinsic Fabry-Pérot interferometric sensor for high-pressure sensing under high-temperature conditions. Meas. Sci. Technol. 2019, 30, 025104. [Google Scholar] [CrossRef]
- Guo, X.; Zhou, J.; Du, C.; Wang, X. Highly sensitive miniature all-silica fiber tip fabry-perot pressure sensor. IEEE Photon. Technol. Lett. 2019, 31, 689–692. [Google Scholar] [CrossRef]
- Zhu, C.; Chen, Y.; Zhuang, Y.; Fang, G.; Liu, X.; Huang, J. Optical Interferometric Pressure Sensor Based on a Buckled Beam with Low-Temperature Cross-Sensitivity. IEEE Trans. Instrum. Meas. 2018, 67, 950–955. [Google Scholar] [CrossRef]
- Tan, X.; Li, X.; Geng, Y.; Yin, Z.; Wang, L.; Wang, W.; Deng, Y. Polymer Microbubble-Based Fabry-Perot Fiber Interferometer and Sensing Applications. IEEE Photon. Technol. Lett. 2015, 27, 2035–2038. [Google Scholar] [CrossRef]
- Li, X.; Shao, Y.; Yu, Y.; Zhang, Y.; Wei, S. A highly sensitive fiber-optic fabry–perot interferometer based on internal reflection mirrors for refractive index measurement. Sensors 2016, 16, 794. [Google Scholar] [CrossRef] [Green Version]
- Yang, B.; Yang, B.; Zhang, J.; Yin, Y.; Niu, Y.; Ding, M. A sensing peak identification method for fiber extrinsic fabry–perot interferometric refractive index sensing. Sensors 2019, 19, 96. [Google Scholar] [CrossRef] [Green Version]
- Tian, J.; Lu, Z.; Quan, M.; Jiao, Y.; Yao, Y. Fast response Fabry–Perot interferometer microfluidic refractive index fiber sensor based on concave-core photonic crystal fiber. Opt. Express 2016, 24, 20132. [Google Scholar] [CrossRef]
- Liu, F.; He, X.; Yu, L.; Pan, Y.; Xie, B.; Yi, D.; Gu, L.; Zhang, M. The Applications of Interferometric Fiber-Optic Sensors in Oilfield. In Proceedings of the 2018 Progress in Electromagnetics Research Symposium (PIERS-Toyama), Toyama, Japan, 1–4 August 2018; pp. 1664–1671. [Google Scholar] [CrossRef]
- Zhao, Y.; Zhao, H.; Lv, R.Q.; Zhao, J. Review of optical fiber Mach–Zehnder interferometers with micro-cavity fabricated by femtosecond laser and sensing applications. Opt. Lasers Eng. 2019, 117, 7–20. [Google Scholar] [CrossRef]
- Engholm, M.; Hammarling, K.; Andersson, H.; Sandberg, M.; Nilsson, H.E. A bio-compatible fiber optic pH sensor based on a thin core interferometric technique. Photonics 2019, 6, 11. [Google Scholar] [CrossRef] [Green Version]
- Ahsani, V.; Ahmed, F.; Jun, M.B.G.; Bradley, C. Tapered fiber-optic mach-zehnder interferometer for ultra-high sensitivity measurement of refractive index. Sensors 2019, 19, 1652. [Google Scholar] [CrossRef] [Green Version]
- Zhao, Y.; Li, X.G.; Cai, L. A highly sensitive Mach-Zehnder interferometric refractive index sensor based on core-offset single mode fiber. Sens. Actuators A Phys. 2015, 223, 119–124. [Google Scholar] [CrossRef]
- Yu, F.; Xue, P.; Zheng, J. Enhancement of Refractive Index Sensitivity by Bending a Core-Offset In-Line Fiber Mach-Zehnder Interferometer. IEEE Sens. J. 2019, 19, 3328–3334. [Google Scholar] [CrossRef]
- Bian, C.; Cheng, Y.; Zhu, W.; Tong, R.; Hu, M.; Gang, T. A Novel Optical Fiber Mach-Zehnder Interferometer Based on the Calcium Alginate Hydrogel Film for Humidity Sensing. IEEE Sens. J. 2020, 20, 5759–5765. [Google Scholar] [CrossRef]
- Marrujo-Garcia, S.; Hernandez-Romano, I.; Torres-Cisneros, M.; May-Arrioja, D.A.; Minkovich, V.P.; Monzon-Hernandez, D. Temperature-independent curvature sensor based on in-fiber mach-zehnder interferometer using hollow-core fiber. J. Light. Technol. 2020, 38, 4166–4173. [Google Scholar] [CrossRef]
- Bhardwaj, V.; Singh, V.K. Fabrication and characterization of cascaded tapered Mach-Zehnder interferometer for refractive index sensing. Sens. Actuators A Phys. 2016, 244, 30–34. [Google Scholar] [CrossRef]
- Harris, J.; Lu, P.; Larocque, H.; Chen, L.; Bao, X. In-fiber Mach-Zehnder interferometric refractive index sensors with guided and leaky modes. Sens. Actuators B Chem. 2015, 206, 246–251. [Google Scholar] [CrossRef]
- Li, L.; Xia, L.; Xie, Z.; Liu, D. All-fiber Mach-Zehnder interferometers for sensing applications. Opt. Express 2012, 20, 11109. [Google Scholar] [CrossRef] [PubMed]
- Lokman, A.; Arof, H.; Harun, S.W.; Harith, Z.; Rafaie, H.A.; Nor, R.M. Optical Fiber Relative Humidity Sensor Based on Inline Mach-Zehnder Interferometer With ZnO Nanowires Coating. IEEE Sens. J. 2016, 16, 312–316. [Google Scholar] [CrossRef]
- Fan, X.; Wang, Q.; Zhou, M.; Liu, F.; Shen, H.; Wei, Z.; Wang, F.; Tan, C.; Meng, H. Humidity sensor based on a graphene oxide-coated few-mode fiber Mach-Zehnder interferometer. Opt. Express 2020, 28, 24682. [Google Scholar] [CrossRef] [PubMed]
- Fu, H.; Jiang, Y.; Ding, J.; Zhang, J. Low Temperature Cross-Sensitivity Humidity Sensor Based on a U-Shaped Microfiber Interferometer. IEEE Sens. J. 2017, 17, 644–649. [Google Scholar] [CrossRef]
- Li, C.; Ning, T.; Li, J.; Zhang, C.; Zhang, C.; Lin, H.; Pei, L. Fiber-Optic Laser Sensor Based on All-Fiber Multipath Mach—Zehnder Interferometer. IEEE Photon. Technol. Lett. 2016, 28, 1908–1911. [Google Scholar] [CrossRef]
- Guan, C.; Zhong, X.; Mao, G.; Yuan, T.; Yang, J.; Yuan, L. In-line Mach-Zehnder interferometric sensor based on a linear five-core fiber. IEEE Photon. Technol. Lett. 2015, 27, 635–638. [Google Scholar] [CrossRef]
- Wang, Y.; Zhou, Y.; Liu, Z.; Chen, D.; Lu, C.; Tam, H.-Y. Sensitive Mach–Zehnder interferometric sensor based on a grapefruit microstructured fiber by lateral offset splicing. Opt. Express 2020, 28, 26564. [Google Scholar] [CrossRef]
- Dhara, P.; Singh, V.K. Effect of MMF stub on the sensitivity of a photonic crystal fiber interferometer sensor at 1550 nm. Opt. Fiber Technol. 2015, 21, 154–159. [Google Scholar] [CrossRef]
- Castellani, C.E.S.; Ximenes, H.C.B.; Silva, R.L.; Frizera-Neto, A.; Ribeiro, M.R.N.; Pontes, M.J. Multi-Parameter Interferometric Sensor Based on a Reduced Diameter Core Axial Offseted Fiber. IEEE Photon. Technol. Lett. 2017, 29, 239–242. [Google Scholar] [CrossRef]
- Huang, Y.; Tian, Z.; Sun, L.-P.; Sun, D.; Li, J.; Ran, Y.; Guan, B.-O. High-sensitivity DNA biosensor based on optical fiber taper interferometer coated with conjugated polymer tentacle. Opt. Express 2015, 23, 26962. [Google Scholar] [CrossRef] [PubMed]
- Ahmed, F.; Ahsani, V.; Nazeri, K.; Marzband, E.; Bradley, C.; Toyserkani, E.; Jun, M.B.G. Monitoring of carbon dioxide using hollow-core photonic crystal fiber mach–zehnder interferometer. Sensors 2019, 19, 3357. [Google Scholar] [CrossRef] [Green Version]
- Fu, H.; Wang, Q.; Ding, J.; Zhu, Y.; Zhang, M.; Yang, C.; Wang, S. Fe2O3 nanotube coating micro-fiber interferometer for ammonia detection. Sens. Actuators B Chem. 2020, 303, 127186. [Google Scholar] [CrossRef]
- Huerta-Mascotte, E.; Sierra-Hernandez, J.M.; Mata-Chavez, R.I.; Jauregui-Vazquez, D.; Castillo-Guzman, A.; Estudillo-Ayala, J.M.; Guzman-Chavez, A.D.; Rojas-Laguna, R. A core-offset mach zehnder interferometer based on a non-zero dispersion-shifted fiber and its torsion sensing application. Sensors 2016, 16, 856. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Yu, X.; Bu, D.; Chen, X.; Zhang, J.; Liu, S. Lateral Stress Sensor Based on an In-Fiber Mach-Zehnder Interferometer and Fourier Analysis. IEEE Photon. J. 2016, 8, 1–10. [Google Scholar] [CrossRef] [Green Version]
- Li, Z.; Liao, C.; Wang, Y.; Xu, L.; Wang, D.; Dong, X.; Liu, S.; Wang, Q.; Yang, K.; Zhou, J. Highly-sensitive gas pressure sensor using twin-core fiber based in-line Mach-Zehnder interferometer. Opt. Express 2015, 23, 6673. [Google Scholar] [CrossRef] [PubMed]
- Zheng, Y.; Dong, X.; Chan, C.C.; Shum, P.P.; Su, H. Optical fiber magnetic field sensor based on magnetic fluid and microfiber mode interferometer. Opt. Commun. 2015, 336, 5–8. [Google Scholar] [CrossRef]
- Hu, X.; Shen, X.; Wu, J.; Peng, J.; Yang, L.; Li, J.; Li, H.; Dai, N. All fiber M-Z interferometer for high temperature sensing based on a hetero-structured cladding solid-core photonic bandgap fiber. Opt. Express 2016, 24, 21693. [Google Scholar] [CrossRef] [PubMed]
- Gao, S.; Ji, C.; Ning, Q.; Chen, W.; Li, J. High-sensitive Mach-Zehnder interferometric temperature fiber-optic sensor based on core-offset splicing technique. Opt. Fiber Technol. 2020, 56, 102202. [Google Scholar] [CrossRef]
- Naeem, K.; Kim, B.H.; Kim, B.; Chung, Y. High-Sensitivity Temperature Sensor Based on a. IEEE Sens. J. 2015, 15, 3998–4003. [Google Scholar] [CrossRef]
- Pallarés-Aldeiturriaga, D.; Rodriguez-Cobo, L.; Quintela, A.; Lopez-Higuera, J.M. In-fiber Mach-Zehnder interferometer inscribed with femtosecond laser for high temperature sensing. In Proceedings of the 2017 25th Optical Fiber Sensors Conference (OFS), Jeju, Korea, 24–28 April 2017. [Google Scholar] [CrossRef] [Green Version]
- Wang, X.; Chen, D.; Li, H.; Feng, G.; Yang, J. In-Line Mach-Zehnder Interferometric Sensor Based on a Seven-Core Optical Fiber. IEEE Sens. J. 2017, 17, 100–104. [Google Scholar] [CrossRef]
- Raji, Y.M.; Lin, H.S.; Ibrahim, S.A.; Mokhtar, M.R.; Yusoff, Z. Intensity-modulated abrupt tapered Fiber Mach-Zehnder Interferometer for the simultaneous sensing of temperature and curvature. Opt. Laser Technol. 2016, 86, 8–13. [Google Scholar] [CrossRef] [Green Version]
- Hu, P.; Chen, Z.; Yang, M.; Yang, J.; Zhong, C. Highly sensitive liquid-sealed multimode fiber interferometric temperature sensor. Sens. Actuators A Phys. 2015, 223, 114–118. [Google Scholar] [CrossRef]
- Hernandez-Romano, I.; Monzon-Hernandez, D.; Moreno-Hernandez, C.; Moreno-Hernandez, D.; Villatoro, J. Highly Sensitive Temperature Sensor Based on a Polymer-Coated Microfiber Interferometer. IEEE Photon. Technol. Lett. 2015, 27, 2591–2594. [Google Scholar] [CrossRef]
- Yi, D.; Huo, Z.; Geng, Y.; Li, X.; Hong, X. PDMS-coated no-core fiber interferometer with enhanced sensitivity for temperature monitoring applications. Opt. Fiber Technol. 2020, 57, 102185. [Google Scholar] [CrossRef]
- Wu, J.; Miao, Y.; Song, B.; Lin, W.; Zhang, K.; Zhang, H.; Liu, B.; Yao, J. Simultaneous measurement of displacement and temperature based on thin-core fiber modal interferometer. Opt. Commun. 2015, 340, 136–140. [Google Scholar] [CrossRef]
- Kong, J.; Ouyang, X.; Zhou, A.; Yuan, L. Highly Sensitive Directional Bending Sensor Based on Eccentric Core Fiber Mach-Zehnder Modal Interferometer. IEEE Sens. J. 2016, 16, 6899–6902. [Google Scholar] [CrossRef]
- Wang, R.; Zhao, J.; Sun, Y.; Yu, H.; Zhou, N.; Zhang, H.; Jia, D. Wearable respiration monitoring using an in-line few-mode fiber Mach-Zehnder interferometric sensor. Biomed. Opt. Express 2020, 11, 316. [Google Scholar] [CrossRef]
- Dass, S.; Jha, R. Micrometer wire assisted inline Mach-Zehnder interferometric curvature sensor. IEEE Photon. Technol. Lett. 2015, 28, 31–34. [Google Scholar] [CrossRef]
- Luo, H.; Sun, Q.; Li, X.; Yan, Z.; Li, Y.; Liu, D.; Zhang, L. Refractive index sensitivity characteristics near the dispersion turning point of the multimode microfiber-based Mach–Zehnder interferometer. Opt. Lett. 2015, 40, 5042. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wang, Q.; Kong, L.; Dang, Y.; Xia, F.; Zhang, Y.; Zhao, Y.; Hu, H.; Li, J. High sensitivity refractive index sensor based on splicing points tapered SMF-PCF-SMF structure Mach-Zehnder mode interferometer. Sens. Actuators B Chem. 2016, 225, 213–220. [Google Scholar] [CrossRef] [Green Version]
- Nazeri, K.; Ahmed, F.; Ahsani, V.; Joe, H.; Bradley, C.; Toyserkani, E.; Jun, M.B.G. Interferometer for Gas Sensing. Sensors 2020, 20, 2807. [Google Scholar] [CrossRef] [PubMed]
- Yu, F.; Liu, B.; Zou, Q.; Xue, P.; Zheng, J. Influence of temperature on the refractive index sensor based on a core-offset in-line fiber Mach-Zehnder interferometer. Opt. Fiber Technol. 2020, 58, 102293. [Google Scholar] [CrossRef]
- Yu, F.; Xue, P.; Zhao, X.; Zheng, J. Investigation of an in-line fiber Mach–Zehnder interferometer based on peanut-shape structure for refractive index sensing. Opt. Commun. 2019, 435, 173–177. [Google Scholar] [CrossRef]
- Wang, Q.; Wei, W.; Guo, M.; Zhao, Y. Optimization of cascaded fiber tapered Mach-Zehnder interferometer and refractive index sensing technology. Sens. Actuators B Chem. 2016, 222, 159–165. [Google Scholar] [CrossRef]
- Zhao, Y.; Li, X.G.; Cai, L.; Yang, Y. Refractive index sensing based on photonic crystal fiber interferometer structure with up-tapered joints. Sens. Actuators B Chem. 2015, 221, 406–410. [Google Scholar] [CrossRef] [Green Version]
- Yu, F.; Xue, P.; Zheng, J. Study of an in-line fiber Mach-Zehnder interferometer with peanut-shape structure for refractive index sensing. In Proceedings of the Asia Communications and Photonics Conference, Hangzhou, China, 26–29 October 2018. [Google Scholar] [CrossRef]
- Gong, T.; Liu, X.; Wang, Z.; Liu, Y. Ultrasensitivity steel surface corrosion noncontacted monitoring based on a mismatching fused Mach-Zehnder interferometric fiber sensor. IEEE Sens. J. 2020, 2018, 12732–12738. [Google Scholar] [CrossRef]
- Guo, D.; Wu, L.; Yu, H.; Zhou, A.; Li, Q.; Mumtaz, F.; Du, C.; Hu, W. Tapered multicore fiber interferometer for refractive index sensing with graphene enhancement. Appl. Opt. 2020, 59, 3927. [Google Scholar] [CrossRef] [PubMed]
- Dash, J.N.; Jha, R. PCF Modal Interferometer Based on Macrobending for Refractive Index Sensing. IEEE Sens. J. 2015, 15, 5291–5295. [Google Scholar] [CrossRef]
- Fu, H.; Li, H.; Shao, M.; Zhao, N.; Liu, Y.; Li, Y.; Yan, X.; Liu, Q. TCF-MMF-TCF fiber structure based interferometer for refractive index sensing. Opt. Lasers Eng. 2015, 69, 58–61. [Google Scholar] [CrossRef]
- Nguyen, L.V.; Warren-Smith, S.C.; Ebendorff-Heidepriem, H.; Monro, T.M. Interferometric high temperature sensor using suspended-core optical fibers. Opt. Express 2016, 24, 8967. [Google Scholar] [CrossRef]
- Yin, J.; Liu, T.; Jiang, J.; Liu, K.; Wang, S.; Zou, S.; Wu, F. Assembly-Free-Based Fiber-Optic Micro-Michelson Interferometer for High Temperature Sensing. IEEE Photon. Technol. Lett. 2016, 28, 625–628. [Google Scholar] [CrossRef]
- Sun, H.; Shao, M.; Han, L.; Liang, J.; Zhang, R.; Fu, H. Large core-offset based in-fiber Michelson interferometer for humidity sensing. Opt. Fiber Technol. 2020, 55, 102153. [Google Scholar] [CrossRef]
- Zhou, J.; Wang, Y.; Liao, C.; Sun, B.; He, J.; Yin, G.; Liu, S.; Li, Z.; Wang, G.; Zhong, X.; et al. Intensity modulated refractive index sensor based on optical fiber Michelson interferometer. Sens. Actuators B Chem. 2015, 208, 315–319. [Google Scholar] [CrossRef]
- Sun, Q.; Luo, H.; Luo, H.; Lai, M.; Liu, D.; Zhang, L. Multimode microfiber interferometer for dual-parameters sensing assisted by Fresnel reflection. Opt. Express 2015, 23, 12777. [Google Scholar] [CrossRef]
- Salceda-Delgado, G.; Martinez-Rios, A.; Selvas-Aguilar, R.; Álvarez-Tamayo, R.I.; Castillo-Guzman, A.; Ibarra-Escamilla, B.; Durán-Ramírez, V.M.; Enriquez-Gomez, L.F. Adaptable optical fiber displacement-curvature sensor based on a modal michelson interferometer with a tapered single mode fiber. Sensors 2017, 17, 1259. [Google Scholar] [CrossRef] [Green Version]
- Hu, P.; Dong, X.; Ni, K.; Chen, L.H.; Wong, W.C.; Chan, C.C. Sensitivity-enhanced Michelson interferometric humidity sensor with waist-enlarged fiber bitaper. Sens. Actuators B Chem. 2014, 194, 180–184. [Google Scholar] [CrossRef]
- Shao, M.; Sun, H.; Liang, J.; Han, L.; Feng, D. In-Fiber Michelson Interferometer in Photonic Crystal Fiber for Humidity Measurement. IEEE Sens. J. 2021, 21, 1561–1567. [Google Scholar] [CrossRef]
- Duan, L.; Zhang, P.; Tang, M.; Wang, R.; Zhao, Z.; Fu, S.; Gan, L.; Zhu, B.; Tong, W.; Liu, D.; et al. Heterogeneous all-solid multicore fiber based multipath Michelson interferometer for high temperature sensing. Opt. Express 2016, 24, 20210. [Google Scholar] [CrossRef] [PubMed]
- Zhao, N.; Fu, H.; Shao, M.; Yan, X.; Li, H.; Liu, Q.; Gao, H.; Liu, Y.; Qiao, X. High temperature probe sensor with high sensitivity based on Michelson interferometer. Opt. Commun. 2015, 343, 131–134. [Google Scholar] [CrossRef]
- Cardona-Maya, Y.; Botero-Cadavid, J.F. Refractive index desensitized optical fiber temperature sensor. Rev. Fac. Ing. 2017, 86–90. [Google Scholar] [CrossRef]
- Song, B.; Zhang, H.; Miao, Y.; Lin, W.; Wu, J.; Liu, H.; Yan, D.; Liu, B. Highly sensitive twist sensor employing Sagnac interferometer based on PM-elliptical core fibers. Opt. Express 2015, 23, 15372. [Google Scholar] [CrossRef]
- Li, A.; Wang, Y.; Hu, Q.; Shieh, W. Few-mode fiber based optical sensors. Opt. Express 2015, 23, 1139. [Google Scholar] [CrossRef] [PubMed]
- Sun, L.P.; Li, J.; Jin, L.; Ran, Y.; Guan, B.O. High-birefringence microfiber Sagnac interferometer based humidity sensor. Sens. Actuators B Chem. 2016, 231, 696–700. [Google Scholar] [CrossRef] [Green Version]
- Htein, L.; Gunawardena, D.S.; Liu, Z.; Tam, H.-Y. Two semicircular-hole fiber in a Sagnac loop for simultaneous discrimination of torsion, strain and temperature. Opt. Express 2020, 28, 33841. [Google Scholar] [CrossRef]
- He, X.; Ma, C.; Wang, X.; Wang, Z.; Yuan, L. Pressure vector sensor based on an orthogonal optical path Sagnac interferometer. Opt. Express 2020, 28, 7969. [Google Scholar] [CrossRef]
- Feng, W.Q.; Liu, Z.Y.; Tam, H.Y.; Yin, J.H. The pore water pressure sensor based on Sagnac interferometer with polarization-maintaining photonic crystal fiber for the geotechnical engineering. Meas. J. Int. Meas. Confed. 2016, 90, 208–214. [Google Scholar] [CrossRef]
- Zhao, Y.; Wu, D.; Lv, R.Q.; Li, J. Magnetic Field Measurement Based on the Sagnac Interferometer With a Ferrofluid-Filled High-Birefringence Photonic Crystal Fiber. IEEE Trans. Instrum. Meas. 2016, 65, 1503–1507. [Google Scholar] [CrossRef]
- Lv, F.; Han, C.; Ding, H.; Wu, Z.; Li, X. Magnetic Field Sensor Based on Microfiber Sagnac Loop Interferometer and Ferrofluid. IEEE Photon. Technol. Lett. 2015, 27, 2327–2330. [Google Scholar] [CrossRef]
- Liu, Q.; Li, S.G.; Shi, M. Fiber Sagnac interferometer based on a liquid-filled photonic crystal fiber for temperature sensing. Opt. Commun. 2016, 381, 1–6. [Google Scholar] [CrossRef]
- Shao, L.Y.; Zhang, X.; He, H.; Zhang, Z.; Zou, X.; Luo, B.; Pan, W.; Yan, L. Optical fiber temperature and torsion sensor based on Lyot-Sagnac interferometer. Sensors 2016, 16, 1774. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Liu, Y.; Li, X.; Zhang, Y.N.; Zhao, Y. Fiber-optic sensors based on Vernier effect. Meas. J. Int. Meas. Confed. 2021, 167, 108451. [Google Scholar] [CrossRef]
- Cui, Y.; Jiang, Y.; Liu, T.; Hu, J.; Jiang, L. A Dual-Cavity Fabry-Perot Interferometric Fiber-Optic Sensor for the Simultaneous Measurement of High-Temperature and High-Gas-Pressure. IEEE Access 2020, 8, 80582–80587. [Google Scholar] [CrossRef]
- Fan, H.; Chen, L.; Bao, X. Fiber-Optic Sensor Based on Core-Offset Fused Unequal-Length Fiber Segments to Improve Ultrasound Detection Sensitivity. IEEE Sens. J. 2020, 20, 9148–9154. [Google Scholar] [CrossRef]
- Zheng, Y.; Chen, L.H.; Dong, X.; Yang, J.; Long, H.Y.; So, P.L.; Chan, C.C. Miniature pH Optical Fiber Sensor Based on Fabry-Perot Interferometer. IEEE J. Sel. Top. Quantum Electron. 2016, 22, 331–335. [Google Scholar] [CrossRef]
- Zhang, P.; Tang, M.; Gao, F.; Zhu, B.; Zhao, Z.; Duan, L.; Fu, S.; Ouyang, J.; Wei, H.; Shum, P.P.; et al. Simplified hollow-core fiber-based fabry-perot interferometer with modified vernier effect for highly sensitive high-temperature measurement. IEEE Photon. J. 2015, 7, 1–10. [Google Scholar] [CrossRef]
- Li, X.G.; Zhao, Y.; Cai, L.; Wang, Q. Simultaneous Measurement of RI and Temperature Based on a Composite Interferometer. IEEE Photon. Technol. Lett. 2016, 28, 1839–1842. [Google Scholar] [CrossRef]
- Dominguez-Flores, C.E.; Rodriguez-Quiroz, O.; Monzon-Hernandez, D.; Ascorbe, J.; Corres, J.M.; Arregui, F.J. Dual-Cavity Fiber Fabry-Perot Interferometer Coated with SnO2for Relative Humidity and Temperature Sensing. IEEE Sens. J. 2020, 20, 14195–14201. [Google Scholar] [CrossRef]
- Zhao, Y.; Tong, R.J.; Chen, M.Q.; Peng, Y. Relative humidity sensor based on Vernier effect with GQDs-PVA un-fully filled in hollow core fiber. Sensors Actuators A Phys. 2019, 285, 329–337. [Google Scholar] [CrossRef]
- Hu, J.; Smietana, M.; Wang, T.; Lang, T.; Shao, L.; Gu, G.; Zhang, X.; Liu, Y.; Song, X.; Song, Z.; et al. Dual Mach-Zehnder Interferometer Based on Side-Hole Fiber for High-Sensitivity Refractive Index Sensing. IEEE Photon. J. 2019, 11. [Google Scholar] [CrossRef]
- Lin, H.; Liu, F.; Guo, H.; Zhou, A.; Dai, Y. Ultra-highly sensitive gas pressure sensor based on dual side-hole fiber interferometers with Vernier effect. Opt. Express 2018, 26, 28763. [Google Scholar] [CrossRef]
- Zhang, S.; Yin, L.; Zhao, Y.; Zhou, A.; Yuan, L. Bending sensor with parallel fiber Michelson interferometers based on Vernier-like effect. Opt. Laser Technol. 2019, 120, 105679. [Google Scholar] [CrossRef]
- Shao, L.Y.; Luo, Y.; Zhang, Z.; Zou, X.; Luo, B.; Pan, W.; Yan, L. Sensitivity-enhanced temperature sensor with cascaded fiber optic Sagnac interferometers based on Vernier-effect. Opt. Commun. 2015, 336, 73–76. [Google Scholar] [CrossRef]
- Ushakov, N.A.; Markvart, A.A.; Liokumovich, L.B. Pulse Wave Velocity Measurement with Multiplexed Fiber Optic Fabry-Perot Interferometric Sensors. IEEE Sens. J. 2020, 20, 11302–11312. [Google Scholar] [CrossRef]
- Liao, H.; Lu, P.; Fu, X.; Jiang, X.; Ni, W.; Liu, D.; Zhang, J. Sensitivity amplification of fiber-optic in-line Mach–Zehnder Interferometer sensors with modified Vernier-effect. Opt. Express 2017, 25, 26898. [Google Scholar] [CrossRef] [PubMed]
- Wang, Z.; Huang, L.; Liu, C.; Wang, H.; Sun, S.; Yang, D. Sensitivity-Enhanced Fiber Temperature Sensor Based on Vernier Effect and Dual In-Line Mach-Zehnder Interferometers. IEEE Sens. J. 2019, 19, 7983–7987. [Google Scholar] [CrossRef]
- Xie, L.; Sun, B.; Chen, M.; Zhang, Z. Sensitivity enhanced temperature sensor with serial tapered two-mode fibers based on the Vernier effect. Opt. Express 2020, 28, 32447. [Google Scholar] [CrossRef] [PubMed]
- Wang, S.; Wang, S.; Jin, R.B.; Feng, M.; Wu, S.; Zhang, L.; Lu, P. All-Optical demodulation fiber acoustic sensor with real-Time controllable sensitivity based on optical vernier effect. IEEE Photon. J. 2019, 11. [Google Scholar] [CrossRef]
- Yang, Y.; Wang, Y.; Zhao, Y.; Jiang, J.; He, X.; Yang, W.; Zhu, Z.; Gao, W.; Li, L. Sensitivity-enhanced temperature sensor by hybrid cascaded configuration of a Sagnac loop and a F-P cavity. Opt. Express 2017, 25, 33290. [Google Scholar] [CrossRef]
- Claes, T.; Bogaerts, W.; Bienstman, P. Experimental characterization of a silicon photonic biosensor consisting of two cascaded ring resonators based on the Vernier-effect and introduction of a curve fitting method for an improved detection limit. Opt. Express 2010, 18, 22747. [Google Scholar] [CrossRef] [Green Version]
- Zhang, P.; Tang, M.; Gao, F.; Zhu, B.; Fu, S.; Ouyang, J.; Shum, P.P.; Liu, D. Cascaded fiber-optic Fabry-Perot interferometers with Vernier effect for highly sensitive measurement of axial strain and magnetic field. Opt. Express 2014, 22, 19581. [Google Scholar] [CrossRef] [PubMed]
- Ma, C.; Wang, A. Signal processing of white-light interferometric low-finesse fiber-optic Fabry—Perot sensors. Appl. Opt. 2013, 52, 127–138. [Google Scholar] [CrossRef]
- Yu, Z.; Wang, A. Fast White Light Interferometry Demodulation Algorithm for Low-Finesse Fabry-Pérot Sensors. IEEE Photon. Technol. Lett. 2015, 27, 817–820. [Google Scholar] [CrossRef]
- Bhatia, V.; Murphy, K.A.; Claus, R.O.; Tran, T.A.; Greene, J.A. Recent developments in optical-fiber-based extrinsic Fabry-Perot interferometric strain sensing technology. Smart Mater. Struct. 1995, 4, 246–251. [Google Scholar] [CrossRef]
- Yu, Q.; Zhou, X. Pressure sensor based on the fiber-optic extrinsic fabry-perot interferometer. Photonic Sens. 2011, 1, 72–83. [Google Scholar] [CrossRef] [Green Version]
- Xie, J.; Wang, F.; Pan, Y.; Wang, J.; Hu, Z.; Hu, Y. High resolution signal-processing method for extrinsic Fabry-Perot interferometric sensors. Opt. Fiber Technol. 2015, 22, 1–6. [Google Scholar] [CrossRef]
- Zhou, X.; Yu, Q. Wide-Range Displacement Sensor Based on Fiber-Optic Fabry–Perot Interferometer for Subnanometer Measurement. IEEE Sens. J. 2011, 11, 1602–1606. [Google Scholar] [CrossRef]
- Li, C.; Chen, S.; Zhu, Y. Maximum Likelihood Estimation of Optical Path Length in Spectral Interferometry. J. Light. Technol. 2017, 35, 4880–4887. [Google Scholar] [CrossRef]
- Qi, Y.; Chen, W.; Xie, J.; Lei, X. A fast Maximum Likelihood Estimation algorithm for demodulating Fiber White-Light Interferometry. J. Phys. Conf. Ser. 2018, 1065. [Google Scholar] [CrossRef]
- Wu, Y.; Xia, L.; Cai, N.; Zhu, L. A Highly Precise Demodulation Method for Fiber Fabry-Perot Cavity through Spectrum Reconstruction. IEEE Photon. Technol. Lett. 2018, 30, 435–438. [Google Scholar] [CrossRef]
- Chen, H.; Liu, J.; Zhang, X.; Wang, W.; Ma, Z.; Lv, W.; Guo, Z. High-Order Harmonic-Frequency Cross-Correlation Algorithm for Absolute Cavity Length Interrogation of White-Light Fiber-Optic Fabry-Perot Sensors. J. Light. Technol. 2020, 38, 953–960. [Google Scholar] [CrossRef]
- Wang, H.; Meng, H.; Xiong, R.; Wang, Q.; Huang, B.; Zhang, X.; Yu, W.; Tan, C.; Huang, X. Simultaneous measurement of refractive index and temperature based on asymmetric structures modal interference. Opt. Commun. 2016, 364, 191–194. [Google Scholar] [CrossRef]
- Ni, X.; Wang, M.; Guo, D.; Hao, H.; Zhu, J. A Hybrid Mach-Zehnder Interferometer for Refractive Index and Temperature Measurement. IEEE Photon. Technol. Lett. 2016, 28, 1850–1853. [Google Scholar] [CrossRef]
- Mayrargue, S.; Blu, T. Relationship between high-resolution methods and discrete Fourier transform. Proc. ICASSP, IEEE Int. Conf. Acoust. Speech Signal Process. 1991, 5, 3321–3324. [Google Scholar] [CrossRef] [Green Version]
- Russell, P. Applied physics: Photonic crystal fibers. Science 2003, 299, 358–362. [Google Scholar] [CrossRef] [PubMed]
- Weihs, G.; Reck, M.; Weinfurter, H.; Zeilinger, A. All-fiber three-path Mach–Zehnder interferometer. Opt. Lett. 1996, 21, 302. [Google Scholar] [CrossRef] [Green Version]
- Chen, G.Y. A Review of Microfiber and Nanofiber Based Optical Sensors. Open Opt. J. 2014, 7, 32–57. [Google Scholar] [CrossRef]
- Li, Z.; Liao, C.; Song, J.; Wang, Y.; Wang, Y. Ultrasensitive magnetic field sensor based on in-fiber Mach-Zehnder interferometer and magnetic fluid. Opt. InfoBase Conf. Pap. 2014, 4, 197–201. [Google Scholar] [CrossRef]

| Sensor Design | Sensitivity (pm/°C) | Range (°C) | Applications | Ref. |
|---|---|---|---|---|
| Microfiber taper/SMF | 11,800 | 43–50 | Biochemical reaction, food storage | [54] |
| SMF/air/SMF | 1 | RT-700 | Various applications | [55] |
| SMF/air/SMF wax embedded | 60 | |||
| SMF/Endless single-mode PCF | 10 | up to 1200 | Wide-range of applications | [56] |
| SMF/side hole fiber/SMF | 1.1 | 20–100 | Biochemical | [57] |
| MMF/sapphire wafer | 29.9 | up to 800 | Extremely harsh environment | [5] |
| SMF/solid film | 200 | 20–100 | Various applications | [58] |
| Fiber-optic cantilever sensor | 83,000 | 0–80 | Multiparameter measurements | [17] |
| SMF/curved polymer tip | 249 | ---- | Harsh environment | [16] |
| SMF/Hollow Core Fiber/SMF | 0.82 | 19–600 | Multiparameter measurements | [15] |
| 3D printed | 160.2 | 20–70 | Various applications | [47] |
| SMF/air/SMF | 260,700 | 25.2–28.2 | Enhanced sensitivity | [40] |
| SMF/silicon | 84.8 | 0–100 | Various applications | [9] |
| SMF-a solid-core PCF | 12 | ---- | Various applications | [59] |
| Introduction of nanograting inside the core of SMF | 11,100 | <300 | Extreme environmental conditions | [60] |
| 14,400 | >300 |
| Sensor Design | Sensitivity (nm/MPa) | Range (MPa) | Applications | Ref. |
|---|---|---|---|---|
| Embedded SMF/diaphragm | ---- | ±0.1 | Harsh environment | [4] |
| MEMS-based FOFPI | 1598 | 0–1 | Atmospheric | [61] |
| SMF/Silica diaphragm | 70.8 | 0–10 | High-temperature | [62] |
| Diaphragm-based all-silica | 12,400 | 6900–48,300 | Medical | [63] |
| Buckled beam-based sensor | 169,000 | 0–2 | Environmental | [64] |
| Polymer microbubble-based sensor | 310 | 0–0.1 | Biomedical and microfluidic | [65] |
| SMF/curved polymer tip | 1,130,000 | 0.1–2.5 | Harsh environment | [16] |
| Sensor Design | Sensitivity (nm/RIU) | Range (RIU) | Applications | Ref. |
|---|---|---|---|---|
| SMF/Microcavity SMF | 1160 | 0.0058 | Biochemical & environmental | [66] |
| SMF/Microcavity/SMF | 979.7 | 0.042 | Pollution & density | [67] |
| SMF/concave-core photonic crystal fiber | 1635.62 | 0.0529 | Chemical and biological | [68] |
| SMF/Medium/Si plate | ---- | 0.41 | Liquid concentration | [18] |
| SMF/side-hole fiber/SMF | 1250 | 0.007 | Biochemical | [57] |
| SMF-a solid-core PCF | 30 | ---- | Various | [59] |
| Sensor Design | Sensitivity (pm/°C) | Range (°C) | Applications | Ref. |
|---|---|---|---|---|
| FOMZI cascading two hump-shaped tapers in SMF | 49 | 20–80 | Machinery processing & Health monitoring | [20] |
| Hetero-structured cladding solid-core photonic bandgap fiber | 90 | 25–1000 | High temperature | [95] |
| Four-core fiber spliced between two SMFs | 209 | 0–50 | Chemical Industry & Health monitoring | [21] |
| Asymmetrical FOMZI with a peanut-like section and an abrupt taper with PCF segment | 11.7 | 25–95 | Curvature sensing applications | [22] |
| SMF-Quartz capillary-SMF (core offset) | 21,200 | 20–27 | Security, Industrial & Biomedical | [96] |
| FOMZI with two-core PCF selectively filled with polymer material | 1595 | 25–100 | Wide range | [97] |
| FOMZI with inscription of a secondary waveguide | High | 450–650 | High temperature | [98] |
| MMF-seven-core fiber-MMF | 55.81 | 25–175 | Wide range | [99] |
| Abrupt tapered fiber FOMZI | 0.0833 dBm/°C | ---- | Nondestructive structural monitoring | [100] |
| SMF-offset fiber with reduced core-MMF | 39.2 | 22–55 | Wide range | [87] |
| FOMZI based on the grapefruit micro-structured fiber | 37 | 30–180 | Wide range | [85] |
| SMF-No Core Fiber -SMF embedded in a liquid-sealed capillary | 5150 | 10–30 | Various | [101] |
| Microfiber mode interferometer embedded in PDMS polymer | 3101.8 | 20–48 | Various | [102] |
| SMF-No Core Fiber-SMF with a PDMS layer | 200.2 | 40–80 | Health monitoring | [103] |
| SMF- Thin Core Fiber- SMF | 9.42–4.93 | 20–90 | Multiparameter measurements | [104] |
| Tapered Photonic Crystal Fiber (PCF) with up-tapered collapsed region | 51.6 | 40–90 | Various | [25] |
| Sensor Design | Sensitivity (nm/m−1) | Range (m−1) | Applications | Ref. |
|---|---|---|---|---|
| FOMZI cascading two hump-shaped tapers in SMF | 10.224 and −4.973 | 0–1.2 | Machinery processing & Health monitoring | [20] |
| Four-core fiber spliced between two SMFs | 20.18 | 0.08–0.22 | Chemical Industry & Health monitoring | [21] |
| Asymmetrical FOMZI with a peanut-like section and an abrupt taper with PCF segment | 50.5 | 0–2.8 | Wide range | [22] |
| Four-core fiber spliced between two SMFs | 2.25 | 1.2316–1.4599 | ---- | [83] |
| Eccentric core fiber in between two SMFs with a small core-offset | 13.49 and −18.4 | 0–1.11 | Wide range | [105] |
| SMF- five-core fiber-SMF | 10.37 | 0–2 | Wide range | [84] |
| MMF-seven-core fiber-MMF | 31.57 | 0.5–1 | Wide range | [76] |
| Abrupt tapered fiber FOMZI | 12.4885 dBm/m−1 | ---- | Nondestructive structural monitoring | [100] |
| SMF-Few mode fiber-SMF | 8.53 dB/m−1 | ---- | Biomedical | [106] |
| Two tapered SMF separated by a small distance | 11.92 dB/m−1 | 0–1 | Various | [107] |
| Tapered Photonic Crystal Fiber (PCF) with up-tapered collapsed region | 7.56 | 0–0.55 | Various | [25] |
| MMF-Hollow Core Fiber-MMF | −11.80 ± 1.30 dB/m−1 | 0.95–2.68 | Various | [76] |
| Sensor Design | Sensitivity (nm/RIU) | Range (RIU) | Applications | Ref. |
|---|---|---|---|---|
| SMF-SMF-tapered fiber with core offset- SMF | 78.7 | 1.33–1.374 | Measure environmental RI | [73] |
| Four-core fiber spliced between two SMFs | 20.18 | 0.08–0.22 | Chemical Industry & Health monitoring | [21] |
| SMF-1st tapered microfiber MMF-2nd taped microfiber MMF- SMF | 10,777.8 | around 1.3334 | Ultra-high RI sensitivity | [108] |
| SMF–MMF–PCF–SMF | 440.32 μw/RIU | 1.33–1.42 | Chemical & Biological | [86] |
| SMF-bend core offset region-SMF | 358.039 | 1.33–1.37 | High sensitivity | [74] |
| FOMZI double tapered SMFs | 380 | 1.33–1.349 | Glucose solution of various concentrations | [77] |
| Four-core fiber spliced between two SMFs | 113.27 | 1.3353 to 1.3549 | ---- | [83] |
| SMF-1st tapered PCF-2nd taped microfiber PCF- SMF | 224.2 | 1.3333–1.3737 | ---- | [109] |
| SMF-Hollow core PCF-SMF | 4629 | 1.0000347–1.000436 | Gas detection | [110] |
| SMF-core offset SMF-SMF | 200 | 1.33–1.37 | Temperature insensitive | [111] |
| Two cascades peanut shape structures | 67.355 | 1.33–1.37 | ---- | [112] |
| SMF-offset fiber with reduced core-MMF | 8.8 | 1.35–1.42 | Wide range | [87] |
| SMF-1st tapered SMF-2nd taped microfiber SMF- SMF | 158.4 | 1.33 to 1.3792 | Wide range | [113] |
| SMF-PCF with core offset -SMF | 252 | 1.333–1.379 | Low temperature sensitivity | [114] |
| A peanut-based shape FOMZI | 67.953 | 1.33–1.36 | ---- | [115] |
| Sharp tapered SMF FOMZI | 415 1103 4234 | 1.332 to 1.384 1.384 to 1.4204 1.4204 to 1.4408 | chemical and biochemical | [72] |
| Mismatched fused FOMZI | 10,372 | ---- | Monitoring corrosion and small compositional changes in NaCl solutions | [116] |
| SMF-tapered multicore fiber-SMF covered by monolayer graphene | 12,617.6 | 1.4144 to 1.4159 | Various | [117] |
| SMF-bend Photonic Crystal Fiber-SMF | 258 | ---- | Various | [118] |
| SMF-TCF-MMF-TCF-SMF fiber structure | 433.60 | 1.3333 to 1.4182 | Biochemical, medical industry | [119] |
| Sensor Design | Sensitivity (pm/°C) | Range (°C) | Applications | Ref. |
|---|---|---|---|---|
| SMF structure with a 45° angle reflector | 13.32 | 19–950 | Extremely harsh environment | [121] |
| SMF -seven-core fiber and a spherical reflective structure | 165 | 250–900 | Extremely harsh environment | [128] |
| Bi-taper at the splicing point of SMF and Thin Core Fiber | 140 | 30–800 | Extremely harsh environment | [129] |
| SMF-SMF with a lateral offset | 5.4 | 39.3–96.9 | Oil/gas exploration and high-voltage power systems | [130] |
| SMF-MMF-Dispersion Compensating Fiber | 47.4 | 30–70 | Wide range | [23] |
| SMF-2 Suspended Core Fiber-SMF | 11 | 20–1100 | High temperature | [120] |
| SMF-Multimode microfiber-SMF | 68.122 | ---- | Various | [124] |
| Sensor Design | Sensitivity (dB/RIU) | Range (RIU) | Applications | Ref. |
|---|---|---|---|---|
| SMF-Thin Core Fiber with lateral offset | −202.46 | 1.42 | For applications insensitive to temperature | [123] |
| SMF-MMF-Dispersion Compensating Fiber | −22 to −5 | 1.30–1.38 | Wide range | [23] |
| SMF-Multimode microfiber-SMF | −17 | ---- | Various | [124] |
| Sensor Design | Sensitivity (nm/°C) | Range (°C) | Applications | Ref. |
|---|---|---|---|---|
| FOSI based on the square-lattice photonic crystal fiber | 7.54 | 25–85 | Wide range | [139] |
| FOSI based on a two semicircular-hole fiber (TSHF) with a germanium (Ge)-doped elliptical core | 0.16 | 40–160 | ----- | [134] |
| FOSI based on two sections of high-birefringence (HiBi) fiber | 17.99 | 40–41 | ----- | [140] |
| Sensor Design | Type | Sensitivity | Amplification Factor | Ref. |
|---|---|---|---|---|
| Two Fabry-Perot (Relative Humidity) | Cascaded | 78.86% RH | 4.8 | [148] |
| Two MZIs (Refractive IndeX) | Cascaded | 44,000 nm/RIU | 3.1 | [149] |
| Two MZIs (Pressure) | Parallel | −60 nm/MPa | 7 | [150] |
| Two Michelson (Curvature) | Parallel | 38.53 nm/m−1 | ---- | [151] |
| Two MZIs (Temperature) | Parallel | 528.5 pm/oC | 17.5 | [155] |
| Sensor Design | Type | Sensitivity | Amplification Factor | Ref. |
|---|---|---|---|---|
| Two Sagnac (Temperature) | Common Cascaded | 13.36 nm/°C | 9 | [152] |
| Two MZIs (Temperature & Curvature) | Common Cascaded | 397.36 pm/°C −36.26 nm/m−1 | 9 | [154] |
| Two MZIs (Temperature) | Common Parallel | −3.348 nm/°C | 11.3 | [156] |
| Sagnac and Fabry-Perot (Temperature) | Different | −29.0 nm/°C | 20.7 | [158] |
| Sagnac and Fabry-Perot (Pressure) | Different | 37.1 nm/Pa | 10 | [157] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Miliou, A. In-Fiber Interferometric-Based Sensors: Overview and Recent Advances. Photonics 2021, 8, 265. https://doi.org/10.3390/photonics8070265
Miliou A. In-Fiber Interferometric-Based Sensors: Overview and Recent Advances. Photonics. 2021; 8(7):265. https://doi.org/10.3390/photonics8070265
Chicago/Turabian StyleMiliou, Amalia. 2021. "In-Fiber Interferometric-Based Sensors: Overview and Recent Advances" Photonics 8, no. 7: 265. https://doi.org/10.3390/photonics8070265
APA StyleMiliou, A. (2021). In-Fiber Interferometric-Based Sensors: Overview and Recent Advances. Photonics, 8(7), 265. https://doi.org/10.3390/photonics8070265





