Adaptation of the Standard Off-Axis Digital Holographic Microscope to Achieve Variable Magnification
Abstract
:1. Introduction
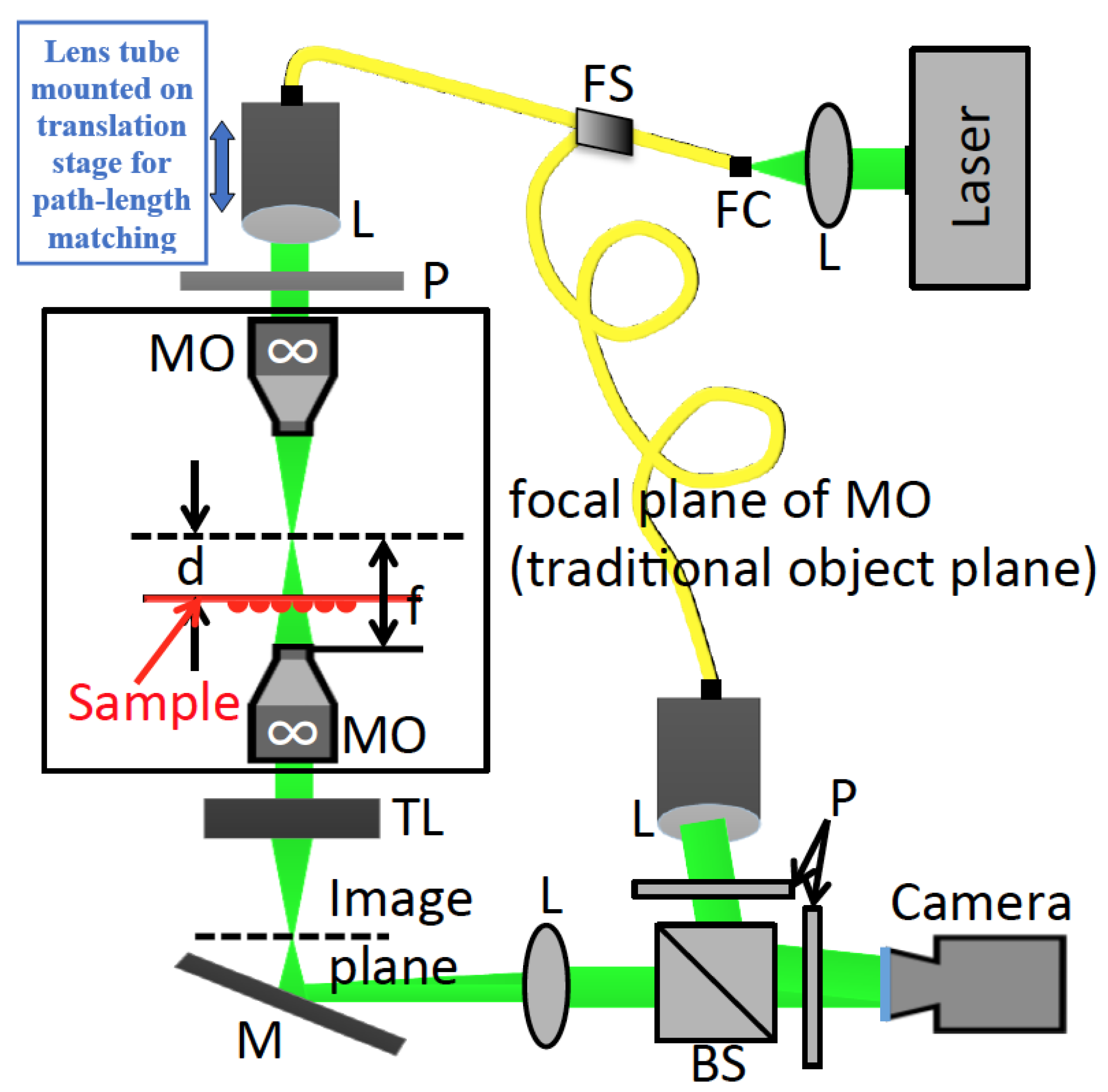
2. Optical System
2.1. Experimental Setup
2.2. Comparison with Setup for Traditional Off-Axis Digital Holographic Microscopy
- The distance between the condenser lens and the MO is altered to ensure that they are physically separated by a distance equal to the sum of their working distances. Since both objectives are infinity corrected objectives, this ensures that the focal planes of both objectives are coplanar. Furthermore, it ensures that a collimated laser beam that enters the back aperture of the condenser will result in a collimated laser beam exiting the back aperture of the MO.
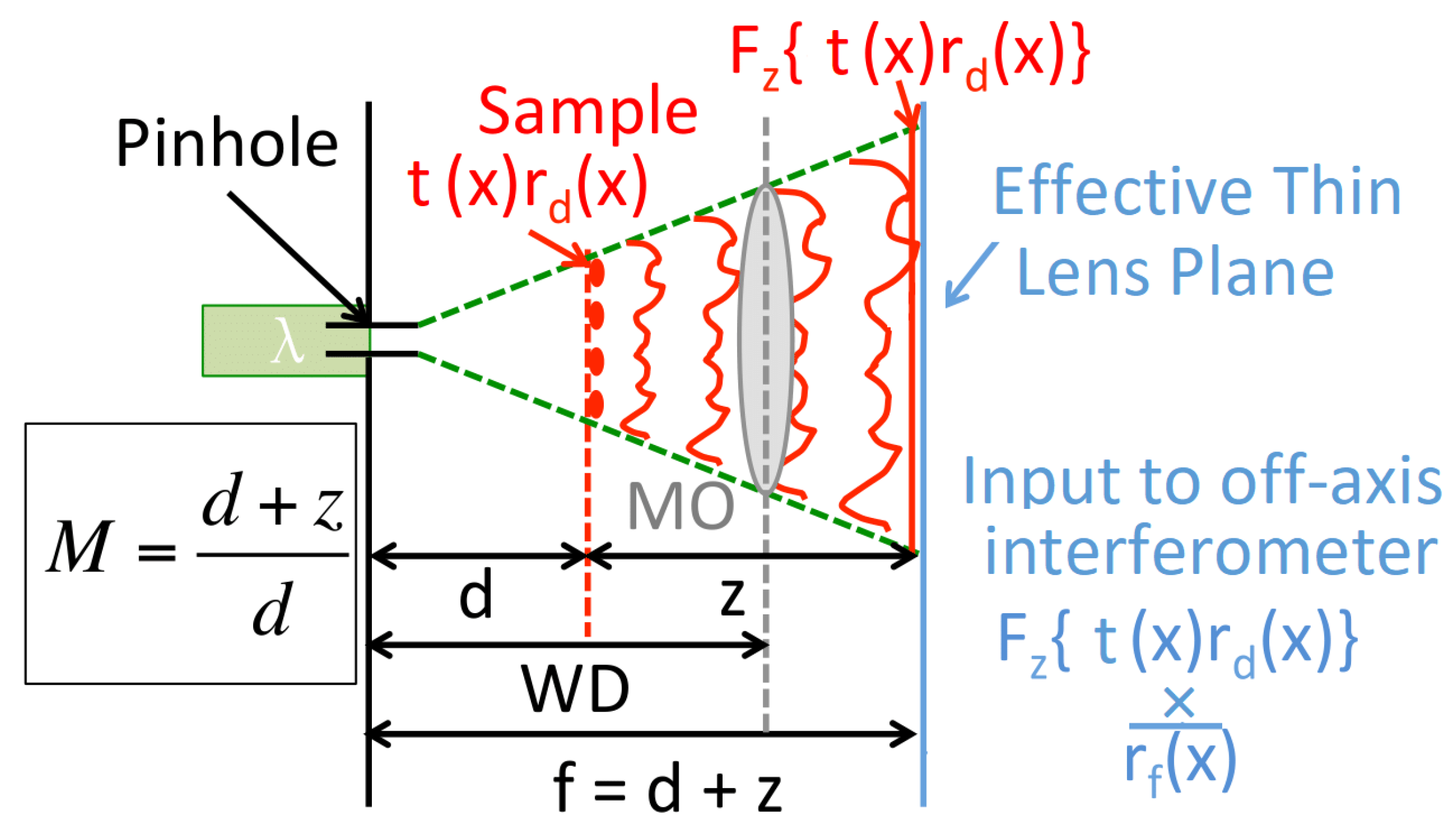
- The sample is no longer limited to a single position at the focal plane of the MO as for the case of traditional off-axis DHM. Instead, the sample can occupy any plane between the focal plane of the MO and the surface of the MO. The location of the sample in this range will determine the magnification of the imaging system with a maximum value of infinity, when the sample is located at the focal plane, and a minimum value that must be less than unity, when the sample is located at the surface of the MO. The relationship between sample location and magnification is explored in more detail in Section 5.
- The camera is not positioned in the traditional image plane of the microscope (i.e., at the focal plane of the tube lens). Instead, a Fourier transforming lens is inserted between the image plane and the camera plane. Thus, a collimated laser beam entering the condenser aperture, and exiting the back aperture of the MO, will be imaged onto the camera. This step guarantees that the full Field-of-View that is afforded by any given (variable) magnification can be captured by the system. This step also ensures that there will be a simple relationship between the object plane and camera plane for any arbitrary object location and resultant magnification, which is described in more detail in Section 4.
3. Extending the Principles of DIHM to Off-Axis DHM with a Microscope Objective
4. Numerical Reconstruction
5. Numerical Aperture, Field-of-View, Magnification
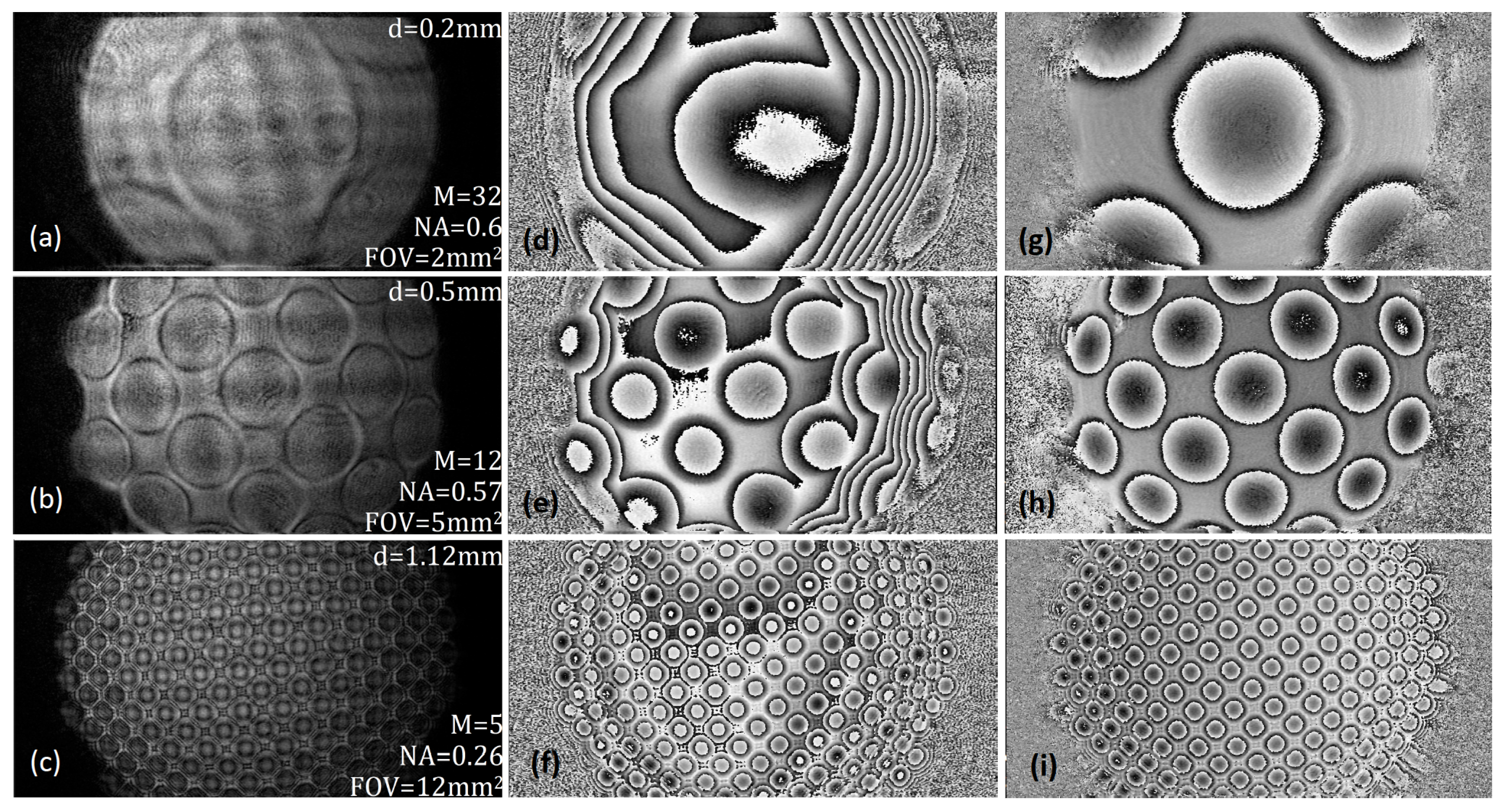
6. Preliminary Results Using a Micro-Lens Array
7. Discussion
7.1. Aberration
7.2. Relationship of the Proposed System to Off-Axis DIHM
7.3. Laser Source
8. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
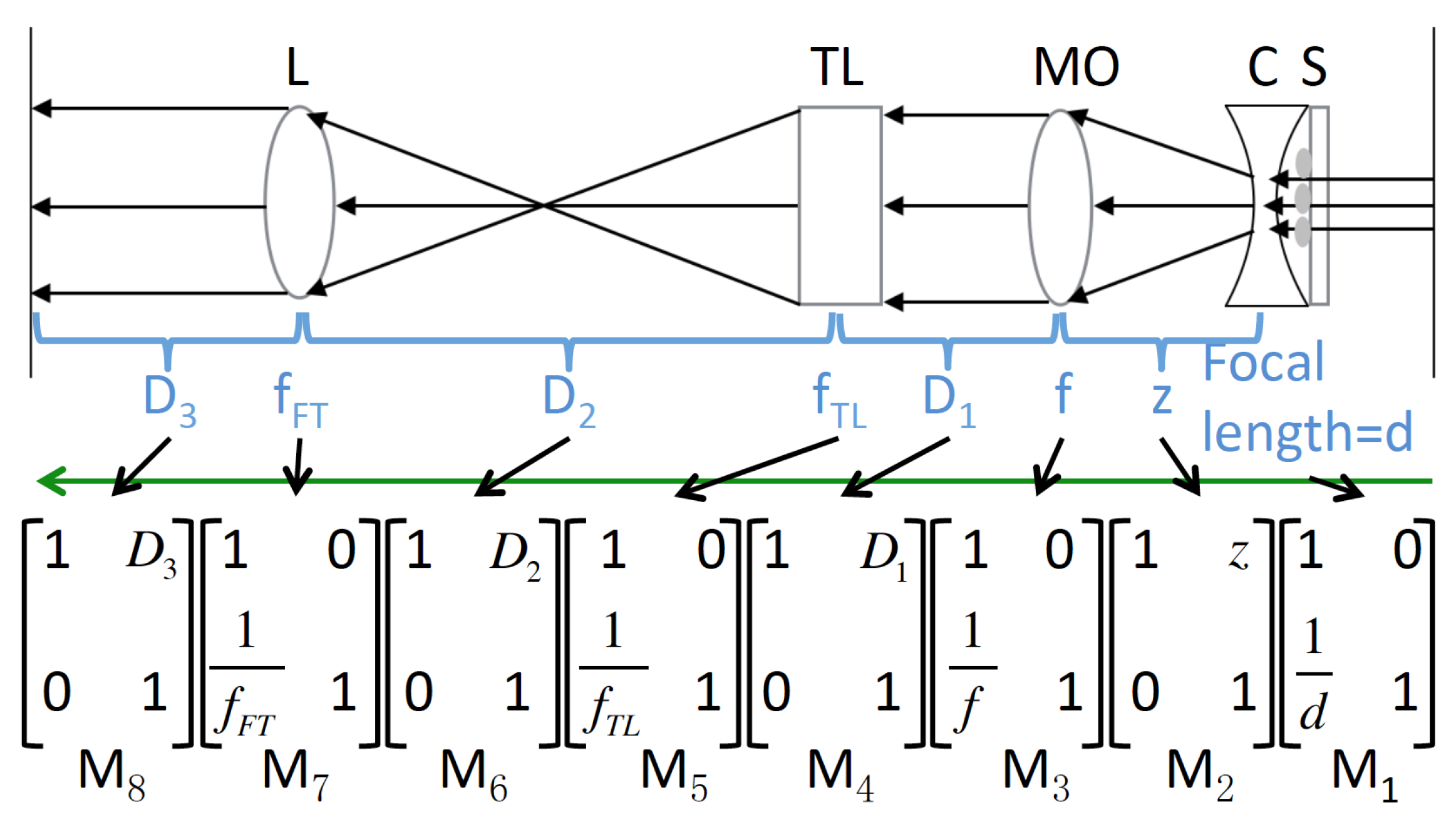
Appendix A. The Ray Transfer Matrix and Its Relationship to Wave Optics
Appendix B. Digital In-Line Holographic Microscopy and Variable Magnification

References
- Gabor, D. A new microscopic principle. Nature 1948, 161, 777–778. [Google Scholar] [CrossRef]
- Leith, E.N.; Upatnieks, J. Wavefront reconstruction with diffused illumination and three-dimensional objects. Josa 1964, 54, 1295–1301. [Google Scholar] [CrossRef]
- Kreis, T.M. Handbook of Holographic Interferometry; Wiley-VCH: Weinheim, Germany, 2005. [Google Scholar]
- Schnars, U.; Jüptner, W.P.O. Digital Holography; Springer: Berlin/Heidelberg, Germany, 2004. [Google Scholar]
- Cuche, E.; Marquet, P.; Depeursinge, C. Spatial filtering for zero-order and twin-image elimination in digital off-axis holography. Appl. Opt. 2000, 39, 4070–4075. [Google Scholar] [CrossRef] [PubMed]
- Kim, M.K. Digital holographic microscopy. In Digital Holographic Microscopy; Springer: Berlin/Heidelberg, Germany, 2011; pp. 149–190. [Google Scholar]
- Molony, K.M.; Hennelly, B.M.; Kelly, D.P.; Naughton, T.J. Reconstruction algorithms applied to in-line Gabor digital holographic microscopy. Opt. Commun. 2010, 283, 903–909. [Google Scholar] [CrossRef] [Green Version]
- Hennelly, B.M.; Kelly, D.P.; Monaghan, D.S.; Pandey, N. Zoom algorithms for digital holography. In Information Optics and Photonics; Springer: Berlin/Heidelberg, Germany, 2010; pp. 187–204. [Google Scholar]
- Kemper, B.; von Bally, G. Digital holographic microscopy for live cell applications and technical inspection. Appl. Opt. 2008, 47, A52–A61. [Google Scholar] [CrossRef]
- Fan, X.; Healy, J.J.; O’Dwyer, K.; Hennelly, B.M. Label-free color staining of quantitative phase images of biological cells by simulated Rheinberg illumination. Appl. Opt. 2019, 58, 3104–3114. [Google Scholar] [CrossRef] [PubMed]
- Garcia-Sucerquia, J.; Xu, W.; Jericho, S.K.; Klages, P.; Jericho, M.H.; Kreuzer, H.J. Digital in-line holographic microscopy. Appl. Opt. 2006, 45, 836–850. [Google Scholar] [CrossRef] [PubMed]
- Garcia-Sucerquia, J.; Xu, W.; Jericho, M.; Kreuzer, H.J. Immersion digital in-line holographic microscopy. Opt. Lett. 2006, 31, 1211–1213. [Google Scholar] [CrossRef]
- Jericho, S.; Garcia-Sucerquia, J.; Xu, W.; Jericho, M.; Kreuzer, H. Submersible digital in-line holographic microscope. Rev. Sci. Instrum. 2006, 77, 043706. [Google Scholar] [CrossRef]
- Garcia-Sucerquia, J. Color lensless digital holographic microscopy with micrometer resolution. Opt. Lett. 2012, 37, 1724–1726. [Google Scholar] [CrossRef] [PubMed]
- Jericho, M.; Kreuzer, H.; Kanka, M.; Riesenberg, R. Quantitative phase and refractive index measurements with point-source digital in-line holographic microscopy. Appl. Opt. 2012, 51, 1503–1515. [Google Scholar] [CrossRef]
- Kreuzer, H.J. Holographic Microscope and Method of Hologram Reconstruction. U.S. Patent 6,411,406, 25 June 2002. [Google Scholar]
- Luo, W.; Zhang, Y.; Göröcs, Z.; Feizi, A.; Ozcan, A. Propagation phasor approach for holographic image reconstruction. Sci. Rep. 2016, 6, 22738. [Google Scholar] [CrossRef]
- Ozcan, A.; Greenbaum, A. Maskless Imaging of Dense Samples Using Multi-Height Lensfree Microscope. U.S. Patent 9,715,099, 25 July 2017. [Google Scholar]
- Rivenson, Y.; Zhang, Y.; Günaydın, H.; Teng, D.; Ozcan, A. Phase recovery and holographic image reconstruction using deep learning in neural networks. Light. Sci. Appl. 2018, 7, 17141. [Google Scholar] [CrossRef]
- Goodman, J.W. Introduction to Fourier Optics; Roberts & Company Publishers: Greenwood Village, CO, USA, 2004. [Google Scholar]
- Greenbaum, A.; Ozcan, A. Maskless imaging of dense samples using pixel super-resolution based multi-height lensfree on-chip microscopy. Opt. Express 2012, 20, 3129–3143. [Google Scholar] [CrossRef]
- Waller, L.; Luo, Y.; Yang, S.Y.; Barbastathis, G. Transport of intensity phase imaging in a volume holographic microscope. Opt. Lett. 2010, 35, 2961–2963. [Google Scholar] [CrossRef]
- Fan, X.; Healy, J.J.; Hennelly, B.M. Investigation of sparsity metrics for autofocusing in digital holographic microscopy. Opt. Eng. 2017, 56, 053112. [Google Scholar] [CrossRef]
- Rhodes, W.T. Light Tubes, Wigner Diagrams, and Optical Wave Propagation Simulation. In Optical Information Processing: A Tribute to Adolf Lohmann; SPIE Press: Bellingham, WA, USA, 2002; p. 343. [Google Scholar]
- Hennelly, B.M.; Sheridan, J.T. Generalizing, optimizing, and inventing numerical algorithms for the fractional Fourier, Fresnel, and linear canonical transforms. JOSA A 2005, 22, 917–927. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Mendlovic, D.; Zalevsky, Z.; Konforti, N. Computation considerations and fast algorithms for calculating the diffraction integral. J. Mod. Opt. 1997, 44, 407–414. [Google Scholar] [CrossRef]
- Memmolo, P.; Distante, C.; Paturzo, M.; Finizio, A.; Ferraro, P.; Javidi, B. Automatic focusing in digital holography and its application to stretched holograms. Opt. Lett. 2011, 36, 1945–1947. [Google Scholar] [CrossRef] [PubMed]
- Colomb, T.; Kühn, J.; Charriere, F.; Depeursinge, C.; Marquet, P.; Aspert, N. Total aberrations compensation in digital holographic microscopy with a reference conjugated hologram. Opt. Express 2006, 14, 4300–4306. [Google Scholar] [CrossRef] [PubMed]
- Colomb, T.; Cuche, E.; Charrière, F.; Kühn, J.; Aspert, N.; Montfort, F.; Marquet, P.; Depeursinge, C. Automatic procedure for aberration compensation in digital holographic microscopy and applications to specimen shape compensation. Appl. Opt. 2006, 45, 851–863. [Google Scholar] [CrossRef] [Green Version]
- Colomb, T.; Montfort, F.; Kühn, J.; Aspert, N.; Cuche, E.; Marian, A.; Charrière, F.; Bourquin, S.; Marquet, P.; Depeursinge, C. Numerical parametric lens for shifting, magnification, and complete aberration compensation in digital holographic microscopy. JOSA A 2006, 23, 3177–3190. [Google Scholar] [CrossRef]
- Ferraro, P.; De Nicola, S.; Finizio, A.; Coppola, G.; Grilli, S.; Magro, C.; Pierattini, G. Compensation of the inherent wave front curvature in digital holographic coherent microscopy for quantitative phase-contrast imaging. Appl. Opt. 2003, 42, 1938–1946. [Google Scholar] [CrossRef]
- Cotte, Y.; Toy, F.; Jourdain, P.; Pavillon, N.; Boss, D.; Magistretti, P.; Marquet, P.; Depeursinge, C. Marker-free phase nanoscopy. Nat. Photonics 2013, 7, 113–117. [Google Scholar] [CrossRef]
- Serabyn, E.; Liewer, K.; Wallace, J. Resolution optimization of an off-axis lensless digital holographic microscope. Appl. Opt. 2018, 57, A172–A180. [Google Scholar] [CrossRef] [PubMed]
- Serabyn, E.; Liewer, K.; Lindensmith, C.; Wallace, K.; Nadeau, J. Compact, lensless digital holographic microscope for remote microbiology. Opt. Express 2016, 24, 28540–28548. [Google Scholar] [CrossRef]
- Girshovitz, P.; Shaked, N.T. Compact and portable low-coherence interferometer with off-axis geometry for quantitative phase microscopy and nanoscopy. Opt. Express 2013, 21, 5701–5714. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bishara, W.; Su, T.W.; Coskun, A.F.; Ozcan, A. Lensfree on-chip microscopy over a wide field-of-view using pixel super-resolution. Opt. Express 2010, 18, 11181–11191. [Google Scholar] [CrossRef] [PubMed]
- Collins, S.A. Lens-system diffraction integral written in terms of matrix optics. JOSA 1970, 60, 1168–1177. [Google Scholar] [CrossRef]
- Healy, J.J.; Kutay, M.A.; Ozaktas, H.M.; Sheridan, J.T. Linear Canonical Transforms: Theory and Applications; Springer: Berlin/Heidelberg, Germany, 2015; Volume 198. [Google Scholar]
- Sheridan, J.T.; Hennelly, B.M.; Kelly, D.P. Motion detection, the Wigner distribution function, and the optical fractional Fourier transform. Opt. Lett. 2003, 28, 884–886. [Google Scholar] [CrossRef] [Green Version]
- Kelly, D.P.; Hennelly, B.M.; Rhodes, W.T.; Sheridan, J.T. Analytical and numerical analysis of linear optical systems. Opt. Eng. 2006, 45, 088201-1–088201-12. [Google Scholar] [CrossRef]
- Latychevskaia, T.; Fink, H.W. Solution to the twin image problem in holography. Phys. Rev. Lett. 2007, 98, 233901. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wu, X.; Sun, J.; Zhang, J.; Lu, L.; Chen, R.; Chen, Q.; Zuo, C. Wavelength-scanning lensfree on-chip microscopy for wide-field pixel-super-resolved quantitative phase imaging. Opt. Lett. 2021, 46, 2023–2026. [Google Scholar] [CrossRef] [PubMed]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fan, X.; Healy, J.J.; O’Dwyer, K.; Winnik, J.; Hennelly, B.M. Adaptation of the Standard Off-Axis Digital Holographic Microscope to Achieve Variable Magnification. Photonics 2021, 8, 264. https://doi.org/10.3390/photonics8070264
Fan X, Healy JJ, O’Dwyer K, Winnik J, Hennelly BM. Adaptation of the Standard Off-Axis Digital Holographic Microscope to Achieve Variable Magnification. Photonics. 2021; 8(7):264. https://doi.org/10.3390/photonics8070264
Chicago/Turabian StyleFan, Xin, John J. Healy, Kevin O’Dwyer, Julianna Winnik, and Bryan M. Hennelly. 2021. "Adaptation of the Standard Off-Axis Digital Holographic Microscope to Achieve Variable Magnification" Photonics 8, no. 7: 264. https://doi.org/10.3390/photonics8070264
APA StyleFan, X., Healy, J. J., O’Dwyer, K., Winnik, J., & Hennelly, B. M. (2021). Adaptation of the Standard Off-Axis Digital Holographic Microscope to Achieve Variable Magnification. Photonics, 8(7), 264. https://doi.org/10.3390/photonics8070264





