1. Introduction
The question of generation and propagation of light fields containing optical vortices [
1,
2,
3] is getting attention in many fields of modern optical science nowadays. The fundamental case of such a field is vortex beam-the well-defined, single beam (as, for example, LG beam [
4,
5]), carrying the optical vortex of any order. Many different problems concerning the generation and propagation of the vortex beam have been considered in the literature so far. Some of them consider optical fields containing the lattice of vortices generated, for example, by three or more plane or spherical waves interference [
6,
7,
8,
9]; or so called composed vortices, i.e., vortices which are generated by two or more overlapping beams [
10,
11,
12,
13,
14,
15,
16].
The study on single vortex beam propagation has started with the most basic problem, that is propagation of the fundamental vortex beam in a free space [
17,
18,
19]. Next, the problem of vortex beam generation by the simple optical element revealing circular symmetry was reported [
20,
21]. Here, the most important part for our study are papers devoted to Gaussian beam propagation through the spiral phase plate (SPP) [
22,
23,
24,
25,
26,
27,
28]. SPP is now one of the most common ways of introducing optical vortex into the laser beam. In more advanced approaches the propagation of vortex beam with broken symmetry or through the system with broken symmetry (like, for example, diffraction by half-plane [
29,
30] or a phase step [
31,
32]) was studied. Most of these asymmetrical cases were studied combining numerical and/or strongly approximated analytical method, especially in case of higher order vortices [
33,
34,
35,
36,
37,
38,
39,
40,
41]. Another highly asymmetrical problem is a vortex beam propagation through the turbid media (e.g., atmosphere) [
42,
43,
44] or vortex generation with polarization optics such as q-plates [
45].
In the paper [
34] the analysis of the Gaussian beam propagation through the off-axis SPP in Fraunhofer approximation is studied. Authors have focused their attention on the vortex point displacement measured by inspecting the asymmetry in intensity distribution at the far field. In the present paper we analyze asymmetrical optical system with Fresnel diffraction theory, which is more general than Fraunhofer one. The analysis of the off-axis high-order vortices is a difficult task. The integrals become highly complicated and some typical tricks often used in the calculations cannot be applied. The good example is stationary phase method [
46], which cannot be used since the phase changes very fast in the vicinity of the vortex point. That is the reason why there are only few publications regarding the exact solutions of asymmetric higher order vortex propagation. In paper [
47] the elliptic vortex beam propagation is studied. The paper [
48] describes the generation of the higher order vortex beam by discretizing spiral phase plate. In paper [
49] the generation of vortex beam through fractional spiral phase plate is studied. In papers [
50,
51] the propagation through off-axis hologram generating the optical vortices is analyzed, also including the effects of misalignment.
In papers [
52,
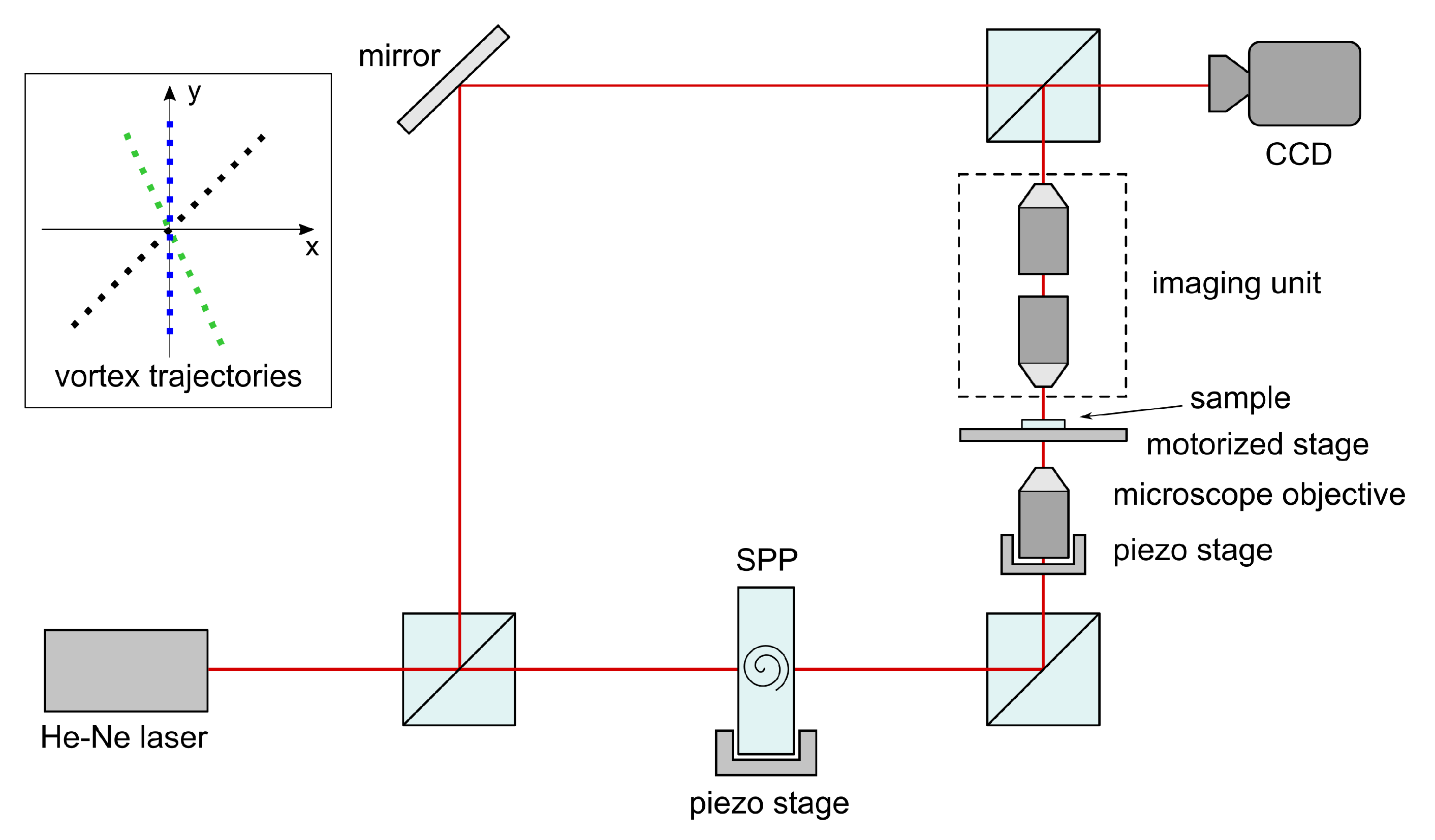
53] we have provided a solution for asymmetrical vortex propagation in optical vortex scanning microscope (OVSM) presented schematically in
Figure 1. In this paper we propose more general solution in terms of Kummer confluent hypergeometric function which can be used for a system of arbitrary number of lenses. This function has been already used for defining the new beam family produced by the four-component system (of circular symmetry)–logarithmic axicon, SPP, amplitude power function and Gaussian function [
54]. Furthermore, the asymmetrical Gaussian vortex beam generated by the off-axis SPP or fork-like hologram has been studied in paper [
55] but in a different way–no propagation through the classical optical system was included. The context of photon entanglement via angular momentum [
56,
57] mentioned in the paper [
55] shows that the analysis of the off-axis Gaussian vortices is important to this very hot topics in modern physics.
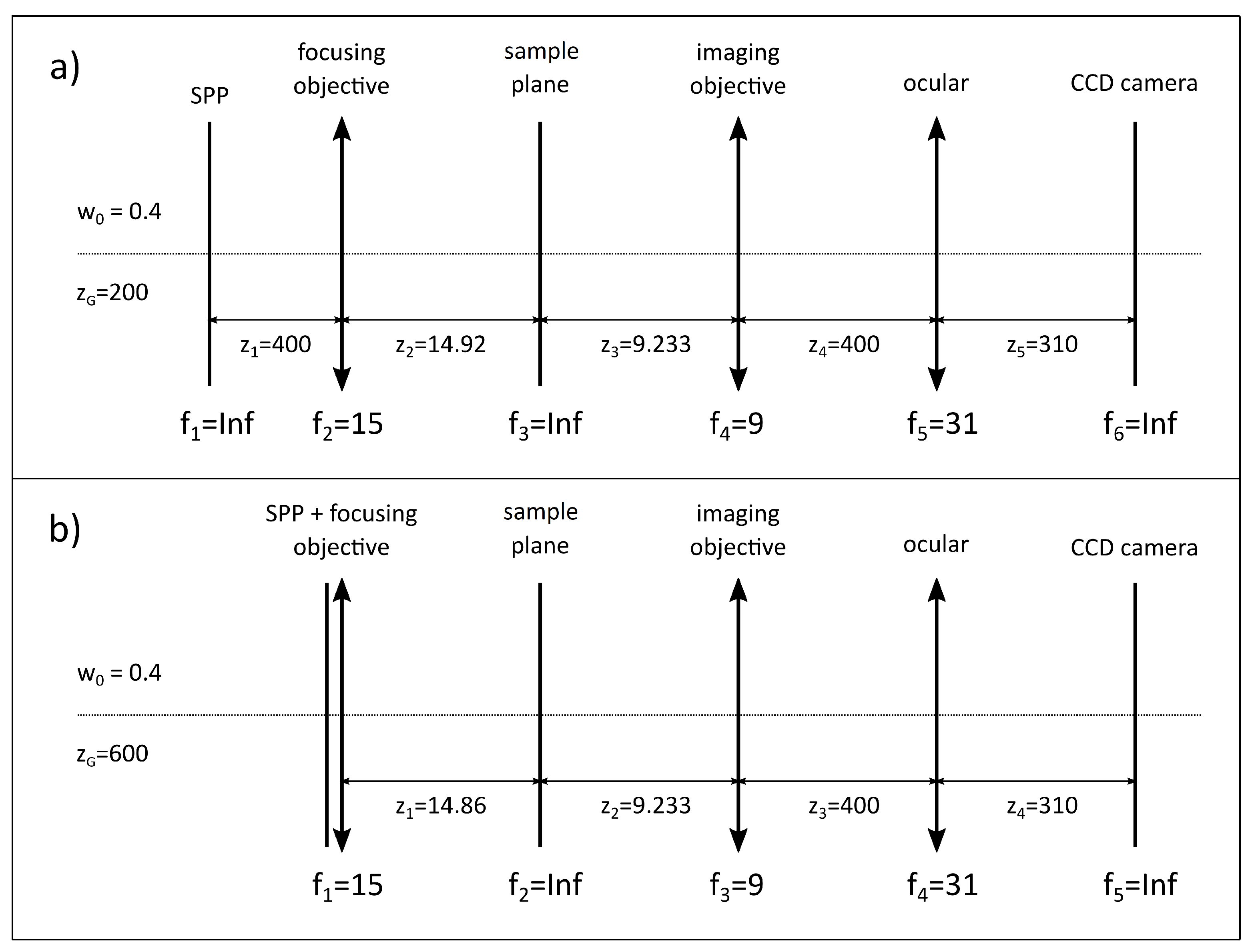
In this paper the optical system shown schematically in
Figure 2 is studied. The system represents the object arm of the OVSM shown in
Figure 1 (in experiment, the reference arm is necessary for reconstructing both amplitude and phase of the object beam. Obviously, in analytical and numerical calculations we do not need it). The system is divided into blocks. Each block consists of one or more elements and is represented by its transmittance function (in case of single element) or the product of the transmittance functions (when there are two or more elements in the given block). In our first approach [
52] the OVSM was reduced to a single block consisting of three elements, i.e., incident Gaussian beam, SPP and focusing lens considered as a single thin element. The image was calculated at the sample plane (noted as sample in
Figure 1). It should be noticed that the SPP can be moved perpendicularly to the optical axis, which breaks the system symmetry. As a result, the vortex point moves inside the focused beam, but the range of this movement is highly reduced due to focusing lens. The inset in
Figure 1 shows the exemplary vortex trajectories as registered in our experimental system. In this way the sample can be scanned with the vortex point (i.e., point where the phase is singular). This technique is named the Internal Scanning Method (ISM) [
37,
39,
52,
53,
58,
59]. In the paper [
53] the system built of three blocks was analyzed. The first block consisted of incident Gaussian beam, SPP and focusing lens, the second was just the sample plane, and the third contained a single imaging lens. Here, we extend the analysis to the fully expanded system shown in
Figure 2b.
Our analysis was performed within the frame of scalar diffraction theory in Fresnel approximation [
60]. The Fresnel diffraction integral for the first block shown in
Figure 2b has a form
where
k is a wave number,
is a wavelength,
is a transmittance function of the SPP,
is an incident Gaussian beam,
is a transmittance function of the focusing lens.
m is a topological charge of the optical vortex; it is an integer–positive or negative,
is a beam waist (of the Gaussian beam),
is a focal length of the focusing lens.
Instead of moving the SPP off the optical axis by the distance
we moved the rest of the system (including the screen) by the same
, which simplified further calculations. As was shown in [
52] the integral Equation (
1) had a solution
where
The parameters
and
are
The main part of this solution is function Kappa
K.
where
We have obtained an interesting result showing that a system having more blocks (with lenses or just planes) can still be described by Kappa function, but with different coefficients
,
,
,
,
. The number of blocks in the system is indicated by superscript (
q) as it has been already done in Equations (
3) and (4). In paper [
53] we calculated explicitly the coefficients
,
,
,
,
up to
, and postulated that it can be done for any (
q). In this paper we derived a recurrence formula for the coefficient for any number of blocks (any (
q)), which is actually a formal proof of our previous claim.
The function Kappa was denoted previously as
G [
52,
53]. Since that time we have improved and generalized our solution. Now the function
G is rewritten in more useful form. In order not to confuse both versions, we denote the present version by capital Kappa
K.
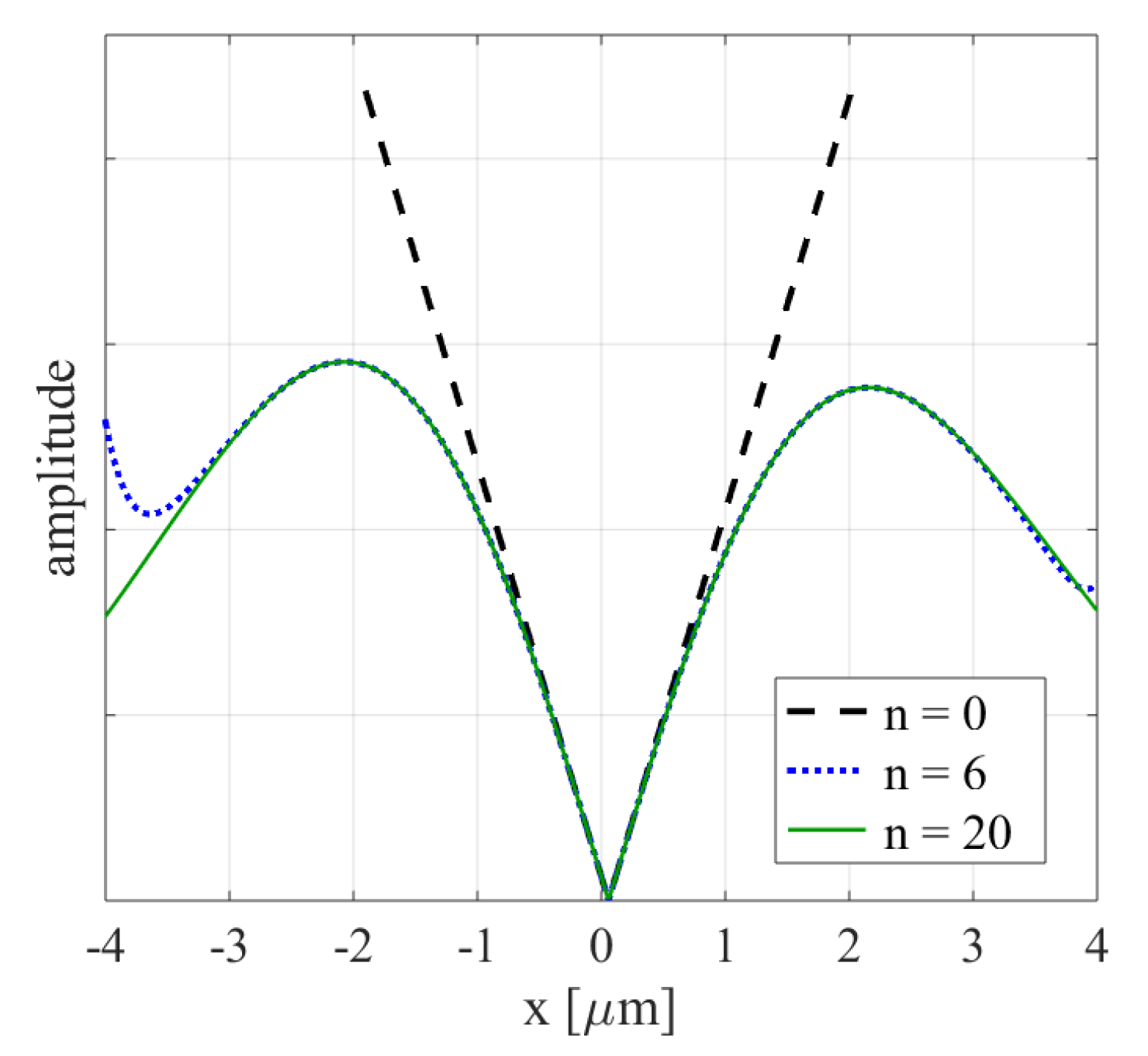
As it was shown in papers [
52,
53], close to the vortex axis the
term is sufficient to evaluate the vortex beam.
Figure 3 illustrates this fact, but now it is plotted for the four-block system shown in
Figure 2b. This is a useful result for us, as we analyze the OVSM images at the central part of the beam, where the
approximation well represents phase and amplitude distribution of our beam.
Our goal was to represent the entire object arm of the OVSM as presented in
Figure 2a. Now the SPP can be separated from the focusing lens which means that focal length for the first block (i.e., SPP block) is
. Next there is a focusing lens with focal length
mm followed by the sample plane for which the focal length is also infinite
. The third block is the imaging lens with focal length
mm and the last one is the ocular lens with focal length
mm, after which we have observation plane (screen). Unfortunately, for the reasons given later in the manuscript the full system could not be analyzed with Kappa function. The SPP cannot be separated from the focusing lens. Therefore, we have to switch to the system shown in
Figure 2b. In this paper we will analyze this system showing the efficiency of our formulas. As can be noticed from
Figure 2b, in our model a sample plane is treated as a separate block. In this way we are able to enhance our calculations in order to analyze the influence of a simple phase object on the vortex beam. Thus, the system shown in
Figure 2b prepares us for this next step.
Certainly, all the blocks may have different focal lengths and positions than the ones shown in
Figure 2 and the Kappa function will still work. However, the important thing is that the first element must be the block containing the SPP, focusing lens and Gaussian beam. Thus, we can model a wide range of classical optical systems with the incident vortex beam generated by the SPP. The analytical modelling means that the analyzed optical system is highly simplified, but on the other hand supports a general insight which is still valuable in solving practical problems. Our work can be also considered as solving the diffraction problem in the paraxial approximation for the specific optical system. However, the expression “analytical modelling” is more preferable since it emphasizes our focus on practical aspects, i.e., development of the OVSM system for which our former results have been already used [
58,
59].
We realize that after this long introduction the Reader may be lost concerning the question of what is new in the presented paper. To avoid confusion the new aspects of this paper are summarized in brief. At first, we extend our previous formulas from three to any number of elements in the optical system. Then, the formulas are written in closed form using the special functions. This step speeds up all calculations and allows proving the hypothesis that the higher order vortices propagate in a stable way in our system, which is a surprising result. We also study in detail the role of coefficient A which plays a role similar to the Gouy phase in case of Gaussian beam. Finally, we show that vortex trajectory behaves like a rigid body while being rotated due to observation plane shift.
The present paper is organized as follows. In
Section 2 the extension of our formulas as well as their closed form for the system consisting of any number of lenses is discussed. In
Section 3 we discussed the role of coefficient
A. In
Section 4 we show the efficiency of our formulas by discussing the effect of vortex trajectory rotation.
Section 5 and
Section 6 discuss and conclude the paper.
2. Analytical Part
In this part our previous results will be extended. In order to build more relevant model of the OVSM we have derived the explicit formula for coefficients
,
,
,
,
up to
, just to analyze the OVSM system shown in
Figure 2b.
where the meaning of
and
can be read from
Figure 2,
,…,
are lens thicknesses. In case of a simple plane we put
.
Following this path a general iterative formulas for coefficient of any (
q) index can be derived. The formulas have a form
The derivation of the above is presented in
Appendix A.
The sums in Kappa function are convergent provided that a series of conditions are hold
Very similar formulas can be derived for negative vortex charge. In that case we can use the same Kappa function but with some multiplying expression and
coefficient multiplied by
.
The derivation of this formula is presented in
Appendix B.
We have also derived a closed formula, but with a special function, which is given below. The derivation is discussed in the
Appendix C.
where
is the Kummer confluent hypergeometric function [
61]. The calculations using closed formula are much faster, but as we will show both formulas Equations (
3) and (11) are helpful in understanding the vortex beam propagation through our system.
The sum of two
B coefficients in Equation (8b,c) is a complex expression which can be easily decomposed into real and imaginary part. From Equation (8b,c) we got, for the real part,
For the, imaginary part, we got
The form of coefficients
,
,
depends on the value of
q.
For example for
we get
Formulas in Equations (
12) and (
13) show that y-coordinate is a member of imaginary part and x-coordinate is a member of real part of the
expression. This leads us to simple formulas for vortex point trajectory. The vortex point (zero amplitude point) is at the place where both imaginary and real part are equal to zero [
1,
2,
3].
From the above formula we may conclude that the vortex trajectory as a function of
, for the given z is a straight line. We may also find a plane where the vortex trajectory is perpendicular to the SPP shift. Using Equation (
16b) the condition is
In the paper [
52] we have formulated the hypothesis that the higher order vortices (
) do not split even when
. The higher order vortices are classified as structurally unstable [
62], i.e., they are supposed to split into single order vortices even under small phase or amplitude perturbation [
63,
64,
65]. Nevertheless, the formulas in Equation (11) proof the stability hypothesis in an explicit form. The factors in front of the Kummer confluent hypergeometric function are just a vortex term.
The place where the real and imaginary part of
+
is zero indicates the position of the vortex point of the
m-th order. Since the whole term is at power
m, the
m-order vortex does not split for any
. The result is very interesting, which is illustrated in
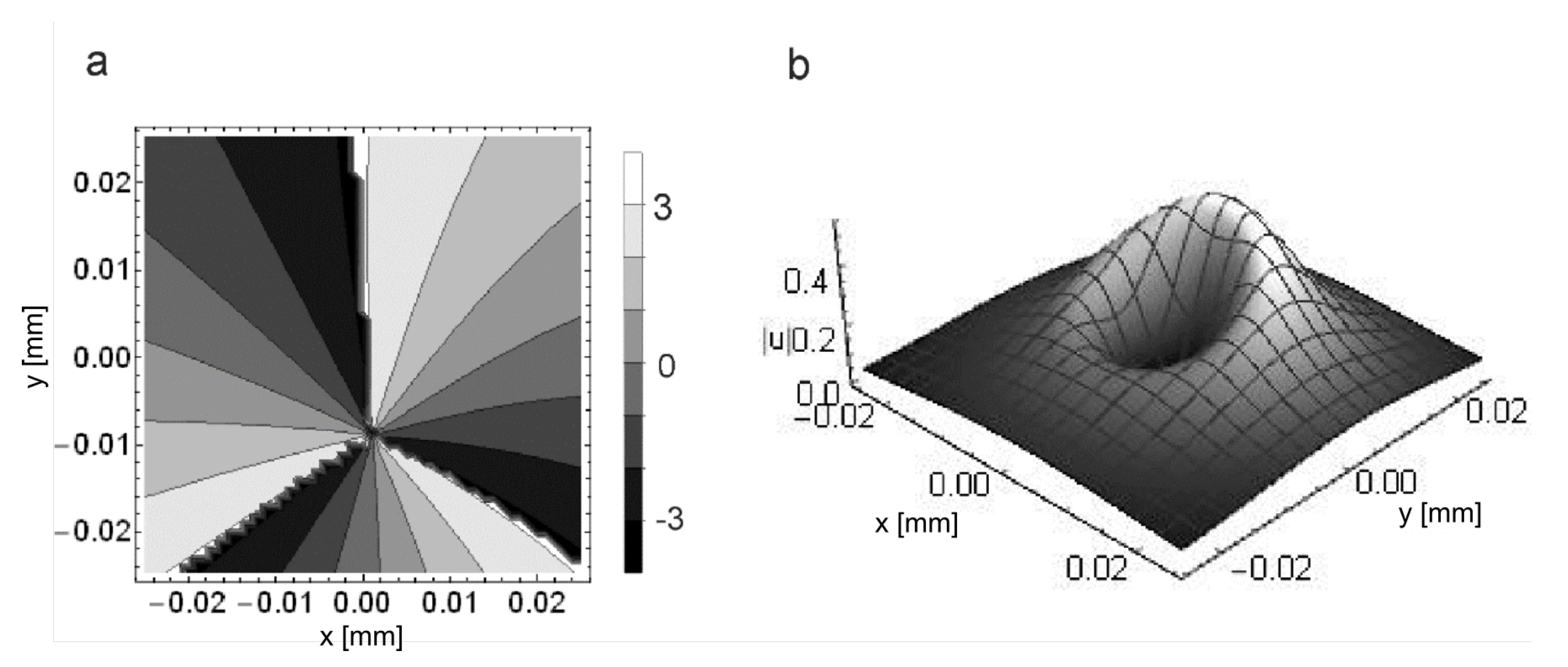
Figure 4.
The situation would not be strange if the higher order optical vortex were stable for a given non-zero value
. However, changing the
continuously breaks the circular symmetry of the phase distribution at the SPP plane with no harm for the higher order OV stability. We could also start our analysis at the sample plane, where both phase and amplitude distribution symmetry are broken (
Figure 5). Nevertheless, the propagation of this input beam through any number of lenses will not split the higher order vortices. In paper [
55] similar results were obtained for a simpler system, i.e., Gaussian beam illuminating the off-axis SPP.
We can go even further. Adding the term inside the bracket in Equation (
18) and multiplying
or
coefficients by any number, but in such a way that the coefficients by
remain real (or become imaginary) and coefficient by
remains imaginary (or becomes real) may change the position of the vortex point and its phase distribution (
Figure 5c) but still the higher order vortices will be stable while propagating through our system. However, changing other parameters, like, for example, binomial factor by coefficients
B in Equation (5) splits the higher order vortices immediately (
Figure 6).
To summarize this part: We have shown that the higher order vortices when propagating through the set of classical lenses described in paraxial approximation will not split regardless of asymmetry introduced by the off axis position of the SPP. Moreover, we may perform some more symmetry breaking operation (as shown in
Figure 4c) in Equation (8c,d), provided that the coefficients by
x remain real (or imaginary) and by
y imaginary (or real). So, the conclusion is that the very basic optical system does not split the higher order vortices even if the input phase and amplitude distribution is highly non-symmetrical. In many cases, such an unusual stability results from deeper physical rules. There is a question if this is also a case here. So far we have no answer to this question. What we can learn now is that classical optical system is somewhat special, at least when being described in paraxial approximation and illuminated by Gaussian beam with the vortex beam introduced by SPP. In the next section the special role of the coefficient
A will be studied.
3. Coefficient A
In our further discussion we will refer to two specific examples of the OVSM models. One with the separated SPP plate and focusing lens (
Figure 2a) and the second one with SPP plate and focusing lens working as single thin element (
Figure 2b).
The coefficient has relatively simple form. It depends neither on the x and y coordinates nor on SPP shift . However, it plays a crucial role in vortex beam phase evolution. Unfortunately it cannot be totally taken outside the first sum in Kappa function. On the contrary, in Equation (4) it can be entirely assimilated inside the first sum, but in the present form some mathematical aspects can be noticed in a more clear way.
The first important point is that the coefficient
A is responsible for breaking the Kappa function convergence. In the OVSM this happens when the SPP is separated from the focusing lens, and coefficient
A has a singular point (
Figure 7a,b). At first we will analyze the system with two blocks, the SPP and the focusing lens (sample plane is an observation plane in this case), so we need the second order coefficient
. The coefficient
contributes to the Kappa function as
, so the term in front of the first sum takes form (for
).
There exists a range of such positive
for which the conditions in Equation (
9) fail. In particular there is a
value that the
equals zero and the whole term in Equation (
19) becomes zero. Thus, the whole Kappa function is equal to zero, which shows that it does not reflect the true behavior of the focused vortex beam. Moreover, the Kappa function encounters a
-jump in this point. This
value can be computed from the formula
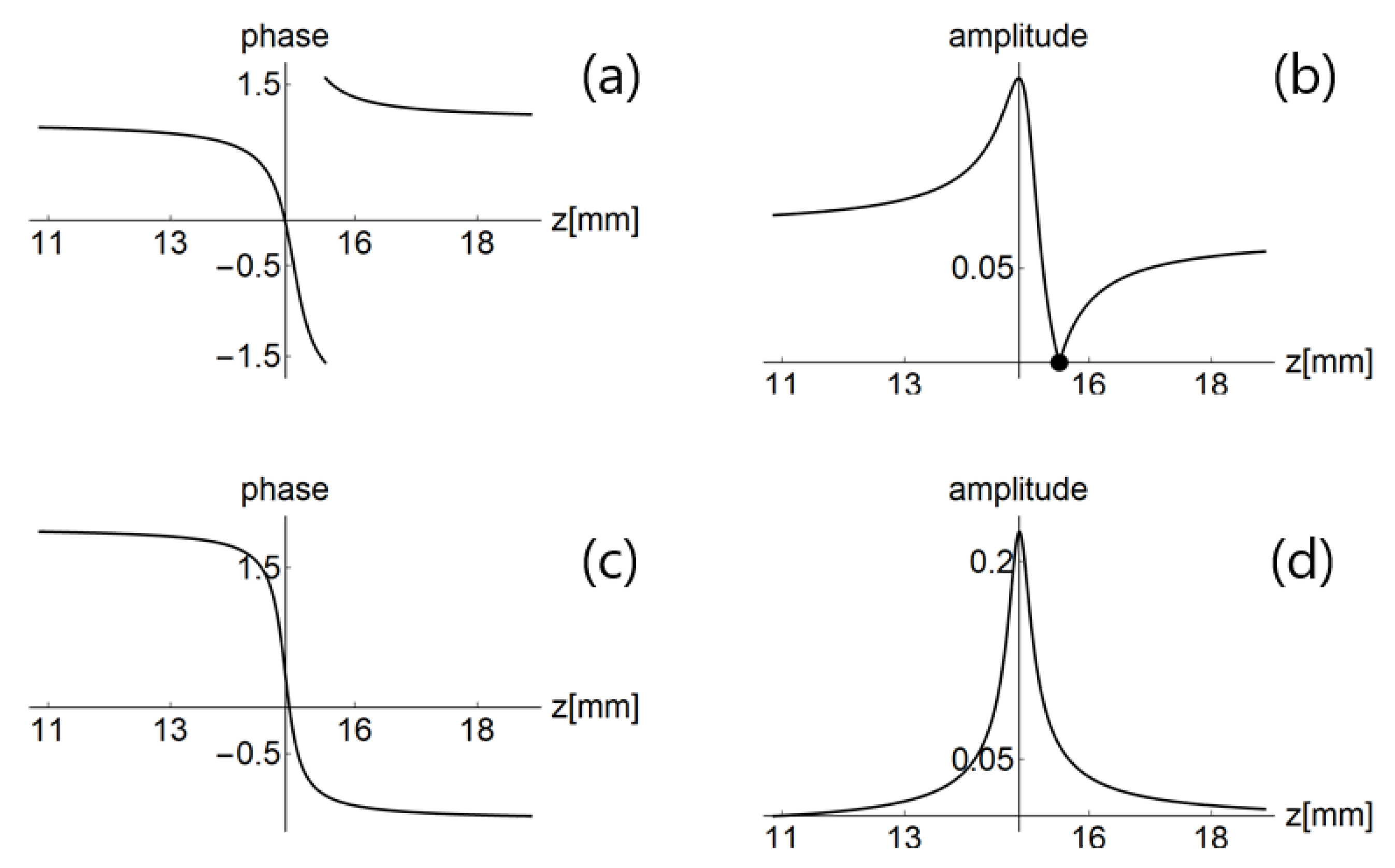
Figure 7a,b illustrates the problem. As we can see for the
mm (calculated from Equation (
20)) the
term equals zero.
When the SPP and focusing lens are joined, we only need a coefficient
which has a simple form, free of our problem (at least for the OVSM optical system). This is illustrated in
Figure 7c,d. The higher order terms
(
) behave in a similar way. When the SPP and focusing lens work as a single element, they meet the conditions in Equation (
9), for a reasonable OVSM configuration. When
the set of conditions in Equation (
9) is more complicated. The detailed study of this problem would explode the volume of this paper, so we will not follow this path. Importantly, when the SPP and focusing lens work separately, the condition in Equation (
9) fails for any
in case of reasonable OVSM configurations. From a practical point of view, it is enough to check if the
factor behaves like in
Figure 7c,d, which is the case in the system shown in
Figure 2b. It is worth noticing here that when using the ISM, all
parameters are fixed, so the test for conditions in Equation (
9) is not difficult. When we move any element of our system along the optical axis the conditions in Equation (
9) have to be checked for the whole range of
coordinates.
For the reason explained above we cannot use our formulas to the system shown in
Figure 2a. Instead, we have to limit our study to the system shown in
Figure 2b, when both SPP and focusing lens work together. Certainly for the forbidden area the numerical modeling of our optical system is still possible and effective.
We can also find
coefficient inside the first sum in Kappa function. Now, we multiply this term by
, located in front of the first sum, so we have
The first term (for
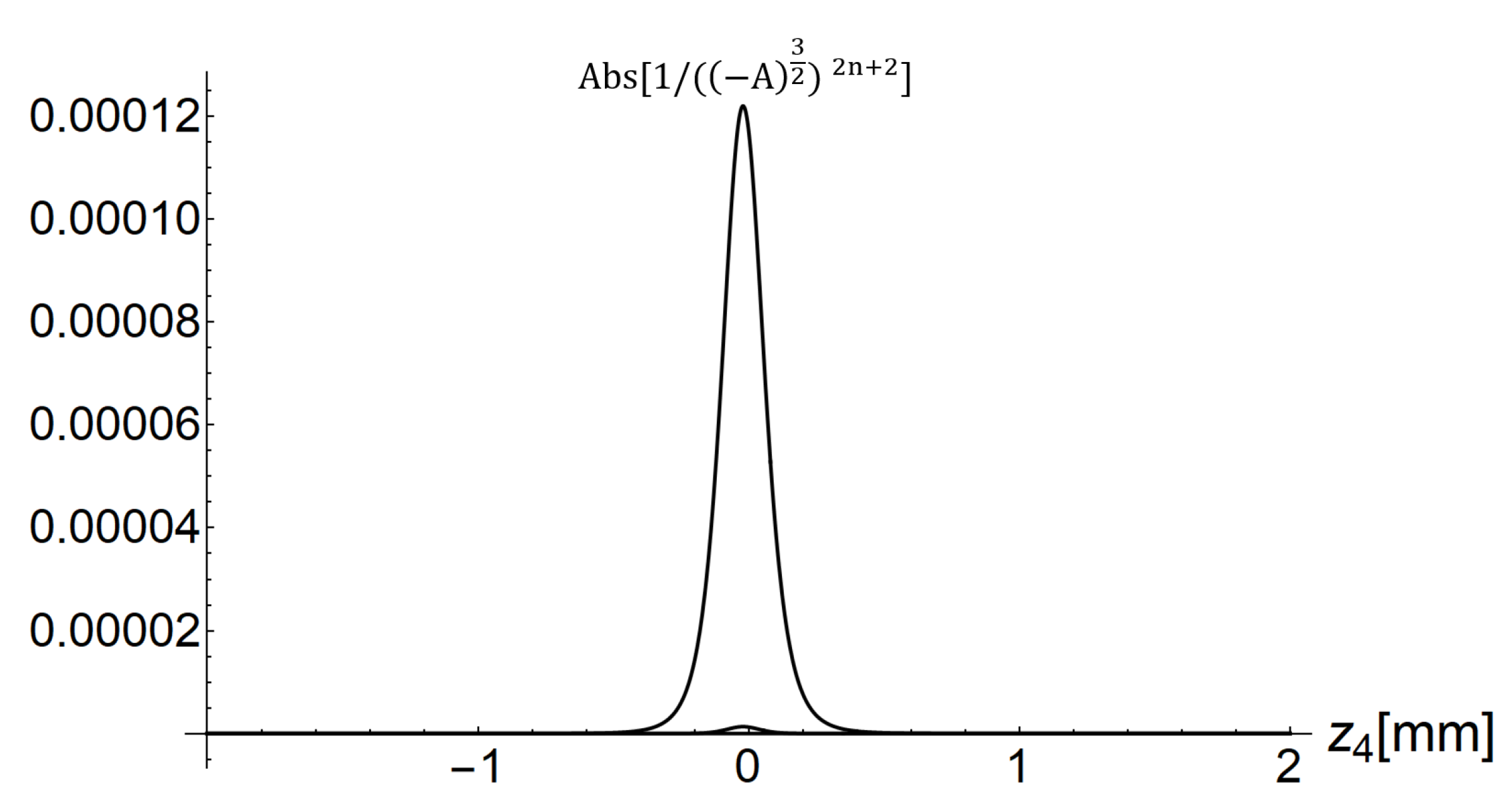
) has the largest influence on the phase and amplitude of the Kappa function The next terms rapidly drop in their values. The plot in
Figure 8 is done for
, but it represents the typical curve for expressions in Equation (21a,b) for any
q, provided that we avoid the forbidden area defined by conditions (9). As we can see the part for
strongly dominates over the part for
. The next terms (for
) are invisible in the figure scale. This domination is particularly strong at the beam center, when
and
coordinates are small (
Figure 3). When the
and
become larger, the coefficient grows rapidly with increasing
n and things become more complicated.
Figure 7c suggests that the
coefficient may play a primary role in phase evolution after the SPP, i.e., when changing the position of any element behind the SPP or SPP and focusing lens themselves. This is illustrated in
Figure 9a. If
and
, there is no phase rotation when changing the position of the focusing lens along the z-axis. We can conclude that in the OVSM system the coefficient
plays, in some respect, the similar role as the Gouy phase in the Gaussian beam. When the
things become more complicated. The off-axis position of the SPP plate introduces a phase value dependence on
being inside the coefficient
(
Figure 9b).
The other coefficients (but
,
) play minor role. The coefficient
and
are responsible for the vortex trajectory evolution as a function of SPP shift
. This dependence is linear, but as has been already shown, the direction of vortex trajectory becomes perpendicular to SPP trajectory when the condition in Equation (
17) holds. In the previous papers [
52,
53] this fact was proved for the small
. Having the formulas in Equation (11) we can conclude that they hold for any
. In paper [
59] precise experiments were reported which confirm the theoretical results.
The coefficient collects all constant factors. It must be observed when we analyze the rotation of the beam phase while moving the first block in the optical system along the z-axis. When the first element moves away or toward the laser source, the phase of the incident Gaussian beam changes. This incident phase is a part of coefficient .
The coefficient
multiplies the first sum in function Kappa. Due to the
factor, the
C coefficient is responsible for the equiphase lines curvature (
Figure 10). When
the equiphase lines are straight (for
). Since the
coefficient contributes to the Kappa function as
, where
is an imaginary part of the
C coefficient, and typically
its contribution to the amplitude is small, especially for small
.
4. The Ice-Skater Effect
To see the effectiveness of our formulas we study the correlation between the focused beam radius and vortex trajectory rotation (see the inset in
Figure 1). The hypothesis was that the vortex trajectory behaves like a rigid body. Consequently we assumed that in the closed system of a focused vortex beam the angular momentum
L is conserved, thus
. Regardless of the assumed model (point mass, flat disk, cylinder, etc.), the moment of inertia
I is always proportional to the squared distance
r from the rotation axis, i.e.,
. Therefore, the rotational speed
of the vortex trajectory shall satisfy
. In our case,
is the first derivative of the trajectory inclination angle with respect to
z (
) and
r is the radius of the vortex bright ring, i.e., the distance between points of zero and maximal intensity (e.g.,
mm for the vortex analyzed in
Figure 3). Both the radius of the converging vortex beam and the rotational speed of the trajectory depend on the axial distance
z. Obviously, as
z approaches the focal point, the vortex radius decreases whereas the rotations speed up. As the beam focusses, the radius reaches its minimum and the speed is maximal. This is a direct analogy to the ice skater pulling their arms in for a faster spin. Such a rigid body mechanics approach to the vortex beam has been already studied by Bekshaev et al. [
11], for free propagating Gaussian beam with optical vortex. The authors concluded that it is related to the Gouy phase dynamics. We have stated that the coefficient
A plays the role of the Gouy phase in our case. Indeed, from Equations (
12) and (
13) we may calculate the angle of the trajectory inclination as
Calculating the angle of the equiphase line for the A coefficient we get exactly the same formula.
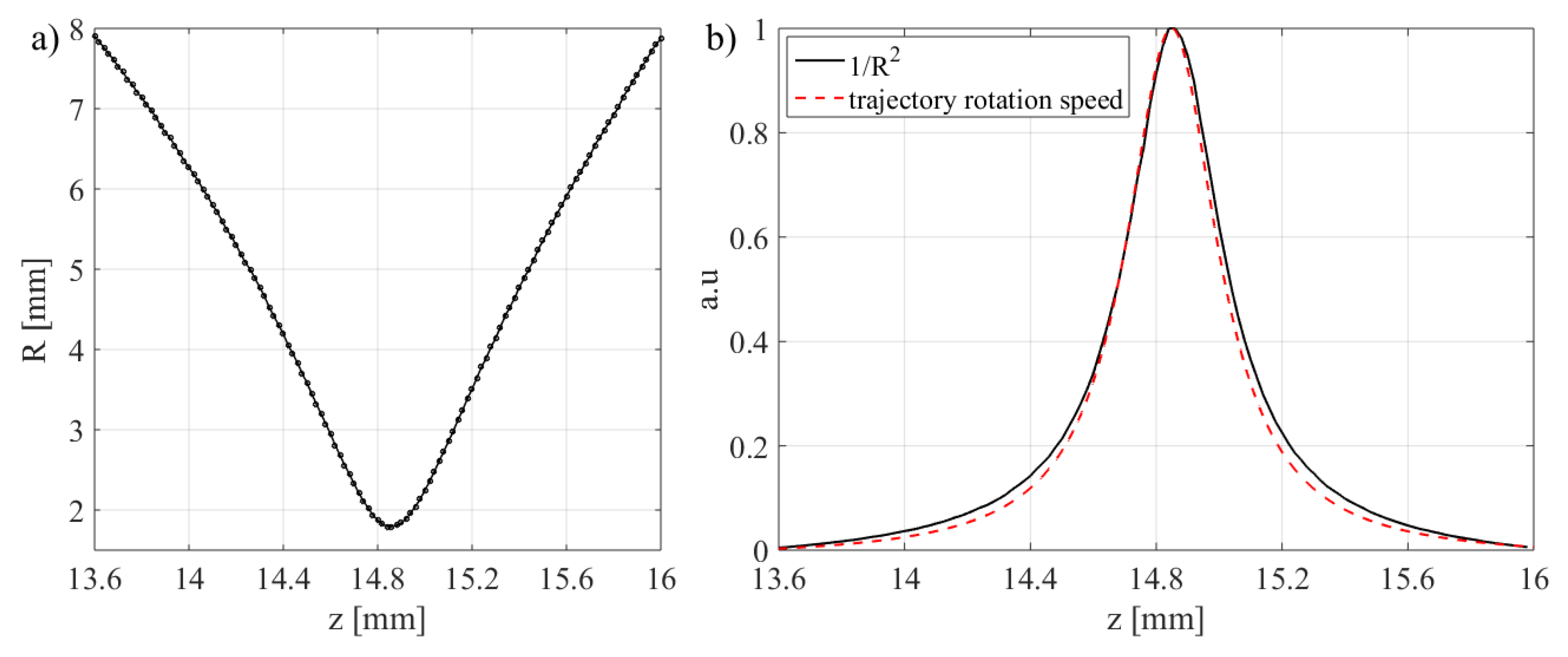
Here we present the results calculated at the exit of the setup (camera plane) shown in
Figure 2b. The default z-position is where the the focal point of the beam lies very close to the critical plane (plane where the trajectory is perpendicular to the SPP shift
). Defocusing is performed by moving the focusing objective along the optical axis without changing the position of other elements. Thus, the plane at which the vortex beam is imaged is at different distances from the focus resulting in different vortex radii, as shown in
Figure 11a. These calculations require running the Kappa function once for each point on the graph. On the other hand, computing the vortex trajectory rotation speed
is much easier. In paper [
39] the formula for
was given as:
In fact the formula in Equation (
24) was derived for the vortex trajectory rotation at the sample plane for the approximated linear case (
). However, the new formulas in Equation (11) allow to extent their applicability for general case. The classical imaging preserves the vortex trajectory orientation as was shown experimentally in [
53,
58]. So we expect that the angle rotation at the image plane is the same as in the sample plane.
The obtained relation between the rotational speed and the defocusing is presented in
Figure 11b, red. Applying the above rigid-body reasoning,
was compared with the inverse square of the vortex radius (
Figure 11b, black). There is a clear agreement between the two curves supporting the hypothesis of rigid-like behavior of vortex trajectory.
5. Discussion
There is a growing interest in the exact theory describing the propagation of the optical vortex beams in the optical system, in particular in a system with broken symmetry. The reasons are both understanding the physics of electromagnetic waves (also in quantum picture) and practical applications. Some examples concerning both science or applications can be found in [
11,
14,
24,
33,
34,
55,
57,
59,
63,
65,
66,
67], but the list of papers is much longer. In this paper we have enhanced the results presented in our former works [
52,
53]. The Kappa function describing our system can be applied for any numbers of lenses. We have derived the coefficient for Kappa function for any numbers of elements. We have also enhanced the formulas for the vortex trajectory. Moreover, we have found a closed form of our solution in Equation (11) using the special function. This new form proved that in classical optical system the higher order vortices do not split even if the circular symmetry is broken. This is true under the conditions of paraxial approximation. This result is surprising and it is hard to check it experimentally. We cannot use an optical system in paraxial approximation. Moreover, any real system introduces errors which break the beam symmetry in a way different than allowed by our theory. This immediately splits the higher order vortices forming a constellation of the first order ones. Nevertheless, the experiment reported in [
52] suggested the mass center of such a constellation moves as ideal higher order vortex. There is also an open question: what is the reason for such a special behavior?
The study on
coefficient have shown that it is responsible for breaking the conditions in Equation (
9). As a result, we cannot analyze the OVSM system with separated SPP plate and focusing lens. The
coefficient has an important role in the rotation of vortex beam phase, which is similar to the role of the Gouy phase in Gaussian beam. The second important factor is the SPP shift
. When we are close to vortex core we can limit the sum range (in Kappa function) to
, which is also the result of the
coefficient specific behavior (
Figure 8).
We have shown that the vortex trajectory behaves like a rigid body. The range of the trajectory shrinks when the beam converge, so its rotation speed increases according to the rules describing the rigid body behavior. The vortex trajectory rotation is strictly related to the phase rotation of the
coefficient. In [
11] the same relation was shown for free propagation of axial Gaussian vortex beam and the Gouy phase. It is worth noticing here that our theoretical results concerning the vortex trajectory dynamics as well as the shape of the beam at the observation plane were in agreement with numerical simulations performed with the software dedicated for solving diffraction problems.
The question is if we can enhance our results for more general optical systems like, for example, the system generating Laguerre–Gaussian beam with the SPP of the special profile [
28]. There are various types of vortex beams which are studied for their practical potential in optical metrology, manipulation and telecommunication. Unfortunately, we have not found any general way for transforming our results to a wider class of possible vortex beams. So far any system variation generates difficult diffraction problems which must be solved separately.