A Highly Sensitive, Polarization Maintaining Photonic Crystal Fiber Sensor Operating in the THz Regime
Abstract
1. Introduction
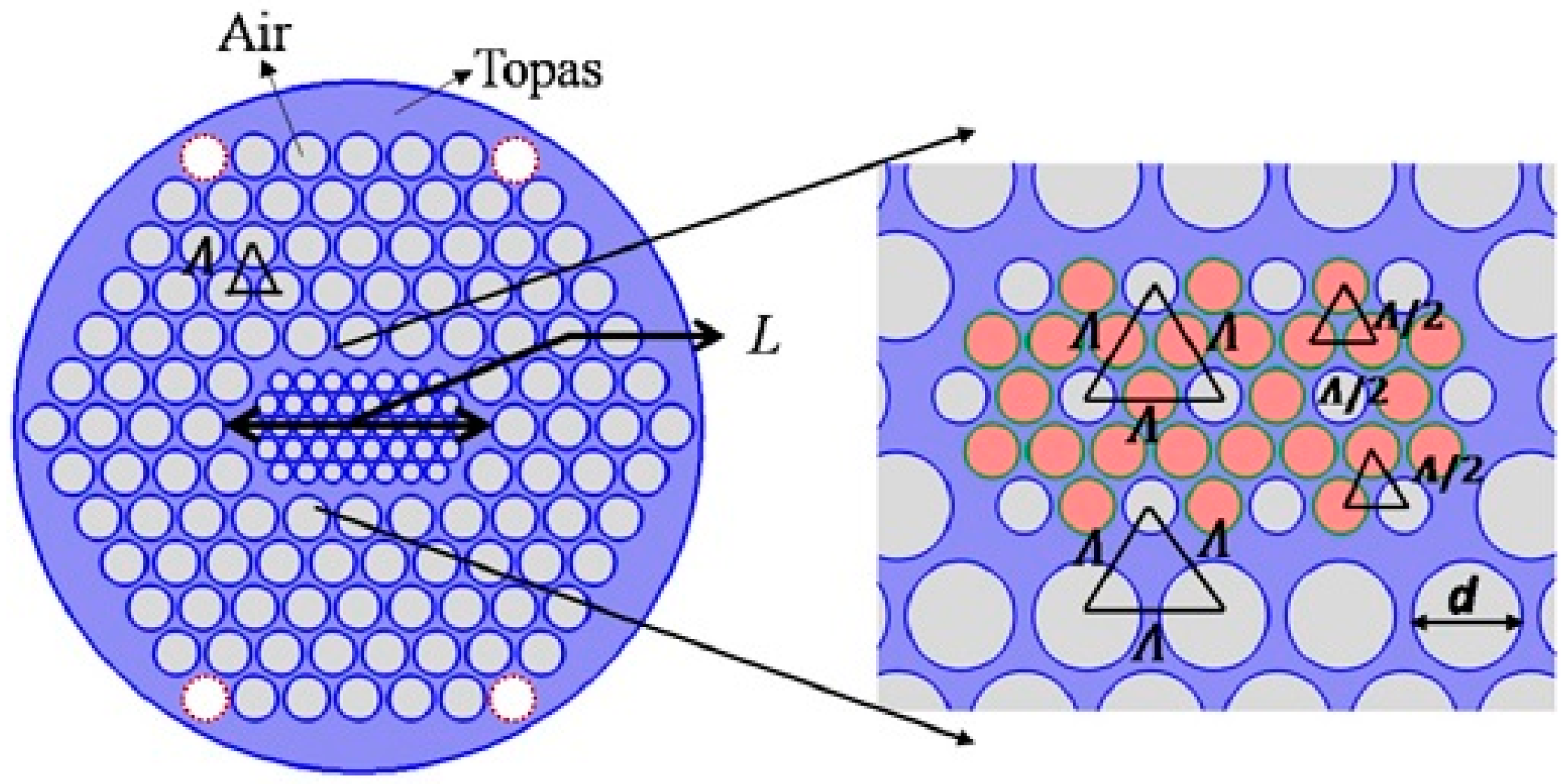
2. Geometric Structure
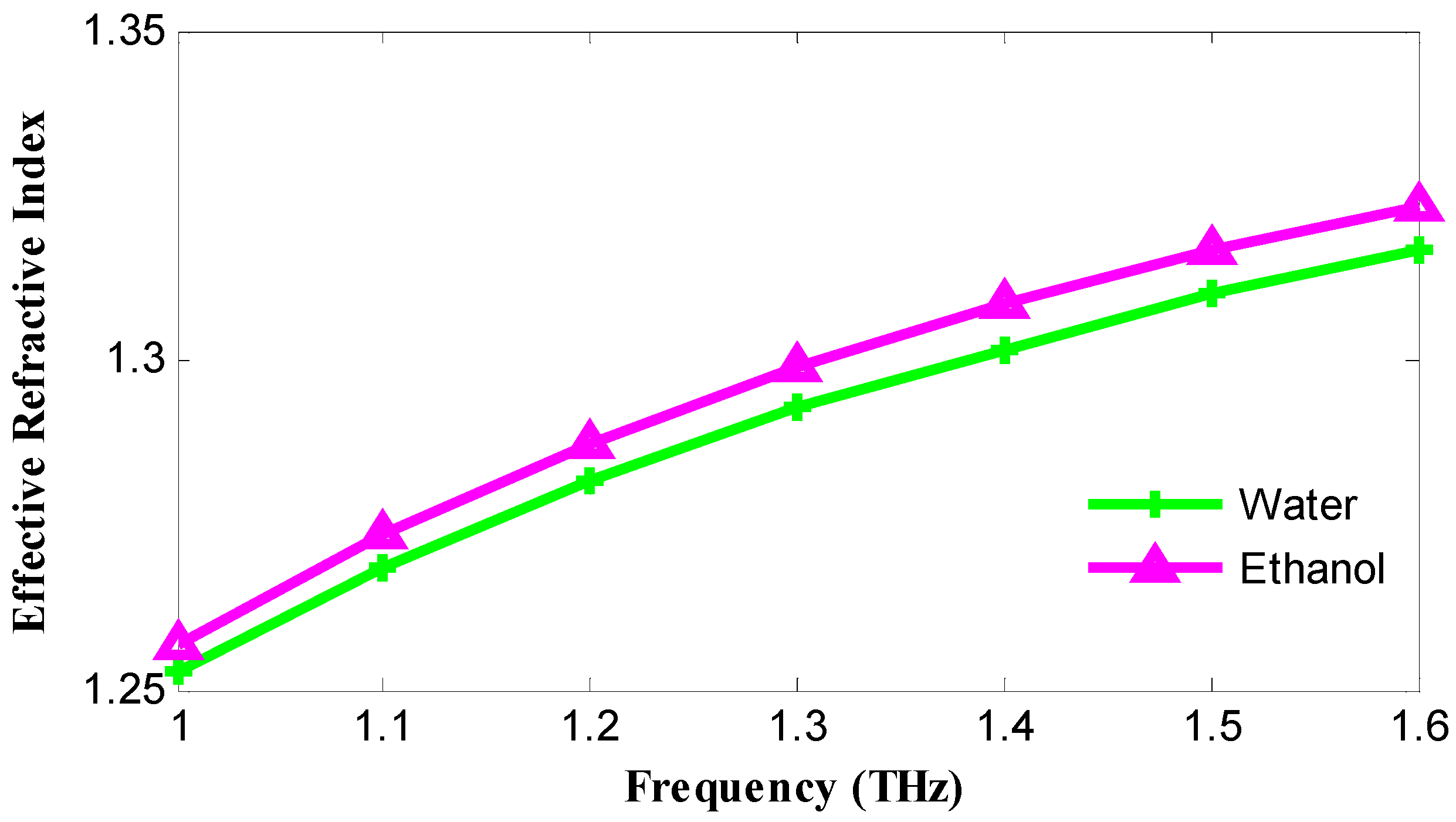
3. Results and Discussion
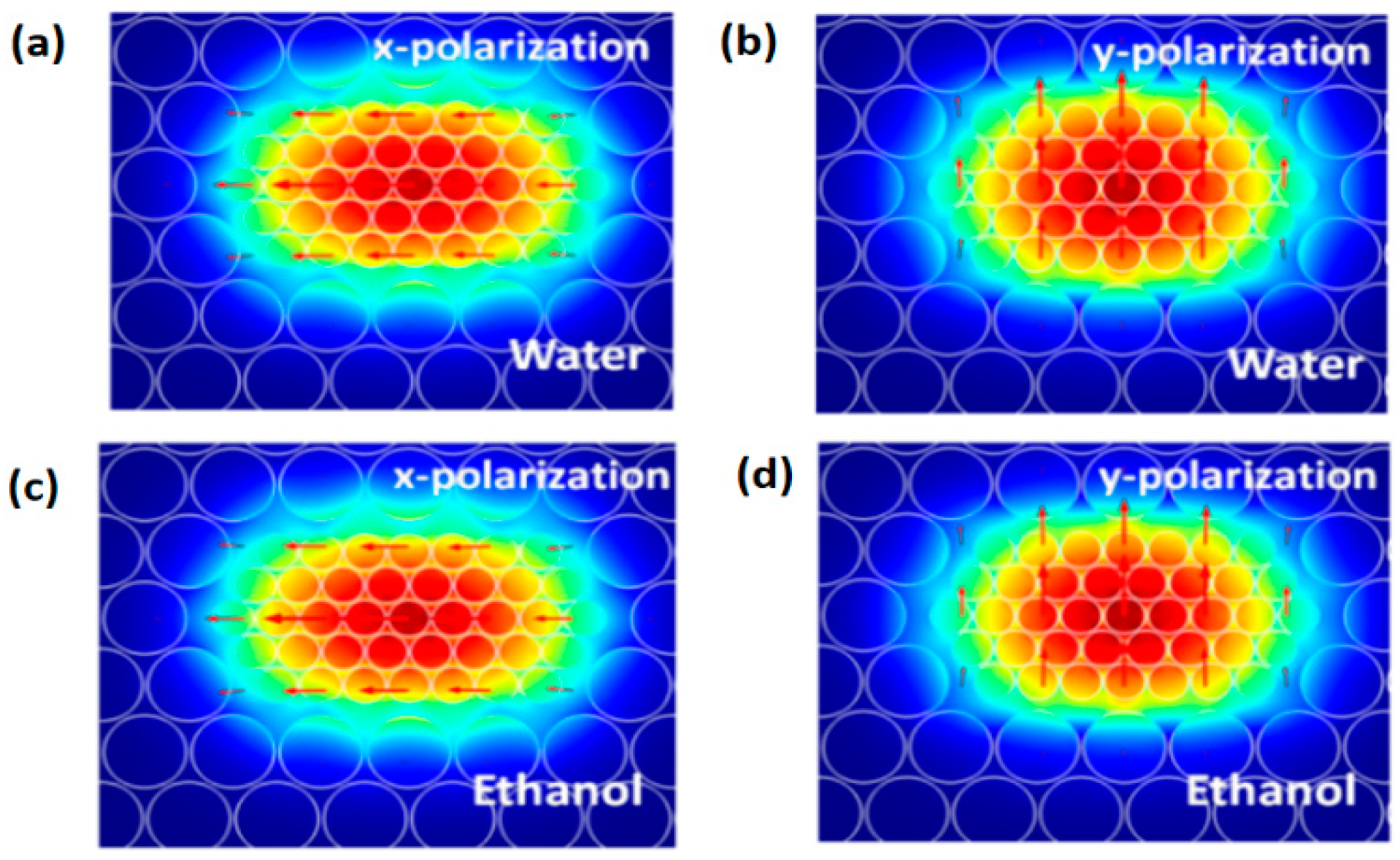
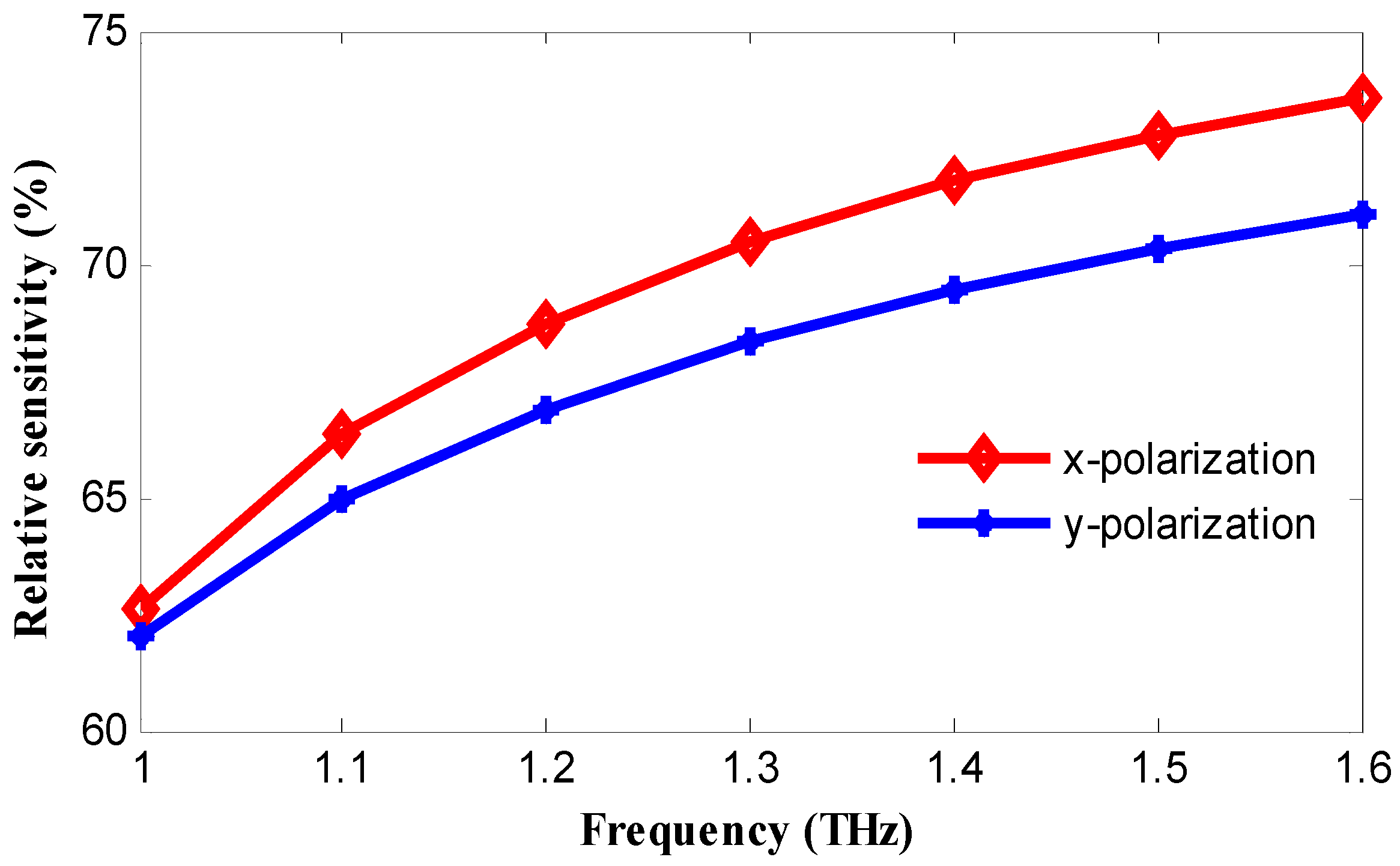
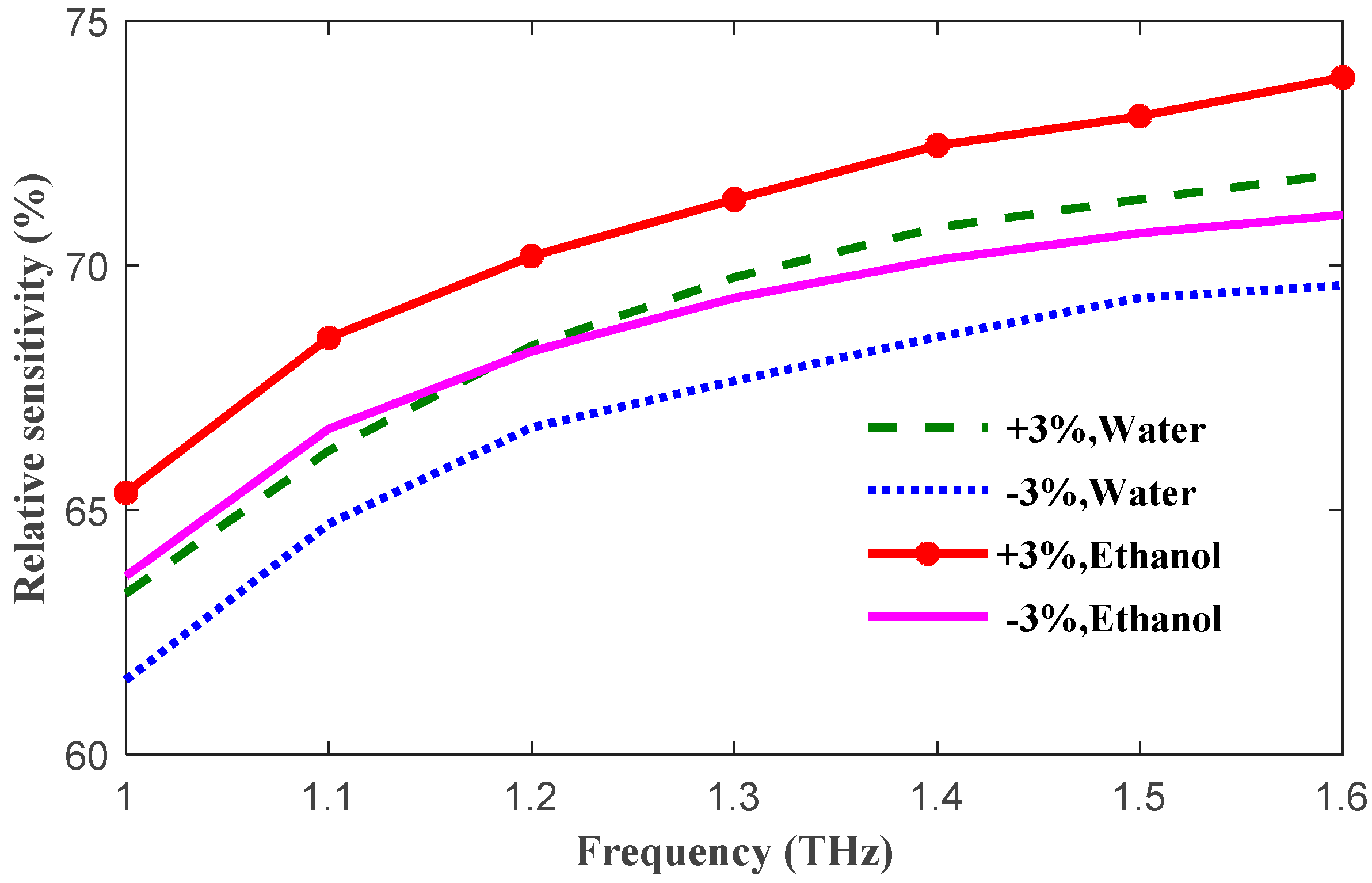
3.1. Relative Sensitivity
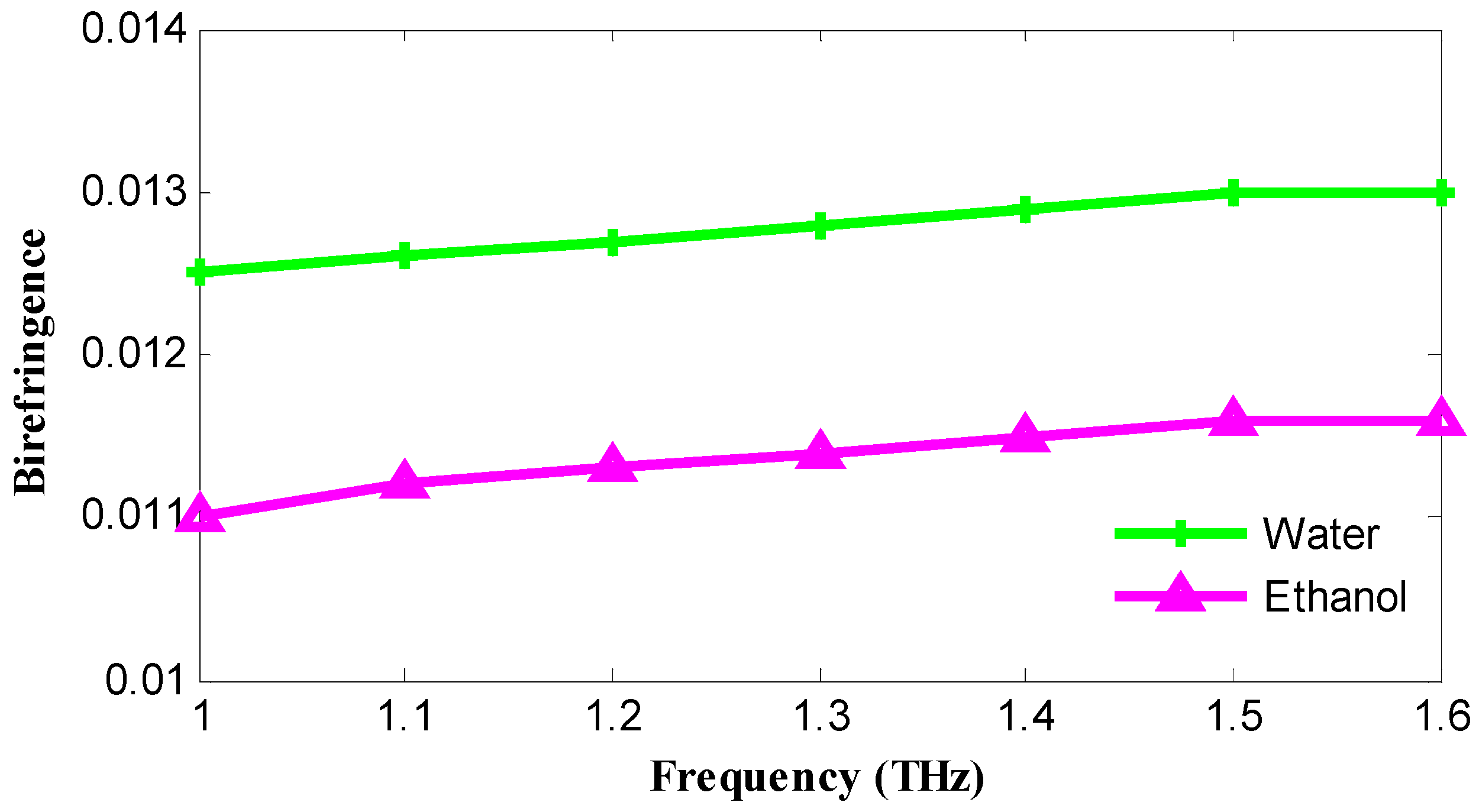
3.2. Modal Birefringence
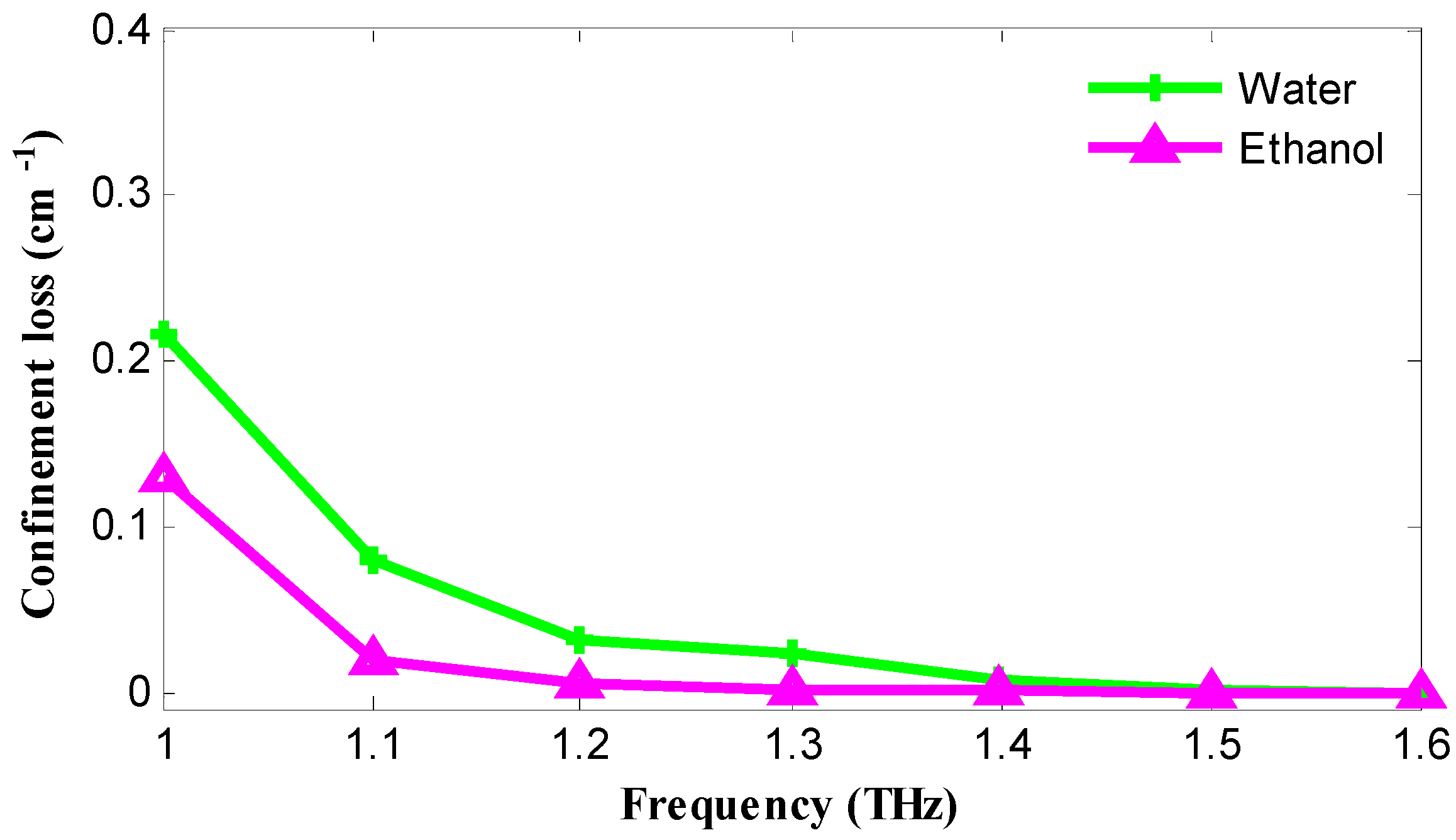
3.3. Confinement Loss
4. Fabrication Methods
5. Conclusion
Author Contributions
Funding
Conflicts of Interest
References
- Awad, M.M.; Cheville, R.A. Transmission tera-hertzwaveguide based imaging below the diffraction limit. Appl. Phys. Lett. 2005, 86, 221107. [Google Scholar] [CrossRef]
- Cook, D.J.; Decker, B.K.; Allen, M.G. Quantitative THz spectroscopy of explosive materials. In Proceedings of the 2005 Optical Terahertz Science and Technology, Orlando, FL, USA, 14–16 March 2005; p. MA6. [Google Scholar] [CrossRef]
- Fischer, B.M.; Helm, H.; Jepsen, P.U. Chemical recognition with broadband THz spectroscopy. Proc. IEEE. 2007, 95, 1592–1604. [Google Scholar] [CrossRef]
- Fischer, B.M.; Hoffmann, M.; Helm, H.; Modjesch, G.; Jepsen, P.U. Chemical recognition in terahertz time-domain spectroscopy and imaging. Semicond. Sci. Technol. 2005, 20, S246. Available online: stacks.iop.org/SST/20/S246 (accessed on 20 September 2018). [CrossRef]
- Zhang, J.Q.; Grischkowsky, D. Waveguide terahertz time-domain spectroscopy of manometer water layers. Opt. Lett. 2004, 29, 1617–1619. [Google Scholar] [CrossRef] [PubMed]
- Nagel, M.; Bolivar, P.H.; Brucherseifer, M.; Kurz, H.; Bosserhoff, A.; Bttner, R. Integrated THz technology for label-free genetic diagnostics. Appl. Phys. Lett. 2002, 80, 154–156. [Google Scholar] [CrossRef]
- Fischer, B.M. Terahertz time-domain spectroscopy and imaging of artificial RNA. Opt. Express 2005, 13, 5205–5215. [Google Scholar] [CrossRef] [PubMed]
- Cheon, H.; Yang, H.J.; Son, J.H. Toward clinical cancer imaging using terahertz spectroscopy. IEEE J. Sel. Top. Quant. Electron. 2017, 23, 1–9. [Google Scholar] [CrossRef]
- Zaytsev, K.I.; Kudrin, K.G.; Koroleva, S.A.; Fokina, I.N.; Volodarskaya, S.I.; Novitskaya, E.V.; Perov, A.N.; Karasik, V.E.; Yurchenko, S.O. Medical diagnostics using terahertz pulsed spectroscopy. J. Phys. Conf. Ser. 2014, 486, 012014. Available online: http://iopscience.iop.org/article/10.1088/1742-6596/486/1/012014/meta (accessed on 20 September 2018). [CrossRef]
- Kiwa, T.; Kondo, J.; Oka, S.; Kawayama, I.; Yamada, H.; Tonouchi, M.; Tsukada, K. Chemical sensing plate with a laser-terahertz monitoring system. Appl. Opt. 2008, 47, 3324–3327. [Google Scholar] [CrossRef] [PubMed]
- Islam, M.S.; Sultana, J.; Ahmed, K.; Islam, M.R.; Dinovitser, A.; Ng, B.W.H.; Abbott, D. A novel approach for spectroscopic chemical identification using photonic crystal fiber in the terahertz regime. IEEE Sens. J. 2018, 18, 575–582. [Google Scholar] [CrossRef]
- Islam, M.S.; Sultana, J.; Rifat, A.A.; Dinovitser, A.; Ng, B.W.H.; Abbott, D. Terahertz sensing in a hollow core photonic crystal fiber. IEEE Sens. J. 2018, 18, 4073–4080. [Google Scholar] [CrossRef]
- Sultana, J.; Islam, M.S.; Ahmed, K.; Dinovitser, A.; Ng, B.W.H.; Abbott, D. Terahertz detection of alcohol using photonic crystal fiber sensor. Appl. Opt. 2018, 57, 2426–2433. [Google Scholar] [CrossRef] [PubMed]
- Akowuah, E.K.; Ademgil, H.; Haxha, S.; Malek, F.A. An endlessly single-mode photonic crystal fiber with low chromatic dispersion, and bend and rotational insensitivity. J. Lightwave Technol. 2009, 27, 3940–3947. [Google Scholar] [CrossRef]
- Yue, Y.; Kai, G.; Wang, Z.; Sun, T.; Jin, L.; Lu, Y.; Zhang, C.; Liu, J.; Li, Y.; Liu, Y. Highly birefringent elliptic-hole photonic crystal fibrewith squeezed hexagonal lattice. Opt. Lett. 2007, 32, 469–471. [Google Scholar] [CrossRef] [PubMed]
- Saitoh, K.; Koshiba, M.; Hasegawa, T.; Sasaoka, E. Chromatic dispersion control in photonic crystal fibres: Application to ultra—Flattened dispersion. Opt. Express 2003, 11, 843–852. [Google Scholar] [CrossRef] [PubMed]
- Liu, S.; Wang, Y.; Hou, M.; Guo, J.; Li, Z.; Lu, P. Anti-resonant reflecting guidance in alcohol-filled hollow core photonic crystal fiber for sensing applications. Opt. Express 2013, 21, 31690–31697. [Google Scholar] [CrossRef] [PubMed]
- Hansen, K.P. Dispersion flattened hybrid-core nonlinear photonic crystal fiber. Opt. Express 2003, 11, 1503–1509. [Google Scholar] [CrossRef] [PubMed]
- Elbaz, D.; Malka, D.; Zalevsky, Z. Photonic crystal fiber based 1 × N intensity and wavelength splitters/couplers. Electromagnetics 2012, 32, 209–220. [Google Scholar] [CrossRef]
- Malka, D.; Zalevsky, Z. Multicore photonic crystal fiber based 1 × 8 two-dimensional intensity splitters/couplers. Electromagnetics 2013, 33, 413–420. [Google Scholar] [CrossRef]
- Sintov, Y.; Malka, D.; Zalevsky, Z. Prospects for diode-pumped alkali-atom-based hollow-core photonic-crystal fiber lasers. Opt. lett. 2014, 39, 4655–4658. [Google Scholar] [CrossRef] [PubMed]
- Malka, D.; Sintov, Y.; Zalevsky, Z. Fiber-laser monolithic coherent beam combiner based on multicore photonic crystal fiber. Opt. Eng. 2014, 54. [Google Scholar] [CrossRef]
- Malka, D.; Cohen, E.; Zalevsky, Z. Design of 4 × 1 power beam combiner based on multiCore photonic crystal fiber. Appl. Sci. 2017, 7, 695. [Google Scholar] [CrossRef]
- Rana, S.; Rakin, A.S.; Subbaraman, H.; Leonhardt, R.; Abbott, D. Low loss and low dispersion fiber for transmission applications in the terahertz regime. IEEE Photonics Technol. Lett. 2017, 29, 830–833. [Google Scholar] [CrossRef]
- Islam, M.S.; Faisal, M.; Razzak, S.M.A. Extremely low loss porous-core photonic crystal fiber with ultra-flat dispersion in terahertz regime. J. Opt. Soc. Am. B 2017, 34, 1747–1754. [Google Scholar] [CrossRef]
- Rana, S.; Rakin, A.S.; Hasan, M.R.; Reza, M.S.; Leonhartd, R.; Abbott, D.; Subbaraman, H. Low loss and flat dispersion Kagome photonic crystal fiber in the terahertz regime. Opt. Commun. 2017, 410, 452–456. [Google Scholar] [CrossRef]
- Nielsen, K.; Rasmussen, H.K.; Adam, A.J.L.; Planken, P.C.M.; Bang, O.; Jepsen, P.U. Bendable, low-loss TOPAS fibers for the terahertz frequency range. Opt. Express 2009, 17, 8592–8601. [Google Scholar] [CrossRef] [PubMed]
- Yuan, W.; Khan, L.; Webb, D.J.; Kalli, K.; Rasmussen, H.K.; Stefani, A.; Bang, O. Humidity insensitive TOPAS polymer fiber Bragg grating sensor. Opt. Express 2011, 19, 19731–19739. [Google Scholar] [CrossRef] [PubMed]
- Rana, S.; Islam, M.S.; Faisal, M.; Roy, K.C.; Islam, R.; Kaijage, S.F. Single-mode porous fiber for low-loss polarization maintaining terahertz transmission. Opt. Eng. 2016, 55, 076114. [Google Scholar] [CrossRef]
- Ademgil, H.; Haxha, S. Highly birefringent nonlinear PCF for optical sensing of analytes in aqueous solutions. Optik Int. J. Light Electron Opt. 2014, 125, 6274–6278. [Google Scholar] [CrossRef]
- Atakaramians, S.; Afshar, S.; Ebendorff-Heidepriem, H.; Nagel, M.; Fischer, B.M.; Abbott, D.; Monro, T.M. THz porous fibers: Design, fabrication and experimental characterization. Opt. Express 2009, 17, 14053–14062. [Google Scholar] [CrossRef] [PubMed]
- Issa, N.A.; van Eijkelenborg, M.A.; Fellew, M.; Cox, F.; Henry, G.; Large, M.C. Fabrication and study of microstructured optical fibers with elliptical holes. Opt. Lett. 2004, 29, 1336–1338. [Google Scholar] [CrossRef] [PubMed]
- Pysz, D.; Kujawa, I.; Stępień, R.; Klimczak, M.; Filipkowski, A.; Franczyk, M.; Kociszewski, L.; Buźniak, J.; Haraśny, K.; Buczyński, R. Stack and draw fabrication of soft glass microstructured fiber optics. Bull. Pol. Ac. Tech. 2014, 62, 667–682. [Google Scholar] [CrossRef]

| Ref. | Core Air Hole Shape | Cladding Air Hole Shape | Relative Sensitivity | Birefringence | Fabrication Feasibility |
|---|---|---|---|---|---|
| 11 | Rectangular slot | Kagome structure | 85.7% | 0.005 | difficult |
| 12 | Rectangular slot | Rectangular slot | 96.8% | 0.0154 | difficult |
| 13 | Elliptical | Circular | 68.8% | 0.0176 | difficult |
| Proposed (this work) | Circular | Circular | 73.5% | 0.013 | compatible with the state-of-the-art process |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rana, S.; Kandadai, N.; Subbaraman, H. A Highly Sensitive, Polarization Maintaining Photonic Crystal Fiber Sensor Operating in the THz Regime. Photonics 2018, 5, 40. https://doi.org/10.3390/photonics5040040
Rana S, Kandadai N, Subbaraman H. A Highly Sensitive, Polarization Maintaining Photonic Crystal Fiber Sensor Operating in the THz Regime. Photonics. 2018; 5(4):40. https://doi.org/10.3390/photonics5040040
Chicago/Turabian StyleRana, Sohel, Nirmala Kandadai, and Harish Subbaraman. 2018. "A Highly Sensitive, Polarization Maintaining Photonic Crystal Fiber Sensor Operating in the THz Regime" Photonics 5, no. 4: 40. https://doi.org/10.3390/photonics5040040
APA StyleRana, S., Kandadai, N., & Subbaraman, H. (2018). A Highly Sensitive, Polarization Maintaining Photonic Crystal Fiber Sensor Operating in the THz Regime. Photonics, 5(4), 40. https://doi.org/10.3390/photonics5040040







