Smartphone-Readable Optical-Fiber Quasi-Distributed Phosphorescent Temperature Sensor
Abstract
1. Introduction
2. Principle of Operation
2.1. Temperature-Sensitive Phosphorescent Materials
2.1.1. Strontium Aluminates
2.1.2. Temperature-Dependent Intensity Change
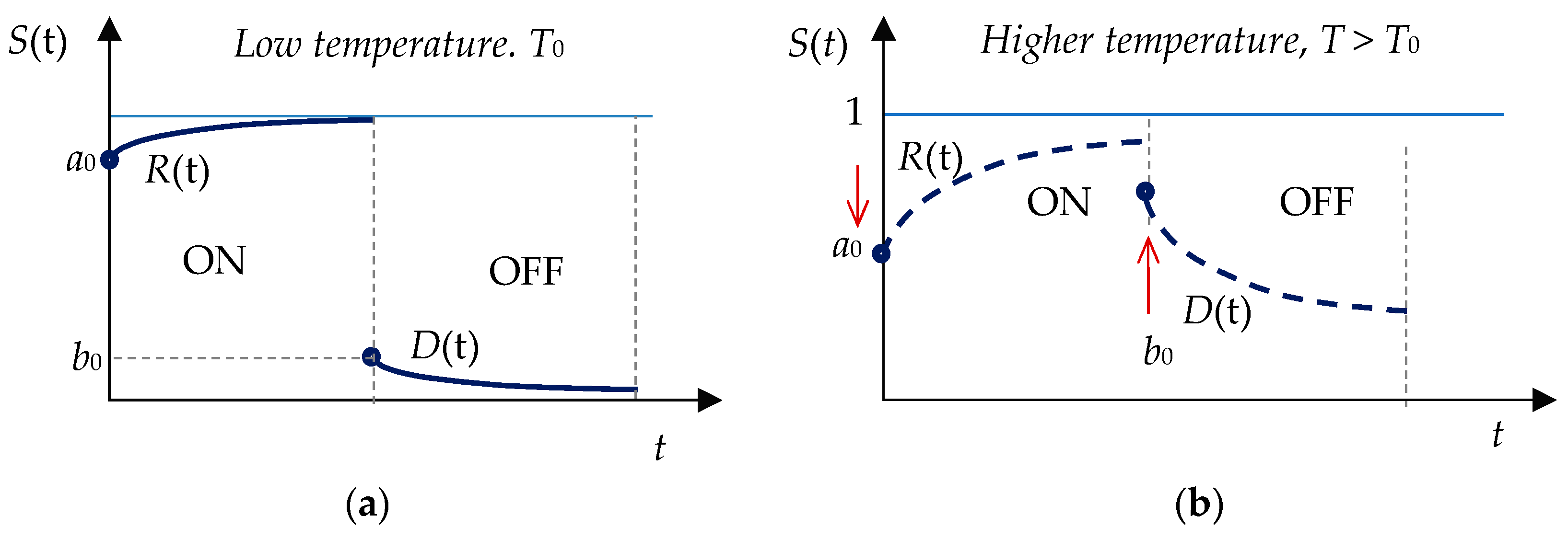
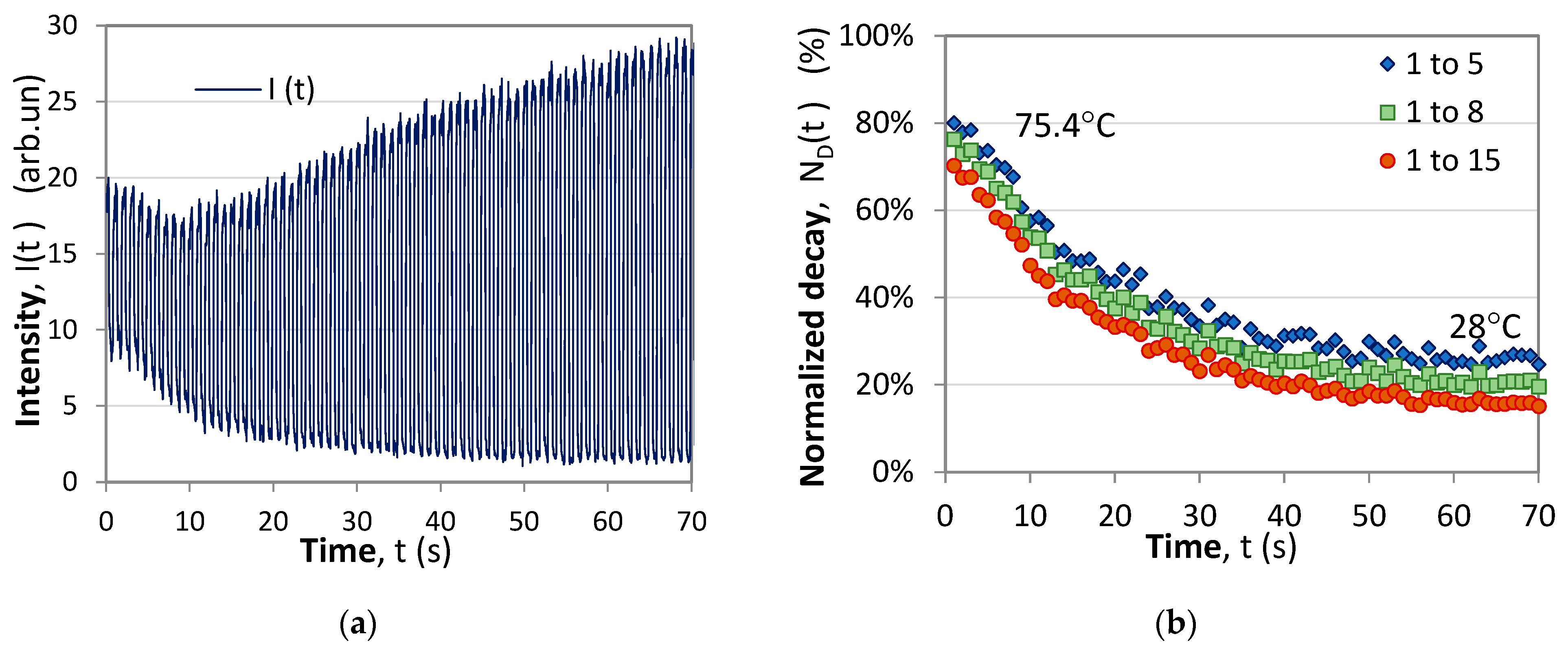
2.1.3. Rise and Decay Time Responses
2.2. Smartphone-Based Interrogation of Space-Multiplexed Temperature Sensors
2.2.1. The Smartphone as a Detection Unit
- It is sensitive in the 400 nm to 700 nm visible range where most of the phosphors emit;
- It can measure time-dependent light emissions at rates of 30 fps (33 ms) or 60 fps (16.7 ms) which allow typical phosphorescent time responses to be tracked;
- As the camera is based on 2D CCD arrays it can simultaneously measure a considerable number of independent spatially distributed luminescent point sensors;
- Touchless remote interrogation of spatially distributed sensors;
- Data processing capabilities;
- Connectivity to wireless sensing networks using Wi-Fi and Bluetooth technology.
2.2.2. Possible Interrogation Schemes
2.3. Optical-Fiber Excitation Platform
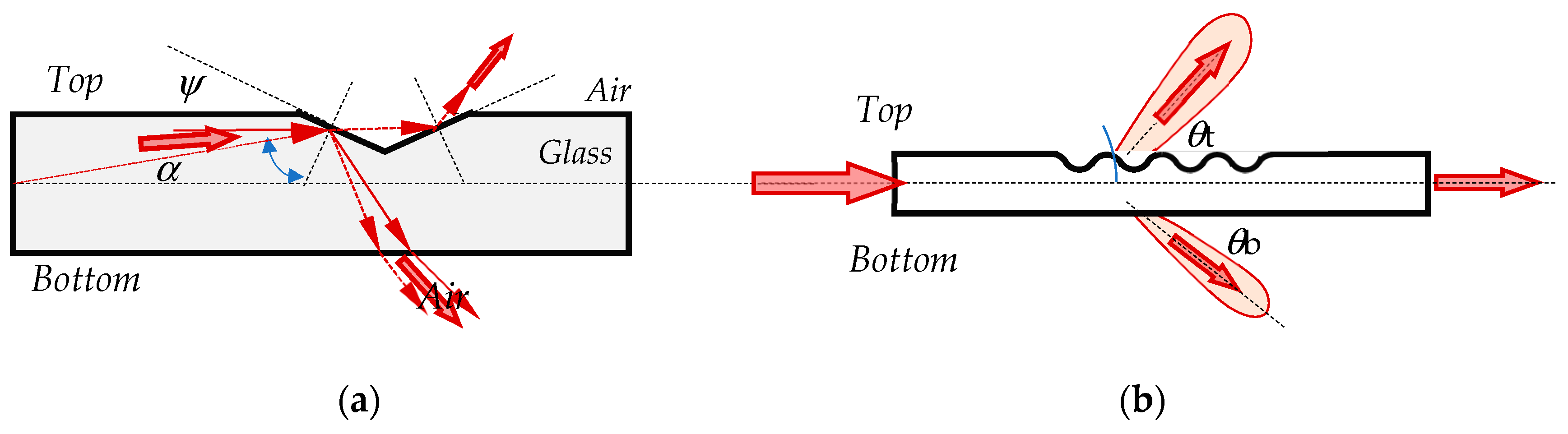
2.3.1. CO2 Laser-Written Corrugated Long-Period Gratings (C-LPG)
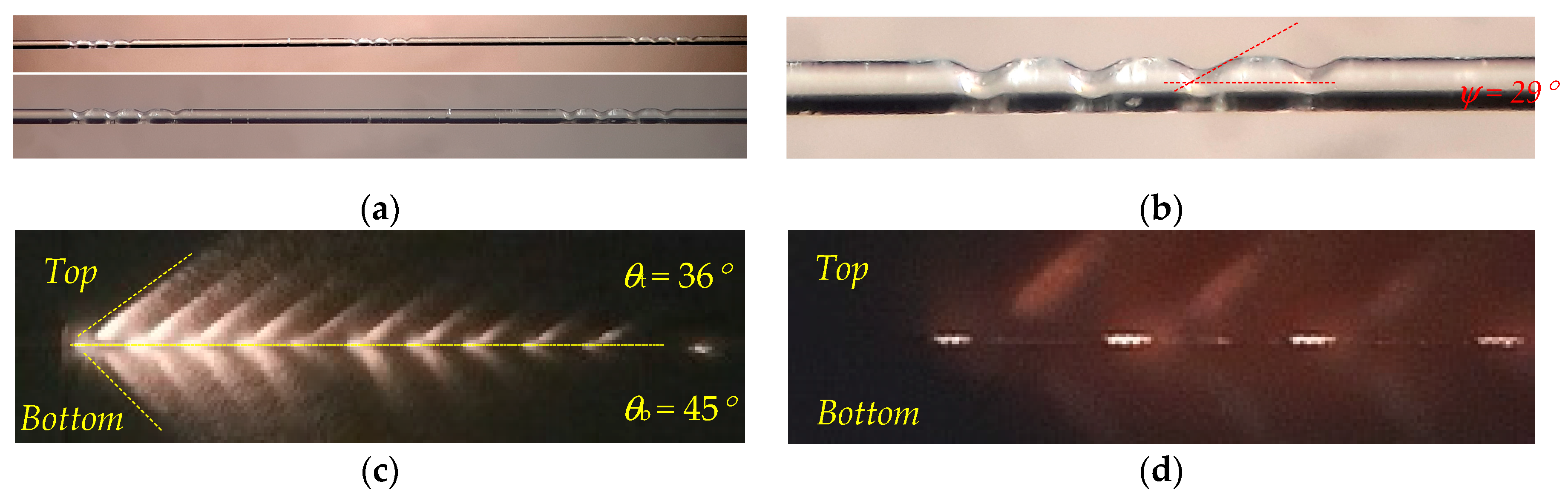
2.3.2. Radiation Diagram of a Corrugated LPG
3. Experiments and Results
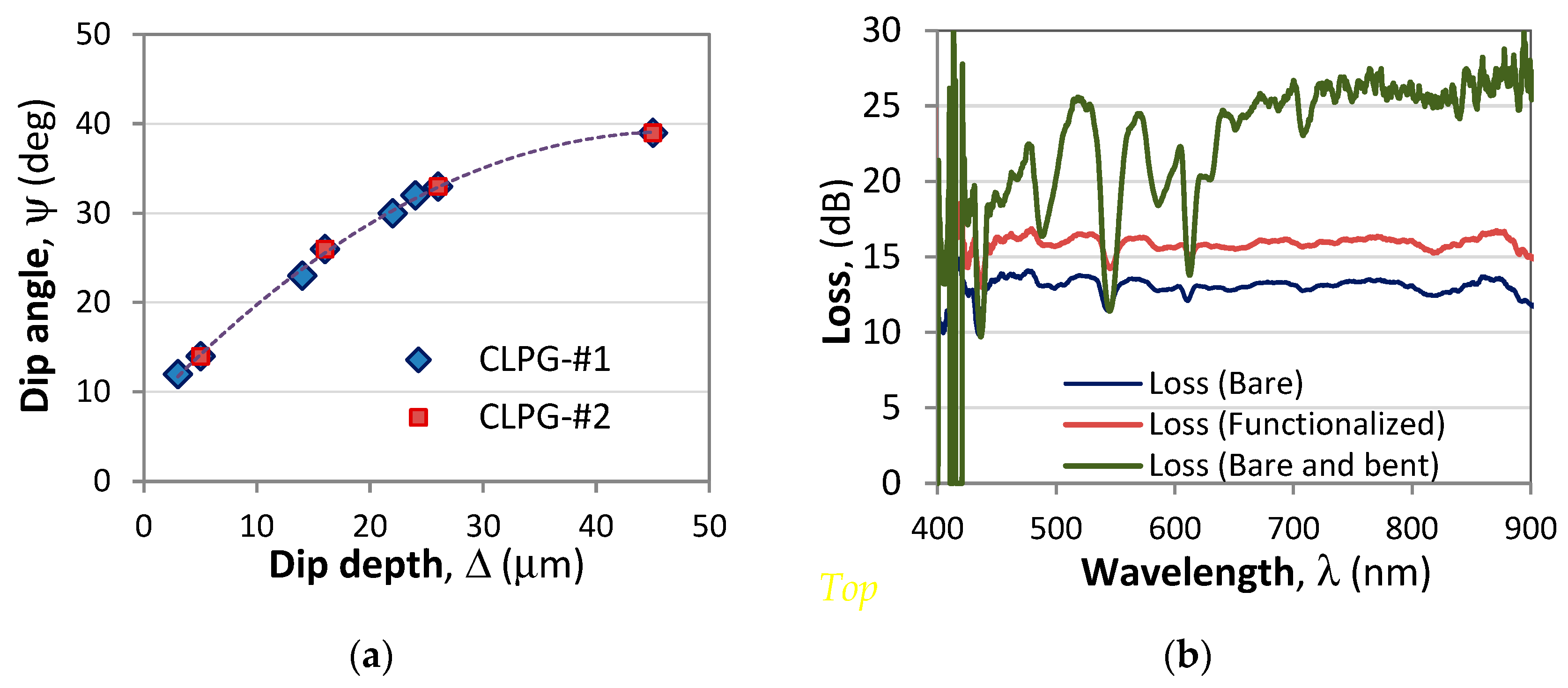
3.1. Inscription, Characterization and Functionalization of the Corrugated LPGs
- (i)
- Inscription of the array of CLPGs;
- (ii)
- Measurement of the basic parameters of the corrugated structures;
- (iii)
- Deposition of the phosphorescent powder around the C-LPG.
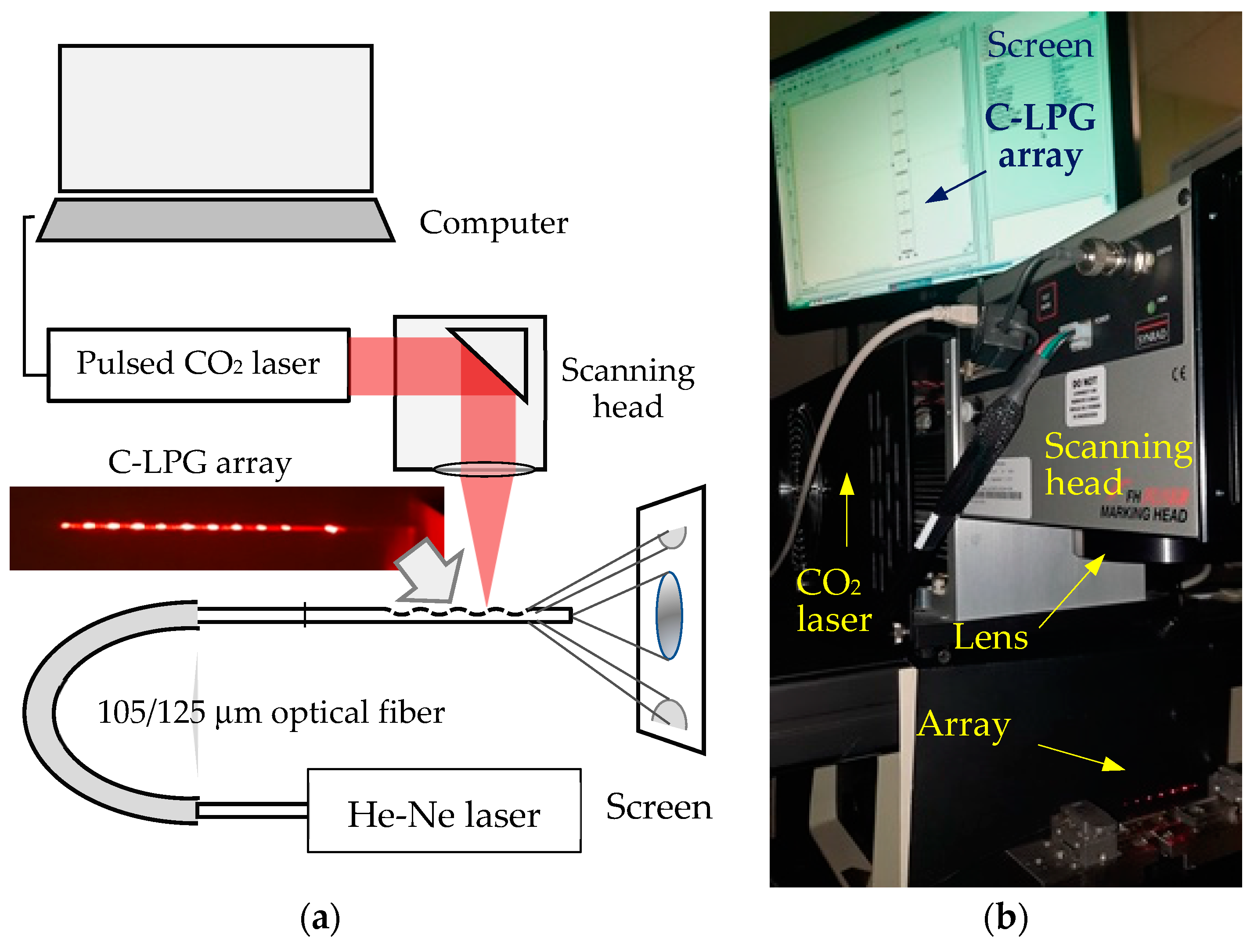
3.1.1. Experimental Setup for C-LPG Inscription
3.1.2. Characteristics of the Fabricated Corrugated Structures
- To individually fine tune the dip depth and hence the radiation pattern and irradiated power;
- To excite the fiber from both ends;
- To deposit a reflector at the fiber output tip.
3.2. Performance of the Quasi-Distributed Sensor
3.2.1. Measurement Set-Up
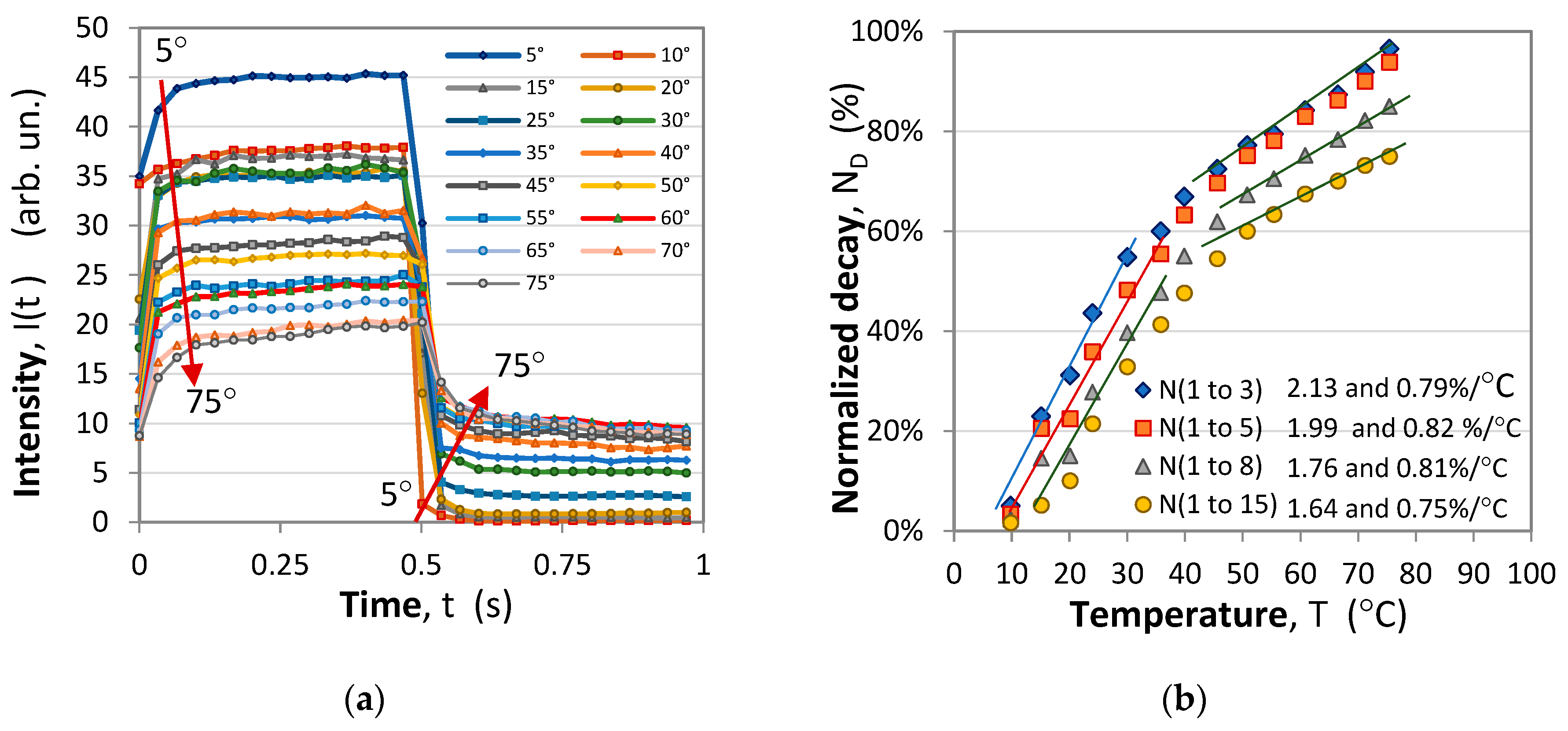
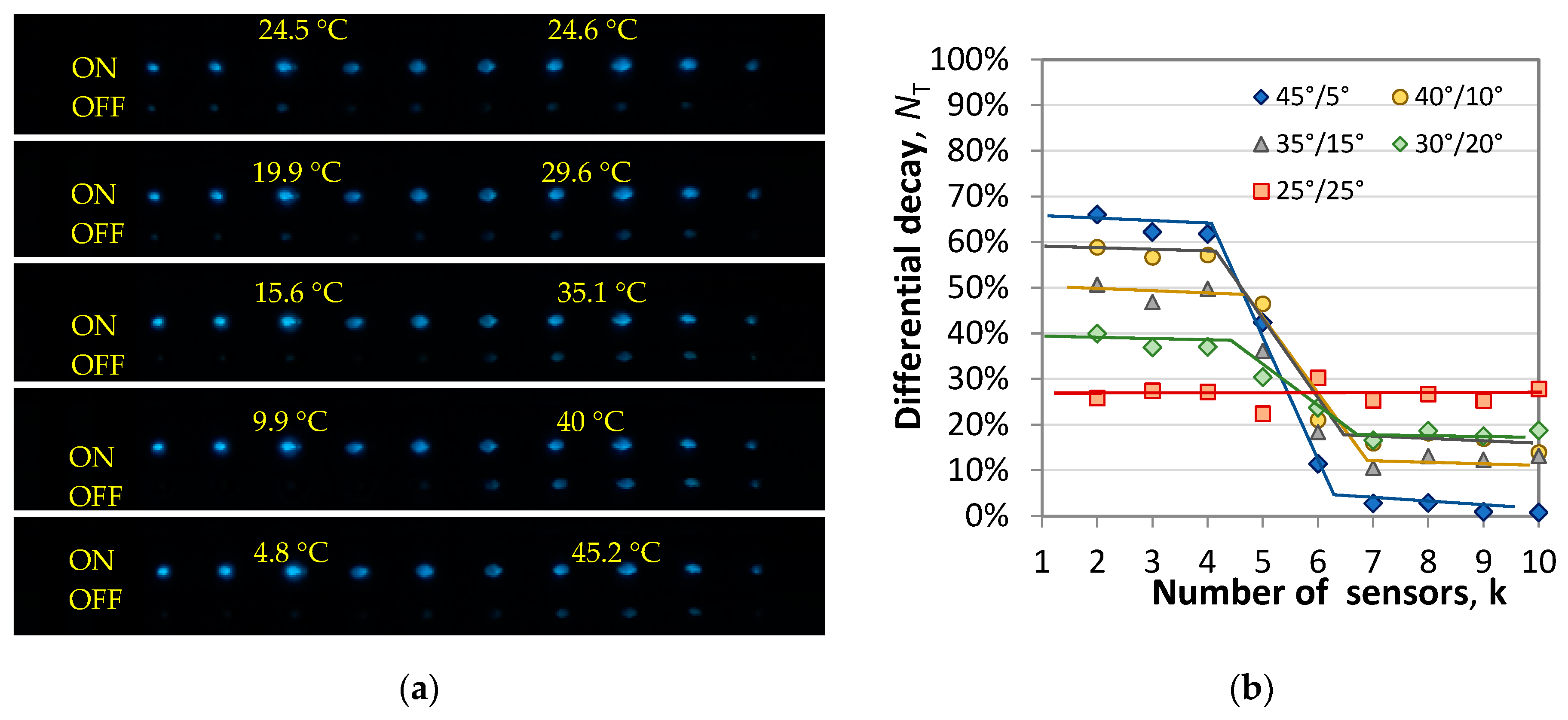
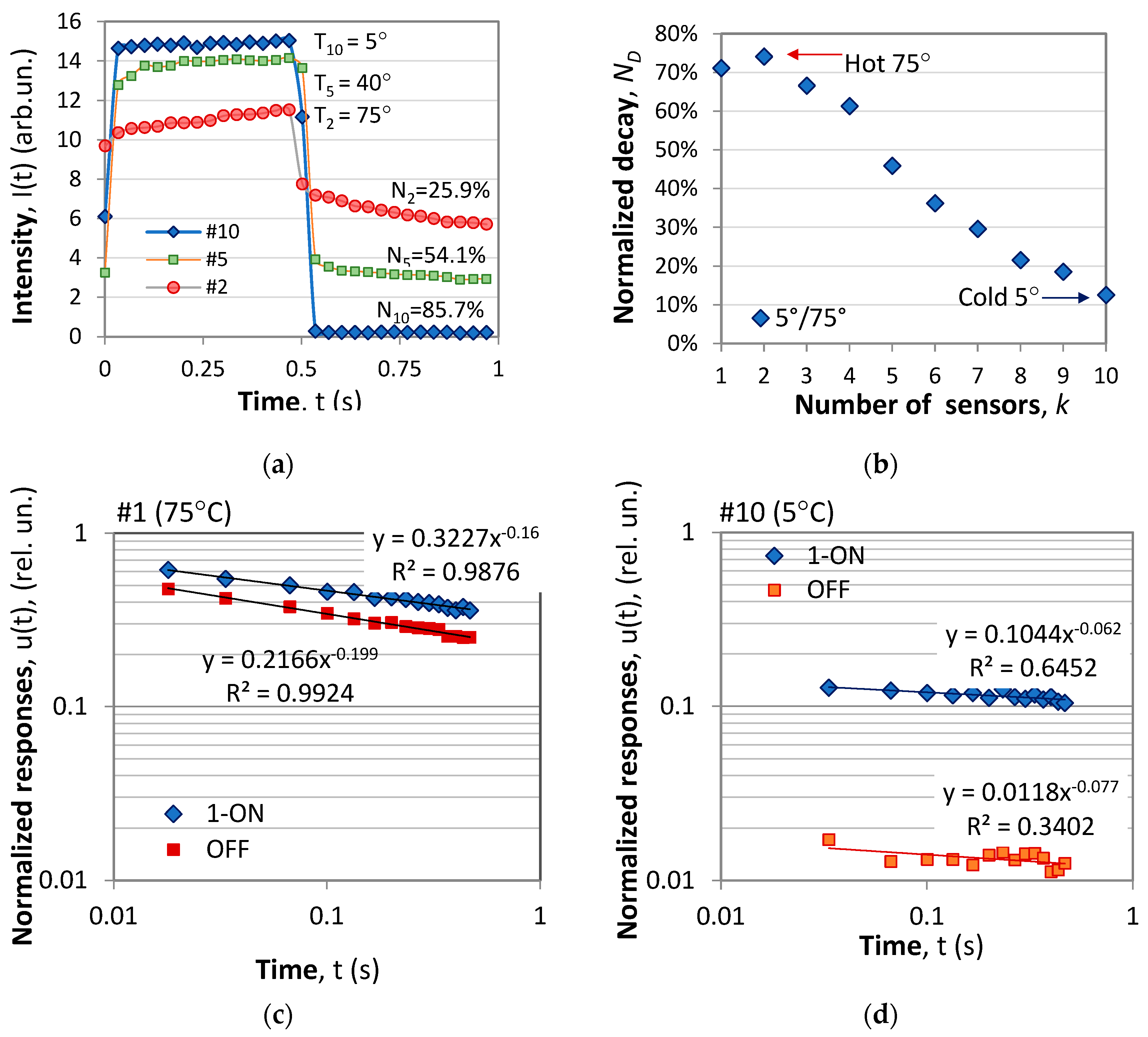
3.2.2. Results
4. Discussion
- (i)
- (ii)
- The duration over which rise and decay summation (integration) is carried out;
- (iii)
- The duty factor η.
- (iv)
- Fifth, the response time of the sensor essentially is affected by the following:
- (v)
- The rise/decay times of the phosphors; so to reduce it to the order of seconds, if needed, phosphors with similar decay times must be used;
- (vi)
- The modulation frequency (limited by the rise/decay times of the responses), whose increase will reduce the response time;
- (vii)
- The number of cycles over which the signal is averaged to reduce fluctuations, at the expense of response time;
- (viii)
- The frame rate of the smartphone whose increase (to 60 fps, for example) will reduce the response time.
- (i)
- It offers contactless simultaneous interrogation using smartphones or web cameras linked via Wi-Fi and Bluetooth to the internet as a part of a broader sensing network. None of the reported temperature sensors has this feature.
- (ii)
- It makes use of standard components of the lowest cost such as fibers and phosphorescent materials. It can also use polymer fiber. Interferometric sensors [21,22], be they Mach-Zender (MZI), Michelson (MI) or Fabry Perror (FP), require single-mode or specialty photonic crystal (PCF), holey (HOF) or microstructured (MOF) optical fibers.
- (iii)
- A single low-cost LED can excite and a single camera can interrogate several sensing arrays. Similar is the case of a quasi-distributed FBG sensing network using wavelength multiplexing whose temperature range is up to 100 °C. In contrast, all of the non-phosphorescent types of sensors are based on considerably more costly sources and detection equipment. The fluorescence-based sensors [21] require pulsed lasers and fast electronics or spectrometers to measure the intensity ratio of different fluorescence. Practically all of the interferometric and fiber Bragg grating (FBG) sensors function in the infrared communication band (1260 nm to 1630 nm) and employ orders of magnitude more costly sources and interrogation equipment and thus make practical sense mostly for multiplexed sensing networks.
- (iv)
- It is easy to fabricate by a high-productivity pulsed CO2 laser technique. In contrast, FBGs are made using an order of magnitude more costly excimer or femtosecond lasers [23]. The variety of interferometric sensors need more sophisticated splicers and for the micro-cavity-based MZI and FP interferometric sensors—a femtosecond laser [23].
- (v)
- The excitation fiber allows wiring of the sensing array along arbitrary paths to measure spatial distribution of temperature and thermal flows. This feature is similar to distributed temperature sensors (Raman, Brillouin [23] or Rayleigh type [22]) and quasi-distributed FBG sensors, which however need a special attachment in order to eliminate strain unless they measure both strain and temperature.
- (vi)
- Depending on the phosphor used, temperatures as high as several hundred degrees can be reached, which is what most of the silica-based fiber sensors offer. The sensor proposed here, however, offers no possibility for high temperature (above several hundred degrees) measurement.
- (vii)
- It can also be made from a polymer fiber [21], in which case the fabrication of the dips along the fiber would be even less costly. However, losses in the UV will be considerably higher so shorter fiber spans should be used.
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Aizawa, H.; Toba, E.; Katsumata, T.; Takahashi, J.; Matsunaga, K.; Komuro, S.; Morikawa, T. Long duration phosphor for a fiber-optic thermometer. In Proceedings of the 41st SICE Annual Conference, Osaka, Japan, 5–7 August 2002; pp. 2972–2975. [Google Scholar]
- McSherry, M.; Fitzpatrick, C.; Lewis, E. Review of luminescent based fibre optic temperature sensors. Sens. Rev. 2005, 25, 56–62. [Google Scholar] [CrossRef]
- Bao, Y.; Huang, Y.; Hoehler, M.; Chen, G. Review of fiber optic sensors for structural fire engineering. Sensors 2019, 19, 877. [Google Scholar] [CrossRef] [PubMed]
- Wang, L.; Shang, Z.; Shi, M.; Cao, P.; Yang, B.; Zou, J. Preparing and testing the reliability of long afterglow SrAl2O4:Eu2+, Dy3+ phosphor flexible films for temperature sensing. RSC Adv. 2020, 10, 11418. [Google Scholar] [CrossRef]
- Li, J.; Chen, H.; Wang, X.; Li, P.; Lv, M.; Hao, H.; Meng, L. An optical fiber temperature sensor based on fluorescence intensity ratio in Er3+/Yb3+ co-doped Gd2O3 phosphors. Ceram. Int. 2023, 49, 28913–28919. [Google Scholar] [CrossRef]
- Zhao, Y.; Wang, X.; Zhang, Y.; Li, Y.; Yao, X. Optical temperature sensing of up-conversion luminescent materials: Fundamentals and progress. J. Alloys Compd. 2020, 817, 152691. [Google Scholar] [CrossRef]
- Carrillo-Betancourt, R.A.; López-Camero, A.D.; Hernández-Cordero, J. Luminescent Polymer Composites for Optical Fiber Sensors. Polymers 2023, 15, 505. [Google Scholar] [CrossRef] [PubMed]
- Miluski, P.; Dorosz, D.; Mojda, J.; Kochanowicz, M.; Dorosz, J. Luminescent Polymer Optical Fibre Sensor for Temperature Measurement. Acta Phys. Pol. A 2015, 127, 730–733. [Google Scholar] [CrossRef]
- Li, H.; Zhang, Y.; Shao, L.; Htwe, Z.; Yuan, P. Luminescence probe for temperature sensor based on fluorescence intensity ratio. Opt. Mater. Express 2017, 7, 1077. [Google Scholar] [CrossRef]
- Harun, S.W.; Yasin, M.; Hamzah, A.; Arof, H.; Ahmad, H. Temperature sensor based on lifetime measurement of Erbium fluorescence. J. Optoelectr. Adv. Mater. 2011, 13, 69–71. [Google Scholar]
- Fouzar, S.; Eftimov, T.; Kostova, I.; Dimitrova, T.L.; Benmounah, A.; Lakhssassi, A. A Simple Fiber Optic Temperature Sensor for Fire Detection In Hazardous Environment Based On Differential Time Rise/Decay Phosphorescence Response. IEEE Trans. Instr. Meas. 2022, 71, 9508908. [Google Scholar] [CrossRef]
- Fouzar, S.; Kostova, I.; Eftimov, T.A.; Nikolov, K. Phosphorescent Fibre Optic Tip Temperature Sensor. In Proceedings of the 4th International Conference on Phosphor Thermometry, Becici, Montenegro, 26–30 August 2024. Accepted. [Google Scholar]
- Eftimov, T.; Kostova, I.; Arapova, A.; Patronov, G. Rise and decay time responses of Sr aluminate phosphorescent materials. J. Lumin. 2021, 235, 117985. [Google Scholar] [CrossRef]
- Fouzar, S.; Kostova, I.; Eftimov, T.; Benmounah, A.; Ouchabane, M.; Lakhssassi, A. Excitation and spectral dependence of the rise and decay time responses of Eu2+ and Dy3+ doped strontium aluminates. J. Mater. Sci. Mater. Electron. 2022, 33, 20962–20980. [Google Scholar] [CrossRef]
- Fouzar, S.; Eftimov, T.; Kostova, I.; Benmounah, A.; Lakhssassi, A. Effects of temperature on the time responses of strontium aluminates. Opt. Mater. 2021, 122, 111619. [Google Scholar] [CrossRef]
- Eftimov, T.A.; Kostova, I.; Peltekov, A.; Hristova, K.; Brabant, D.; Fouzar, S.; Nikolov, K. A Study of Strontium Aluminates for All Optical Contactless Sensing Applications Using Smartphone Interrogation. Opt. Laser Technol. 2024, 176, 110965. [Google Scholar] [CrossRef]
- Fratto, B.E.; Culver, E.L.; Davis, G.; Deans, R.; Goods, J.B.; Hwang, S.; Keller, N.K.; Lawrence, J.A.; Petty, A.R.; Swager, T.M.; et al. Leveraging a smartphone to perform time-gated luminescence measurements. PLoS ONE 2023, 18, e0293740. [Google Scholar] [CrossRef] [PubMed]
- Chiniforooshan, Y.; Ma, J.; Bock, W.J.; Hao, W.; Wang, Z.Y. Corrugated Fiber Grating for Detection of Lead Ions in Water. J. Light. Technol. 2015, 33, 2549–2553. [Google Scholar] [CrossRef]
- Irawan, R.; Chuan, T.S.; Meng, T.C.; Ming, T.K. Rapid Constructions of Microstructures for Optical Fiber Sensors Using a Commercial CO2 Laser System. Open Biomed. Eng. J. 2008, 2, 28–35. [Google Scholar] [CrossRef] [PubMed]
- Tan, C.; Zhao, L.; Chen, M.; Cheng, J.; Yin, Z.; Liu, Q.; Yang, H.; Liao, W. Physical mechanism of pulsed laser interaction with fused silica optics during CO2 laser mitigation process. J. Non-Cryst. Solids 2021, 558, 120662. [Google Scholar] [CrossRef]
- Szczerska, M. Temperature Sensors Based on Polymer Fiber Optic Interferometer. Chemosensors 2022, 10, 228. [Google Scholar] [CrossRef]
- Yang, T.; Wang, X. Decoupling and Simultaneous Measurement of Nonuniform Strain and Temperature Using a Single Distributed Optical Fiber Ring. Exp. Mech. 2022, 62, 1531–1552. [Google Scholar] [CrossRef]
- Ma, S.; Xu, Y.; Pang, Y.; Zhao, X.; Li, Y.; Qin, Z.; Liu, Z.; Lu, P.; Bao, X. Optical Fiber Sensors for High-Temperature Monitoring: A Review. Sensors 2022, 22, 5722. [Google Scholar] [CrossRef] [PubMed]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Eftimov, T.; Kostova, I.; Fouzar, S.; Brabant, D.; Nikolov, K.; Vladev, V. Smartphone-Readable Optical-Fiber Quasi-Distributed Phosphorescent Temperature Sensor. Photonics 2024, 11, 694. https://doi.org/10.3390/photonics11080694
Eftimov T, Kostova I, Fouzar S, Brabant D, Nikolov K, Vladev V. Smartphone-Readable Optical-Fiber Quasi-Distributed Phosphorescent Temperature Sensor. Photonics. 2024; 11(8):694. https://doi.org/10.3390/photonics11080694
Chicago/Turabian StyleEftimov, Tinko, Irena Kostova, Samia Fouzar, Daniel Brabant, Kristian Nikolov, and Veselin Vladev. 2024. "Smartphone-Readable Optical-Fiber Quasi-Distributed Phosphorescent Temperature Sensor" Photonics 11, no. 8: 694. https://doi.org/10.3390/photonics11080694
APA StyleEftimov, T., Kostova, I., Fouzar, S., Brabant, D., Nikolov, K., & Vladev, V. (2024). Smartphone-Readable Optical-Fiber Quasi-Distributed Phosphorescent Temperature Sensor. Photonics, 11(8), 694. https://doi.org/10.3390/photonics11080694






