Role of Pyramidal Low-Dimensional Semiconductors in Advancing the Field of Optoelectronics
Abstract
1. Introduction
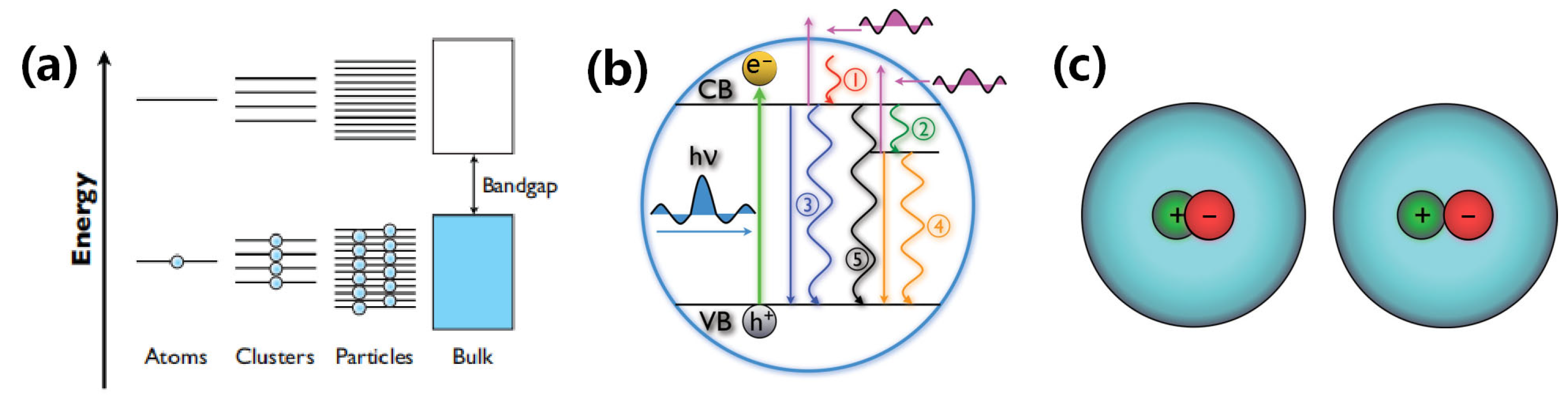
1.1. Structures and Properties of Low-Dimensional Nanomaterials
1.2. Influences of Low-Dimensional Semiconductor Morphologies on Optoelectronics
2. Structure, Growth, and Preparation of Pyramidal Low-Dimensional Semiconductors
2.1. Pyramidal Semiconductor Quantum Dots with Different Components
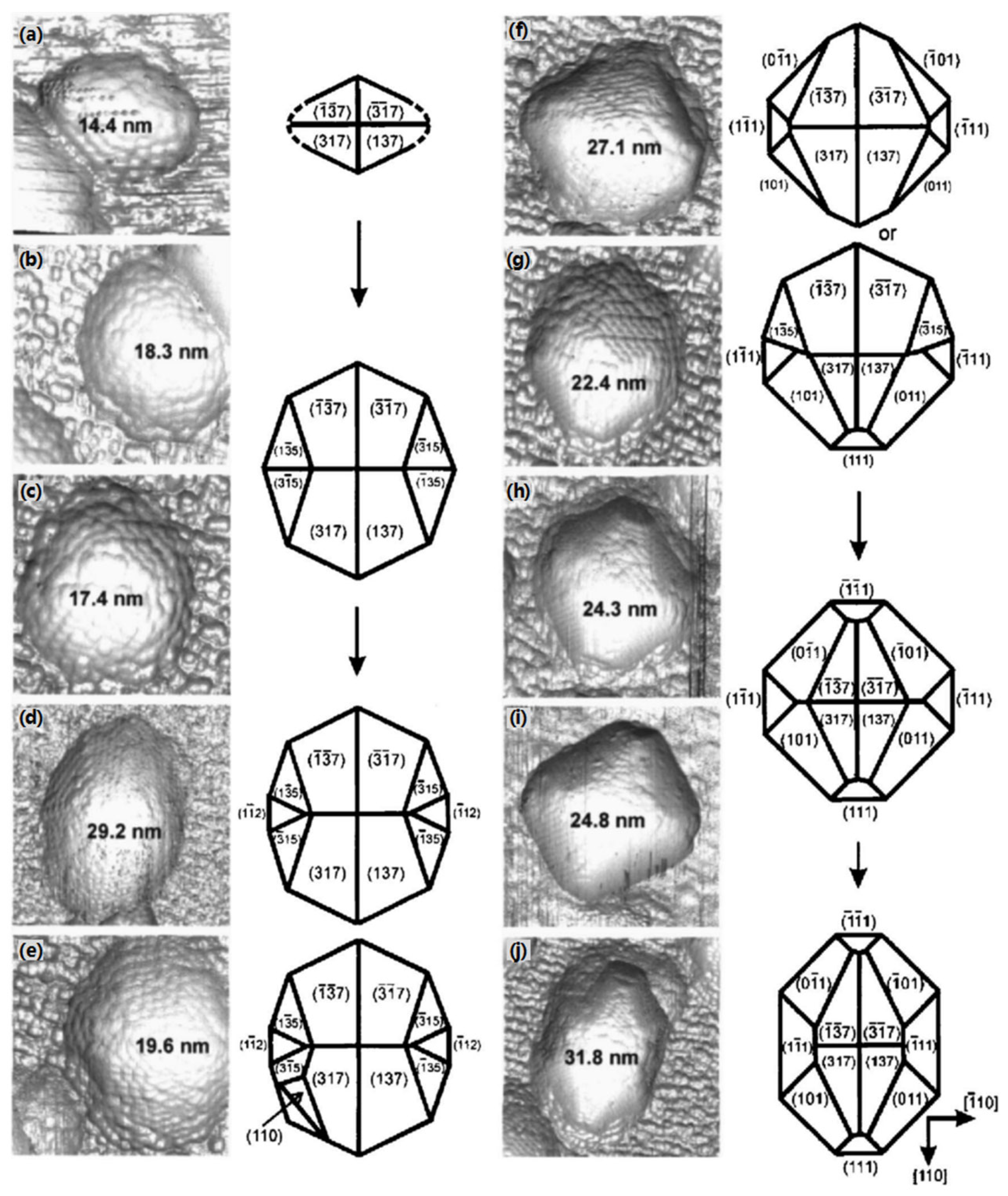
2.1.1. Structure and Growth of Pyramidal SiGe/Si Quantum Dots
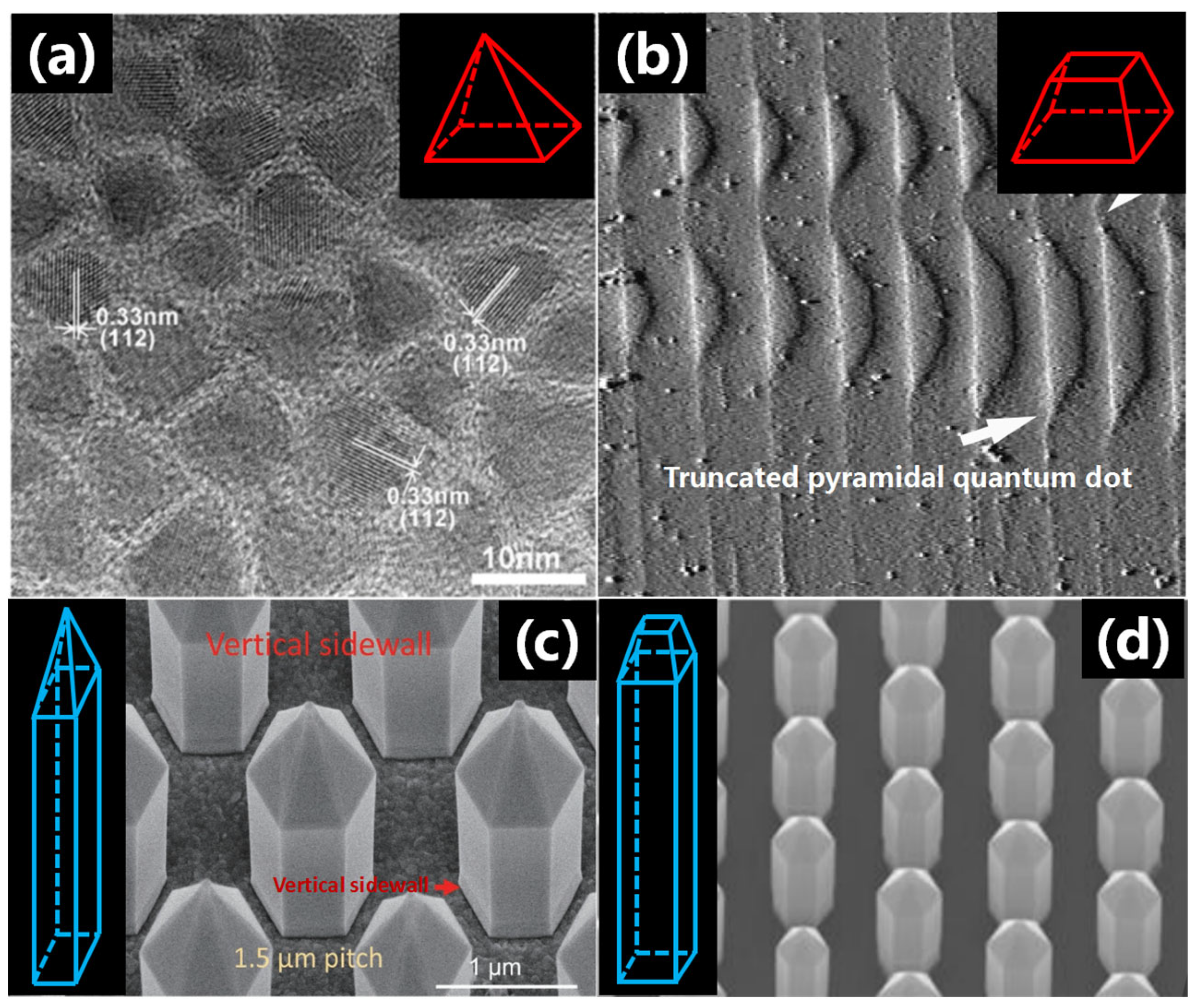
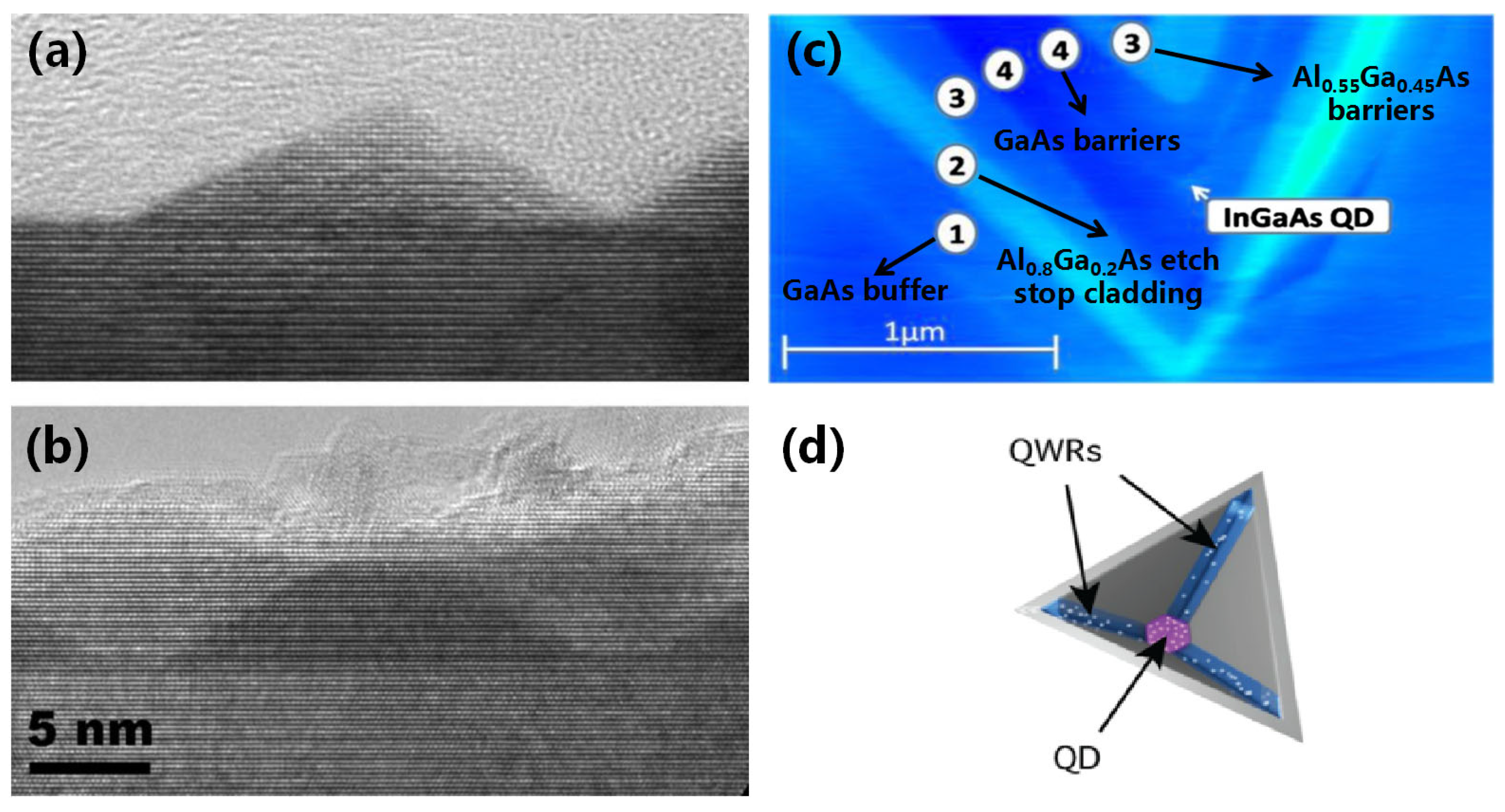
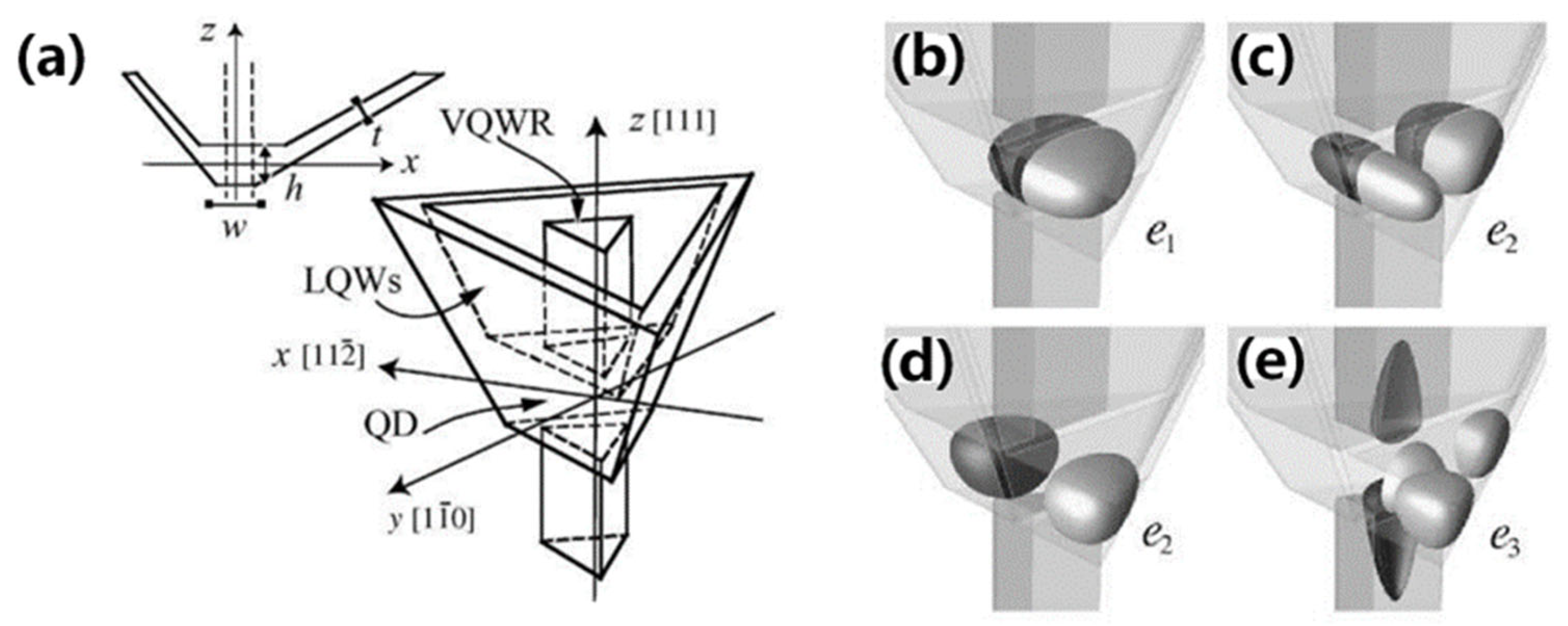
2.1.2. Structure and Growth of Pyramidal III–V Quantum Dots
2.1.3. Structure and Growth of Pyramidal III-Nitride Quantum Dots
2.1.4. Structure and Growth of Pyramidal II–VI Quantum Dots
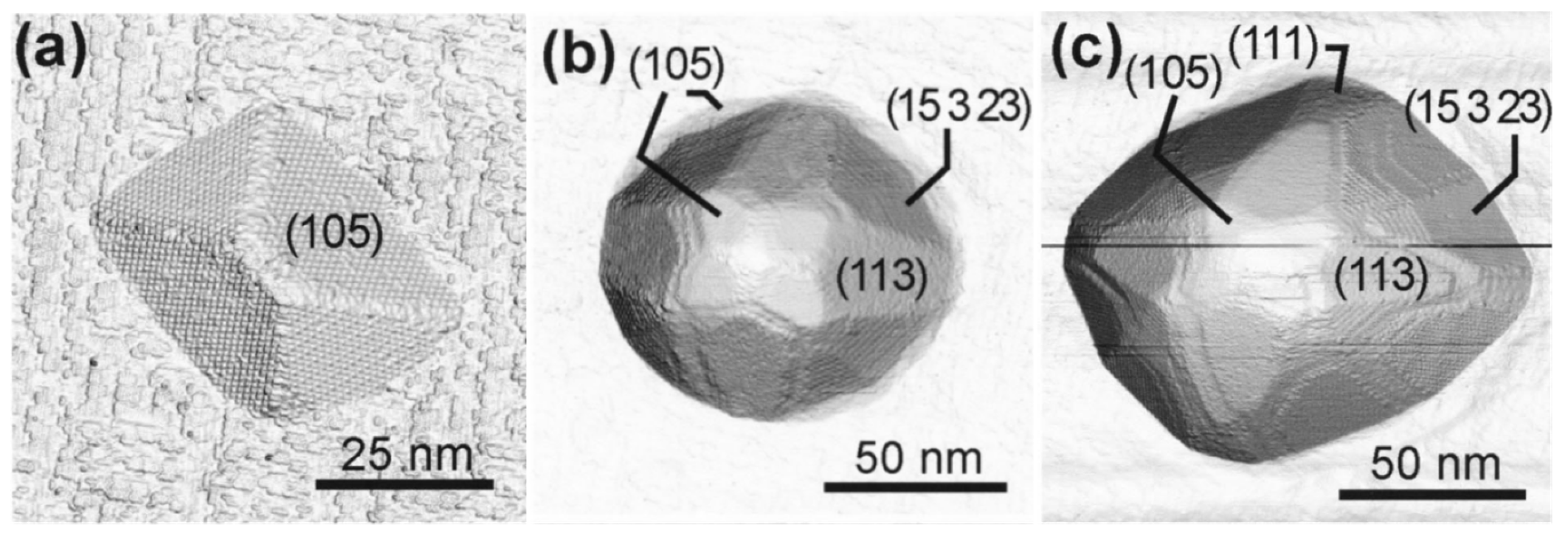
2.1.5. Structure and Growth of Pyramidal IV–VI Quantum Dots
2.1.6. Preparation Methodology of Pyramidal Semiconductor Quantum Dots
2.2. Pyramidal Semiconductor Nanorods (Nanowires) with Different Components
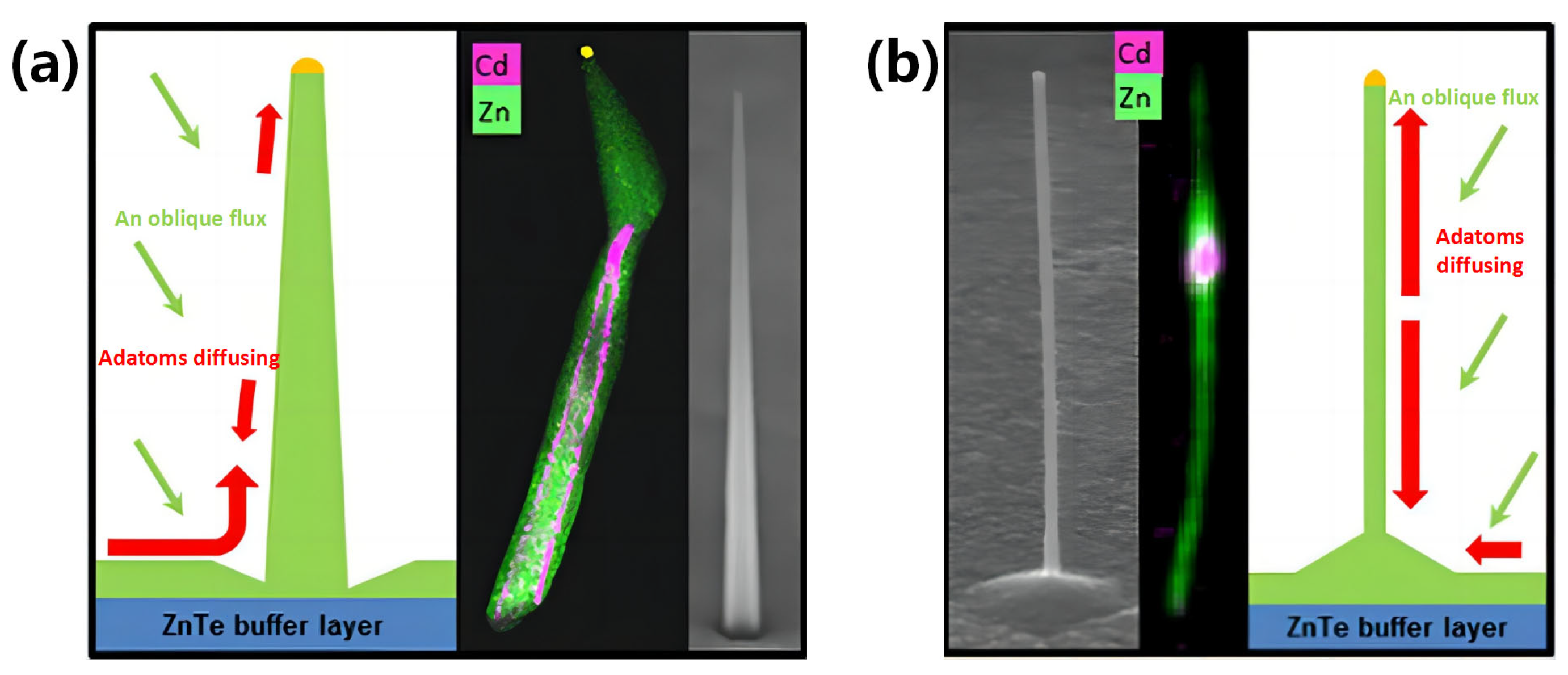
2.2.1. Structure and Growth of Pyramidal II–VI Nanorods (Nanowires)
2.2.2. Other Pyramidal Semiconductor Nanorods (Nanowires)
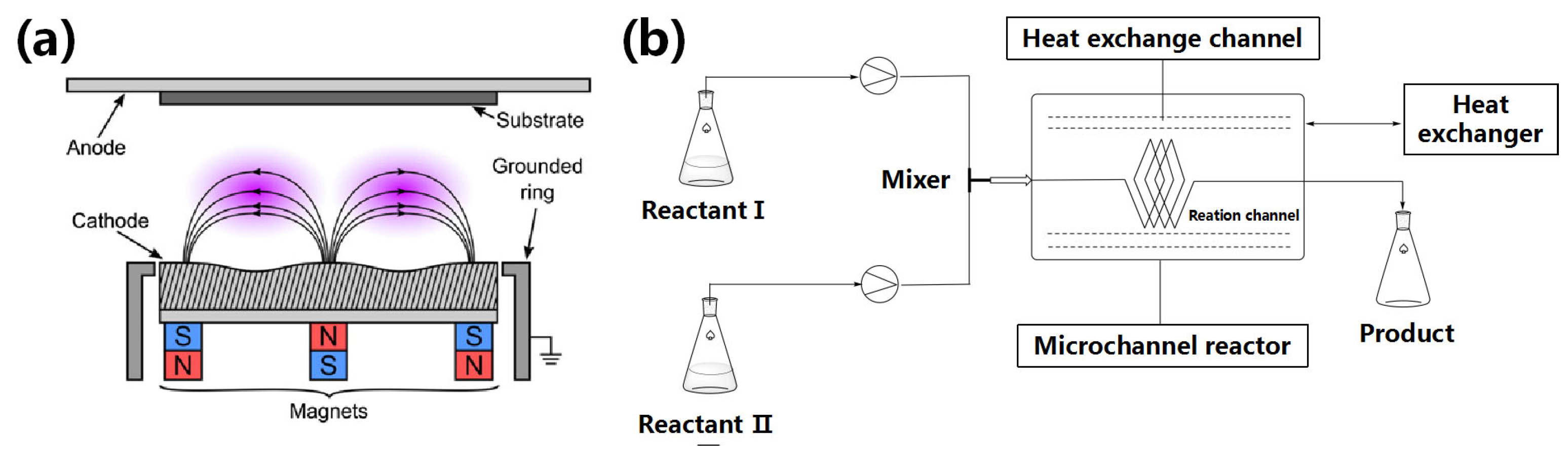
2.2.3. Preparation Methodology of Pyramidal Semiconductor Nanorods (Nanowires)
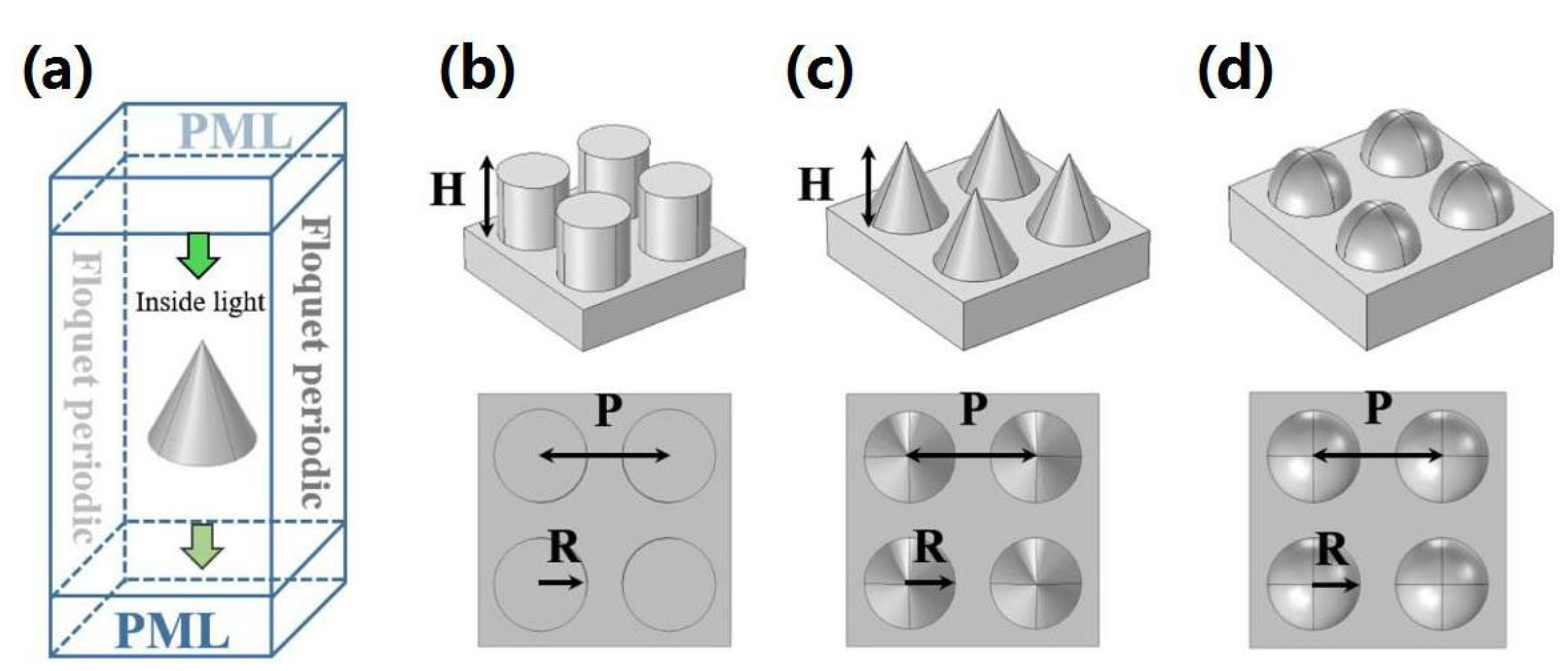
3. Energy Band Structures and Electronic Properties of Pyramidal Low-Dimensional Semiconductors
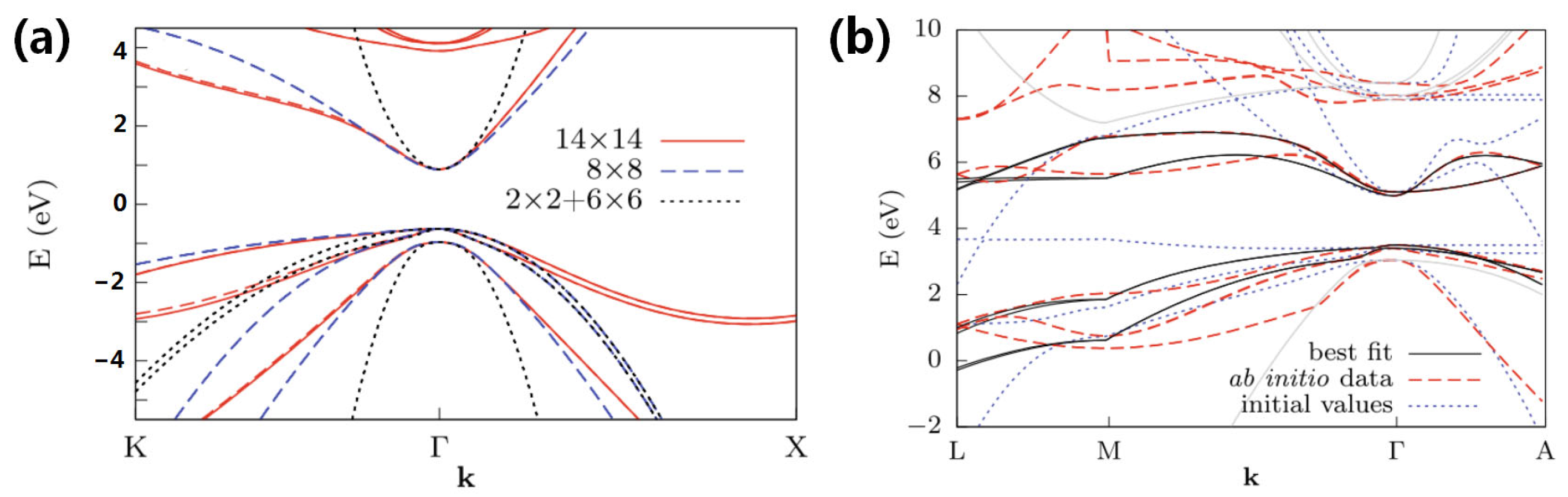
3.1. Definition of the k·p Perturbation Method
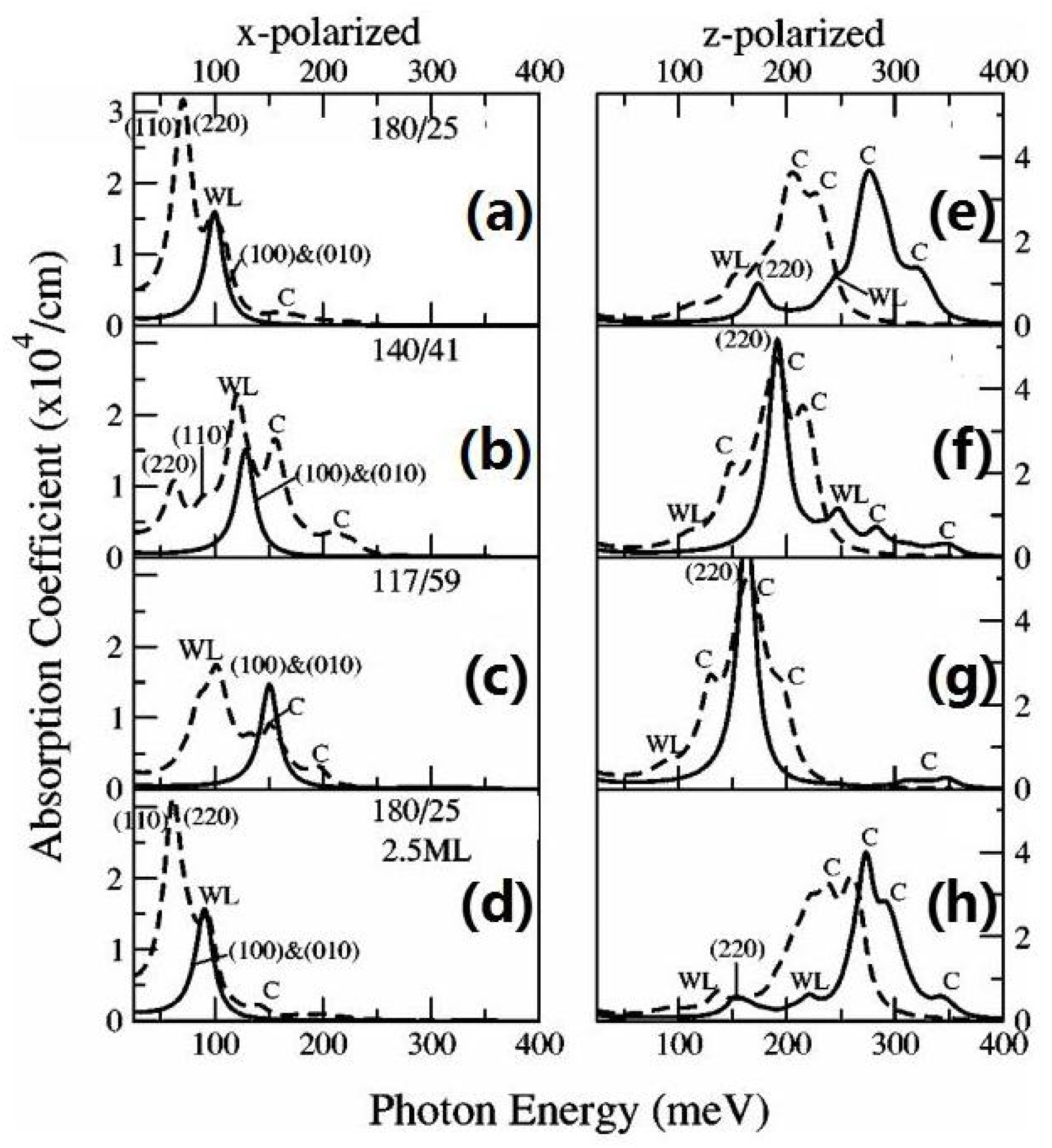
3.2. III–V Pyramidal Semiconductor Quantum Dots
3.3. III-Nitride Pyramidal Semiconductor Quantum Dots
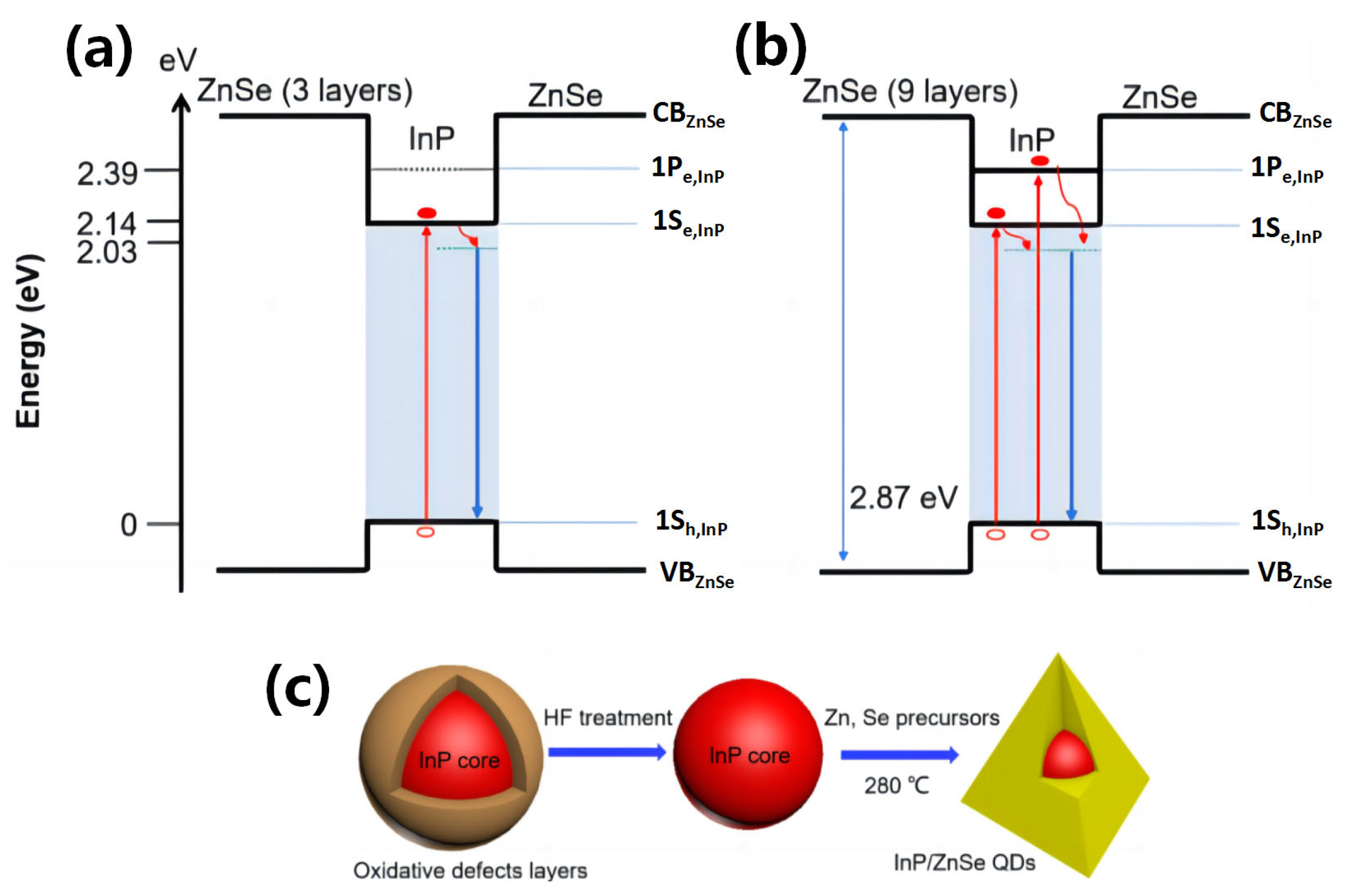
3.4. II–VI Pyramidal Semiconductor Quantum Dots
3.5. Summary
4. Fundamental Optical Processes Based on Pyramidal Low-Dimensional Semiconductors
5. Applications of Pyramidal Low-Dimensional Semiconductors in Optoelectronics
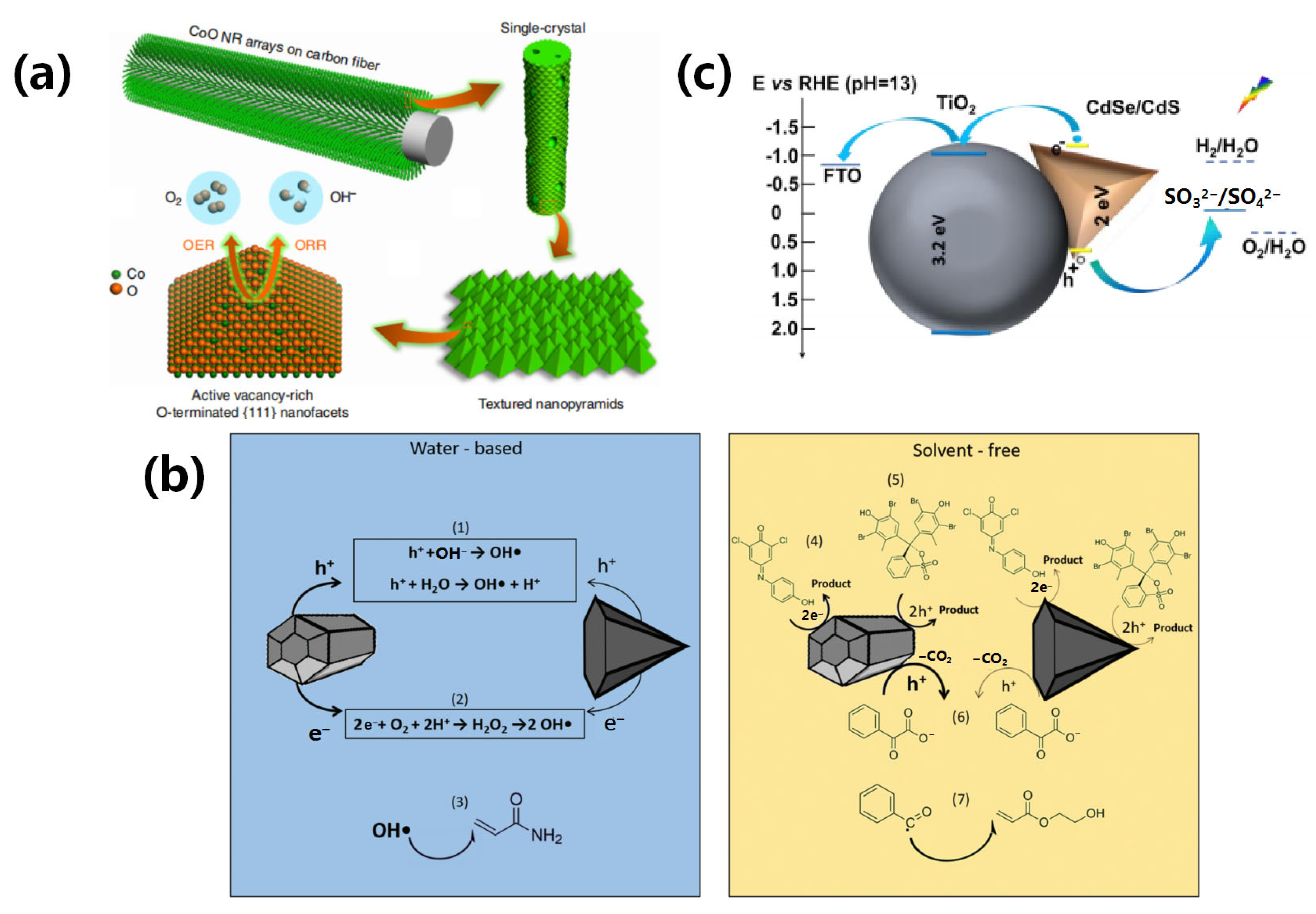
5.1. Photocatalysts Based on Pyramidal Low-Dimensional Semiconductors
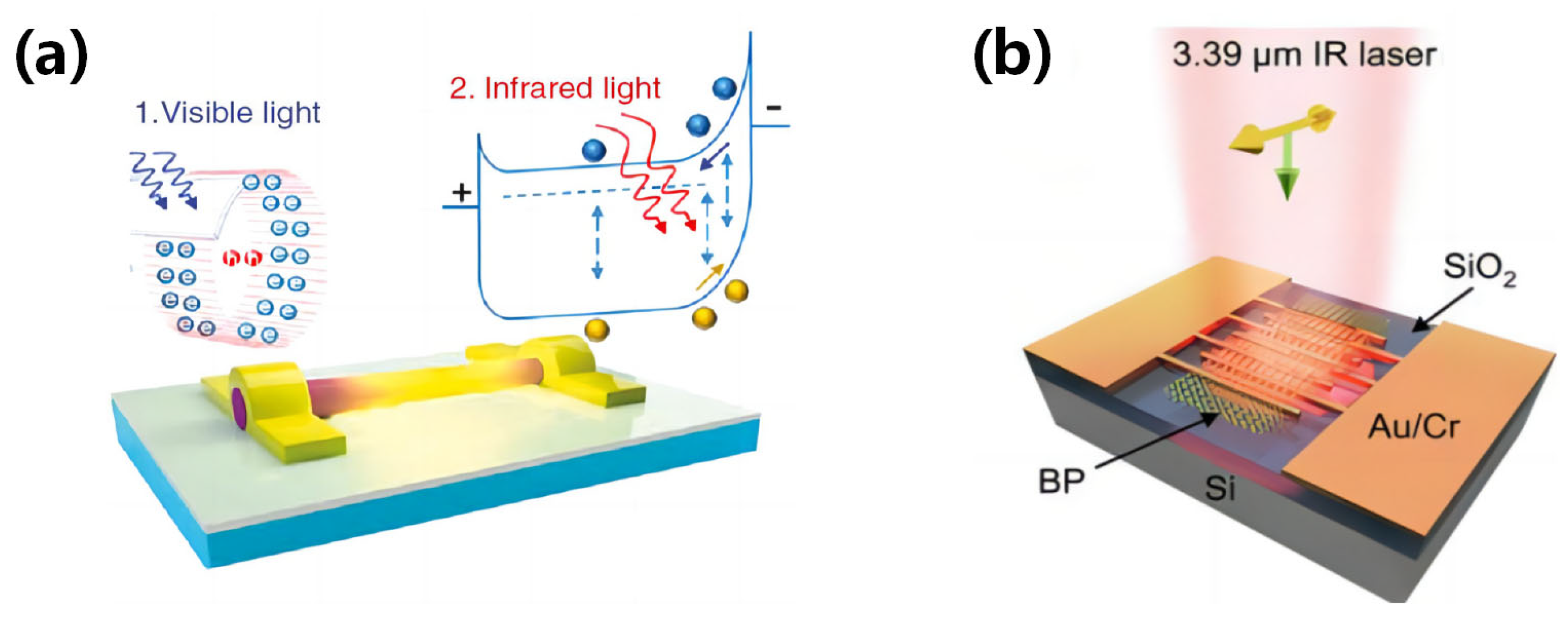
5.2. Photodetectors Based on Pyramidal Low-Dimensional Semiconductors
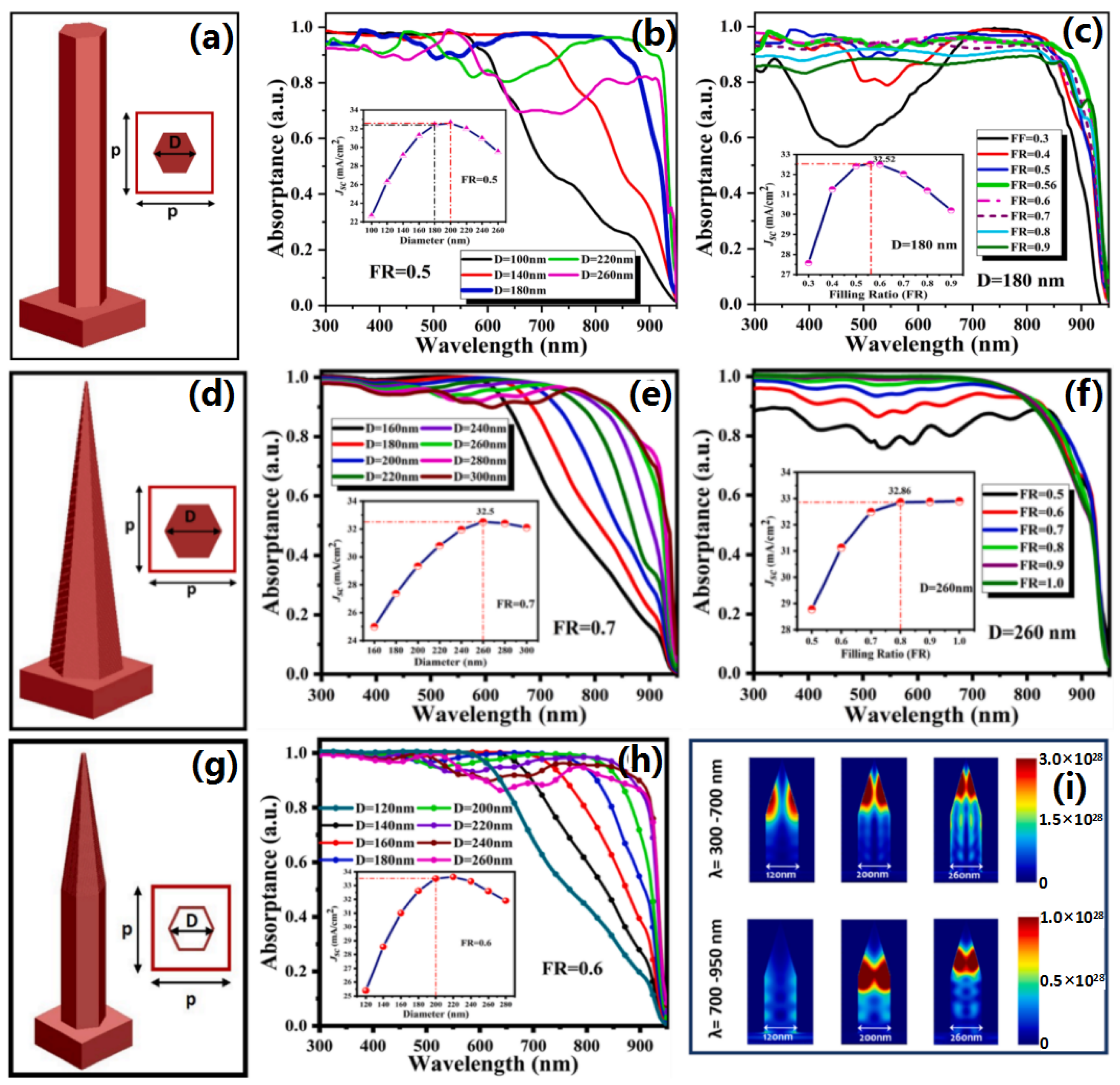
5.3. Solar Cells Based on Pyramidal Low-Dimensional Semiconductors
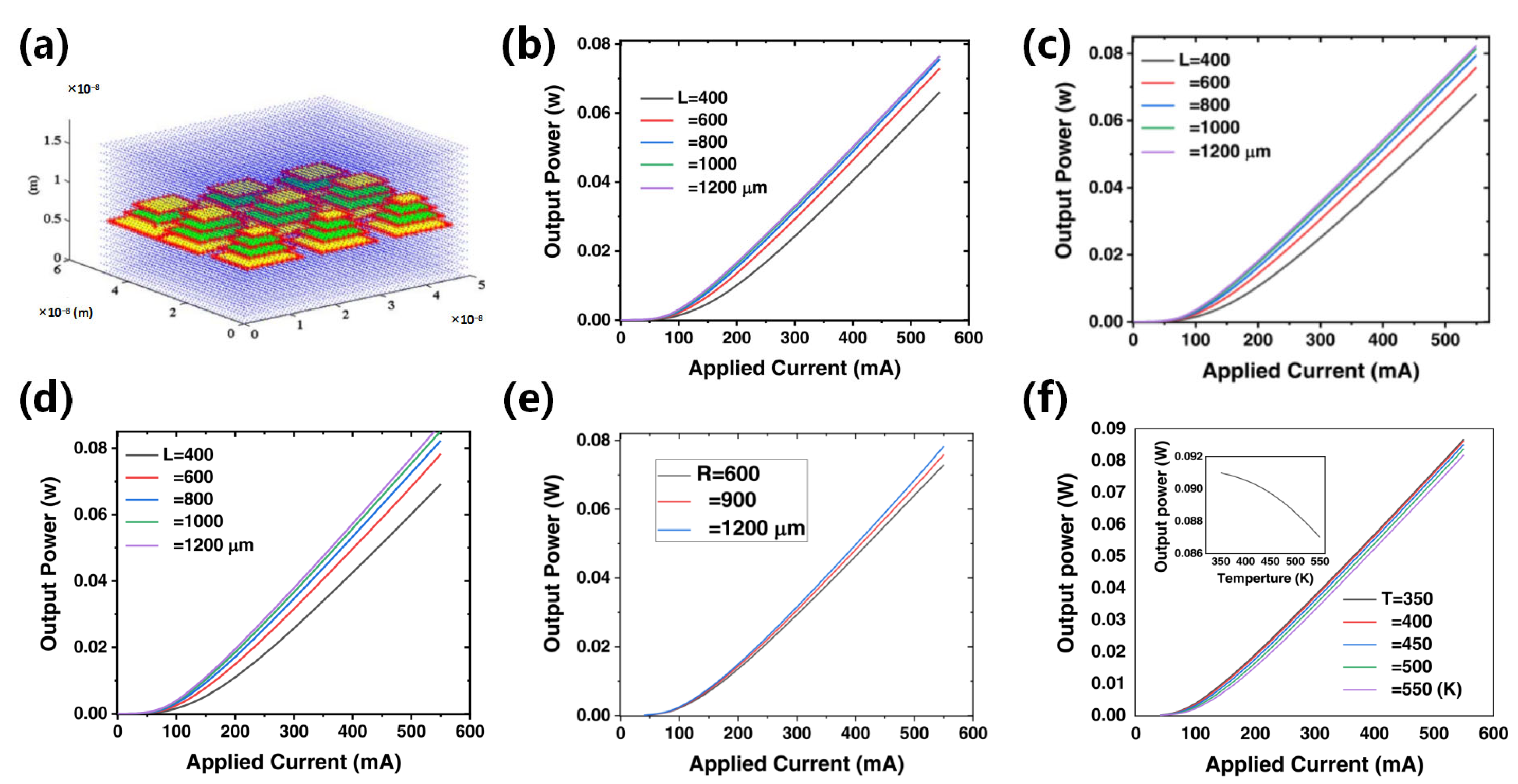
5.4. Luminescent Devices Based on Pyramidal Low-Dimensional Semiconductors
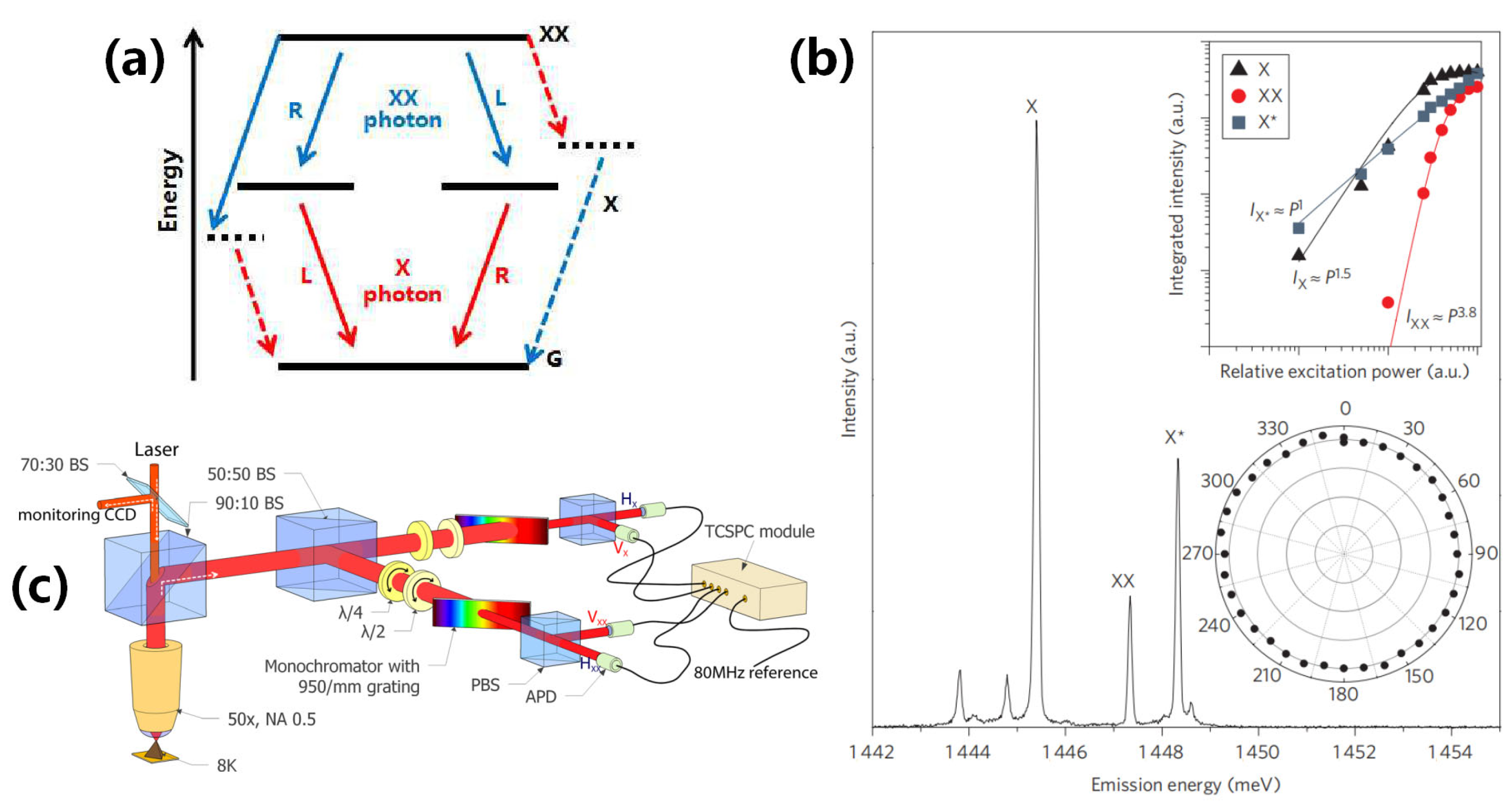
5.5. Quantum Information Applications Based on Pyramidal Low-Dimensional Semiconductors
6. Summary and Perspectives
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Yetisen, A.K.; Qu, H.; Manbachi, A.; Butt, H.; Dokmeci, M.R.; Hinestroza, J.P.; Skorobogatiy, M.; Khademhosseini, A.; Yun, S.H. Nanotechnology in textiles. ACS Nano 2016, 10, 3042–3068. [Google Scholar] [CrossRef] [PubMed]
- Malik, S.; Muhammad, K.; Waheed, Y. Nanotechnology: A revolution in modern industry. Molecules 2023, 28, 661. [Google Scholar] [CrossRef] [PubMed]
- Hussein, A.K. Applications of nanotechnology in renewable energies—A comprehensive overview and understanding. Renew. Sustain. Energy Rev. 2015, 42, 460–476. [Google Scholar] [CrossRef]
- Guerra, F.D.; Attia, M.F.; Whitehead, D.C.; Alexis, F. Nanotechnology for environmental remediation: Materials and applications. Molecules 2018, 23, 1760. [Google Scholar] [CrossRef] [PubMed]
- Ramesh, M.; Janani, R.; Deepa, C.; Rajeshkumar, L. Nanotechnology-enabled biosensors: A review of fundamentals, design principles, materials, and applications. Biosensors 2022, 13, 40. [Google Scholar] [CrossRef]
- Blancon, J.C.; Even, J.; Stoumpos, C.C.; Kanatzidis, M.G.; Mohite, A.D. Semiconductor physics of organic–inorganic 2D halide perovskites. Nat. Nanotechnol. 2020, 15, 969–985. [Google Scholar] [CrossRef] [PubMed]
- Hirohata, A.; Takanashi, K. Future perspectives for spintronic devices. J. Phys. D Appl. Phys. 2014, 47, 193001. [Google Scholar] [CrossRef]
- Farahani, R.D.; Dubé, M.; Therriault, D. Three-dimensional printing of multifunctional nanocomposites: Manufacturing techniques and applications. Adv. Mater. 2016, 28, 5794–5821. [Google Scholar] [CrossRef] [PubMed]
- Abo-Hamad, A.; Hayyan, M.; AlSaadi, M.A.; Hashim, M.A. Potential applications of deep eutectic solvents in nanotechnology. Chem. Eng. J. 2015, 273, 551–567. [Google Scholar] [CrossRef]
- Makam, P.; Gazit, E. Minimalistic peptide supramolecular co-assembly: Expanding the conformational space for nanotechnology. Chem. Soc. Rev. 2018, 47, 3406–3420. [Google Scholar] [CrossRef] [PubMed]
- Amarnath, C.A.; Nanda, S.S.; Papaefthymiou, G.C.; Yi, D.K.; Paik, U. Nanohybridization of low-dimensional nanomaterials: Synthesis, classification, and application. Crit. Rev. Solid State Mater. Sci. 2013, 38, 1–56. [Google Scholar] [CrossRef]
- Cao, Y.; Fatemi, V.; Fang, S.; Watanabe, K.; Taniguchi, T.; Kaxiras, E.; Jarillo-Herrero, P. Unconventional superconductivity in magic-angle graphene superlattices. Nature 2018, 556, 43–50. [Google Scholar] [CrossRef] [PubMed]
- Chiang, W.S.; Fratini, E.; Baglioni, P.; Chen, J.H.; Liu, Y. Pore size effect on methane adsorption in mesoporous silica materials studied by small-angle neutron scattering. Langmuir 2016, 32, 8849–8857. [Google Scholar] [CrossRef] [PubMed]
- Niu, D.; Tang, G.H. The effect of surface wettability on water vapor condensation in nanoscale. Sci. Rep. 2016, 6, 19192. [Google Scholar] [CrossRef] [PubMed]
- Awschalom, D.D.; Smyth, J.F.; Grinstein, G.; Divincenzo, D.P.; Loss, D. Macroscopic quantum tunneling in magnetic proteins. Phys. Rev. Lett. 1992, 68, 3092–3095. [Google Scholar] [CrossRef] [PubMed]
- Wu, P.; Xu, Y.X.; Zhan, J.Y.; Li, Y.; Xue, H.G.; Pang, H. The research development of quantum dots in electrochemical energy storage. Small 2018, 14, 1801479. [Google Scholar] [CrossRef] [PubMed]
- Vajner, D.A.; Rickert, L.; Gao, T.; Kaymazlar, K.; Heindel, T. Quantum communication using semiconductor quantum dots. Adv. Quantum Technol. 2022, 5, 2100116. [Google Scholar] [CrossRef]
- Malhotra, A.; Maldovan, M. Phononic pathways towards rational design of nanowire heat conduction. Nanotechnology 2019, 30, 372002. [Google Scholar] [CrossRef] [PubMed]
- Wu, G.L.; Cheng, Y.H.; Yang, Z.H.; Jia, Z.R.; Wu, H.J.; Yang, L.J.; Li, H.L.; Guo, P.Z.; Lv, H.L. Design of carbon sphere/magnetic quantum dots with tunable phase compositions and boost dielectric loss behavior. Chem. Eng. J. 2018, 333, 519–528. [Google Scholar] [CrossRef]
- Yin, R.; Yang, S.Y.; Li, Q.M.; Zhang, S.D.; Liu, H.; Han, J.; Liu, C.T.; Shen, C.Y. Flexible conductive Ag nanowire/cellulose nanofibril hybrid nanopaper for strain and temperature sensing applications. Sci. Bull. 2020, 65, 899–908. [Google Scholar] [CrossRef] [PubMed]
- Mitzinger, S.; Broeckaert, L.; Massa, W.; Weigend, F.; Dehnen, S. Understanding of multimetallic cluster growth. Nat. Commun. 2016, 7, 10480. [Google Scholar] [CrossRef]
- Zhang, J.; Zhang, S.H.; Zhang, Y.L.; Al-Hartomy, O.; Wageh, S.; Al-Sehemi, A.G.; Hao, Y.B.; Gao, L.F.; Wang, H.; Zhang, H. Colloidal quantum dots: Synthesis, composition, structure, and emerging optoelectronic applications. Laser Photonics Rev. 2023, 17, 2200551. [Google Scholar] [CrossRef]
- Maenosono, S.; Okubo, T.; Yamaguchi, Y. Overview of nanoparticle array formation by wet coating. J. Nanopart. Res. 2003, 5, 5–15. [Google Scholar] [CrossRef]
- Rackauskas, S.; Nasibulin, A.G. Nanowire growth without catalysts: Applications and mechanisms at the atomic scale. ACS Appl. Nano Mater. 2020, 3, 7314–7324. [Google Scholar] [CrossRef]
- Drake, G.A.; Keating, L.P.; Shim, M. Design principles of colloidal nanorod heterostructures. Chem. Rev. 2022, 123, 3761–3789. [Google Scholar] [CrossRef]
- Tenne, R.; Rao, C.N.R. Inorganic nanotubes. Philos. Trans. R. Soc. Lond. Ser. A Math. Phys. Eng. Sci. 2004, 362, 2099–2125. [Google Scholar] [CrossRef] [PubMed]
- Li, D.M.; Cheng, Y.Y.; Luo, Y.X.; Teng, Y.Q.; Liu, Y.H.; Feng, L.B.; Wang, N.; Zhao, Y. Electrospun nanofiber materials for photothermal interfacial evaporation. Materials 2023, 16, 5676. [Google Scholar] [CrossRef]
- Wang, Z.L. ZnO nanowire and nanobelt platform for nanotechnology. Mater. Sci. Eng. R Rep. 2009, 64, 33–71. [Google Scholar] [CrossRef]
- Liu, Y.X.; Chen, E.Q. Polymer crystallization of ultrathin films on solid substrates. Coord. Chem. Rev. 2010, 254, 1011–1037. [Google Scholar] [CrossRef]
- Dai, Z.; Ansaloni, L.; Deng, L. Recent advances in multi-layer composite polymeric membranes for CO2 separation: A review. Green Energy Environ. 2016, 1, 102–128. [Google Scholar] [CrossRef]
- Ning, J.J.; Duan, Z.H.; Kershaw, S.V.; Rogach, A.L. Phase-controlled growth of CuInS2 shells to realize colloidal CuInSe2/CuInS2 core/shell nanostructures. ACS Nano 2020, 14, 11799–11808. [Google Scholar] [CrossRef] [PubMed]
- Ouattara, L.; Mikkelsen, A.; Lundgren, E.; Borgström, M.; Samuelson, L.; Seifert, W. Stacked InAs quantum dots in InP studied by cross-sectional scanning tunnelling microscopy. Nanotechnology 2004, 15, 1701–1707. [Google Scholar] [CrossRef]
- Adhikari, S.; Lem, O.L.C.; Kremer, F.; Vora, K.; Brink, F.; Lysevych, M.; Tan, H.H.; Jagadish, C. Nonpolar AlxGa1–xN/AlyGa1–yN multiple quantum wells on GaN nanowire for UV emission. Nano Res. 2022, 15, 7670–7680. [Google Scholar] [CrossRef]
- Tu, C.G.; Zhang, X.; Chou, K.P.; Tse, W.F.; Hsu, Y.C.; Chen, Y.P.; Kiang, Y.W.; Yang, C.C. AlGaN nano–shell structure on a GaN nanorod formed with the pulsed MOCVD growth. Nanotechnology 2019, 30, 275201. [Google Scholar] [CrossRef] [PubMed]
- Chen, X.Q.; Liu, Y.H.; Wang, B.B.; Liu, X.T.; Lu, C. Understanding role of microstructures of nanomaterials in electrochemiluminescence properties and their applications. TrAC Trends Anal. Chem. 2023, 162, 117030. [Google Scholar] [CrossRef]
- Mishra, N.; Vasavi Dutt, V.G.; Arciniegas, M.P. Recent progress on metal chalcogenide semiconductor tetrapod-shaped colloidal nanocrystals and their applications in optoelectronics. Chem. Mater. 2019, 31, 9216–9242. [Google Scholar] [CrossRef]
- Goyal, M.; Singh, M. Size and shape dependence of optical properties of nanostructures. Appl. Phys. A 2020, 126, 176. [Google Scholar] [CrossRef]
- Wang, C.H.; Zhang, X.T.; Zhang, Y.L.; Jia, Y.; Yuan, B.; Yang, J.K.; Sun, P.P.; Liu, Y.C. Morphologically-tunable TiO2 nanorod film with high energy facets: Green synthesis, growth mechanism and photocatalytic activity. Nanoscale 2012, 4, 5023–5030. [Google Scholar] [CrossRef] [PubMed]
- Rawat, K.; Goyal, M. Modelling to determine the variation of magnetic properties with size and shape in the nanomaterials. Pramana 2021, 95, 184. [Google Scholar] [CrossRef]
- Huang, M.H. Facet-dependent optical properties of semiconductor nanocrystals. Small 2019, 15, 1804726. [Google Scholar] [CrossRef] [PubMed]
- Regulacio, M.D.; Han, M.Y. Composition-tunable alloyed semiconductor nanocrystals. Acc. Chem. Res. 2010, 43, 621–630. [Google Scholar] [CrossRef] [PubMed]
- Burda, C.; Chen, X.B.; Narayanan, R.; El-Sayed, M.A. Chemistry and properties of nanocrystals of different shapes. Chem. Rev. 2005, 105, 1025–1102. [Google Scholar] [CrossRef] [PubMed]
- Esteves, A.C.; Trindade, T. Synthetic studies on II/VI semiconductor quantum dots. Curr. Opin. Solid State Mater. Sci. 2002, 6, 347–353. [Google Scholar] [CrossRef]
- Jang, Y.J.; Shapiro, A.; Isarov, M.; Rubin-Brusilovski, A.; Safran, A.; Budniak, A.K.; Horani, F.; Dehnel, J.; Sashchiuk, A.; Lifshitz, E. Interface control of electronic and optical properties in IV–VI and II–VI core/shell colloidal quantum dots: A review. Chem. Commun. 2017, 53, 1002–1024. [Google Scholar] [CrossRef] [PubMed]
- Fan, G.; Wang, C.; Fang, J. Solution-based synthesis of III–V quantum dots and their applications in gas sensing and bio-imaging. Nano Today 2014, 9, 69–84. [Google Scholar] [CrossRef]
- Wong, P.S.; Liang, B.; Huffaker, D.L. InAs quantum dots on nanopatterned GaAs (001) surface: The growth, optical properties, and device implementation. J. Nanosci. Nanotechnol. 2010, 10, 1537–1550. [Google Scholar] [CrossRef] [PubMed]
- Lopez, M.; Ishikawa, T.; Nomura, Y. Molecular-beam epitaxial growth of pyramidal structures on patterned GaAs [100] substrates for three-dimensionally confined structures. Electron. Lett. 1993, 25, 2225–2227. [Google Scholar] [CrossRef]
- Taher, M.; Al-yousif, S.; Ahmed, N.M. Atomistic modeling of InGaN/GaN quantum dots-in-nanowire for graded surface-emitting low-threshold, blue exciton laser. Results Phys. 2021, 20, 103732. [Google Scholar] [CrossRef]
- Ranjbar Jahromi, I.; Juska, G.; Varo, S.; Basset, F.B.; Salusti, F.; Trotta, R.; Gocalinska, A.; Mattana, F.; Pelucchi, E. Optical properties and symmetry optimization of spectrally (excitonically) uniform site-controlled GaAs pyramidal quantum dots. Appl. Phys. Lett. 2021, 118, 073103. [Google Scholar] [CrossRef]
- Holsgrove, K.M.; O’Reilly, T.I.; Varo, S.; Gocalinska, A.; Juska, G.; Kepaptsoglou, D.M.; Pelucchi, E.; Arredondo, M. Towards 3D characterisation of site-controlled InGaAs pyramidal QDs at the nanoscale. J. Mater. Sci. 2022, 57, 16383–16396. [Google Scholar] [CrossRef] [PubMed]
- Liu, Y.; Bose, S.; Fan, W. Effect of size and shape on electronic and optical properties of CdSe quantum dots. Optik 2018, 155, 242–250. [Google Scholar] [CrossRef]
- Liang, L.; Xie, W. Influence of the shape of quantum dots on their optical absorptions. Phys. B Condens. Matter 2015, 462, 15–17. [Google Scholar] [CrossRef]
- Califano, M. Tetrahedral vs spherical nanocrystals: Does the shape really matter? Chem. Mater. 2024, 36, 1162–1171. [Google Scholar] [CrossRef]
- Diroll, B.T.; Guzelturk, B.; Po, H.; Dabard, C.; Fu, N.Y.; Makke, L.; Lhuillier, E.; Ithurria, S. 2D II–VI semiconductor nanoplatelets: From material synthesis to optoelectronic integration. Chem. Rev. 2023, 123, 3543–3624. [Google Scholar] [CrossRef]
- Mantashian, G.A. The contribution of edge number on the optical properties in ZnO pyramidal quantum dots. In Proceedings of the Conference on Quantum Optics and Photon Counting, Prague, Czech Republic, 26–27 April 2023. [Google Scholar]
- Oberli, D.Y.; Byszewski, M.; Chalupar, B.; Pelucchi, E.; Rudra, A.; Kapon, E. Coulomb correlations of charged excitons in semiconductor quantum dots. Phys. Rev. B 2009, 80, 165312. [Google Scholar] [CrossRef]
- Oberli, D.Y. Intertwining of Zeeman and Coulomb interactions on excitons in highly symmetric semiconductor quantum dots. Phys. Rev. B 2012, 85, 155305. [Google Scholar] [CrossRef]
- Surrente, A.; Felici, M.; Gallo, P.; Rudra, A.; Dwir, B.; Kapon, E. Dense arrays of site-controlled quantum dots with tailored emission wavelength: Growth mechanisms and optical properties. Appl. Phys. Lett. 2017, 111, 221102. [Google Scholar] [CrossRef]
- Zekavat, M.A.; Sabaeian, M.; Solookinejad, G. Graphene plasmonic coupling with intersubband radiation of truncated pyramidal-shaped InAs/GaAs quantum dots. JOSA B 2021, 38, 1824–1833. [Google Scholar] [CrossRef]
- Baira, M.; Salem, B.; Madhar, N.A.; Ilahi, B. Intersubband optical nonlinearity of GeSn quantum dots under vertical electric field. Micromachines 2019, 10, 243. [Google Scholar] [CrossRef] [PubMed]
- Lox, J.F.L.; Dang, Z.Y.; Dzhagan, V.M.; Spittel, D.; Martin-Garcia, B.; Moreels, I.; Zahn, D.R.T.; Lesnyak, V. Near-infrared Cu–In–Se-based colloidal nanocrystals via cation exchange. Chem. Mater. 2018, 30, 2607–2617. [Google Scholar] [CrossRef]
- Cho, B.; Baek, S.; Woo, H.G.; Sohn, H. Synthesis of silicon quantum dots showing high quantum efficiency. J. Nanosci. Nanotechnol. 2014, 14, 5868–5872. [Google Scholar] [CrossRef] [PubMed]
- Wei, F.D.; Lu, X.L.; Wu, Y.Z.; Cai, Z.; Liu, L.P.; Zhou, P.; Hu, Q. Synthesis of highly luminescent CdTe/CdS/ZnS quantum dots by a one-pot capping method. Chem. Eng. J. 2013, 226, 416–422. [Google Scholar] [CrossRef]
- Huang, F.; Huang, B. Aqueous synthesis of water-soluble citrate-modified cadmium selenide/cadmium sulfide/zinc sulfide quantum dots. Spectrosc. Lett. 2015, 48, 422–426. [Google Scholar] [CrossRef]
- Murphy, J.E.; Beard, M.C.; Norman, A.G.; Ahrenkiel, S.P.; Johnson, J.C.; Yu, P.R.; Micic, O.I.; Ellingson, R.J.; Nozik, A.J. PbTe colloidal nanocrystals: Synthesis, characterization, and multiple exciton generation. J. Am. Chem. Soc. 2006, 128, 3241–3247. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Dai, Q.Q.; Li, X.B.; Liang, J.Y.; Colvin, V.L.; Wang, Y.D.; Yu, W.W. PbSe/CdSe and PbSe/CdSe/ZnSe hierarchical nanocrystals and their photoluminescence. Langmuir 2011, 27, 9583–9587. [Google Scholar] [CrossRef] [PubMed]
- Laufersky, G.; Bradley, S.; Frécaut, E.; Lein, M.; Nann, T. Unraveling aminophosphine redox mechanisms for glovebox-free InP quantum dot syntheses. Nanoscale 2018, 10, 8752–8762. [Google Scholar] [CrossRef] [PubMed]
- Cao, Y.W.; Banin, U. Growth and properties of semiconductor core/shell nanocrystals with InAs cores. J. Am. Chem. Soc. 2000, 122, 9692–9702. [Google Scholar] [CrossRef]
- Shamsi, J.; Rastogi, P.; Caligiuri, V.; Abdelhady, A.L.; Spirito, D.; Manna, L.; Krahne, R. Bright-emitting perovskite films by large-scale synthesis and photoinduced solid-state transformation of CsPbBr3 nanoplatelets. ACS Nano 2017, 11, 10206–10213. [Google Scholar] [CrossRef]
- Takeda, M.; Yokoyama, M.; Umemoto, K.; Lyu, B.; Takahashi, Y.; Rodbuntum, S.; Enomoto, J.; Tozawa, K.; Nohara, T.; Tabata, K.; et al. Synthesis of highly luminescent CH3NH3PbBr3 perovskite nanocrystals via a forced thin film reactor. Jpn. J. Appl. Phys. 2020, 59, SIIG02. [Google Scholar] [CrossRef]
- Saidzhonov, B.M.; Zaytsev, V.B.; Berekchiian, M.V.; Vasiliev, R.B. Highly luminescent copper-doped ultrathin CdSe nanoplatelets for white-light generation. J. Lumin. 2020, 222, 117134. [Google Scholar] [CrossRef]
- Kelestemur, Y.; Guzelturk, B.; Erdem, O.; Olutas, M.; Gungor, K.; Demir, H.V. Platelet-in-box colloidal quantum wells: CdSe/CdS@CdS core/crown@shell heteronanoplatelets. Adv. Funct. Mater. 2016, 26, 3570–3579. [Google Scholar] [CrossRef]
- Tan, R.; Yuan, Y.C.; Nagaoka, Y.; Eggert, D.; Wang, X.D.; Thota, S.; Guo, P.; Yang, H.R.; Zhao, J.; Chen, O. Monodisperse hexagonal pyramidal and bipyramidal wurtzite CdSe-CdS core-shell nanocrystals. Chem. Mater. 2017, 29, 4097–4108. [Google Scholar] [CrossRef]
- Langevin, M.A.; Ritcey, A.M.; Allen, C.N. Air-stable near-infrared AgInSe2 nanocrystals. ACS Nano 2014, 8, 3476–3482. [Google Scholar] [CrossRef] [PubMed]
- Li, R.F.; Lee, J.; Yang, B.C.; Horspool, D.N.; Aindow, M.; Papadimitrakopoulos, F. Amine-assisted facetted etching of CdSe nanocrystals. J. Am. Chem. Soc. 2005, 127, 2524–2532. [Google Scholar] [CrossRef] [PubMed]
- Chetty, S.S.; Praneetha, S.; Murugan, A.V.; Govarthanan, K.; Verma, R.S. Microwave-assisted synthesis of quasi-pyramidal CuInS2–ZnS nanocrystals for enhanced near-Infrared targeted fluorescent imaging of subcutaneous melanoma. Adv. Biosyst. 2019, 3, 1800127. [Google Scholar] [CrossRef] [PubMed]
- Hollinger, R.; Gupta, D.; Zapf, M.; Röder, R.; Kartashov, D.; Ronning, C.; Spielmann, C. Single nanowire defined emission properties of ZnO nanowire arrays. J. Phys. D Appl. Phys. 2019, 52, 295101. [Google Scholar] [CrossRef]
- Atkinson, J.; Goldthorpe, I.A. Near-infrared properties of silver nanowire networks. Nanotechnology 2020, 31, 365201. [Google Scholar] [CrossRef] [PubMed]
- Abhilash, T.S.; Mathew, J.P.; Sengupta, S.; Gokhale, M.R.; Bhattacharya, A.; Deshmukh, M.M. Wide bandwidth nanowire electromechanics on insulating substrates at room temperature. Nano Lett. 2012, 12, 6432–6435. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Qi, J.W.; Li, Y.D.; Yang, M.; Wu, Q.; Chen, Z.Q.; Peng, J.Y.; Liu, Y.; Wang, W.D.; Yu, X.Y.; Sun, Q.; et al. Fabrication of nanowire network AAO and its application in SERS. Nanoscale Res. Lett. 2013, 8, 495. [Google Scholar]
- Morales, A.M.; Lieber, C.M. A laser ablation method for the synthesis of crystalline semiconductor nanowires. Science 1998, 279, 208–211. [Google Scholar] [CrossRef] [PubMed]
- Zhang, W.; Yang, S. In situ fabrication of inorganic nanowire arrays grown from and aligned on metal substrates. Acc. Chem. Res. 2009, 42, 1617–1627. [Google Scholar] [CrossRef] [PubMed]
- Xiong, S.; Zhang, X.; Qian, Y. CdS with various novel hierarchical nanostructures by nanobelts/nanowires self-assembly: Controllable preparation and their optical properties. Cryst. Growth Des. 2009, 9, 5259–5265. [Google Scholar] [CrossRef]
- Zhu, J.; Xu, Z.H.; Ha, S.H.; Li, D.K.; Zhang, K.X.; Zhang, H.; Feng, J.J. Gallium oxide for gas sensor applications: A comprehensive review. Materials 2022, 15, 7339. [Google Scholar] [CrossRef] [PubMed]
- Shah, K.A.; Tali, B.A. Synthesis of carbon nanotubes by catalytic chemical vapour deposition: A review on carbon sources, catalysts and substrates. Mater. Sci. Semicond. Process. 2016, 41, 67–82. [Google Scholar] [CrossRef]
- Ruach-Nir, I.; Zhang, Y.; Popovitz-Biro, R.; Rubinstein, I.; Hodes, G. Shape control in electrodeposited, epitaxial CdSe nanocrystals on (111) gold. J. Phys. Chem. B 2003, 107, 2174–2179. [Google Scholar] [CrossRef]
- Li, Y.; Qian, F.; Xiang, J.; Lieber, C.M. Nanowire electronic and optoelectronic devices. Mater. Today 2006, 9, 18–27. [Google Scholar] [CrossRef]
- Jia, C.C.; Lin, Z.Y.; Huang, Y.; Duan, X.F. Nanowire electronics: From nanoscale to macroscale. Chem. Rev. 2019, 119, 9074–9135. [Google Scholar] [CrossRef] [PubMed]
- Shinagawa, T.; Shibata, K.; Shimomura, O.; Chigane, M.; Nomura, R.; Izaki, M. Solution-processed high-haze ZnO pyramidal textures directly grown on a TCO substrate and the light-trapping effect in Cu2O solar cells. J. Mater. Chem. C 2014, 2, 2908–2917. [Google Scholar] [CrossRef]
- Riley, J.R.; Padalkar, S.; Li, Q.M.; Lu, P.; Koleske, D.D.; Wierer, J.J.; Wang, G.T.; Lauhon, L.J. Three-dimensional mapping of quantum wells in a GaN/InGaN core–shell nanowire light-emitting diode array. Nano Lett. 2013, 13, 4317–4325. [Google Scholar] [CrossRef] [PubMed]
- Chen, K.M.; Jesson, D.E.; Pennycook, S.J.; Thundat, T.; Warmack, R.J. Critical nuclei shapes in the stress-driven 2D-to-3D transition. Phys. Rev. B 1997, 56, R1700–R1703. [Google Scholar] [CrossRef]
- Vailionis, A.; Cho, B.; Glass, G.; Desjardins, P.; Cahill, D.G.; Greene, J.E. Pathway for the strain-driven two-dimensional to three-dimensional transition during growth of Ge on Si(001). Phys. Rev. Lett. 2000, 85, 3672–3675. [Google Scholar] [CrossRef] [PubMed]
- Mo, Y.W.; Savage, D.E.; Swartzentruber, B.S.; Lagally, M.G. Kinetic pathway in the Stranski–Krastanow growth of Ge on Si(001). Phys. Rev. Lett. 1990, 65, 1020–1023. [Google Scholar] [CrossRef] [PubMed]
- Sutter, E.; Sutter, P.; Bernard, J.E. Extended shape evolution of low-mismatch Si1−xGex alloy islands on Si(100). Appl. Phys. Lett. 2004, 84, 2262–2264. [Google Scholar] [CrossRef]
- Stoffel, M.; Rastelli, A.; Tersoff, J.; Merdzhanova, T.; Schmidt, O.G. Local equilibrium and global relaxation of strained SiGe/Si(001) layers. Phys. Rev. B 2006, 74, 155326. [Google Scholar] [CrossRef]
- Rastelli, A.; von Känel, H. Island formation and faceting in the SiGe/Si(001) system. Surf. Sci. 2003, 532, 769–773. [Google Scholar] [CrossRef]
- Medeiros-Ribeiro, G.; Bratkovski, A.M.; Kamins, T.I.; Ohlberg, D.A.A.; Williams, R.S. Shape transition of germanium nanocrystals on a silicon (001) surface from pyramids to domes. Science 1998, 279, 353–355. [Google Scholar] [CrossRef] [PubMed]
- Ohlsson, B.J.; Björk, M.T.; Persson, A.I.; Thelander, C.; Wallenberg, L.R.; Magnusson, M.H.; Deppert, K.; Samuelson, L. Growth and characterization of GaAs and InAs nano-whiskers and InAs/GaAs heterostructures. Phys. E Low-Dimens. Syst. Nanostruct. 2002, 13, 1126–1130. [Google Scholar] [CrossRef]
- Imran, A.; Jiang, J.L.; Eric, D.; Zahid, M.N.; Yousaf, M.; Shah, Z.H. Optical properties of InAs/GaAs quantum dot superlattice structures. Results Phys. 2018, 9, 297–302. [Google Scholar] [CrossRef]
- Xu, M.C.; Temko, Y.; Suzuki, T.; Jacobi, K. Shape transition of InAs quantum dots on GaAs(001). J. Appl. Phys. 2005, 98, 083525. [Google Scholar] [CrossRef]
- Kratzer, P.; Liu, Q.K.K.; Acosta-Diaz, P.; Manzano, C.; Costantini, G.; Songmuang, R.; Rastelli, A.; Schmidt, O.G.; Kern, K. Shape transition during epitaxial growth of InAs quantum dots on GaAs(001): Theory and Experiment. Phys. Rev. B 2006, 73, 205347. [Google Scholar] [CrossRef]
- Fukui, T.; Kumakura, K.; Nakakoshi, K.; Motohisa, J. Pyramidal quantum dot structures by self-limited selective area metalorganic vapor phase epitaxy. Solid-State Electron. 1996, 40, 799–802. [Google Scholar]
- Hartmann, A.; Loubies, L.; Reinhardt, F.; Kapon, E. Self-limiting growth of quantum dot heterostructures on nonplanar {111}B substrates. Appl. Phys. Lett. 1997, 71, 1314–1316. [Google Scholar] [CrossRef]
- Pelucchi, E.; Baier, M.; Ducommun, Y.; Watanabe, S.; Kapon, E. High-quality InxGa1–xAs/Al0.30Ga0.70As quantum dots grown in inverted pyramids. Phys. Status Solidi B 2003, 238, 233–236. [Google Scholar] [CrossRef]
- Dimastrodonato, V.; Mereni, L.O.; Young, R.J.; Pelucchi, E. Growth and structural characterization of pyramidal site-controlled quantum dots with high uniformity and spectral purity. Phys. Status Solidi B 2010, 247, 1862–1866. [Google Scholar] [CrossRef]
- Prohl, C.; Lenz, A.; Roy, D.; Schuppang, J.; Stracke, G.; Strittmatter, A.; Pohl, U.W.; Bimberg, D.; Eisele, H.; Dähne, M. Spatial structure of In0. 25Ga0. 75As/GaAs/GaP quantum dots on the atomic scale. Appl. Phys. Lett. 2013, 102, 123102. [Google Scholar] [CrossRef]
- Gajjela, R.S.R.; Hendriks, A.L.; Douglas, J.O.; Sala, E.M.; Steindl, P.; Klenovsky, P.; Bagot, P.A.J.; Moody, M.P.; Bimberg, D.; Koenraad, P.M. Structural and compositional analysis of (InGa)(AsSb)/GaAs/GaP Stranski–Krastanov quantum dots. Light Sci. Appl. 2021, 10, 125. [Google Scholar] [CrossRef] [PubMed]
- Hasan, S.; Merckling, C.; Pantouvaki, M.; Meersschaut, J.; Van Campenhout, J.; Vandervorst, W. Thermodynamic modelling of InAs/InP(001) growth towards quantum dots formation by metalorganic vapor phase epitaxy. J. Cryst. Growth 2019, 509, 133–140. [Google Scholar] [CrossRef]
- Weng, G.E.; Ling, A.K.; Lv, X.Q.; Zhang, J.Y.; Zhang, B.P. III-Nitride-based quantum dots and their optoelectronic applications. Nano-Micro Lett. 2011, 3, 200–207. [Google Scholar] [CrossRef]
- Ramvall, P.; Riblet, P.; Nomura, S.; Aoyagi, Y.; Tanaka, S. Optical properties of GaN quantum dots. J. Appl. Phys. 2000, 87, 3883–3890. [Google Scholar] [CrossRef]
- Korytov, M.; Benaissa, M.; Brault, J.; Huault, T.; Neisius, T.; Vennégués, P. Effects of capping on GaN quantum dots deposited on Al0.5Ga0.5N by molecular beam epitaxy. Appl. Phys. Lett. 2009, 94, 143105. [Google Scholar] [CrossRef]
- Dimastrodonato, V.; Mereni, L.O.; Juska, G.; Pelucchi, E. Impact of nitrogen incorporation on pseudomorphic site-controlled quantum dots grown by metalorganic vapor phase epitaxy. Appl. Phys. Lett. 2010, 97, 072115. [Google Scholar] [CrossRef]
- Carron, R.; Gallo, P.; Dwir, B.; Rudra, A.; Kapon, E. Dilute-nitride GaInAsN/GaAs site-controlled pyramidal quantum dots. Appl. Phys. Lett. 2011, 99, 181113. [Google Scholar] [CrossRef]
- Al-Douri, Y.; Khan, M.; Jennings, J.R. Synthesis and optical properties of II-VI semiconductor quantum dots: A review. J. Mater. Sci. Mater. Electron. 2023, 34, 993. [Google Scholar] [CrossRef]
- Suemune, I.; Yoshida, K.; Kumano, H.; Tawara, T.; Ueta, A.; Tanaka, S. II-VI quantum dots grown by MOVPE. J. Cryst. Growth 2003, 248, 301–309. [Google Scholar] [CrossRef]
- Scheerschmidt, K.; Conrad, D.; Kirmse, H.; Schneider, R.; Neumann, W. Electron microscope characterization of CdSe/ZnSe quantum dots based on molecular dynamics structure relaxations. Ultramicroscopy 2000, 81, 289–300. [Google Scholar] [CrossRef] [PubMed]
- Yanover, D.; Vaxenburg, R.; Tilchin, J.; Rubin-Brusilovski, A.; Zaiats, G.; Capek, R.K.; Sashchiuk, A.; Lifshitz, E. Significance of small-sized PbSe/PbS core/shell colloidal quantum dots for optoelectronic applications. J. Phys. Chem. C 2014, 118, 17001–17009. [Google Scholar] [CrossRef]
- Fu, H.; Tsang, S.W. Infrared colloidal lead chalcogenide nanocrystals: Synthesis, properties, and photovoltaic applications. Nanoscale 2012, 4, 2187–2201. [Google Scholar] [CrossRef] [PubMed]
- Zhang, X.; Shen, J.X.; Van de Walle, C.G. Anomalous auger recombination in PbSe. Phys. Rev. Lett. 2020, 125, 037401. [Google Scholar] [CrossRef] [PubMed]
- Abtin, L.; Springholz, G.; Holy, V. Surface exchange and shape transitions of PbSe quantum dots during overgrowth. Phys. Rev. Lett. 2006, 97, 266103. [Google Scholar] [CrossRef] [PubMed]
- Preobrajenski, A.B.; Barucki, K.; Chassé, T. Exploiting the difference in lattice structures for formation of self-assembled PbS dots on InP (110). Phys. Rev. Lett. 2000, 85, 4337–4340. [Google Scholar] [CrossRef] [PubMed]
- Choy, K.L. Chemical vapour deposition of coatings. Prog. Mater. Sci. 2003, 48, 57–170. [Google Scholar] [CrossRef]
- Johansson, J.; Carlsson, N.; Seifert, W. Manipulations of size and density of self-assembled quantum dots grown by MOVPE. Phys. E Low-Dimens. Syst. Nanostruct. 1998, 2, 667–671. [Google Scholar] [CrossRef]
- Kapon, E. Pyramidal quantum dots grown by organometallic chemical vapor deposition on patterned substrates. In Lateral Aligment of Epitaxial Quantum Dots, 1st ed.; Schmidt, O., Ed.; Springer: Berlin/Heidelberg, Germany, 2007; Volume 1, pp. 591–638. [Google Scholar]
- Pelucchi, E.; Moroni, S.T.; Dimastrodonato, V.; Vvedensky, D.D. Self-ordered nanostructures on patterned substrates: Experiment and theory of metalorganic vapor-phase epitaxy of V-groove quantum wires and pyramidal quantum dots. J. Mater. Sci. Mater. Electron. 2018, 29, 952–967. [Google Scholar] [CrossRef]
- Cho, A.Y. Advances in molecular beam epitaxy (MBE). J. Cryst. Growth 1991, 111, 1–13. [Google Scholar] [CrossRef]
- Yang, F.; Liang, Y.; Liu, L.X.; Zhu, Q.; Wang, W.H.; Zhu, X.T.; Guo, J.D. Controlled growth of complex polar oxide films with atomically precise molecular beam epitaxy. Front. Phys. 2018, 13, 136802. [Google Scholar] [CrossRef]
- Gurioli, M.; Wang, Z.M.; Rastelli, A.; Kuroda, T.; Sanguinetti, S. Droplet epitaxy of semiconductor nanostructures for quantum photonic devices. Nat. Mater. 2019, 18, 799–810. [Google Scholar] [CrossRef] [PubMed]
- Sanguinetti, S.; Koguchi, N.; Mano, T.; Kuroda, T. Droplet epitaxy quantum ring structures. J. Nanoelectron. Optoelectron. 2011, 6, 34–50. [Google Scholar] [CrossRef]
- Vichi, S.; Bietti, S.; Khalili, A.; Costanzo, M.; Cappelluti, F.; Esposito, L.; Somaschini, C.; Fedorov, A.; Tsukamoto, S.; Rauter, P.; et al. Droplet epitaxy quantum dot based infrared photodetectors. Nanotechnology 2020, 31, 245203. [Google Scholar] [CrossRef] [PubMed]
- Wu, J.; Shao, D.L.; Dorogan, V.G.; Li, A.Z.; Li, S.B.; DeCuir, E.A.; Manasreh, M.O.; Wang, Z.M.; Mazur, Y.I.; Salamo, G.J. Intersublevel infrared photodetector with strain-free GaAs quantum dot pairs grown by high-temperature droplet epitaxy. Nano Lett. 2010, 10, 1512–1516. [Google Scholar] [CrossRef] [PubMed]
- Watanabe, K.; Koguchi, N.; Gotoh, Y. Fabrication of GaAs quantum dots by modified droplet epitaxy. Jpn. J. Appl. Phys. 2000, 39, L79–L81. [Google Scholar] [CrossRef]
- Pruna, A.; Shao, Q.; Kamruzzaman, M.; Li, Y.Y.; Zapien, J.A.; Pullini, D.; Mataix, D.B.; Ruotolo, A. Effect of ZnO core electrodeposition conditions on electrochemical and photocatalytic properties of polypyrrole-graphene oxide shelled nanoarrays. Appl. Surf. Sci. 2017, 392, 801–809. [Google Scholar] [CrossRef]
- Raha, S.; Ahmaruzzaman, M. ZnO nanostructured materials and their potential applications: Progress, challenges and perspectives. Nanoscale Adv. 2022, 4, 1868–1925. [Google Scholar] [CrossRef]
- Janotti, A.; Van de Walle, C.G. Fundamentals of zinc oxide as a semiconductor. Rep. Prog. Phys. 2009, 72, 126501. [Google Scholar] [CrossRef]
- Sharma, D.K.; Shukla, S.; Sharma, K.K.; Kumar, V. A review on ZnO: Fundamental properties and applications. Mater. Today Proc. 2022, 49, 3028–3035. [Google Scholar] [CrossRef]
- Fan, J.C.; Sreekanth, K.M.; Xie, Z.; Chang, S.L.; Rao, K.V. p-Type ZnO materials: Theory, growth, properties and devices. Prog. Mater. Sci. 2013, 58, 874–985. [Google Scholar] [CrossRef]
- Yuan, X.M.; Yang, J.B.; He, J.; Tan, H.H.; Jagadish, C. Role of surface energy in nanowire growth. J. Phys. D Appl. Phys. 2018, 51, 283002. [Google Scholar] [CrossRef]
- Ghorbani, L.; Nasirian, S. Zinc oxide nanorods assisted by polyaniline network as a flexible self-powered ultraviolet photodetector: A comprehensive study. Appl. Surf. Sci. 2020, 527, 146786. [Google Scholar] [CrossRef]
- Ali, N.M.; Ali, T.A.; Rafat, N.H. Modeling of perovskite solar cells containing hexagonal- shaped nanorods. Opt. Quantum Electron. 2022, 54, 97. [Google Scholar] [CrossRef]
- McPeak, K.M.; Baxter, J.B. ZnO nanowires grown by chemical bath deposition in a continuous flow microreactor. Cryst. Growth Des. 2009, 9, 4538–4545. [Google Scholar] [CrossRef]
- Tharsika, T.; Haseeb, A.S.M.A.; Akbar, S.A.; Thanihaichelvan, M. Tailoring ZnO nanostructures by spray pyrolysis and thermal annealing. Ceram. Int. 2015, 41, 5205–5211. [Google Scholar] [CrossRef]
- Patra, M.K.; Manzoor, K.; Manoth, M.; Vadera, S.R.; Kumar, N. Studies on structural and magnetic properties of Co-doped pyramidal ZnO nanorods synthesized by solution growth technique. J. Phys. Chem. Solids 2009, 70, 659–664. [Google Scholar] [CrossRef]
- Kim, Y.; Kong, B.H.; Cho, H.K. Vertically arrayed Ga-doped ZnO nanorods grown by magnetron sputtering: The effect of Ga contents and microstructural evaluation. J. Cryst. Growth 2011, 330, 17–21. [Google Scholar] [CrossRef]
- Seo, J.U.; Park, C.M. ZnTe and ZnTe/C nanocomposite: A new electrode material for high-performance rechargeable Li-ion batteries. J. Mater. Chem. A 2014, 2, 20075–20082. [Google Scholar] [CrossRef]
- Seifert, T.; Jaiswal, S.; Martens, U.; Hannegan, J.; Braun, L.; Maldonado, P.; Freimuth, F.; Kronenberg, A.; Henrizi, J.; Radu, I.; et al. Efficient metallic spintronic emitters of ultrabroadband terahertz radiation. Nat. Photonics 2016, 10, 483–488. [Google Scholar] [CrossRef]
- Erwin, S.C.; Zu, L.J.; Haftel, M.I.; Efros, A.L.; Kennedy, T.A.; Norris, D.J. Doping semiconductor nanocrystals. Nature 2005, 436, 91–94. [Google Scholar] [CrossRef] [PubMed]
- Rueda-Ponseca, P.; Bellet-Amalric, E.; Vigliaturo, R.; den Hertog, M.; Genuist, Y.; André, R.; Robin, E.; Artioli, A.; Stepanov, P.; Ferrand, D.; et al. Structure and morphology in diffusion-driven growth of nanowires: The case of ZnTe. Nano Lett. 2014, 14, 1877–1883. [Google Scholar] [CrossRef] [PubMed]
- Khan, K.; Jian, Z.; Li, J.A.T.; Sun, K.; Ahmadi, E. Selective-area growth of GaN and AlGaN nanowires on N-polar GaN templates with 4° miscut by plasma-assisted molecular beam epitaxy. J. Cryst. Growth 2023, 611, 127181. [Google Scholar] [CrossRef]
- Regonini, D.; Bowen, C.R.; Jaroenworaluck, A.; Stevens, R. A review of growth mechanism, structure and crystallinity of anodized TiO2 nanotubes. Mater. Sci. Eng. R Rep. 2013, 74, 377–406. [Google Scholar] [CrossRef]
- Katal, R.; Masudy-Panah, S.; Tanhaei, M.; Farahani, M.H.D.A.; Hu, J.Y. A review on the synthesis of the various types of anatase TiO2 facets and their applications for photocatalysis. Chem. Eng. J. 2020, 384, 123384. [Google Scholar] [CrossRef]
- Yadav, H.M.; Kim, J.S.; Pawar, S.H. Developments in photocatalytic antibacterial activity of nano TiO2: A review. Korean J. Chem. Eng. 2016, 33, 1989–1998. [Google Scholar] [CrossRef]
- Baraton, M.I. Nano-TiO2 for dye-sensitized solar cells. Recent Pat. Nanotechnol. 2012, 6, 10–15. [Google Scholar] [CrossRef] [PubMed]
- Yang, L.X.; Luo, S.L.; Cai, Q.Y.; Yao, S.Z. A review on TiO2 nanotube arrays: Fabrication, properties, and sensing applications. Chin. Sci. Bull. 2010, 55, 331–338. [Google Scholar] [CrossRef]
- Ge, S.H.; Sang, D.D.; Zou, L.R.; Yao, Y.; Zhou, C.D.; Fu, H.L.; Xi, H.Z.; Fan, J.C.; Meng, L.J.; Wang, C. A review on the progress of optoelectronic devices based on TiO2 thin films and nanomaterials. Nanomaterials 2023, 13, 1141. [Google Scholar] [CrossRef] [PubMed]
- Xu, Y.M.; Fang, X.M.; Xiong, J.A.; Zhang, Z.G. Hydrothermal transformation of titanate nanotubes into single-crystalline TiO2 nanomaterials with controlled phase composition and morphology. Mater. Res. Bull. 2010, 45, 799–804. [Google Scholar] [CrossRef]
- Chizhov, A.; Rumyantseva, M.; Gaskov, A. Light activation of nanocrystalline metal oxides for gas sensing: Principles, achievements, challenges. Nanomaterials 2021, 11, 892. [Google Scholar] [CrossRef] [PubMed]
- Ellmer, K. Past achievements and future challenges in the development of optically transparent electrodes. Nat. Photonics 2012, 6, 809–817. [Google Scholar] [CrossRef]
- Zhang, Z.G.; Wang, X.X.; Zhang, J.; Yu, M.; Zhang, J.C.; Zhang, H.D.; Long, Y.Z. Recent advances in 1D micro-and nanoscale indium oxide structures. J. Alloys Compd. 2018, 752, 359–375. [Google Scholar] [CrossRef]
- Hsin, C.L.; Wang, S.M. Structural, electrical, and optical properties of faceted In2O3 nanostructures. IEEE Trans. Nanotechnol. 2014, 13, 172–175. [Google Scholar] [CrossRef]
- Chen, K.; Zhang, Y.C.; Zhang, J.C.; Wang, X.; Yao, Y.X.; Ma, J.B.; Hao, Y. Ultrathin GaN film and AlGaN/GaN heterostructure grown on thick AlN buffer by MOCVD. Ceram. Int. 2022, 48, 36193–36200. [Google Scholar] [CrossRef]
- Wang, Y.C.; Li, H.L.; Yang, T.F.; Zou, Z.X.; Qi, Z.Y.; Ma, L.; Chen, J.Y. Space-confined physical vapour deposition of high quality ZnTe nanosheets for optoelectronic application. Mater. Lett. 2019, 238, 309–312. [Google Scholar] [CrossRef]
- Sun, H.; Li, W.J.; Jia, Z.X.; Zhang, Y.; Yin, L.Y.; Jie, W.Q.; Xu, Y.D. Effect of ACRT technology on the large size ZnTe crystals grown by solution method and corresponding terahertz properties. J. Inorg. Mater. 2023, 38, 310–315. [Google Scholar] [CrossRef]
- Gudmundsson, J.T. Physics and technology of magnetron sputtering discharges. Plasma Sources Sci. Technol. 2020, 29, 113001. [Google Scholar] [CrossRef]
- Sun, M.; Du, Y.; Hao, W.C.; Xu, H.Z.; Yu, Y.X.; Wang, T.M. Fabrication and wettability of ZnO nanorod array. J. Mater. Sci. Technol. 2009, 25, 53–57. [Google Scholar]
- Kim, C.Y.; Oh, H.B.; Ryu, H.; Yun, J.; Lee, W.J. ZnO nanorod growth by plasma-enhanced vapor phase transport with different growth durations. J. Vac. Sci. Technol. A 2014, 32, 051505. [Google Scholar] [CrossRef]
- Wu, Y.; Wang, D.; Li, Y. Understanding of the major reactions in solution synthesis of functional nanomaterials. Sci. China Mater. 2016, 59, 938–996. [Google Scholar] [CrossRef]
- Boles, M.A.; Engel, M.; Talapin, D.V. Self-assembly of colloidal nanocrystals: From intricate structures to functional materials. Chem. Rev. 2016, 116, 11220–11289. [Google Scholar] [CrossRef] [PubMed]
- Feng, B.C.; Hou, X.C.; Wang, T.L.; Lu, J.Q.; Jin, Y. Synthesis of Bicyclic Esters in a Continuous-Flow Microreactor. ChemistrySelect 2020, 5, 952–956. [Google Scholar]
- Li, C.L.; Iqbal, M.; Lin, J.J.; Luo, X.L.; Jiang, B.; Malgras, V.; Wu, K.C.W.; Kim, J.; Yamauchi, Y. Electrochemical deposition: An advanced approach for templated synthesis of nanoporous metal architectures. Acc. Chem. Res. 2018, 51, 1764–1773. [Google Scholar] [CrossRef] [PubMed]
- Cao, B.Q.; Li, Y.; Duan, G.T.; Cai, W.P. Growth of ZnO nanoneedle arrays with strong ultraviolet emissions by an electrochemical deposition method. Cryst. Growth Des. 2006, 6, 1091–1095. [Google Scholar] [CrossRef]
- Alimanesh, M.; Hassan, Z.; Zainal, N. Electrochemical growth of controlled tip shapes of ZnO nanorod arrays on silicon substrate and enhanced photoluminescence emission from nanopyramid arrays compared with flat-head nanorods. Opt. Mater. 2017, 72, 276–282. [Google Scholar] [CrossRef]
- Sriram, S.R.; Parne, S.R.; Pothukanuri, N.; Edla, D.R. Prospects of spray pyrolysis technique for gas sensor applications—A comprehensive review. J. Anal. Appl. Pyrolysis 2022, 164, 105527. [Google Scholar] [CrossRef]
- Liu, B.; Zeng, H.C. Hydrothermal synthesis of ZnO nanorods in the diameter regime of 50 nm. J. Am. Chem. Soc. 2003, 125, 4430–4431. [Google Scholar] [CrossRef] [PubMed]
- Fu, H.; Wang, L.W.; Zunger, A. Applicability of the k·p method to the electronic structure of quantum dots. Phys. Rev. B 1998, 57, 9971–9987. [Google Scholar] [CrossRef]
- Marquardt, O. Simulating the electronic properties of semiconductor nanostructures using multiband k·p models. Comput. Mater. Sci. 2021, 194, 110318. [Google Scholar] [CrossRef]
- Grundmann, M.; Stier, O.; Bimberg, D. InAs/GaAs pyramidal quantum dots: Strain distribution, optical phonons, and electronic structure. Phys. Rev. B 1995, 52, 11969–11981. [Google Scholar] [CrossRef] [PubMed]
- Kim, J.; Wang, L.W.; Zunger, A. Comparison of the electronic structure of InAs/GaAs pyramidal quantum dots with different facet orientations. Phys. Rev. B 1998, 57, R9408–R9411. [Google Scholar] [CrossRef]
- Cusack, M.A.; Briddon, P.R.; Jaros, M. Electronic structure, impurity binding energies, absorption spectra of InAs/GaAs quantum dots. Phys. B Condens. Matter 1998, 253, 10–27. [Google Scholar] [CrossRef]
- Wang, L.W.; Kim, J.; Zunger, A. Electronic structures of [110]-faceted self-assembled pyramidal InAs/GaAs quantum dots. Phys. Rev. B 1999, 59, 5678–5687. [Google Scholar] [CrossRef]
- Fonseca, L.R.C.; Jimenez, J.L.; Leburton, J.P.; Martin, R.M. Self-consistent electronic structure, coulomb interaction, and spin effects in self-assembled strained InAs-GaAs quantum dot structures. Phys. E Low-Dimens. Syst. Nanostruct. 1998, 2, 743–747. [Google Scholar] [CrossRef]
- Klenovský, P.; Schliwa, A.; Bimberg, D. Electronic states of (InGa)(AsSb)/GaAs/GaP quantum dots. Phys. Rev. B 2019, 100, 115424. [Google Scholar] [CrossRef]
- Stoleru, V.G.; Pal, D.; Towe, E. Self-assembled (In,Ga)As/GaAs quantum-dot nanostructures: Strain distribution and electronic structure. Phys. E Low-Dimens. Syst. Nanostruct. 2002, 15, 131–152. [Google Scholar] [CrossRef]
- Califano, M.; Harrison, P. Composition, volume, and aspect ratio dependence of the strain distribution, band lineups and electron effective masses in self-assembled pyramidal In1-xGaxAs/GaAs and SixGe1-x/Si quantum dots. J. Appl. Phys. 2002, 91, 389–398. [Google Scholar] [CrossRef][Green Version]
- Lee, J.; Chou, W.C.; Yang, C.S.; Jan, G.J. Eigen-energies and eigen-functions of symmetroidal quantum dots. Chin. J. Phys. 2004, 42, 102–115. [Google Scholar]
- Kapon, E.; Pelucchi, E.; Watanabe, S.; Malko, A.; Baier, M.H.; Leifer, K.; Dwir, B.; Michelini, F.; Dupertuis, M.A. Site-and energy-controlled pyramidal quantum dot heterostructures. Phys. E Low-Dimens. Syst. Nanostruct. 2004, 25, 288–297. [Google Scholar] [CrossRef]
- Nakaoka, T.; Saito, T.; Tatebayashi, J.; Arakawa, Y. Size, shape, and strain dependence of the g factor in self-assembled In(Ga)As quantum dots. Phys. Rev. B 2004, 70, 235337. [Google Scholar] [CrossRef]
- Tomić, S.; Sunderland, A.G.; Bush, I.J. Parallel multi-band k·p code for electronic structure of zinc blend semiconductor quantum dots. J. Mater. Chem. 2006, 16, 1963–1972. [Google Scholar] [CrossRef]
- Peng, X.G.; Schlamp, M.C.; Kadavanich, A.V.; Alivisatos, A.P. Epitaxial growth of highly luminescent CdSe/CdS core/shell nanocrystals with photostability and electronic accessibility. J. Am. Chem. Soc. 1997, 119, 7019–7029. [Google Scholar] [CrossRef]
- Park, S.H.; Hong, A.; Kim, J.H.; Yang, H.; Lee, K.; Jang, H.S. Highly bright yellow-green-emitting CuInS2 colloidal quantum dots with core/shell/shell architecture for white light-emitting diodes. ACS Appl. Mater. Interfaces 2015, 7, 6764–6771. [Google Scholar] [CrossRef] [PubMed]
- Dorfs, D.; Eychmüller, A. Multishell semiconductor nanocrystals. Z. Phys. Chem. 2006, 220, 1539–1552. [Google Scholar] [CrossRef]
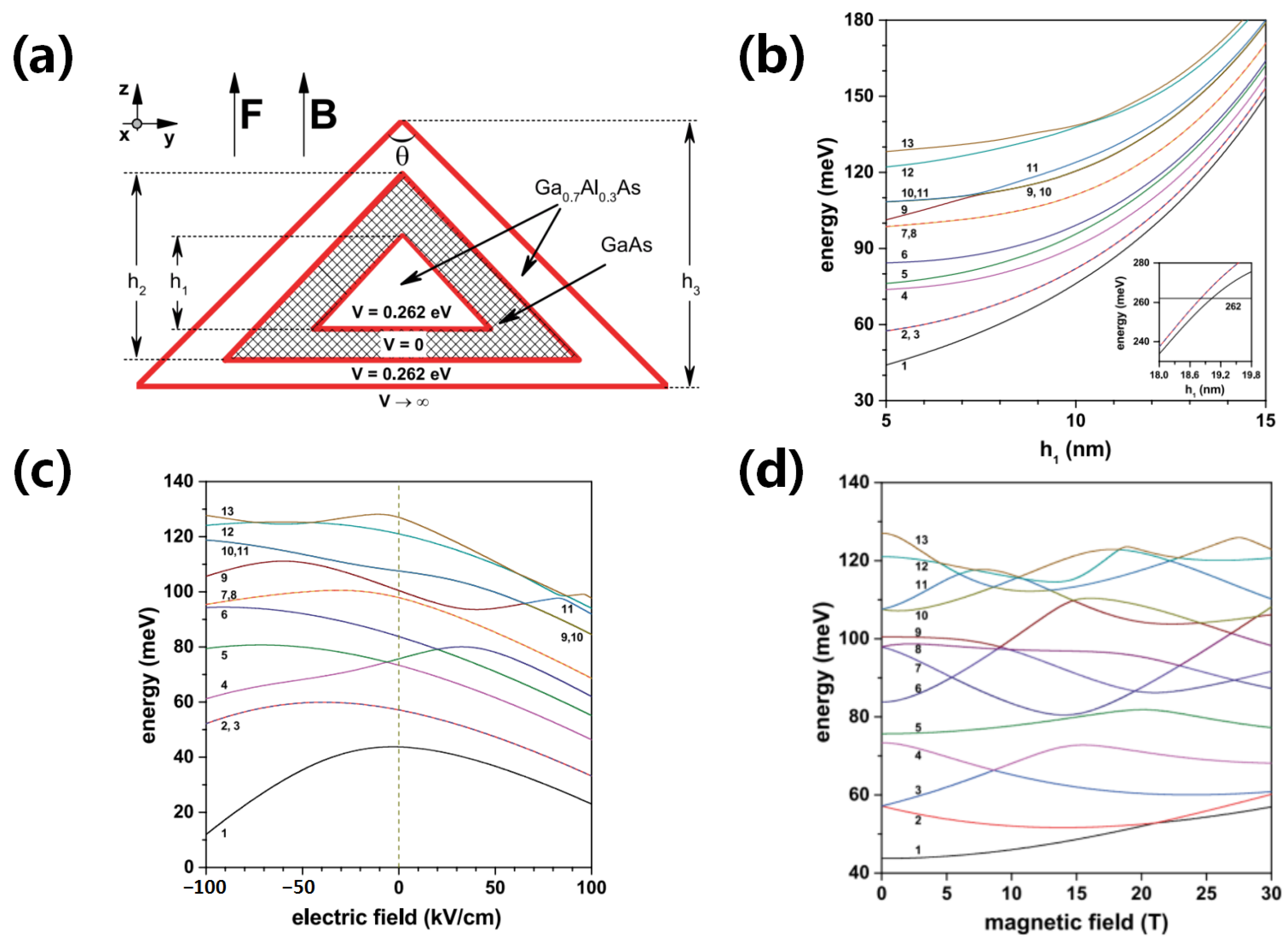
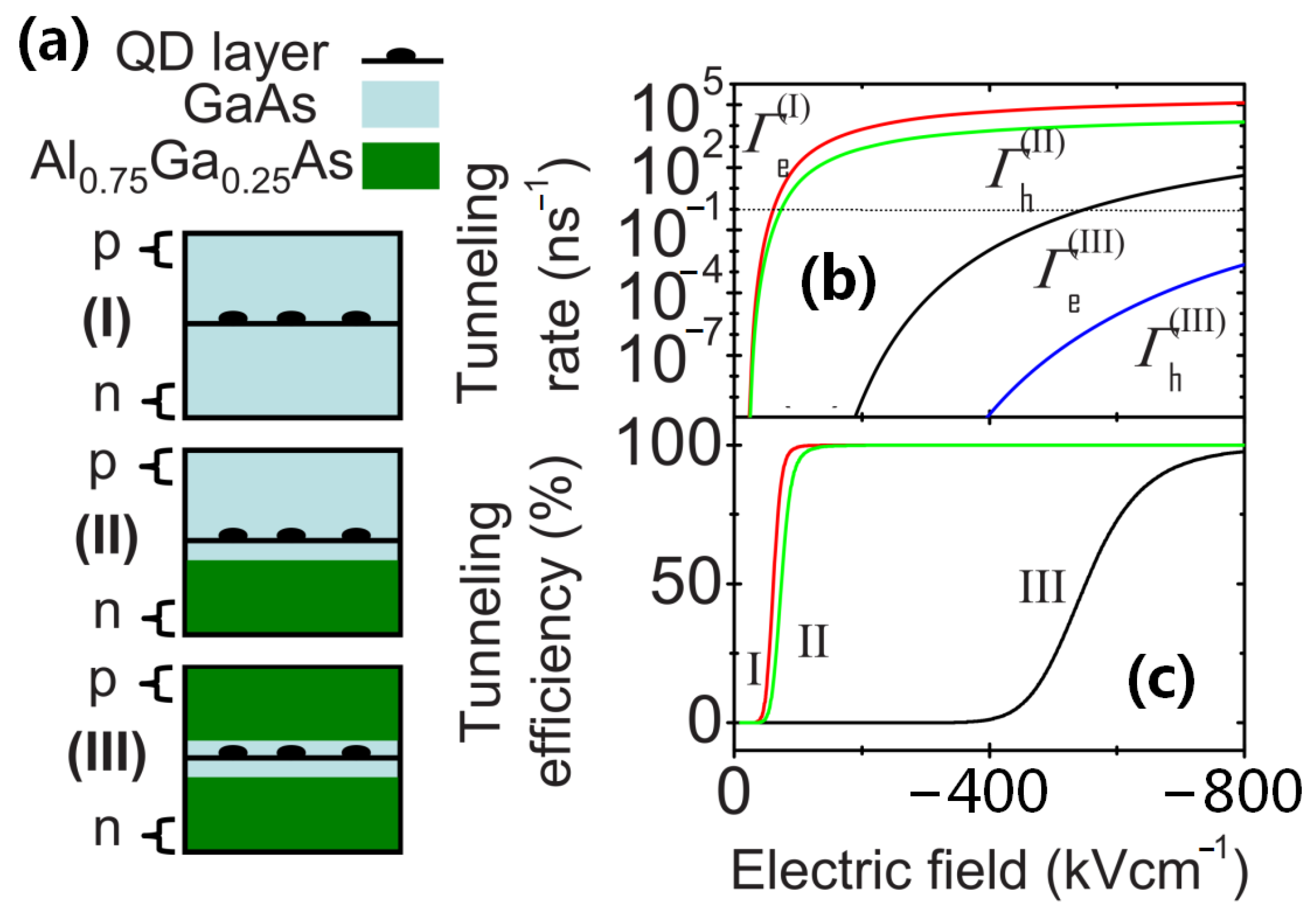
- Osorio, J.A.; Caicedo-Paredes, D.; Vinasco, J.A.; Morales, A.L.; Radu, A.; Restrepo, R.L.; Martínez-Orozco, J.C.; Tiutiunnyk, A.; Laroze, D.; Hieu, N.N.; et al. Pyramidal core-shell quantum dot under applied electric and magnetic fields. Sci. Rep. 2020, 10, 8961. [Google Scholar] [CrossRef] [PubMed]
- Jaskólski, W.; Zieliński, M.; Bryant, G.W. Electronic properties of quantum-dot molecules. Phys. E Low-Dimens. Syst. Nanostruct. 2003, 17, 40–41. [Google Scholar] [CrossRef]
- Markiewicz, M.; Voss, H. Electronic states in three dimensional quantum dot/wetting layer structures. In Proceedings of the International Conference on Computational Science and Its Applications, Glasgow, UK, 8–11 May 2006. [Google Scholar]
- Saito, T.; Schulman, J.N.; Arakawa, Y. Strain-energy distribution and electronic structure of InAs pyramidal quantum dots with uncovered surfaces: Tight-binding analysis. Phys. Rev. B 1998, 57, 13016–13019. [Google Scholar] [CrossRef]
- Williamson, A.J.; Zunger, A. InAs quantum dots: Predicted electronic structure of free-standing versus GaAs-embedded structures. Phys. Rev. B 1999, 59, 15819–15824. [Google Scholar] [CrossRef]
- Pryor, C. Geometry and material parameter dependence of InAs/GaAs quantum dot electronic structure. Phys. Rev. B 1999, 60, 2869–2874. [Google Scholar] [CrossRef]
- Lu, Y.; Chen, W. Sub-nanometre sized metal clusters: From synthetic challenges to the unique property discoveries. Chem. Soc. Rev. 2012, 41, 3594–3623. [Google Scholar] [CrossRef] [PubMed]
- Viswanath, A.K. From clusters to semiconductor nanostructures. J. Nanosci. Nanotechnol. 2014, 14, 1253–1281. [Google Scholar] [CrossRef] [PubMed]
- Philip, R.; Chantharasupawong, P.; Qian, H.F.; Jin, R.C.; Thomas, J. Evolution of nonlinear optical properties: From gold atomic clusters to plasmonic nanocrystals. Nano Lett. 2012, 12, 4661–4667. [Google Scholar]
- Pozhar, L.A.; Yeates, A.T.; Szmulowicz, F.; Mitchel, W.C. Structure, composition and optoelectronic properties of small pyramidal semiconductor quantum dots of Ga and In atoms with As. In Proceedings of the Symposium on Progress in Semiconductor Materials V held at the 2005 MRS Fall Meeting, Boston, MA, USA, 28 November–1 December 2005. [Google Scholar]
- Bennett, A.J.; Patel, R.B.; Skiba-Szymanska, J.; Nicoll, C.A.; Farrer, I.; Ritchie, D.A.; Shields, A.J. Giant Stark effect in the emission of single semiconductor quantum dots. Appl. Phys. Lett. 2010, 97, 031104. [Google Scholar] [CrossRef]
- Fry, P.W.; Itskevich, I.E.; Mowbray, D.J.; Skolnick, M.S.; Barker, J.; O’Reilly, E.P.; Hopkinson, M.; Al-Khafaji, M.; Cullis, A.G.; Hill, G.; et al. Quantum confined Stark effect and permanent dipole moment of InAs-GaAs self-assembled quantum dots. Phys. Status Solidi A 2000, 178, 269–275. [Google Scholar] [CrossRef]
- Sheng, W.; Leburton, J.P. 2D–3D transitions in the quantum Stark effect in self-assembled InAs/GaAs quantum dots. Phys. E Low-Dimens. Syst. Nanostruct. 2003, 17, 50–55. [Google Scholar] [CrossRef]
- Jin, P.; Li, C.M.; Zhang, Z.Y.; Liu, F.Q.; Chen, Y.H.; Ye, X.L.; Xu, B.; Wang, Z.G. Quantum-confined Stark effect and built-in dipole moment in self-assembled InAs/GaAs quantum dots. Appl. Phys. Lett. 2004, 85, 2791–2793. [Google Scholar] [CrossRef]
- Wang, Y.; Negro, D.; Djie, H.S.; Ooi, B.S.; Osinski, M.; Henneberger, F.; Arakawa, Y. Quantum-confined Stark effects in interdiffused semiconductor quantum dots. In Physics and Simulation of Optoelectronic Devices XV; SPIE: Bellingham, WA, USA, 2007; Volume 6468, pp. 364–373. [Google Scholar]
- Troncale, V.; Karlsson, K.F.; Kapon, E. Dynamic switching of hole character and single photon polarization using the quantum confined Stark effect in quantum dot-in-dot structures. Nanotechnology 2010, 21, 285202. [Google Scholar] [CrossRef] [PubMed]
- Rinke, P.; Winkelnkemper, M.; Qteish, A.; Bimberg, D.; Neugebauer, J.; Scheffler, M. Consistent set of band parameters for the group-III nitrides AlN, GaN, and InN. Phys. Rev. B 2008, 77, 075202. [Google Scholar] [CrossRef]
- Andreev, A.D.; O’Reilly, E.P. Theoretical analysis of the electronic structure of truncated-pyramidal GaN/AlN quantum dots. Phys. E Low-Dimens. Syst. Nanostruct. 2001, 10, 553–560. [Google Scholar] [CrossRef]
- Marquardt, O.; Mourad, D.; Schulz, S.; Hickel, T.; Czycholl, G.; Neugebauer, J. Comparison of atomistic and continuum theoretical approaches to determine electronic properties of GaN/AlN quantum dots. Phys. Rev. B 2008, 78, 235302. [Google Scholar] [CrossRef]
- Hong, W.; Park, S. Effects of the capping and the wetting layers on the electronic properties of self-assembled pyramidal CdTe/ZnTe quantum dots. J. Korean Phys. Soc. 2009, 55, 1607–1614. [Google Scholar] [CrossRef]
- Park, S.H.; Hong, W.P.; Kim, J. Quantum-confined Stark effects of strained CdTe/ZnTe pyramidal quantum dots. J. Korean Phys. Soc. 2011, 59, 2817–2820. [Google Scholar] [CrossRef]
- Betcke, M.; Voss, H. Numerical simulation of electronic properties of coupled quantum dots on wetting layers. Nanotechnology 2008, 19, 165204. [Google Scholar] [CrossRef] [PubMed]
- Yek, W.C.; Gopir, G.; Othman, A.P. Calculation of electronic properties of InAs/GaAs cubic, spherical and pyramidal quantum dots with finite difference method. Adv. Mater. Res. 2012, 501, 347–351. [Google Scholar] [CrossRef]
- Gong, L.; Shu, Y.C.; Xu, J.J.; Wang, Z.G. Numerical computation of pyramidal quantum dots with band non-parabolicity. Superlattices Microstruct. 2013, 61, 81–90. [Google Scholar] [CrossRef]
- Zhao, T.; Hwang, F.N.; Cai, X.C. Parallel two-level domain decomposition based Jacobi-Davidson algorithms for pyramidal quantum dot simulation. Comput. Phys. Commun. 2016, 204, 74–81. [Google Scholar] [CrossRef]
- Parto, E.; Rezaei, G.; Eslami, A.M.; Jalali, T. Finite difference time domain simulation of arbitrary shapes quantum dots. Eur. Phys. J. B 2019, 92, 246. [Google Scholar] [CrossRef]
- Nenashev, A.V.; Dvurechenskii, A.V. Variational method of energy level calculation in pyramidal quantum dots. J. Appl. Phys. 2020, 127, 154301. [Google Scholar] [CrossRef]
- Kockum, A.F.; Miranowicz, A.; De Liberato, S.; Savasta, S.; Nori, F. Ultrastrong coupling between light and matter. Nat. Rev. Phys. 2019, 1, 19–40. [Google Scholar] [CrossRef]
- Buschlinger, R.; Lorke, M.; Peschel, U. Light-matter interaction and lasing in semiconductor nanowires: A combined finite-difference time-domain and semiconductor Bloch equation approach. Phys. Rev. B 2015, 91, 045203. [Google Scholar] [CrossRef]
- Wheeler, D.A.; Zhang, J.Z. Exciton dynamics in semiconductor nanocrystals. Adv. Mater. 2013, 25, 2878–2896. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.Z.; Zhong, Y.Q.; Zi, J.Z.; Lian, Z.C. Type-I CdSe@CdS@ZnS heterostructured nanocrystals with long fluorescence lifetime. Materials 2023, 16, 7007. [Google Scholar] [CrossRef] [PubMed]
- Pietryga, J.M.; Park, Y.S.; Lim, J.H.; Fidler, A.F.; Bae, W.K.; Brovelli, S.; Klimov, V.I. Spectroscopic and device aspects of nanocrystal quantum dots. Chem. Rev. 2016, 116, 10513–10622. [Google Scholar] [CrossRef] [PubMed]
- Yakimov, A.I.; Stepina, N.P.; Dvurechenskii, A.V.; Nikiforov, A.I.; Nenashev, A.V. Excitons in charged Ge/Si type-II quantum dots. Semicond. Sci. Technol. 2000, 15, 1125–1130. [Google Scholar] [CrossRef]
- Yakimov, A.I.; Stepina, N.P.; Dvurechenskii, A.V.; Nikiforov, A.I.; Nenashev, A.V. Many-particle effects in excitonic transitions in type-II Ge/Si quantum dots. Phys. E Low-Dimens. Syst. Nanostruct. 2002, 13, 1026–1029. [Google Scholar] [CrossRef]
- Anitha, A.; Arulmozhi, M. Exciton binding energy in a pyramidal quantum dot. Pramana 2018, 90, 57. [Google Scholar] [CrossRef]
- Heitz, R.; Mukhametzhanov, I.; Stier, O.; Madhukar, A.; Bimberg, D. Phonon-assisted polar exciton–transitions in self-organized InAs/GaAs quantum dots. Phys. E Low-Dimens. Syst. Nanostruct. 2000, 7, 398–402. [Google Scholar] [CrossRef]
- Heitz, R.; Rodt, S.; Schliwa, A.; Bimberg, D. Shape-dependent properties of self-organized quantum dots: Few-particle states and exciton-phonon coupling. Phys. Status Solidi B 2003, 238, 273–280. [Google Scholar] [CrossRef]
- Schliwa, A.; Stier, O.; Heitz, R.; Grundmann, M.; Bimberg, D. Exciton level crossing in coupled InAs/GaAs quantum dot pairs. Phys. Status Solidi B 2001, 224, 405–408. [Google Scholar] [CrossRef]
- Pohl, U.W.; Seguin, R.; Rodt, S.; Schliwa, A.; Pötschke, K.; Bimberg, D. Control of structural and excitonic properties of self-organized InAs/GaAs quantum dots. Phys. E Low-Dimens. Syst. Nanostruct. 2006, 35, 285–292. [Google Scholar] [CrossRef]
- Kim, D.; Sheng, W.D.; Poole, P.J.; Dalacu, D.; Lefebvre, J.; Lapointe, J.; Reimer, M.E.; Aers, G.C.; Williams, R.L. Tuning the exciton g factor in single InAs/InP quantum dots. Phys. Rev. B 2009, 79, 045310. [Google Scholar] [CrossRef]
- Gawełczyk, M. Atypical dependence of excited exciton energy levels and electron-hole correlation on emission energy in pyramidal InP-based quantum dots. Sci. Rep. 2022, 12, 164. [Google Scholar] [CrossRef] [PubMed]
- Dufåker, D.; Mereni, L.O.; Karlsson, K.F.; Dimastrodonato, V.; Juska, G.; Holtz, P.O.; Pelucchi, E. Exciton-phonon coupling in single quantum dots with different barriers. Appl. Phys. Lett. 2011, 98, 251911. [Google Scholar] [CrossRef]
- Jarlov, C.; Gallo, P.; Calic, M.; Dwir, B.; Rudra, A.; Kapon, E. Bound and anti-bound biexciton in site-controlled pyramidal GaInAs/GaAs quantum dots. Appl. Phys. Lett. 2012, 101, 191101. [Google Scholar] [CrossRef]
- Dupertuis, M.A.; Karlsson, K.F.; Oberli, D.Y.; Pelucchi, E.; Rudra, A.; Holtz, P.O.; Kapon, E. Symmetries and the polarized optical spectra of exciton complexes in quantum dots. Phys. Rev. Lett. 2011, 107, 127403. [Google Scholar] [CrossRef] [PubMed]
- Steindl, P.; Sala, E.M.; Alén, B.; Marrón, D.F.; Bimberg, D.; Klenovsky, P. Optical response of (InGa)(AsSb)/GaAs quantum dots embedded in a GaP matrix. Phys. Rev. B 2019, 100, 195407. [Google Scholar] [CrossRef]
- Park, S.H.; Hong, W.P.; Kim, J.J.; Ahn, D. Effects of wetting layer on exciton binding energy of strained CdTe/ZnTe pyramidal quantum dots. Solid State Commun. 2015, 204, 61–63. [Google Scholar] [CrossRef]
- Hong, W.P.; Park, S.H. Optical characteristics of type-II ZnTe/ZnSe quantum dots for visible wavelength device applications. J. Korean Phys. Soc. 2022, 80, 1–4. [Google Scholar] [CrossRef]
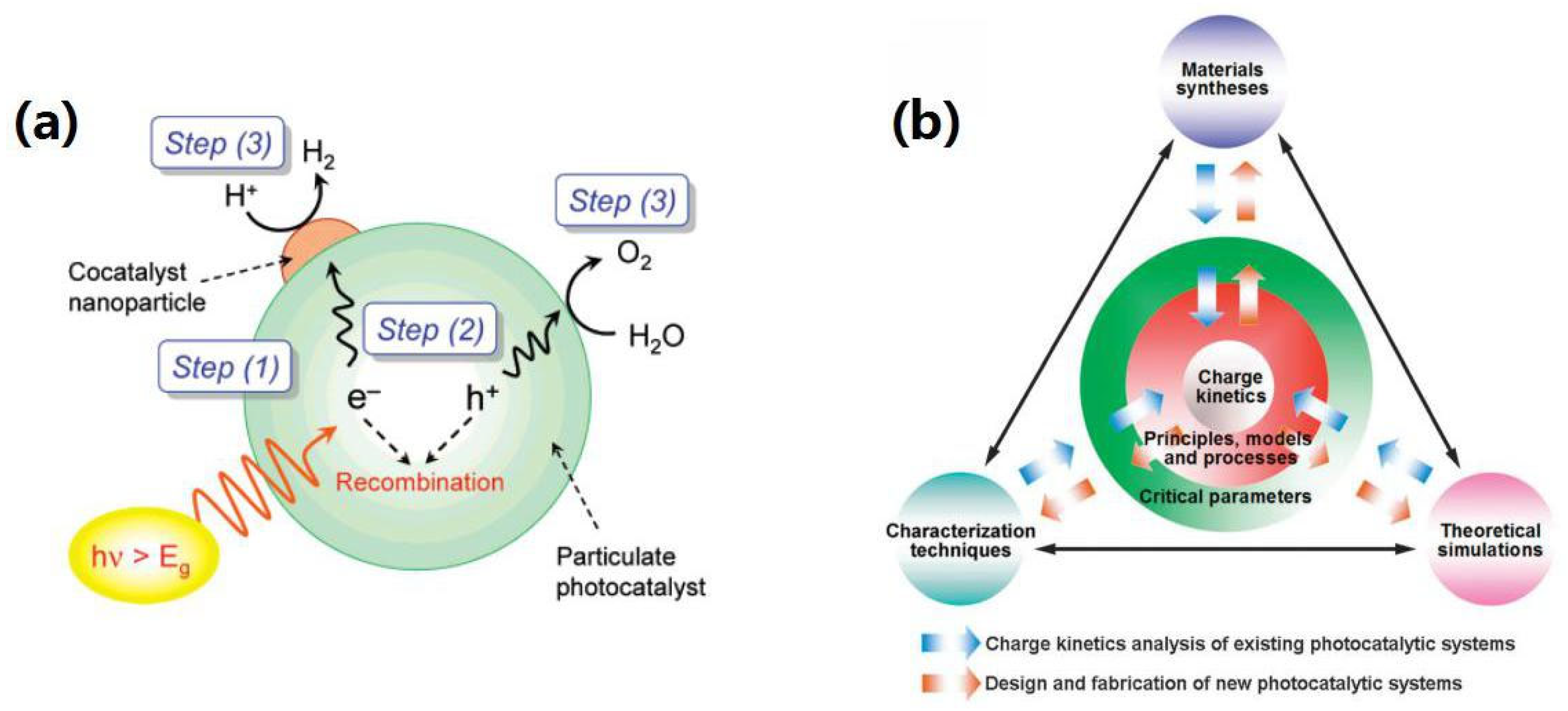
- Maeda, K.; Domen, K. New non-oxide photocatalysts designed for overall water splitting under visible light. J. Phys. Chem. C 2007, 111, 7851–7861. [Google Scholar] [CrossRef]
- Bai, S.; Jiang, J.; Zhang, Q.; Xiong, Y.J. Steering charge kinetics in photocatalysis: Intersection of materials syntheses, characterization techniques and theoretical simulations. Chem. Soc. Rev. 2015, 44, 2893–2939. [Google Scholar] [CrossRef]
- Ling, T.; Yan, D.Y.; Jiao, Y.; Wang, H.; Zheng, Y.; Zheng, X.L.; Mao, J.; Du, X.W.; Hu, Z.P.; Jaroniec, M.; et al. Engineering surface atomic structure of single-crystal cobalt (II) oxide nanorods for superior electrocatalysis. Nat. Commun. 2016, 7, 12876. [Google Scholar] [CrossRef] [PubMed]
- Pinkas, A.; Waiskopf, N.; Gigi, S.; Naor, T.; Layani, A.; Banin, U. Morphology effect on zinc oxide quantum photoinitiators for radical polymerization. Nanoscale 2021, 13, 7152–7160. [Google Scholar] [CrossRef] [PubMed]
- Zhao, H.G.; Liu, G.J.; Vidal, F.; Wang, Y.Q.; Vomiero, A. Colloidal thick-shell pyramidal quantum dots for efficient hydrogen production. Nano Energy 2018, 53, 116–124. [Google Scholar] [CrossRef]
- Tan, C.L.; Mohseni, H. Emerging technologies for high performance infrared detectors. Nanophotonics 2018, 7, 169–197. [Google Scholar] [CrossRef]
- Wang, F.; Wang, Z.X.; Yin, L.; Cheng, R.Q.; Wang, J.J.; Wen, Y.; Shifa, T.A.; Wang, F.M.; Zhang, Y.; Zhan, X.Y.; et al. 2D library beyond graphene and transition metal dichalcogenides: A focus on photodetection. Chem. Soc. Rev. 2018, 47, 6296–6341. [Google Scholar] [CrossRef] [PubMed]
- Wang, S.Y.; Li, Z.J.; Zhou, X.S.; Zhao, C.; Zu, X.T.; Fu, Y.Q. Advances in nanostructured acoustic wave technologies for ultraviolet sensing. Nanosci. Nanotechnol. Lett. 2015, 7, 169–192. [Google Scholar] [CrossRef]
- Huo, N.; Konstantatos, G. Recent progress and future prospects of 2D-based photodetectors. Adv. Mater. 2018, 30, 1801164. [Google Scholar] [CrossRef] [PubMed]
- Vandervelde, T.E.; Krishna, S. Progress and prospects for quantum dots in a well infrared photodetectors. J. Nanosci. Nanotechnol. 2010, 10, 1450–1460. [Google Scholar] [CrossRef] [PubMed]
- Bin Hafiz, S.; Scimeca, M.; Sahu, A.; Ko, D.K. Colloidal quantum dots for thermal infrared sensing and imaging. Nano Converg. 2019, 6, 7. [Google Scholar] [CrossRef] [PubMed]
- Martyniuk, P.; Rogalski, A. Quantum-dot infrared photodetectors: Status and outlook. Prog. Quantum Electron. 2008, 32, 89–120. [Google Scholar] [CrossRef]
- Reiter, D.E.; Kuhn, T.; Axt, V.M. Distinctive characteristics of carrier-phonon interactions in optically driven semiconductor quantum dots. Adv. Phys. X 2019, 4, 1655478. [Google Scholar] [CrossRef]
- Melnychuk, C.; Guyot-Sionnest, P. Multicarrier dynamics in quantum dots. Chem. Rev. 2021, 121, 2325–2372. [Google Scholar] [CrossRef] [PubMed]
- Geller, M. Nonequilibrium carrier dynamics in self-assembled quantum dots. Appl. Phys. Rev. 2019, 6, 031306. [Google Scholar] [CrossRef]
- Kershaw, S.V.; Rogach, A.L. Carrier multiplication mechanisms and competing processes in colloidal semiconductor nanostructures. Materials 2017, 10, 1095. [Google Scholar] [CrossRef] [PubMed]
- Boucaud, P.; Le Thanh, V.; Sauvage, S.; Brunhes, T.; Fortuna, F.; Debarre, D.; Bouchier, D. Intraband absorption in Ge/Si self-assembled quantum dots. Appl. Phys. Lett. 1999, 74, 401–403. [Google Scholar] [CrossRef][Green Version]
- Zhang, J.Z.; Galbraith, I. Intraband absorption for InAs/GaAs quantum dot infrared photodetectors. Appl. Phys. Lett. 2004, 84, 1934–1936. [Google Scholar] [CrossRef]
- Chaganti, V.R.; Apalkov, V. Intraband optical transition in InGaAs/GaAs pyramidal quantum dots. J. Appl. Phys. 2013, 113, 064310. [Google Scholar] [CrossRef]
- Mohan, A.; Nevou, L.; Gallo, P.; Dwir, B.; Rudra, A.; Kapon, E.; Faist, J. Photocurrent spectroscopy of site-controlled pyramidal quantum dots. Appl. Phys. Lett. 2012, 101, 031110. [Google Scholar] [CrossRef]
- Amtout, A.; Raghavan, S.; Rotella, P.; von Winckel, G.; Stintz, A.; Krishna, S. Theoretical modeling and experimental characterization of InAs/InGaAs quantum dots in a well detector. J. Appl. Phys. 2004, 96, 3782–3786. [Google Scholar] [CrossRef]
- Naser, M.A.; Deen, M.J.; Thompson, D.A. Spectral function of InAs/InGaAs quantum dots in a well detector using Green’s function. J. Appl. Phys. 2006, 100, 093102. [Google Scholar] [CrossRef]
- Fazlalipour, H.; Asgari, A.; Darvish, G. Modeling of pyramidal shape quantum dot infrared photodetector: The effects of temperature and quantum dot size. J. Nanophotonics 2018, 12, 026006. [Google Scholar] [CrossRef]
- Yang, X.F.; Chen, X.S.; Lu, W.; Fu, Y. Effects of shape and strain distribution of quantum dots on optical transition in the quantum dot infrared photodetectors. Nanoscale Res. Lett. 2008, 3, 534–539. [Google Scholar] [CrossRef] [PubMed]
- Mir, A.; Ahmadi, V. Design and analysis of a new structure of InAs/GaAs QDIP for 8-12 μm infrared windows with low dark current. J. Mod. Opt. 2009, 56, 1704–1712. [Google Scholar] [CrossRef]
- Lv, Z.S.; Liu, L.; Zhangyang, X.Y.; Lu, F.F.; Tian, J. Enhanced absorptive characteristics of GaN nanowires for ultraviolet (UV) photocathode. Appl. Phys. A 2020, 126, 152. [Google Scholar] [CrossRef]
- Lv, Z.S.; Liu, L.; Zhangyang, X.Y.; Lu, F.F.; Tian, J. Optical absorption enhancement in GaN nanowire arrays with hexagonal periodic arrangement for UV photocathode. J. Nanopart. Res. 2021, 23, 24. [Google Scholar] [CrossRef]
- Liu, Y.H.; Li, B.W.; Ma, C.Q.; Huang, F.; Feng, G.T.; Chen, H.Z.; Hou, J.H.; Yan, L.P.; Wei, Q.Y.; Luo, Q.; et al. Recent progress in organic solar cells (Part I material science). Sci. China Chem. 2022, 65, 224–265. [Google Scholar] [CrossRef]
- Huang, K.; Liu, J.L.; Yuan, J.J.; Zhao, W.C.; Zhao, K.; Zhou, Z.H. Perovskite-quantum dot hybrid solar cells: A multi-win strategy for high performance and stability. J. Mater. Chem. A 2023, 11, 4487–4509. [Google Scholar] [CrossRef]
- Rashidinejad, E.; Naderi, A.A. Analytical study of electro-elastic fields in quantum nanostructure solar cells: The inter-nanostructure couplings and geometrical effects. Acta Mech. 2018, 229, 3089–3106. [Google Scholar] [CrossRef]
- Jaouane, M.; Fakkahi, A.; Ed-Dahmouny, A.; El-Bakkari, K.; Tuzemen, A.T.; Arraoui, R.; Sali, A.; Ungan, F. Modeling and simulation of the influence of quantum dots density on solar cell properties. Eur. Phys. J. Plus 2023, 138, 148. [Google Scholar] [CrossRef]
- Sun, Y.; Liu, L.; Lv, Z.S.; Zhangyang, X.Y.; Lu, F.F.; Tian, J. Theoretical study on optoelectronic properties of GaAs nanostructures. Mater. Sci. Semicond. Process. 2021, 122, 105498. [Google Scholar] [CrossRef]
- Dash, R.; Jena, S. Finite element analysis of the effect of wetting layer on the electronic eigenstates of InP/InGaP pyramidal quantum dots solar cell. Mater. Today Proc. 2021, 39, 2015–2021. [Google Scholar] [CrossRef]
- Arefinia, Z.; Asgari, A. The intrinsic losses of quantum dot intermediate band solar cells. In Proceedings of the 17th International Conference on Numerical Simulation of Optoelectronic Devices NUSOD, Santa Barbara, CA, USA, 24–28 July 2017. [Google Scholar]
- Kim, M.R.; Xu, Z.H.; Chen, G.Z.; Ma, D.L. Semiconductor and metallic core-shell nanostructures: Synthesis and applications in solar cells and catalysis. Chem.-A Eur. J. 2014, 20, 11256–11275. [Google Scholar] [CrossRef] [PubMed]
- Jing, Q.; Meng, X.Y.; Wang, C.; Zhao, H.G. Exciton dynamic in pyramidal InP/ZnSe quantum dots for luminescent solar concentrators. ACS Appl. Nano Mater. 2023, 6, 4449–4454. [Google Scholar] [CrossRef]
- Chandrakala, V.; Bachan, N.; Kumar, P.N.; Pugazhendhi, K.; Praveen, B.; Tenkyong, T.; Shyla, J.M. Investigation of the morphology based properties of multidimensional titanate nanostructures for application as proficient photo anodes. Mater. Today Proc. 2019, 9, 217–236. [Google Scholar] [CrossRef]
- Huang, F.F.; Guo, B.; Li, S.; Fu, J.C.; Zhang, L.; Lin, G.H.; Yang, Q.R.; Cheng, Q.J. Plasma-produced ZnO nanorod arrays as an antireflective layer in c-Si solar cells. J. Mater. Sci. 2019, 54, 4011–4023. [Google Scholar] [CrossRef]
- Wang, L.; Chen, K.C.; Tong, H.; Wang, K.; Tao, L.; Zhang, Y.X.; Zhou, X.F. Inverted pyramid Er3+ and Yb3+ co-doped TiO2 nanorod arrays based perovskite solar cell: Infrared response and improved current density. Ceram. Int. 2020, 46, 12073–12079. [Google Scholar] [CrossRef]
- Agnihotri, S.K.; Prashant, D.V.; Samajdar, D.P. A modified hexagonal pyramidal InP nanowire solar cell structure for efficiency improvement: Geometrical optimisation and device analysis. Sol. Energy 2022, 237, 293–300. [Google Scholar] [CrossRef]
- Mishra, S.M.; Dey, S.; Singha, T.; Mandal, S.; Dehury, A.K.; Chaudhary, Y.S.; Satpati, B. Enhanced optical properties and dark I-V characteristics of silicon nanowire-carbon quantum dots heterostructures. Mater. Res. Bull. 2023, 164, 112262. [Google Scholar] [CrossRef]
- Owen, J.; Brus, L. Chemical synthesis and luminescence applications of colloidal semiconductor quantum dots. J. Am. Chem. Soc. 2017, 139, 10939–10943. [Google Scholar] [CrossRef] [PubMed]
- Zak, P.P.; Lapina, V.A.; Pavich, T.A.; Trofimov, A.V.; Trofimova, N.N.; Tsaplev, Y.B. Luminescent materials for modern light sources. Russ. Chem. Rev. 2017, 86, 831–844. [Google Scholar] [CrossRef]
- Cui, Z.Y.; Abdurahman, A.; Ai, X.; Li, F. Stable luminescent radicals and radical-based LEDs with doublet emission. CCS Chem. 2020, 2, 1129–1145. [Google Scholar] [CrossRef]
- Dai, X.L.; Deng, Y.Z.; Peng, X.G.; Jin, Y.Z. Quantum-dot light-emitting diodes for large-area displays: Towards the dawn of commercialization. Adv. Mater. 2017, 29, 1607022. [Google Scholar] [CrossRef] [PubMed]
- Ryu, J.E.; Park, S.; Park, Y.; Ryu, S.W.; Hwang, K.; Jang, H.W. Technological breakthroughs in chip fabrication, transfer, and color conversion for high-performance micro-LED displays. Adv. Mater. 2023, 35, 2204947. [Google Scholar] [CrossRef] [PubMed]
- Demir, H.V.; Nizamoglu, S.; Erdem, T.; Mutlugun, E.; Gaponik, N.; Eychmüller, A. Quantum dot integrated LEDs using photonic and excitonic color conversion. Nano Today 2011, 6, 632–647. [Google Scholar] [CrossRef]
- Ji, W.Y.; Tian, Y.; Zeng, Q.H.; Qu, S.N.; Zhang, L.G.; Jing, P.T.; Wang, J.; Zhao, J.L. Efficient quantum dot light-emitting diodes by controlling the carrier accumulation and exciton formation. ACS Appl. Mater. Interfaces 2014, 6, 14001–14007. [Google Scholar] [CrossRef] [PubMed]
- Deng, Y.; Lin, X.; Fang, W.; Di, D.; Wang, L.; Friend, R.H.; Peng, X.; Jin, Y. Deciphering exciton-generation processes in quantum-dot electroluminescence. Nat. Commun. 2020, 11, 2309. [Google Scholar] [CrossRef] [PubMed]
- Baier, M.H.; Constantin, C.; Pelucchi, E.; Kapon, E. Electroluminescence from a single pyramidal quantum dot in a light-emitting diode. Appl. Phys. Lett. 2004, 84, 1967–1969. [Google Scholar] [CrossRef]
- Chung, T.H.; Juska, G.; Moroni, S.T.; Pescaglini, A.; Gocalinska, A.; Pelucchi, E. Selective carrier injection into patterned arrays of pyramidal quantum dots for entangled photon light-emitting diodes. Nat. Photonics 2016, 10, 782–787. [Google Scholar] [CrossRef]
- Chua, S.J.; Soh, C.B.; Liu, W.; Teng, J.H.; Ang, S.S.; Teo, S.L. Quantum dots excited InGaN/GaN phosphor-free white LEDs. Phys. Status Solidi C 2008, 5, 2189–2191. [Google Scholar] [CrossRef]
- Rokhi, M.M.; Asgari, A. Power improvement in ridge bent waveguide superluminescent light-emitting diodes based on GaN quantum dots. Phys. Scr. 2021, 96, 125520. [Google Scholar] [CrossRef]
- Liang, Y.; Li, C.; Huang, Y.Z.; Zhang, Q. Plasmonic nanolasers in on-chip light sources: Prospects and challenges. ACS Nano 2020, 14, 14375–14390. [Google Scholar] [CrossRef] [PubMed]
- Norman, J.C.; Mirin, R.P.; Bowers, J.E. Quantum dot lasers—History and future prospects. J. Vac. Sci. Technol. A 2021, 39, 020802. [Google Scholar] [CrossRef]
- Bhattacharya, P.; Mi, Z. Quantum-dot optoelectronic devices. Proc. IEEE 2007, 95, 1723–1740. [Google Scholar] [CrossRef]
- Asryan, L.V.; Grundmann, M.; Ledentsov, N.N.; Stier, O.; Suris, R.A.; Bimberg, D. Effect of excited-state transitions on the threshold characteristics of a quantum dot laser. IEEE J. Quantum Electron. 2001, 37, 418–425. [Google Scholar] [CrossRef]
- Qasaimeh, O. Effect of inhomogeneous line broadening on gain and differential gain of quantum dot lasers. IEEE Trans. Electron Devices 2003, 50, 1575–1581. [Google Scholar] [CrossRef]
- Vukmirovic, N.; Ikonic, Z.; Jovanovic, V.D.; Indjin, D.; Harrison, P. Optically pumped intersublevel midinfrared lasers based on InAs-GaAs quantum dots. IEEE J. Quantum Electron. 2005, 41, 1361–1368. [Google Scholar] [CrossRef]
- Bhattacharya, P.; Kamath, K.K.; Singh, J.; Klotzkin, D.; Phillips, J.; Jiang, H.T.; Chervela, N.; Norris, T.B.; Sosnowski, T.; Laskar, J.; et al. In(Ga)As/GaAs self-organized quantum dot lasers: DC and small-signal modulation properties. IEEE Trans. Electron Devices 1999, 46, 871–883. [Google Scholar] [CrossRef]
- Borji, M.A.; Rajaei, E. Energy level engineering in InxGa1–xAs/GaAs quantum dots applicable to quantum dot-lasers by changing the stoichiometric percentage. J. Nanoelectron. Optoelectron. 2016, 11, 315–322. [Google Scholar] [CrossRef]
- Northup, T.E.; Blatt, R. Quantum information transfer using photons. Nat. Photonics 2014, 8, 356–363. [Google Scholar] [CrossRef]
- Basset, F.B.; Valeri, M.; Neuwirth, J.; Polino, E.; Rota, M.B.; Poderini, D.; Pardo, C.; Rodari, G.; Roccia, E.; da Silva, S.F.C.; et al. Daylight entanglement-based quantum key distribution with a quantum dot source. Quantum Sci. Technol. 2023, 8, 025002. [Google Scholar] [CrossRef]
- Tomm, N.; Javadi, A.; Antoniadis, N.O.; Najer, D.; Löbl, M.C.; Korsch, A.R.; Schott, R.; Valentin, S.R.; Wieck, A.D.; Ludwig, A.; et al. A bright and fast source of coherent single photons. Nat. Nanotechnol. 2021, 16, 399–403. [Google Scholar] [CrossRef] [PubMed]
- Coste, N.; Fioretto, D.A.; Belabas, N.; Wein, S.C.; Hilaire, P.; Frantzeskakis, R.; Gundin, M.; Goes, B.; Somaschi, N.; Morassi, M.; et al. High-rate entanglement between a semiconductor spin and indistinguishable photons. Nat. Photonics 2023, 17, 582–587. [Google Scholar]
- Baier, M.H.; Pelucchi, E.; Kapon, E.; Varoutsis, S.; Gallart, M.; Robert-Philip, I.; Abram, I. Single photon emission from site-controlled pyramidal quantum dots. Appl. Phys. Lett. 2004, 84, 648–650. [Google Scholar] [CrossRef]
- Tsuruoka, T.; Ohizumi, Y.; Ushioda, S. Light intensity imaging of single InAs quantum dots using scanning tunneling microscope. Appl. Phys. Lett. 2003, 82, 3257–3259. [Google Scholar] [CrossRef]
- Winden, A.; Mikulics, M.; Grützmacher, D.; Hardtdegen, H. Vertically integrated (Ga,In)N nanostructures for future single photon emitters operating in the telecommunication wavelength range. Nanotechnology 2013, 24, 405302. [Google Scholar] [CrossRef]
- Pérez-Solórzano, V.; Gröning, A.; Jetter, M.; Riemann, T.; Christen, J. Near-red emission from site-controlled pyramidal InGaN quantum dots. Appl. Phys. Lett. 2005, 87, 163121. [Google Scholar] [CrossRef]
- Stachurski, J.; Tamariz, S.; Callsen, G.; Butté, R.; Grandjean, N. Single photon emission and recombination dynamics in self-assembled GaN/AlN quantum dots. Light Sci. Appl. 2022, 11, 114. [Google Scholar] [CrossRef] [PubMed]
- Kim, S.; Gong, S.H.; Cho, J.H.; Cho, Y.H. Unidirectional emission of a site-controlled single quantum dot from a pyramidal structure. Nano Lett. 2016, 16, 6117–6123. [Google Scholar] [CrossRef] [PubMed]
- Zhang, H.W.; Guo, Z.Y.; Zhang, J.; Li, Y.; Hou, Y.F. Fiber-based unidirectional emission with enhanced extraction efficiency of a single quantum dot from an optimized structure. Opt. Commun. 2020, 455, 124480. [Google Scholar] [CrossRef]
- Gershoni, D. Pyramidal quantum dots. Nat. Photonics 2010, 4, 271–272. [Google Scholar] [CrossRef]
- Juska, G.; Chung, T.H.; Moroni, S.T.; Gocalinska, A.; Pelucchi, E. A site-controlled quantum dot light-emitting diode of polarization-entangled photons, violating Bell’s inequality. In Proceedings of the Conference on Lasers and Electro-Optics (CLEO), San Jose, CA, USA, 5–10 June 2016. [Google Scholar]
- Huang, J.H.; Liu, W.; Miranda, A.; Dwir, B.; Rudra, A.; Kapon, E.; Wong, C.W. ontrollable pure dephasing pathways in single site-controlled pyramidal quantum dot–nanocavity system. In Proceedings of the Conference on Lasers and Electro-Optics (CLEO), San Jose, CA, USA, 9–14 May 2021. [Google Scholar]
- Juska, G.; Dimastrodonato, V.; Mereni, L.O.; Gocalinska, A.; Pelucchi, E. Towards quantum-dot arrays of entangled photon emitters. Nat. Photonics 2013, 7, 527–531. [Google Scholar] [CrossRef]
- Juska, G.; Murray, E.; Dimastrodonato, V.; Chung, T.H.; Moroni, S.T.; Gocalinska, A.; Pelucchi, E. Conditions for entangled photon emission from (111)B site-controlled pyramidal quantum dots. J. Appl. Phys. 2015, 117, 134302. [Google Scholar] [CrossRef]










| Shape | Material | Size | Maximum PLQY | Reference |
|---|---|---|---|---|
| Sphere | Si | 3–4 nm | 85% | [62] |
| CdTe/CdS/ZnS | 10 nm | 73% | [63] | |
| CdSe/CdS/ZnS | 3.6 nm | 37.3% | [64] | |
| PbTe | 2.6–8.3 nm | 52% | [65] | |
| PbSe/CdSe | 6.2 nm | 70% | [66] | |
| InP/ZnS | 10 nm | 66.5% | [67] | |
| InAs/ZnSe | 2.8 nm | 20% | [68] | |
| Plate | CsPbBr3 | 27–33 nm | 65% | [69] |
| CH3NH3PbBr3 | 4–10 nm | 80% | [70] | |
| Cu-doped CdSe | 34–40 nm | 95% | [71] | |
| CdSe/CdS@CdS | 12.7–16.1 nm | 90% | [72] | |
| Pyramid | CdSe/CdS | 5.5–6 nm | 94% | [73] |
| AgInSe2 | 7–12 nm | 21% | [74] | |
| CdSe | 4.9–5.7 nm | 41% | [75] | |
| CuInS2/ZnS | 4.8–7.2 nm | 76% | [76] |
| Preparation Method | Material | Reference |
|---|---|---|
| MOCVD | AlGaAs/GaAs | [103] |
| InGaAs/AlGaAs | [104] | |
| InGaAs/GaAs | [105] | |
| CdS/ZnS | [115] | |
| InGaAsN | [112] | |
| InAs/InP | [108] | |
| In0.25Ga0.75As/GaAs/GaP | [106] | |
| (InGa)(AsSb)/GaAs/GaP | [107] | |
| MBE | SiGe/Si | [94] |
| InAs/GaAs | [100] | |
| PbS | [121] | |
| PbSe | [120] | |
| CdSe/ZnSe | [116] | |
| GaN | [111] | |
| GaAs/AlGaAs | [132] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jiang, A.; Xing, S.; Lin, H.; Chen, Q.; Li, M. Role of Pyramidal Low-Dimensional Semiconductors in Advancing the Field of Optoelectronics. Photonics 2024, 11, 370. https://doi.org/10.3390/photonics11040370
Jiang A, Xing S, Lin H, Chen Q, Li M. Role of Pyramidal Low-Dimensional Semiconductors in Advancing the Field of Optoelectronics. Photonics. 2024; 11(4):370. https://doi.org/10.3390/photonics11040370
Chicago/Turabian StyleJiang, Ao, Shibo Xing, Haowei Lin, Qing Chen, and Mingxuan Li. 2024. "Role of Pyramidal Low-Dimensional Semiconductors in Advancing the Field of Optoelectronics" Photonics 11, no. 4: 370. https://doi.org/10.3390/photonics11040370
APA StyleJiang, A., Xing, S., Lin, H., Chen, Q., & Li, M. (2024). Role of Pyramidal Low-Dimensional Semiconductors in Advancing the Field of Optoelectronics. Photonics, 11(4), 370. https://doi.org/10.3390/photonics11040370







