Strain Monitoring and Installation Adjustment of Satellite–Rocket Connection Device Based on Distributed Optical Fibers
Abstract
1. Introduction
2. Methods
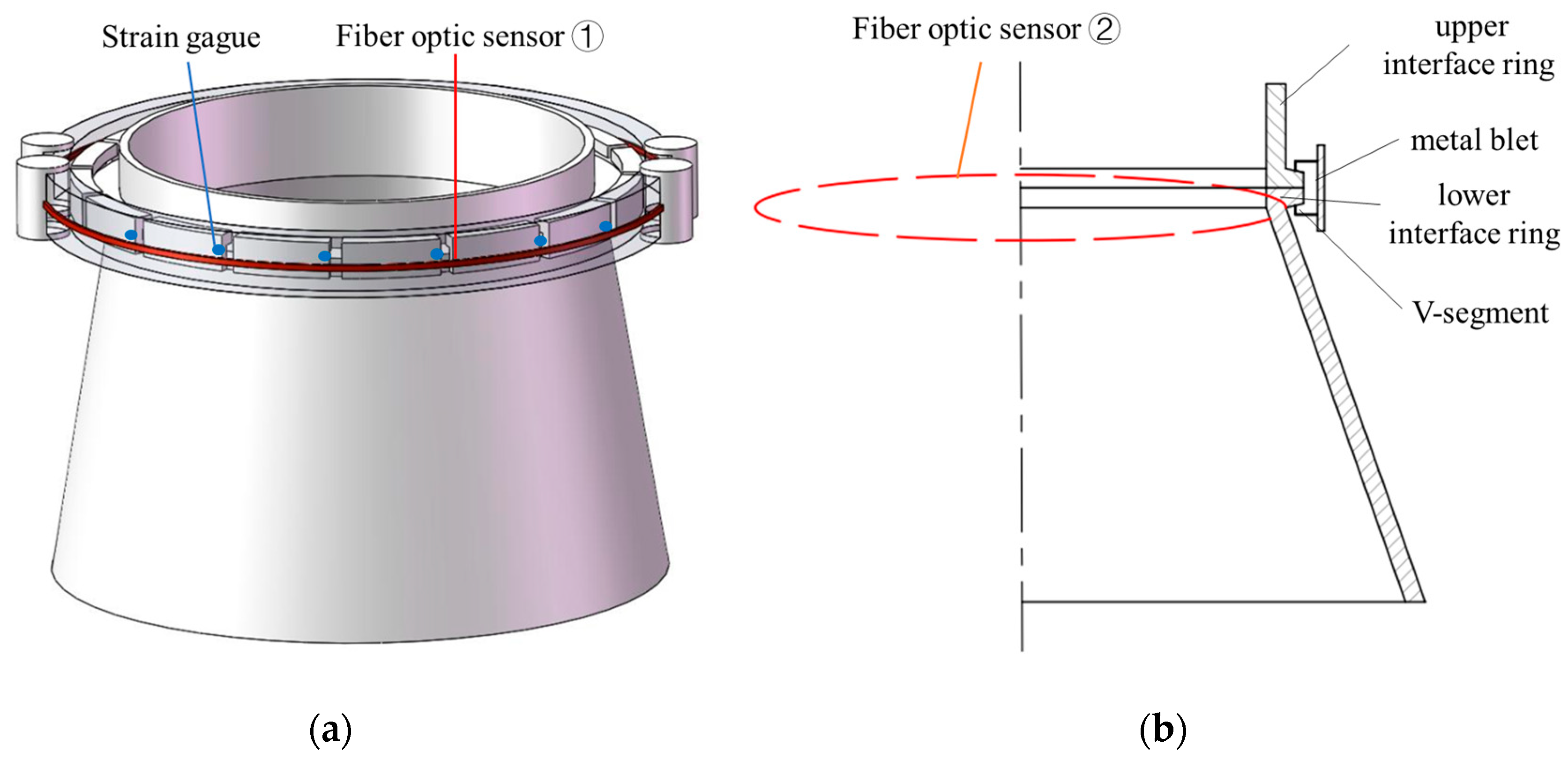
2.1. Description of the Clamp Band System
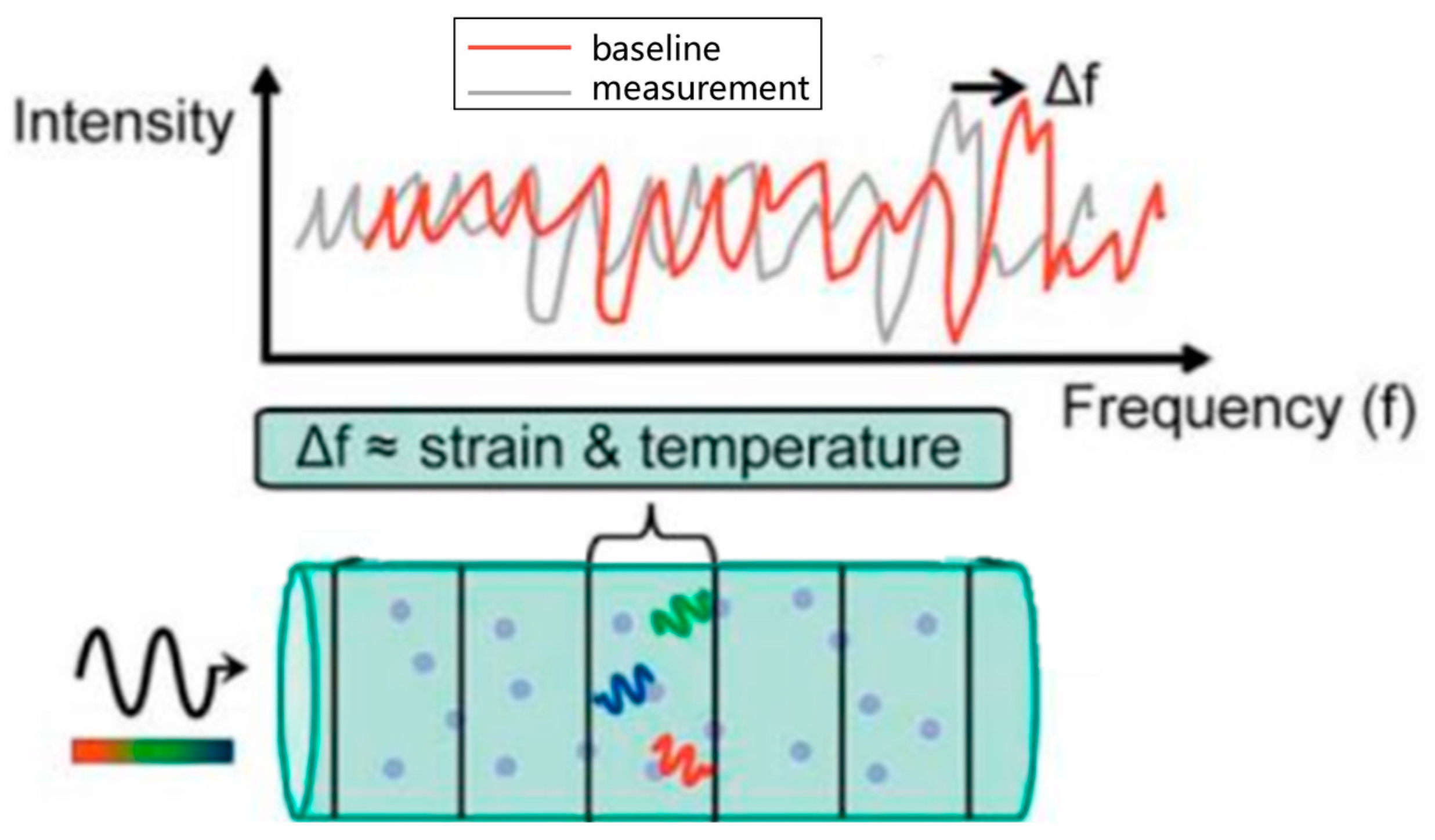
2.2. The Foundation of Fiber Optic Monitoring
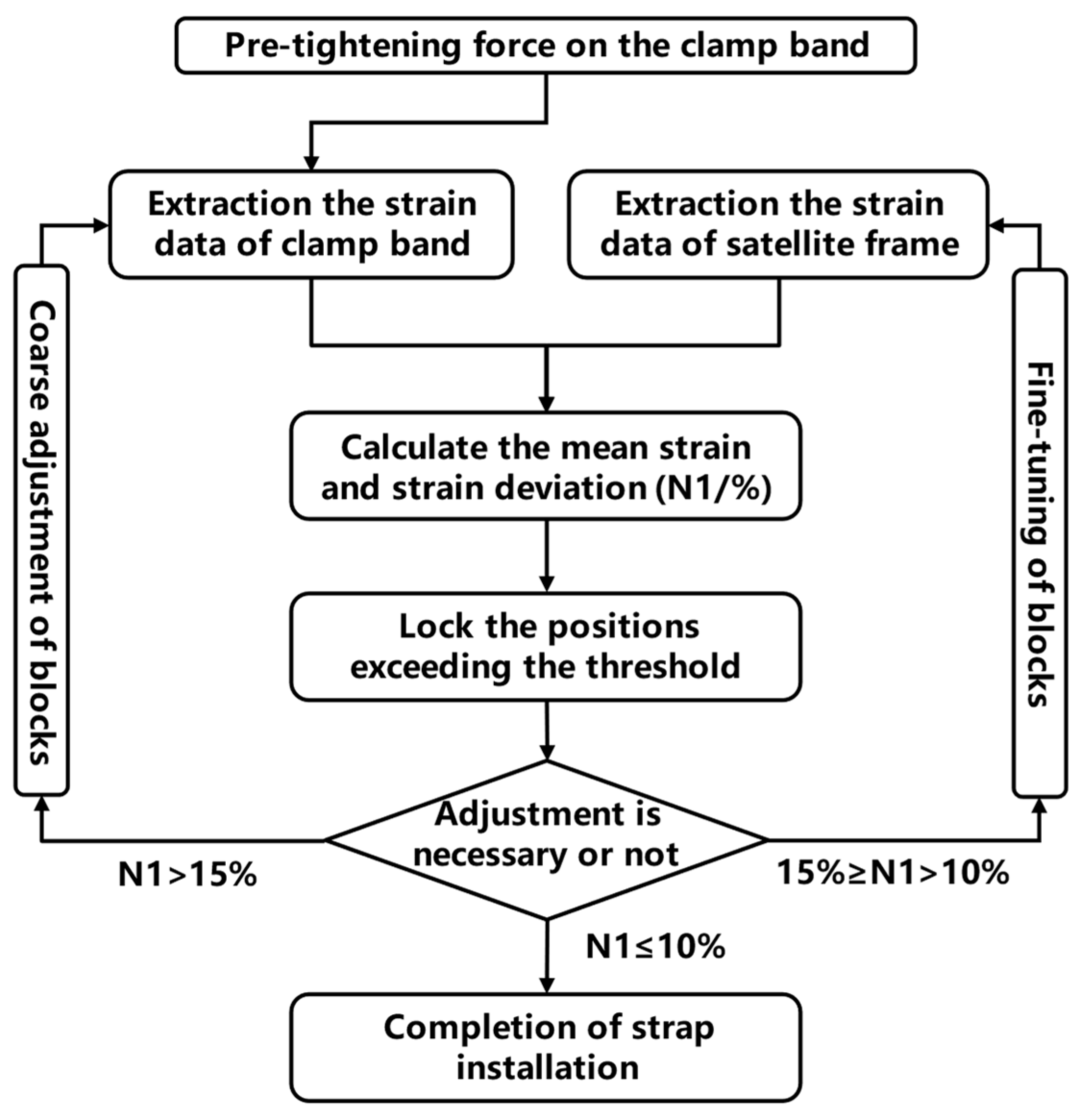
2.3. Strain Monitoring Method for Uniform Preload
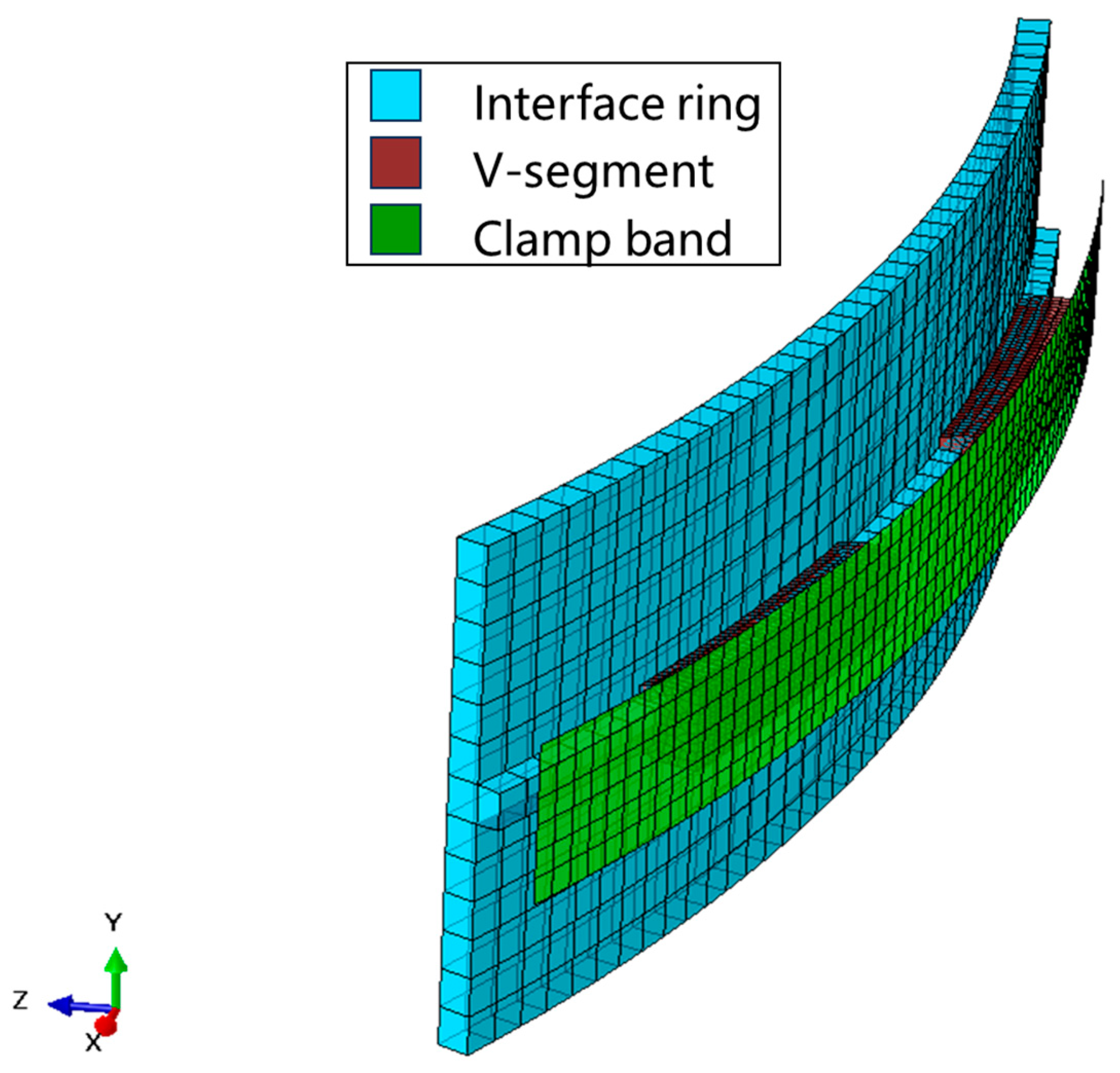
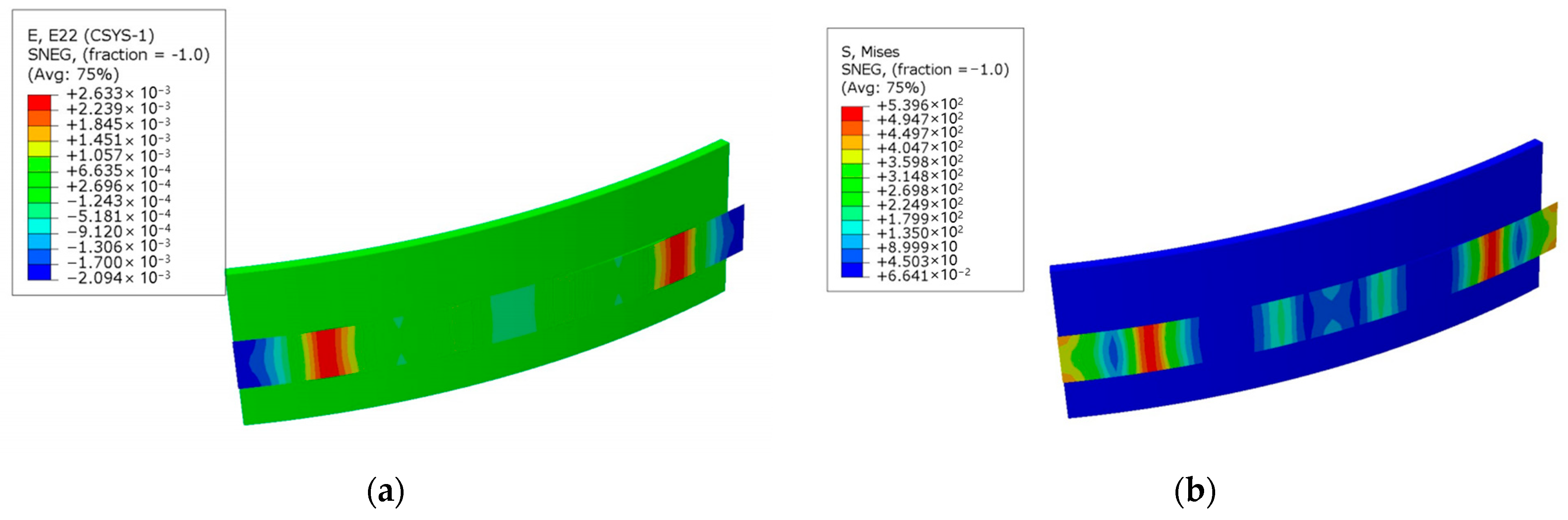
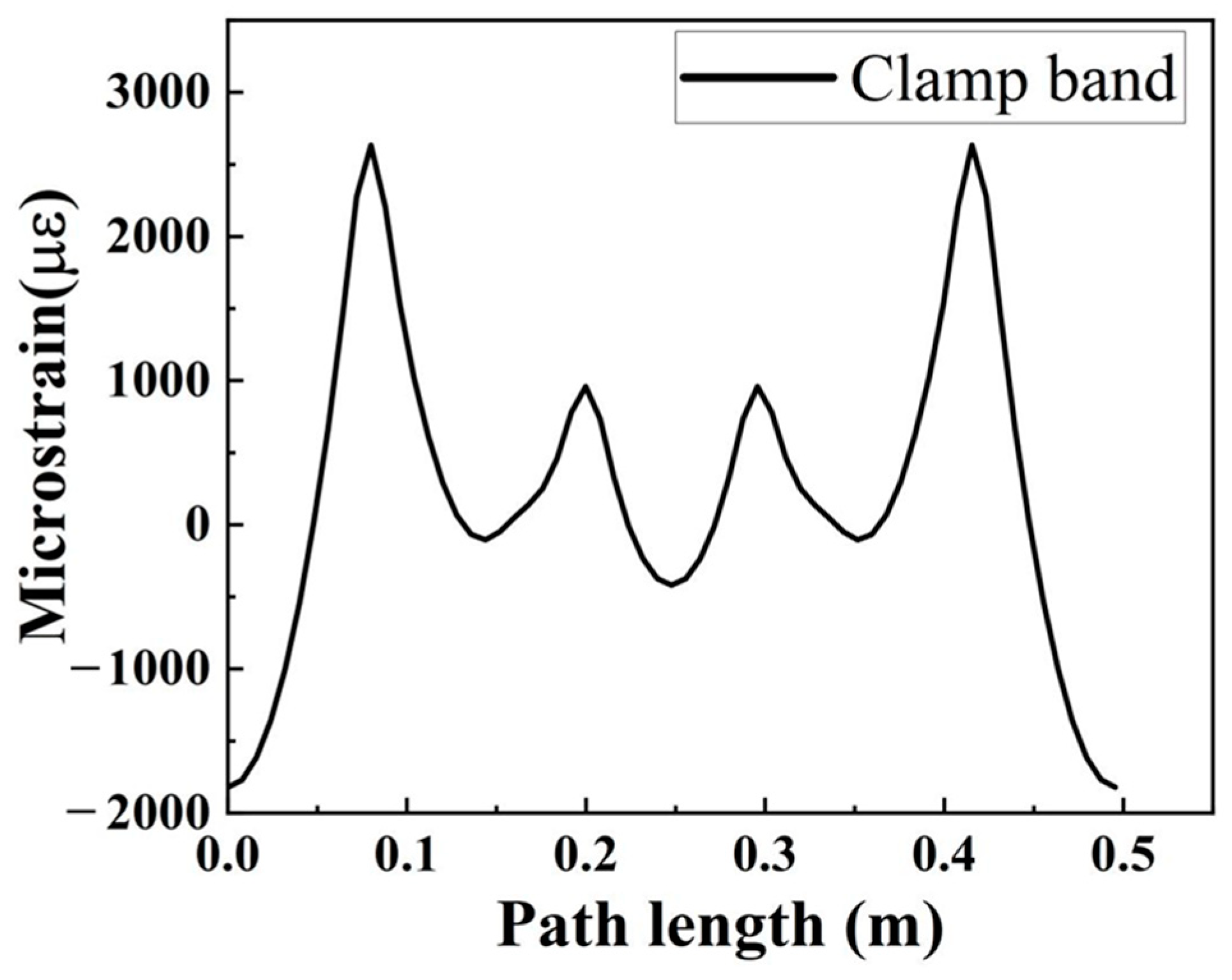
3. Finite Element Model
4. Experiment Test
4.1. Test Setup
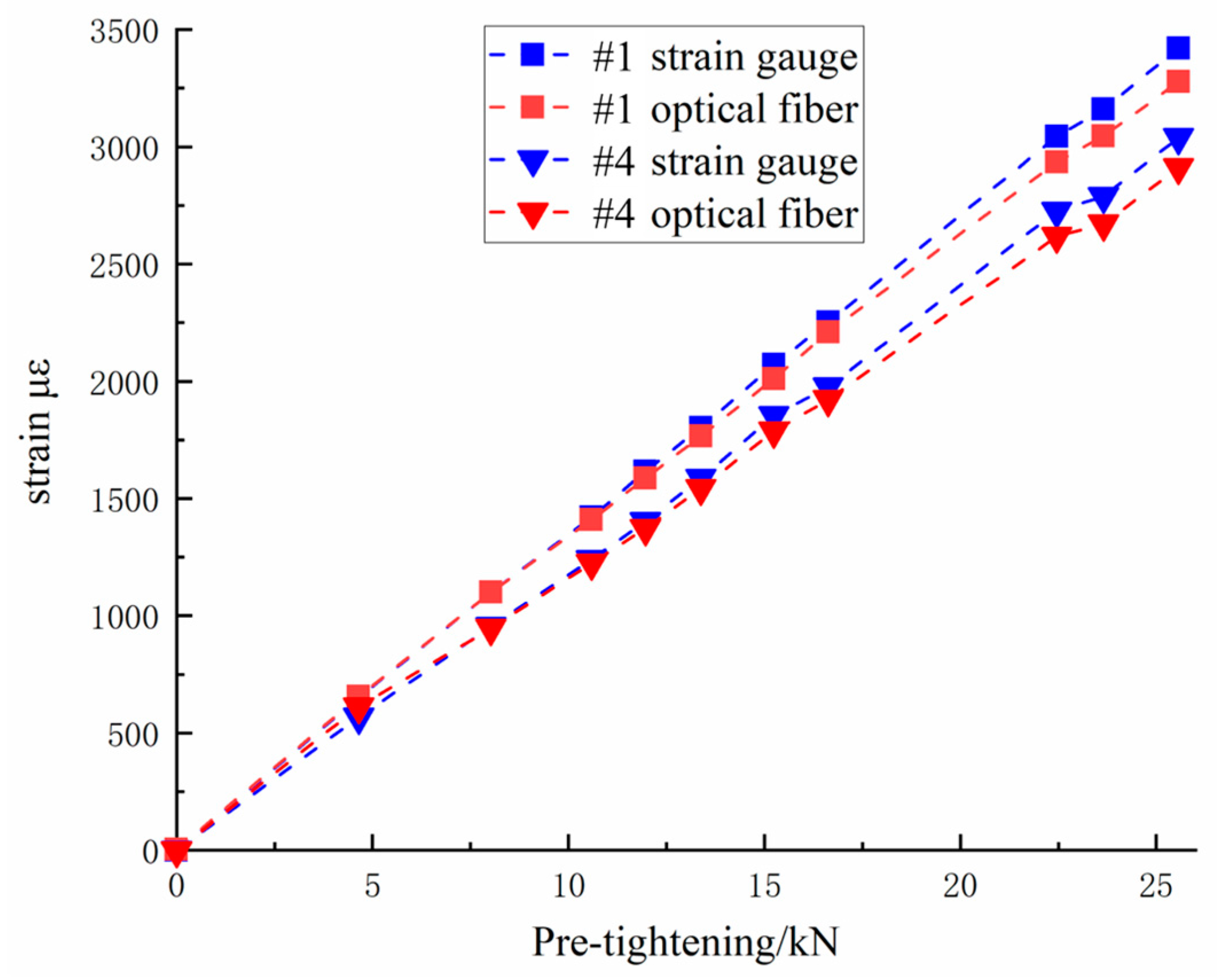
4.2. Effect of Preload
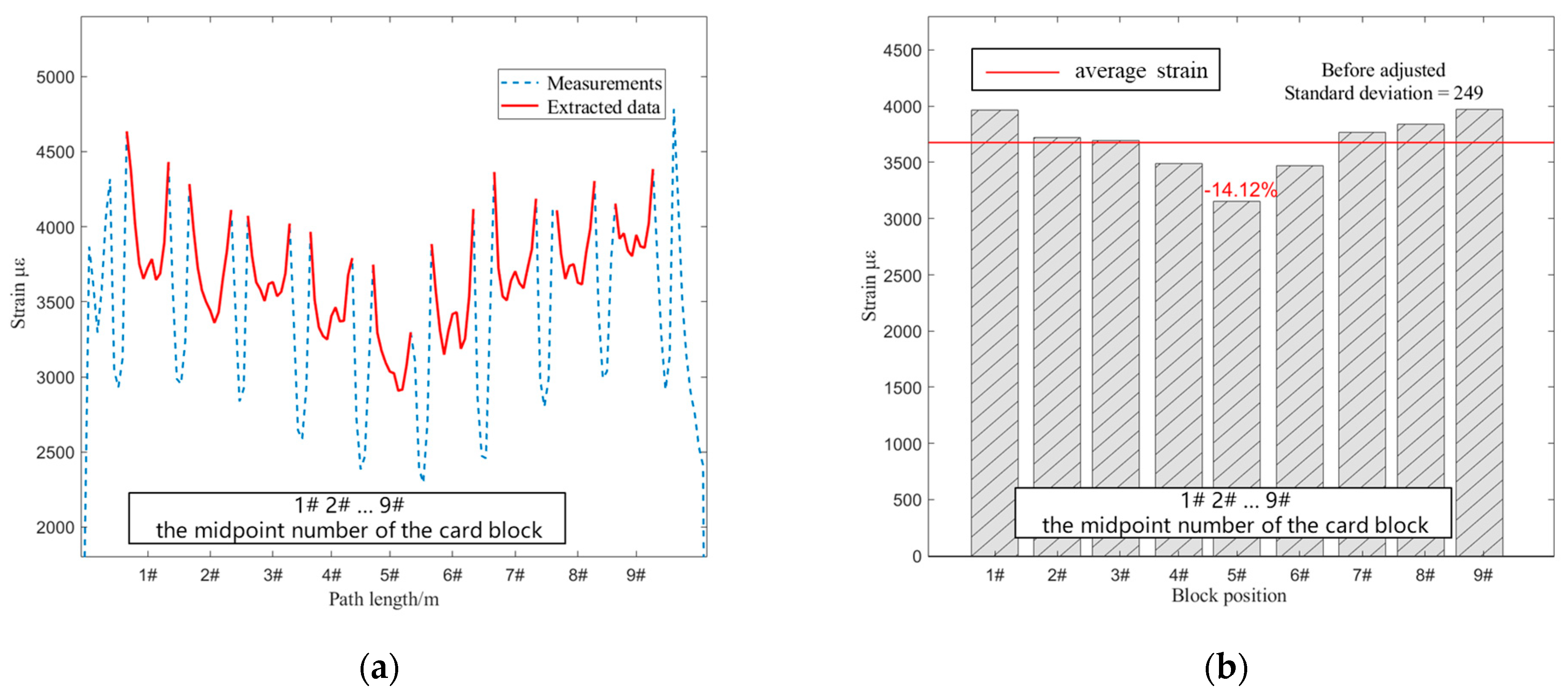
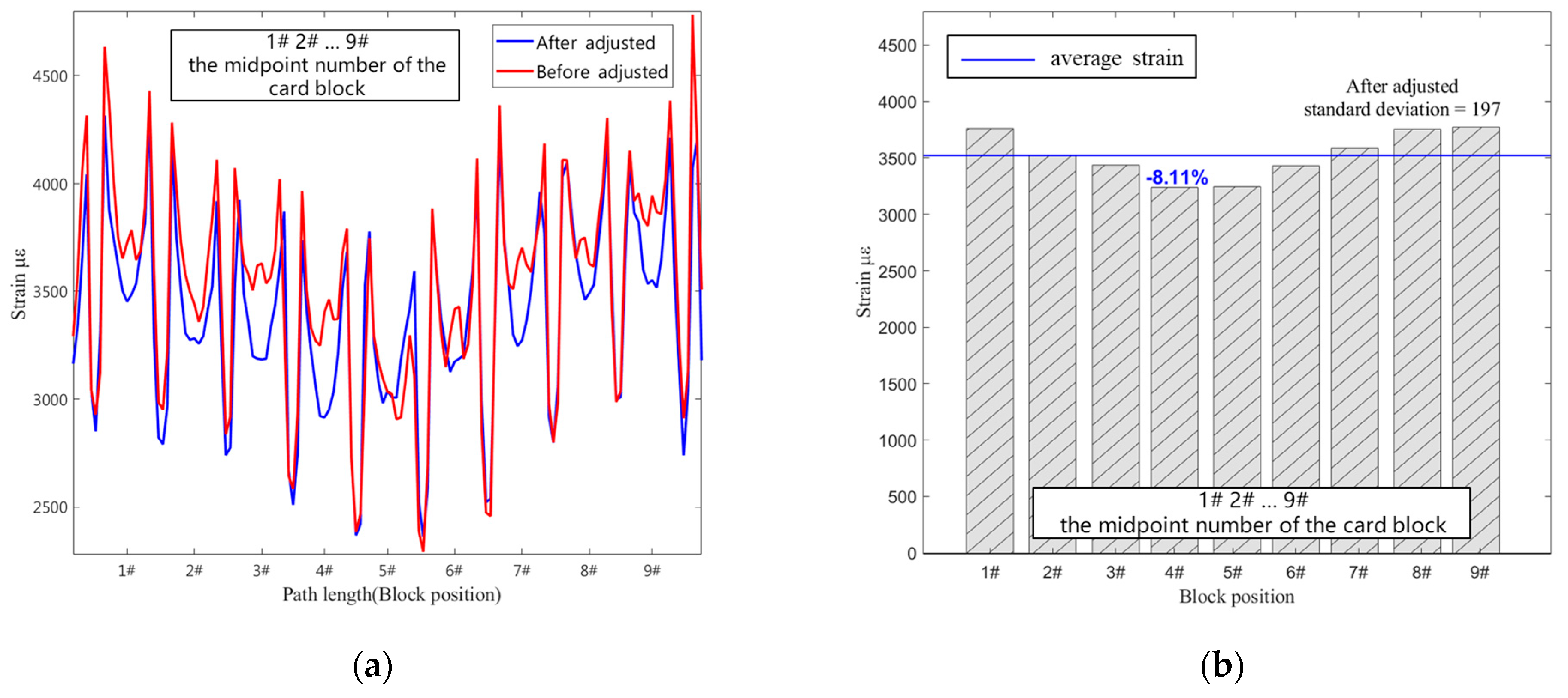
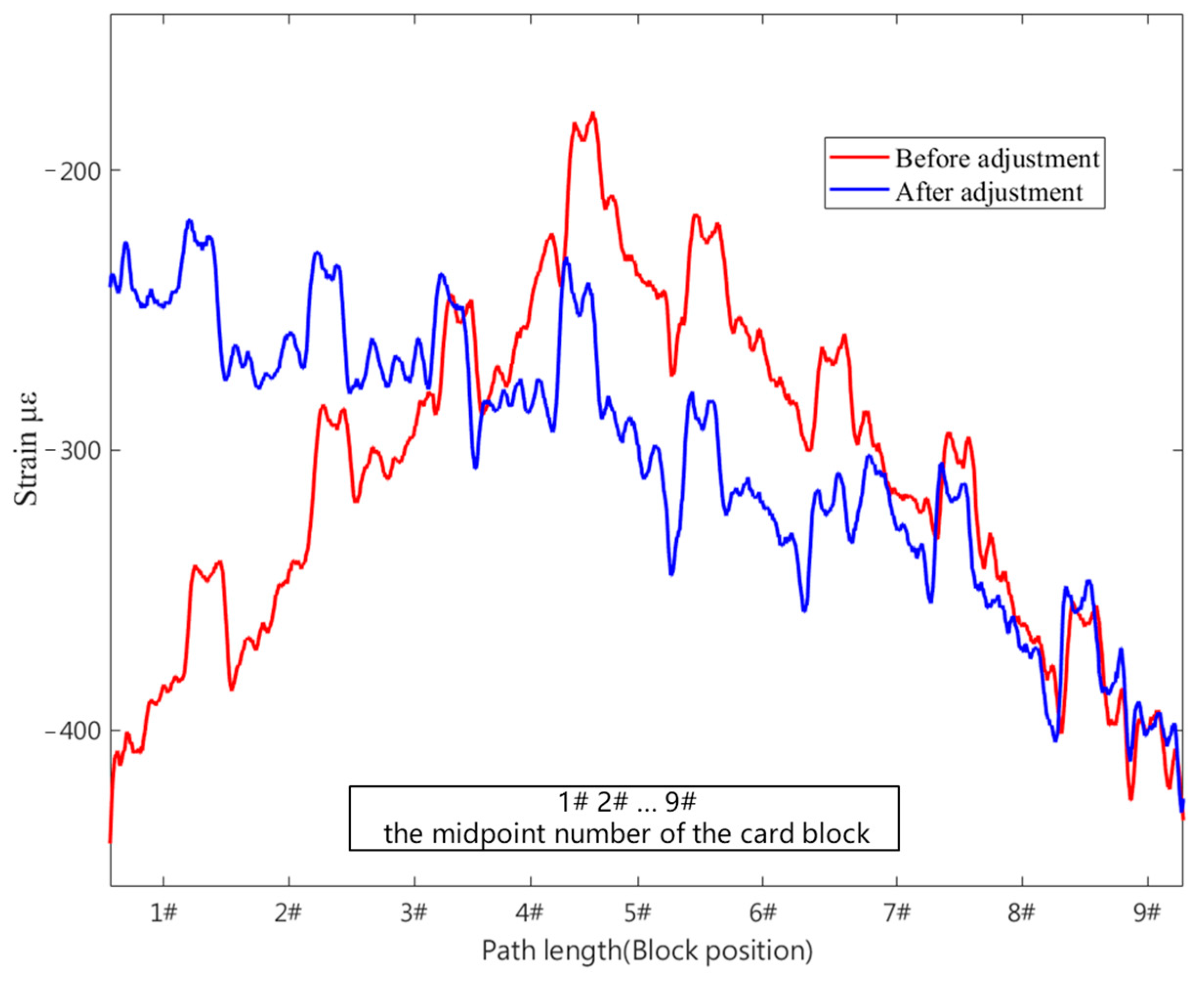
4.3. Adjustment Result
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- NASA. Marman clamp system design guidelines. In Guideline; Goddard Space Flight Centre: Greenbelt, MD, USA, 2000. [Google Scholar]
- Ali, A.; Barrans, S.; Ghiradi, F. Empirical method for determining axial load distribution in joints formed by V-band clamps. In Proceedings of the 2017 8th International Conference on Mechanical and Aerospace Engineering (ICMAE), Prague, Czech Republic, 22–25 July 2017; pp. 11–15. [Google Scholar]
- Di Tolla, R.; Ernst, M. Nonlinear finite element evaluation of marman clamp structural capability. In Proceedings of the AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Hilton Head, SC, USA, 18–20 April 1994; pp. 320–330. [Google Scholar]
- Jacob, I.; Vinay, K.; Nicholas, E. Techniques for finite element analysis of clamp band systems. In Proceedings of the 50th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Palm Springs, CA, USA, 4–7 May 2009; pp. 1–9. [Google Scholar]
- Ladisa, P.; Santonico, G. Non linear finite element verification approach for marman fitting in space AIT operations. SAE Int. J. Aerosp. 2014, 7, 292–308. [Google Scholar] [CrossRef]
- Guo, X.; Zhu, S.; Liu, G.; Yu, B.; Zhang, Q.; Lei, Y. Nonlinear Static Analysis and Structural Optimization of Rigid Clamp Band Connection Device between Launch Vehicle and Satellite. J. Northwestern Polytech. Univ. 2020, 38, 1122–1128. [Google Scholar] [CrossRef]
- Qin, Z.; Cui, D.; Yan, S.; Chu, F. Hysteresis modeling of clamp band joint with macro-slip. Mech. Syst. Signal Process. 2016, 66, 89–110. [Google Scholar] [CrossRef]
- Qin, Z.; Yan, S.; Chu, F. Finite element analysis of the clamp band joint. Appl. Math. Model. 2012, 36, 463–477. [Google Scholar] [CrossRef]
- Qin, Z.; Yan, S.; Chu, F. Analytical modeling of clamp band joint under external bending moment. Aerosp. Sci. Technol. 2013, 25, 45–55. [Google Scholar] [CrossRef]
- Barrans, S.; Khodabakhshi, G.; Xu, Q. Contact pressure distribution in joints formed by V-band clamps. Adv. Mater. Res. 2014, 1016, 34–38. [Google Scholar] [CrossRef]
- Barrans, S.; Waterworth, A.; Sahboun, S. Analysis of the torsional load capacity of V-section band clamps. Adv. Mater. Res. 2014, 1016, 59–64. [Google Scholar] [CrossRef]
- Muller, M.; Barrans, S.; Blunt, L. Predicting plastic deformation and work hardening during V-band formation. J. Mater. Process. Technol. 2011, 211, 627–636. [Google Scholar] [CrossRef]
- Zhang, Z.; Wu, P.; Zhang, H.H. Contact stress analysis of interference fit between V-band clamp and piping systems. Int. J. Eng. Innov. Technol. 2014, 4, 48–54. [Google Scholar]
- Wang, W.; Zhang, M.; Xue, J. Development of Fiber Sensors integrated with Aerospace Composites for Structure Health Monitoring. Adv. Eng. Technol. Res. 2023, 8, 242. [Google Scholar] [CrossRef]
- NASA expands fiber-optic sensor studies. Int. Aviat. 2008, 7, 17–19.
- Soller, B.J.; Gifford, D.K.; Wolfe, M.S.; Froggatt, M.E. High resolution optical frequency domain reflectometry for characterization of components and assemblies. Opt. Express 2005, 13, 666–674. [Google Scholar] [CrossRef] [PubMed]
- Gifford, D.K.; Froggatt, M.E.; Kreger, S.T. High precision, high sensitivity distributed displacement and temperature measurements using OFDR-based phase tracking. In Proceedings of the 21st International Conference on Optical Fiber Sensors, Ottawa, Canada, 15–19 May 2011; pp. 536–539. [Google Scholar]
- Iadicicco, A.; Natale, D.; Di Palma, P.; Spinaci, F.; Apicella, A.; Campopiano, S. Strain monitoring of a composite drag strut in aircraft landing gear by fiber bragg grating sensors. Sensors 2019, 19, 2239. [Google Scholar] [CrossRef]
- Hardt, M.; Ayuso, A.; Cocho, D.; Urmston, P.; Gracia, O. Multibody dynamic considerations in the redesign of the international berthing and docking mechanism (IBDM). In Proceedings of the 1st ESA Workshop on Multibody Dynamics for Space Applications, ESA/ESTEC, Noordwijk, The Netherlands, 2–3 February 2010; pp. 1–9. [Google Scholar]
- LaBauve, T.E. Low Impact Docking System (LIDS)—NASA Technical Reports Server (NTRS); No. JSC-17710; US Gov Publications: Washington, DC, USA, 2009.
- Parma, G.F. Overview of the NASA docking system (NDS) and the international docking system standard (IDSS). In Proceedings of the AIAA Houston Section Annual Technical Symposium, Houston, TX, USA, 13 November 2021. [Google Scholar]
- Cabaleiro, M.; Conde, B.; González-Gaya, C.; Barros, B. Removable, reconfigurable, and sustainable steel structures: A state-of-the-art review of clamp-based steel connections. Sustainability 2023, 15, 7808. [Google Scholar] [CrossRef]
- Kreger, S.T.; Rahim, N.A.A.; Garg, N.; Klute, S.M.; Metrey, D.R.; Beaty, N.; Jeans, J.W.; Gamber, R. Optical frequency domain reflectometry: Principles and applications in fiber optic sensing. In Proceedings of the Fiber Optic Sensors and Applications XIII, Baltimore, MD, USA, 12 May 2016; pp. 218–227. [Google Scholar]
- Palmieri, L.; Schenato, L. Distributed optical fiber sensing based on Rayleigh scattering. Open Opt. J. 2013, 7, 104–127. [Google Scholar] [CrossRef]
- Palmieri, L.; Schenato, L.; Santagiustina, M.; Galtarossa, A. Rayleigh-based distributed optical fiber sensing. Sensors 2022, 22, 6811. [Google Scholar] [CrossRef] [PubMed]
- Yang, X.; Zhan, L.; Jiang, C.; Zhang, Y.; Hu, J. Influence of mould materials on curing process and deformation of composite part. J. Nanjing Univ. Aeronaut. Astronaut. 2018, 50, 24–29. [Google Scholar]




| Part(s) | Material | E/GPa | μ |
|---|---|---|---|
| Interface ring and V-segment | Aluminum alloy | 70 | 0.3 |
| Clamp band | High-tensile steel | 160 | 0.3 |
| Loading Level | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 |
|---|---|---|---|---|---|---|---|---|---|---|---|
| Preload/kN | 0 | 4.65 | 8.02 | 10.59 | 11.96 | 13.38 | 15.24 | 16.63 | 22.46 | 23.65 | 25.57 |
| Parameter | Value |
|---|---|
| Distance range (standard mode) | up to 50 m |
| Strain range | ±5000 με |
| Spatial resolution (gauge spacing) | 5 mm |
| Gauge length | 10 mm |
| Strain measurement resolution | ±1 με |
| Number of Block | #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | Mean Value | Standard Deviation | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Before adjustment | Mean strain | 3962 | 3718 | 3696 | 3491 | 3156 | 3468 | 3768 | 3842 | 3947 | 3675 | 249 |
| deviation (%) | 7.82 | 1.16 | 0.57 | −5.01 | −14.1 | −5.64 | 2.53 | 4.55 | 8.14 | |||
| After adjustment | Mean strain | 3761 | 3520 | 3436 | 3241 | 3242 | 3433 | 3587 | 3752 | 3773 | 3527 | 197 |
| deviation (%) | 6.62 | −0.22 | −2.6 | −8.11 | −8.07 | −2.67 | 1.69 | 6.38 | 6.96 | |||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qu, X.; Zhao, S.; Ma, F.; Li, J.; Li, H.; Yang, Z.; Xu, H.; Yang, L.; Wu, Z. Strain Monitoring and Installation Adjustment of Satellite–Rocket Connection Device Based on Distributed Optical Fibers. Photonics 2024, 11, 335. https://doi.org/10.3390/photonics11040335
Qu X, Zhao S, Ma F, Li J, Li H, Yang Z, Xu H, Yang L, Wu Z. Strain Monitoring and Installation Adjustment of Satellite–Rocket Connection Device Based on Distributed Optical Fibers. Photonics. 2024; 11(4):335. https://doi.org/10.3390/photonics11040335
Chicago/Turabian StyleQu, Xiaoxi, Shiyuan Zhao, Fuqiang Ma, Jianle Li, Hanke Li, Zhengyan Yang, Hao Xu, Lei Yang, and Zhanjun Wu. 2024. "Strain Monitoring and Installation Adjustment of Satellite–Rocket Connection Device Based on Distributed Optical Fibers" Photonics 11, no. 4: 335. https://doi.org/10.3390/photonics11040335
APA StyleQu, X., Zhao, S., Ma, F., Li, J., Li, H., Yang, Z., Xu, H., Yang, L., & Wu, Z. (2024). Strain Monitoring and Installation Adjustment of Satellite–Rocket Connection Device Based on Distributed Optical Fibers. Photonics, 11(4), 335. https://doi.org/10.3390/photonics11040335







