Narrow Linewidth 510 nm Laser via Single-Pass Frequency-Tripling by Waveguide PPLNs
Abstract
1. Introduction
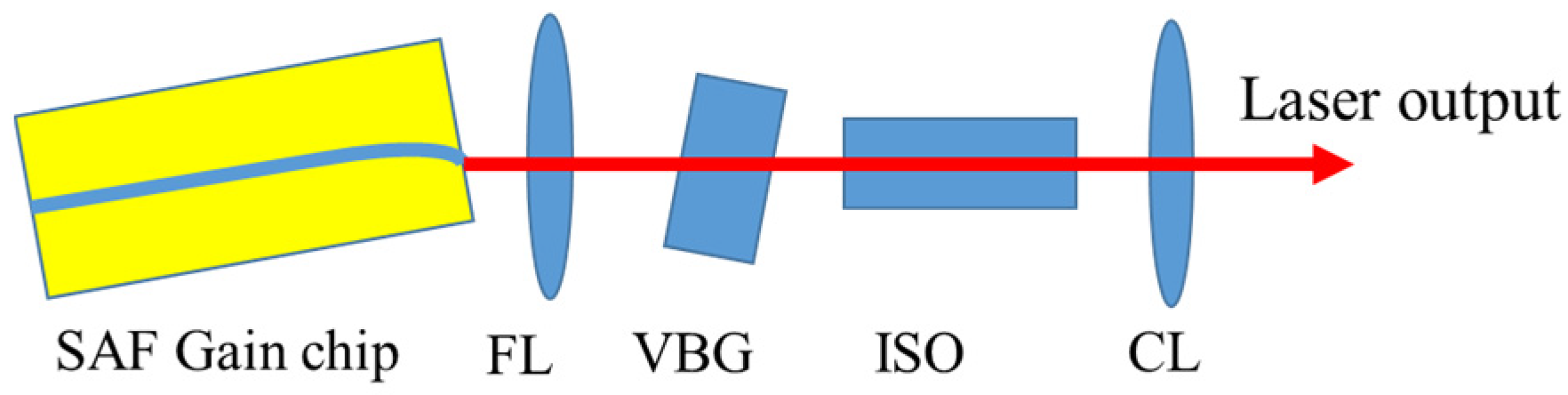
2. Methods and Experiments
3. Results and Analysis
3.1. Optical Spectrum
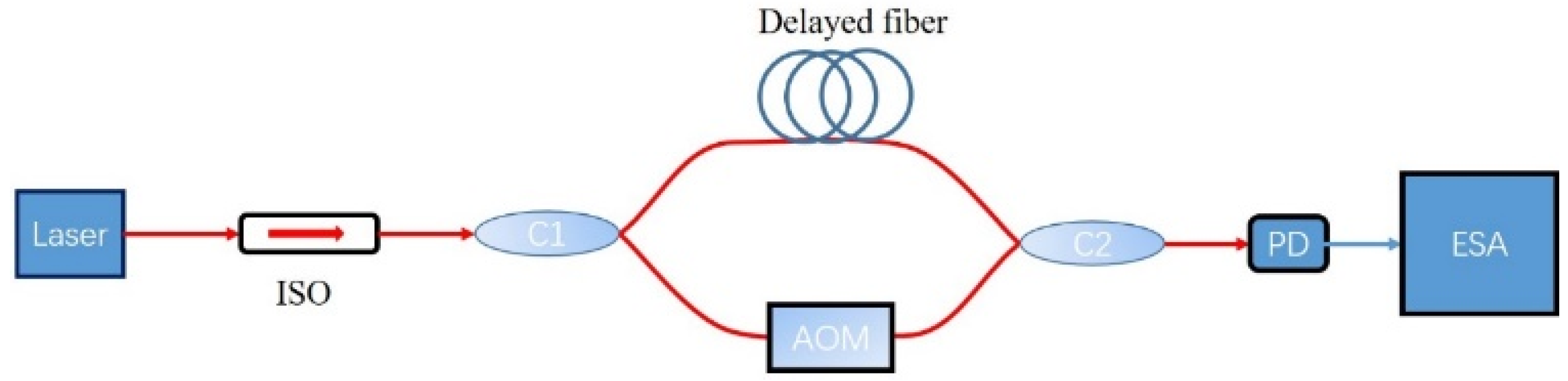
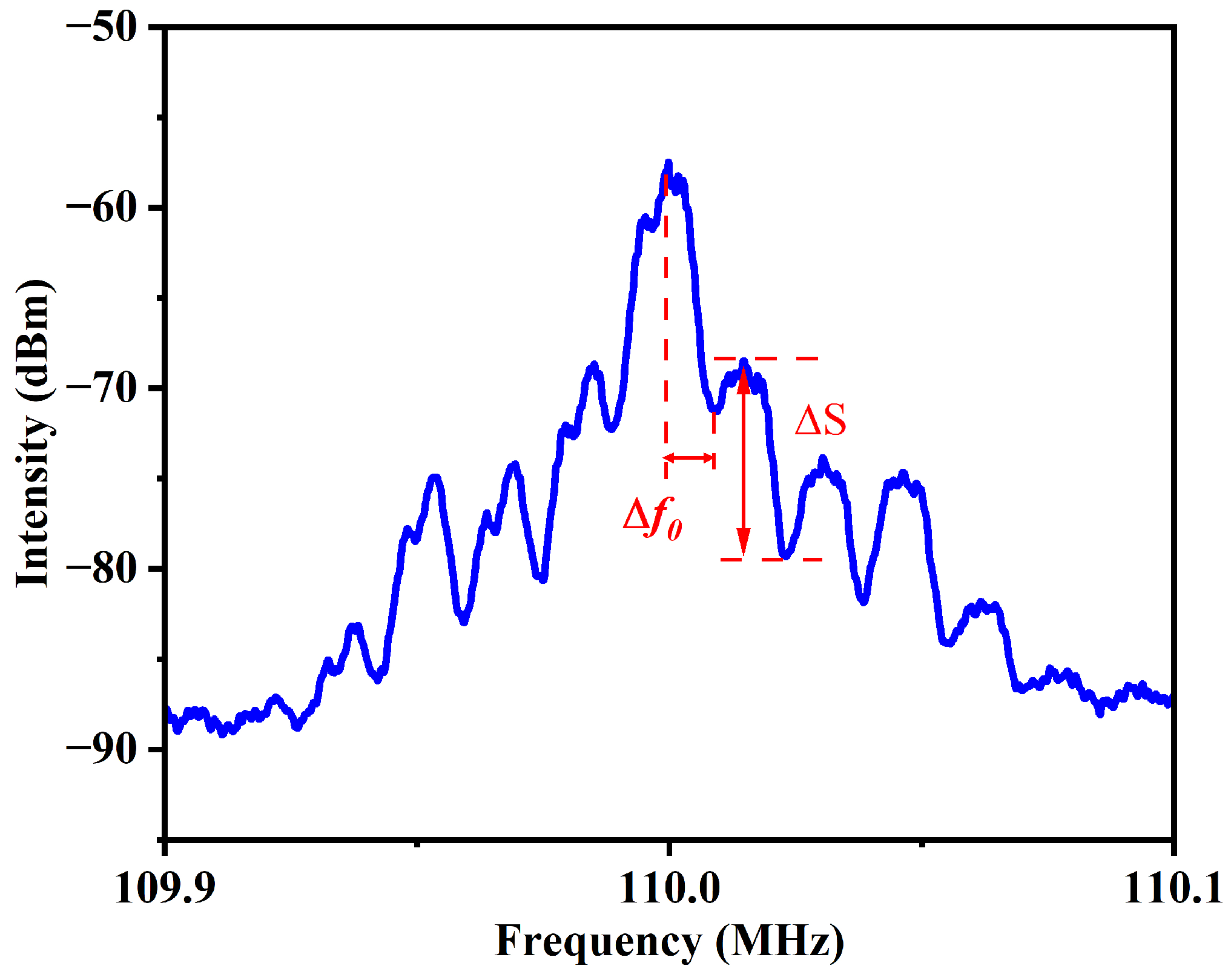
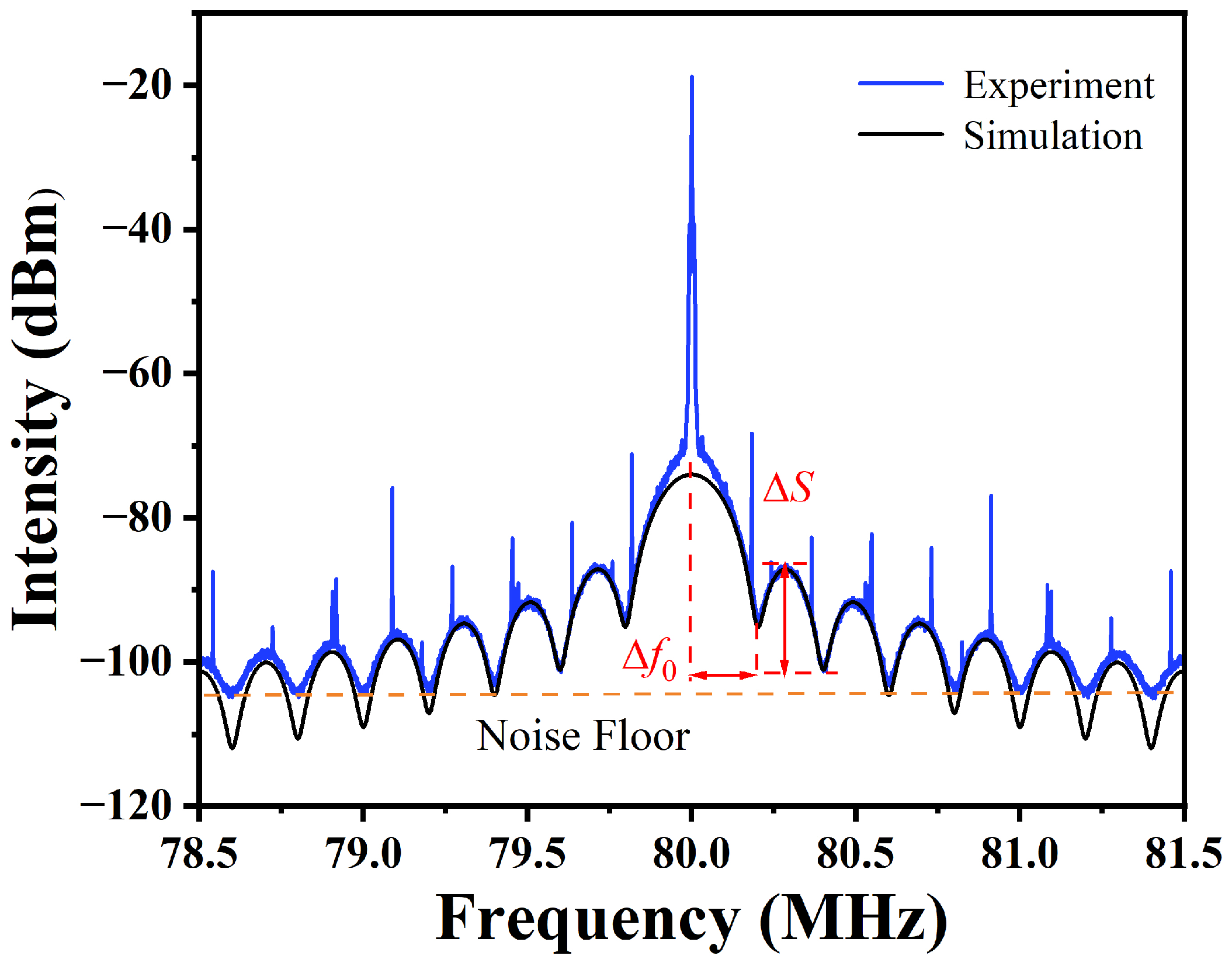
3.2. The Linewidth Measurement
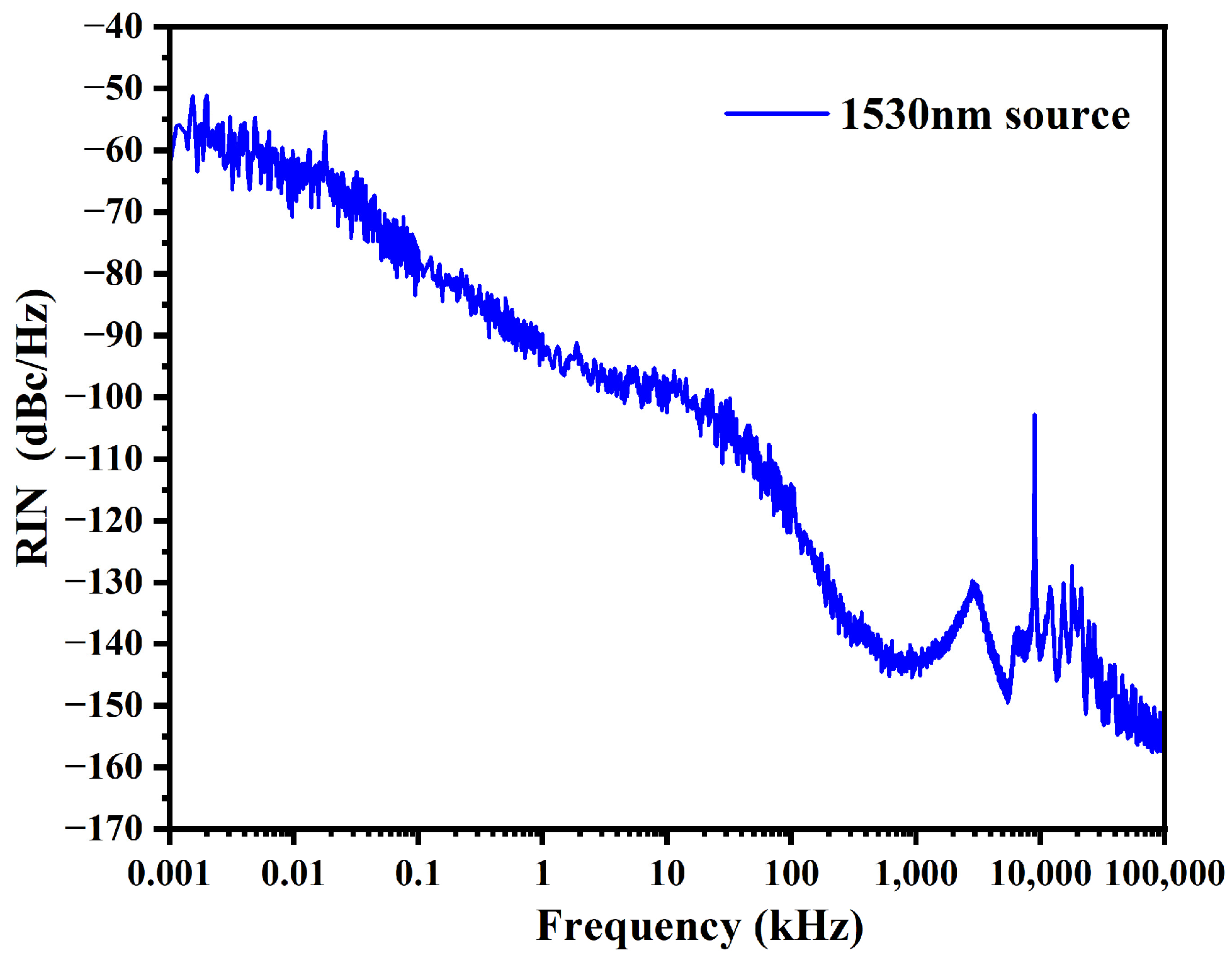
3.3. The Relative Intensity Noise (RIN) Measuremant
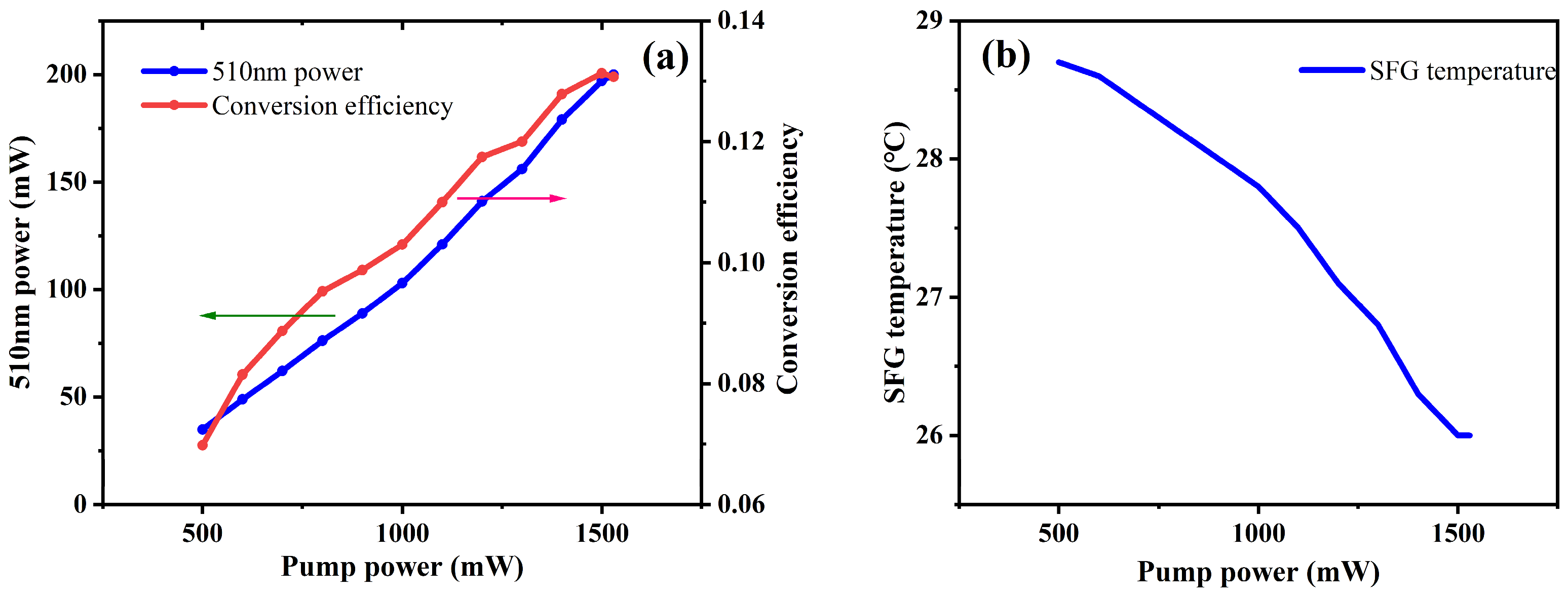
3.4. The Nonlinear Optical Conversion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Anderson, D.A.; Sapiro, R.E.; Gonıfmmode, L.F.; Cardman, R.; Raithel, G. Optical Radio-Frequency Phase Measurement with an Internal-State Rydberg Atom Interferometer. Phys. Rev. Appl. 2022, 17, 044020. [Google Scholar] [CrossRef]
- Li, G.; Zhou, Q.; Xu, G.; Wang, X.; Han, W.; Wang, J.; Zhang, G.; Zhang, Y.; Yuan, Z.; Song, S.; et al. Lidar-Radar for Underwater Target Detection Using a Modulated Sub-Nanosecond Q-Switched Laser. Opt. Laser Technol. 2021, 142, 107234. [Google Scholar] [CrossRef]
- Lee, M.-W.C. Combination 532-nm and 1064-nm Lasers for Noninvasive Skin Rejuvenation and Toning. Arch. Dermatol. 2003, 139, 1265–1276. [Google Scholar] [CrossRef]
- Hanson, F.; Radic, S. High Bandwidth Underwater Optical Communication. Appl. Opt. 2008, 47, 277–283. [Google Scholar] [CrossRef]
- Tawfieq, M.; Jensen, O.B.; Hansen, A.K.; Sumpf, B.; Paschke, K.; Andersen, P.E. Efficient Generation of 509 nm Light by Sum-Frequency Mixing between Two Tapered Diode Lasers. Opt. Commun. 2015, 339, 137–140. [Google Scholar] [CrossRef][Green Version]
- Chi, M.; Jensen, O.B.; Petersen, P.M. Green High-Power Tunable External-Cavity GaN Diode Laser at 515 nm. Opt. Lett. 2016, 41, 4154–4157. [Google Scholar] [CrossRef] [PubMed]
- Corato-Zanarella, M.; Gil-Molina, A.; Ji, X.; Shin, M.C.; Mohanty, A.; Lipson, M. Widely Tunable and Narrow-Linewidth Chip-Scale Lasers from near-Ultraviolet to near-Infrared Wavelengths. Nat. Photonics 2023, 17, 157–164. [Google Scholar] [CrossRef]
- Qian, J.; Zhang, L.; Jiang, H.; Cui, S.; Zhou, J.; Feng, Y. 2 W Single-Frequency, Low-Noise 509 nm Laser via Single-Pass Frequency Doubling of an ECDL-Seeded Yb Fiber Amplifier. Appl. Opt. 2018, 57, 8733–8737. [Google Scholar] [CrossRef]
- Philippe, C.; Chea, E.; Nishida, Y.; du Burck, F.; Acef, O. Efficient Third Harmonic Generation of a CW-Fibered 1.5 Μm Laser Diode. Appl. Phys. B 2016, 122, 265. [Google Scholar] [CrossRef]
- Lu, D.; Fang, Q.; Yu, X.; Han, X.; Wang, J.; Yu, H.; Zhang, H. Power Scaling of the Self-Frequency-Doubled Quasi-Two-Level Yb:YCOB Laser with a 30% Slope Efficiency. Opt. Lett. 2019, 44, 5157–5160. [Google Scholar] [CrossRef] [PubMed]
- Chen, X.; Xu, Y.; Tsai, C.-C.; Chen, Y.-H.; Chen, N.-K.; Chui, H.-C. An Injection-Locked Green InGaN Diode Laser. Microw. Opt. Technol. Lett. 2023, 65, 1037–1041. [Google Scholar] [CrossRef]
- Huang, S.; Zhu, T.; Yin, G.; Lan, T.; Huang, L.; Li, F.; Bai, Y.; Qu, D.; Huang, X.; Qiu, F. Tens of Hertz Narrow-Linewidth Laser Based on Stimulated Brillouin and Rayleigh Scattering. Opt. Lett. 2017, 42, 5286–5289. [Google Scholar] [CrossRef] [PubMed]
- Chen, M.; Meng, Z.; Liu, H.; Lu, Y.; Hu, X.; Wang, J. 140-Hz Narrow-Linewidth Brillouin/Erbium- Ytterbium Fiber Laser. IEEE Photonics Technol. Lett. 2023, 35, 521–524. [Google Scholar] [CrossRef]
- Debut, A.; Randoux, S.; Zemmouri, J. Experimental and Theoretical Study of Linewidth Narrowing in Brillouin Fiber Ring Lasers. J. Opt. Soc. Am. B 2001, 18, 556–567. [Google Scholar] [CrossRef]
- Debut, A.; Randoux, S.; Zemmouri, J. Linewidth Narrowing in Brillouin Lasers: Theoretical Analysis. Phys. Rev. A 2000, 62, 023803. [Google Scholar] [CrossRef]
- Okada, K.; Hashimoto, K.; Shibata, T.; Nagaki, Y. Novel Method for High Resolution Measurement of Laser Output Spectrum. Electron. Lett. 1980, 16, 630. [Google Scholar] [CrossRef]
- Peng, Y. A Novel Scheme for Hundred-Hertz Linewidth Measurements with the Self-Heterodyne Method. Chin. Phys. Lett. 2013, 30, 084208. [Google Scholar] [CrossRef]
- Bennetts, S.; McDonald, G.D.; Hardman, K.S.; Debs, J.E.; Kuhn, C.C.N.; Close, J.D.; Robins, N.P. External Cavity Diode Lasers with 5 kHz Linewidth and 200 nm Tuning Range at 1.55 μm and Methods for Linewidth Measurement. Opt. Express 2014, 22, 10642–10654. [Google Scholar] [CrossRef]
- Huang, S.; Zhu, T.; Cao, Z.; Liu, M.; Deng, M.; Liu, J.; Li, X. Laser Linewidth Measurement Based on Amplitude Difference Comparison of Coherent Envelope. IEEE Photonics Technol. Lett. 2016, 28, 759–762. [Google Scholar] [CrossRef]
- He, Y.; Hu, S.; Liang, S.; Li, Y. High-Precision Narrow Laser Linewidth Measurement Based on Coherent Envelope Demodulation. Opt. Fiber Technol. 2019, 50, 200–205. [Google Scholar] [CrossRef]
- Bai, Z.; Zhao, Z.; Qi, Y.; Ding, J.; Li, S.; Yan, X.; Wang, Y.; Lu, Z. Narrow-Linewidth Laser Linewidth Measurement Technology. Front. Phys. 2021, 9, 768165. [Google Scholar] [CrossRef]
- Wang, Z.; Ke, C.; Zhong, Y.; Xing, C.; Wang, H.; Yang, K.; Cui, S.; Liu, D. Ultra-Narrow-Linewidth Measurement Utilizing Dual-Parameter Acquisition through a Partially Coherent Light Interference. Opt. Express 2020, 28, 8484–8493. [Google Scholar] [CrossRef]
- Xu, D.; Yang, F.; Chen, D.; Wei, F.; Cai, H.; Fang, Z.; Qu, R. Laser Phase and Frequency Noise Measurement by Michelson Interferometer Composed of a 3 × 3 Optical Fiber Coupler. Opt. Express 2015, 23, 22386–22393. [Google Scholar] [CrossRef]
- Zhao, Z.; Bai, Z.; Jin, D.; Chen, X.; Qi, Y.; Ding, J.; Yan, B.; Wang, Y.; Lu, Z.; Mildren, R.P. The Influence of Noise Floor on the Measurement of Laser Linewidth Using Short-Delay-Length Self-Heterodyne/Homodyne Techniques. Micromachines 2022, 13, 1311. [Google Scholar] [CrossRef]
- Foster, S.B.; Tikhomirov, A.E. Pump-Noise Contribution to Frequency Noise and Linewidth of Distributed-Feedback Fiber Lasers. IEEE J. Quantum Electron. 2010, 46, 734–741. [Google Scholar] [CrossRef]
- Herbers, S.; Dörscher, S.; Benkler, E.; Lisdat, C. Phase Noise of Frequency Doublers in Optical Clock Lasers. Opt. Express 2019, 27, 23262–23273. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Fonseca-Campos, J.; Liang, W.; Xu, C.-Q.; Vargas-Baca, I. Noise Analysis of Second-Harmonic Generation in Undoped and MgO-Doped Periodically Poled Lithium Niobate. Adv. OptoElectron. 2008, 2008, 428971. [Google Scholar] [CrossRef]
- Tehranchi, A.; Kashyap, R. Design of Novel Unapodized and Apodized Step-Chirped Quasi-Phase Matched Gratings for Broadband Frequency Converters Based on Second-Harmonic Generation. J. Light. Technol. 2008, 26, 343–349. [Google Scholar] [CrossRef]




| Work | Laser Type | Wavelength (Turning Range) | Power | Efficiency | Linewidth |
|---|---|---|---|---|---|
| Qian et al. [8] | SHG | 509 nm (~) | 2 W | 20% | ~40 kHz |
| Philippe et al. [9] | SHG + SFG | 514 nm (~) | 290 mW | 36% | ~ |
| Lu et al. [10] | SFD | 513 nm (~) | 6.2 W | 30% | ~ |
| Chi et al. [6] | ECDL | 515 nm (9.2 nm) | 480 mW | ~ | ~0.5 nm |
| Mateus et al. [7] | micro-ring | 520 nm (6.0 nm) | 10.75 mW | ~ | 26 kHz |
| Chen et al. [11] | injection-lock | 519 nm (~) | 60 mW | ~ | 6.5 MHz |
| Tawfieq et al. [5] | SFG | 509 nm (~) | 1.7 W | 12.1% | ~ |
| This work | SHG + SFG | 510 nm (9 pm) | 200 mW | 13.3% | 4.8 kHz |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, Y.; Zhang, J.; Qiu, X.; Wang, S.; Li, C.; Yu, H.; Wei, X. Narrow Linewidth 510 nm Laser via Single-Pass Frequency-Tripling by Waveguide PPLNs. Photonics 2024, 11, 269. https://doi.org/10.3390/photonics11030269
Chen Y, Zhang J, Qiu X, Wang S, Li C, Yu H, Wei X. Narrow Linewidth 510 nm Laser via Single-Pass Frequency-Tripling by Waveguide PPLNs. Photonics. 2024; 11(3):269. https://doi.org/10.3390/photonics11030269
Chicago/Turabian StyleChen, Yanlin, Jing Zhang, Xiaolang Qiu, Suo Wang, Chuanchuan Li, Haiyang Yu, and Xin Wei. 2024. "Narrow Linewidth 510 nm Laser via Single-Pass Frequency-Tripling by Waveguide PPLNs" Photonics 11, no. 3: 269. https://doi.org/10.3390/photonics11030269
APA StyleChen, Y., Zhang, J., Qiu, X., Wang, S., Li, C., Yu, H., & Wei, X. (2024). Narrow Linewidth 510 nm Laser via Single-Pass Frequency-Tripling by Waveguide PPLNs. Photonics, 11(3), 269. https://doi.org/10.3390/photonics11030269






