Fabrication of a 4 m SiC Aspheric Mirror Using an Optimized Strategy of Dividing an Error Map
Abstract
1. Introduction
- Zernike polynomials can provide a smooth error map fit without high-frequency oscillation.
- Zernike polynomials are orthogonal and do not contribute to the rest of the error.
- Zernike polynomials can characterize the errors caused by the design tolerance. Errors of tolerance can be eliminated by adjusting the coefficient.
2. Principle of Error Map Dividing Strategy
2.1. Relationship between Tool Size and Surface Figuring Efficiency
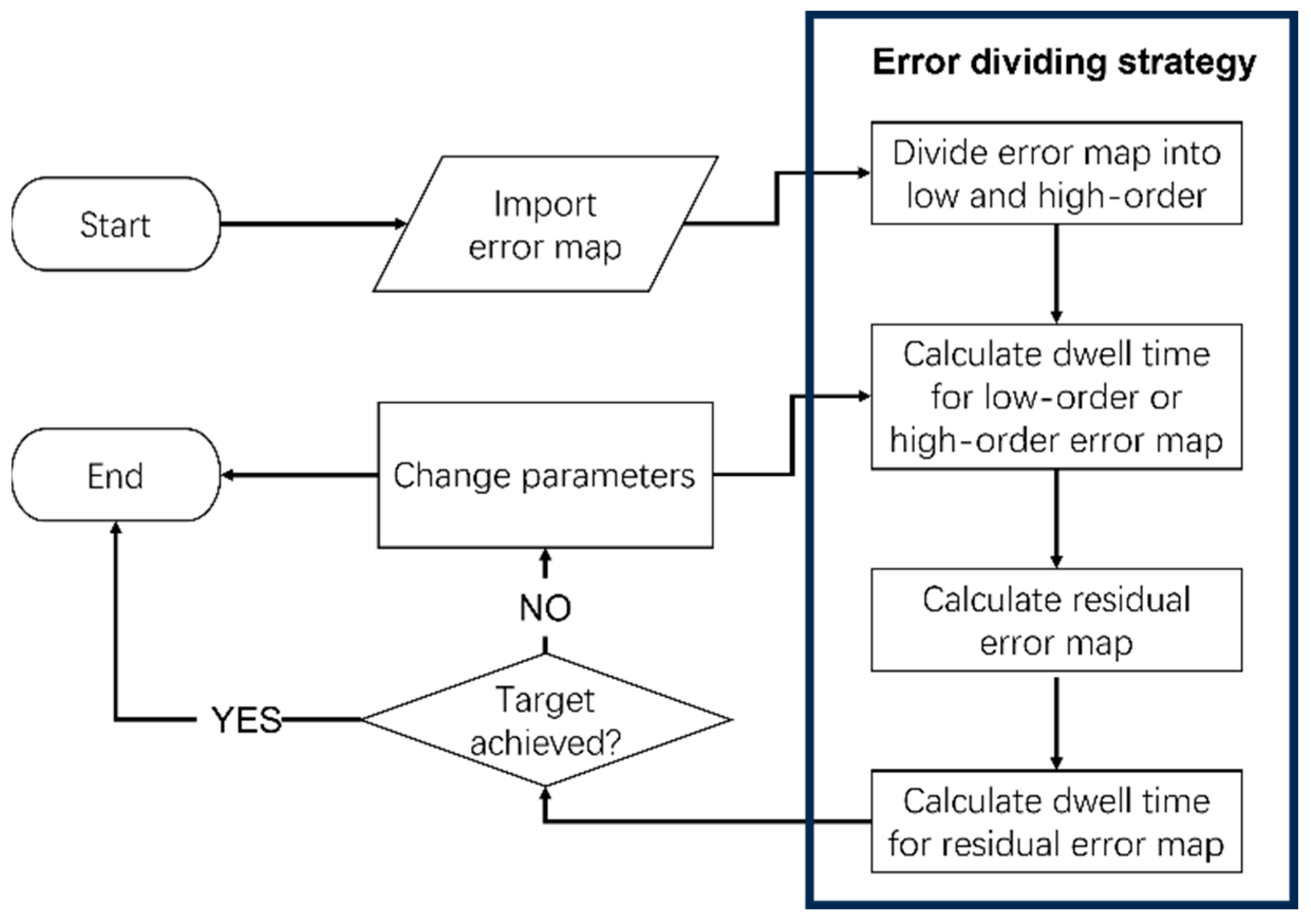
2.2. Principle of the Error Dividing Strategy
2.3. Mathematical Model of the Strategy
- (1)
- The error map is changed from the Cartesian coordinate system (xi, yi, zi) to the polar coordinate system (ρi, φi, zi).
- (2)
- By using Zernike polynomials, error zi can be expressed aswhere ki is the coefficient of the ith terms in the polynomial. Based on the errors at all the points, we can obtain the least square solution of the ki fitting.
- (3)
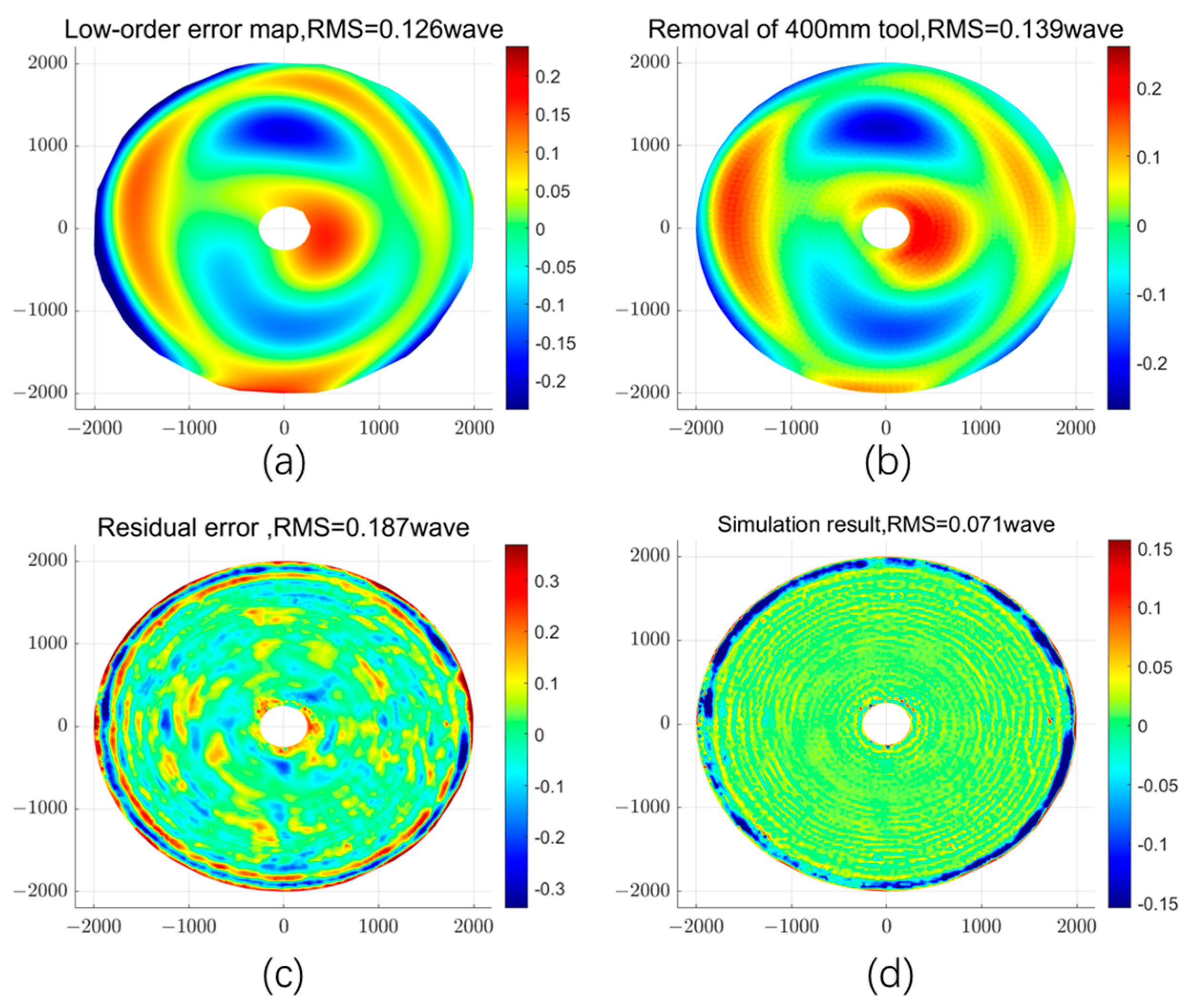
- The first 36 terms of the polynomials are chosen to describe the error map, which forms the low-order frequency of the error, Zlow.
2.4. Dwell Time Optimization Process
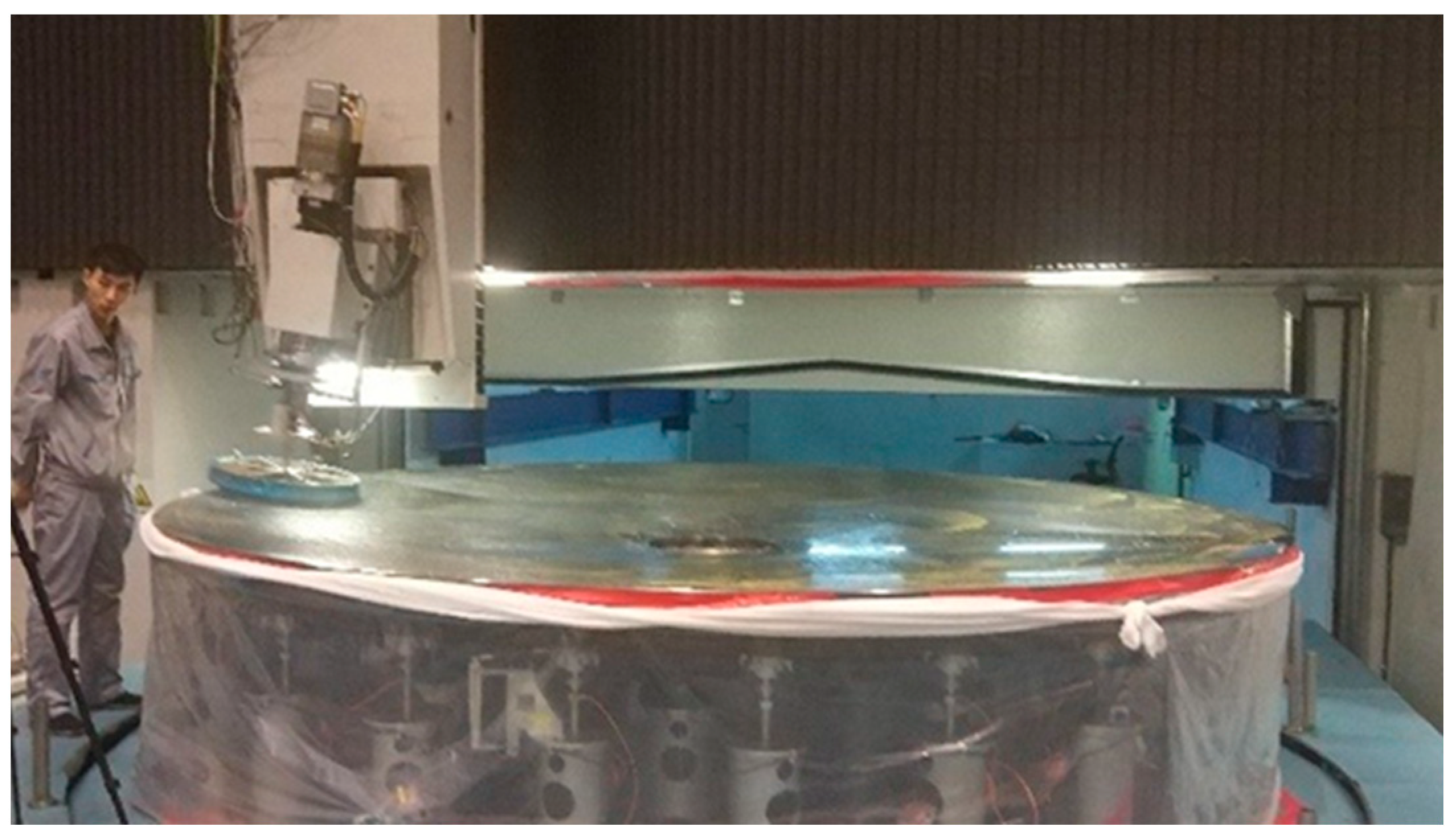
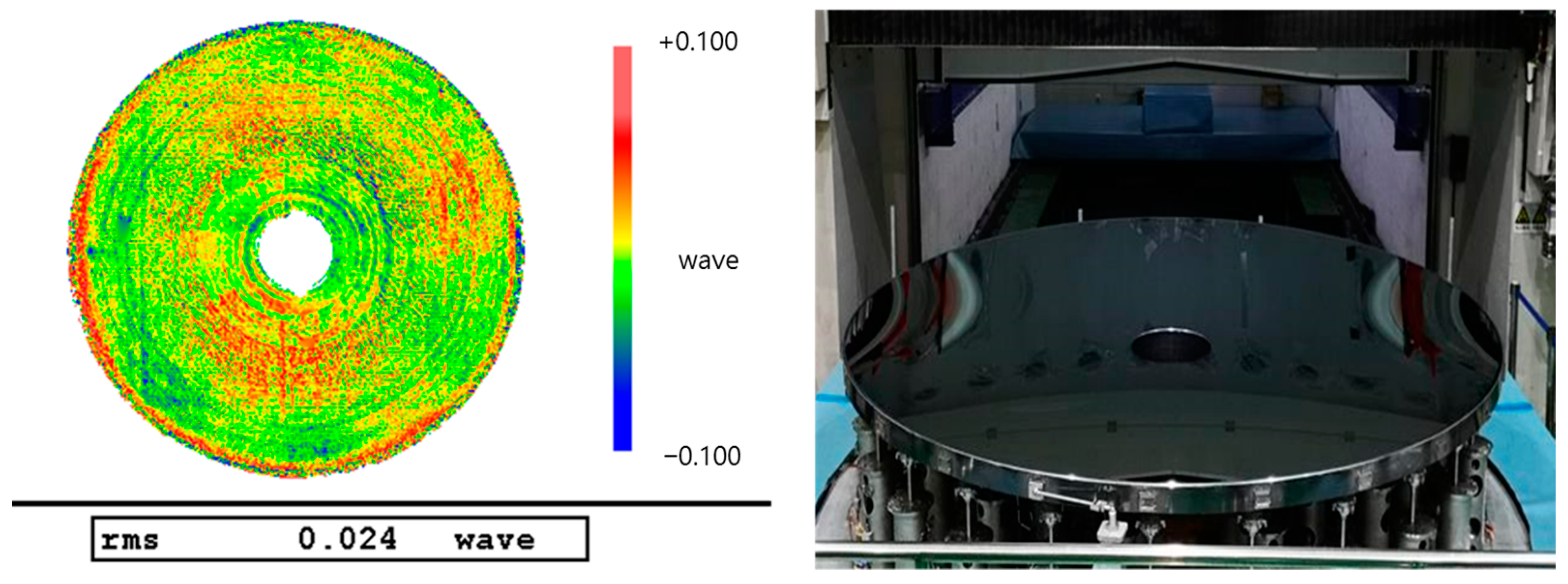
3. Simulation and Experiment
4. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Daukantas, P.; Carts, Y. James Webb Space Telescope. Proc. Int. Astron. Union 2007, 2, 522–525. [Google Scholar]
- Hill, J.M. The large binocular telescope. Appl. Opt. 2010, 49, 115–122. [Google Scholar] [CrossRef] [PubMed]
- Kaifu, A.N. SUBARU Telescope. Proc. SPIE 1998, 15, 14–22. [Google Scholar]
- Luehe, O.V.D.; Derie, F.; Koehler, B.; Paresce, F.; D’Arcio, L. Interferometry with the ESO Very Large Telescope. Proc. SPIE—Int. Soc. Opt. Eng. 1995, 2566, 124–133. [Google Scholar]
- Martin, H.M.; Allen, R.G.; Burge, J.H.; Davis, J.M.; Davison, W.B.; Johns, M.; Kim, D.W.; Kingsley, J.S.; Law, K.; Lutz, R.D.; et al. Production of primary mirror segments for the Giant Magellan Telescope. SPIE Astron. Telesc. Instrum. 2014, 9151, 91510J. [Google Scholar]
- Cole, G.C. Optical fabrication and metrology for the thirty meter telescope primary mirror segments. In Proceedings of the Optical Fabrication and Testing 2017, Denver, CO, USA, 9–13 July 2017; p. OW1B.4. [Google Scholar]
- Rees, P.; Gray, C. Metrology requirements for the serial production of ELT primary mirror segments. SPIE Opt. Eng. Appl. 2015, 9575, 957508. [Google Scholar]
- Stepp, L.M.; Huang, E.; Cho, M.K. Gemini primary mirror support system. In Advanced Technology Optical Telescopes V; International Society for Optics and Photonics: Washington, DC, USA, 1994; pp. 223–237. [Google Scholar]
- European Southern Observatory. The European Extremely Large Telescope (EELT). Available online: https://elt.eso.org/mirror/M1/ (accessed on 21 December 2023).
- Geyl, R.; Leplan, H.; Chaussat, G. Pushing SiC polishing technology for advanced applications. In Proceedings of the SPIE Optical Fabrication, Testing, and Metrology VI, Frankfurt, Germany, 14–17 May 2018; Volume 10692, p. 106920X. [Google Scholar]
- Zhang, X.; Zeng, X.; Hu, H.; Luo, X. Manufacturing and testing large SiC mirrors in an efficient way. In Proceedings of the SPIE Optical Systems Design 2015: Optical Fabrication, Testing, and Metrology V, Jena, Germany, 7–10 September 2015; Volume 9628, p. 96280S. [Google Scholar]
- Rodolfo, J. SiC mirrors polishing. In Proceedings of the SPIE International Conference on Space Optics—ICSO 2014, Tenerife, Canary Islands, Spain, 6–10 October 2014; Volume 10563, p. 105631Z. [Google Scholar]
- Bougoin, M.; Lavenac, J.; Gerbert-Gaillard, A.; Pierot, D. The SiC primary mirror of the EUCLID telescope. In Proceedings of the SPIE International Conference on Space Optics—ICSO 2016, Biarritz, France, 18–21 October 2016; Volume 10562, p. 105623Q. [Google Scholar]
- Pilbratt, G.L. Herschel mission overview and key programmes. In Proceedings of the SPIE Space Telescopes and Instrumentation 2008, Marseille, France, 23–28 June 2008; Volume 7010, p. 701002. [Google Scholar]
- Jones, R.A. Fabrication using the computer controlled polisher. Appl. Opt. 1978, 17, 1889–1892. [Google Scholar] [CrossRef] [PubMed]
- Aspden, R.; Mcdonough, R.; Nitchie, F.R. Computer assisted optical surfacing. Appl. Opt. 1972, 11, 2739–2747. [Google Scholar] [CrossRef] [PubMed]
- Walker, D.D.; Brooks, D.; King, A.; Freeman, R.; Morton, R.; Mccavana, G.; Kim, S.-W. The ‘Precessions’ tooling for polishing and figuring flat, spherical and aspheric surfaces. Opt. Express 2003, 11, 958–964. [Google Scholar] [CrossRef] [PubMed]
- Kim, D.W.; Kim, S.W.; Burge, J. Non-sequential optimization technique for a computer controlled optical surfacing process using multiple tool influence functions. Opt. Express 2009, 17, 21850. [Google Scholar] [CrossRef] [PubMed]
- Liu, Z. Dwell time algorithm for multi-mode optimization in manufacturing large optical mirrors. In Proceedings of the SPIE 9281: 7th International Symposium on Advanced Optical Manufacturing and Testing Technologies: Advanced Optical Manufacturing Technologies, Harbin, China, 6 August 2014; p. 92810G. [Google Scholar]
- Luo, X. High-precision fabrication of 4m SiC aspheric mirror. Light Sci. Appl. 2023, 12, 4. [Google Scholar] [CrossRef] [PubMed]
- Zhang, X.; Hu, H.; Wang, X.; Luo, X.; Zhang, G.; Zhao, W.; Wang, X.; Liu, Z.; Xiong, L.; Qi, E.; et al. Challenges and strategies in high-accuracy manufacturing of the world’s largest SiC aspheric mirror. Light Sci. Appl. 2022, 11, 310. [Google Scholar] [CrossRef] [PubMed]
- Bauer, A.; Schiesser, E.M.; Rolland, J.P. Starting geometry creation and design method for freeform optics. Nat. Commun. 2018, 9, 1756. [Google Scholar] [CrossRef] [PubMed]
- Ibañez, D.R.; Gomez-Pedrero, J.A.; Alonso, J.; Quiroga, J.A. Robust fitting of Zernike polynomials to noisy point clouds defined over connected domains of arbitrary shape. Opt. Express 2016, 24, 5918–5933. [Google Scholar] [CrossRef] [PubMed]
- Walker, D.D.; Yu, W.; Yu, G.; Li, H.; Zhang, W.; Lu, C. Insight into aspheric misfit with hard tools: Mapping the island of low mid-spatial frequencies. Appl. Opt. 2017, 56, 9925–9931. [Google Scholar] [CrossRef]
- Du, H.; Song, C.; Li, S.; Peng, X.; Shi, F.; Zhao, M. Multi-tool selection model for the error control of mid- to-high frequency and rapid fabrication on large-scale aspheric optics. Optik 2020, 216, 164635. [Google Scholar] [CrossRef]
- Zhu, W.; Beaucamp, A. Zernike mapping of optimum dwell time in deterministic fabrication of freeform optics. Opt. Express 2019, 27, 28692–28706. [Google Scholar] [CrossRef] [PubMed]
- Lee, H.; Yang, M. Dwell time algorithm for computer-controlled polishing of small axis-symmetrical aspherical lens mold. Opt. Eng. 2001, 40, 1936–1943. [Google Scholar]
- Goodwin, E.P.; Wyant, J.C. Field Guide to Interferometric Optical Testing; SPIE: Bellingham, WA, USA, 2006; p. 25. ISBN 0-8194-6510-0. [Google Scholar]
- Carnal, C.L.; Egert, C.M.; Hylton, K.W. Advanced matrix-based algorithms for ion beam milling of optical components. In Proceedings of the SPIE 1752: Current Developments in Optical Design and Optical Engineering II, San Diego, CA, USA, 22 July 1992; pp. 54–62. [Google Scholar]
- Deng, W.; Zheng, L.-G.; Shi, Y.-L.; Wang, X.-K.; Zhang, X. Dwell time algorithm based on matrix algebra and regularization method. Opt. Precis. Eng. 2007, 15, 1009–1015. (In Chinese) [Google Scholar]


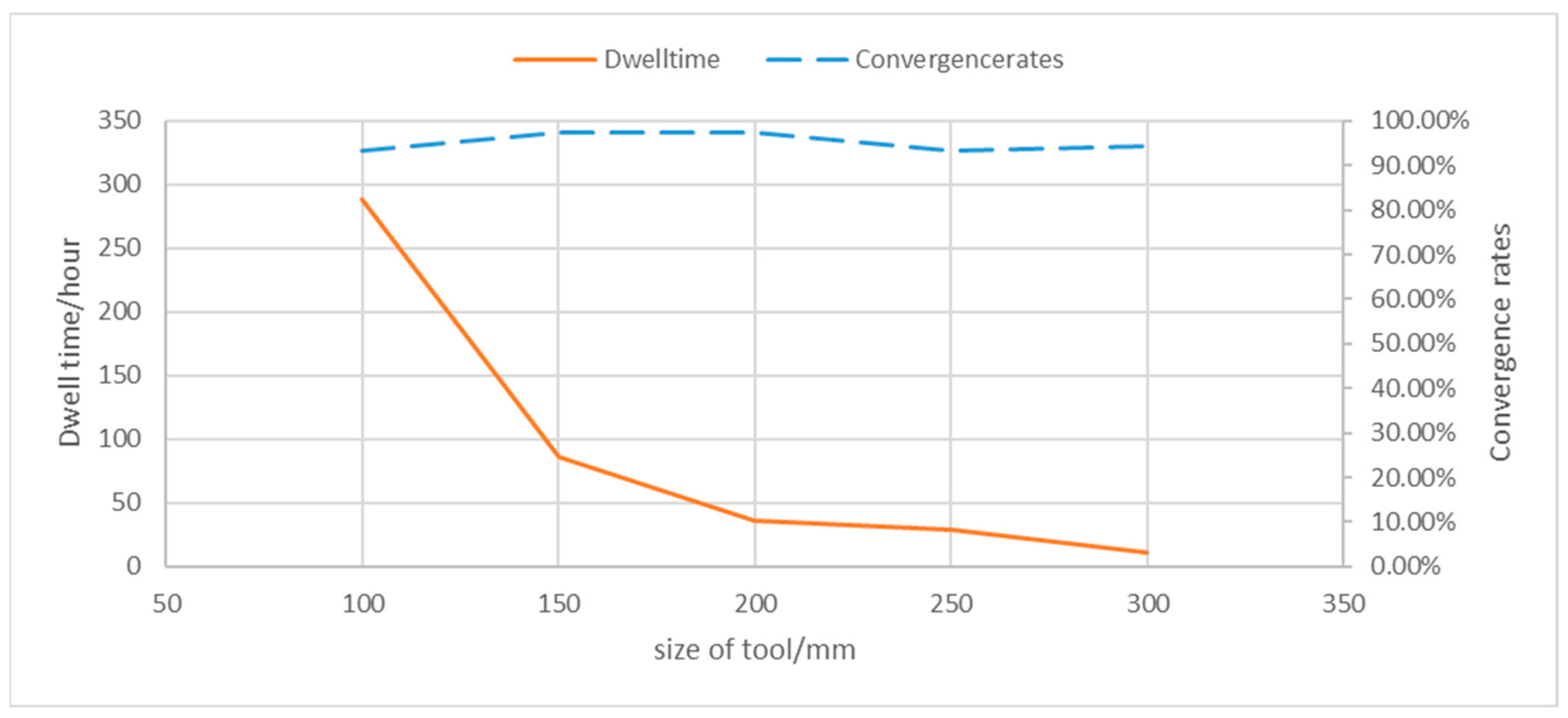


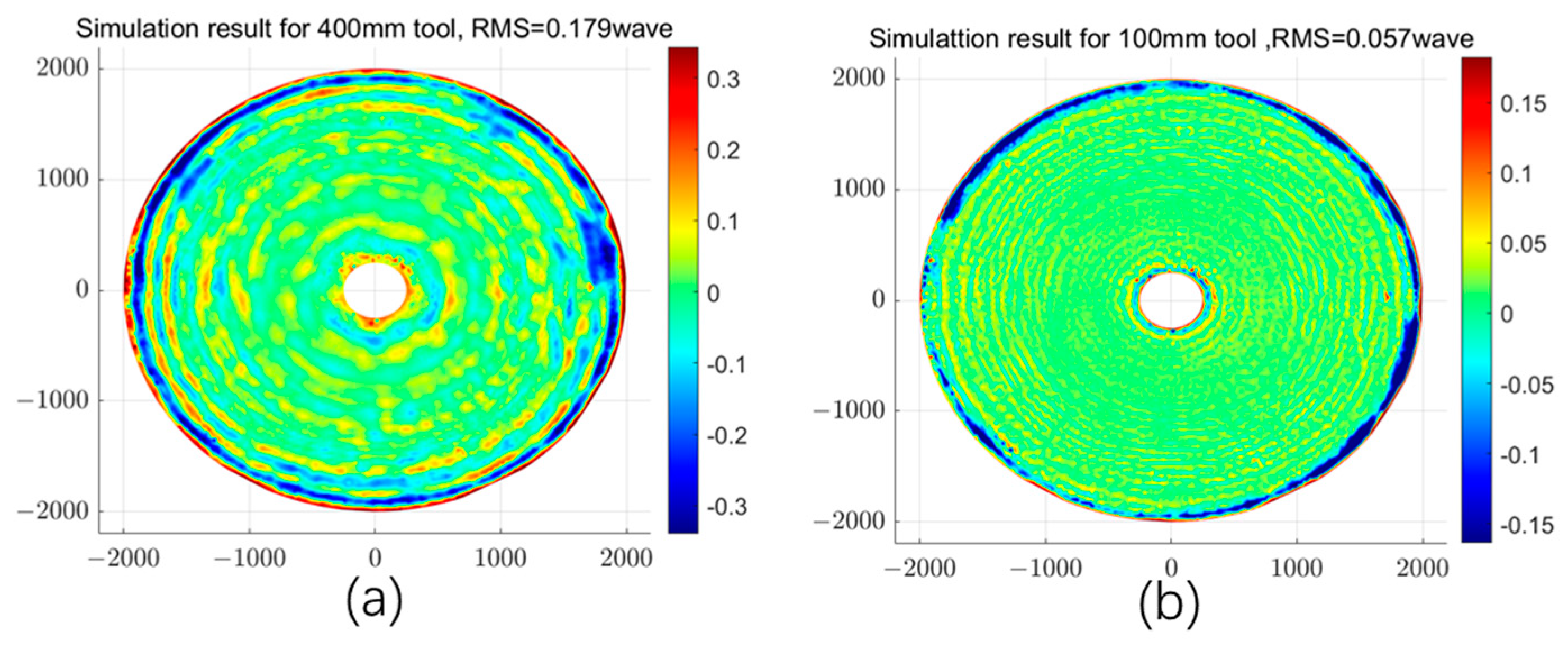

| Tool Size (mm) | 100 | 150 | 200 | 250 | 300 |
|---|---|---|---|---|---|
| Convergence rates | 93.4% | 97.4% | 97.5% | 93.4% | 94.3% |
| Dwell time (h) | 288.8 | 85.7 | 36.2 | 29.2 | 10.8 |
| Tool Size (mm) | 100 | 400 | 400 and 100 |
| Initial RMS (λ) | 0.207 | ||
| Residual RMS (λ) | 0.057 | 0.179 | 0.071 |
| Convergence rate | 72.46% | 13.53% | 65.70% |
| Time (hour) | 100.6 | 26.6 | 24.1(small) + 11.7(large) = 35.8 |
| Convergence efficiency | 0.72 | 0.51 | 1.84 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Z.; Li, L.; Qi, E.; Hu, H.; Luo, X. Fabrication of a 4 m SiC Aspheric Mirror Using an Optimized Strategy of Dividing an Error Map. Photonics 2024, 11, 125. https://doi.org/10.3390/photonics11020125
Liu Z, Li L, Qi E, Hu H, Luo X. Fabrication of a 4 m SiC Aspheric Mirror Using an Optimized Strategy of Dividing an Error Map. Photonics. 2024; 11(2):125. https://doi.org/10.3390/photonics11020125
Chicago/Turabian StyleLiu, Zhenyu, Longxiang Li, Erhui Qi, Haixiang Hu, and Xiao Luo. 2024. "Fabrication of a 4 m SiC Aspheric Mirror Using an Optimized Strategy of Dividing an Error Map" Photonics 11, no. 2: 125. https://doi.org/10.3390/photonics11020125
APA StyleLiu, Z., Li, L., Qi, E., Hu, H., & Luo, X. (2024). Fabrication of a 4 m SiC Aspheric Mirror Using an Optimized Strategy of Dividing an Error Map. Photonics, 11(2), 125. https://doi.org/10.3390/photonics11020125






