Anisotropic Purcell Effect and Quantum Interference in Fractal Aggregates of Nanoparticles
Abstract
1. Introduction
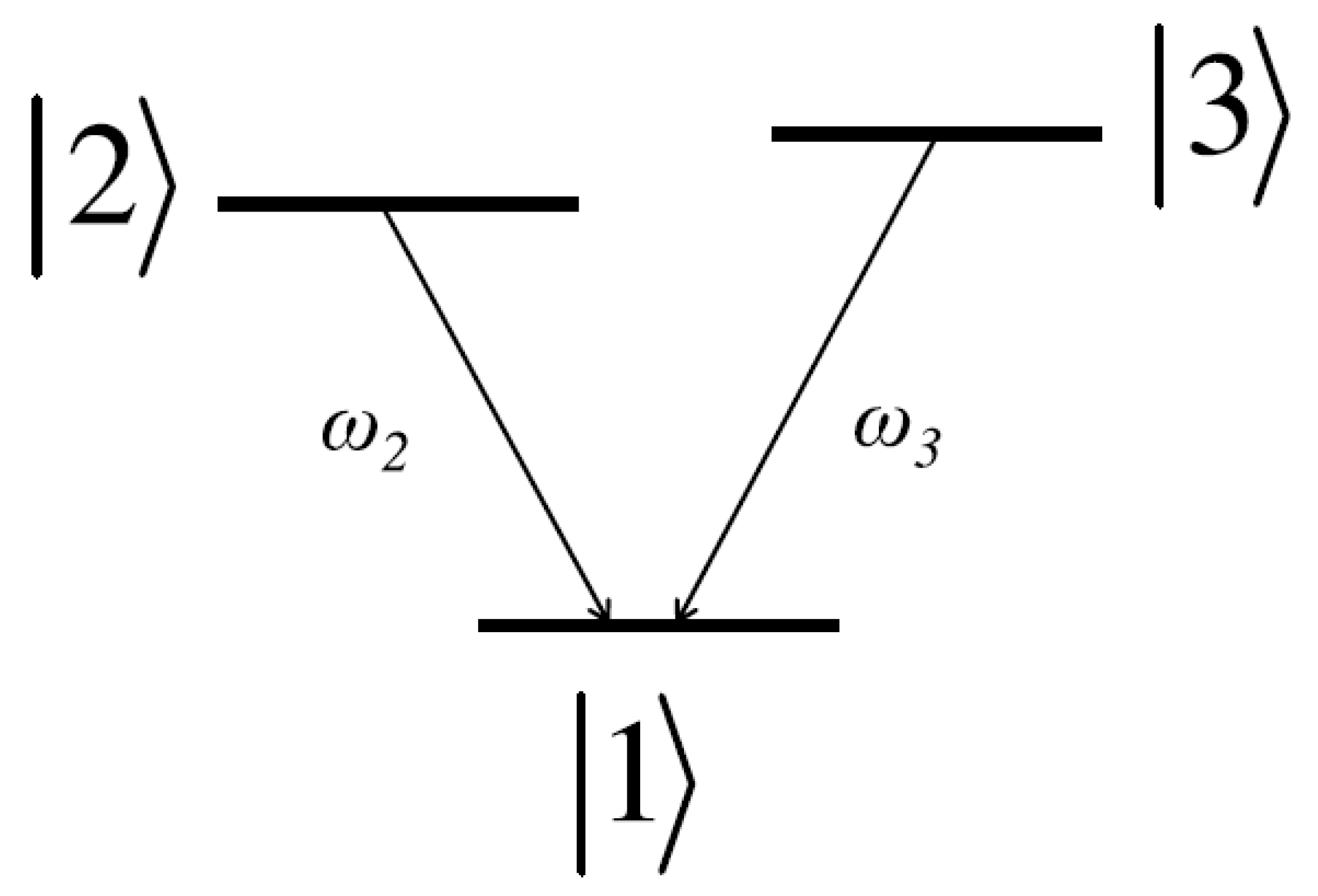
2. EM Green’s Tensor in the Coupled-Dipole Method
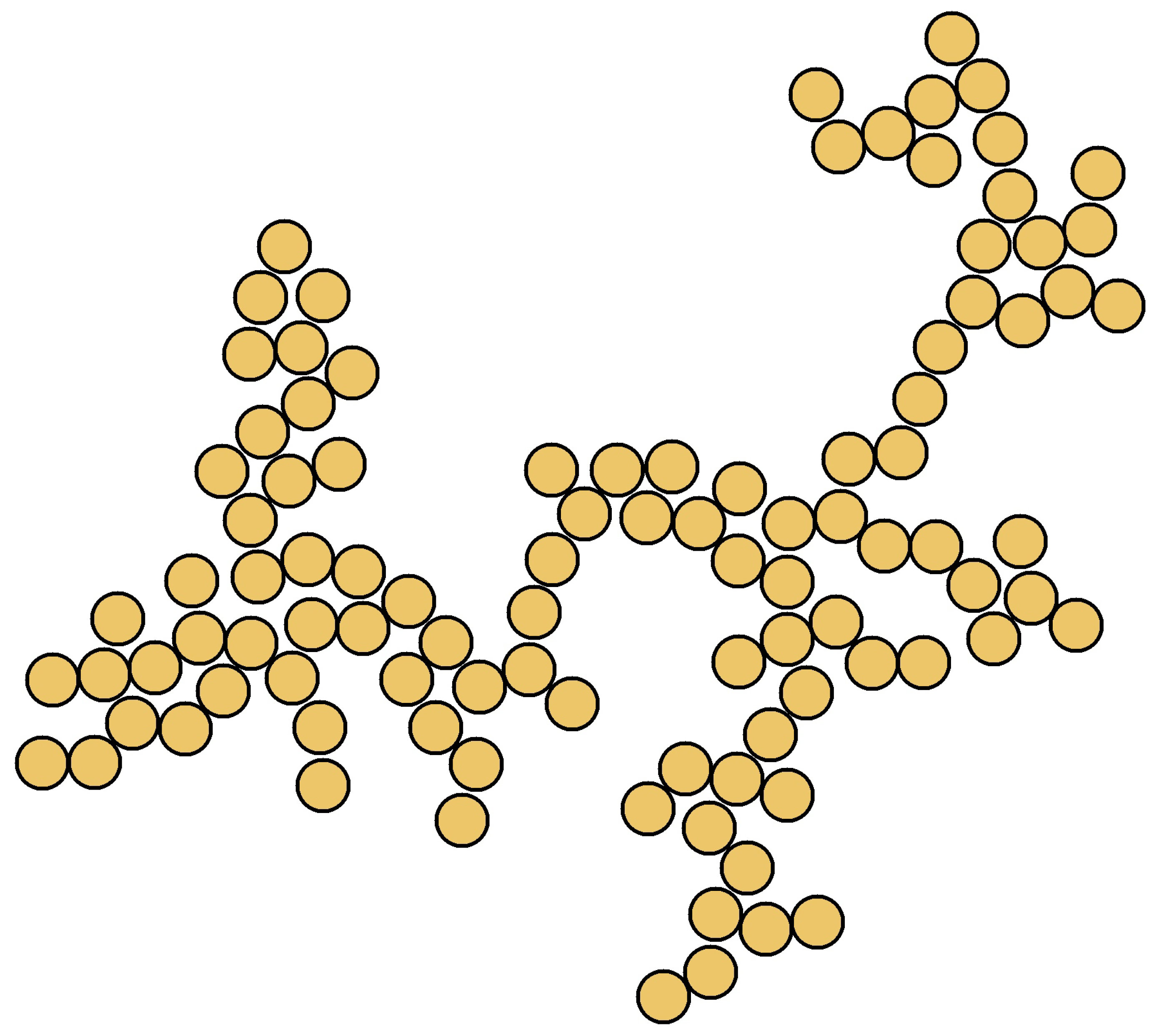
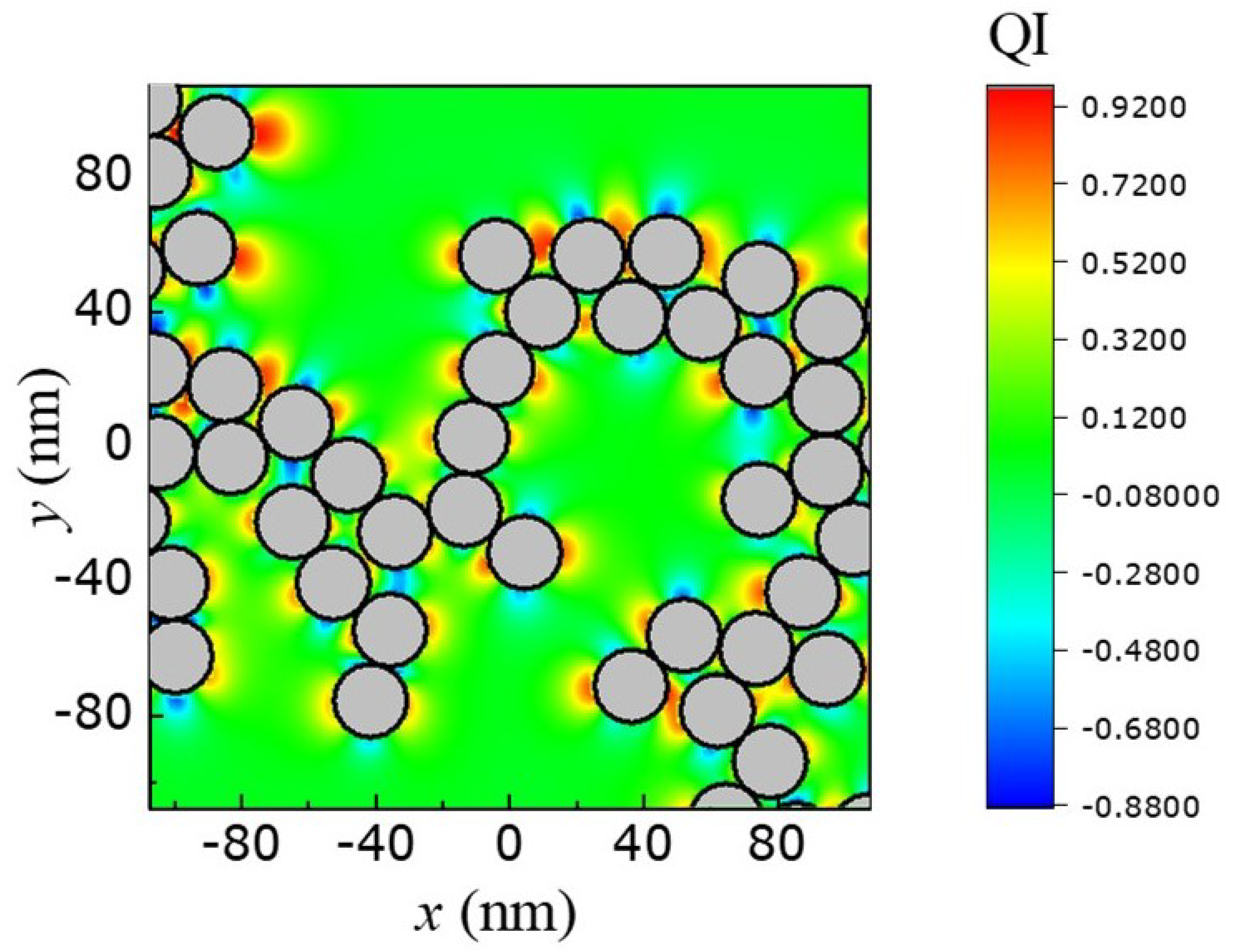
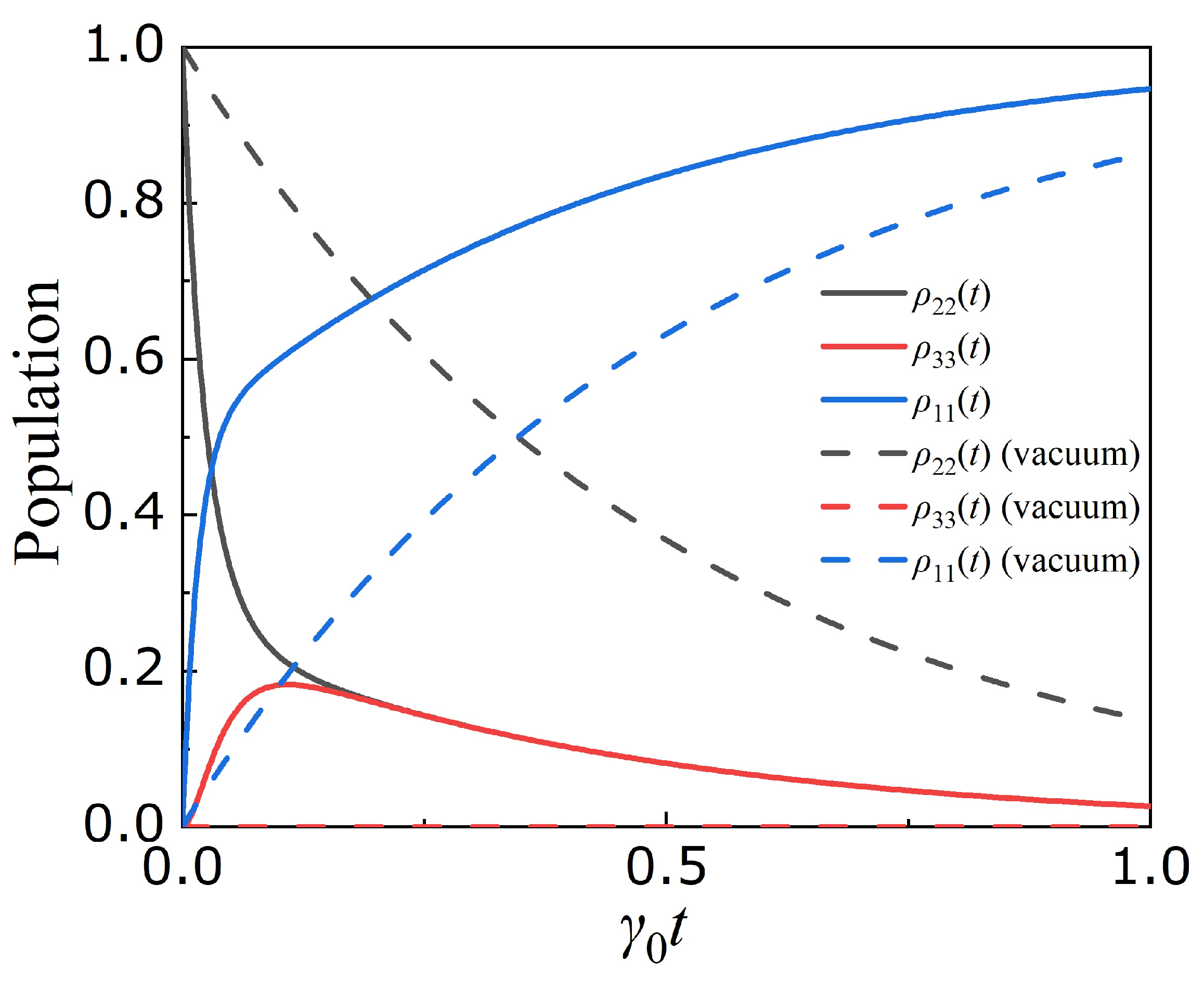
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Purcell, E.M. Spontaneous emission probabilities at radio frequencies. Phys. Rev. 1946, 69, 681. [Google Scholar]
- Drexhage, K.H. Influence of a dielectric interface on fluorescence decay time. J. Luminesc. 1970, 1, 693–701. [Google Scholar] [CrossRef]
- Drexhage, K.H. Interaction of light with monomolecular dye lasers. Prog. Opt. 1974, 12, 163–232. [Google Scholar]
- Tame, M.S.; McEnery, K.R.; Özdemir, Ş.K.; Lee, J.; Maier, S.A.; Kim, M.S. Quantum Plasmonics. Nat. Phys. 2013, 9, 329–340. [Google Scholar] [CrossRef]
- Chang, D.E.; Sørensen, A.S.; Hemmer, P.R.; Lukin, M.D. Quantum Optics with Surface Plasmons. Phys. Rev. Lett. 2006, 97, 053002. [Google Scholar] [CrossRef]
- Vahala, K.J. Optical Microcavities. Nature 2003, 424, 839–846. [Google Scholar] [CrossRef]
- Noginov, M.A.; Zhu, G.; Belgrave, A.M.; Bakker, R.; Shalaev, V.M.; Narimanov, E.E.; Stout, S.; Herz, E.; Suteewong, T.; Wiesner, U. Demonstration of a Spaser-Based Nanolaser. Nature 2009, 460, 1110–1112. [Google Scholar] [CrossRef] [PubMed]
- Atwater, H.A.; Polman, A. Plasmonics for Improved Photovoltaic Devices. Nat. Mater. 2010, 9, 205–213. [Google Scholar] [CrossRef] [PubMed]
- Catchpole, K.R.; Polman, A. Plasmonic Solar Cells. Opt. Express 2008, 16, 21793–21800. [Google Scholar] [CrossRef] [PubMed]
- Pillai, S.; Catchpole, K.R.; Trupke, T.; Green, M.A. Surface Plasmon Enhanced Silicon Solar Cells. J. Appl. Phys. 2007, 101, 093105. [Google Scholar] [CrossRef]
- Ferry, V.E.; Verschuuren, M.A.; Li, H.B.T.; Verhagen, E.; Walters, R.J.; Schropp, R.E.I.; Atwater, H.A.; Polman, A. Light Trapping in Ultrathin Plasmonic Solar Cells. Opt. Express 2010, 18, A237–A245. [Google Scholar] [CrossRef] [PubMed]
- Kneipp, K.; Kneipp, H.; Kneipp, J. Surface-Enhanced Raman Spectroscopy and Biophysics. J. Phys. Condens. Matter 1997, 9, 7671–7683. [Google Scholar]
- Moskovits, M. Surface-Enhanced Spectroscopy. Rev. Mod. Phys. 1985, 57, 783–826. [Google Scholar] [CrossRef]
- Stiles, P.L.; Dieringer, J.A.; Shah, N.C.; Van Duyne, R.P. Surface-Enhanced Raman Spectroscopy. Annu. Rev. Anal. Chem. 2008, 1, 601–626. [Google Scholar] [CrossRef]
- Xu, H.X.; Bjerneld, E.J.; Käll, M.; Börjesson, L. Spectroscopy of Single Hemoglobin Molecules by Surface Enhanced Raman Scattering. Phys. Rev. Lett. 1999, 83, 4357. [Google Scholar] [CrossRef]
- Zalogina, A.S.; Savelev, R.S.; Zograf, G.P.; Komissarenko, F.E.; Milichko, V.A.; Makarov, S.V.; Zuev, D.A.; Shadrivov, I.D. Purcell effect in active diamond nanoantennas. Nanoscale 2018, 10, 8721. [Google Scholar] [CrossRef]
- Lodahl, P.; Mahmoodian, S.; Stobbe, S. Interfacing single photons and single quantum dots with photonic nanostructures. Rev. Mod. Phys. 2015, 87, 347–400. [Google Scholar] [CrossRef]
- Akimov, A.V.; Mukherjee, A.; Yu, C.L.; Chang, D.E.; Zibrov, A.S.; Hemmer, P.R.; Park, H.; Lukin, M.D. Generation of Single Optical Plasmons in Metallic Nanowires Coupled to Quantum Dots. Nature 2007, 450, 402–406. [Google Scholar] [CrossRef]
- Kimble, H.J. The Quantum Internet. Nature 2008, 453, 1023–1030. [Google Scholar] [CrossRef]
- Ritter, S.; Nölleke, C.; Hahn, C.; Reiserer, A.; Neuzner, A.; Uphoff, M.; Mücke, M.; Figueroa, E.; Bochmann, J.; Rempe, G. An elementary quantum network of single atoms in optical cavities. Nature 2012, 484, 195–200. [Google Scholar] [CrossRef]
- Krasnok, A.; Glybovski, S.; Petrov, M.; Makarov, S.; Savelev, R.; Belov, P.; Simovski, C.; KIvshar, Y. Demonstration of the enhanced Purcell factor in all-dielectric structures. Appl. Phys. Lett. 2016, 108, 211105. [Google Scholar] [CrossRef]
- Qian, Z.; Shan, L.; Zhang, X.; Liu, Q.; Ma, Y.; Gong, Q.; Gu, Y. Spontaneous emission in micro- or nanophotonic structures. PhotoniX 2021, 2, 21. [Google Scholar] [CrossRef]
- Yannopapas, V.; Paspalakis, E.; Vitanov, N.V. Plasmon-induced enhancement of quantum interference near metallic nanostructures. Phys. Rev. Lett. 2009, 103, 063602. [Google Scholar] [CrossRef] [PubMed]
- Li, G.X.; Li, F.-L.; Zhu, S.-Y. Quantum interference between decay channels of a three-level atom in a multilayer dielectric medium. Appl. Phys. A 2001, 64, 013819. [Google Scholar] [CrossRef]
- Yang, Y.; Xu, J.; Chen, H.; Zhu, S. Quantum Interference Enhancement with Left-Handed Materials. Phys. Rev. Lett. 2008, 100, 043601. [Google Scholar] [CrossRef] [PubMed]
- Kästel, J.; Fleischhauer, M. Suppression of spontaneous emission and superradiance over macroscopic distances in media with negative refraction. Phys. Rev. A 2005, 71, 011804(R). [Google Scholar] [CrossRef]
- Agarwal, G.S. Anisotropic Vacuum-Induced Interference in Decay Channels. Phys. Rev. Lett. 2000, 84, 5500–5503. [Google Scholar] [CrossRef]
- Paspalakis, E.; Keitel, C.H.; Knight, P.L. Fluorescence control through multiple interference mechanisms. Phys. Rev. A 1998, 58, 4868. [Google Scholar] [CrossRef]
- Ficek, Z.; Swain, S. Simulating quantum interference in a three-level system with perpendicular transition dipole moments. Phys. Rev. A 2004, 69, 023401. [Google Scholar] [CrossRef]
- Zhu, S.-Y.; Scully, M.O. Spectral line elimination and spontaneous emission cancellation via quantum interference. Phys. Rev. Lett. 1996, 76, 388–391. [Google Scholar] [CrossRef]
- Menon, S.; Agarwal, G.S. Gain components in the Autler-Townes doublet from quantum interferences in decay channels. Phys. Rev. A 1999, 61, 013807. [Google Scholar] [CrossRef]
- Hughes, S.; Agarwal, G.S. Anisotropy-Induced Quantum Interference and Population Trapping between Orthogonal Quantum Dot Exciton States in Semiconductor Cavity Systems. Phys. Rev. Lett. 2017, 118, 063601. [Google Scholar] [CrossRef] [PubMed]
- Das, S.; Agarwal, G.S. Protecting bipartite entanglement by quantum interferences. Phys. Rev. A 2010, 81, 052341. [Google Scholar] [CrossRef]
- Iliopoulos, N.; Terzis, A.F.; Yannopapas, V.; Paspalakis, E. Prolonging entanglement dynamics near periodic plasmonic nanostructures. Phys. Rev. B 2017, 96, 075405. [Google Scholar] [CrossRef]
- Sangshekan, B.; Sahrai, M.; Asadpour, S.H. Controllable atom-photon entanglement via quantum interference near plasmonic nanostructure. Sci. Rep. 2022, 12, 677. [Google Scholar] [CrossRef]
- Zhou, P.; Swain, S. Quantum interference in probe absorption: Narrow resonances, transparency, and gain without population inversion. Phys. Rev. Lett. 1997, 78, 832–835. [Google Scholar] [CrossRef]
- Paspalakis, E.; Gong, S.-Q.; Knight, P.L. Spontaneous emission induced coherent effects in absorption and dispersion of a V-type three-level atom. Opt. Commun. 1998, 152, 293–298. [Google Scholar] [CrossRef]
- Zhou, P.; Swain, S. Ultranarrow spectral lines via quantum interference. Phys. Rev. Lett. 1996, 77, 3995. [Google Scholar] [CrossRef]
- Wang, C.-L.; Kang, Z.-H.; Tian, S.-C.; Jiang, Y.; Gao, J.-Y. Effect of spontaneously generated coherence on absorption in a V-type system: Investigation in dressed states. Phys. Rev. A 2009, 79, 043810. [Google Scholar] [CrossRef]
- Evangelou, S.; Yannopapas, V.; Paspalakis, E. Transparency and slow light in a four-level quantum system near a plasmonic nanostructure. Phys. Rev. A 2012, 86, 053811. [Google Scholar] [CrossRef]
- Li, L.; Nie, W.; Chen, A. Transparency and tunable slow and fast light in a nonlinear optomechanical cavity. Sci. Rep. 2016, 6, 35090. [Google Scholar] [CrossRef] [PubMed]
- Frogley, M.D.; Dynes, J.F.; Beck, M.; Faist, J.; Phillips, C.C. Gain without inversion in semiconductor nanostructures. Nat. Mater 2006, 5, 175–178. [Google Scholar] [CrossRef]
- Paspalakis, E.; Kylstra, N.J.; Knight, P.L. Transparency induced via decay interference. Phys. Rev. Lett. 1999, 82, 2079. [Google Scholar] [CrossRef]
- Bortman-Arbiv, D.; Wilson-Gordon, A.D.; Friedmann, H. Phase control of group velocity: From subluminal to superluminal light propagation. Phys. Rev. A 2001, 63, 043818. [Google Scholar] [CrossRef]
- Gurudev Dutt, M.V.; Cheng, J.; Li, B.; Xu, X.; Li, X.; Berman, P.R.; Steel, D.G.; Bracker, A.S.; Gammon, D.; Economou, S.E.; et al. Stimulated and spontaneous optical generation of electron spin coherence in charged GaAs quantum dots. Phys. Rev. Lett. 2005, 94, 227403. [Google Scholar] [CrossRef]
- Niu, Y.-P.; Gong, S.-Q. Enhancing Kerr nonlinearity via spontaneously generated coherence. Phys. Rev. A 2006, 73, 053811. [Google Scholar] [CrossRef]
- Asadpour, S.H.; Sahrai, M.; Soltani, A.; Hamedi, H.R. Enhanced Kerr nonlinearity via quantum interference from spontaneous emission. Phys. Lett. A 2012, 376, 147–152. [Google Scholar] [CrossRef]
- Mandelbrot, B.B. The Fractal Geometry of Nature; Freeman: San Francisco, CA, USA, 1982. [Google Scholar]
- Sapoval, B. Fractals; Aditech: Paris, France, 1990. [Google Scholar]
- Jullien, R.; Botet, R. Aggregation and Fractal Aggregates; World Scientific: Singapore, 1987. [Google Scholar]
- Shalaev, V.M. Nonlinear Optics of Random Media; Springer: Berlin, Germany, 2000. [Google Scholar]
- Markel, V.A.; Muratov, L.S.; Stockman, M.I.; George, T.F. Theory and numerical simulation of optical properties of fractal clusters. Phys. Rev. B 1991, 43, 8183. [Google Scholar] [CrossRef]
- Tsai, D.P.; Kovacs, J.; Wang, Z.; Moskovits, M.; Shalaev, V.M.; Suh, J.; Botet, R. Photon scanning tunneling microscopy images of optical excitations of fractal metal colloid clusters. Phys. Rev. Lett. 1994, 72, 4149. [Google Scholar] [CrossRef]
- Zhang, P.; Haslett, T.L.; Douketis, C.; Moskovits, M. Mode localization in self-affine fractal interfaces observed by near-field microscopy. Phys. Rev. B 1998, 57, 15513. [Google Scholar] [CrossRef]
- Stockman, M.I.; Pandey, L.N.; Muratov, L.S.; George, T.F. Giant fluctuations of local optical fields in fractal clusters. Phys. Rev. Lett. 1994, 72, 2486. [Google Scholar] [CrossRef] [PubMed]
- Stockman, M.I.; Pandey, L.N.; Muratov, L.S.; George, T.F. Optical absorption and localization of eigenmodes in disordered clusters. Phys. Rev. B 1995, 51, 185. [Google Scholar] [CrossRef]
- Stockman, M.I.; Pandey, L.N.; George, T.F. Inhomogeneous localization of polar eigenmodes in fractals. Phys. Rev. B 1996, 53, 2183. [Google Scholar] [CrossRef] [PubMed]
- Stockman, M.I. Chaos and Spatial Correlations for Dipolar Eigenproblems. Phys. Rev. Lett. 1997, 79, 4562. [Google Scholar] [CrossRef]
- Safonov, V.P.; Shalaev, V.M.; Markel, V.A.; Danilova, Y.E.; Lepeshkin, N.N.; Kim, W.; Rautian, S.G.; Armstrong, R.L. Spectral Dependence of Selective Photomodification in Fractal Aggregates of Colloidal Particles. Phys. Rev. Lett. 1998, 80, 1102. [Google Scholar] [CrossRef]
- Shubin, V.A.; Kim, W.; Safonov, V.P.; Sarychev, A.K.; Armstrong, R.L.; Shalaev, V.M. Surface-plasmon-enhanced radiation effects in confined photonic systems. J. Light. Technol. 1999, 17, 2183. [Google Scholar] [CrossRef]
- Chatzidakis, G.D.; Yannopapas, V. A multiple-scattering polaritonic-operator method for hybrid arrays of metal nanoparticles and quantum emitters. J. Mod. Opt. 2018, 65, 951–959. [Google Scholar] [CrossRef]
- Yannopapas, V. Localized Heating of Nanostructures by Coherent Laser Pulses. J. Phys. Chem. C 2013, 117, 14183. [Google Scholar] [CrossRef]
- West, P.R.; Ishii, S.; Naik, G.V.; Emani, N.K.; Shalaev, V.M.; Boltasseva, A. Searching for better plasmonic materials. Laser Photon. Rev. 2010, 4, 795. [Google Scholar] [CrossRef]
- Naik, G.V.; Shalaev, V.M.; Boltasseva, A. Alternative plasmonic materials: Beyond gold and silver. Adv. Mater. 2013, 25, 3264. [Google Scholar] [CrossRef]
- Kinsey, N.; Ferrera, M.; Shalaev, V.M.; Boltasseva, A. Examining nanophotonics for integrated hybrid systems: A review of plasmonic interconnects and modulators using traditional and alternative materials. J. Opt. Soc. Am. B Opt. Phys. 2015, 32, 121. [Google Scholar] [CrossRef]
- Tonkaev, P.; KiIvshar, Y. All-dielectric resonant metaphotonics: Opinion. Opt. Mater. Express 2022, 12, 2879. [Google Scholar] [CrossRef]
- Verre, R.; Baranov, D.G.; Munkhbat, B.; Cuadra, J.; Käll, M.; Shegai, T. Transition metal dichalcogenide nanodisks as high-index dielectric Mie nanoresonators. Nat. Nanotech. 2019, 14, 679. [Google Scholar] [CrossRef] [PubMed]
- Tiguntseva, E.Y.; Zograf, G.P.; Komissarenko, F.E.; Zuev, D.A.; Zakhidov, A.A.; Makarov, S.V.; Kivshar, Y.S. Light-Emitting Halide Perovskite Nanoantennas. Nano Lett. 2018, 18, 1185. [Google Scholar] [CrossRef] [PubMed]
- Thanopulos, I.; Yannopapas, V.; Paspalakis, E. Non-Markovian dynamics in plasmon-induced spontaneous emission interference. Phys. Rev. B 2017, 95, 075412. [Google Scholar] [CrossRef]
- López-Suárez, A.; Torres-Torres, C.; Can-Uc, B.; Rangel-Rojo, R.; Valencia, C.E.; Oliver, A. Third-order nonlinear optical properties exhibited by a bilayer configuration of silver nanoparticles integrated to silicon nanocrystals embedded in ion-implanted silica. J. Opt. Soc. Am. B 2015, 32, 805. [Google Scholar] [CrossRef]
- Joannopoulos, J.D.; Johnson, S.G.; Winn, J.N.; Meade, R.D. Photonics Crystals: Molding the Flow of Light; Princeton University Press: Princeton, NJ, USA, 2008. [Google Scholar]
- Evangelou, S.; Yannopapas, V.; Paspalakis, E. Simulating quantum interference in spontaneous decay near plasmonic nanostructures: Population dynamics. Phys. Rev. A 2011, 83, 055805. [Google Scholar] [CrossRef]
- Karaoulanis, D.; Paspalakis, E.; Yannopapas, V. Quantum interference near bismuth-chalcogenide microstructures. J. Opt. Soc. Am. B 2021, 38, 3301. [Google Scholar] [CrossRef]
- Kyvelos, N.; Tsigaridas, G.; Paspalakis, E.; Yannopapas, V. Quantum Interference in Spontaneous Decay of a Quantum Emitter Placed in a Dimer of Bismuth-Chalcogenide Microparticles. Photonics 2022, 9, 596. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yannopapas, V.; Paspalakis, E. Anisotropic Purcell Effect and Quantum Interference in Fractal Aggregates of Nanoparticles. Photonics 2023, 10, 898. https://doi.org/10.3390/photonics10080898
Yannopapas V, Paspalakis E. Anisotropic Purcell Effect and Quantum Interference in Fractal Aggregates of Nanoparticles. Photonics. 2023; 10(8):898. https://doi.org/10.3390/photonics10080898
Chicago/Turabian StyleYannopapas, Vassilios, and Emmanuel Paspalakis. 2023. "Anisotropic Purcell Effect and Quantum Interference in Fractal Aggregates of Nanoparticles" Photonics 10, no. 8: 898. https://doi.org/10.3390/photonics10080898
APA StyleYannopapas, V., & Paspalakis, E. (2023). Anisotropic Purcell Effect and Quantum Interference in Fractal Aggregates of Nanoparticles. Photonics, 10(8), 898. https://doi.org/10.3390/photonics10080898





