Energy Efficiency Optimization for SLIPT-Enabled NOMA System
Abstract
1. Introduction
2. System Model
2.1. Transmitter
2.2. Channel Model
2.3. Receiver
3. Problem Formulation
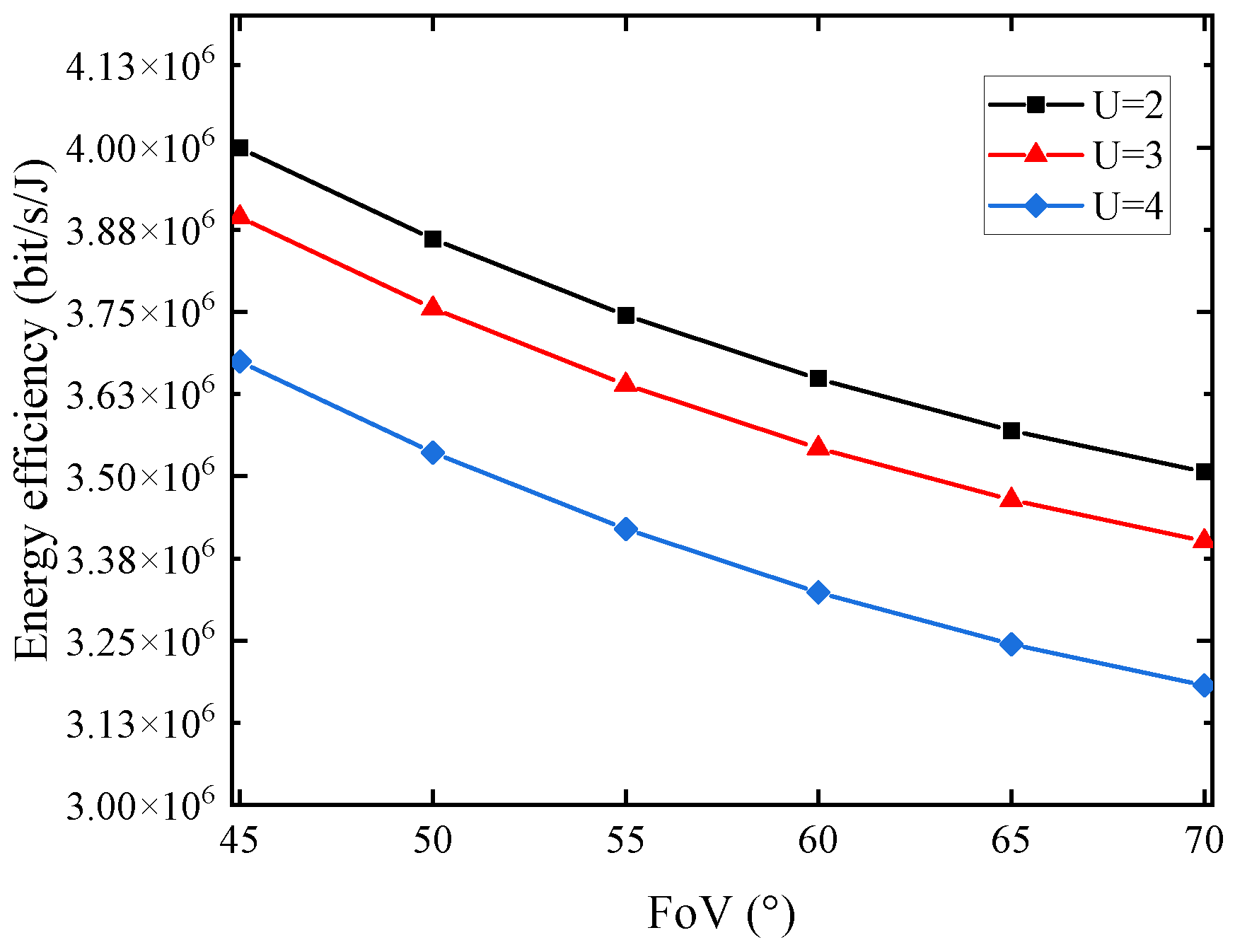
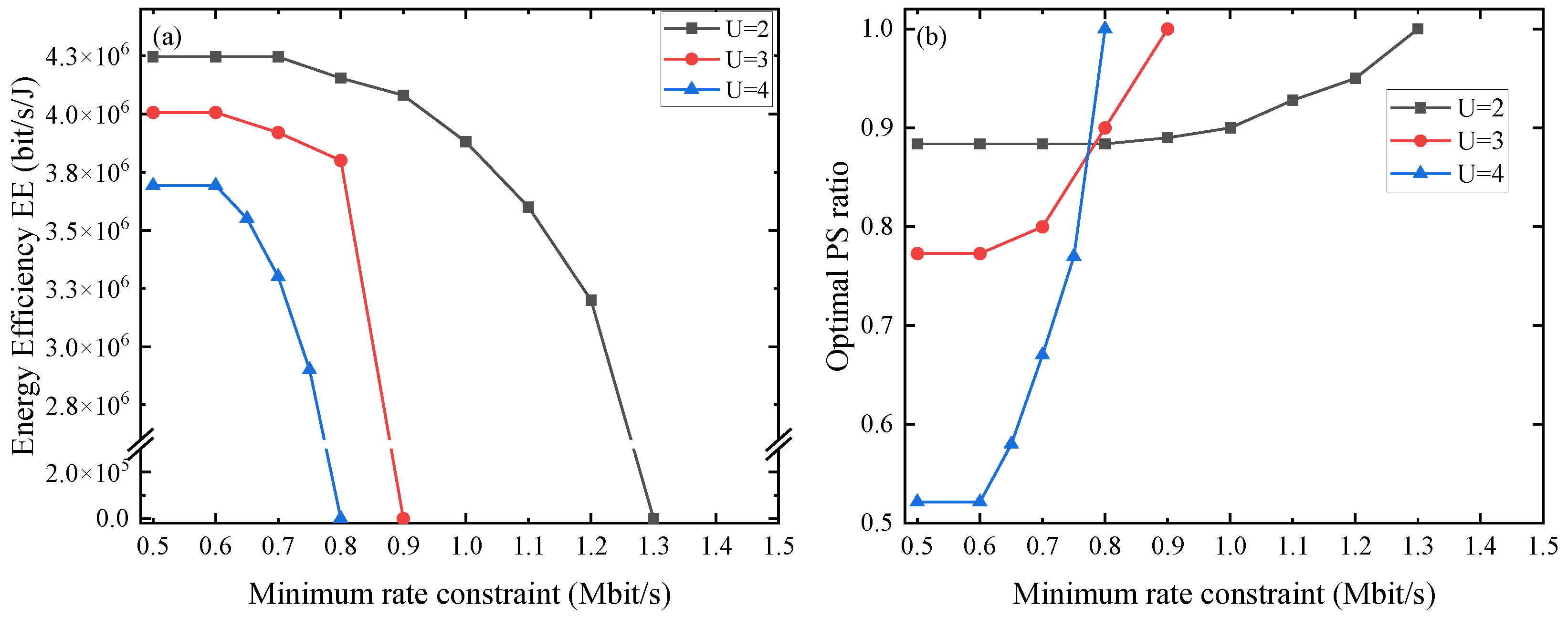
4. Results and Discussions
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Saad, W.; Bennis, M.; Chen, M. A vision of 6G wireless systems: Applications, trends, technologies, and open research problems. IEEE Netw. 2019, 34, 134–142. [Google Scholar] [CrossRef]
- Truitt, A.; Mahmoodi, S.N. A review on active wind energy harvesting designs. Int. J. Precis. Eng. Manuf. 2013, 14, 1667–1675. [Google Scholar] [CrossRef]
- Hao, D.; Qi, L.; Tairab, A.M.; Ahmed, A.; Azam, A.; Luo, D.; Pan, Y.; Zhang, Z.; Yan, J. Solar energy harvesting technologies for PV self-powered applications: A comprehensive review. Renew. Energy 2022, 188, 678–697. [Google Scholar] [CrossRef]
- Qian, H. Design of Forest IoT Monitoring Nodes Based on Energy Harvesting Technology. Master’s Thesis, Nanjing University of Information Engineering, Nanjing, China, 2022. [Google Scholar]
- Zhang, R.; Ho, C.K. MIMO broadcasting for simultaneous wireless information and power transfer. IEEE Trans. Wirel. Commun. 2013, 12, 1989–2001. [Google Scholar] [CrossRef]
- Abuella, H.; Elamassie, M.; Uysal, M.; Xu, Z.; Serpedin, E.; Qaraqe, K.A.; Ekin, S. Hybrid RF/VLC systems: A comprehensive survey on network topologies, performance analyses, applications, and future directions. IEEE Access 2021, 9, 160402–160436. [Google Scholar] [CrossRef]
- Chi, N.; Zhou, Y.; Wei, Y.; Hu, F. Visible light communication in 6G: Advances, challenges, and prospects. IEEE Veh. Technol. Mag. 2020, 15, 93–102. [Google Scholar] [CrossRef]
- Diamantoulakis, P.D.; Karagiannidis, G.K. Simultaneous lightwave information and power transfer (SLIPT) for indoor IoT applications. In Proceedings of the GLOBECOM 2017 IEEE Global Communications Conference, Singapore, 4–8 December 2017; IEEE: New York, NY, USA, 2017; pp. 1–6. [Google Scholar]
- Abdelhady, A.M.; Amin, O.; Chaaban, A.; Alouini, M.S. Resource allocation for outdoor visible light communications with energy harvesting capabilities. In Proceedings of the 2017 IEEE Globecom Workshops (GC Wkshps), Singapore, 4–8 December 2017; IEEE: New York, NY, USA, 2017; pp. 1–6. [Google Scholar]
- Ye, K.; Wang, T.; Yang, F. Rate optimization for relaying VLC system with simultaneous lightwave information and power transfer. Opt. Express 2021, 29, 2184–2192. [Google Scholar] [CrossRef] [PubMed]
- Wang, Z.; Tsonev, D.; Videv, S.; Haas, H. On the design of a solar-panel receiver for optical wireless communications with simultaneous energy harvesting. IEEE J. Sel. Areas Commun. 2015, 33, 1612–1623. [Google Scholar] [CrossRef]
- Pan, G.; Diamantoulakis, P.D.; Ma, Z.; Ding, Z.; Karagiannidis, G.K. Simultaneous lightwave information and power transfer: Policies, techniques, and future directions. IEEE Access 2019, 7, 28250–28257. [Google Scholar] [CrossRef]
- Wang, H.; Wang, F.; Li, R. Enhancing power allocation efficiency of NOMA aided-MIMO downlink VLC networks. Opt. Commun. 2020, 454, 124497. [Google Scholar] [CrossRef]
- Chen, Z.; Ding, Z.; Dai, X.; Zhang, R. An optimization perspective of the superiority of NOMA compared to conventional OMA. IEEE Trans. Signal Process. 2017, 65, 5191–5202. [Google Scholar] [CrossRef]
- Sung, J.Y.; Yeh, C.H.; Chow, C.W.; Lin, W.F.; Liu, Y. Orthogonal frequency-division multiplexing access (OFDMA) based wireless visible light communication (VLC) system. Opt. Commun. 2015, 355, 261–268. [Google Scholar] [CrossRef]
- Anzagira, A.; Edmonson, W. Non-orthogonal multiple access (NOMA) for LED-based visible light inter-satellite communications. In Proceedings of the 2018 6th IEEE International Conference on Wireless for Space and Extreme Environments (WiSEE), Huntsville, AL, USA, 11–13 December 2018; IEEE: New York, NY, USA, 2018; pp. 24–29. [Google Scholar]
- Marshoud, H.; Kapinas, V.M.; Karagiannidis, G.K.; Muhaidat, S. Non-orthogonal multiple access for visible light communications. IEEE Photonics Technol. Lett. 2015, 28, 51–54. [Google Scholar] [CrossRef]
- Tennakoon, P.; Jayakody, D.N.K.; Affes, S. Simultaneous Lightwave Information and Power Transfer with Non-orthogonal Multiple Access. In Proceedings of the 2021 10th International Conference on Information and Automation for Sustainability (ICIAfS), Negambo, Sri Lanka, 11–13 August 2021; IEEE: New York, NY, USA, 2021; pp. 214–219. [Google Scholar]
- de Oliveira Filho, J.I.; Trichili, A.; Ooi, B.S.; Alouini, M.S.; Salama, K.N. Toward self-powered internet of underwater things devices. IEEE Commun. Mag. 2020, 58, 68–73. [Google Scholar] [CrossRef]
- Obeed, M.; Dahrouj, H.; Salhab, A.M.; Zummo, S.A.; Alouini, M.S. DC-bias and power allocation in cooperative VLC networks for joint information and energy transfer. IEEE Trans. Wirel. Commun. 2019, 18, 5486–5499. [Google Scholar] [CrossRef]
- Komine, T.; Nakagawa, M. Fundamental analysis for visible-light communication system using LED lights. IEEE Trans. Consum. Electron. 2004, 50, 100–107. [Google Scholar] [CrossRef]
- Kizilirmak, R.C.; Rowell, C.R.; Uysal, M. Non-orthogonal multiple access (NOMA) for indoor visible light communications. In Proceedings of the 2015 4th International Workshop on Optical Wireless Communications (IWOW), Istanbul, Turkey, 7–8 September 2015; IEEE: New York, NY, USA, 2015; pp. 98–101. [Google Scholar]
- Li, C.; Jia, W.; Tao, Q.; Sun, M. Solar cell phone charger performance in indoor environment. In Proceedings of the 2011 IEEE 37th Annual Northeast Bioengineering Conference (NEBEC), Troy, NY, USA, 1–3 April 2011; IEEE: New York, NY, USA, 2011; pp. 1–2. [Google Scholar]
- Rakia, T.; Yang, H.C.; Gebali, F.; Alouini, M.S. Dual-hop VLC/RF transmission system with energy harvesting relay under delay constraint. In Proceedings of the 2016 IEEE Globecom Workshops (GC Wkshps), Washington, DC, USA, 4–8 December 2016; IEEE: New York, NY, USA, 2016; pp. 1–6. [Google Scholar]
- Masaracchia, A.; Da Costa, D.B.; Duong, T.Q.; Nguyen, M.N.; Nguyen, M.T. A PSO-based approach for user-pairing schemes in NOMA systems: Theory and applications. IEEE Access 2019, 7, 90550–90564. [Google Scholar] [CrossRef]
- Hasan, I.; Shamshiri, M.; Gan, C.K.; Ghani, M.; Yusoff, M. Using Particle Swarm Optimization Algorithm in the Distribution System Planning. Aust. J. Basic Appl. Sci. 2013, 7, 85–92. [Google Scholar]





| Parameters | Symbols | Values |
|---|---|---|
| Bandwidth (Hz) | ||
| Maximum DC bias (mA) | 12 | |
| Minimum DC bias (mA) | 0 | |
| Semi-angle of half power () | 60 | |
| Thermal voltage (mV) | 25 | |
| Dark saturation current (nA) | 0.1 | |
| Physical area of solar panel (cm ) | A | 4 |
| Thermal voltage (mV) | 25 | |
| Power of noise (W) | ||
| Responsivity (A/W) | 1.5 | |
| Fill factor | f | 0.75 |
| Height of room(m) | H | 3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, D.; Wang, Q.; Wang, J.; Li, Z.; Wu, S.; Hao, R.; Fan, K.; Lu, H.; Jin, J. Energy Efficiency Optimization for SLIPT-Enabled NOMA System. Photonics 2023, 10, 791. https://doi.org/10.3390/photonics10070791
Chen D, Wang Q, Wang J, Li Z, Wu S, Hao R, Fan K, Lu H, Jin J. Energy Efficiency Optimization for SLIPT-Enabled NOMA System. Photonics. 2023; 10(7):791. https://doi.org/10.3390/photonics10070791
Chicago/Turabian StyleChen, Danyang, Qingxuan Wang, Jianping Wang, Zhao Li, Shuai Wu, Rui Hao, Kai Fan, Huimin Lu, and Jianli Jin. 2023. "Energy Efficiency Optimization for SLIPT-Enabled NOMA System" Photonics 10, no. 7: 791. https://doi.org/10.3390/photonics10070791
APA StyleChen, D., Wang, Q., Wang, J., Li, Z., Wu, S., Hao, R., Fan, K., Lu, H., & Jin, J. (2023). Energy Efficiency Optimization for SLIPT-Enabled NOMA System. Photonics, 10(7), 791. https://doi.org/10.3390/photonics10070791





