A Review of Variable-Beam Divergence Angle FSO Communication Systems
Abstract
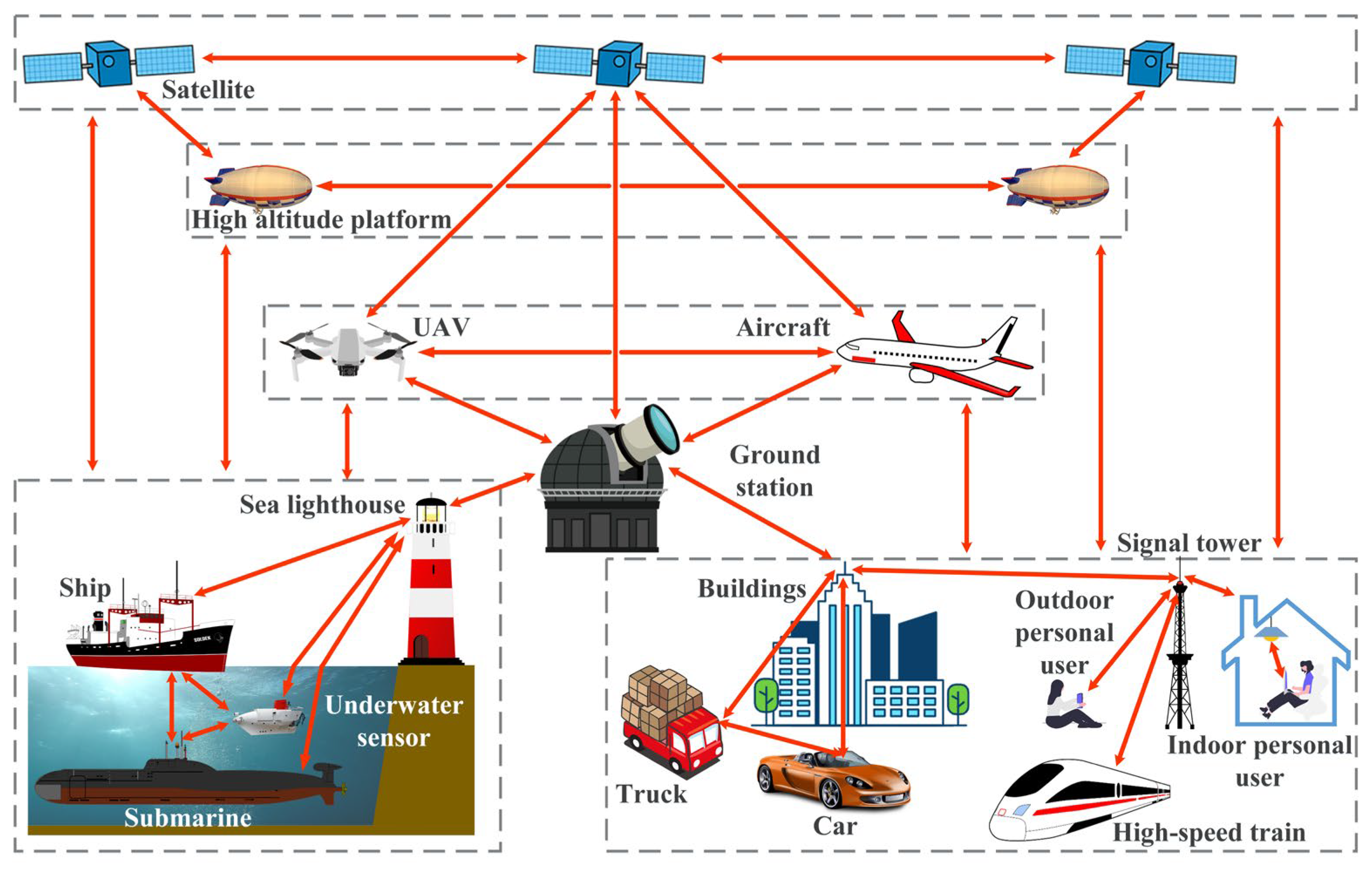
1. Introduction
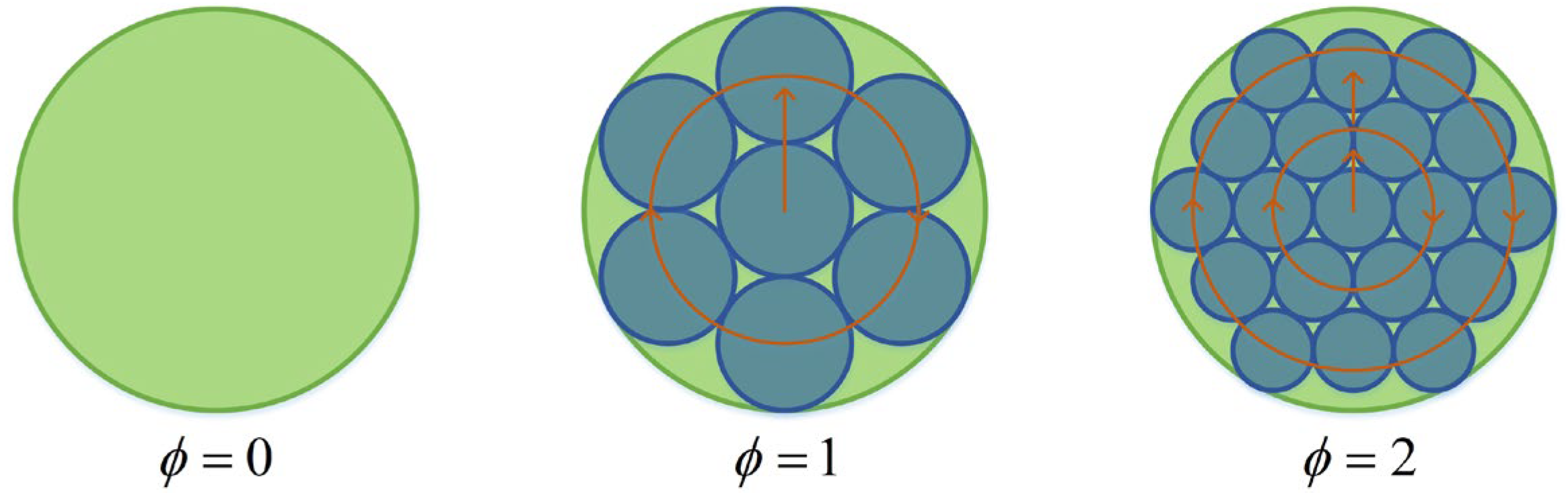
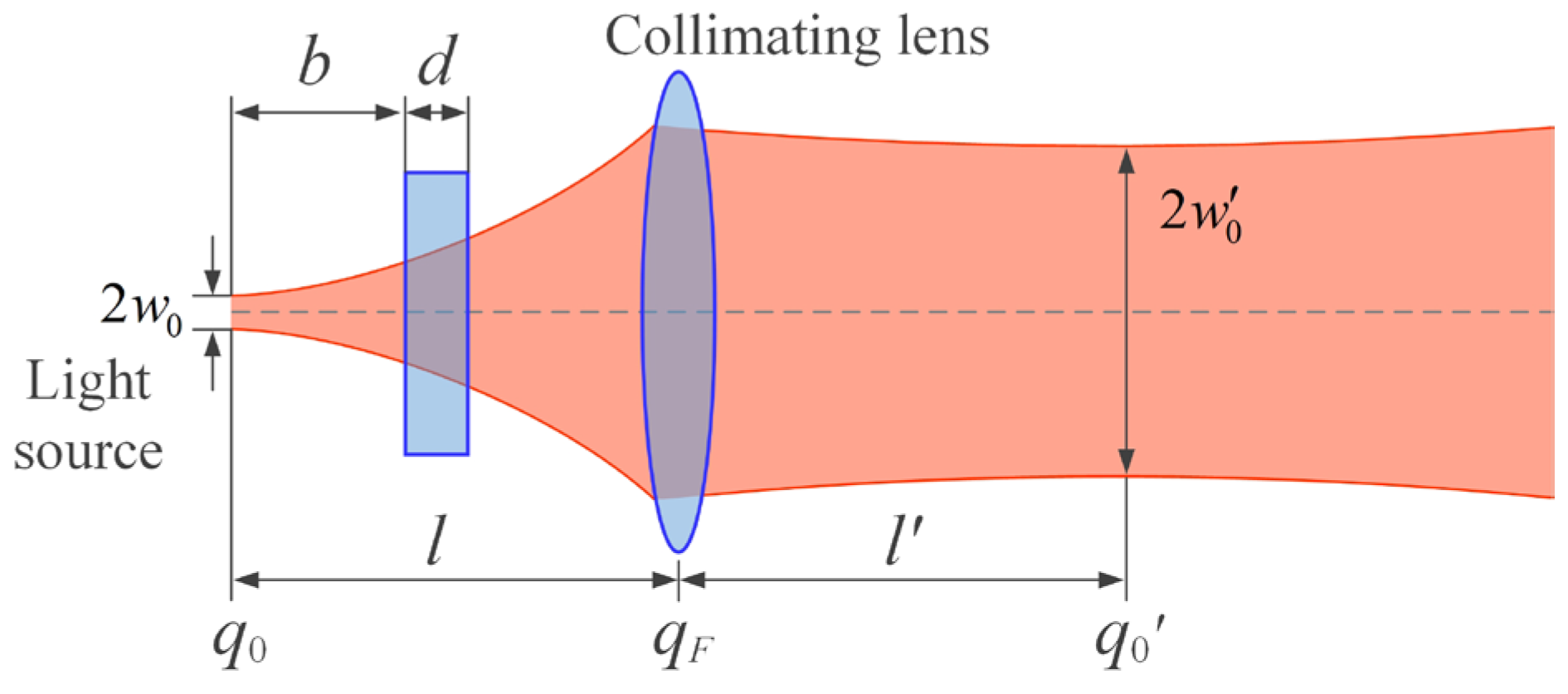
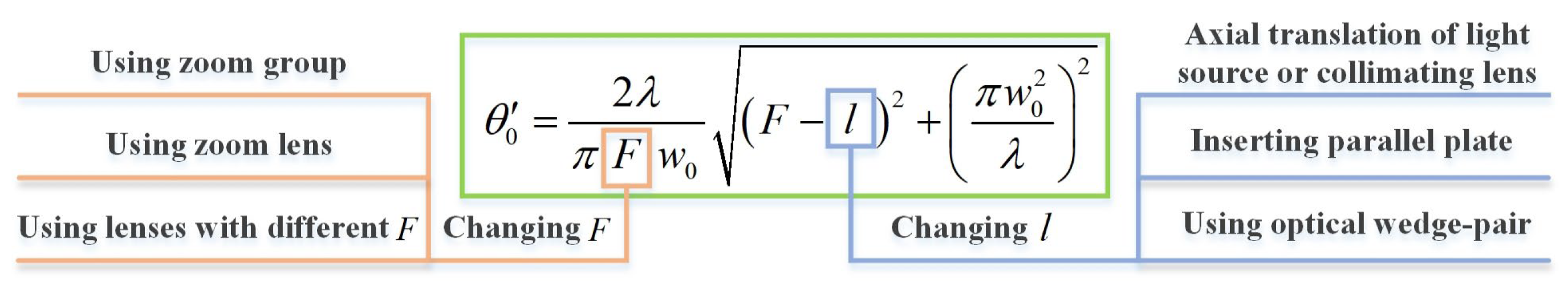
2. Optical Principle of VBDA Method
3. Existing Methods for VBDA
3.1. Vary Beam Divergence by Adjusting the Focal Length F of the Collimating Lens
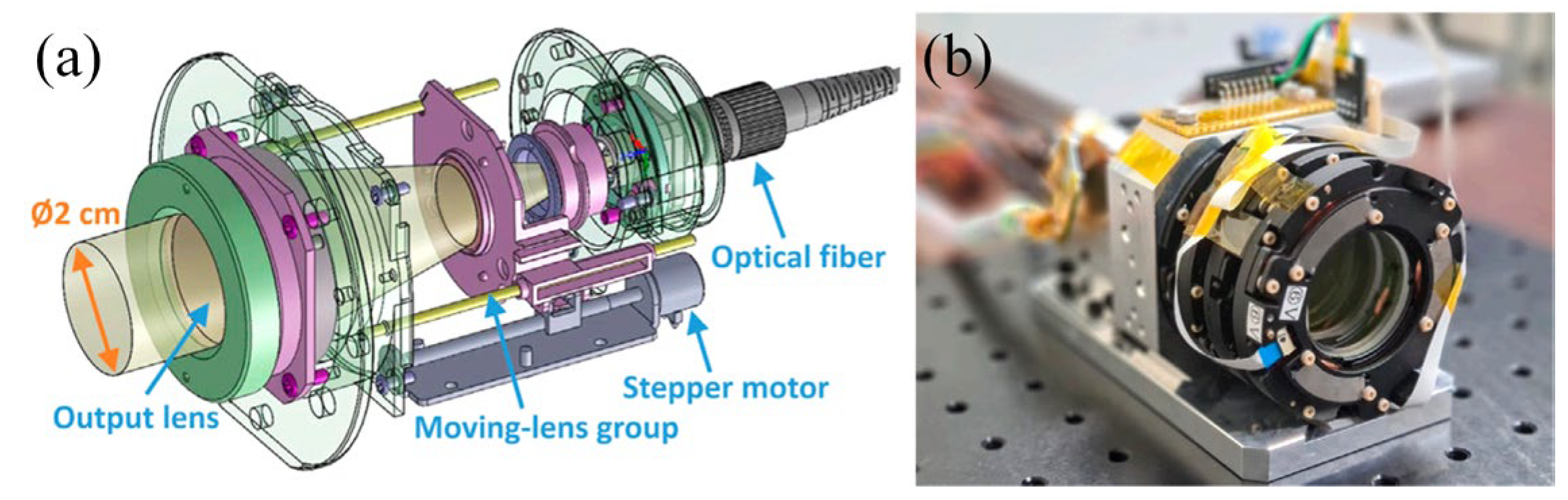
3.1.1. Using Zoom Group
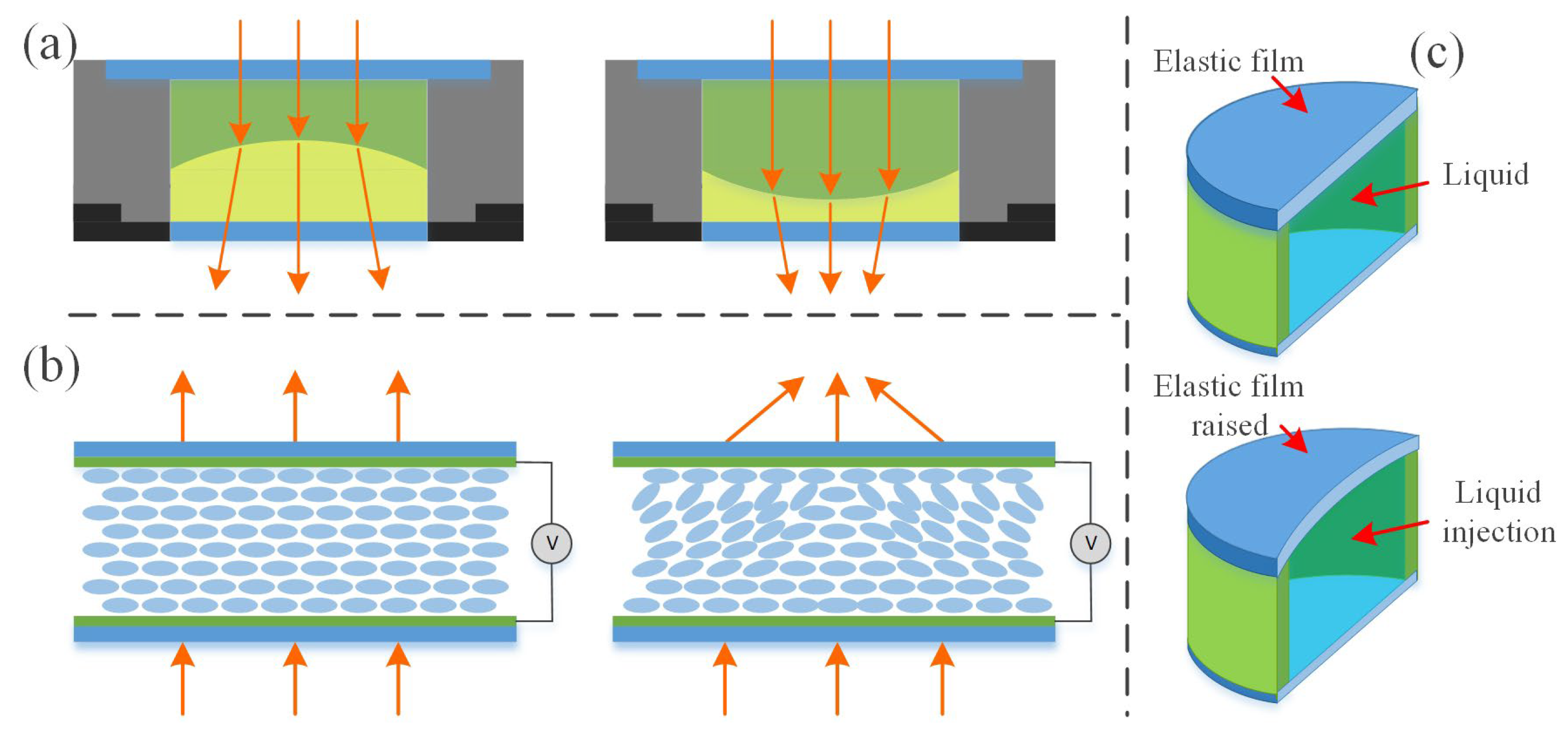
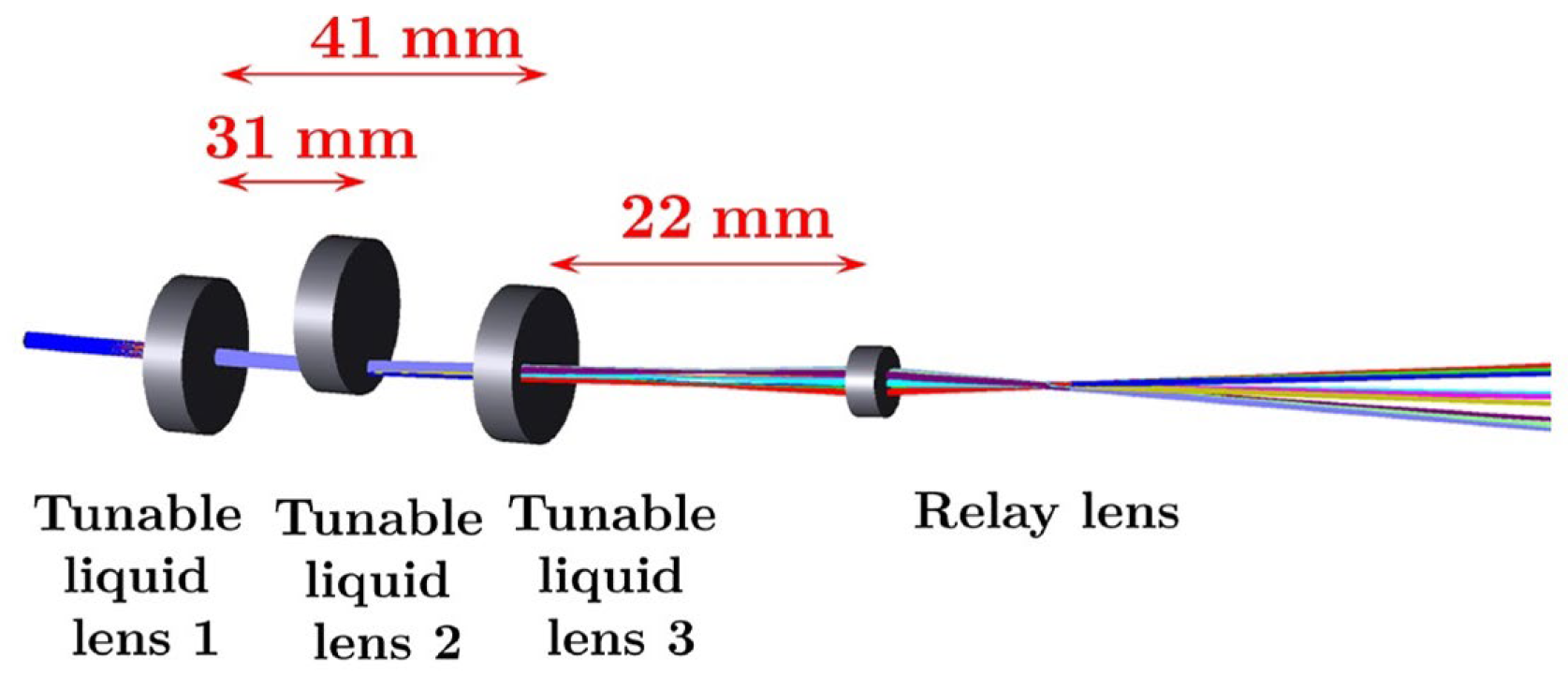
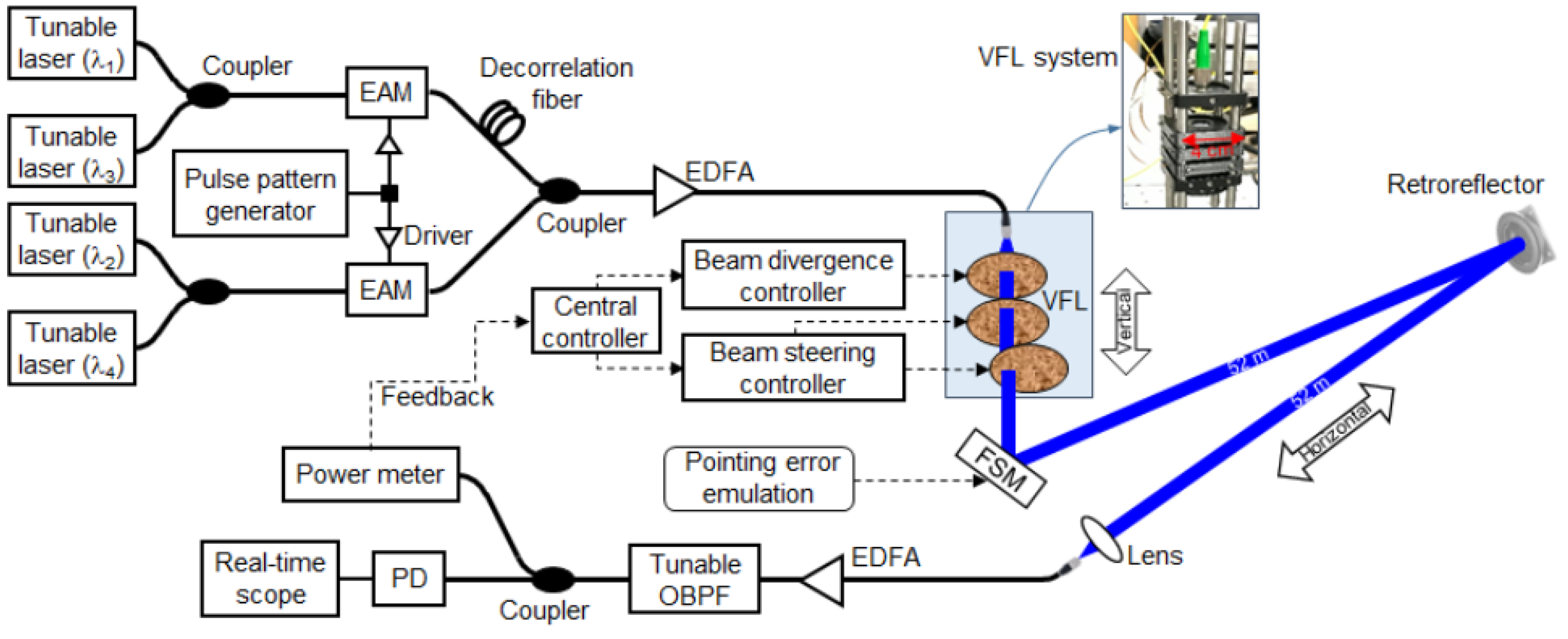
3.1.2. Using Zoom Lens
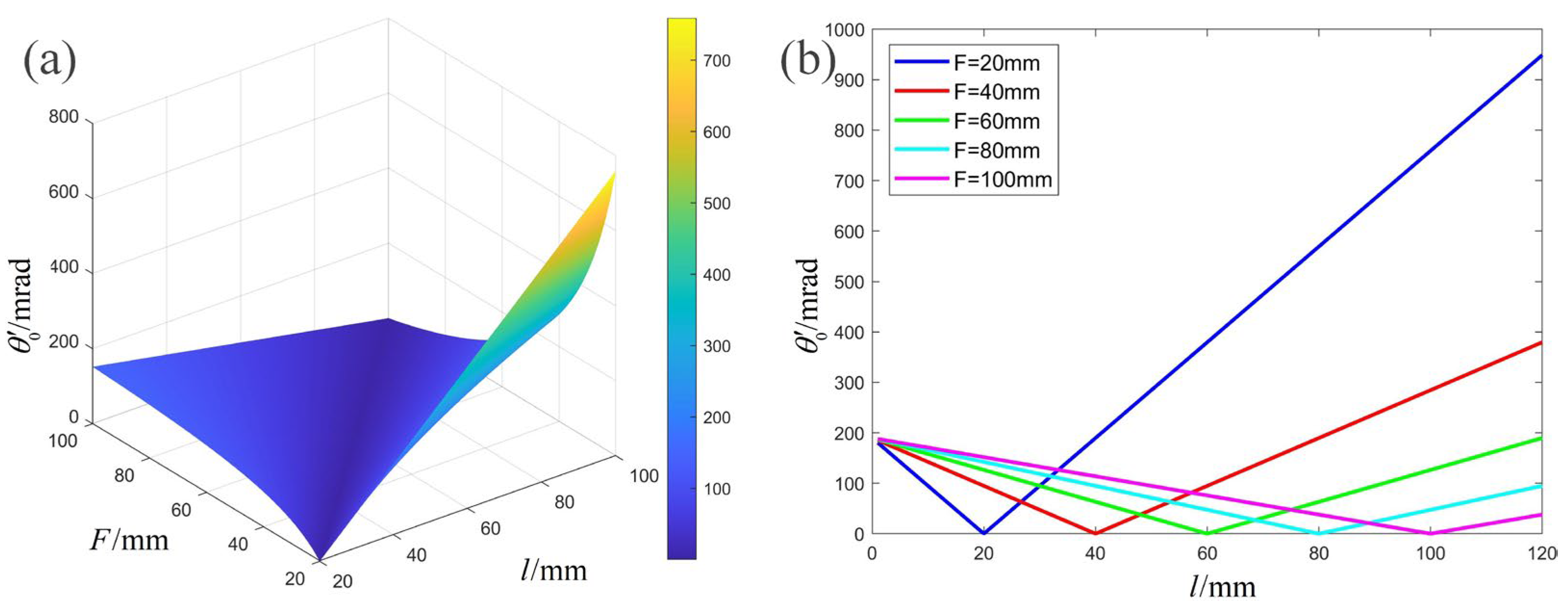
3.1.3. Using Lens with Different F
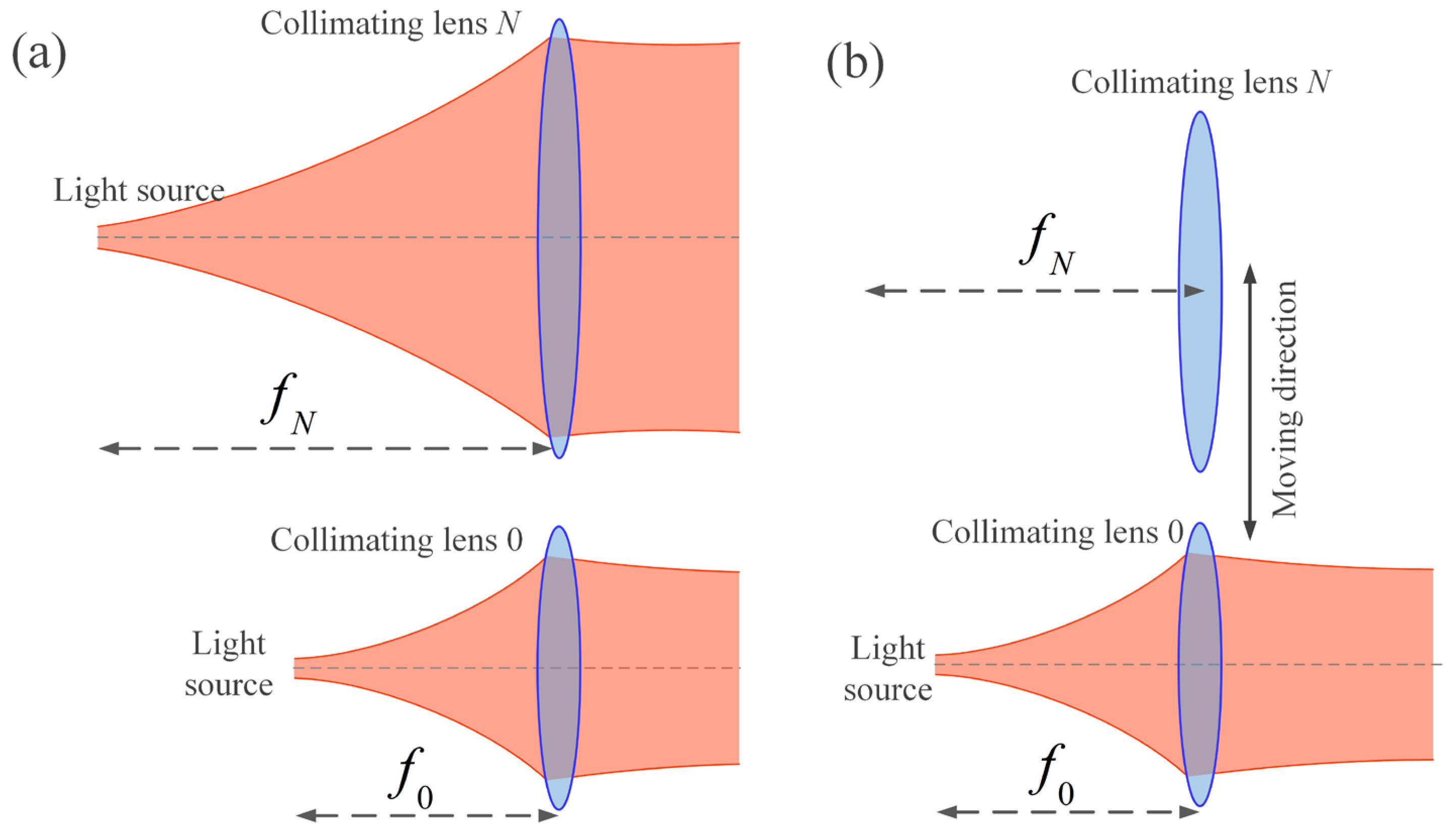
3.2. Vary Beam Divergence by Adjusting the Optical Path Length between the Beam Waist and the Collimating Lens
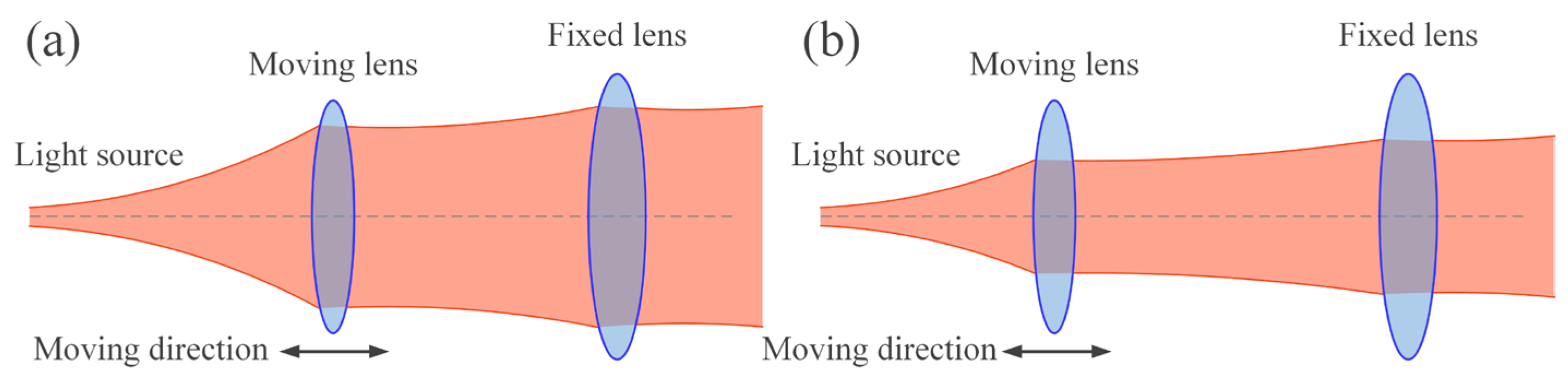
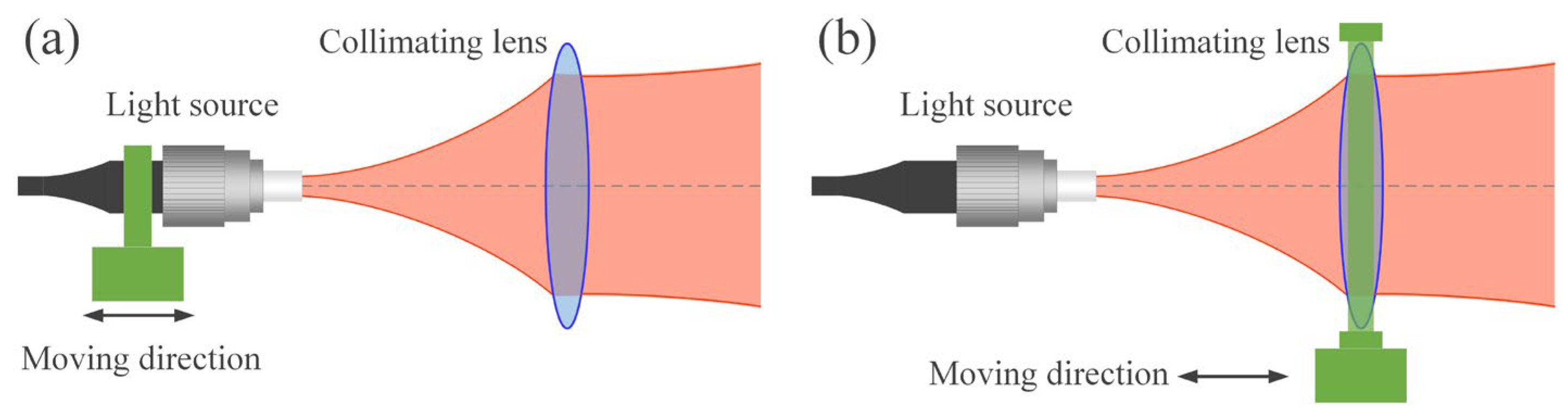
3.2.1. Axial Translation of Light Source or Collimating Lens
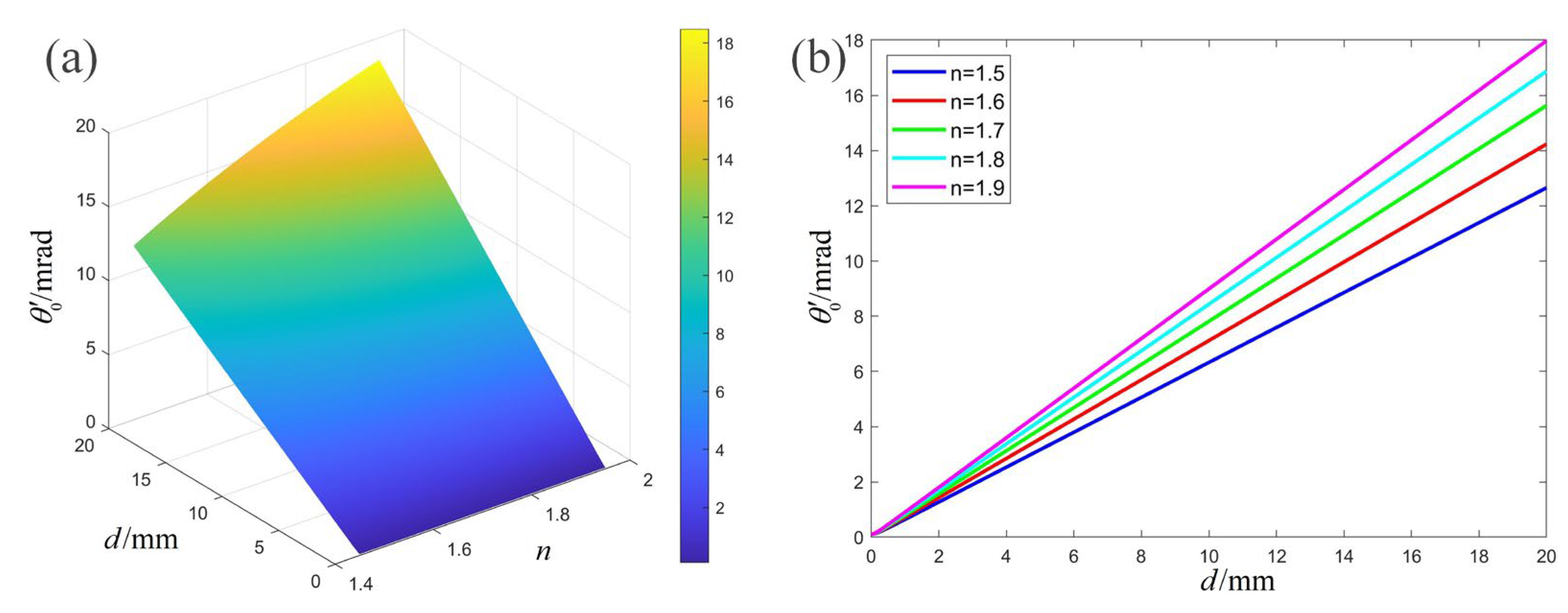
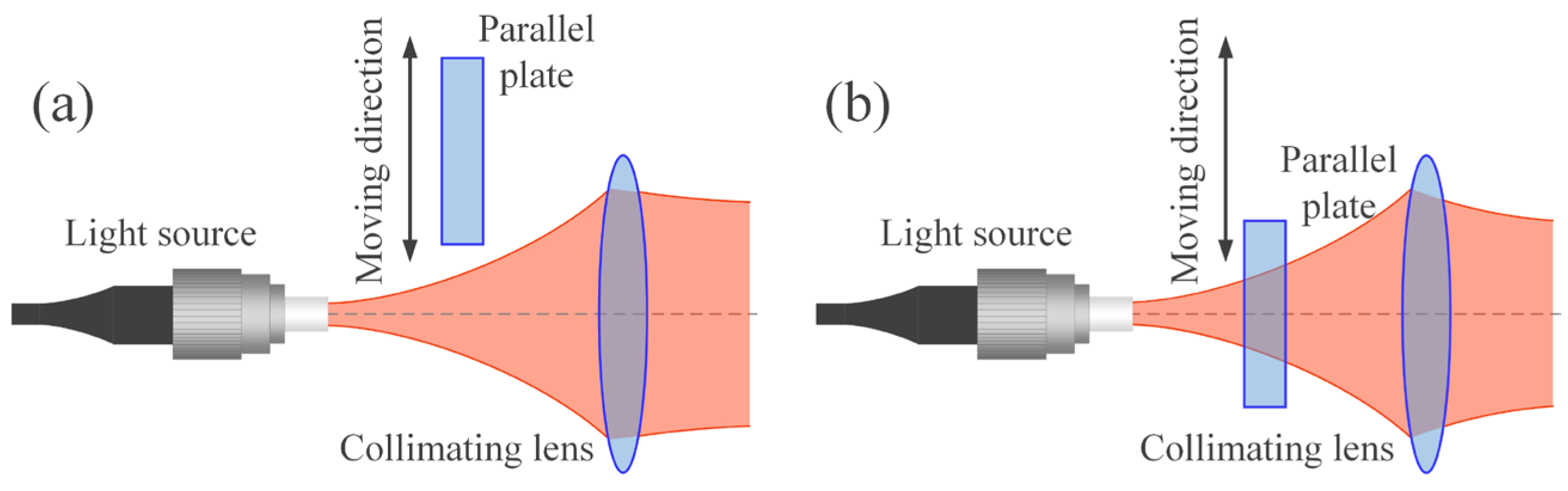
3.2.2. Inserting Parallel Plate
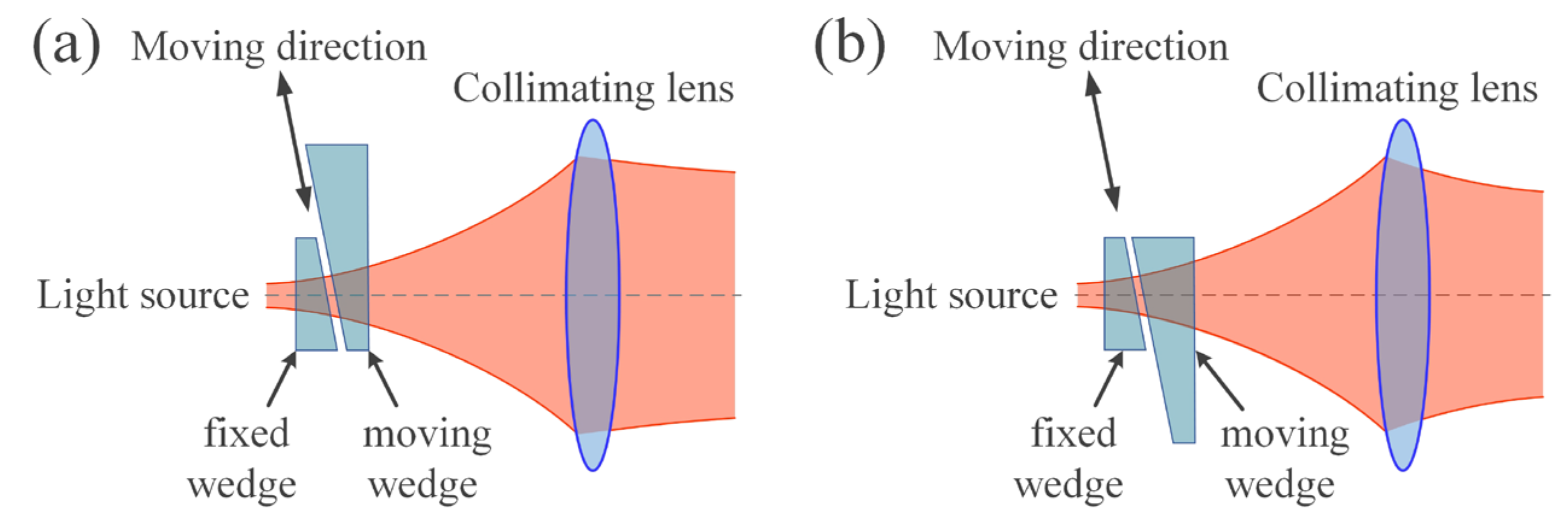
3.2.3. Using Optical Wedge Pair
4. Challenges and Future Work
4.1. Reduce the Computational Cost of OBDA
- Algorithm Optimization: Use different algorithms of varying complexity to compute the OBDA for different types of terminals. Large communication terminals, such as ground stations, can use relatively complex and advanced OBDA algorithms due to their high tolerance for high computing costs. For remote small units, relatively simple OBDA algorithms can be used, and the computational accuracy can be appropriately reduced to alleviate the computational pressure;
- Distributed Computing: Assign the task of computing the OBDA for each communication party to a node with powerful computing capabilities. And the computing results are then transmitted to the remote communication node via FSO communication or RF link. This measure could reduce the computational burden and power consumption of small communication nodes. However, it introduces additional delay. Therefore, it is essential to comprehensively consider the capabilities of terminal and relay equipment, communication environment, and application requirements to achieve optimal performance and efficiency;
- Machine Learning Techniques: Utilize machine learning to improve the accuracy and efficiency of the OBDA algorithm. By leveraging the complex relationship between the link factors and OBDA, machine learning can improve accuracy and efficiency, resulting in reduced computational costs. In addition, neural network hardware accelerators combined with field-programmable gate array (FPGA) can be reconfigured for different target applications while maintaining low power consumption. This measure leverages the ability of FPGA parallel computing to achieve low-delay processing.
4.2. Achieve Better Reliability and Environmental Adaptability
- Reducing the impact of temperature: There are two ways to approach this issue. ① Selecting materials with low thermal expansion coefficients. This helps to maintain the stability and performance of the VBDA mechanism across a wide range of temperatures. ② Adding a temperature compensation mechanism. This compensates for any thermal-induced changes, ensuring the VBDA mechanism remains within the appropriate temperature range;
- Real-time measurement of transmitted beam divergence angle: By continuously measuring the beam divergence angle in real time, feedback can be provided to the VBDA mechanism. This feedback can be adjusted immediately to compensate for changes caused by temperature fluctuations, ensuring accurate beam alignment and reliable communication;
- Performing temperature tests and creating a lookup table: Conducting temperature tests on the VBDA mechanism helps to understand its performance characteristics under different temperature conditions. Based on the results, a lookup table can be created that maps temperature variations to the corresponding correction values for the beam divergence angle. This lookup table can then be used to apply real-time corrections during operation, compensating for the effects of temperature changes.
4.3. Reduce Hardware Costs
- Modular design: Implement a modular design for the VBDA system, where different components are divided into modules that can be easily replaced when a failure occurs. This can significantly reduce the cost of maintenance and repair, improving the system’s overall maintainability. Additionally, by adding new modules, the system’s functionality can be easily extended without significantly affecting its overall design. This makes the system more adaptable and able to meet various application scenarios and requirements;
- Design for Manufacturing (DFM): The design should apply principles that prioritize ease of manufacturing and assembly. It should be simplified to reduce the number of complex parts and manufacturing steps. The use of materials should be optimized to minimize waste and lower production costs. It is important to ensure that the design is compatible with existing manufacturing processes and technologies, thereby avoiding the need for expensive production modifications;
- Utilize commercially manufactured components: Consider using commercially available components to minimize the number of unique parts needed. This can significantly reduce the costs and time associated with producing custom components. Commercial components are often mass produced and have established quality standards, which ensure the reliability and consistency of the final product.
4.4. Other Future Trends
4.4.1. To Realize UAV Swarm Communication and Positioning under Electromagnetic Suppression Conditions
4.4.2. Applied to Quantum Key Distribution (QKD)
4.4.3. Applied to MIMO
5. Conclusions
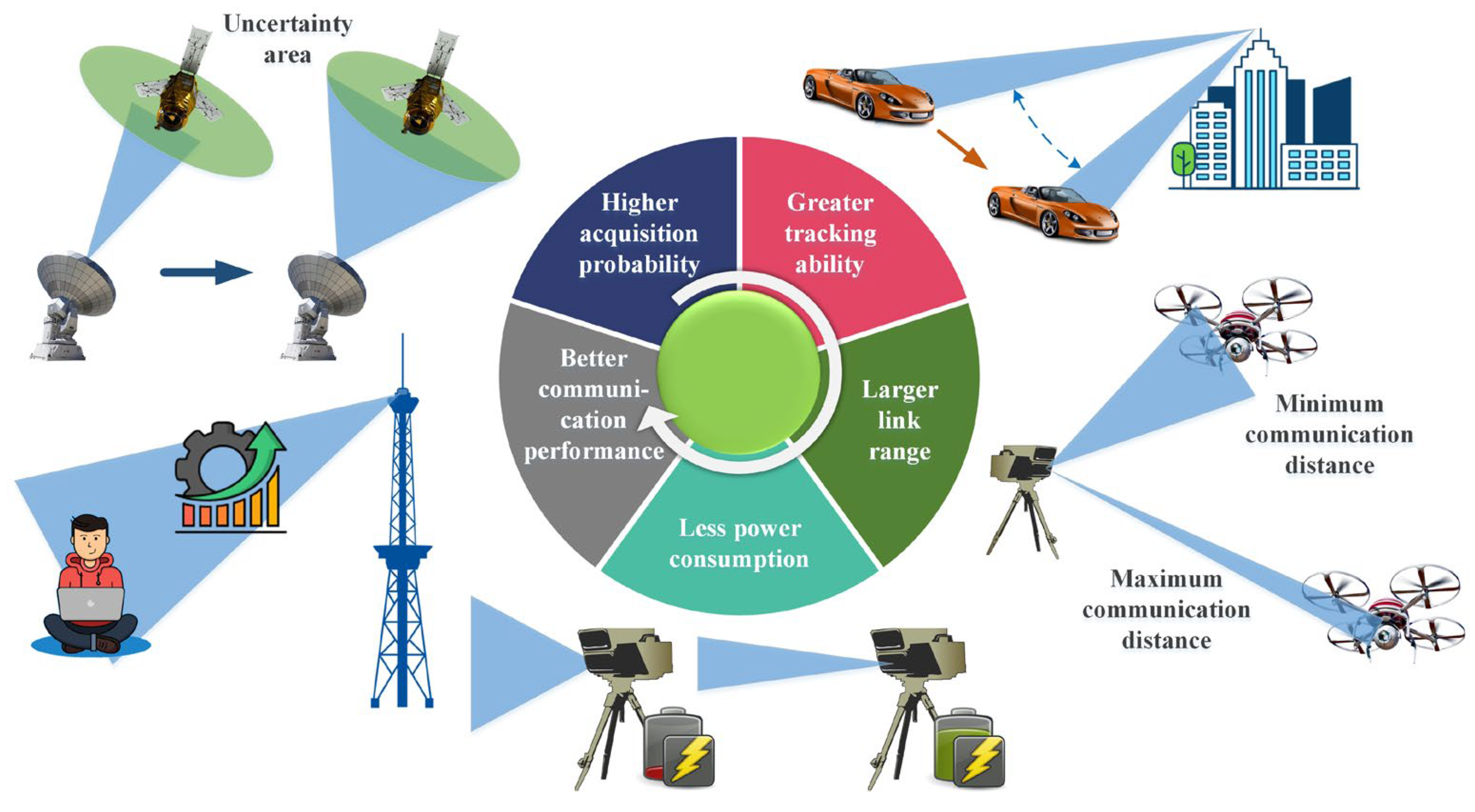
- Explanation of the application significance of VBDA technology in FSO communication, emphasizing its ability to enhance system performance in terms of higher acquisition probability, greater tracking ability, larger communication link range, and lower power consumption;
- Introduction to the transmission theory of Gaussian beams and the basic principle of the VBDA method, laying the foundation for the following discussion;
- In-depth research on different types of VBDA methods, including:
- a.
- Using zoom groups or axial translation of fiber or lens: This method has the advantage of mature zoom lens groups technology and simple principles. However, it requires high-quality guide rails and actuators;
- b.
- Using zoom lenses: This method has the advantages of having no mechanical mechanism and being small in size, but the system may be affected by gravity, and enlarging the aperture may be difficult;
- c.
- Using lenses with different F or inserting parallel plates: These methods, respectively, have the advantage of simplicity and can greatly relax the requirement for lateral positioning accuracy. However, the communication link will experience an outage when switching angles;
- d.
- Using an optical wedge-pair: The advantage is that the influence on the optical axis deflection is small. However, a trade-off must be made between the VBDA range and larger astigmatism;
- e.
- The research on using VBDA technologies for different types of links and how they can enhance performance.
- 4.
- Emphasis on the challenges faced by VBDA technology and potential future research directions.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Majumdar, A.K. Laser Communication with Constellation Satellites, UAVs, HAPs and Balloons: Fundamentals and Systems Analysis for Global Connectivity; Springer International Publishing: Cham, Switzerland, 2022; p. 48. ISBN 978-3-031-03971-3. [Google Scholar]
- Alraih, S.; Shayea, I.; Behjati, M.; Nordin, R.; Abdullah, N.F.; Abu-Samah, A.; Nandi, D. Revolution or Evolution? Technical Requirements and Considerations towards 6G Mobile Communications. Sensors 2022, 22, 762. [Google Scholar] [CrossRef] [PubMed]
- Jahid, A.; Alsharif, M.H.; Hall, T.J. A contemporary survey on free space optical communication: Potentials, technical challenges, recent advances and research direction. J. Netw. Comput. Appl. 2022, 200, 103311. [Google Scholar] [CrossRef]
- Al-Gailani, S.A.; Mohd Salleh, M.F.; Salem, A.A.; Shaddad, R.Q.; Sheikh, U.U.; Algeelani, N.A.; Almohamad, T.A. A Survey of Free Space Optics (FSO) Communication Systems, Links, and Networks. IEEE Access 2020, 9, 7353–7373. [Google Scholar] [CrossRef]
- Chowdhury, M.Z.; Hossan, M.T.; Islam, A.; Jang, Y.M. A Comparative Survey of Optical Wireless Technologies: Architectures and Applications. IEEE Access 2018, 6, 9819–9840. [Google Scholar] [CrossRef]
- Mohsan, S.A.H.; Khan, M.A.; Amjad, H. Hybrid FSO/RF networks: A review of practical constraints, applications and challenges. Opt. Switch. Netw. 2023, 47, 100697. [Google Scholar] [CrossRef]
- Ali, M.H.; Ajel, R.I.; Abdul-Kader Hussain, S. Performance analysis of beam divergence propagation through rainwater and snow pack in free space optical communication. Bull. Electr. Eng. Inform. 2021, 10, 1395–1404. [Google Scholar] [CrossRef]
- Laycock, L.; Rowe, D.P.; Williams, A.J.; Griffith, M.S.; McCarthy, A.G. Acquisition and tracking for underwater optical communications. In Advanced Free-Space Optical Communication Techniques and Applications III; SPIE: Warsaw, Poland, 2017; p. 1043707. [Google Scholar] [CrossRef]
- Harada, R.; Shibata, N.; Kaneko, S.; Imai, T.; Kani, J.-I.; Yoshida, T. Adaptive Beam Divergence for Expanding Range of Link Distance in FSO with Moving Nodes toward 6G. IEEE Photonics Technol. Lett. 2022, 34, 1061–1064. [Google Scholar] [CrossRef]
- Heng, K.H.; Zhong, W.-D.; Cheng, T.H.; Liu, N.; He, Y. Beam divergence changing mechanism for short-range inter-unmanned aerial vehicle optical communications. Appl. Opt. 2009, 48, 1565–1572. [Google Scholar] [CrossRef]
- Trinh, P.V.; Carrasco-Casado, A.; Okura, T.; Tsuji, H.; Kolev, D.R.; Shiratama, K.; Munemasa, Y.; Toyoshima, M. Experimental Channel Statistics of Drone-to-Ground Retro-Reflected FSO Links with Fine-Tracking Systems. IEEE Access 2021, 9, 137148–137164. [Google Scholar] [CrossRef]
- Ansari, I.S.; Al-Ahmadi, S.; Yilmaz, F.; Alouini, M.-S.; Yanikomeroglu, H. A New Formula for the BER of Binary Modulations with Dual-Branch Selection over Generalized-K Composite Fading Channels. IEEE Trans. Commun. 2011, 59, 2654–2658. [Google Scholar] [CrossRef]
- Sandalidis, H.G.; Tsiftsis, T.A.; Karagiannidis, G.K.; Uysal, M. BER Performance of FSO Links over Strong Atmospheric Turbulence Channels with Pointing Errors. IEEE Commun. Lett. 2008, 12, 44–46. [Google Scholar] [CrossRef]
- Dabiri, M.T.; Sadough, S.M.S.; Khalighi, M.A. Channel Modeling and Parameter Optimization for Hovering UAV-Based Free-Space Optical Links. IEEE J. Sel. Areas Commun. 2018, 36, 2104–2113. [Google Scholar] [CrossRef]
- Navidpour, S.M.; Uysal, M.; Kavehrad, M. BER Performance of Free-Space Optical Transmission with Spatial Diversity. IEEE Trans. Wirel. Commun. 2007, 6, 2813–2819. [Google Scholar] [CrossRef]
- Zedini, E.; Ansari, I.S.; Alouini, M.-S. Performance Analysis of Mixed Nakagami- m and Gamma–Gamma Dual-Hop FSO Transmission Systems. IEEE Photonics J. 2014, 7, 2381657. [Google Scholar] [CrossRef]
- Zedini, E.; Soury, H.; Alouini, M.-S. Dual-Hop FSO Transmission Systems Over Gamma–Gamma Turbulence with Pointing Errors. IEEE Trans. Wirel. Commun. 2016, 16, 784–796. [Google Scholar] [CrossRef]
- Ansari, I.S.; Alouini, M.-S.; Yilmaz, F. A Unified Performance Analysis of Free-Space Optical Links over Gamma-Gamma Turbulence Channels with Pointing Errors. 2013. Available online: https://eprints.gla.ac.uk/171230/ (accessed on 8 June 2023).
- Ding, J.; Xie, X.; Wang, L.; Tan, L.; Ma, J.; Kang, D. Performance of dual-hop FSO/RF systems with fixed-gain relaying over Fisher–Snedecor F and κ-μ shadowed fading channels. Appl. Opt. 2022, 61, 2079. [Google Scholar] [CrossRef]
- Xu, G.; Zhang, N.; Xu, M.; Xu, Z.; Zhang, Q.; Song, Z. Outage Probability and Average BER of UAV-assisted Dual-hop FSO Communication with Amplify-and-Forward Relaying. IEEE Trans. Veh. Technol. 2023, 1–16. [Google Scholar] [CrossRef]
- Singh, D.; Swaminathan, R. Comprehensive Performance Analysis of Hovering UAV-Based FSO Communication System. IEEE Photonics J. 2022, 14, 3205704. [Google Scholar] [CrossRef]
- Farid, A.A.; Hranilovic, S. Outage Capacity Optimization for Free-Space Optical Links with Pointing Errors. J. Light Technol. 2007, 25, 1702–1710. [Google Scholar] [CrossRef]
- Zhang, K.; Zhu, B.; Zhang, Z.; Wang, H. Tracking System for Fast Moving Nodes in Optical Mobile Communication and the Design Rules. IEEE Trans. Wirel. Commun. 2020, 20, 2716–2728. [Google Scholar] [CrossRef]
- Sandalidis, H.G.; Tsiftsis, T.A.; Karagiannidis, G.K. Optical Wireless Communications with Heterodyne Detection Over Turbulence Channels with Pointing Errors. J. Light Technol. 2009, 27, 4440–4445. [Google Scholar] [CrossRef]
- Song, T.; Wang, Q.; Wu, M.-W.; Kam, P.-Y. Performance of laser inter-satellite links with dynamic beam waist adjustment. Opt. Express 2016, 24, 11950–11960. [Google Scholar] [CrossRef] [PubMed]
- Mai, V.V.; Kim, H. Adaptive beam control techniques for airborne free-space optical communication systems. Appl. Opt. 2018, 57, 7462–7471. [Google Scholar] [CrossRef] [PubMed]
- Mitsev, T.; Kovachev, Y. Availability of Free-Space Optical Systems Depending on Atmospheric Conditions and System Parameters. Int. J. Wirel. Commun. Netw. Technol. 2016, 5, 21–27. [Google Scholar]
- Ma, J.; Ma, L.; Yang, Q.; Ran, Q. Statistical model of the efficiency for spatial light coupling into a single-mode fiber in the presence of atmospheric turbulence. Appl. Opt. 2015, 54, 9287–9293. [Google Scholar] [CrossRef]
- Wu, J.B.; Chen, Y.S.; Gao, S.J.; Wu, Z.Y. High precision spot position detection model for the near infrared light. Infrared Laser Eng. 2016, 45, 0717001:1–0717001:7. (In Chinese) [Google Scholar]
- Li, L.; Geng, T.; Wang, Y.; Li, X.; Wu, J.; Li, Y.; Ma, S.; Gao, S.; Wu, Z. Free-Space Optical Communication Using Coherent Detection and Double Adaptive Detection Thresholds. IEEE Photonics J. 2018, 11, 2885542. [Google Scholar] [CrossRef]
- Ma, L.; Gao, S.; Chen, B.; Liu, Y. Theoretical and Experimental Analysis on Statistical Properties of Coupling Efficiency for Single-Mode Fiber in Free-Space Optical Communication Link Based on Non-Kolmogorov Turbulence. Appl. Sci. 2022, 12, 6075. [Google Scholar] [CrossRef]
- Li, Y.-T.; Geng, T.-W.; Gao, S.-J. Likelihood based synchronization algorithms in optical pulse position modulation systems with photon-counting receivers. Opt. Express 2022, 30, 31472. [Google Scholar] [CrossRef]
- Gao, S.J.; Li, Y.T.; Geng, T.W. Deep Reinforcement Learning-Based Relay Selection Algorithm in Free-Space Optical Cooperative Communications. Appl. Sci. 2022, 12, 4881. [Google Scholar] [CrossRef]
- Li, Y.T.; Geng, T.W.; Gao, S.J. Improve the Throughput of M-to-1 Free-Space Optical Systems by Employing Uniquely Decodable Codes. Chin. Opt. Lett. 2023, 21, 030603.35. [Google Scholar] [CrossRef]
- Zhou, B.K.; Gao, Y.Z.; Chen, T.R.; Chen, J.H. Laser Principle, 7th ed.; Defense Industry Press: Beijing, China, 2014; pp. 70–96. ISBN 978-7-118-09665-1. (In Chinese) [Google Scholar]
- Han, K.K. Study of Optical System for Divergence Angle Changed of The System on Laser Radar Cross Section. Master’s Thesis, Xidian University, Xian, China, 2014. (In Chinese). [Google Scholar]
- Ma, J.J.; Sun, H.; Zhu, H.T.; Guo, J.; Jiang, P.; Wei, M.; Wu, Y.M.; Deng, C.; Wang, C.; Long, Y.Z. Wireless Light Communication Antenna Variable-Divergence Angle Light Beam Transmitting Device. CN Patent 106772809A, 31 May 2017. (In Chinese). [Google Scholar]
- Yang, Y.L.; Liu, B.; Zhang, W.M. Design and Simulation of Divergence Angle of Atmospheric Laser Communication System. Semicond. Optoelectron. 2018, 39, 294–297+304. (In Chinese) [Google Scholar] [CrossRef]
- Xie, X.L.; Lu, G.Y.; Wen, L.K.; Xue, J.Y.; Wang, W.Z.; Ding, R.W.; Luo, T.; Zhang, Q.Y. Method for Controlling Beam Divergence Angle, Laser Transmitting System, Terminal and Storage Medium. CN Patent 115242305A, 25 October 2022. (In Chinese). [Google Scholar]
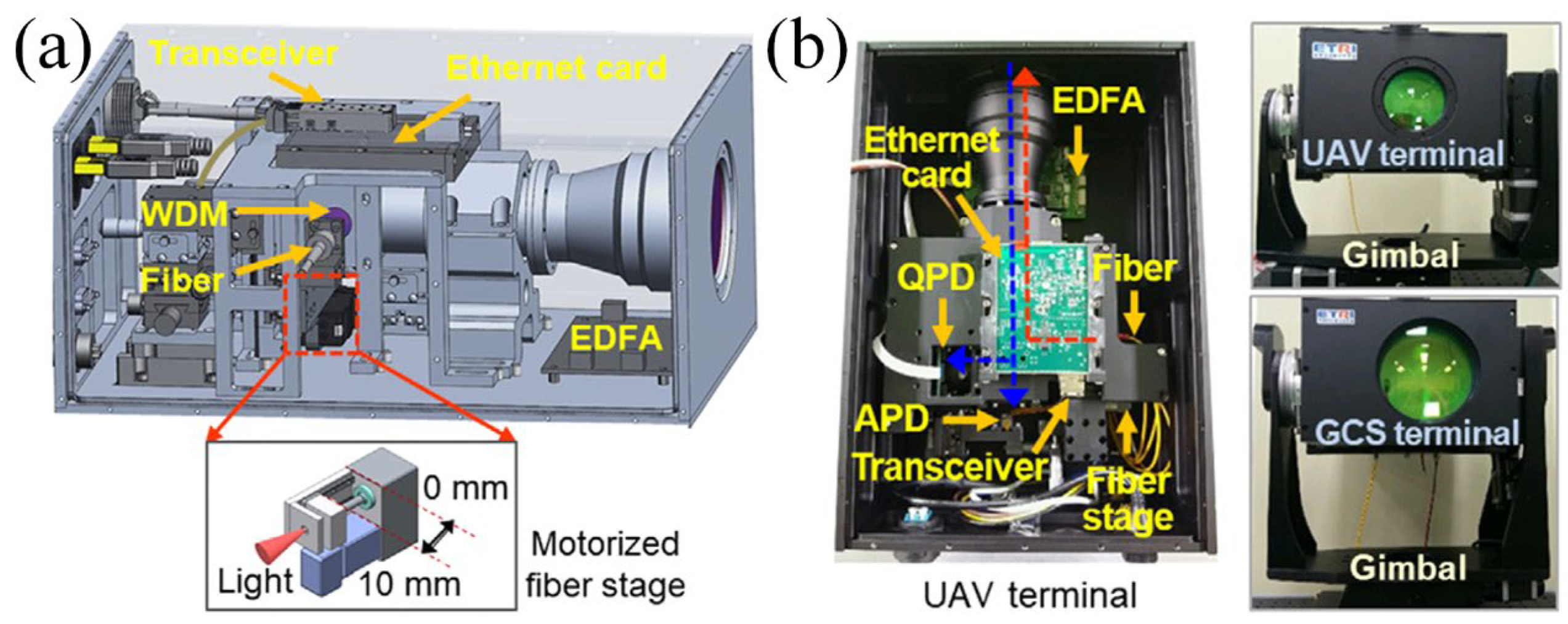
- Carrasco-Casado, A.; Shiratama, K.; Kolev, D.; Trinh, P.V.; Fuse, T.; Fuse, S.; Kawaguchi, K.; Hashimoto, Y.; Hyodo, M.; Sakamoto, T.; et al. Prototype Development and Validation of a Beam-Divergence Control System for Free-Space Laser Communications. Front. Phys. 2022, 10, 878488. [Google Scholar] [CrossRef]
- Gu, H.P.; Hong, H.J.; Fan, J.H. Research status and development of liquid lens. J. Appl. Opt. 2019, 40, 1126–1138. [Google Scholar] [CrossRef]
- Lv, W.M. Simulation and Experiment Study of Liquid Adaptive Lens. Master’s Thesis, Tianjin University, Tianjin, China, 2018. (In Chinese). [Google Scholar]
- Zhang, W.; Liu, P.; Wei, X.; Zhuang, S.; Yang, B. The Analysis of the Wavefront Aberration Caused by the Gravity of the Tunable-focus Liquid-Filled Membrane Lens. Master’s Thesis, Tianjin University, Tianjin, China, 2018. (In Chinese). [Google Scholar]
- Huang, X.; Lin, S.Y.; Gu, D.D.; Bu, Z.X.; Yi, W.J.; Xie, P.Q.; Wang, L.Y. Review on progress of variable-focus liquid lens. Chin. Opt. 2019, 12, 1179–1194. [Google Scholar] [CrossRef]
- Gao, K.; Bhowmik, A.; McGinty, C.; Bos, P.J. A non-mechanical zoom lens fabricated from liquid crystal reactive mesogens. In Proceedings of the Liquid Crystals XX, San Diego, CA, USA, 23 September 2016; SPIE: Warsaw, Poland, 2016; Volume 9940, pp. 125–133. (In Chinese). [Google Scholar]
- Lu, J.W.; Yuan, D.C.; Liu, Q. Study on characteristics of transmission wavefront of liquid lens. J. Appl. Opt. 2021, 42, 339–345. [Google Scholar] [CrossRef]
- Gan, J.J.; Li, L. PDMS liquid lens with corrected abberations. Opto-Electron. Eng. 2022, 49, 65–72. (In Chinese) [Google Scholar] [CrossRef]
- Zhao, Y.R.; Xu, J.B.; Liu, C.; Wang, Q.H. Large Aperture Electrowetting-Based Liquid Lens. Chin. J. Vac. Sci. Technol. 2021, 41, 877–882. (In Chinese) [Google Scholar] [CrossRef]
- Mai, V.V.; Kim, H. Beaconless PAT and adaptive beam control using variable focus lens for free-space optical communication systems. APL Photon. 2021, 6, 020801. [Google Scholar] [CrossRef]
- Zohrabi, M.; Cormack, R.H.; Gopinath, J.T. Wide-angle nonmechanical beam steering using liquid lenses. Opt. Express 2016, 24, 23798–23809. [Google Scholar] [CrossRef]
- Mai, V.V.; Kim, H. Non-Mechanical Beam Steering and Adaptive Beam Control Using Variable Focus Lenses for Free-Space Optical Communications. J. Light Technol. 2021, 39, 7600–7608. [Google Scholar] [CrossRef]
- Mai, V.; Kim, H. Beam Steering and Divergence Control Using Variable Focus Liquid Lenses for WDM FSO Communications. IEEE Photonics Technol. Lett. 2022, 34, 1226–1229. [Google Scholar] [CrossRef]
- Mai, V.V.; Kim, H. Variable Focus Lens-Based Beam Steering and Divergence Control for WDM Free-Space Optical Communication. In Proceedings of the Optical Fiber Communication Conference (OFC), San Diego, CA, USA, 6–10 March 2022; Optica Publishing Group: Washington, DC, USA, 2022; pp. M1C.6:1–M1C.6:3. [Google Scholar]
- Mai, V.V.; Kim, H. Optical Beam Control Based on Variable Focus Lenses for WDM FSO Communications. In Proceedings of the Conference on Lasers and Electro-Optics, San Jose, CA, USA, 15–20 May 2022; Optica Publishing Group: Washington, DC, USA, 2022; pp. STh2M.6:1–STh2M.6:2. [Google Scholar]
- Heng, K.H.; Liu, N.; He, Y.; Zhong, W.D.; Cheng, T.H. Adaptive Beam Divergence for Inter-UAV Free Space Optical Communications. In Proceedings of the 2008 IEEE PhotonicsGlobal@Singapore, Singapore, 8–11 December 2008; IEEE: Singapore, 2008; pp. 1–4. [Google Scholar]
- Hu, Y.; Gao, T.Y.; Jiang, L.; Cheng, D.W.; Dong, K.Y. Intensity Distribution and Realization Method of Divergence Angle Amplification in Ground Test of Space Laser Communication. Opt. Eng. 2015, 42, 82–88. (In Chinese) [Google Scholar] [CrossRef]
- Yang, N.; Lv, S.M.; Xie, T. An Optical Antenna with Variable Divergence Angle for Laser Communication. CN Patent 217443581U, 16 September 2022. (In Chinese). [Google Scholar]
- Hinrichs, K.M.; DeCew, A.E.; Narkewich, L.E.; Williams, T.H. Continuous beam divergence control via wedge-pair for laser communication applications. In Continuous Beam Divergence Control via Wedge-Pair for Laser Communication Applications; Hemmati, H., Boroson, D.M., Eds.; SPIE: San Francisco, CA, USA; Warsaw, Poland, 2015; pp. 166–177. [Google Scholar] [CrossRef]
- Presby, H.M.; Tyson, J.A. Method and Apparatus for Controlling Received Power Levels within a Free Space Optical Communication System. U.S. Patent 6,643,467, 4 November 2003. [Google Scholar]
- Zhu, L.; Song, C.J.; Zhang, J.; Wang, J.S.; Tian, B.; Liu, J.L.; Zhong, Y.; Cui, Y.P. Fiber Free Laser Communication Optical Transceiver with Variable Beam Divergence Angle and Its Control Method. CN Patent 1457154A, 19 November 2003. (In Chinese). [Google Scholar]
- Gianfranco, V. Method and Device for the Control of the Power Radiated in a Free-Space Optical Transmission System. EP Patent EP1411653A3, 8 June 2004. [Google Scholar]
- Ma, J.; Tan, L.Y.; Liu, J.F.; Han, Q.Q.; Yu, S.Y.; Yu, J.J.; Yang, Y.Q. High Precision Variable Beam Divergence Laser Emission Device Based on Precision Displacement Sensor. CN Patent 101210818A, 2 July 2008. (In Chinese). [Google Scholar]
- Park, S.; Yeo, C.I.; Heo, Y.S.; Ryu, J.H.; Kang, H.S.; Kim, S.C.; Jang, J.H. Common path-based mobile free-space optical terminal with adaptive beamforming function for Gbps out-of-band full-duplex connectivity to UAVs. Opt. Commun. 2021, 494, 127041. [Google Scholar] [CrossRef]
- Park, S.; Yeo, C.I.; Heo, Y.S.; Ryu, J.H.; Kang, H.S.; Lee, D.-S.; Jang, J.-H. Tracking Efficiency Improvement According to Incident Beam Size in QPD-Based PAT System for Common Path-Based Full-Duplex FSO Terminals. Sensors 2022, 22, 7770. [Google Scholar] [CrossRef]
- Lopresti, P.; Refai, H.; Sluss, J.; Varela-Cuadrado, I. Adaptive divergence and power for improving connectivity in free-space optical mobile networks. Appl. Opt. 2006, 45, 6591–6597. [Google Scholar] [CrossRef] [PubMed]
- Mitchell, G.A. Focus Adjustment for Motion-Picture Sound Pickups. U.S. Patent 2554679, 29 May 1951. [Google Scholar]
- Schuma, R.F.; Teppo, E. Optical Wedges Used in Beam Expander for Divergence Control of Laser. U.S. Patent 4846550, 11 July 1989. [Google Scholar]
- Roth, J.M.; Ramakrishnan, S.; Murphy, R.J.; Volpicell, A.M.; Rauch, S.; Andrle, M.S.; King, A.J.; Reynolds, B.; Taylor, J.A.; Parenti, R.R.; et al. Variable, two-color acquisition beam for free-space laser communication terminals. In Free-Space Laser Communications XXXI; SPIE: Warsaw, Poland, 2019; pp. 10910:144–10910:159. [Google Scholar] [CrossRef]
- Arnon, S. Optimization of urban optical wireless communication systems. IEEE Trans. Wirel. Commun. 2003, 24, 626–629. [Google Scholar] [CrossRef]
- Sandalidis, H. Optimization Models for Misalignment Fading Mitigation in Optical Wireless Links. IEEE Commun. Lett. 2008, 12, 395–397. [Google Scholar] [CrossRef]
- Bonev, B.G. Influence of random fluctuations of laser beam propagation direction on FSO power design. In Proceedings of the 2013 Conference on Microwave Techniques (COMITE), Pardubice, Czech Republic, 17–18 April 2013; IEEE: Piscataway, NJ, USA, 2013; pp. 95–100. [Google Scholar]
- Bonev, B. Impact of Laser Beam Divergence on Power Design of Free Space Optics Communication Systems. Available online: http://rcvt.tu-sofia.bg/ICEST2013_1_91.pdf (accessed on 25 April 2013).
- Safi, H.; Dargahi, A.; Cheng, J.; Safari, M. Analytical Channel Model and Link Design Optimization for Ground-to-HAP Free-Space Optical Communications. J. Light Technol. 2020, 38, 5036–5047. [Google Scholar] [CrossRef]
- Kaymak, Y.; Ansari, N.; Zhou, M. On Divergence-Angle Efficiency of a Laser Beam in Free-Space Optical Communications for High-Speed Trains. IEEE Trans. Veh. Technol. 2017, 66, 7677–7687. [Google Scholar] [CrossRef]
- Khallaf, H.S.; Uysal, M. Comprehensive study on UAV-based FSO links for high-speed train backhauling. Appl. Opt. 2021, 60, 8239–8247. [Google Scholar] [CrossRef] [PubMed]
- Al-Mohammed, H.A.; Yaacoub, E. Free Space Optics Communication for Ultra-High-Speed Train Running in Evacuated Tube. Appl. Sci. 2022, 12, 8545. [Google Scholar] [CrossRef]
- Vaiopoulos, N.; Sandalidis, H.G.; Varoutas, D. Using a HAP Network to Transfer WiMAX OFDM Signals: Outage Probability Analysis. J. Opt. Commun. Netw. 2013, 5, 711–721. [Google Scholar] [CrossRef]
- Mai, V.V.; Kim, H. Mitigation of Effects of Angle-of-Arrival Fluctuation and Pointing Error on Airborne Free-Space Optical Systems. In Proceedings of the Optical Fiber Communication Conference (OFC) 2019, San Diego, CA, USA, 3–7 March 2019; OSA: Washington, DC, USA, 2019; pp. W2A.40:1–W2A.40:4. [Google Scholar] [CrossRef]
- Mai, V.V.; Kim, H. Beam Size Optimization and Adaptation for High-Altitude Airborne Free-Space Optical Communication Systems. IEEE Photonics J. 2019, 11, 2901952. [Google Scholar] [CrossRef]
- Mai, V.V.; Kim, H. Beam Control and Tracking Techniques for High- Altitude Airborne Free-Space Optical Communication Systems. In Proceedings of the 2020 International Topical Meeting on Microwave Photonics (MWP), Matsue, Japan, 24–26 November 2020; IEEE: Piscataway, NJ, USA, 2020; pp. 5–8. [Google Scholar] [CrossRef]
- Arnon, S. Minimization of outage probability of WiMAX link supported by laser link between a high-altitude platform and a satellite. J. Opt. Soc. Am. A 2009, 26, 1545–1552. [Google Scholar] [CrossRef] [PubMed]
- Song, T.; Wang, Q.; Wu, M.-W.; Ohtsuki, T.; Gurusamy, M.; Kam, P.-Y. Impact of Pointing Errors on the Error Performance of Intersatellite Laser Communications. J. Light Technol. 2017, 35, 3082–3091. [Google Scholar] [CrossRef]
- Wan, X.; Hao, S.; Zhang, D.; Zhao, Q.; Tang, J.; Xu, C. Dynamic Beam Waist Adjustment of Inter-Satellite Optical Communication Based on Marcum Q-Function. Acta Opt. Sin. 2018, 38, 0906005. [Google Scholar] [CrossRef]
- Do, P.X.; Carrasco-Casado, A.; Hosonuma, T.; Toyoshima, M.; Nakasuka, S. A Study on Optimizing Laser Beam Waist for LEO-To-GEO Communication for 100 Kg-Class Satellite. In Proceedings of the 2020 International Conference on Communications, Computing, Cybersecurity, and Informatics (CCCI), Sharjah, United Arab Emirates, 3–5 November 2020; IEEE: Piscataway, NJ, USA, 2020; pp. 1–6. [Google Scholar] [CrossRef]
- Do, P.X.; Carrasco-Casado, A.; Van Vu, T.; Hosonuma, T.; Toyoshima, M.; Nakasuka, S. Numerical and analytical approaches to dynamic beam waist optimization for LEO-to-GEO laser communication. OSA Contin. 2020, 3, 3508. [Google Scholar] [CrossRef]
- Farid, A.A.; Hranilovic, S. Optimization of beam width, bit error rate and availability for free-space optical links. In Proceedings of the 2008 6th International Symposium on Communication Systems, Networks and Digital Signal Processing, Porto, Portugal, 29 August 2008; IEEE: Piscataway, NJ, USA, 2008; pp. 92–96. [Google Scholar] [CrossRef]
- Liu, C.; Yao, Y.; Sun, Y.X.; Xiao, J.J.; Zhao, X.H. Average capacity optimization in free-space optical communication system over atmospheric turbulence channels with pointing errors. Opt. Lett. 2010, 35, 3171–3173. [Google Scholar] [CrossRef]
- Zhao, Z.; Liao, R.; Zhang, Y. Impacts of laser beam diverging angle on free-space optical communications. In Proceedings of the 2011 Aerospace Conference, Big Sky, MT, USA, 5–12 March 2011; IEEE: Piscataway, NJ, USA, 2011; pp. 1–10. [Google Scholar] [CrossRef]
- Mitsev, T.; Dimitrov, K.; Ivanov, H.; Kolev, N. Optimum Divergence of Laser Radiation in FSO Systems. In Proceedings of the 7th International Conference on Communications, Electromanetics and Medical Applications, Athens, Greece, 10–15 June 2012; pp. 42–45. [Google Scholar]
- Soni, G.; Malhotra, J.S. Impact of Beam Divergence on the Performance of Free Space Optical System. Int. J. Sci. Res. Publ. 2012, 2, 57–61. [Google Scholar]
- Poliak, J.; Pezzei, P.; Leitgeb, E.; Wilfert, O. Analytical Expression of FSO Link Misalignments Considering Gaussian Beam. In Proceedings of the 2013 18th European Conference on Network and Optical Communications & 2013 8th Conference on Optical Cabling and Infrastructure (NOC-OC&I), Graz, Austria, 10 July 2013; IEEE: Piscataway, NJ, USA, 2013; pp. 99–104. [Google Scholar]
- Mitsev, T.A.; Kolev, N.K. Optimal Divergence of Laser Beam in Optical Wireless Communication Systems. Elektrotechnica Elektron. 2014, 49, 15–20. [Google Scholar]
- Mitsev, T.; Ferdinandov, E. Methodology for realization of given BER in FSO systems under effect of internal and external noises. In Proceedings of the 2015 Conference on Microwave Techniques (COMITE), Pardubice, Czech Republic, 22–23 April 2015; IEEE: Piscataway, NJ, USA, 2015; pp. 1–4. [Google Scholar] [CrossRef]
- Ivanov, H.; Leitgeb, E.; Plank, T.; Bekhrad, P.; Mitsev, T. Link Budget Optimization of Free Space Optical Systems in Relation to the Beam Diverging Angle. In Proceedings of the 2015 13th International Conference on Telecommunications (ConTEL), Graz, Austria, 13–15 July 2015; IEEE: Piscataway, NJ, USA, 2015; pp. 1–5. [Google Scholar]
- Liu, X.S.; Zou, G.N.; Zhou, W.F. Optimization Analysis of the Beam Divergence Angle over Pointing Error and Gamma-Gamma Atmospheric Turbulence. Semicond. Optoelectron. 2017, 38, 857–861. (In Chinese) [Google Scholar] [CrossRef]
- Bedington, R.; Arrazola, J.M.; Ling, A. Progress in satellite quantum key distribution. NPJ Quantum Inf. 2017, 3, 30. [Google Scholar] [CrossRef]
- Dervisevic, E.; Mehic, M. Overview of Quantum Key Distribution Technique within IPsec Architecture. arXiv 2021, arXiv:2112.13105. [Google Scholar]
- Filgueiras, H.R.D.; Lima, E.S.; Cunha, M.S.B.; Lopes, C.H.d.S.; De Souza, L.C.; Borges, R.M.; Pereira, L.A.M.; Brandao, T.H.; Andrade, T.P.V.; Alexandre, L.C.; et al. Wireless and Optical Convergent Access Technologies Toward 6G. IEEE Access 2023, 11, 9232–9259. [Google Scholar] [CrossRef]
- Khorasani, M.; Ghasemi, A.; Leary, M.; Sharabian, E.; Cordova, L.; Gibson, I.; Downing, D.; Bateman, S.; Brandt, M.; Rolfe, B. The effect of absorption ratio on meltpool features in laser-based powder bed fusion of IN718. Opt. Laser Technol. 2022, 153, 108263. [Google Scholar] [CrossRef]
- Weaver, J.; Schlenoff, A.; Deisenroth, D.; Moylan, S. Assessing the influence of non-uniform gas speed on the melt pool depth in laser powder bed fusion additive manufacturing. Rapid Prototyp. J. 2023; ahead-of-print. [Google Scholar] [CrossRef]
- Stutzman, C.; Przyjemski, A.; Nassar, A.R. Effects of gas flow speed on bead geometry and optical emissions during laser powder bed fusion additive manufacturing. Rapid Prototyp. J. 2023; ahead-of-print. [Google Scholar] [CrossRef]
- Nasr, N.H.; Mohamed, M.-D.A.; Khairy, M. Dartboard Scheme for Rate Enhancement of Mobile Free-Space Optical Channels. In Proceedings of the 2018 IEEE Global Communications Conference (GLOBECOM), Abu Dhabi, United Arab Emirates, 9–13 December 2018; IEEE: Piscataway, NJ, USA, 2018; pp. 1–6. [Google Scholar]



| Method | Year | Author | Wavelength/nm | Experiment/Simulation | Beam Divergence Angle Range | Power Range | Communication Distance | Date Rate | BER |
|---|---|---|---|---|---|---|---|---|---|
| Using zoom group | 2014 | K.K. Han et al. [36] | 532 | Experiment | 1–200 mrad | Constant | |||
| 2016 | J.J. Ma et al. [37] | Patent | |||||||
| 2018 | Y.L. Yang [38] | 1550 | Experiment | 0.7–1.2 mrad | Constant | 3 km | 1.25 Gbps | ||
| 2022 | X.L. Xie et al. [39] | Patent | |||||||
| 2022 | A, C.C. et al. [40] | 1550 | Experiment | 90–6250 µrad (FWHM) | Maximum 2 W | 600 km: 10 Gb/s; 1200 km: 2.5 Gb/s | |||
| Using zoom lens | 2016 | M. Zohrabi [50] | visible and near–infrared | Experiment | few mm to few cm beam diameter | ||||
| 2017 | J.W. Andrew et al. [8] | 486/520 | Experiment | collimated to 13° | |||||
| 2021 | V.V Mai et al. [49] | 1530 | Experiment | 377.1–1131.4 μrad | 104 m | 10 Gbps | 1 × 10−3 | ||
| 2021 | V.V Mai et al. [51] | 1530 | Experiment | 0.84–2.2 mrad | 1 × 10−3 | ||||
| 2022 | V.V Mai et al. [52,53,54] | 1549.2/ 1550.0/ 1550.8/1551.6 | Experiment | 0.84–2.2 mrad | 4 × 10 Gbps (WDM) | 3.5 × 10−6 | |||
| Using lens with different F | 2008 | K.H. Heng et al. [10,55] | Simulation | Constant | Maximum 4 km | ||||
| 2009 | 6–96 mrad (Discrete) | 800 mW | 300 m–10 km | ||||||
| 2015 | Y. Hu et al. [56] | 1550 | Simulation | 15/500 μrad | Constant | 5/10/20 km | |||
| 2022 | Y. Ning et al. [57] | Patent | |||||||
| Axial translation of light source or collimating lens | 2003 | L. Zhu et al. [60] | Patent | 1.5–30 mrad | |||||
| 2003 | C.M. Kelway [59] | Patent | |||||||
| 2004 | V. Gianfranco [61] | Patent | |||||||
| 2008 | J. Ma et al. [62] | Patent | 0.1–1 mrad | ||||||
| 2006 | P. LoPresti [65] | 632.8 | Experiment | 1–100 mW | 2/1.75/1.5/1.25/1/0.5 km | ||||
| 2021 | S. Park et al. [63,64] | 1530/ 1590 | Experiment | Maximum 8.84 mrad | Maximum 200 mW | 13.6 km | 1.25 Gbit/s | 1 × 10−12 | |
| 2022 | 200 mW | 50 m | |||||||
| 2022 | R. Harada et al. [9] | 1550 | Simulation | 0.4–6 mrad | 10 dBm | 5–200 m | 100 Gbit/s | ||
| Inserting parallel plate | 1951 | G.A. Mitchell [66] | Patent | ||||||
| Using optical wedge-pair | 1989 | R.F. Schuma et al. [67] | Patent | ||||||
| 2015 | K.M. Hinrichs et al. [58] | 1530– 1570 | Experiment | 58.8–509 μrad | Constant | ||||
| 2019 | J.M. Roth et al. [68] | Experiment | 64.5–679 μrad (Discrete) | 0–1 mW |
| Method | VBDA Range * | If Varies Continuously | Response Time | Others |
| Using zoom group | 90 μrad (FWHM)–200 mrad | Yes | Seconds | The technology behind zoom lens groups has reached a relatively mature stage and it requires high-quality guide rails and actuators. |
| Using zoom lens | 377.1 μrad–13° | Yes | Milliseconds to seconds | It is not necessary to use a mechanical mechanism, which could reduce the terminal volume. However, the system may be affected by gravity and enlarging the aperture may be difficult. |
| Using lens with different F | 15 μrad– 96 mrad | No | Milliseconds/seconds | The principle is simple, but the communication link will experience an outage when switching angles. |
| Axial translation of fiber or lens | 100 μrad–30 mrad | Yes | Seconds | The parallelism of linear displacement table is very high. |
| Inserting parallel plate | No | Milliseconds to seconds | The principle is simple and can greatly relax the requirement for lateral positioning accuracy. The communication link will experience an outage when switching angles. | |
| Using optical wedge-pair | 58.8 μrad– 0.679 mrad | Yes | Seconds | The influence on the optical axis deflection is small. As the wedge angle increases, lager astigmatism is introduced. |
| Link Types | Year | Major Contribution | Ref |
|---|---|---|---|
| Ground terminal platform - Ground terminal platform | 2003 | Proposed a function which incorporates building sway statistics, system parameters, and the required BER to minimize transmitter power. The function achieved this optimization by optimizing the transmitter gain. | [69] |
| 2008 | Provided closed-form expressions for BER and outage probability (OP) of IM/DD with OOK FSO system. The OBDA was determined by searching and calculating. | [70] | |
| 2013 | Analyzed the impact of beam radius and divergence angle on the power design of FSO communication, and the OBDA was determined. | [71,72] | |
| 2016 | Derived the OBDA which corresponds to different pointing errors. Found that the system availability can be significantly improved by using the OBDA. | [27] | |
| Ground terminal platform - Air platform | 2020 | Developed closed-form statistical channel models under log-normal (LN) and gamma–gamma (GG) atmospheric turbulence models. The OBDA, which maximizes the average SNR, was given by searching and analyzing. | [73] |
| 2021 | Through indoor 90 m and outdoor >100 m full-duplex 1.25 Gbps communication experiments, it was found that increasing the beam divergence angle can effectively improve the stability of the communication link. | [63] | |
| 2022 | Proposed a PAT system based on quadrant photodiode (QPD) and VBDA mechanism by moving the light source. This PAT system can achieve beacon-free acquisition and shorten the tracking time to 1/4.5 of the original. | [64] | |
| Ground terminal platform - HST platform | 2017 | This is the first work that compared the narrow and wide beam used in FSO communications for HSTs. A wide beam divergence angle range of [0.07, 2.002] was suggested to relax the steering speed of the FSM, as well as to overcome the negative effects of train vibration. | [74] |
| 2021 | Evaluated the complexity of ground and aerial PAT systems using angular velocity and maximum acceptable delay as two indexes. The results showed that the UAV relay performed better than the ground relay. | [75] | |
| 2022 | Obtained the OBDA to maximize the received optical power and thereby reduced the minimum communication distance between a mobile node with a speed of 100 km/h and a fixed terminal from the original 20 m to 5 m. | [9] | |
| 2022 | Proposed a strategy of VBDA according to the communication distance for ultra-high-speed trains in evacuated tubes. This strategy reduced the number of base stations and power consumption. | [76] | |
| Surface terminal platform - Underwater platform | 2017 | Developed a new scanning strategy using VBDA, which decreased the acquisition time by 80% in simulation. The robust acquisition and tracking with only navar-received optical power have been successfully demonstrated. | [8] |
| Air platform - Air platform | 2008 | Found that the OBDA was 1.4 times the angular size of the combination of the certainty area and jitter. In this case, the attenuation caused by geometrical and pointing loss was the smallest. | [55] |
| 2009 | Compared the transmission distances for the systems equipped with VBDA mechanism (A and B) and a system with fixed-beam divergence angle (C). The A and B showed a significant improvement in the maximum transmission distance compared to system C, which was increased from 2 km to 10 km. | [10] | |
| 2013 | Derived the expression of OP, differentiated it with respect to the divergence angle, and gave the expression of the OBDA which minimized the OP. | [77] | |
| 2018 | Used a generalized two-dimensional Gaussian distribution to represent the pointing error, and a closed-form expression of OBDA was derived under two strategies (maximizing link availability and minimizing transmitter power). | [26] | |
| 2019 | Provided a closed-form expression for the OBDA which minimized the OP. A zoom lens was used to adjust the beam size, thereby alleviating the performance degradation caused by angle of arrival (AoA) fluctuations and pointing errors. | [78,79] | |
| 2020 | Derived closed-form expressions of link availability and OBDA. Additionally, the system’s tolerance to AoA fluctuations was increased by 2 times by using VBDA control technology on a 104 m 10 Gb/s FSO link. | [80] | |
| Air platform - Satellite platform | 2009 | Derived a closed-form solution for the transmit gain which minimized the link OP. | [81] |
| Satellite platform - Satellite platform | 2016 | Created a square approximation of the circular detector region to derive a simple algebraic solution for the OBDA and concluded that the communication quality with a dynamic divergence control scheme was much better than that with a fixed divergence control scheme. | [25] |
| 2017 | The effect of pointing error on the average ABEP of intersatellite optical communication link was studied and gave a closed-form expression for calculating the instantaneous channel gain using the Marcum Q-function. | [82] | |
| 2018 | Provided a closed-form expression for calculating the instantaneous channel state by using the Marcum Q-function, without any approximations. Additionally, a simple algebraic expression for the optimal beam waist radius was derived, under the condition that the instantaneous aiming error angle was known. | [83] | |
| 2020 | Gave the best waist width expression to minimize the average BER for LEO satellites in the 100 kg class; the expression was approximated by using the asymptotic form of the improved Bessel function. | [84] | |
| 2020 | Proposed numerical and analytical methods of the channel gain which based on the Marcum Q-function and obtained an accurate approximation of the optimal waist width to achieve the best LEO-to-GEO communication performance. | [85] | |
| 2022 | Determined the OBDA by the attitude error, load pointing error, positioning error, and real-time link distance between satellites and gave the corresponding beam divergence angle control scheme. | [39] | |
| Others | 2008 | Compared the impact of the VBDA scheme and fixed divergence angle scheme on BER and link availability. In the VBDA scheme, a small number of discrete beam divergence angles were set to balance the performance and complexity of the system well. | [86] |
| 2010 | Derived a closed-form expression for the average channel capacity, and the OBDA to maximize the average channel capacity under different pointing errors and different wavelengths was given by search and calculation analysis. | [87] | |
| 2011 | Gave the average BER and the corresponding OBDA for the uncoded and coded systems, respectively. The authors found that the OBDA of coded FSO communication system was smaller than that of uncoded system. | [88] | |
| 2012 | Studied the relationship between the OBDA and the transmitted optical power and the communication distance and found that the OBDA was much more affected by the communication distance than the transmitted optical power. | [89] | |
| 2012 | Used the simulation package OptSim to study the Q-factor, BER, and Eye Map at different divergence angles from 0.1–3 mrad. The results showed that the link could withstand greater attenuation by reducing the beam divergence angle. | [90] | |
| 2013 | Simulated and analyzed the influence of different beam divergence angles on the received optical power and the mitigation effect on transmitter tilt in short-range FSO communication. The authors proposed the need for a trade-off between the received optical power and the mitigation effect on transmitter tilt. | [91] | |
| 2014 | Derived the calculation formula of the OBDA, and it was found that the maximum allowable pointing error could be increased by 121% when the beam was adjusted to the OBDA. | [92] | |
| 2015 | Gave the formula of the required transmitted optical power corresponding to the beam radius of the receiver and obtained the OBDA which minimized the transmitted optical power. | [93] | |
| 2015 | Gave the formula of the maximum allowable pointing error varying with beam divergence angle under different transmitting optical power and obtained the OBDA. | [94] | |
| 2017 | Derived the closed-form expressions for the channel capacity of the direct detection system and the coherent detection system, and the OBDA which maximized the channel capacity under different turbulence conditions was given by searching and calculating methods. | [95] | |
| 2021 | Expressed the OBDA as a closed-form function of the random displacement statistics of the target, in order to maintain sufficient average power and reduce the outage probability; this provided an idea for the system design. | [23] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, G.; Wu, J.; Li, Y.; Wang, X.; Yu, X.; Gao, S.; Ma, L. A Review of Variable-Beam Divergence Angle FSO Communication Systems. Photonics 2023, 10, 756. https://doi.org/10.3390/photonics10070756
Zhang G, Wu J, Li Y, Wang X, Yu X, Gao S, Ma L. A Review of Variable-Beam Divergence Angle FSO Communication Systems. Photonics. 2023; 10(7):756. https://doi.org/10.3390/photonics10070756
Chicago/Turabian StyleZhang, Guoqiang, Jiabin Wu, Yatian Li, Ximing Wang, Xichang Yu, Shijie Gao, and Lie Ma. 2023. "A Review of Variable-Beam Divergence Angle FSO Communication Systems" Photonics 10, no. 7: 756. https://doi.org/10.3390/photonics10070756
APA StyleZhang, G., Wu, J., Li, Y., Wang, X., Yu, X., Gao, S., & Ma, L. (2023). A Review of Variable-Beam Divergence Angle FSO Communication Systems. Photonics, 10(7), 756. https://doi.org/10.3390/photonics10070756





