Inverse Energy Flux in Tight Focusing of Vector Vortex Beam
Abstract
1. Introduction
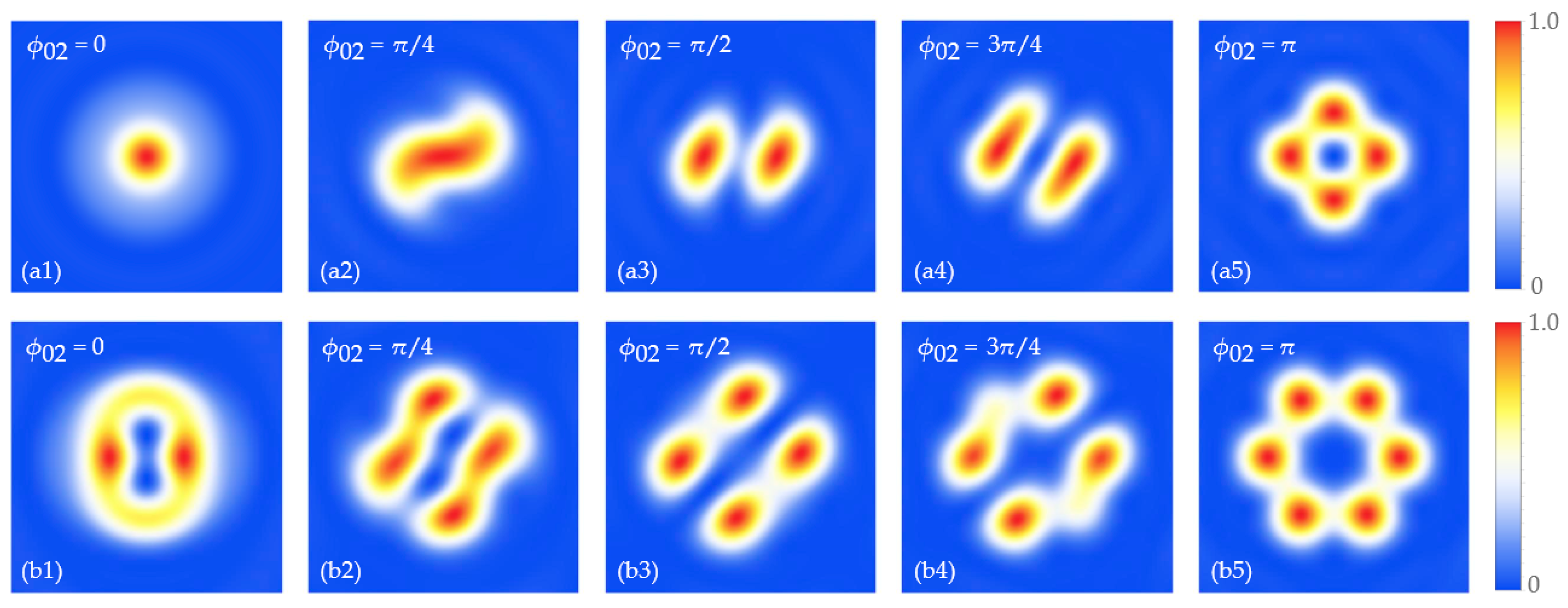
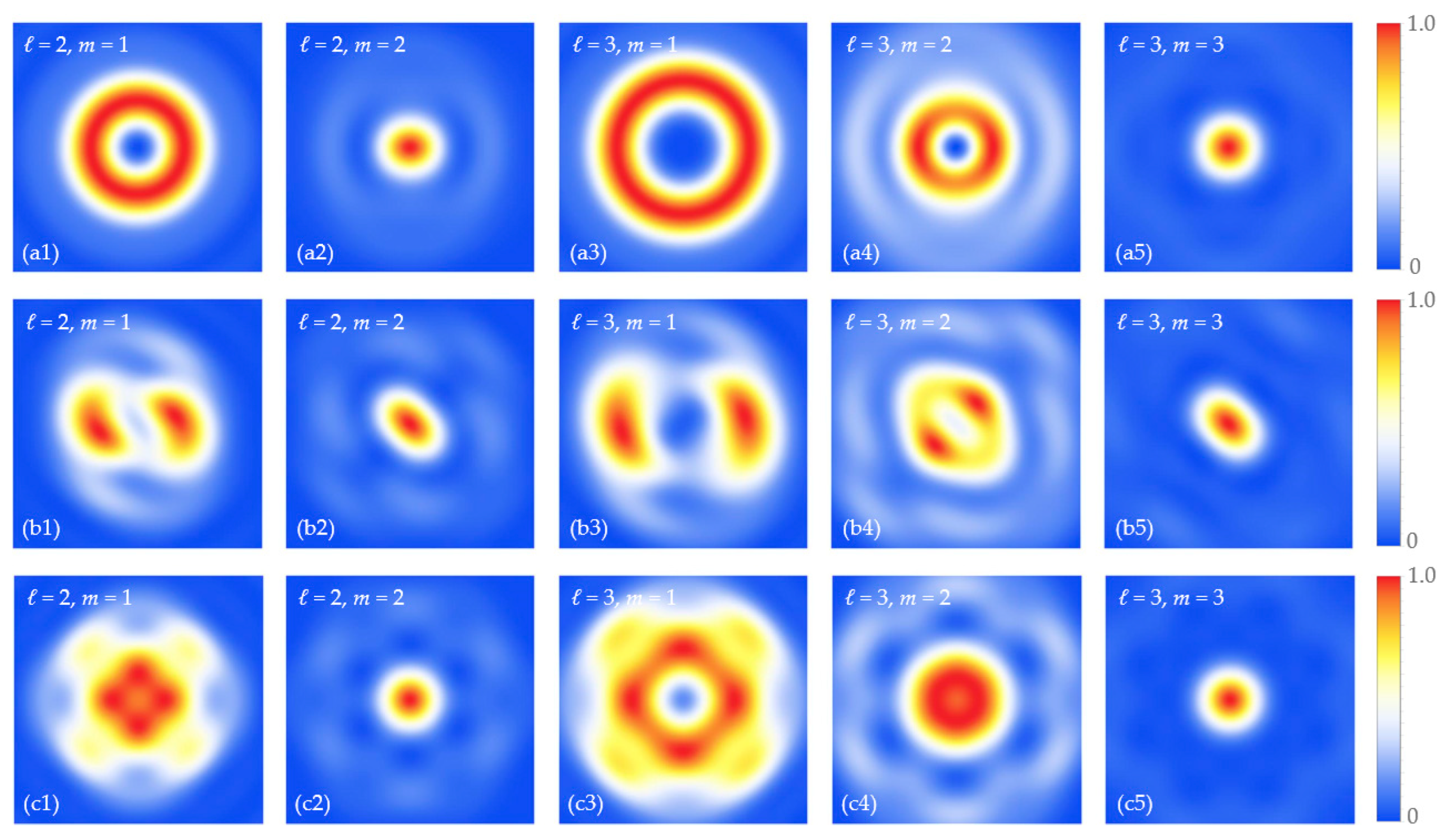
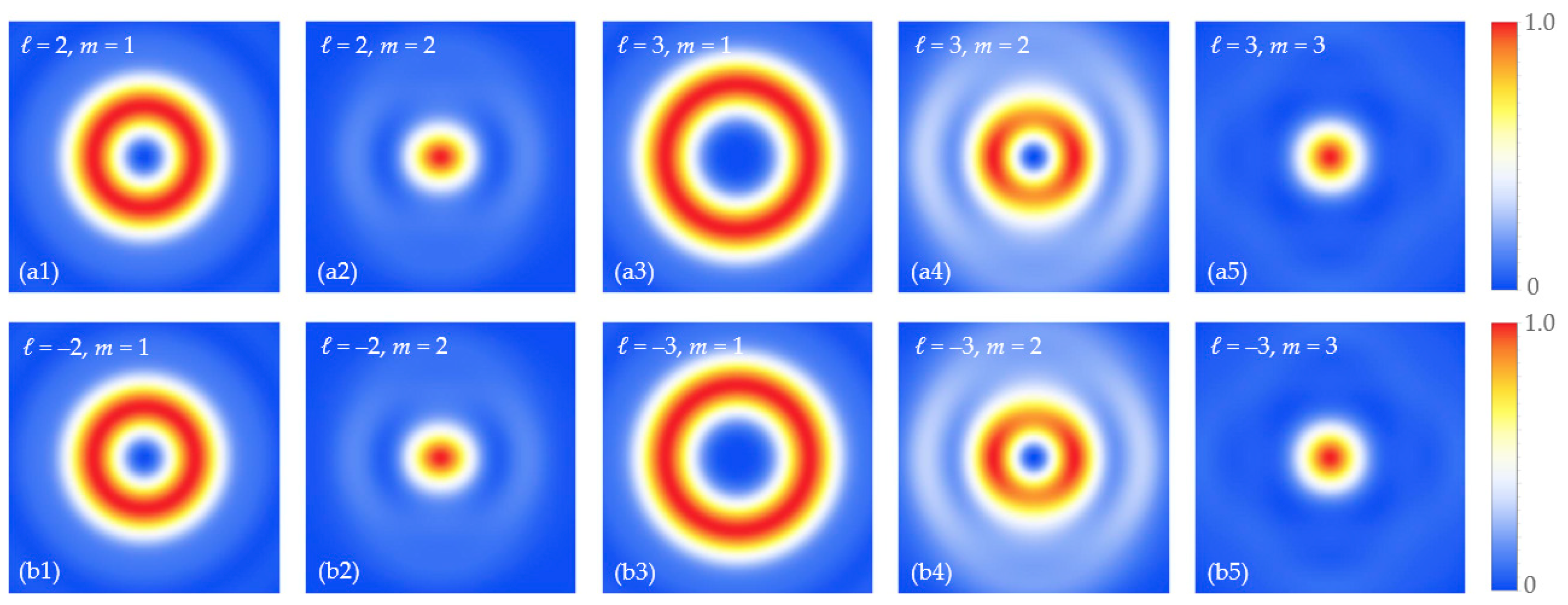
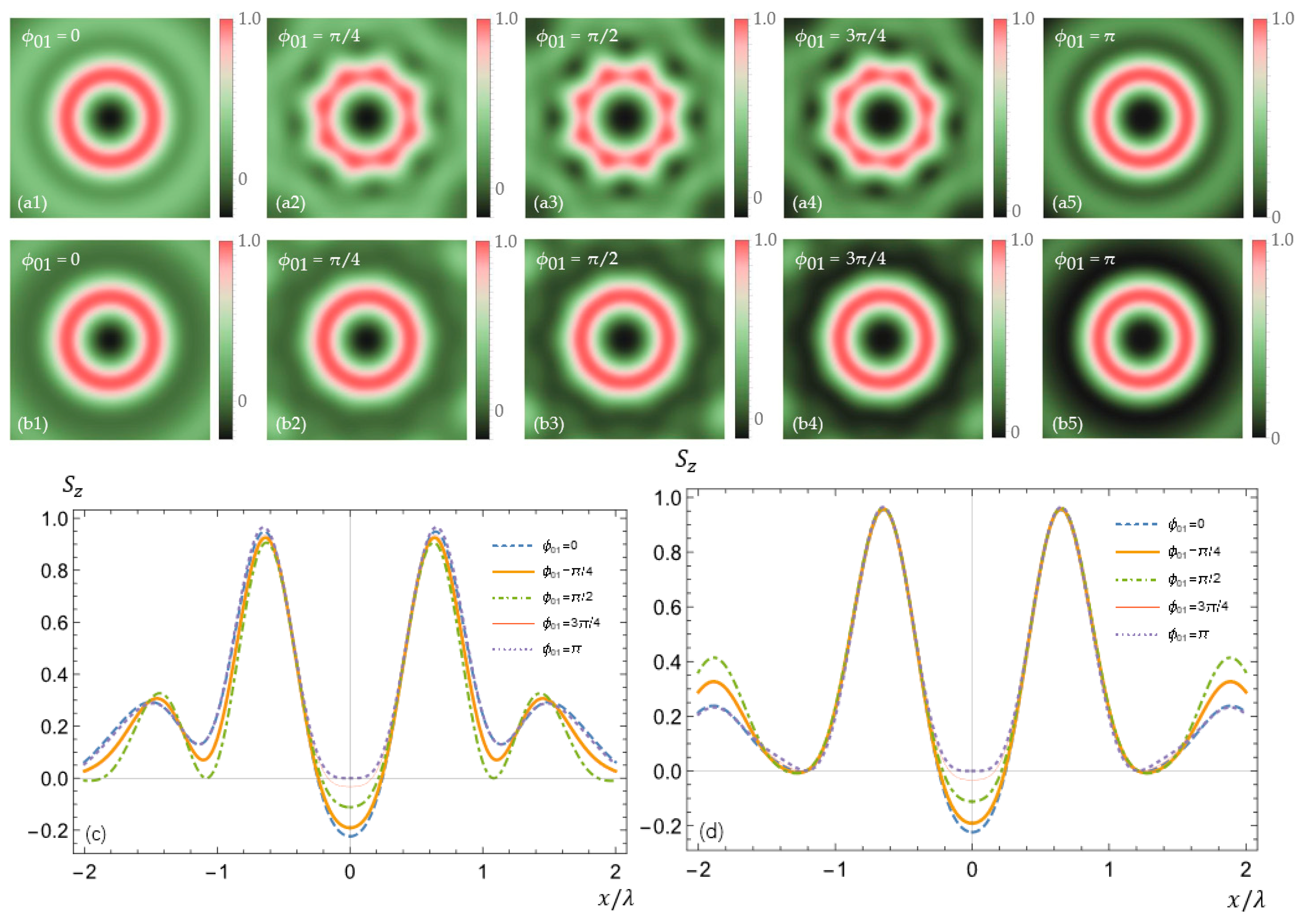
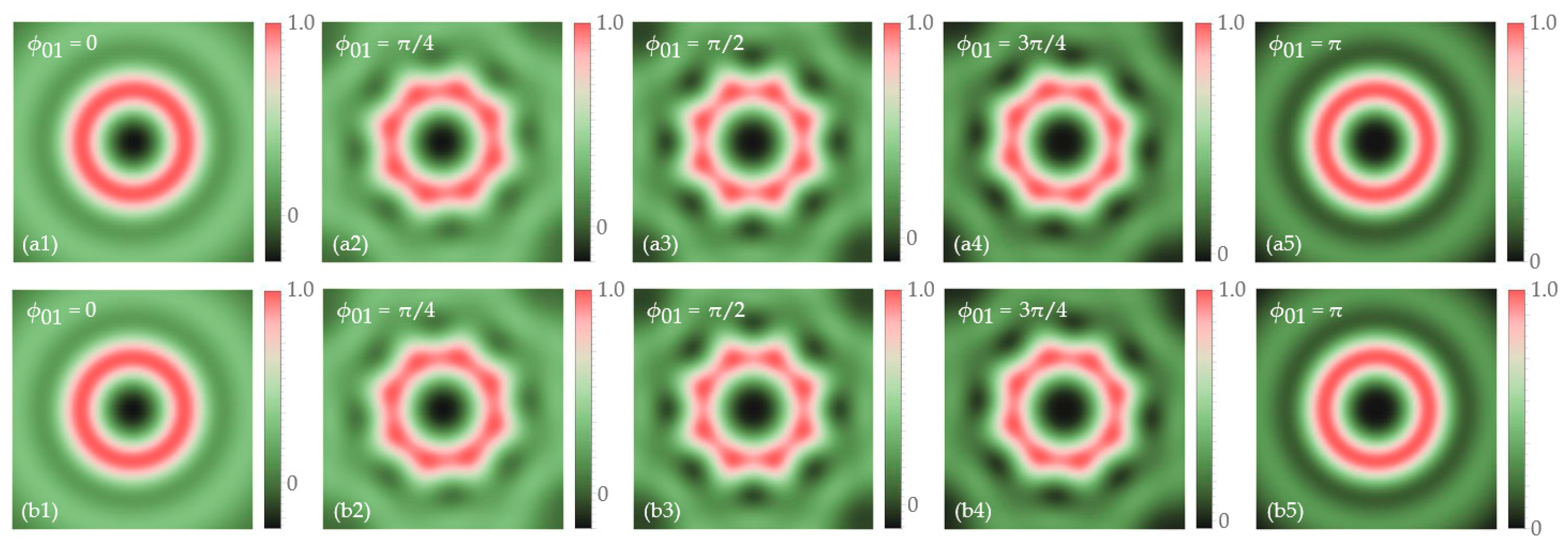
2. Theoretical Model
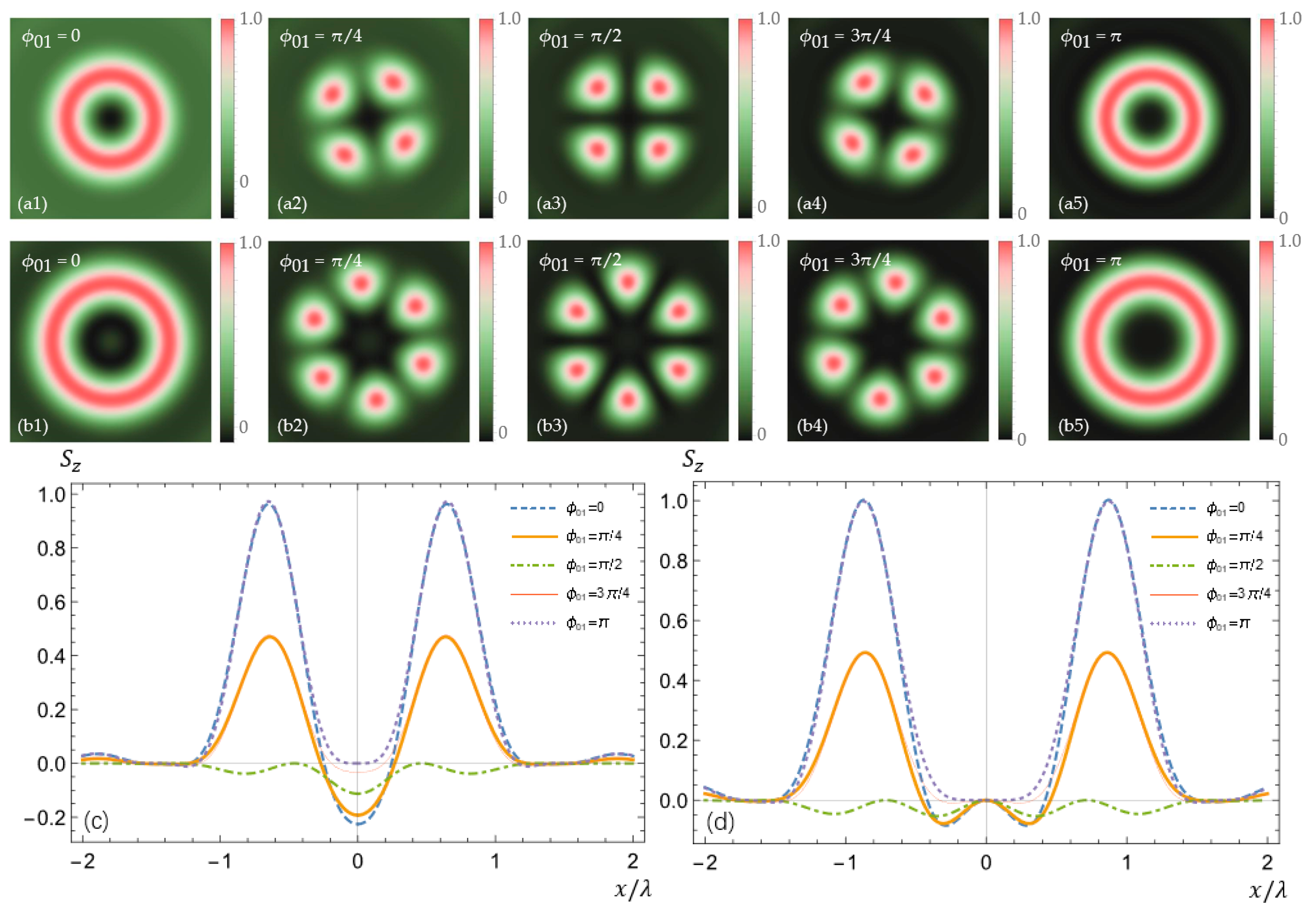
3. Negative Energy Flow in Focal Region
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Rosales-Guzmán, C.; Ndagano, B.; Forbes, A. A Review of Complex Vector Light Fields and Their Applications. J. Opt. 2018, 20, 123001. [Google Scholar] [CrossRef]
- Zhan, Q. Cylindrical Vector Beams: From Mathematical Concepts to Applications. Adv. Opt. Photon. 2009, 1, 1–57. [Google Scholar] [CrossRef]
- Khonina, S.N.; Karpeev, S.V. Grating-Based Optical Scheme for the Universal Generation of Inhomogeneously Polarized Laser Beams. Appl. Opt. 2010, 49, 1734–1738. [Google Scholar] [CrossRef]
- Chen, J.; Wan, C.; Zhan, Q. Vectorial Optical Fields: Recent Advances and Future Prospects. Sci. Bull. 2018, 63, 54–74. [Google Scholar] [CrossRef] [PubMed]
- Berry, M.V.; Jeffrey, M.R. Chapter 2 Conical Diffraction: Hamilton’s Diabolical Point at the Heart of Crystal Optics. Prog. Opt. 2007, 50, 13–50. [Google Scholar]
- He, C.; Shen, Y.; Forbes, A. Towards Higher-Dimensional Structured Light. Light. Sci. Appl. 2022, 11, 205. [Google Scholar] [CrossRef]
- Youngworth, K.; Brown, T. Focusing of High Numerical Aperture Cylindrical-Vector Beams. Opt. Express 2000, 7, 77–87. [Google Scholar] [CrossRef]
- Bhebhe, N.; Rosales-Guzman, C.; Forbes, A. Classical and Quantum Analysis of Propagation Invariant Vector Flat-Top Beams. Appl. Opt. 2018, 57, 5451–5458. [Google Scholar] [CrossRef]
- Michihata, M.; Hayashi, T.; Takaya, Y. Measurement of axial And Transverse Trapping Stiffness of Optical Tweezers In Air Using a Radially Polarized Beam. Appl. Opt. 2009, 48, 6143–6151. [Google Scholar] [CrossRef]
- Kozawa, Y.; Sato, S. Optical Trapping of Micrometer-Sized Dielectric Particles by Cylindrical Vector Beams. Opt. Express 2010, 18, 10828–10833. [Google Scholar] [CrossRef]
- Huang, S.-Y.; Zhang, G.-L.; Wang, Q.; Wang, M.; Tu, C.; Li, Y.; Wang, H.-T. Spin-to-Orbital Angular Momentum Conversion via Light Intensity Gradient. Optica 2021, 8, 1231–1236. [Google Scholar] [CrossRef]
- Segawa, S.; Kozawa, Y.; Sato, S. Resolution Enhancement of Confocal Microscopy by Subtraction Method with Vector Beams. Opt. Lett. 2014, 39, 3118–3121. [Google Scholar] [CrossRef]
- Steger, M.; Gautham, C.; Snoke, D.W.; Pfeiffer, L.; West, K. Slow Reflection and Two-Photon Generation of Microcavity Exciton–Polaritons. Optica 2015, 2, 1–5. [Google Scholar] [CrossRef]
- Zhao, Y.; Wang, J. High-Base Vector Beam Encoding/Decoding for Visible-Light Communications. Opt. Lett. 2015, 40, 4843–4846. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Xue, Y.; Zhu, Z.; Rui, G.; Cui, Y.; Gu, B. Theoretical Investigation on Asymmetrical Spinning and Orbiting Motions of Particles in a Tightly Focused Power-Exponent Azimuthal-Variant Vector Field. Opt. Express 2018, 26, 4318–4329. [Google Scholar] [CrossRef] [PubMed]
- Wang, F.; Zhao, C.; Dong, Y.; Dong, Y.; Cai, Y. Generation and Tight-Focusing Properties of Cylindrical Vector Circular Airy Beams. Appl. Phys. B 2014, 117, 905–913. [Google Scholar] [CrossRef]
- Zhao, Y.; Edgar, J.S.; Jeffries, G.D.M.; McGloin, D.; Chiu, D.T. Spin-to-Orbital Angular Momentum Conversion in a Strongly Focused Optical Beam. Phys. Rev. Lett. 2007, 99, 073901. [Google Scholar] [CrossRef]
- Kotlyar, V.V.; Kovalev, A.A.; Nalimov, A.G. Energy Density and Energy Flux in the Focus of an Optical Vortex: Reverse Flux of Light Energy. Opt. Lett. 2018, 43, 2921–2924. [Google Scholar] [CrossRef] [PubMed]
- Li, H.; Wang, C.; Tang, M.; Li, X. Controlled Negative Energy Flow in the Focus of a Radial Polarized Optical Beam. Opt. Express 2020, 28, 18607–18615. [Google Scholar] [CrossRef]
- Li, H.; Ma, C.; Wang, J.; Tang, M.; Li, X. Spin-Orbit Hall Effect in the Tight Focusing of a Radially Polarized Vortex Beam. Opt. Express 2021, 29, 39419. [Google Scholar] [CrossRef]
- Novitsky, A.; Novitsky, D. Negative Propagation of Vector Bessel Beams. J. Opt. Soc. Am. A 2007, 24, 2844–2849. [Google Scholar] [CrossRef] [PubMed]
- Monteiro, P.B.; Neto, P.A.M.; Nussenzveig, H.M. Angular Momentum of Focused Beams: Beyond the Paraxial Approximation. Phys. Rev. A 2009, 79, 033830. [Google Scholar] [CrossRef]
- Kotlyar, V.V.; Stafeev, S.S.; Nalimov, A.G. Energy Backflow in the Focus of a Light Beam with Phase or Polarization Singularity. Phys. Rev. A 2019, 99, 033840. [Google Scholar] [CrossRef]
- Khonina, S.N.; Ustinov, A.V.; Degtyarev, S.A. Inverse Energy Flux of Focused Radially Polarized Optical Beams. Phys. Rev. A 2018, 98, 043823. [Google Scholar] [CrossRef]
- Helseth, L. Optical Vortices in Focal Regions. Opt. Commun. 2004, 229, 85–91. [Google Scholar] [CrossRef]
- Bliokh, K.Y.; Bekshaev, A.Y.; Nori, F. Dual Electromagnetism: Helicity, Spin, Momentum and Angular Momentum. New J. Phys. 2013, 15, 033026. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, R.; Song, T.; Luo, Y.; Li, H.; Li, X. Inverse Energy Flux in Tight Focusing of Vector Vortex Beam. Photonics 2023, 10, 743. https://doi.org/10.3390/photonics10070743
Chen R, Song T, Luo Y, Li H, Li X. Inverse Energy Flux in Tight Focusing of Vector Vortex Beam. Photonics. 2023; 10(7):743. https://doi.org/10.3390/photonics10070743
Chicago/Turabian StyleChen, Ruixiang, Tiegen Song, Yuee Luo, Hehe Li, and Xinzhong Li. 2023. "Inverse Energy Flux in Tight Focusing of Vector Vortex Beam" Photonics 10, no. 7: 743. https://doi.org/10.3390/photonics10070743
APA StyleChen, R., Song, T., Luo, Y., Li, H., & Li, X. (2023). Inverse Energy Flux in Tight Focusing of Vector Vortex Beam. Photonics, 10(7), 743. https://doi.org/10.3390/photonics10070743




