Atmospheric Turbulence Effects on the Performance of Orbital Angular Momentum Multiplexed Free-Space Optical Links Using Coherent Beam Combining
Abstract
1. Introduction
2. Simulation Analysis Method
2.1. Theoretical Derivation
2.2. Random Phase Screens Method
3. Numerical Results and Discussion
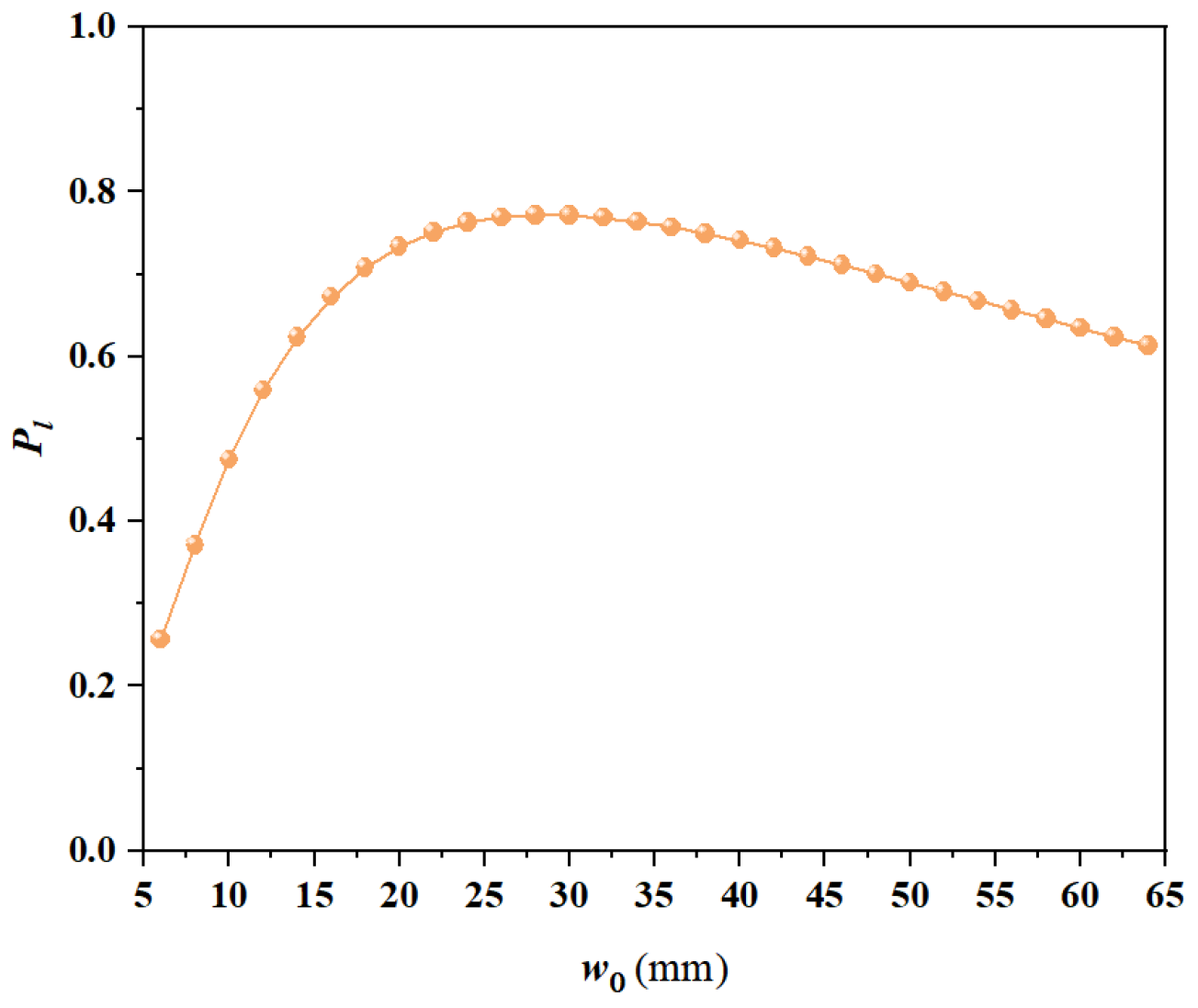
3.1. Numerical Results Based on Theoretical Derivation
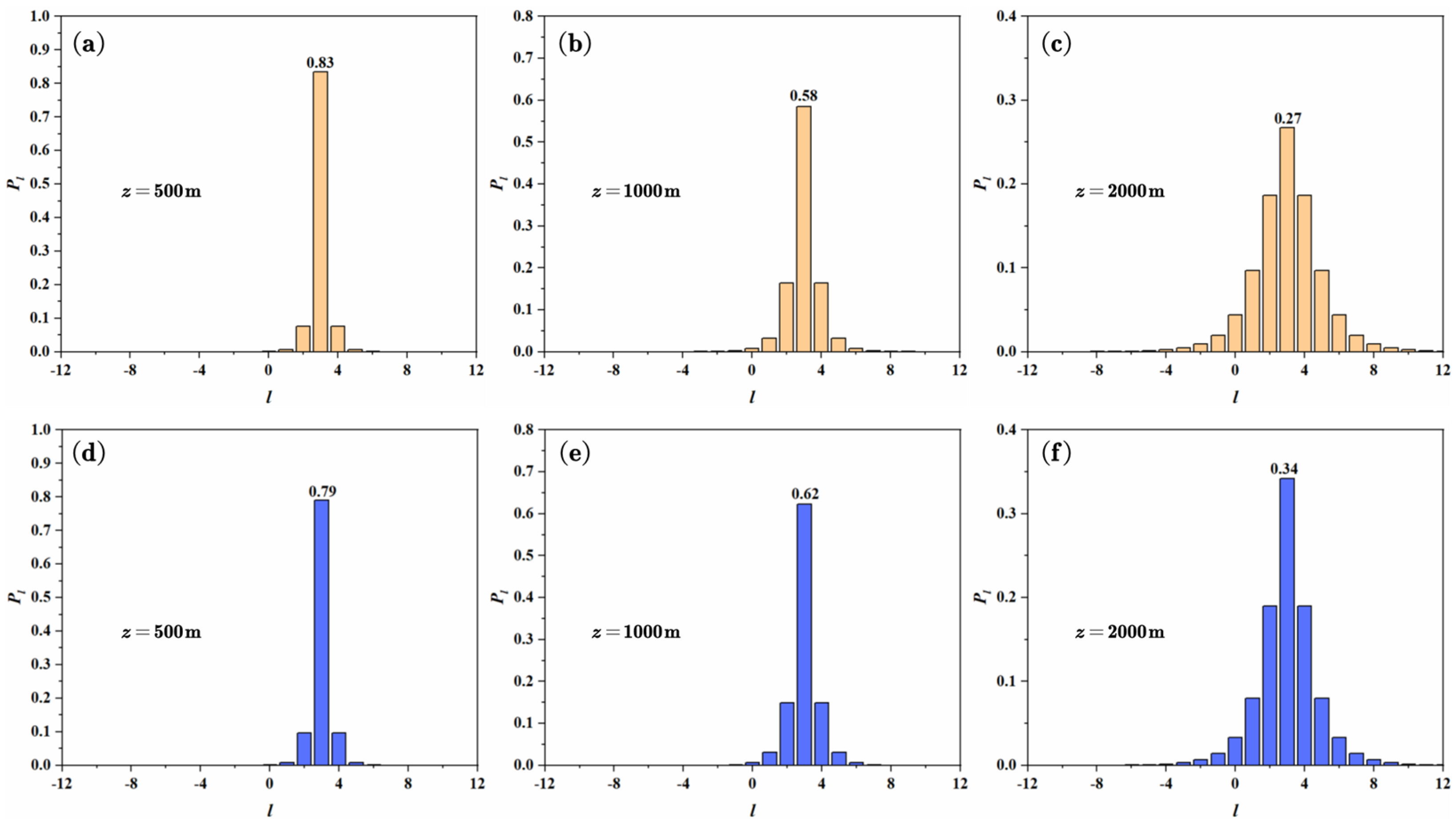
3.2. Numerical Simulation Results Based on Random Phase Screens Method
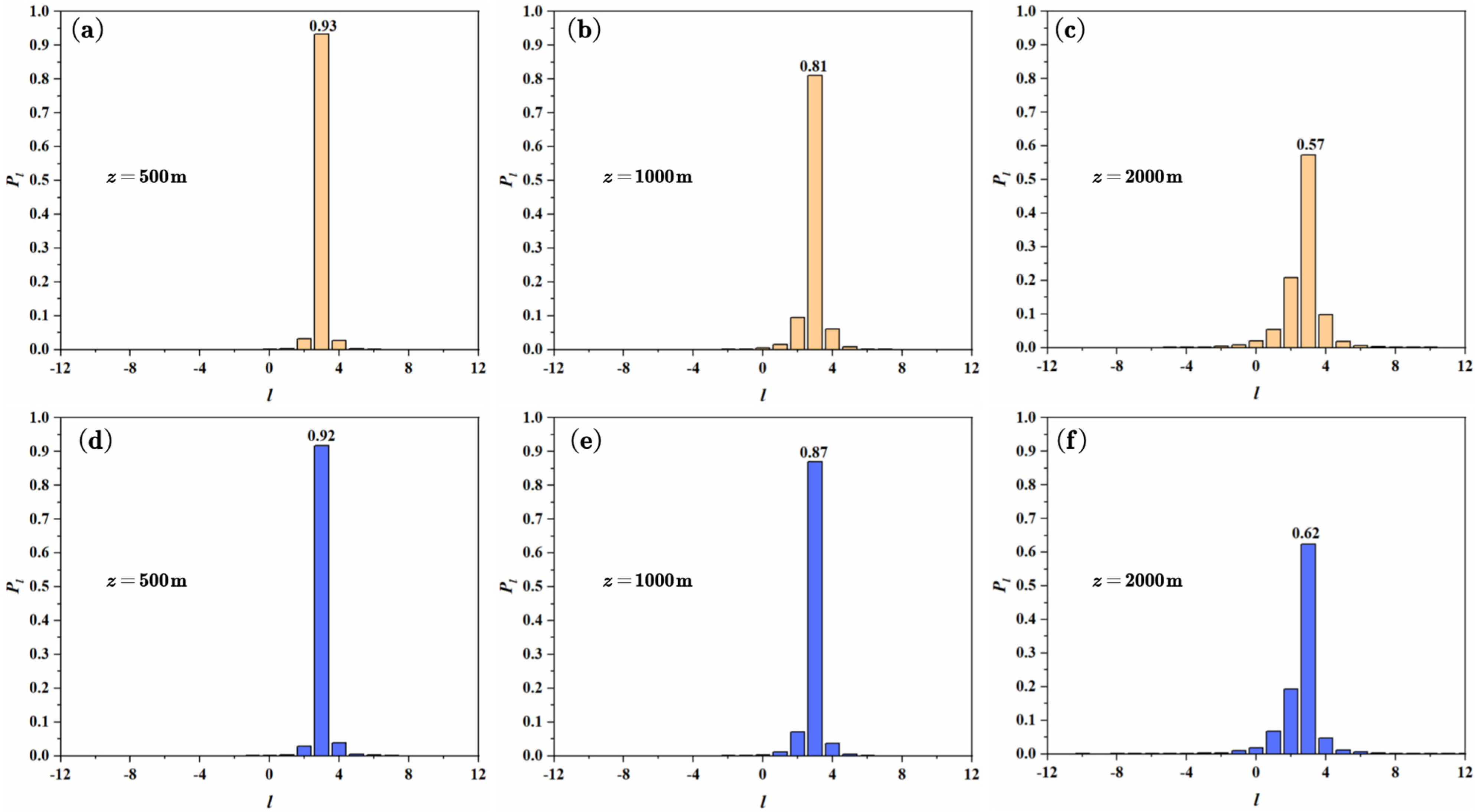
4. Image Transmission Scheme by Employing Coherent Beam Combining
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Allen, L.; Beijersbergen, M.W.; Spreeuw, R.J.C.; Woerdman, J.P. Orbital angular momentum of light and the transformation of Laguerre-Gaussian laser modes. Phys. Rev. A 1992, 45, 8185–8189. [Google Scholar] [CrossRef] [PubMed]
- Yao, A.M.; Padgett, M.J. Orbital angular momentum: Origins, behavior and applications. Adv. Opt. Photon. 2011, 3, 161–204. [Google Scholar] [CrossRef]
- Franke-Arnold, S.; Allen, L.; Padgett, M. Advances in optical angular momentum. Laser Photonics Rev. 2008, 2, 299–313. [Google Scholar] [CrossRef]
- Trichili, A.; Park, K.H.; Zghal, M.; Ooi, B.S.; Alouini, M.S. Communicating Using Spatial Mode Multiplexing: Potentials, Challenges, and Perspectives. IEEE Commun. Surv. Tutor. 2019, 21, 3175–3203. [Google Scholar] [CrossRef]
- Willner, A.E.; Huang, H.; Yan, Y.; Ren, Y.; Ahmed, N.; Xie, G.; Bao, C.; Li, L.; Cao, Y.; Zhao, Z.; et al. Optical communications using orbital angular momentum beams. Adv. Opt. Photon. 2015, 7, 66–106. [Google Scholar] [CrossRef]
- Wang, J. Advances in communications using optical vortices. Photon. Res. 2016, 4, B14–B28. [Google Scholar] [CrossRef]
- Bozinovic, N.; Yue, Y.; Ren, Y.; Tur, M.; Kristensen, P.; Huang, H.; Willner, A.E.; Ramachandran, S. Terabit-Scale Orbital Angular Momentum Mode Division Multiplexing in Fibers. Science 2013, 340, 1545–1548. [Google Scholar] [CrossRef] [PubMed]
- Gibson, G.; Courtial, J.; Padgett, M.J.; Vasnetsov, M.; Pas’ko, V.; Barnett, S.M.; Franke-Arnold, S. Free-space information transfer using light beams carrying orbital angular momentum. Opt. Express 2004, 12, 5448–5456. [Google Scholar] [CrossRef]
- Wang, J.; Yang, J.-Y.; Fazal, I.M.; Ahmed, N.; Yan, Y.; Huang, H.; Ren, Y.; Yue, Y.; Dolinar, S.; Tur, M.; et al. Terabit free-space data transmission employing orbital angular momentum multiplexing. Nat. Photonics 2012, 6, 488–496. [Google Scholar] [CrossRef]
- Wang, J.; Liu, J.; Li, S.; Zhao, Y.; Du, J.; Zhu, L. Orbital angular momentum and beyond in free-space optical communications. Nanophotonics 2022, 11, 645–680. [Google Scholar] [CrossRef]
- Djordjevic, I.B. Deep-space and near-Earth optical communications by coded orbital angular momentum (OAM) modulation. Opt. Express 2011, 19, 14277–14289. [Google Scholar] [CrossRef] [PubMed]
- Sit, A.; Bouchard, F.; Fickler, R.; Gagnon-Bischoff, J.; Larocque, H.; Heshami, K.; Elser, D.; Peuntinger, C.; Günthner, K.; Heim, B.; et al. High-dimensional intracity quantum cryptography with structured photons. Optica 2017, 4, 1006–1010. [Google Scholar] [CrossRef]
- Trichili, A.; Cox, M.A.; Ooi, B.S.; Alouini, M.-S. Roadmap to free space optics. J. Opt. Soc. Am. B 2020, 37, A184–A201. [Google Scholar] [CrossRef]
- Padgett, M.J.; Miatto, F.M.; Lavery, M.P.J.; Zeilinger, A.; Boyd, R.W. Divergence of an orbital-angular-momentum-carrying beam upon propagation. New J. Phys. 2015, 17, 023011. [Google Scholar] [CrossRef]
- Malik, M.; O’Sullivan, M.; Rodenburg, B.; Mirhosseini, M.; Leach, J.; Lavery, M.P.J.; Padgett, M.J.; Boyd, R.W. Influence of atmospheric turbulence on optical communications using orbital angular momentum for encoding. Opt. Express 2012, 20, 13195–13200. [Google Scholar] [CrossRef]
- Anguita, J.A.; Neifeld, M.A.; Vasic, B.V. Turbulence-induced channel crosstalk in an orbital angular momentum-multiplexed free-space optical link. Appl. Opt. 2008, 47, 2414–2429. [Google Scholar] [CrossRef]
- Tyler, G.A.; Boyd, R.W. Influence of atmospheric turbulence on the propagation of quantum states of light carrying orbital angular momentum. Opt. Lett. 2009, 34, 142–144. [Google Scholar] [CrossRef] [PubMed]
- Guo, Y.; Li, L.; Wang, J.; Gao, C.; Fu, S. Distortion Compensation for Orbital Angular Momentum Beams: From Probing to Deep Learning. J. Light. Technol. 2023, 41, 2041–2050. [Google Scholar] [CrossRef]
- Xie, G.; Li, L.; Ren, Y.; Huang, H.; Yan, Y.; Ahmed, N.; Zhao, Z.; Lavery, M.P.J.; Ashrafi, N.; Ashrafi, S.; et al. Performance metrics and design considerations for a free-space optical orbital-angular-momentum-multiplexed communication link. Optica 2015, 2, 357–365. [Google Scholar] [CrossRef]
- Li, L.; Xie, G.; Ren, Y.; Ahmed, N.; Huang, H.; Zhao, Z.; Liao, P.; Lavery, M.P.J.; Yan, Y.; Bao, C.; et al. Orbital-angular-momentum-multiplexed free-space optical communication link using transmitter lenses. Appl. Opt. 2016, 55, 2098–2103. [Google Scholar] [CrossRef]
- Zhu, L.; Wang, A.; Deng, M.; Lu, B.; Guo, X. Free-space optical communication with quasi-ring Airy vortex beam under limited-size receiving aperture and atmospheric turbulence. Opt. Express 2021, 29, 32580–32590. [Google Scholar] [CrossRef] [PubMed]
- Li, L.; Xie, G.; Yan, Y.; Ren, Y.; Liao, P.; Zhao, Z.; Ahmed, N.; Wang, Z.; Bao, C.; Willner, A.J.; et al. Power loss mitigation of orbital-angular-momentum-multiplexed free-space optical links using nonzero radial index Laguerre–Gaussian beams. J. Opt. Soc. Am. B 2017, 34, 1–6. [Google Scholar] [CrossRef]
- Wan, Z.; Shen, Y.; Wang, Z.; Shi, Z.; Liu, Q.; Fu, X. Divergence-degenerate spatial multiplexing towards future ultrahigh capacity, low error-rate optical communications. Light Sci. Appl. 2022, 11, 144. [Google Scholar] [CrossRef] [PubMed]
- Ren, Y.; Xie, G.; Huang, H.; Ahmed, N.; Yan, Y.; Li, L.; Bao, C.; Lavery, M.P.J.; Tur, M.; Neifeld, M.A.; et al. Adaptive-optics-based simultaneous pre- and post-turbulence compensation of multiple orbital-angular-momentum beams in a bidirectional free-space optical link. Optica 2014, 1, 376–382. [Google Scholar] [CrossRef]
- Li, M.; Cvijetic, M.; Takashima, Y.; Yu, Z. Evaluation of channel capacities of OAM-based FSO link with real-time wavefront correction by adaptive optics. Opt. Express 2014, 22, 31337–31346. [Google Scholar] [CrossRef]
- Fu, S.; Zhang, S.; Wang, T.; Gao, C. Pre-turbulence compensation of orbital angular momentum beams based on a probe and the Gerchberg–Saxton algorithm. Opt. Lett. 2016, 41, 3185–3188. [Google Scholar] [CrossRef]
- Zhou, M.; Zhou, Y.; Wu, G.; Cai, Y. Reducing the cross-talk among different orbital angular momentum modes in turbulent atmosphere by using a focusing mirror. Opt. Express 2019, 27, 10280–10287. [Google Scholar] [CrossRef]
- Yuan, Y.; Lei, T.; Li, Z.; Li, Y.; Gao, S.; Xie, Z.; Yuan, X. Beam wander relieved orbital angular momentum communication in turbulent atmosphere using Bessel beams. Sci. Rep. 2017, 7, 42276. [Google Scholar] [CrossRef]
- Zhu, Y.; Zhang, Y.; Yang, G. Evolution of orbital angular momentum mode of the autofocusing Hypergeometric-Gaussian beams through moderate-to-strong anisotropic non-Kolmogorov turbulence. Opt. Commun. 2017, 405, 66–72. [Google Scholar] [CrossRef]
- Yan, X.; Guo, L.; Cheng, M.; Li, J.; Huang, Q.; Sun, R. Probability density of orbital angular momentum mode of autofocusing Airy beam carrying power-exponent-phase vortex through weak anisotropic atmosphere turbulence. Opt. Express 2017, 25, 15286–15298. [Google Scholar] [CrossRef]
- Yue, P.; Hu, J.; Yi, X.; Xu, D.; Liu, Y. Effect of Airy Gaussian vortex beam array on reducing intermode crosstalk induced by atmospheric turbulence. Opt. Express 2019, 27, 37986–37998. [Google Scholar] [CrossRef] [PubMed]
- Cao, J.; Han, L.; Liang, H.; Wu, G.; Pang, X. Orbital angular momentum spectrum of pin-like optical vortex beams in turbulent atmosphere. J. Opt. Soc. Am. A 2022, 39, 1414–1419. [Google Scholar] [CrossRef] [PubMed]
- Fan, T.Y. Laser beam combining for high-power, high-radiance sources. IEEE J. Sel. Top. Quantum Electron. 2005, 11, 567–577. [Google Scholar] [CrossRef]
- Steven Kurti, R.; Halterman, K.; Shori, R.K.; Wardlaw, M.J. Discrete Cylindrical Vector Beam Generation from an Array of Optical Fibers. Opt. Express 2009, 17, 13982–13988. [Google Scholar] [CrossRef] [PubMed]
- Ma, P.; Zhou, P.; Ma, Y.; Wang, X.; Su, R.; Liu, Z. Generation of azimuthally and radially polarized beams by coherent polarization beam combination. Opt. Lett. 2012, 37, 2658–2660. [Google Scholar] [CrossRef] [PubMed]
- Chu, X.; Liu, Z.; Zhou, P. Generation of a high-power Airy beam by coherent combining technology. Laser Phys. Lett. 2013, 10, 125102. [Google Scholar] [CrossRef]
- Veinhard, M.; Bellanger, S.; Daniault, L.; Fsaifes, I.; Bourderionnet, J.; Larat, C.; Lallier, E.; Brignon, A.; Chanteloup, J.-C. Orbital angular momentum beams generation from 61 channels coherent beam combining femtosecond digital laser. Opt. Lett. 2021, 46, 25–28. [Google Scholar] [CrossRef]
- Hou, T.; Zhang, Y.; Chang, Q.; Ma, P.; Su, R.; Wu, J.; Ma, Y.; Zhou, P. High-power vortex beam generation enabled by a phased beam array fed at the nonfocal-plane. Opt. Express 2019, 27, 4046–4059. [Google Scholar] [CrossRef]
- Long, J.; Hou, T.; Chang, Q.; Yu, T.; Su, R.; Ma, P.; Ma, Y.; Zhou, P.; Si, L. Generation of optical vortex lattices by a coherent beam combining system. Opt. Lett. 2021, 46, 3665–3668. [Google Scholar] [CrossRef]
- Yu, T.; Xia, H.; Xie, W.; Xiao, G.; Li, H. The generation and verification of Bessel-Gaussian beam based on coherent beam combining. Results Phys. 2020, 16, 102872. [Google Scholar] [CrossRef]
- Ju, P.; Fan, W.; Gao, W.; Li, Z.; Gao, Q.; Li, G.; Jiang, X.; Zhang, T. Generation of perfect vectorial vortex beams by employing coherent beam combining. Opt. Express 2023, 31, 11885–11898. [Google Scholar] [CrossRef] [PubMed]
- Hou, T.; Chang, Q.; Yu, T.; Long, J.; Chang, H.; Ma, P.; Su, R.; Ma, Y.; Zhou, P. Switching the orbital angular momentum state of light with mode sorting assisted coherent laser array system. Opt. Express 2021, 29, 13428–13440. [Google Scholar] [CrossRef] [PubMed]
- Aksenov, V.P.; Dudorov, V.V.; Kolosov, V.V.; Levitsky, M.E. Synthesized Vortex Beams in the Turbulent Atmosphere. Front. Phys. 2020, 8, 143. [Google Scholar] [CrossRef]
- Zhu, K.; Zhou, G.; Li, X.; Zheng, X.; Tang, H. Propagation of Bessel-Gaussian beams with optical vortices in turbulent atmosphere. Opt. Express 2008, 16, 21315–21320. [Google Scholar] [CrossRef] [PubMed]
- Abeamonwitz, M.; Stegun, I.A. Handbook of Mathematical Functions, 5th ed.; Dover Publications: New York, NY, USA, 1966. [Google Scholar]
- Pinnell, J.; Rodríguez-Fajardo, V.; Forbes, A. How perfect are perfect vortex beams? Opt. Lett. 2019, 44, 5614–5617. [Google Scholar] [CrossRef]
- Paterson, C. Atmospheric Turbulence and Orbital Angular Momentum of Single Photons for Optical Communication. Phys. Rev. Lett. 2005, 94, 153901. [Google Scholar] [CrossRef] [PubMed]
- Molina-Terriza, G.; Torres, J.P.; Torner, L. Management of the Angular Momentum of Light: Preparation of Photons in Multidimensional Vector States of Angular Momentum. Phys. Rev. Lett. 2001, 88, 013601. [Google Scholar] [CrossRef] [PubMed]
- Zhu, Y.; Chen, M.; Zhang, Y.; Li, Y. Propagation of the OAM mode carried by partially coherent modified Bessel–Gaussian beams in an anisotropic non-Kolmogorov marine atmosphere. J. Opt. Soc. Am. A 2016, 33, 2277–2283. [Google Scholar] [CrossRef]
- von Kármán, T. Progress in the Statistical Theory of Turbulence. Proc. National Acad. Sci. USA 1948, 34, 530–539. [Google Scholar] [CrossRef]
- Ostashev, V.E.; Brähler, B.; Mellert, V.; Goedecke, G.H. Coherence functions of plane and spherical waves in a turbulent medium with the von Karman spectrum of medium inhomogeneities. J. Acoust. Soc. Am. 1998, 104, 727–737. [Google Scholar] [CrossRef]
- Fu, S.; Gao, C. Influences of atmospheric turbulence effects on the orbital angular momentum spectra of vortex beams. Photon. Res. 2016, 4, B1–B4. [Google Scholar] [CrossRef]
- Yong, K.; Tang, S.; Yang, X.; Zhang, R. Propagation characteristics of a ring Airy vortex beam in slant atmospheric turbulence. J. Opt. Soc. Am. B 2021, 38, 1510–1517. [Google Scholar] [CrossRef]
- Fleck, J.A.; Morris, J.R.; Feit, M.D. Time-dependent propagation of high energy laser beams through the atmosphere. Appl. Phys. 1976, 10, 129–160. [Google Scholar] [CrossRef]
- Zhu, Z.; Janasik, M.; Fyffe, A.; Hay, D.; Zhou, Y.; Kantor, B.; Winder, T.; Boyd, R.W.; Leuchs, G.; Shi, Z. Compensation-free high-dimensional free-space optical communication using turbulence-resilient vector beams. Nat. Commun. 2021, 12, 1666. [Google Scholar] [CrossRef] [PubMed]






Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ju, P.; Fan, W.; Gao, W.; Li, Z.; Gao, Q.; Jiang, X.; Zhang, T. Atmospheric Turbulence Effects on the Performance of Orbital Angular Momentum Multiplexed Free-Space Optical Links Using Coherent Beam Combining. Photonics 2023, 10, 634. https://doi.org/10.3390/photonics10060634
Ju P, Fan W, Gao W, Li Z, Gao Q, Jiang X, Zhang T. Atmospheric Turbulence Effects on the Performance of Orbital Angular Momentum Multiplexed Free-Space Optical Links Using Coherent Beam Combining. Photonics. 2023; 10(6):634. https://doi.org/10.3390/photonics10060634
Chicago/Turabian StyleJu, Pei, Wenhui Fan, Wei Gao, Zhe Li, Qi Gao, Xiaoqiang Jiang, and Tongyi Zhang. 2023. "Atmospheric Turbulence Effects on the Performance of Orbital Angular Momentum Multiplexed Free-Space Optical Links Using Coherent Beam Combining" Photonics 10, no. 6: 634. https://doi.org/10.3390/photonics10060634
APA StyleJu, P., Fan, W., Gao, W., Li, Z., Gao, Q., Jiang, X., & Zhang, T. (2023). Atmospheric Turbulence Effects on the Performance of Orbital Angular Momentum Multiplexed Free-Space Optical Links Using Coherent Beam Combining. Photonics, 10(6), 634. https://doi.org/10.3390/photonics10060634




