Single-Layered Phase-Change Metasurfaces Achieving Polarization- and Crystallinity-Dependent Wavefront Manipulation
Abstract
1. Introduction
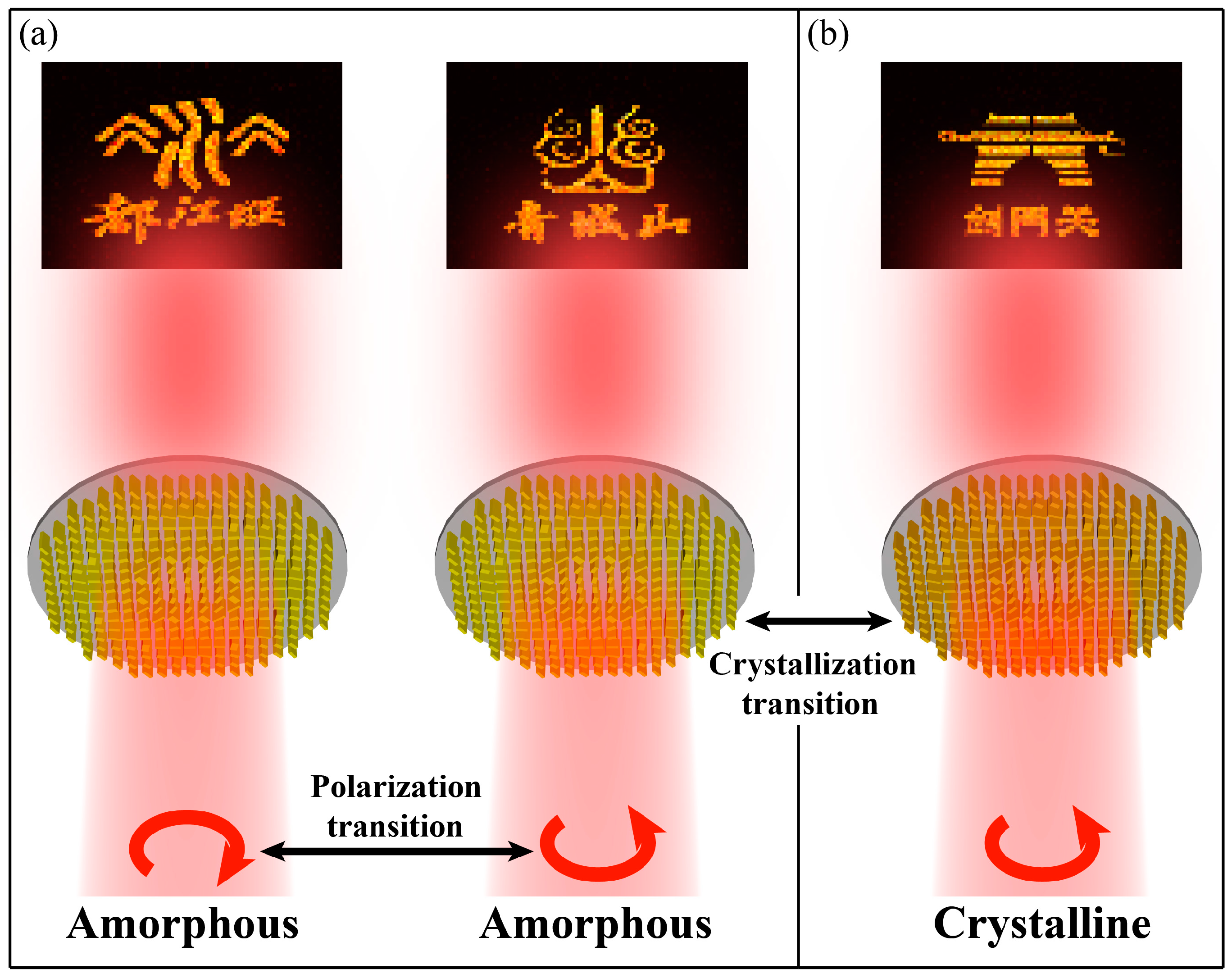
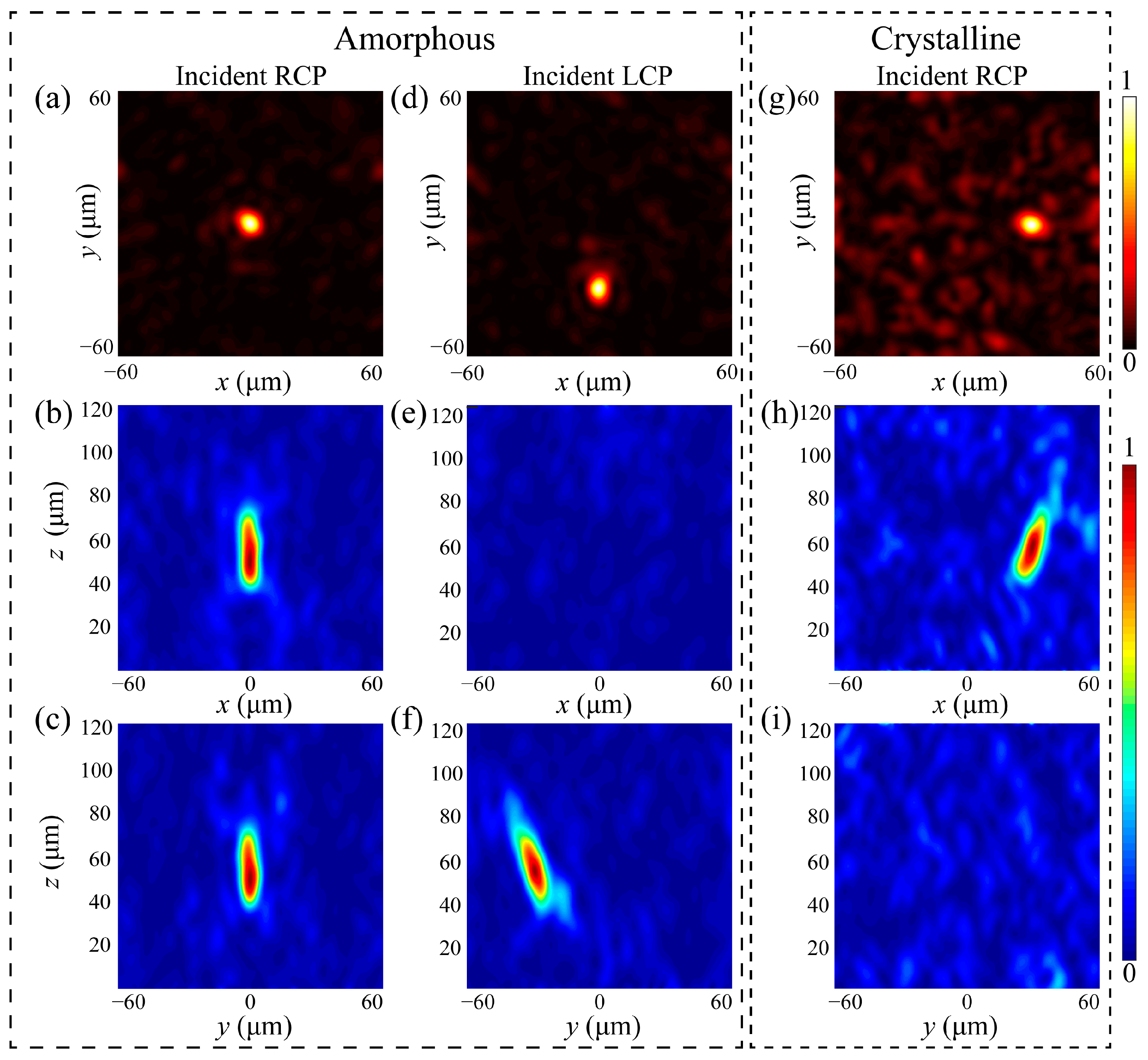
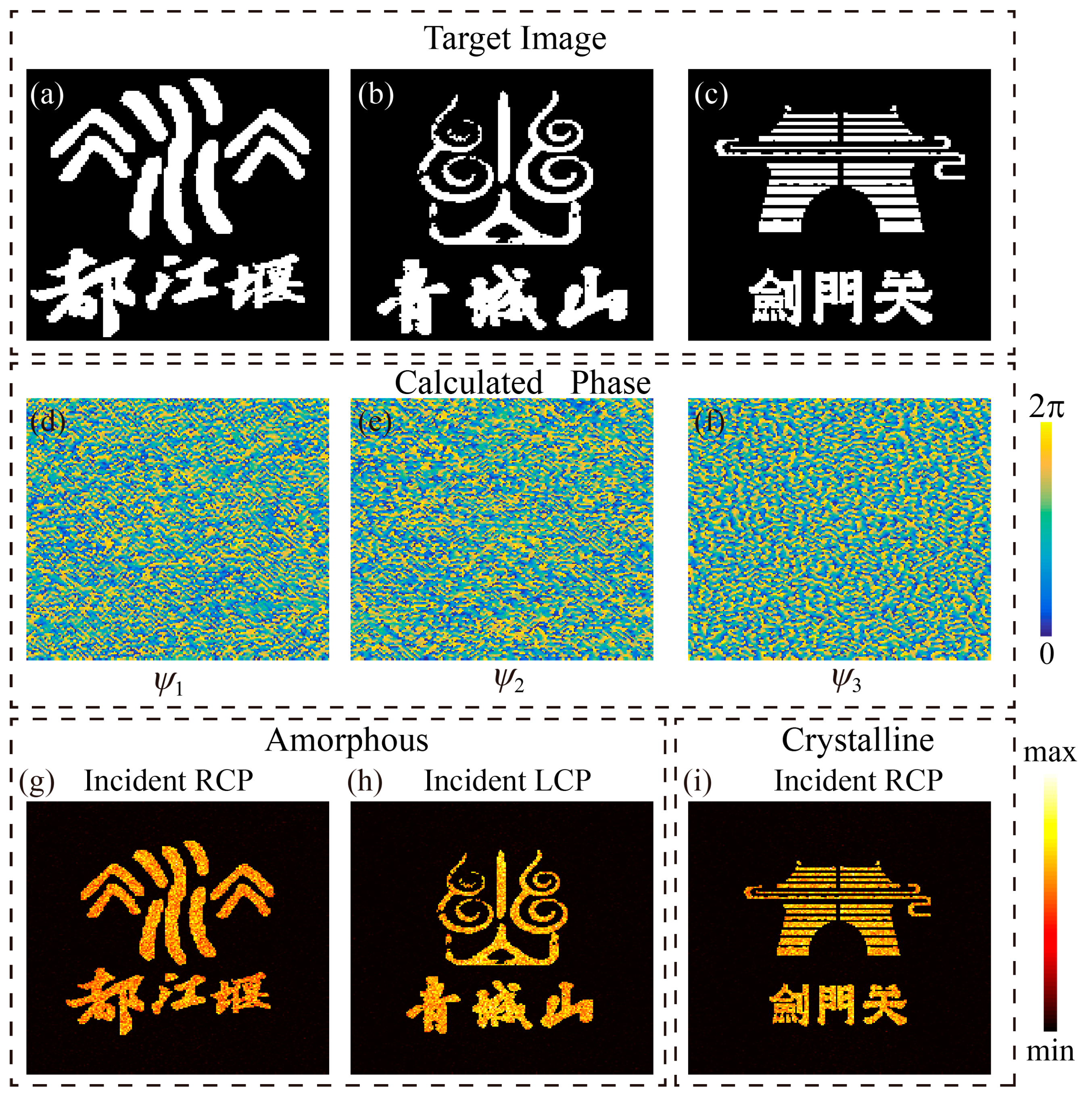
2. Design Principles and Simulation Results
3. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Luo, X. Metamaterials and Metasurfaces. Adv. Opt. Mater. 2019, 7, 1900885. [Google Scholar] [CrossRef]
- Yu, P.; Besteiro, L.V.; Huang, Y.; Wu, J.; Fu, L.; Tan, H.H.; Jagadish, C.; Wiederrecht, G.P.; Govorov, A.O.; Wang, Z. Broadband Metamaterial Absorbers. Adv. Opt. Mater. 2019, 7, 1800995. [Google Scholar] [CrossRef]
- Genevet, P.; Capasso, F.; Aieta, F.; Khorasaninejad, M.; Devlin, R. Recent Advances in Planar Optics: From Plasmonic to Dielectric Metasurfaces. Optica 2017, 4, 139–152. [Google Scholar] [CrossRef]
- Chen, W.T.; Zhu, A.Y.; Capasso, F. Flat Optics with Dispersion-Engineered Metasurfaces. Nat. Rev. Mater. 2020, 5, 604–620. [Google Scholar] [CrossRef]
- Hsiao, H.-H.; Chu, C.H.; Tsai, D.P. Fundamentals and Applications of Metasurfaces. Small Methods 2017, 1, 1600064. [Google Scholar] [CrossRef]
- Chen, M.K.; Wu, Y.; Feng, L.; Fan, Q.; Lu, M.; Xu, T.; Tsai, D.P. Principles, Functions, and Applications of Optical Meta-Lens. Adv. Opt. Mater. 2021, 9, 2001414. [Google Scholar] [CrossRef]
- Cheng, H.; Liu, Z.; Chen, S.; Tian, J. Emergent Functionality and Controllability in Few-Layer Metasurfaces. Adv. Mater. 2015, 27, 5410–5421. [Google Scholar] [CrossRef] [PubMed]
- Yu, N.; Genevet, P.; Kats, M.A.; Aieta, F.; Tetienne, J.-P.; Capasso, F.; Gaburro, Z. Light Propagation with Phase Discontinuities: Generalized Laws of Reflection and Refraction. Science 2011, 6054, 333–337. [Google Scholar] [CrossRef] [PubMed]
- Shi, T.; Wang, Y.; Deng, Z.-L.; Ye, X.; Dai, Z.; Cao, Y.; Guan, B.-O.; Xiao, S.; Li, X. All-Dielectric Kissing-Dimer Metagratings for Asymmetric High Diffraction. Adv. Opt. Mater. 2019, 7, 1901389. [Google Scholar] [CrossRef]
- Huang, Y.; Luo, J.; Pu, M.; Guo, Y.; Zhao, Z.; Ma, X.; Li, X.; Luo, X. Catenary Electromagnetics for Ultra-Broadband Lightweight Absorbers and Large-Scale Flat Antennas. Adv. Sci. 2019, 6, 1801691. [Google Scholar] [CrossRef]
- Huang, Y.; Liu, L.; Pu, M.; Li, X.; Ma, X.; Luo, X. A Refractory Metamaterial Absorber for Ultra-Broadband, Omnidirectional and Polarization-Independent Absorption in the UV-NIR Spectrum. Nanoscale 2018, 10, 8298–8303. [Google Scholar] [CrossRef]
- Song, M.; Feng, L.; Huo, P.; Liu, M.; Huang, C.; Yan, F.; Lu, Y.; Xu, T. Versatile Full-Colour Nanopainting Enabled by a Pixelated Plasmonic Metasurface. Nat. Nanotechnol. 2023, 18, 71–78. [Google Scholar] [CrossRef]
- Huo, P.; Song, M.; Zhu, W.; Zhang, C.; Chen, L.; Lezec, H.J.; Lu, Y.; Agrawal, A.; Xu, T. Photorealistic Full-Color Nanopainting Enabled by a Low-Loss Metasurface. Optica 2020, 7, 1171–1172. [Google Scholar] [CrossRef] [PubMed]
- Fu, R.; Chen, K.; Li, Z.; Yu, S.; Zheng, G. Metasurface-Based Nanoprinting: Principle, Design and Advances. Opto-Electron. Sci. 2022, 1, 220011. [Google Scholar] [CrossRef]
- Adibi, S.; Honarvar, M.A.; Lalbakhsh, A. Lalbakhsh Gain Enhancement of Wideband Circularly Polarized UWB Antenna Using FSS. Radio Sci. 2021, 56, e2020RS007098. [Google Scholar] [CrossRef]
- Lalbakhsh, A.; Afzal, M.U.; Hayat, T.; Esselle, K.P.; Mandal, K. All-Metal Wideband Metasurface for near-Field Transformation of Medium-to-High Gain Electromagnetic Sources. Sci. Rep. 2021, 11, 9421. [Google Scholar] [CrossRef]
- Paul, G.S.; Mandal, K.; Lalbakhsh, A. Single-Layer Ultra-Wide Stop-Band Frequency Selective Surface Using Interconnected Square Rings. AEU-Int. J. Electron. Commun. 2021, 132, 153630. [Google Scholar] [CrossRef]
- Wang, S.; Wu, P.C.; Su, V.-C.; Lai, Y.-C.; Chen, M.-K.; Kuo, H.Y.; Chen, B.H.; Chen, Y.H.; Huang, T.-T.; Wang, J.-H.; et al. A Broadband Achromatic Metalens in the Visible. Nat. Nanotechnol. 2018, 13, 227–232. [Google Scholar] [CrossRef] [PubMed]
- Huo, P.; Zhang, C.; Zhu, W.; Liu, M.; Zhang, S.; Zhang, S.; Chen, L.; Lezec, H.J.; Agrawal, A.; Lu, Y.; et al. Photonic Spin-Multiplexing Metasurface for Switchable Spiral Phase Contrast Imaging. Nano Lett. 2020, 20, 2791–2798. [Google Scholar] [CrossRef]
- Zhang, Y.; Pu, M.; Jin, J.; Lu, X.; Guo, Y.; Cai, J.; Zhang, F.; Ha, Y.; He, Q.; Xu, M.; et al. Crosstalk-Free Achromatic Full Stokes Imaging Polarimetry Metasurface Enabled by Polarization-Dependent Phase Optimization. Opto-Electron. Adv. 2022, 5, 220058. [Google Scholar] [CrossRef]
- Zhang, X.; Pu, M.; Guo, Y.; Jin, J.; Li, X.; Ma, X.; Luo, J.; Wang, C.; Luo, X. Colorful Metahologram with Independently Controlled Images in Transmission and Reflection Spaces. Adv. Funct. Mater. 2019, 29, 1809145. [Google Scholar] [CrossRef]
- Sun, Q.; Zhang, Z.; Huang, Y.; Ma, X.; Pu, M.; Guo, Y.; Li, X.; Luo, X. Asymmetric Transmission and Wavefront Manipulation toward Dual-Frequency Meta-Holograms. ACS Photonics 2019, 6, 1541–1546. [Google Scholar] [CrossRef]
- Wang, D.; Hwang, Y.; Dai, Y.; Si, G.; Wei, S.; Choi, D.-Y.; Gómez, D.E.; Mitchell, A.; Lin, J.; Yuan, X. Broadband High-Efficiency Chiral Splitters and Holograms from Dielectric Nanoarc Metasurfaces. Small 2019, 15, 1900483. [Google Scholar] [CrossRef] [PubMed]
- Yang, Y.; Jing, L.; Zheng, B.; Hao, R.; Yin, W.; Li, E.; Soukoulis, C.M.; Chen, H. Full-Polarization 3D Metasurface Cloak with Preserved Amplitude and Phase. Adv. Mater. 2016, 28, 6866–6871. [Google Scholar] [CrossRef] [PubMed]
- Huang, Y.; Pu, M.; Zhang, F.; Luo, J.; Li, X.; Ma, X.; Luo, X. Broadband Functional Metasurfaces: Achieving Nonlinear Phase Generation toward Achromatic Surface Cloaking and Lensing. Adv. Opt. Mater. 2019, 7, 1801480. [Google Scholar] [CrossRef]
- Chen, K.; Ding, G.; Hu, G.; Jin, Z.; Zhao, J.; Feng, Y.; Jiang, T.; Alù, A.; Qiu, C.-W. Directional Janus Metasurface. Adv. Mater. 2020, 32, 1906352. [Google Scholar] [CrossRef]
- Tang, Z.; Li, L.; Zhang, H.; Yang, J.; Hu, J.; Lu, X.; Hu, Y.; Qi, S.; Liu, K.; Tian, M.; et al. Multifunctional Janus Metasurfaces Achieving Arbitrary Wavefront Manipulation at Dual Frequency. Mater. Des. 2022, 223, 111264. [Google Scholar] [CrossRef]
- Huang, Y.; Xiao, T.; Chen, S.; Xie, Z.; Zheng, J.; Zhu, J.; Su, Y.; Chen, W.; Liu, K.; Tang, M.; et al. All-Optical Controlled-NOT Logic Gate Achieving Directional Asymmetric Transmission Based on Metasurface Doublet. Opto-Electron. Adv. 2023, 6, 220073. [Google Scholar] [CrossRef]
- Lalbakhsh, A.; Simorangkir, R.B.V.B.; Bayat-Makou, N.; Kishk, A.A.; Esselle, K.P. Chapter 2—Advancements and Artificial Intelligence Approaches in Antennas for Environmental Sensing. In Artificial Intelligence and Data Science in Environmental Sensing; Asadnia, M., Razmjou, A., Beheshti, A., Eds.; Academic Press: Cambridge, MA, USA, 2022; pp. 19–38. ISBN 978-0-323-90508-4. [Google Scholar]
- Naveed, M.A.; Kim, J.; Javed, I.; Ansari, M.A.; Seong, J.; Massoud, Y.; Badloe, T.; Kim, I.; Riaz, K.; Zubair, M.; et al. Novel Spin-Decoupling Strategy in Liquid Crystal-Integrated Metasurfaces for Interactive Metadisplays. Adv. Opt. Mater. 2022, 10, 2200196. [Google Scholar] [CrossRef]
- Naveed, M.A.; Ansari, M.A.; Kim, I.; Badloe, T.; Kim, J.; Oh, D.K.; Riaz, K.; Tauqeer, T.; Younis, U.; Saleem, M.; et al. Optical Spin-Symmetry Breaking for High-Efficiency Directional Helicity-Multiplexed Metaholograms. Microsyst. Nanoeng. 2021, 7, 5. [Google Scholar] [CrossRef]
- Nemati, A.; Wang, Q.; Hong, M.; Teng, J. Tunable and Reconfigurable Metasurfaces and Metadevices. Opto-Electron. Adv. 2018, 1, 180009. [Google Scholar] [CrossRef]
- Esfandiyari, M.; Lalbakhsh, A.; Jarchi, S.; Ghaffari-Miab, M.; Mahtaj, H.N.; Simorangkir, R.B.V.B. Tunable Terahertz Filter/Antenna-Sensor Using Graphene-Based Metamaterials. Mater. Des. 2022, 220, 110855. [Google Scholar] [CrossRef]
- Esfandiari, M.; Lalbakhsh, A.; Nasiri Shehni, P.; Jarchi, S.; Ghaffari-Miab, M.; Noori Mahtaj, H.; Reisenfeld, S.; Alibakhshikenari, M.; Koziel, S.; Szczepanski, S. Recent and Emerging Applications of Graphene-Based Metamaterials in Electromagnetics. Mater. Des. 2022, 221, 110920. [Google Scholar] [CrossRef]
- Huang, Y.; Xiao, T.; Xie, Z.; Zheng, J.; Su, Y.; Chen, W.; Liu, K.; Tang, M.; Zhu, J.; Müller-Buschbaum, P.; et al. Multistate Nonvolatile Metamirrors with Tunable Optical Chirality. ACS Appl. Mater. Interfaces 2021, 13, 45890–45897. [Google Scholar] [CrossRef]
- Huang, Y.; Xiao, T.; Xie, Z.; Zheng, J.; Su, Y.; Chen, W.; Liu, K.; Tang, M.; Zhu, J.; Li, L. Reconfigurable Phase-Change Metasurfaces from Efficient Wavefront Manipulation to Perfect Absorption. J. Mater. Sci. 2022, 57, 5426–5437. [Google Scholar] [CrossRef]
- Zhang, F.; Xie, X.; Pu, M.; Guo, Y.; Ma, X.; Li, X.; Luo, J.; He, Q.; Yu, H.; Luo, X. Multistate Switching of Photonic Angular Momentum Coupling in Phase-Change Metadevices. Adv. Mater. 2020, 32, 1908194. [Google Scholar] [CrossRef] [PubMed]
- Choi, C.; Lee, S.-Y.; Mun, S.-E.; Lee, G.-Y.; Sung, J.; Yun, H.; Yang, J.-H.; Kim, H.-O.; Hwang, C.-Y.; Lee, B. Metasurface with Nanostructured Ge2Sb2Te5 as a Platform for Broadband-Operating Wavefront Switch. Adv. Opt. Mater. 2019, 7, 1900171. [Google Scholar] [CrossRef]
- Moitra, P.; Wang, Y.; Liang, X.; Lu, L.; Poh, A.; Mass, T.W.W.; Simpson, R.E.; Kuznetsov, A.I.; Paniagua-Dominguez, R. Programmable Wavefront Control in the Visible Spectrum Using Low-Loss Chalcogenide Phase-Change Metasurfaces. Adv. Mater. 2022, 2205367. [Google Scholar] [CrossRef]
- Liu, T.; Han, Z.; Duan, J.; Xiao, S. Phase-Change Metasurfaces for Dynamic Image Display and Information Encryption. Phys. Rev. Appl. 2022, 18, 044078. [Google Scholar] [CrossRef]
- Ding, F.; Zhong, S.; Bozhevolnyi, S.I. Vanadium Dioxide Integrated Metasurfaces with Switchable Functionalities at Terahertz Frequencies. Adv. Opt. Mater. 2018, 6, 1701204. [Google Scholar] [CrossRef]
- Li, X.; Tang, S.; Ding, F.; Zhong, S.; Yang, Y.; Jiang, T.; Zhou, J. Switchable Multifunctional Terahertz Metasurfaces Employing Vanadium Dioxide. Sci. Rep. 2019, 9, 5454. [Google Scholar] [CrossRef] [PubMed]
- Abdollahramezani, S.; Hemmatyar, O.; Taghinejad, M.; Taghinejad, H.; Krasnok, A.; Eftekhar, A.A.; Teichrib, C.; Deshmukh, S.; El-Sayed, M.; Pop, E.; et al. Electrically Driven Programmable Phase-Change Meta-Switch Reaching 80% Efficiency. arXiv 2021, arXiv:2104.10381. [Google Scholar]
- Zhang, Y.; Fowler, C.; Liang, J.; Azhar, B.; Shalaginov, M.Y.; Deckoff-Jones, S.; An, S.; Chou, J.B.; Roberts, C.M.; Liberman, V.; et al. Electrically Reconfigurable Non-Volatile Metasurface Using Low-Loss Optical Phase-Change Material. Nat. Nanotechnol. 2021, 16, 661–666. [Google Scholar] [CrossRef]
- Wang, Y.; Landreman, P.; Schoen, D.; Okabe, K.; Marshall, A.; Celano, U.; Wong, H.-S.P.; Park, J.; Brongersma, M.L. Electrical Tuning of Phase-Change Antennas and Metasurfaces. Nat. Nanotechnol. 2021, 16, 667–672. [Google Scholar] [CrossRef]
- Abdollahramezani, S.; Hemmatyar, O.; Taghinejad, M.; Taghinejad, H.; Kiarashinejad, Y.; Zandehshahvar, M.; Fan, T.; Deshmukh, S.; Eftekhar, A.A.; Cai, W.; et al. Dynamic Hybrid Metasurfaces. Nano Lett. 2021, 21, 1238–1245. [Google Scholar] [CrossRef] [PubMed]
- Zhang, M.; Pu, M.; Zhang, F.; Guo, Y.; He, Q.; Ma, X.; Huang, Y.; Li, X.; Yu, H.; Luo, X. Plasmonic Metasurfaces for Switchable Photonic Spin–Orbit Interactions Based on Phase Change Materials. Adv. Sci. 2018, 5, 1800835. [Google Scholar] [CrossRef] [PubMed]
- Shalaginov, M.Y.; An, S.; Zhang, Y.; Yang, F.; Su, P.; Liberman, V.; Chou, J.B.; Roberts, C.M.; Kang, M.; Rios, C.; et al. Reconfigurable All-Dielectric Metalens with Diffraction-Limited Performance. Nat. Commun. 2021, 12, 1225. [Google Scholar] [CrossRef] [PubMed]
- Liu, M.; Plum, E.; Li, H.; Duan, S.; Li, S.; Xu, Q.; Zhang, X.; Zhang, C.; Zou, C.; Jin, B.; et al. Switchable Chiral Mirrors. Adv. Opt. Mater. 2020, 8, 2000247. [Google Scholar] [CrossRef]
- Carrillo, S.G.-C.; Trimby, L.; Au, Y.-Y.; Nagareddy, V.K.; Rodriguez-Hernandez, G.; Hosseini, P.; Ríos, C.; Bhaskaran, H.; Wright, C.D. A Nonvolatile Phase-Change Metamaterial Color Display. Adv. Opt. Mater. 2019, 7, 1801782. [Google Scholar] [CrossRef]
- Palik, E.D. Handbook of Optical Constants of Solids; Academic Press: Cambridge, MA, USA, 1998; Volume 3, ISBN 0-12-544423-0. [Google Scholar]
- Zhou, C.; Xie, Z.; Zhang, B.; Lei, T.; Li, Z.; Du, L.; Yuan, X. Reconfigurable Dielectric Metasurface for Active Wavefront Modulation Based on a Phase-Change Material Metamolecule Design. Opt. Express 2020, 28, 38241–38251. [Google Scholar] [CrossRef]
- Zhang, Y.; Chou, J.B.; Li, J.; Li, H.; Du, Q.; Yadav, A.; Zhou, S.; Shalaginov, M.Y.; Fang, Z.; Zhong, H.; et al. Broadband Transparent Optical Phase Change Materials for High-Performance Nonvolatile Photonics. Nat. Commun. 2019, 10, 4279. [Google Scholar] [CrossRef]
- Zhang, F.; Pu, M.; Luo, J.; Yu, H.; Luo, X. Symmetry Breaking of Photonic Spin-Orbit Interactions in Metasurfaces. Opto-Electron. Eng. 2017, 44, 319–325. [Google Scholar] [CrossRef]
- Qin, F.; Liu, B.; Zhu, L.; Lei, J.; Fang, W.; Hu, D.; Zhu, Y.; Ma, W.; Wang, B.; Shi, T.; et al. π-Phase Modulated Monolayer Supercritical Lens. Nat. Commun. 2021, 12, 32. [Google Scholar] [CrossRef]
- Khorasaninejad, M.; Shi, Z.; Zhu, A.Y.; Chen, W.T.; Sanjeev, V.; Zaidi, A.; Capasso, F. Achromatic Metalens over 60 Nm Bandwidth in the Visible and Metalens with Reverse Chromatic Dispersion. Nano Lett. 2017, 17, 1819–1824. [Google Scholar] [CrossRef] [PubMed]
- Huang, Y.; Xiao, T.; Xie, Z.; Zheng, J.; Su, Y.; Chen, W.; Liu, K.; Tang, M.; Müller-Buschbaum, P.; Li, L. Single-Layered Reflective Metasurface Achieving Simultaneous Spin-Selective Perfect Absorption and Efficient Wavefront Manipulation. Adv. Opt. Mater. 2021, 9, 2001663. [Google Scholar] [CrossRef]
- Pu, M.; Li, X.; Ma, X.; Wang, Y.; Zhao, Z.; Wang, C.; Hu, C.; Gao, P.; Huang, C.; Ren, H.; et al. Catenary Optics for Achromatic Generation of Perfect Optical Angular Momentum. Sci. Adv. 2015, 1, e1500396. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hu, J.; Chen, Y.; Zhang, W.; Tang, Z.; Lan, X.; Deng, Q.; Cui, H.; Li, L.; Huang, Y. Single-Layered Phase-Change Metasurfaces Achieving Polarization- and Crystallinity-Dependent Wavefront Manipulation. Photonics 2023, 10, 344. https://doi.org/10.3390/photonics10030344
Hu J, Chen Y, Zhang W, Tang Z, Lan X, Deng Q, Cui H, Li L, Huang Y. Single-Layered Phase-Change Metasurfaces Achieving Polarization- and Crystallinity-Dependent Wavefront Manipulation. Photonics. 2023; 10(3):344. https://doi.org/10.3390/photonics10030344
Chicago/Turabian StyleHu, Jie, Yujie Chen, Wenting Zhang, Ziyi Tang, Xiang Lan, Qinrong Deng, Hengyu Cui, Ling Li, and Yijia Huang. 2023. "Single-Layered Phase-Change Metasurfaces Achieving Polarization- and Crystallinity-Dependent Wavefront Manipulation" Photonics 10, no. 3: 344. https://doi.org/10.3390/photonics10030344
APA StyleHu, J., Chen, Y., Zhang, W., Tang, Z., Lan, X., Deng, Q., Cui, H., Li, L., & Huang, Y. (2023). Single-Layered Phase-Change Metasurfaces Achieving Polarization- and Crystallinity-Dependent Wavefront Manipulation. Photonics, 10(3), 344. https://doi.org/10.3390/photonics10030344





