Spin Hall Effect of Two-Index Paraxial Vector Propagation-Invariant Beams
Abstract
:1. Introduction
2. Field Components and Intensity Distribution of the Two-Index Vector Laguerre–Gaussian Beams
3. Spin Angular Momentum Density of the Two-Index Vector Beams
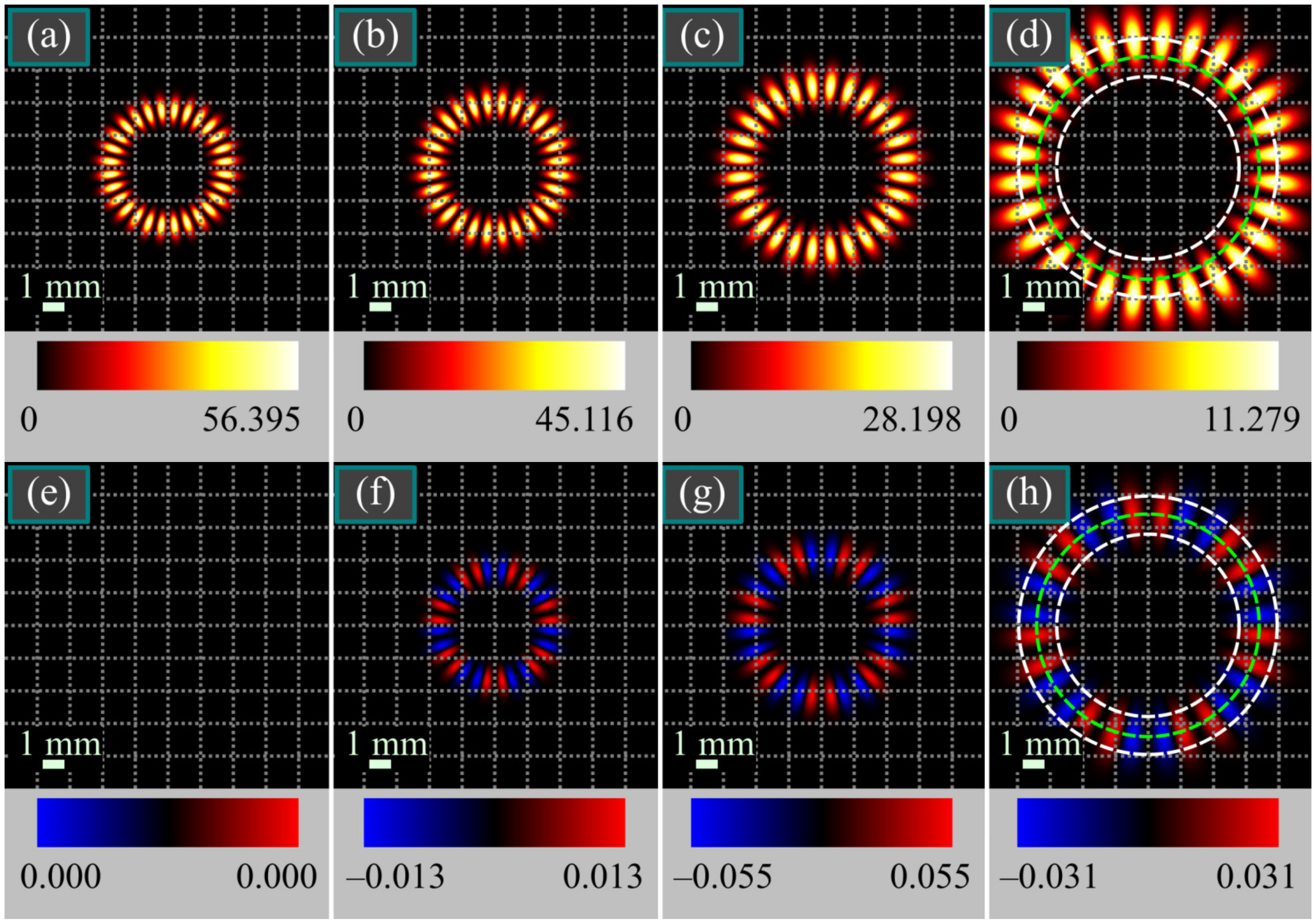
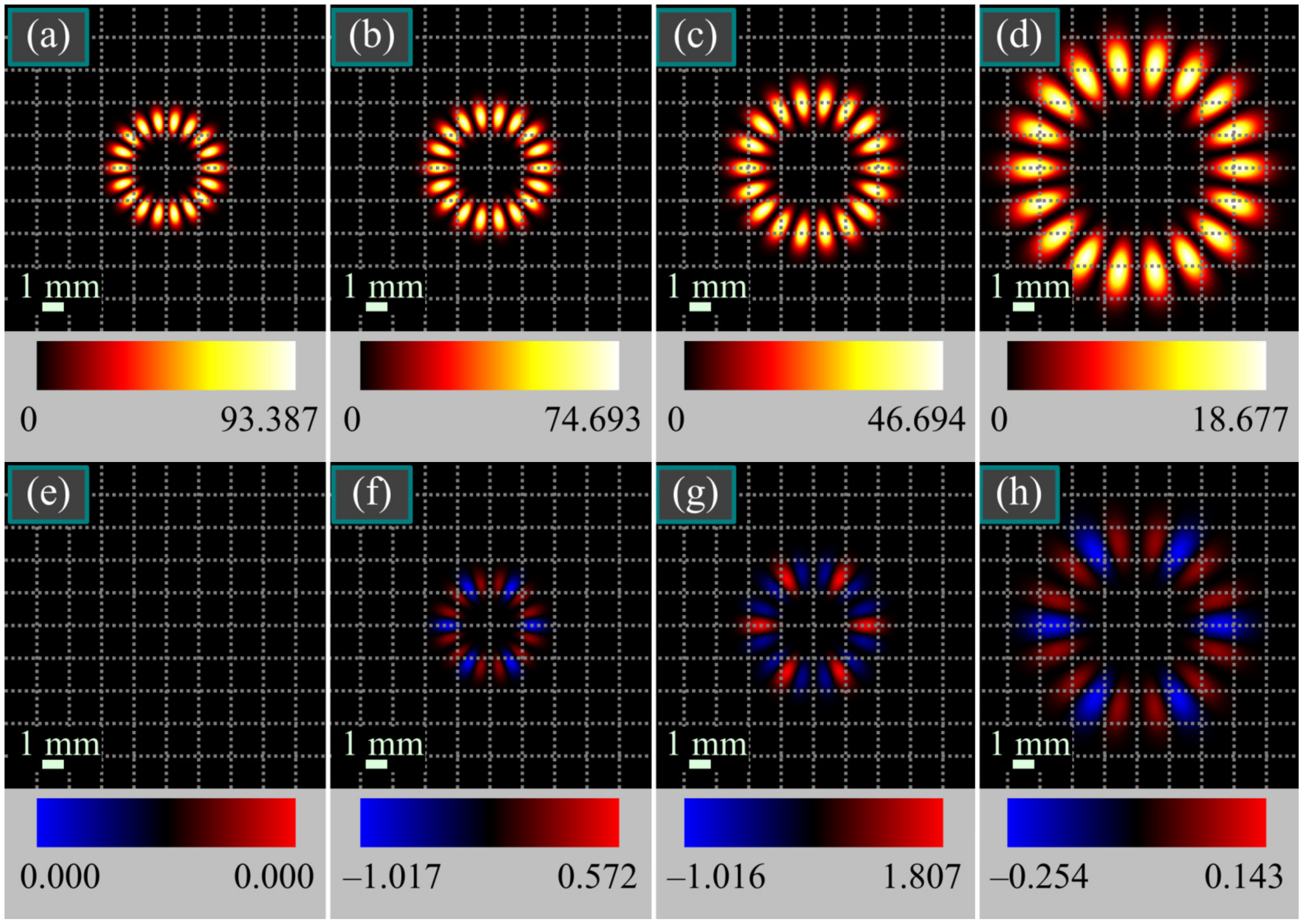
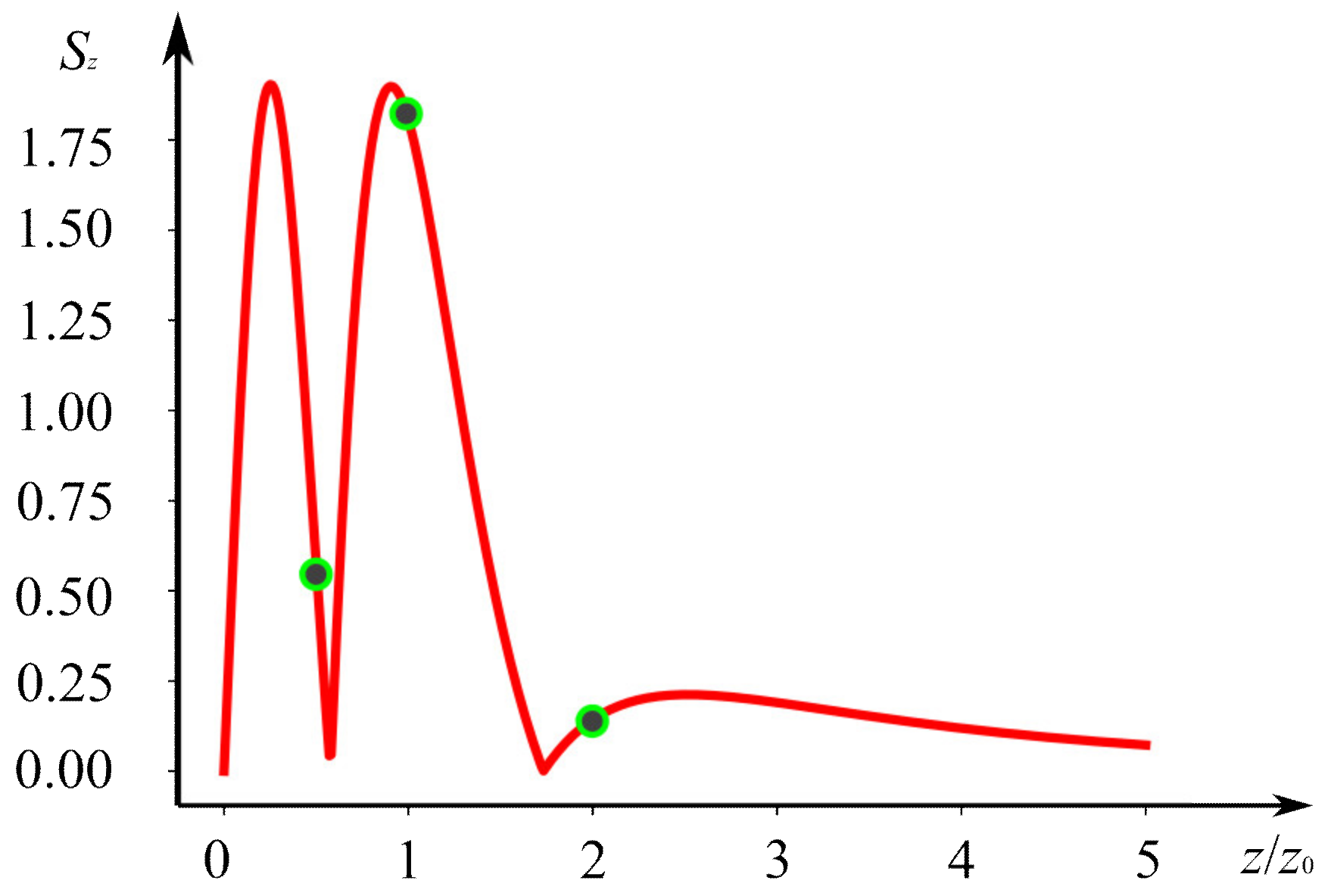
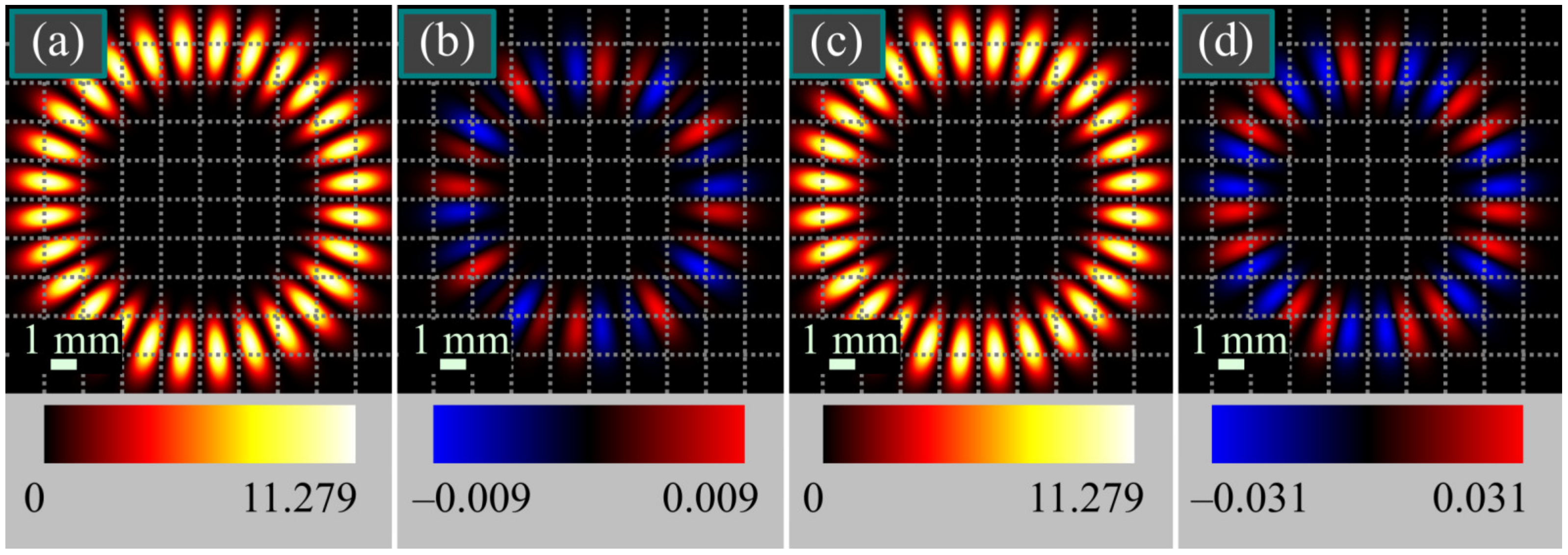
4. Numerical Simulation
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kovalev, A.A.; Kotlyar, V.V. Spin Hall effect of double-index cylindrical vector beams in a tight focus. Micromachines 2023, 14, 494. [Google Scholar] [CrossRef] [PubMed]
- Kovalev, A.A.; Kotlyar, V.V.; Stafeev, S.S. Spin Hall effect in the paraxial light beams with multiple polarization singularities. Micromachines 2023, 14, 777. [Google Scholar] [CrossRef] [PubMed]
- Kovalev, A.A.; Kotlyar, V.V.; Nalimov, A.G. Spin Hall effect in paraxial vectorial light beams with an infinite number of polarization singularities. Micromachines 2023, 14, 1470. [Google Scholar] [CrossRef]
- Kavokin, A.; Malpuech, G.; Glazov, M. Optical spin Hall effect. Phys. Rev. Lett. 2005, 95, 136601. [Google Scholar] [CrossRef] [PubMed]
- Kim, M.; Lee, D.; Kim, T.H.; Yang, Y.; Park, H.J.; Rho, J. Observation of enhanced optical spin Hall effect in a vertical hyperbolic metamaterial. ACS Photonics 2019, 6, 2530–2536. [Google Scholar] [CrossRef]
- Kim, M.; Lee, D.; Ko, B.; Rho, J. Diffraction-induced enhancement of optical spin Hall effect in a dielectric grating. APL Photonics 2020, 5, 066106. [Google Scholar] [CrossRef]
- Stafeev, S.S.; Nalimov, A.G.; Kovalev, A.A.; Zaitsev, V.D.; Kotlyar, V.V. Circular polarization near the tight focus of linearly polarized light. Photonics 2022, 9, 196. [Google Scholar] [CrossRef]
- Youngworth, K.S.; Brown, T.G. Focusing of high numerical aperture cylindrical-vector beams. Opt. Express 2000, 7, 77–87. [Google Scholar] [CrossRef]
- Han, L.; Liu, S.; Li, P.; Zhang, Y.; Cheng, H.; Zhao, J. Catalystlike effect of orbital angular momentum on the conversion of transverse to three-dimensional spin states within tightly focused radially polarized beams. Phys. Rev. A 2018, 97, 053802. [Google Scholar] [CrossRef]
- Li, H.; Ma, C.; Wang, J.; Tang, M.; Li, X. Spin-orbit Hall effect in the tight focusing of a radially polarized vortex beam. Opt. Express 2021, 29, 39419–39427. [Google Scholar] [CrossRef]
- Zhang, X.; Shen, B.; Zhu, Z.; Rui, G.; He, J.; Cui, Y.; Gu, B. Understanding of transverse spin angular momentum in tightly focused linearly polarized vortex beams. Opt. Express 2022, 30, 5121–5130. [Google Scholar] [CrossRef] [PubMed]
- Li, M.; Cai, Y.; Yan, S.; Liang, Y.; Zhang, P.; Yao, B. Orbit-induced localized spin angular momentum in strong focusing of optical vectorial vortex beams. Phys. Rev. A 2018, 97, 053842. [Google Scholar] [CrossRef]
- Meng, P.; Man, Z.; Konijnenberg, A.P.; Urbach, H.P. Angular momentum properties of hybrid cylindrical vector vortex beams in tightly focused optical systems. Opt. Express 2019, 27, 35336–35348. [Google Scholar] [CrossRef] [PubMed]
- Li, M.; Yan, S.; Yao, B.; Liang, Y.; Zhang, P. Spinning and orbiting motion of particles in vortex beams with circular or radial polarizations. Opt. Express 2016, 24, 20604–20612. [Google Scholar] [CrossRef]
- Chen, R.P.; Chew, K.H.; Dai, C.Q.; Zhou, G. Optical spin-to-orbital angular momentum conversion in the near field of a highly nonparaxial optical field with hybrid states of polarization. Phys. Rev. A 2017, 96, 053862. [Google Scholar] [CrossRef]
- Hu, K.; Chen, Z.; Pu, J. Tight focusing properties of hybridly polarized vector beams. J. Opt. Soc. Am. A 2012, 29, 1099–1104. [Google Scholar] [CrossRef]
- Huang, S.Y.; Zhang, G.L.; Wang, Q.; Wang, M.; Tu, C.; Li, Y.; Wang, H.T. Spin-to-orbital angular momentum conversion via light intensity gradient. Optica 2021, 8, 1231–1236. [Google Scholar] [CrossRef]
- Yu, P.; Liu, Y.; Wang, Z.; Li, Y.; Gong, L. Interplay between spin and orbital angular momenta in tightly focused higher-order Poincaré sphere beams. Ann. Phys. 2020, 532, 2000110. [Google Scholar] [CrossRef]
- Bliokh, K.Y.; Ostrovskaya, E.A.; Alonso, M.A.; Rodríguez-Herrera, O.G.; Lara, D.; Dainty, C. Spin-to-orbital angular momentum conversion in focusing, scattering, and imaging systems. Opt. Express 2011, 19, 26132–26149. [Google Scholar] [CrossRef]
- Fadeyeva, T.A.; Rubass, A.F.; Volyar, A.V. Transverse shift of a high-order paraxial vortex-beam induced by a homogeneous anisotropic medium. Phys. Rev. A 2009, 79, 053815. [Google Scholar] [CrossRef]
- Aiello, A.; Banzer, P.; Neugebauer, M.; Leuchs, G. From transverse angular momentum to photonic wheels. Nature Photon. 2015, 9, 789–795. [Google Scholar] [CrossRef]
- Bauer, T.; Banzer, P.; Karimi, E.; Orlov, S.; Rubano, A.; Marrucci, L.; Leuchs, G. Observation of optical polarization Möbius strips. Science 2015, 347, 964–966. [Google Scholar] [CrossRef] [PubMed]
- Berry, M.V. Index formulae for singular lines of polarization. J. Opt. A Pure Appl. Opt. 2004, 6, 675. [Google Scholar] [CrossRef]
- Goorden, S.A.; Bertolotti, J.; Mosk, A.P. Superpixel-based spatial amplitude and phase modulation using a digital micromirror device. Opt. Express 2014, 22, 17999–18009. [Google Scholar] [CrossRef]
- Mendoza-Yero, O.; Mínguez-Vega, G.; Lancis, J. Encoding complex fields by using a phase-only optical element. Opt. Lett. 2014, 39, 1740–1743. [Google Scholar] [CrossRef]
- Ran, W.; Ren, Z.; Wang, P.; Yan, Y.; Zhao, K.; Li, L.; Li, Z.; Wang, L.; Yang, J.; Wei, Z.; et al. Integrated polarization-sensitive amplification system for digital information transmission. Nat. Commun. 2021, 12, 6476. [Google Scholar] [CrossRef]
- Ding, S.; Li, R.; Luo, Y.; Dang, A. Polarization coherent optical communications with adaptive polarization control over atmospheric turbulence. J. Opt. Soc. Am. A 2018, 35, 1204–1211. [Google Scholar] [CrossRef]
- Zhang, J.; Li, Z.; Dang, A. Performance of wireless optical communication systems under polarization effects over atmospheric turbulence. Opt. Commun. 2018, 416, 207–213. [Google Scholar] [CrossRef]
- Yang, Y.; Ren, Y.; Chen, M.; Arita, Y.; Rosales-Guzmán, C. Optical trapping with structured light: A review. Adv. Photon. 2021, 3, 034001. [Google Scholar] [CrossRef]
- Chen, Z.; Segev, M. Highlighting photonics: Looking into the next decade. eLight 2021, 1, 2. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kotlyar, V.V.; Kovalev, A.A. Spin Hall Effect of Two-Index Paraxial Vector Propagation-Invariant Beams. Photonics 2023, 10, 1288. https://doi.org/10.3390/photonics10111288
Kotlyar VV, Kovalev AA. Spin Hall Effect of Two-Index Paraxial Vector Propagation-Invariant Beams. Photonics. 2023; 10(11):1288. https://doi.org/10.3390/photonics10111288
Chicago/Turabian StyleKotlyar, Victor V., and Alexey A. Kovalev. 2023. "Spin Hall Effect of Two-Index Paraxial Vector Propagation-Invariant Beams" Photonics 10, no. 11: 1288. https://doi.org/10.3390/photonics10111288
APA StyleKotlyar, V. V., & Kovalev, A. A. (2023). Spin Hall Effect of Two-Index Paraxial Vector Propagation-Invariant Beams. Photonics, 10(11), 1288. https://doi.org/10.3390/photonics10111288




