Two-Photon Laser Excitation of Rb Rydberg Atoms in the Magneto-Optical Trap and Vapor Cell
Abstract
:1. Introduction
2. Theory of Two-Photon Rydberg Excitation
3. Experimental Results and Their Comparison with Theory
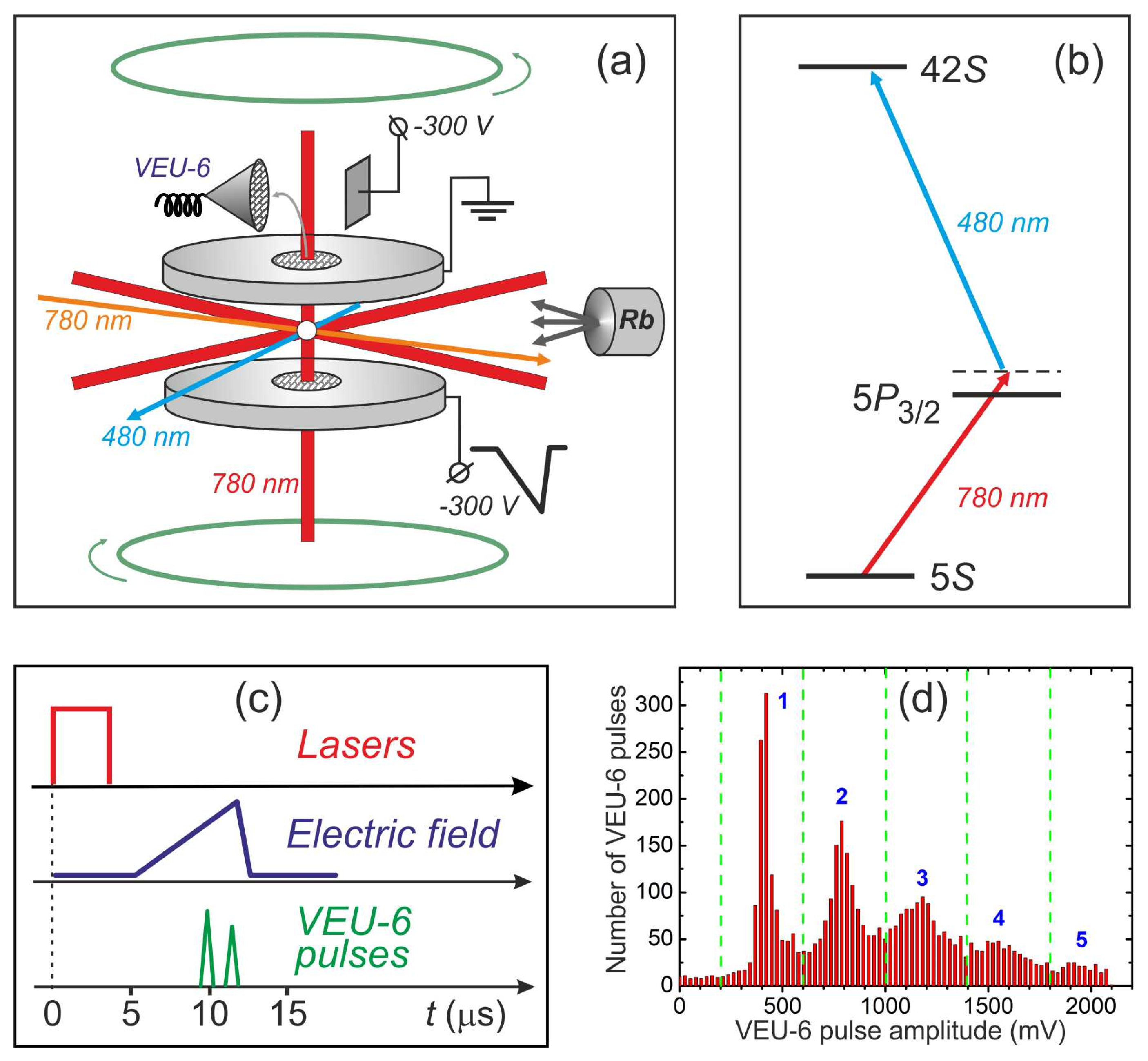
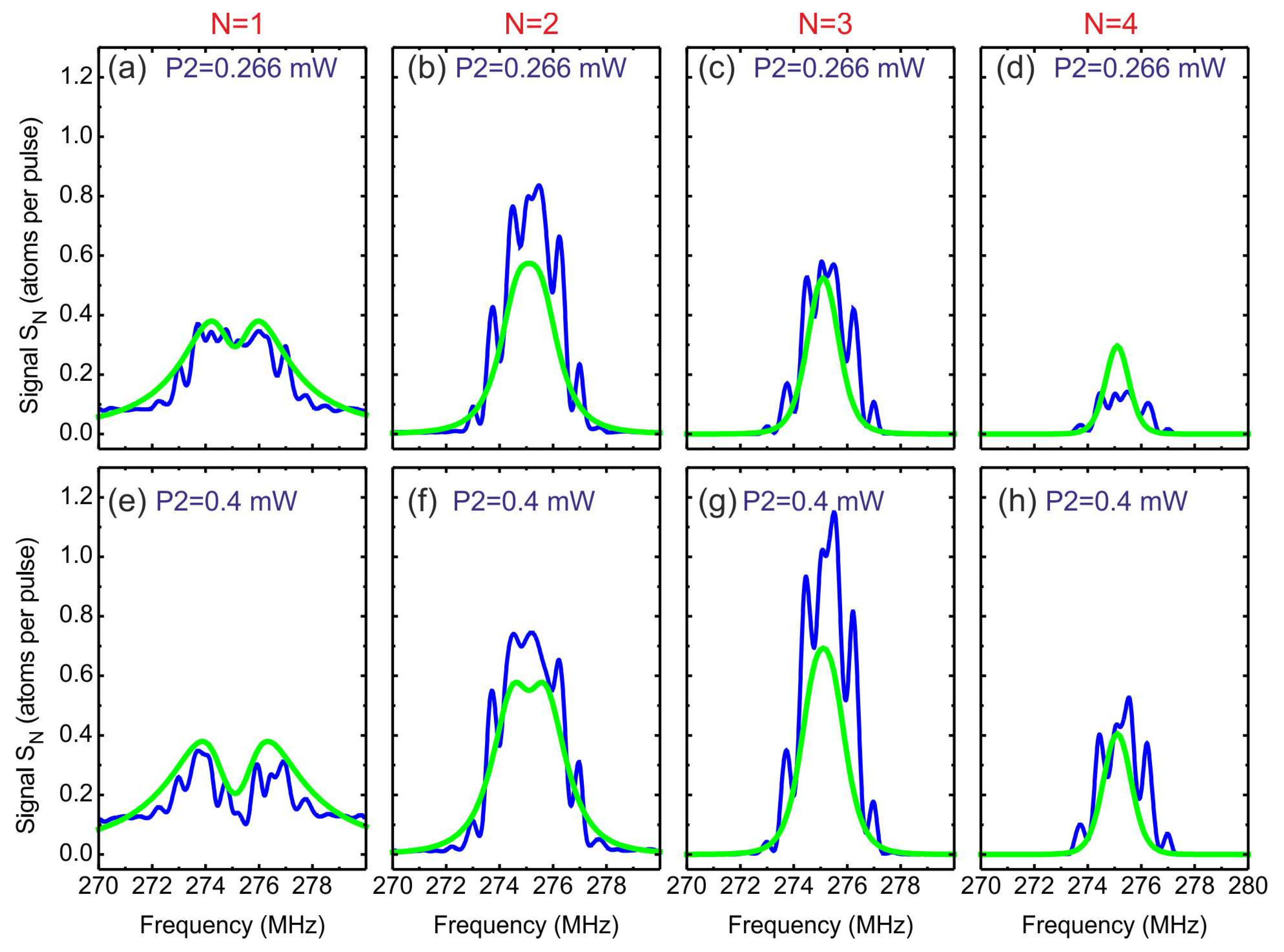
3.1. Experiment with Cold Rb Atoms in a Magneto-Optical Trap
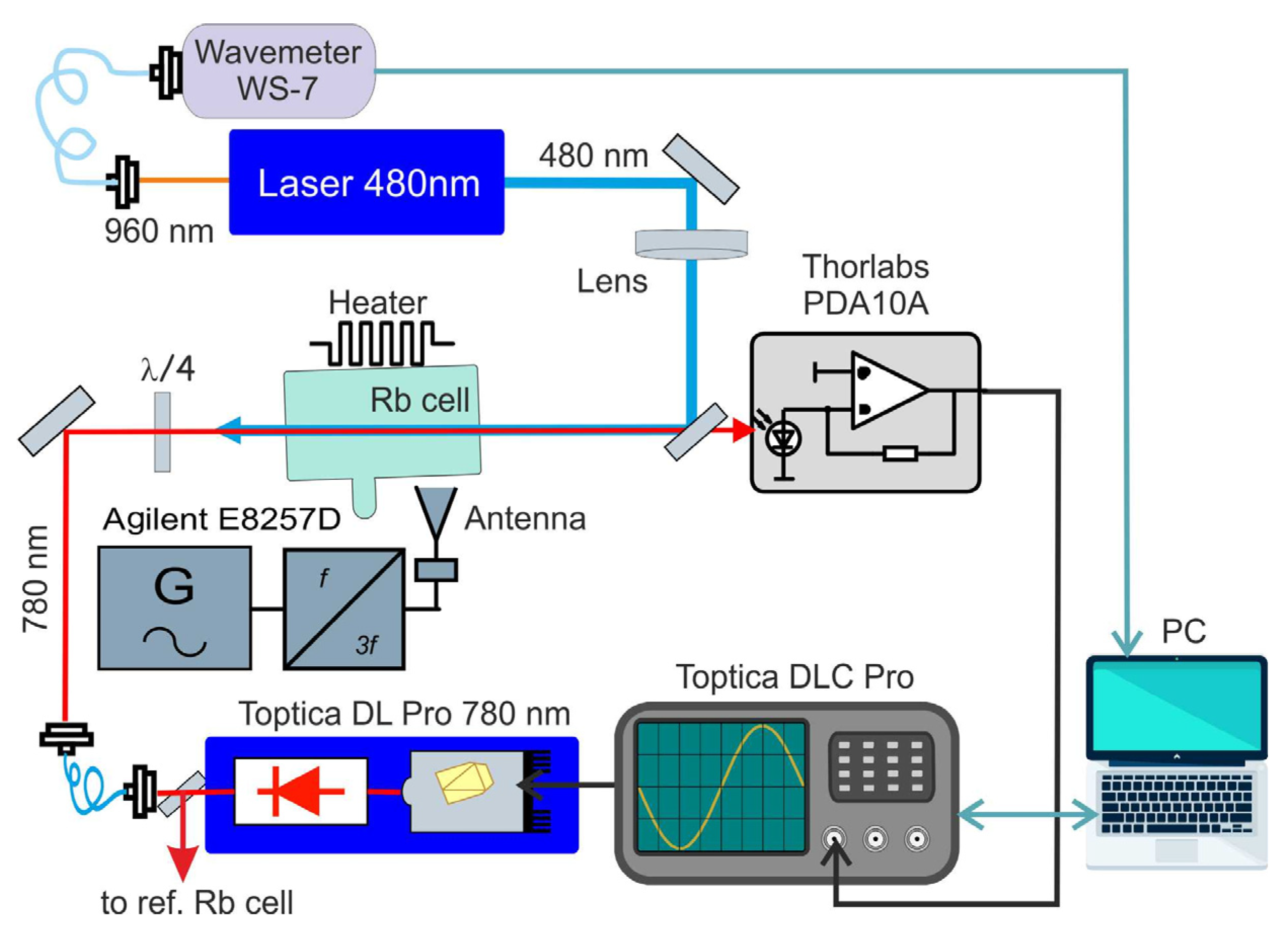
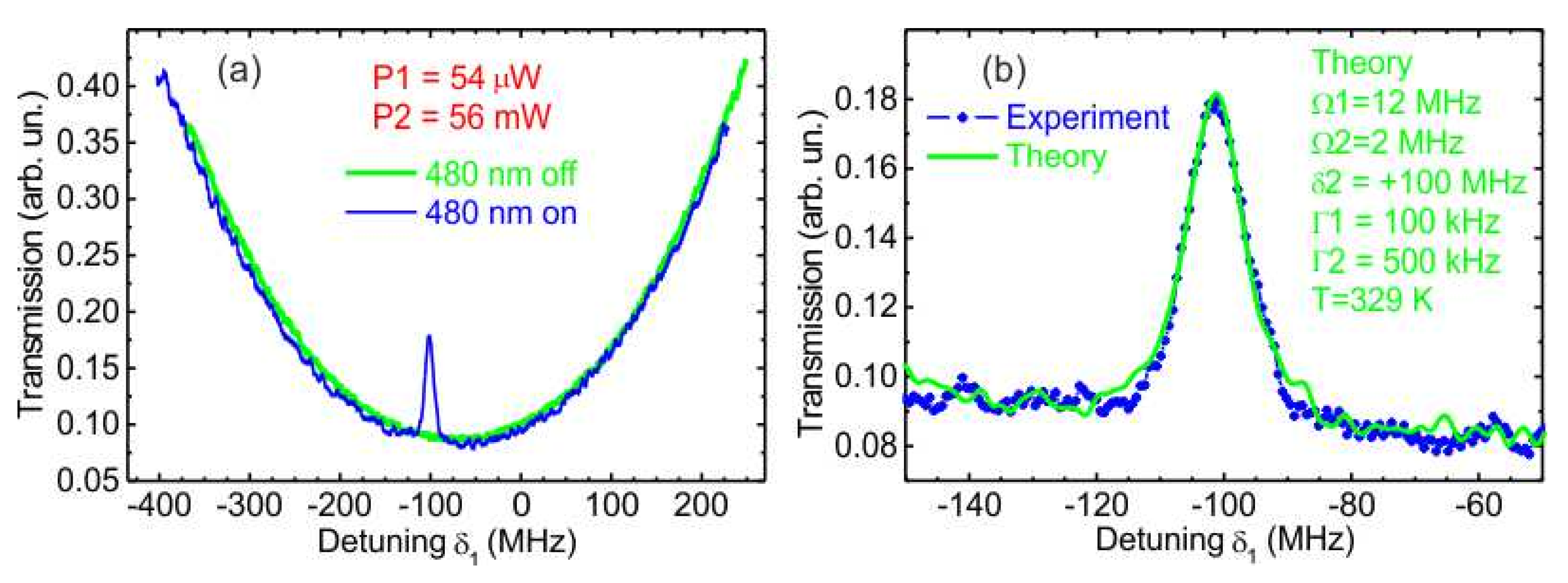
3.2. Experiment with Hot Rb Atoms in a Vapor Cell
4. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Gallagher, T.F. Rydberg Atoms; Cambridge University Press: Cambridge, UK, 1994. [Google Scholar]
- Lukin, M.D.; Fleischhauer, M.; Cote, R.; Duan, L.M.; Jaksch, D.; Cirac, J.I.; Zoller, P. Dipole Blockade and Quantum Information Processing in Mesoscopic Atomic Ensembles. Phys. Rev. Lett. 2001, 87, 037901. [Google Scholar] [CrossRef] [PubMed]
- Saffman, M.; Walker, T.G.; Mølmer, K. Quantum information with Rydberg atoms. Rev. Mod. Phys. 2010, 82, 2313–2363. [Google Scholar] [CrossRef]
- Ryabtsev, I.I.; Beterov, I.I.; Tretyakov, D.B.; Entin, V.M.; Yakshina, E.A. Spectroscopy of cold rubidium Rydberg atoms for applications in quantum information. Phys. Usp. 2016, 59, 196–208. [Google Scholar] [CrossRef]
- Saffman, M. Quantum computing with atomic qubits and Rydberg interactions: Progress and challenges. J. Phys. B 2016, 49, 202001. [Google Scholar] [CrossRef]
- Henriet, L.; Beguin, L.; Signoles, A.; Lahaye, T.; Browaeys, A.; Reymond, G.-O.; Jurczak, C. Quantum computing with neutral atoms. Quantum 2020, 4, 327. [Google Scholar] [CrossRef]
- Holloway, C.L.; Gordon, J.A.; Schwarzkopf, A.; Anderson, D.A.; Miller, S.A.; Thaicharoen, N.; Raithel, G. Sub-wavelength imaging and field mapping via electromagnetically induced transparency and Autler-Townes splitting in Rydberg atoms. Appl. Phys. Lett. 2014, 104, 244102. [Google Scholar] [CrossRef]
- Fan, H.Q.; Kumar, S.; Daschner, R.; Kübler, H.; Shaffer, J.P. Subwavelength microwave electric-field imaging using Rydberg atoms inside atomic vapor cells. Opt. Lett. 2014, 39, 3030–3033. [Google Scholar] [CrossRef]
- Thoumany, P.; Hänsch, T.; Stania, G.; Urbonas, L.; Becker, T. Optical spectroscopy of rubidium Rydberg atoms with a 297 nm frequency-doubled dye laser. Opt. Lett. 2009, 34, 1621–1623. [Google Scholar] [CrossRef]
- Cubel, T.; Teo, B.K.; Malinovsky, V.S.; Guest, J.R.; Reinhard, A.; Knuffman, B.; Berman, P.R.; Raithel, G. Coherent population transfer of ground-state atoms into Rydberg states. Phys. Rev. A 2005, 72, 023405. [Google Scholar] [CrossRef]
- Reetz-Lamour, M.; Deiglmayr, J.; Amthor, T.; Weidemüller, M. Rabi oscillations between ground and Rydberg states and van der Waals blockade in a mesoscopic frozen Rydberg gas. New J. Phys. 2008, 10, 045026. [Google Scholar] [CrossRef]
- Johnson, T.A.; Urban, E.; Henage, T.; Isenhower, L.; Yavuz, D.D.; Walker, T.G.; Saffman, M. Rabi Oscillations between Ground and Rydberg States with Dipole-Dipole Atomic Interactions. Phys. Rev. Lett. 2008, 100, 113003. [Google Scholar] [CrossRef] [PubMed]
- Viteau, M.; Radogostowicz, J.; Chotia, A.; Bason, M.G.; Malossi, N.; Fuso, F.; Ciampini, D.; Morsch, O.; Ryabtsev, I.I.; Arimondo, E. Ion detection in the photoionization of a Rb Bose-Einstein condensate. J. Phys. B 2010, 43, 155301. [Google Scholar] [CrossRef]
- Nipper, J.; Balewski, J.B.; Krupp, A.T.; Butscher, B.; Löw, R.; Pfau, T. Highly Resolved Measurements of Stark-Tuned Förster Resonances between Rydberg Atoms. Phys. Rev. Lett. 2012, 108, 113001. [Google Scholar] [CrossRef] [PubMed]
- de Léséleuc, S.; Barredo, D.; Lienhard, V.; Browaeys, A.; Lahaye, T. Analysis of imperfections in the coherent optical excitation of single atoms to Rydberg states. Phys. Rev. A 2018, 97, 053803. [Google Scholar] [CrossRef]
- Levine, H.; Keesling, A.; Semeghini, G.; Omran, A.; Wang, T.T.; Ebadi, S.; Bernien, H.; Greiner, M.; Vuletić, V.; Pichler, H.; et al. Parallel Implementation of High-Fidelity Multiqubit Gates with Neutral Atoms. Phys. Rev. Lett. 2019, 123, 170503. [Google Scholar] [CrossRef] [PubMed]
- Entin, V.M.; Yakshina, E.A.; Tretyakov, D.B.; Beterov, I.I.; Ryabtsev, I.I. Spectroscopy of the three-photon laser excitation of cold Rubidium Rydberg atoms in a magneto-optical trap. J. Exp. Theor. Phys. 2013, 116, 721–731. [Google Scholar] [CrossRef]
- Yakshina, E.A.; Tretyakov, D.B.; Entin, V.M.; Beterov, I.I.; Ryabtsev, I.I. Three-photon laser excitation of mesoscopic ensembles of cold rubidium Rydberg atoms. Quantum Electron. 2018, 48, 886–893. [Google Scholar] [CrossRef]
- Yakshina, E.A.; Tretyakov, D.B.; Entin, V.M.; Beterov, I.I.; Ryabtsev, I.I. Observation of the Dipole Blockade Effect in Detecting Rydberg Atoms by the Selective Field Ionization Method. J. Exp. Theor. Phys. 2020, 130, 170–182. [Google Scholar] [CrossRef]
- Beterov, I.I.; Yakshina, E.A.; Tretyakov, D.B.; Alyanova, N.V.; Skvortsova, D.A.; Suliman, G.; Zagirov, T.R.; Entin, V.M.; Ryabtsev, I.I. Three-photon laser excitation of single Rydberg rubidium atoms in an optical dipole trap. J. Exp. Theor. Phys. 2023, 137, 246–253. [Google Scholar] [CrossRef]
- Ryabtsev, I.I.; Beterov, I.I.; Tretyakov, D.B.; Entin, V.M.; Yakshina, E.A. Doppler- and recoil-free laser excitation of Rydberg states via three-photon transitions. Phys. Rev. A 2011, 84, 053409. [Google Scholar] [CrossRef]
- Bohaichuk, S.M.; Ripka, F.; Venu, V.; Christaller, F.; Liu, C.; Schmidt, M.; Kübler, H.; Shaffer, J.P. A Three-Photon Rydberg Atom-Based Radio Frequency Sensing Scheme with Narrow Linewidth. arXiv 2023, arXiv:2304.07409. [Google Scholar] [CrossRef]
- Jiao, Y.-C.; Han, X.-X.; Yang, Z.-W.; Zhao, J.-M.; Jia, S.-T. Electromagnetically Induced Transparency in a Cold Gas with Strong Atomic Interactions. Chin. Phys. Lett. 2016, 33, 123201. [Google Scholar] [CrossRef]
- Sedlacek, J.A.; Schwettmann, A.; Kübler, H.; Löw, R.; Pfau, T.; Shaffer, J.P. Microwave electrometry with Rydberg atoms in a vapour cell using bright atomic resonances. Nat. Phys. 2012, 8, 819–824. [Google Scholar] [CrossRef]
- Sautenkov, V.A.; Saakyan, S.A.; Bobrov, A.A.; Vilshanskaya, E.V.; Zelener, B.B.; Zelener, B.V. Differential two-photon spectroscopy for nondestructive temperature measurements of cold light atoms in a magneto-optical trap. J. Opt. Soc. Am. B 2018, 35, 1546–1551. [Google Scholar] [CrossRef]
- Beterov, I.I.; Ryabtsev, I.I.; Tretyakov, D.B.; Entin, V.M. Quasiclassical calculations of blackbody-radiation-induced depopulation rates and effective lifetimes of Rydberg nS, nP, and nD alkali-metal atoms with n ≤ 80. Phys. Rev. A 2009, 79, 052504. [Google Scholar] [CrossRef]
- Mohapatra, A.K.; Jackson, T.R.; Adams, C.S. Coherent optical detection of highly excited Rydberg states using electromagnetically induced transparency. Phys. Rev. Lett. 2007, 98, 113003. [Google Scholar] [CrossRef] [PubMed]
- Stelmashenko, E.F.; Klezovich, O.A.; Baryshev, V.N.; Tishchenko, V.A.; Blinov, I.Y.; Palchikov, V.G.; Ovsyannikov, V.D. Measuring the Electric Field Strength of Microwave Radiation at the Frequency of the Radiation Transition Between Rydberg States of Atoms 85Rb. Opt. Spectrosc. 2020, 128, 1067–1073. [Google Scholar] [CrossRef]
- Agarwal, G.S. Exact Solution for the Influence of Laser Temporal Fluctuations on Resonance Fluorescence. Phys. Rev. Lett. 1976, 37, 1383–1386. [Google Scholar] [CrossRef]
- Ryabtsev, I.I.; Tretyakov, D.B.; Beterov, I.I.; Entin, V.M. Effect of finite detection efficiency on the observation of the dipole-dipole interaction of a few Rydberg atoms. Phys. Rev. A 2007, 76, 012722. [Google Scholar] [CrossRef]
- Tretyakov, D.B.; Beterov, I.I.; Entin, V.M.; Ryabtsev, I.I.; Chapovsky, P.L. Investigation of cold rubidium Rydberg atoms in a magneto-optical trap. J. Exp. Theor. Phys. 2009, 108, 374–383. [Google Scholar] [CrossRef]
- Fancher, C.T.; Scherer, D.R.; St. John, M.C.; Schmittberger Marlow, B.L. Rydberg Atom Electric Field Sensors for Communications and Sensing. IEEE Trans. Quantum Eng. 2021, 2, 3501313. [Google Scholar] [CrossRef]
- Ovsiannikov, V.D.; Glukhov, I.L.; Nekipelov, E.A. Rates of blackbody radiation-induced transitions from Rydberg states of alkali atoms. J. Phys. B 2011, 44, 195010. [Google Scholar] [CrossRef]
- Holloway, C.L.; Simons, M.T.; Kautz, M.D.; Haddab, A.H.; Gordon, J.A.; Crowley, T.P. A quantum-based power standard: Using Rydberg atoms for a SI-traceable radio-frequency power measurement technique in rectangular waveguides. Appl. Phys. Lett. 2018, 113, 094101. [Google Scholar] [CrossRef]
- Anderson, D.A.; Sapiro, R.E.; Raithel, G. A self-calibrating SI-traceable broadband Rydberg atom-based radio-frequency electric field probe and measurement instrument. IEEE Trans. Antennas Propag. 2021, 69, 5931–5941. [Google Scholar] [CrossRef]
- Gerasimov, L.V.; Yusupov, R.R.; Moiseevsky, A.D.; Vybornyi, I.; Tikhonov, K.S.; Kulik, S.P.; Straupe, S.S.; Sukenik, C.I.; Kupriyanov, D.V. Coupled dynamics of spin qubits in optical dipole microtraps: Application to the error analysis of a Rydberg-blockade gate. Phys. Rev. A 2022, 106, 042410. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tretyakov, D.B.; Entin, V.M.; Beterov, I.I.; Yakshina, E.A.; Pechersky, Y.Y.; Gol’dort, V.G.; Ryabtsev, I.I. Two-Photon Laser Excitation of Rb Rydberg Atoms in the Magneto-Optical Trap and Vapor Cell. Photonics 2023, 10, 1201. https://doi.org/10.3390/photonics10111201
Tretyakov DB, Entin VM, Beterov II, Yakshina EA, Pechersky YY, Gol’dort VG, Ryabtsev II. Two-Photon Laser Excitation of Rb Rydberg Atoms in the Magneto-Optical Trap and Vapor Cell. Photonics. 2023; 10(11):1201. https://doi.org/10.3390/photonics10111201
Chicago/Turabian StyleTretyakov, Denis B., Vasily M. Entin, Ilya I. Beterov, Elena A. Yakshina, Yury Ya. Pechersky, Veniamin G. Gol’dort, and Igor I. Ryabtsev. 2023. "Two-Photon Laser Excitation of Rb Rydberg Atoms in the Magneto-Optical Trap and Vapor Cell" Photonics 10, no. 11: 1201. https://doi.org/10.3390/photonics10111201
APA StyleTretyakov, D. B., Entin, V. M., Beterov, I. I., Yakshina, E. A., Pechersky, Y. Y., Gol’dort, V. G., & Ryabtsev, I. I. (2023). Two-Photon Laser Excitation of Rb Rydberg Atoms in the Magneto-Optical Trap and Vapor Cell. Photonics, 10(11), 1201. https://doi.org/10.3390/photonics10111201




