Illustrations of Bessel Beams in s-Polarization, p-Polarization, Transverse Polarization, and Longitudinal Polarization
Abstract
:1. Introduction
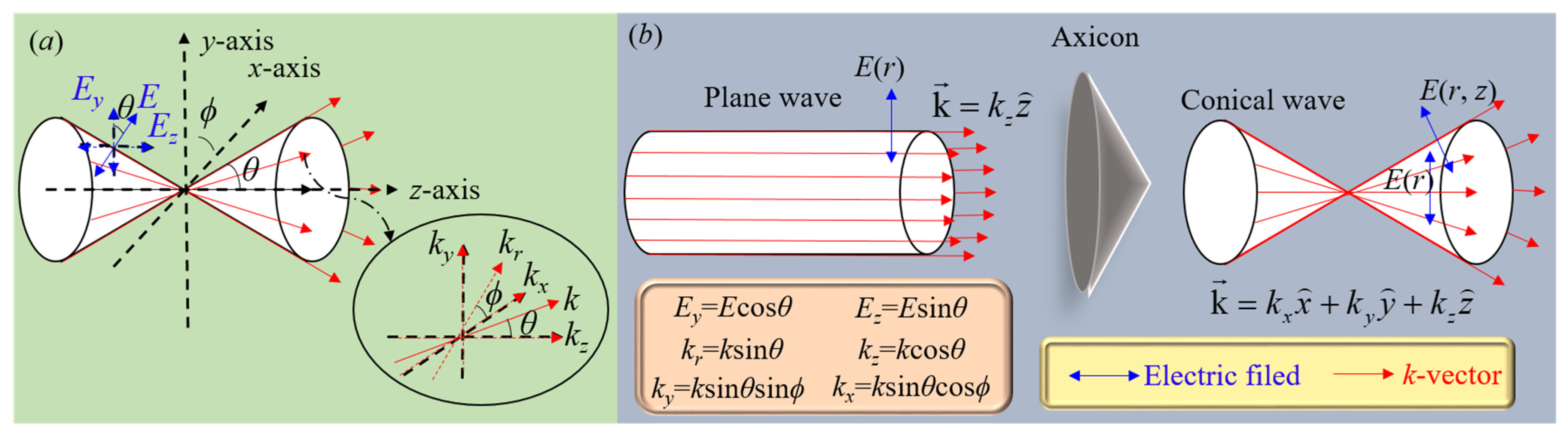
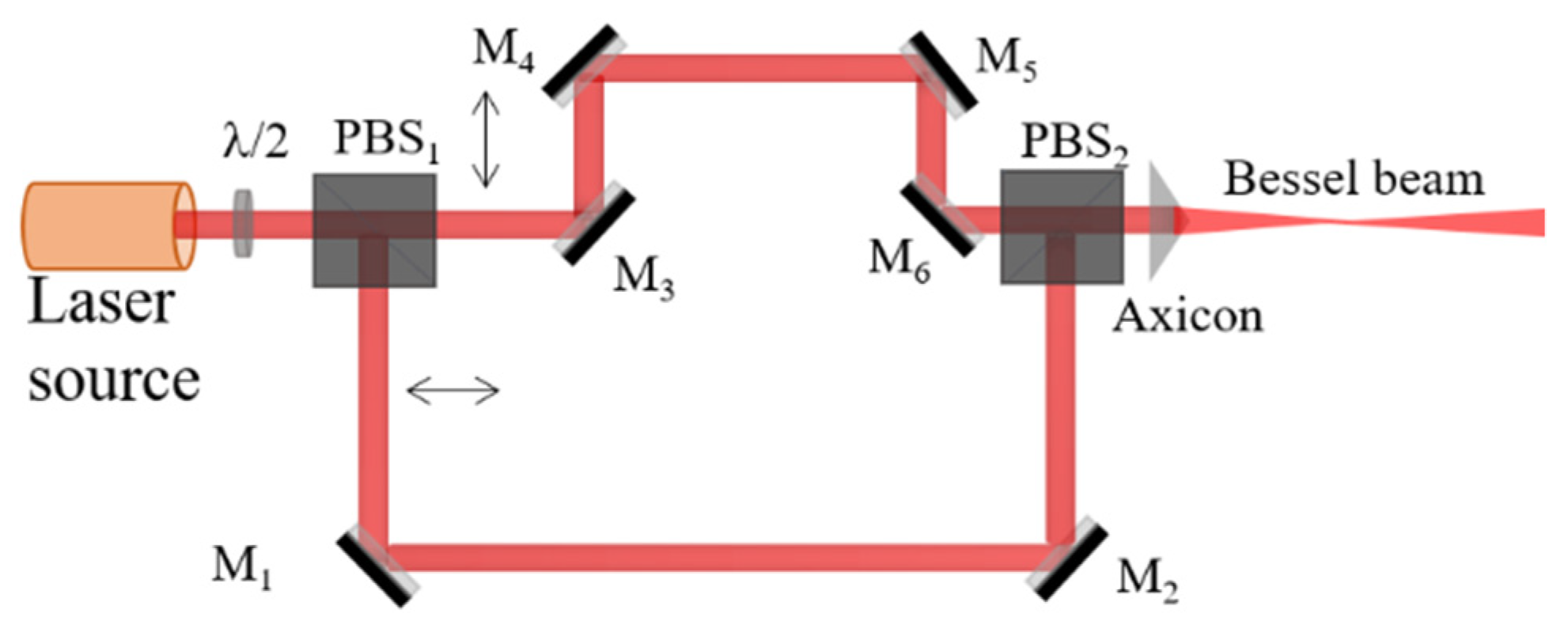
2. Theory
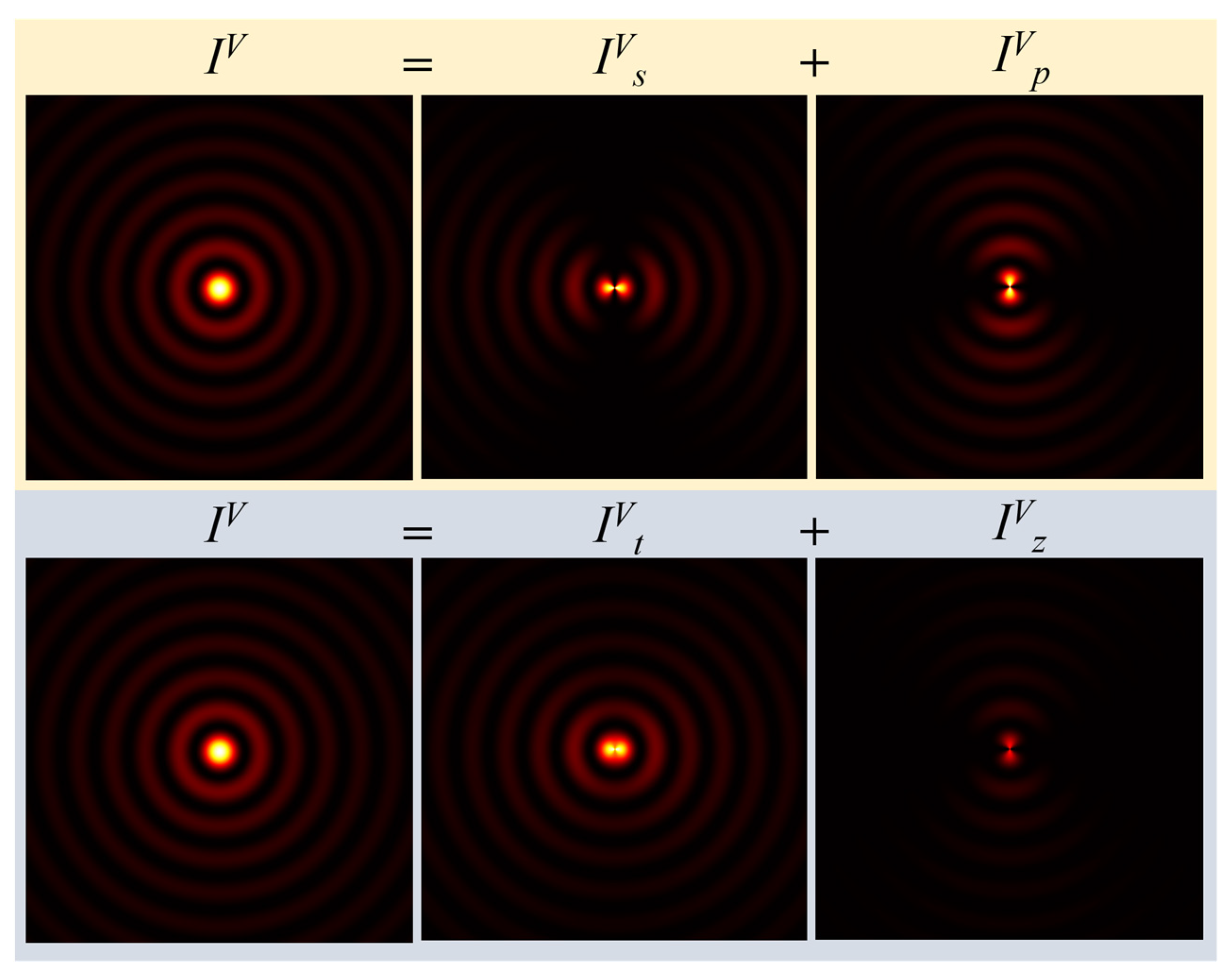
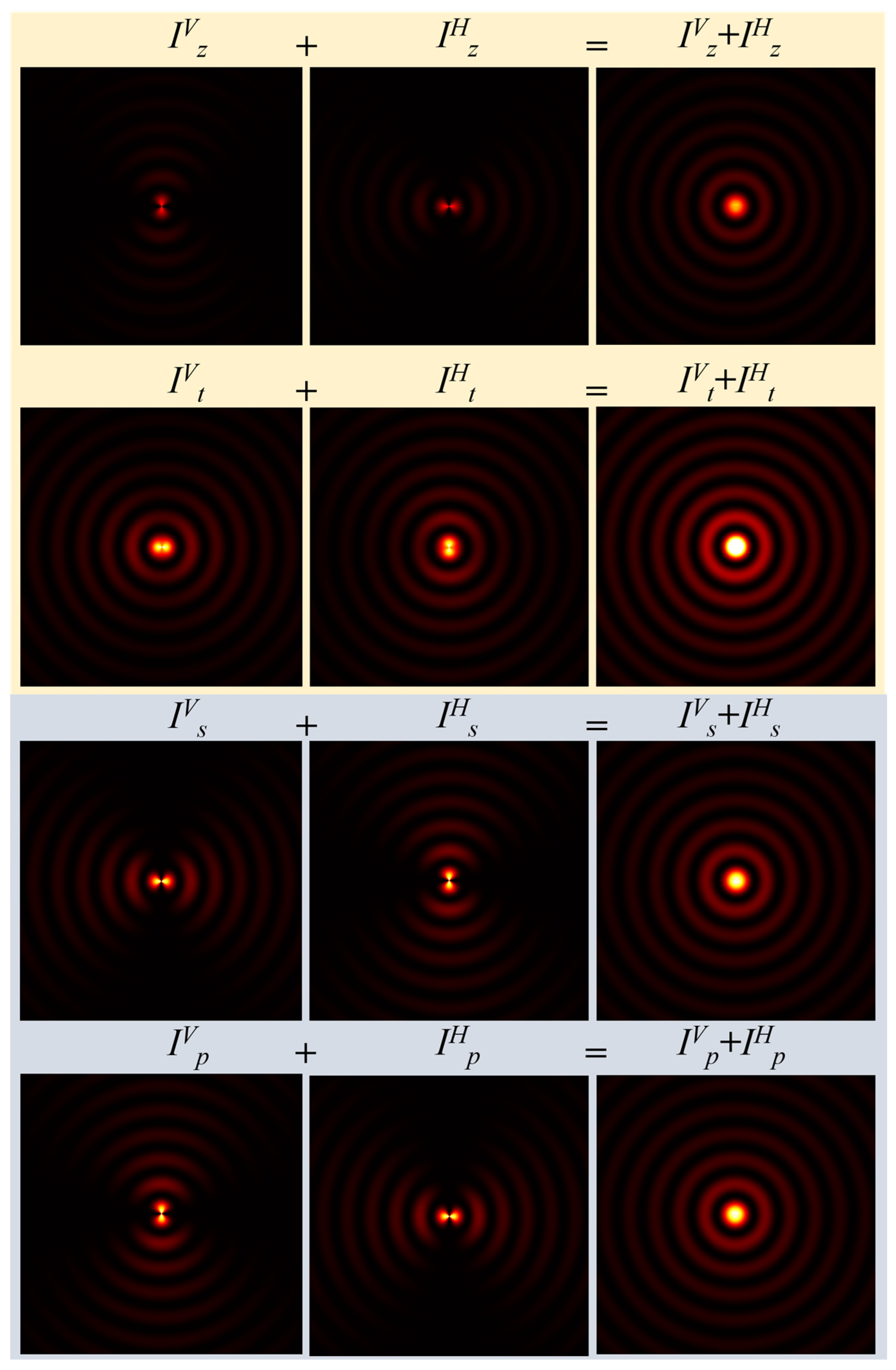
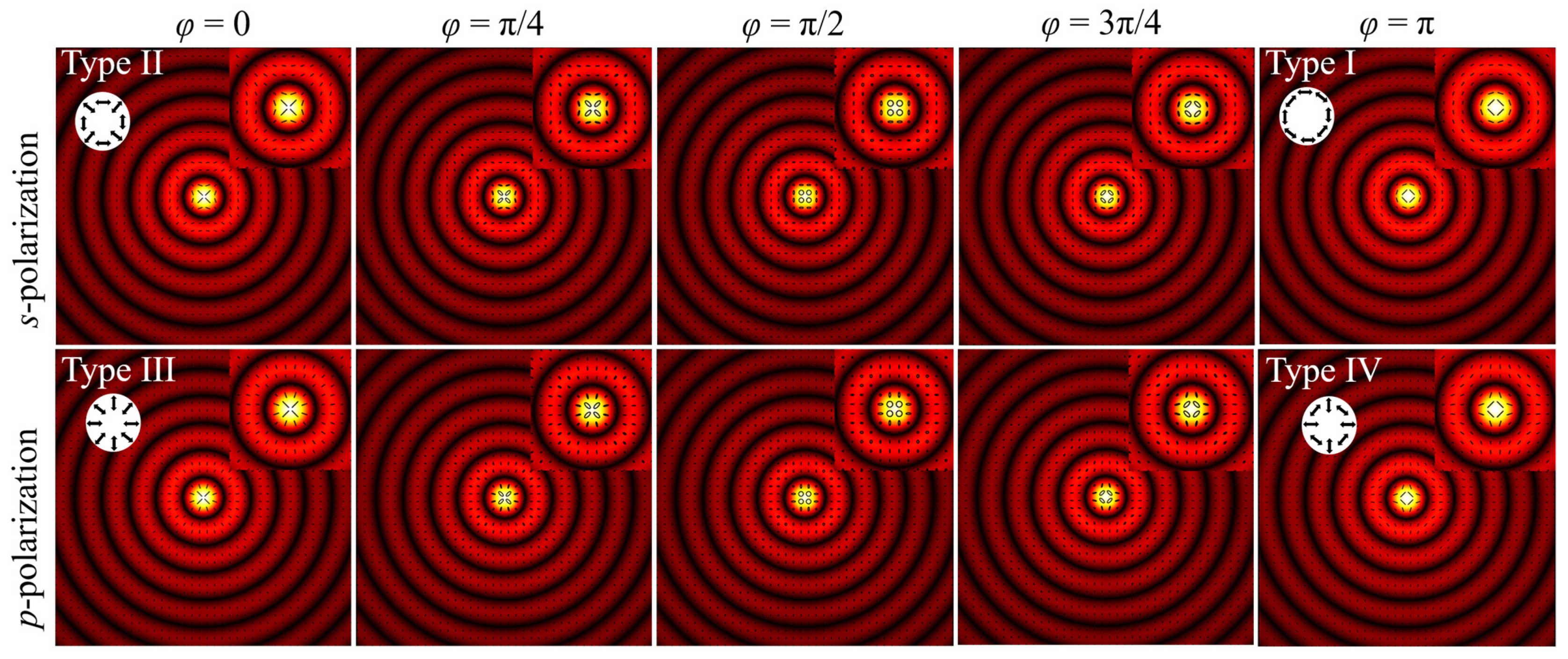
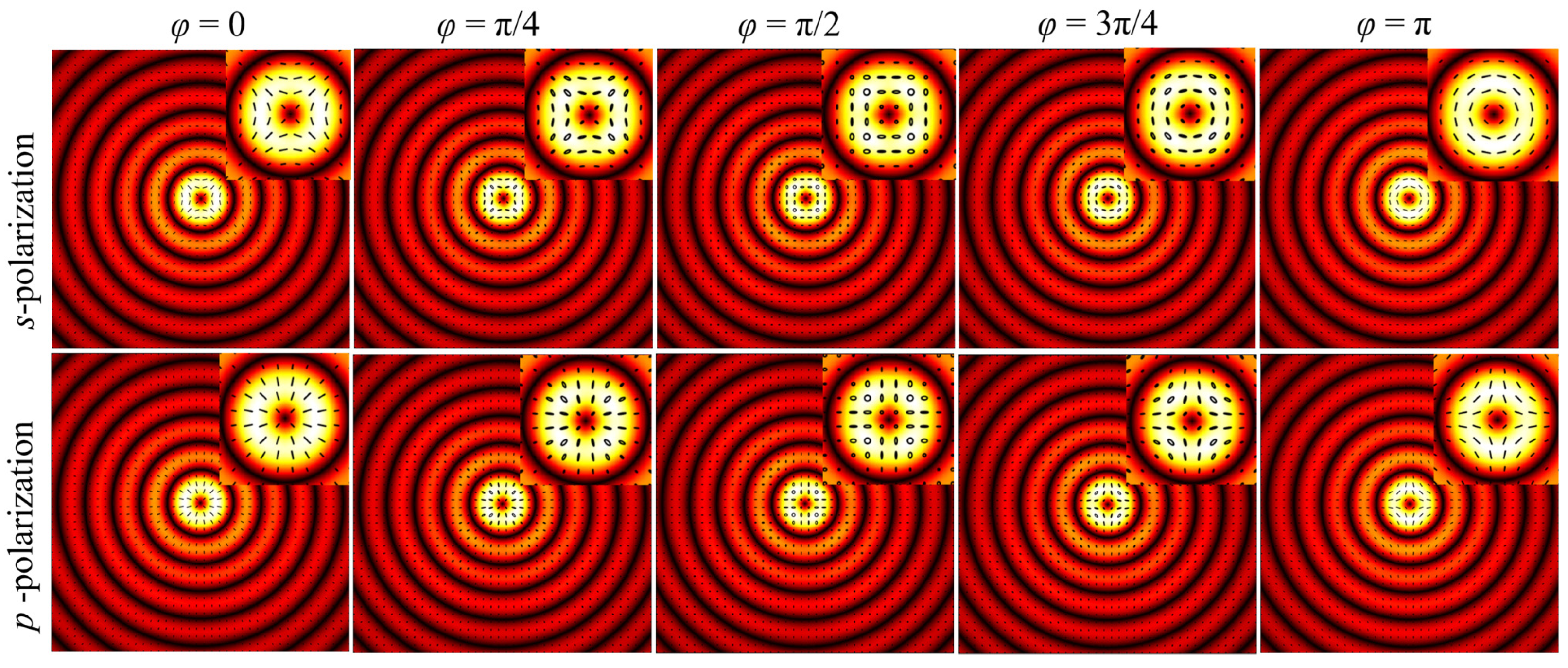
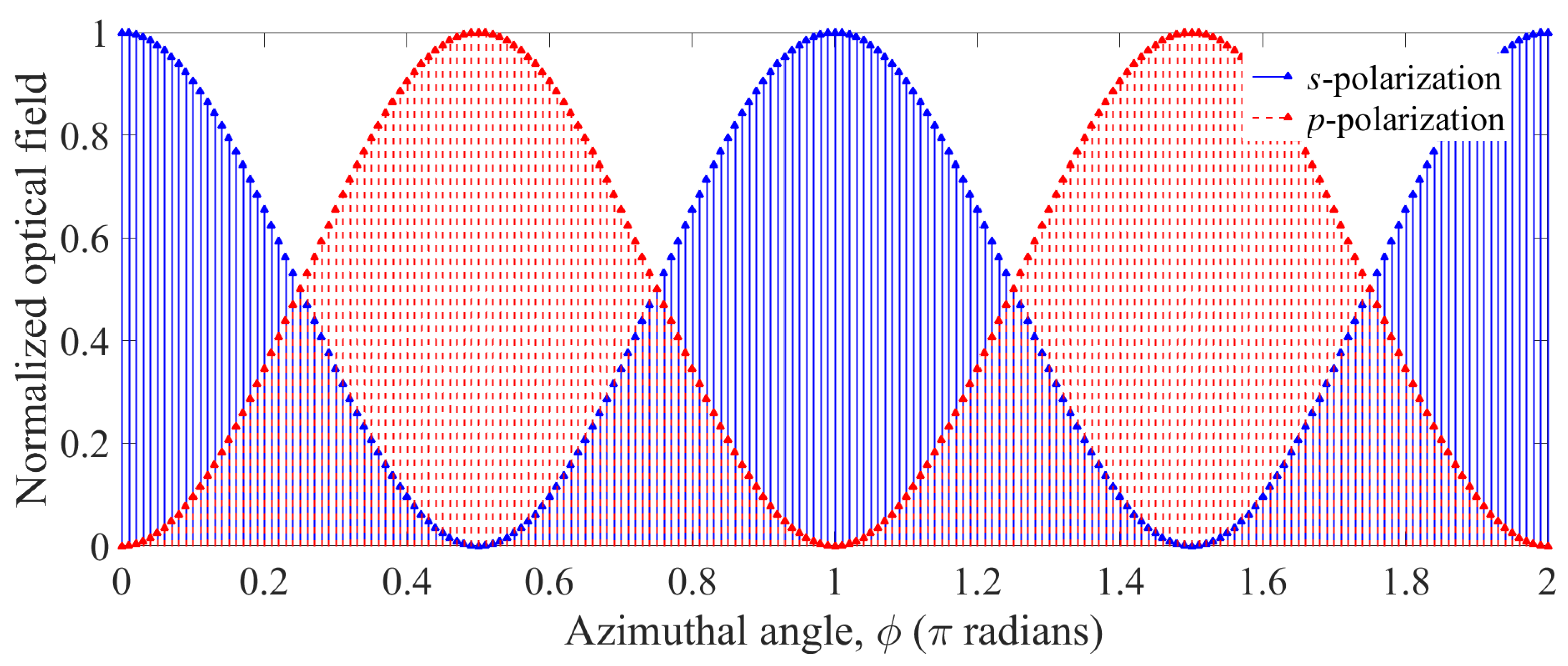
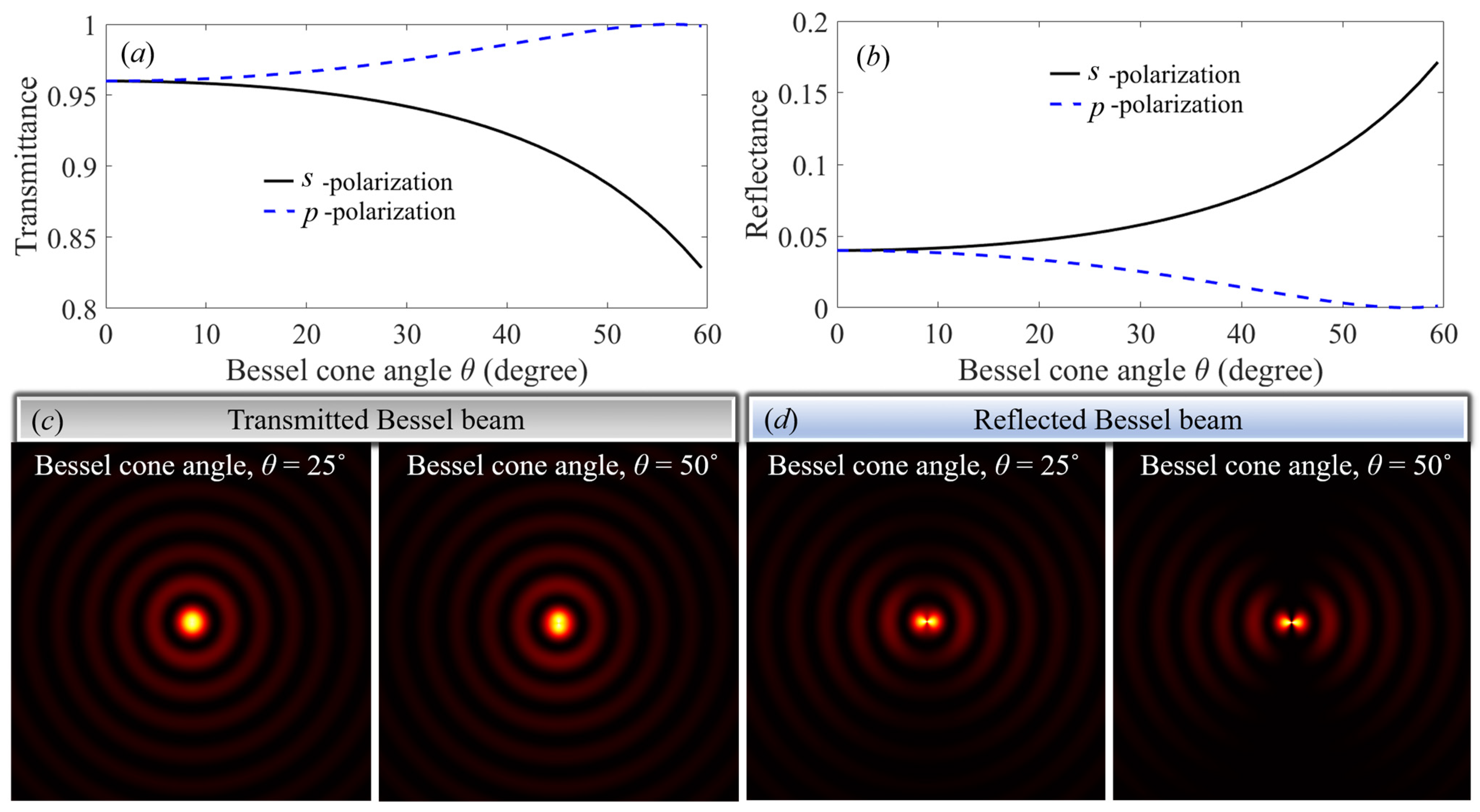
3. Results and Discussion
4. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Durnin, J. Exact solutions for nondiffracting beams. I. The scalar theory. JOSA A 1987, 4, 651–654. [Google Scholar] [CrossRef]
- Durnin, J.; Miceli, J.J., Jr.; Eberly, J.H. Diffraction-free beams. Phys. Rev. Lett. 1987, 58, 15. [Google Scholar] [CrossRef] [PubMed]
- Gori, F.; Guattari, G.; Padovani, C. Bessel-gauss beams. Opt. Commun. 1987, 64, 491–495. [Google Scholar] [CrossRef]
- Li, Y.; Lee, H.; Wolf, E. New generalized Bessel–Gaussian beams. JOSA A 2004, 21, 640–646. [Google Scholar] [CrossRef]
- Khan, S.A. Cross polarization in Gaussian and Bessel light beams. Opt. Commun. 2023, 545, 129728. [Google Scholar] [CrossRef]
- Mishra, S.R. A vector wave analysis of a Bessel beam. Opt. Commun. 1991, 85, 159–161. [Google Scholar] [CrossRef]
- McGloin, D.; Dholakia, K. Bessel beams: Diffraction in a new light. Contemp. Phys. 2005, 46, 15–28. [Google Scholar] [CrossRef]
- Rao, A.S.; Samanta, G.K. On-axis intensity modulation-free, segmented, zero-order Bessel beams with tunable ranges. Opt. Lett. 2018, 43, 3029–3032. [Google Scholar] [CrossRef]
- Chattrapiban, N.; Rogers, E.A.; Cofield, D.; Hill, W.T., III; Roy, R. Generation of nondiffracting Bessel beams by use of a spatial light modulator. Opt. Lett. 2003, 28, 2183–2185. [Google Scholar] [CrossRef]
- Chen, B.; Huang, X.; Gou, D.; Zeng, J.; Chen, G.; Pang, M.; Hu, Y.; Zhao, Z.; Zhang, Y.; Zhou, Z.; et al. Rapid volumetric imaging with Bessel-Beam three-photon microscopy. Biomed. Opt. Exp. 2018, 9, 1992–2000. [Google Scholar] [CrossRef]
- Garcés-Chávez, V.; McGloin, D.; Melville, H.; Sibbett, W.; Dholakia, K. Simultaneous micromanipulation in multiple planes using a self-reconstructing light beam. Nature 2002, 419, 145–147. [Google Scholar] [CrossRef] [PubMed]
- Bhuyan, M.K.; Somayaji, M.; Mermillod-Blondin, A.; Bourquard, F.; Colombier, J.P.; Stoian, R. Ultrafast laser nanostructuring in bulk silica, a “slow” microexplosion. Optica 2017, 4, 951–958. [Google Scholar] [CrossRef]
- Arita, Y.; Lee, J.; Kawaguchi, H.; Matsuo, R.; Miyamoto, K.; Dholakia, K.; Omatsu, T. Photopolymerization with high-order Bessel light beams. Opt. Lett. 2020, 45, 4080–4083. [Google Scholar] [CrossRef] [PubMed]
- Lu, Z.; Guo, Z.; Fan, M.; Guo, M.; Li, C.; Yao, Y.; Zhang, H.; Lin, W.; Liu, H.; Liu, B. Tunable Bessel Beam Shaping for Robust Atmospheric Optical Communication. J. Light. Technol. 2022, 40, 5097–5106. [Google Scholar] [CrossRef]
- Hecht, E. Optics, 4th ed.; Pearson Education: San Francisco, CA, USA, 2001; pp. 121–133. [Google Scholar]
- Li, H.; Honary, F.; Wang, J.; Liu, J.; Wu, Z.; Bai, L. Intensity, phase, and polarization of a vector Bessel vortex beam through multilayered isotropic media. Appl. Opt. 2018, 57, 1967–1976. [Google Scholar] [CrossRef]
- Mugnai, D. Propagation of Bessel beams from a dielectric to a conducting medium. Appl. Opt. 2011, 50, 2654–2658. [Google Scholar] [CrossRef]
- Mugnai, D. Bessel beam through a dielectric slab at oblique incidence: The case of total reflection. Opt. Commun. 2002, 207, 95–99. [Google Scholar] [CrossRef]
- Wang, J.J.; Wriedt, T.; Lock, J.A.; Jiao, Y.C. General description of transverse mode Bessel beams and construction of basis Bessel fields. J. Quant. Spect. Rad. Trans. 2017, 195, 8–17. [Google Scholar] [CrossRef]
- Novitsky, A.V.; Barkovsky, L.M. Vector Bessel beams in bianisotropic media. J. Opt. A Pure Appl. Opt. 2005, 7, 550. [Google Scholar] [CrossRef]
- Manela, O.; Segev, M.; Christodoulides, D.N. Nondiffracting beams in periodic media. Opt. Lett. 2005, 30, 2611–2613. [Google Scholar] [CrossRef]
- Novitsky, A.V.; Barkovsky, L.M. Total internal reflection of vector Bessel beams: Imbert–Fedorov shift and intensity transformation. J. Opt. A Pure Appl. Opt. 2008, 10, 075006. [Google Scholar] [CrossRef]
- Simon, R.; Sudarshan, E.C.G.; Mukunda, N. Cross polarization in laser beams. Appl. Opt. 1987, 26, 1589–1593. [Google Scholar] [CrossRef] [PubMed]
- Sheppard, C.J.; Rehman, S.; Balla., N.K.; Yew., E.Y.; Teng, T.W. Bessel beams: Effects of polarization. Opt. Commun. 2009, 282, 4647–4656. [Google Scholar] [CrossRef]
- Chen, W.; Zhan, Q. Realization of an evanescent Bessel beam via surface plasmon interference excited by a radially polarized beam. Opt. Lett. 2009, 34, 722–724. [Google Scholar] [CrossRef] [PubMed]
- Zhan, Q. Evanescent Bessel beam generation via surface plasmon resonance excitation by a radially polarized beam. Opt. Lett. 2006, 31, 1726–1728. [Google Scholar] [CrossRef] [PubMed]
- Rao, A.S.; Morohashi, T.; Kerridge-Johns, W.R.; Omatsu, T. Generation of higher-order Laguerre–Gaussian modes from a diode-pumped Pr 3+: LiYF 4 laser with an intra-cavity spherical aberration. JOSA B 2023, 40, 406–411. [Google Scholar] [CrossRef]
- Boley, C.D.; Rubenchik, A.M. Modeling of laser interactions with composite materials. Appl. Opt. 2013, 52, 3329–3337. [Google Scholar] [CrossRef]
- Kobayashi, M.; Minár, J.; Khan, W.; Borek, S.; Hai, P.N.; Harada, Y.; Schmitt, T.; Oshima, M.; Fujimori, A.; Tanaka, M.; et al. Minority-spin impurity band in n-type (In, Fe) As: A materials perspective for ferromagnetic semiconductors. Phys. Rev. B 2021, 103, 115111. [Google Scholar] [CrossRef]
- Lio, G.E.; Ferraro, A.; Kowerdziej, R.; Govorov, A.O.; Wang, Z.; Caputo, R. Engineering Fano-Resonant Hybrid Metastructures with Ultra-High Sensing Performances. Adv. Opt. Mat. 2023, 2023, 2203123. [Google Scholar] [CrossRef]
- Gong, Y.; Hu, M.; Harris, N.; Yang, Z.; Xie, T.; Teklu, A.; Kuthirummal, N.; Koenemann, J.; Xu, X.; Cheong, S.W.; et al. Strong laser polarization control of coherent phonon excitation in van der Waals material Fe3GeTe2. npj 2D Mat. Appl. 2022, 6, 9. [Google Scholar] [CrossRef]
- Mugnai, D.; Spalla, P. Electromagnetic propagation of Bessel-like localized waves in the presence of absorbing media. Opt. Commun. 2009, 282, 4668–4671. [Google Scholar] [CrossRef]
- Yang, B.; Ouyang, M.; Ren., H.; Wu, J.; Zhang., Y.; Fu, Y. January. Polarization-Dependent Absorption and Transmission Metasurfaces for Linearly and Circularly Polarized Light in Terahertz Band. Photonics 2023, 10, 100. [Google Scholar] [CrossRef]
- Garcia-Etxarri, A. Optical polarization mobius strips on all-dielectric optical scatterers. ACS Photonics 2017, 4, 1159–1164. [Google Scholar] [CrossRef]
- Matsuoka, Y.; Kizuka, Y.; Inoue, T. The characteristics of laser micro drilling using a Bessel beam. Appl. Phys. A 2006, 84, 423–430. [Google Scholar] [CrossRef]
- Belloni, V.V.; Froehly, L.; Billet, C.; Furfaro, L.; Courvoisier, F. Generation of extremely high-angle Bessel beams. Appl. Opt. 2023, 62, 1765–1768. [Google Scholar] [CrossRef]
- Milne, G.; Jeffries, G.; Chiu, D. Tunable generation of Bessel beams with a fluidic axicon. Appl. Phys. Lett. 2008, 92, 261101. [Google Scholar] [CrossRef]
- Boucher, P.; Del Hoyo, J.; Billet, C.; Pinel, O.; Labroille, G.; Courvoisier, F. Generation of high conical angle Bessel–Gauss beams with reflective axicons. Appl. Opt. 2018, 57, 6725–6728. [Google Scholar] [CrossRef]
- Bergner, K.; Müller, M.; Klas, R.; Limpert, J.; Nolte, S.; Tünnerman, A. Scaling ultrashort laser pulse induced glass modifications for cleaving applications. Appl. Opt. 2018, 57, 5941–5947. [Google Scholar] [CrossRef]
- Maucher, F.; Skupin, S.; Gardiner, S.A.; Hughes, I.G. Creating complex optical longitudinal polarization structures. Phys. Rev. Lett. 2018, 120, 163903. [Google Scholar] [CrossRef]
- Larocque, H.; Sugic, D.; Mortimer, D.; Taylor, A.J.; Fickler, R.; Boyd, R.W.; Dennis, M.R.; Karimi, E. Reconstructing the topology of optical polarization knots. Nat. Phys. 2018, 14, 1079–1082. [Google Scholar] [CrossRef]
- Bauer, T.; Banzer, P.; Karimi, E.; Orlov, S.; Rubano, A.; Marrucci, L.; Santamato, E.; Boyd, R.W.; Leuchs, G. Observation of optical polarization Möbius strips. Science 2015, 347, 964–966. [Google Scholar] [CrossRef]
- Junge, C.; O’shea, D.; Volz, J.; Rauschenbeutel, A. Strong coupling between single atoms and nontransversal photons. Phys. Rev. Lett. 2013, 110, 213604. [Google Scholar] [CrossRef]
- Li, X.; Lan, T.H.; Tien, C.H.; Gu, M. Three-dimensional orientation-unlimited polarization encryption by a single optically configured vectorial beam. Nat. Commun. 2012, 3, 998. [Google Scholar] [CrossRef]
- Masuda, K.; Nakano, S.; Barada, D.; Kumakura, M.; Miyamoto, K.; Omatsu, T. Azo-polymer film twisted to form a helical surface relief by illumination with a circularly polarized Gaussian beam. Opt. Express 2017, 25, 12499–12507. [Google Scholar] [CrossRef]
- Kumar, P.; Nishchal, N.K.; Omatsu, T.; Rao, A.S. Self-referenced interferometry for single-shot detection of vector-vortex beams. Sci. Rep. 2022, 12, 17253. [Google Scholar] [CrossRef]
- Vyas, S.; Kozawa, Y.; Sato, S. Polarization singularities in super- position of vector beams. Opt. Express 2013, 21, 8972–8986. [Google Scholar] [CrossRef]
- Zusin, D.H.; Maksimenka, R.; Filippov, V.V.; Chulkov, R.V.; Perdrix, M.; Gobert, O.; Grabtchikov, A.S. Bessel beam transformation by anisotropic crystals. JOSA A 2010, 27, 1828–1833. [Google Scholar] [CrossRef]
- Riaud, A.; Thomas, J.L.; Baudoin, M.; Matar, O.B. Taming the degeneration of Bessel beams at an anisotropic-isotropic interface: Toward three-dimensional control of confined vortical waves. Phys. Rev. E 2015, 92, 063201. [Google Scholar] [CrossRef]
- Khilo, N.A. Conical diffraction and transformation of Bessel beams in biaxial crystals. Opt. Commun. 2013, 286, 1–5. [Google Scholar] [CrossRef]
- Ruschin, S.; Leizer, A. Evanescent bessel beams. JOSA A 1998, 15, 1139–1143. [Google Scholar] [CrossRef]
- Grosjean, T.; Courjon, D.; Van Labeke, D. Bessel beams as virtual tips for near-field optics. J. Micro. 2003, 210, 319–323. [Google Scholar] [CrossRef] [PubMed]
- Liu, T.; Tan, J.; Lin, J.; Liu, J. Generating super-Gaussian light needle of 0.36 λ beam size and pure longitudinal polarization. Opt. Eng. 2013, 52, 074104. [Google Scholar] [CrossRef]
- Khonina, S.N.; Sergey, V.K.; Sergey, V.A.; Dmitry, A.S.; Janne, L.; Jari, T. Experimental demonstration of the generation of the longitudinal E-field component on the optical axis with high-numerical-aperture binary axicons illuminated by linearly and circularly polarized beams. J. Opt. 2013, 15, 085704. [Google Scholar] [CrossRef]
- Dehez, H.; April, A.; Piché, M. Needles of longitudinally polarized light: Guidelines for minimum spot size and tunable axial extent. Opt. Express 2012, 20, 14891–14905. [Google Scholar] [CrossRef] [PubMed]
- Allam, S.R.; Liu, L.; Cai, Y. Generating approximate non-diffractive three dimensional micro-size optical potentials by superposition. Opt. Commun. 2020, 477, 126297. [Google Scholar] [CrossRef]
- Belyi, V.; Forbes, A.; Kazak, N.; Khilo, N.; Ropot, P. Bessel–like beams with z–dependent cone angles. Opt. Eexpress 2010, 18, 1966–1973. [Google Scholar] [CrossRef]
- Allam, S.R. An intriguing interpretation of Cosine beams. arXiv 2023, arXiv:2307.14201. [Google Scholar]
- Porfirev, A.P.; Skidanov, R.V. Generation of an array of optical bottle beams using a superposition of Bessel beams. Appl. Opt. 2013, 52, 6230–6238. [Google Scholar] [CrossRef]
- Rao, A.S.; Yadav, D.; Samanta, G.K. Nonlinear frequency conversion of 3D optical bottle beams generated using a single axicon. Opt. Lett. 2021, 46, 657–660. [Google Scholar] [CrossRef]
- Wulle, T.; Herminghaus, S. Nonlinear optics of Bessel beams. Phys. Rev. Lett. 1993, 70, 10. [Google Scholar] [CrossRef]
- Rao, A.S. Saturation effects in nonlinear absorption, refraction, and frequency conversion: A review. Optik 2022, 267, 169638. [Google Scholar]
- Sutherland, R.L. Handbook of Nonlinear Optics, 2nd ed.; CRC Press: Boca Raton, FL, USA, 2003; pp. 33–120. [Google Scholar]
- Włodarczyk, P.; Pustelny, S.; Budker, D. System for control of polarization state of light and generation of light with continuously rotating linear polarization. Rev. Sci. Inst. 2019, 90, 013110. [Google Scholar] [CrossRef] [PubMed]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rao, A.S. Illustrations of Bessel Beams in s-Polarization, p-Polarization, Transverse Polarization, and Longitudinal Polarization. Photonics 2023, 10, 1092. https://doi.org/10.3390/photonics10101092
Rao AS. Illustrations of Bessel Beams in s-Polarization, p-Polarization, Transverse Polarization, and Longitudinal Polarization. Photonics. 2023; 10(10):1092. https://doi.org/10.3390/photonics10101092
Chicago/Turabian StyleRao, A. Srinivasa. 2023. "Illustrations of Bessel Beams in s-Polarization, p-Polarization, Transverse Polarization, and Longitudinal Polarization" Photonics 10, no. 10: 1092. https://doi.org/10.3390/photonics10101092
APA StyleRao, A. S. (2023). Illustrations of Bessel Beams in s-Polarization, p-Polarization, Transverse Polarization, and Longitudinal Polarization. Photonics, 10(10), 1092. https://doi.org/10.3390/photonics10101092




