Experimental Study on Flocculation Effect of Tangential Velocity in a Cone-Plate Clarifier

Abstract

A large number of particles with small size and light density in mine water are difficult to remove by traditional separation equipment. In order to improve the efficiency of mine water treatment, a cone-plate clarifier is proposed in this paper. The particle size distribution and their fragmentation fractal dimension were studied in 15 sampling points of the cone-plate clarifier to elucidate the process of floc growth and settling. The influence of the tangential inlet velocity size distribution characteristics was also studied. The results showed that the cone-plate clarifier can effectively improve suspended solids and turbidity removal efficiency. The floc growth and settlement courses are shown in the charts. The cone-plate clarifier was divided into four zones: micro flocculation, floc growing, settlement, and exit pipe. Because the fluid enters the cone-plate clarifier tangentially, the value of the inlet velocity and the tangential velocity are equal at the inlet feed. With the increase in tangential velocity, the flocculation efficiency first increased and then decreased. Comprehensive analysis of the particle greater than 50 μm in the effluent showed that the optimal inlet was qual to 1.63 m/s. By fitting the equations, the optimal SS and turbidity removal efficiency reached the maximum values of 92.04% and 80.18% at the inlet velocity of 1.86 m/s and 1.77 m/s, respectively.

1. Introduction

In recent years, the problem of water pollution is becoming more and more serious, which has attracted the attention of many people [1,2,3]. A large amount of mine water is pumped to the surface in the process of coal mining. In general, the mine water contains a lot of particles such as coal powder, sand, and stone [4,5]. The direct discharge of mine water will cause a waste of water resources and environmental pollution [6,7]. In water treatment, the aggregation of small particles into large particles is the key to removing suspended solids (SS), turbidity, and particle number in water treatment works [8,9]. Due to the small size, light specific gravity, and slow settling speed of particles in mine water, it is difficult to remove them [10]. Therefore, it is very meaningful to study the aggregation and settlement mechanism of particles in mine water.

Particles and natural organic matter in suspension can be effectively removed by flocculation-sedimentation, which is widely used in water treatment engineering [11,12,13]. Magnetic flocculation has high separation efficiency. Magnetic seeds can be recycled, so the operation cost is low [8,14,15]. Numerous flocculation studies have been carried out in jar tests and these experiments proved that the jar test can predict the behavior of the flocculation process in many cases [16,17,18]. It has been confirmed that the hydraulic conditions have an important influence on the floc characteristics in the flocculation process, and further affect the flocculation effect and purification efficiency [19]. Some experts have studied the coagulation-flocculation process and the dynamic separator based on swirling motion to remove the solid phase in the water phase [20]. The factors affecting the floc properties include the type and dosage of coagulant, shear rate, concentration, pH value, temperature, etc. [21,22]. The shear rate is one of the most important factors affecting the collision frequency between particles and flocs. Bamrungsri et al. [23] and Puprasert et al. [24] studied the effect of centrifugal force produced by tangential flow injection on coagulation-flocculation. The results show that the tangential jet produces a strong velocity gradient at the pipe wall, which improves the removal efficiency of turbidity and SS, and promotes the coagulation and flocculation phenomenon. The jar test can reflect the flocculation state in many cases, but the jar test method can only reflect the final state of flocs. At present, the flocculation of flocs in the process of cyclone flocculation has been rarely studied. Becker et al. showed that the flocs produced in the flocculation process can directly affect the precipitation efficiency [25,26]. Therefore, it is feasible to study the different zones of the cyclone flocculation process to elucidate the evolution of flocs in the process of flocculation and sedimentation.

In the flocculation process of water treatment, SS and turbidity are taken as the main indicators to study [27]. In low turbidity water, turbidity cannot fully represent the number of particles in water, so it is necessary to directly measure and detect the number of particles with different sizes [28,29]. Online particle counters can monitor particle size distribution (PSD) and quantify particle number. Yao et al. studied the correlation between particle number and water turbidity [30]. Compared with a turbidimeter, a particle counter can provide better particle characterization information on the micro scale. Because of its high sensitivity to small particles, the measurement results are more accurate. The combination of particle counter and turbidity meter to monitor the operation of water treatment has been more and more widely used in water treatment. However, a large number of experiments have been carried out to study the final results of the jar test, and few studies have been made on the hydrocyclone flocculation process.

In this study, a conical cone-plate clarifier was proposed (Figure 1). It mainly contains a cylindrical body, conical part, cone-plate, feed inlet, underflow, and overflow. After mixing with the coagulant aid, magnetic seed, and flocculant, the mine water enters the cone-plate clarifier tangentially to form a swirling flow field. Under the action of centrifugal force and gravity, particles are collided and gathered. The large flocs moved radially to the outer wall under centrifugal force and settled to the bottom under gravity. The small flocs entered the cone-plate with the flow field. At the cone-plate, due to the principle of shallow pool, some small flocs further aggregated, grew, and settled again. Finally, flocs settled to the underflow and discharged intermittently through the underflow port. The clean water flowed out through the overflow pipe. Compared with the traditional sewage treatment equipment, it can improve the efficiency of particle separation. On one hand, the centrifugal force accelerates the collision rate of flocs; on the other hand, the conical plate can increase the settling area of flocs [31].

In this paper, a series of flocculation and sedimentation experiments were carried out in a cone-plate clarifier at different inlet velocities to study the effects of PSD and fragmentation fractal dimension (D_f) on particle separation efficiency. First, the variation of SS removal efficiency, turbidity removal efficiency, and total particle number at different positions in the cone plate clarifier were studied. Next, the growth law of particles with different sizes was studied, and the change of PSD under different inlet velocity was analyzed. Furthermore, the relationship between the fragmentation fractal dimensions of particles and the growth law of particles was discussed [32,33,34,35,36,37]. Finally, according to the number of particles with different sizes and fragmentation fractal dimensions, the cone-plate clarifier was divided into four zones. According to the PSD of flocs at the end of micro flocculation, sedimentation, and exit pipe (initial floc, growing floc, final floc), and the percentage of particles greater than 50 μm at the end of micro flocculation, the optimal inlet velocity was discussed.

2. Materials and Methods

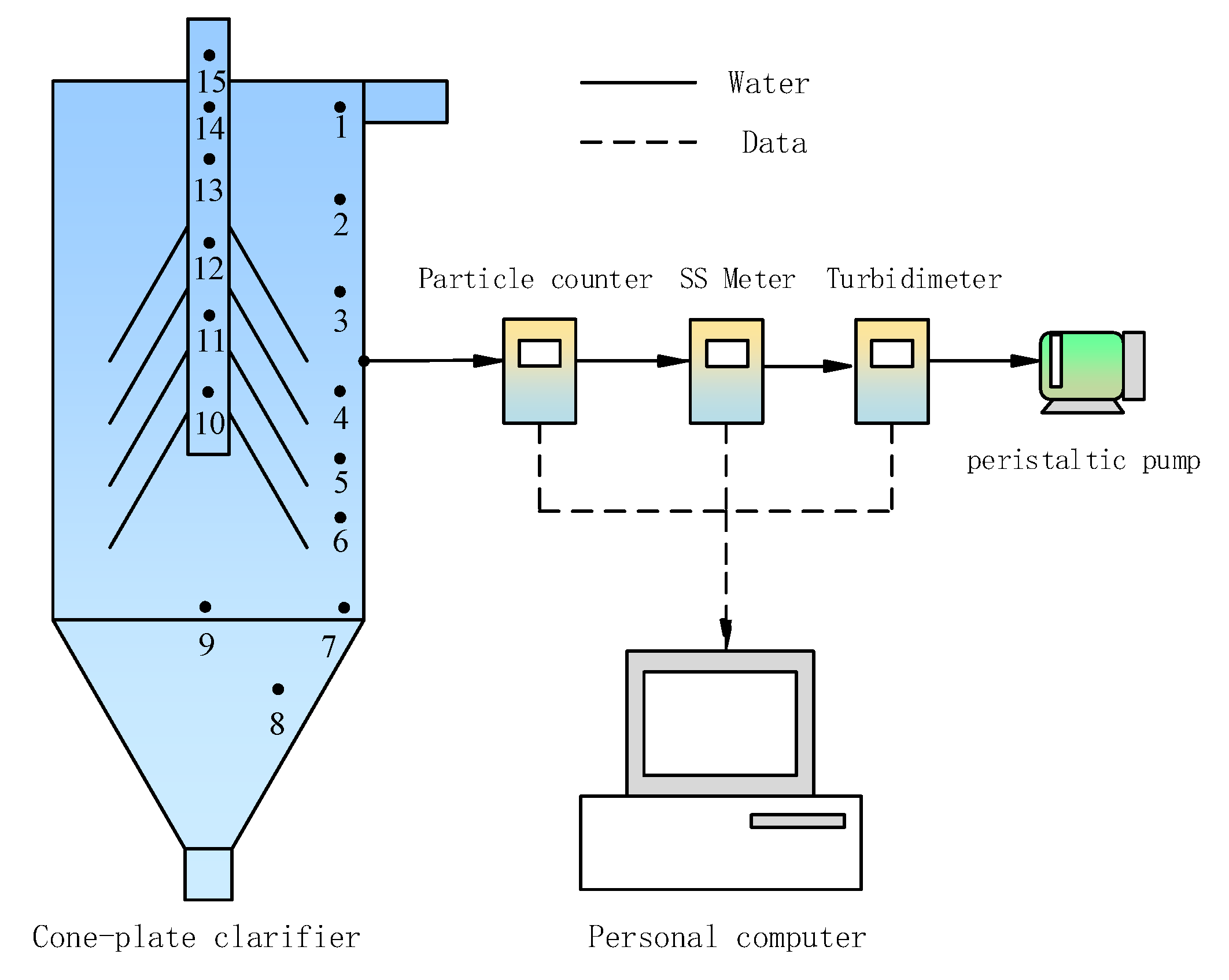
2.1. Materials and Detecting Equipment

The coal mine water used in this research came from a mine in Ningxia, China. The water quality is presented in

Table 1. Water qualities index of the coal mine water.

Property Indexes	SS (mg/L)	Turbidity (NTU)
Value	300–400	50–100

. The SS, turbidity, and pH value of the coal mine water was obtained by the detectors SS-1Z, WGZ-1b, and pH meter, respectively. Particle size analyzers are widely used to monitor the evolution of PSD during flocculation processes, whereas online particle counters have received little use in previous works. Here, a particle counter (2200PCX, HACH, Loveland, CO, USA) was used to measure the number of particles with different particle sizes.

Three materials were used in this experiment: poly-aluminum chloride (PACl), magnetic seed (Fe₃O₄), and polyacrylamide (PAM). The polyaluminum chloride (PACl) was chosen as the coagulant to form colloid aggregates. Stock PACl solutions (1%) were prepared by dissolving the reagent in deionized water. Polyacrylamide (PAM) was selected as the flocculant. The concentration of the aqueous solution was 3‰, and the molecular weight was 12 million. These solutions were used directly, without further preparation. The Fe₃O₄ was used as a magnetic seed. The size of magnetic seed was 200 mesh.

In the process of mine water treatment, the addition of chemicals is a very important link. The optimal dosage of chemicals can be obtained through the beaker test. The dosage of PACl, magnetic seed, and PAM was 60 mg/L, 40 mg/L, and 6 mg/L, respectively. The order of adding the agents was PACl, magnetic seed, and PAM.

2.2. Test System

The test system is mainly composed of a submersible pump, peristaltic pump, pipeline mixer, cone-plate clarifier, and other equipment. The flow chart of the test system is shown in Figure 2. Mine water was pumped into the pipe mixer by a submersible pump. Through the peristaltic pump, the agent was added into the pipe mixer for mixing and flocculation. The order of adding chemicals was PACl, magnetic seed, and PAM. The flocs are fully mixed by the pipe mixer, and then enter the cone-plate clarifier for further growth, separation, and sedimentation.

The detection system is shown in Figure 3. During the experiment, samples were collected from 15 sampling points, and the samples were measured and monitored by the SS meter, turbidity meter, and particle counter in real time to systematically study the whole process of continuous evolution of magnetic floc characteristics in the cone-plate clarifier. The 15 points shown in the cone-plate clarifier are the locations where samples can be taken. These meters were set up in series. For dynamic monitoring, the water sample was circulated from the cone-clarifier at a flow rate of 100 mL/min through transparent plastic tubing with a 5 mm internal diameter by a peristaltic pump. The peristaltic pump was placed on the back of the on-line particle counter, SS meter, and turbidimeter to minimize the damage of floc in the pump clamping part.

A modified version of the standard flocculation test procedure was conducted using an online 2200PCX particle counter to achieve the dynamic characterization of the particle size distribution (PSD). The PSD was represented by the number of particles in various size ranges. Accordingly, the size ranges corresponding to channels (CH1–CH5) in the particle counter are shown in

Table 2. Particle size range setting of the particle counter.

Channel	Minimum Size (μm)	Maximum Size (μm)	Maximum Range (Number/mL)
CH1	2	5	17,000
CH2	5	10	17,000
CH3	10	50	17,000
CH4	50	100	17,000
CH5	100	500	17,000

.

2.3. Fragmentation Fractal Dimensions of Particle Size Distribution

According to the study of O’Melia [38], the particle distribution can be calculated by the following formula:

$$n\left(d_p\right)=\frac{dN}{d\left(d_p\right)}\propto d_p^{-D_P}$$

(1)

where d_p is the diameter of the flocs; n(d_p) is the particle size distribution function; and dN is the number of the flocs for diameters between d_p and d_p plus d(d_p). D_p is the fractal dimensions.

Taking the natural logarithm for both ends of Equation (1) gives:

$$D_p=\frac{-\ln \left[n\left(d_p\right)\right]}{\ln d_p}$$

(2)

Equation (2) has been used to experimentally study the raw water of the Songhua River, the sedimentation tank effluent, and the filter effluent. It was demonstrated that the particle number and the corresponding particle size were approximately linearly related on a double logarithmic coordinate system, and the corresponding β values were measured.

Most water plants use particle counters to check the particle count. Therefore, the calculation of the fragmentation fractal dimensions of particle size by particle count can effectively guide the water treatment in water plants. A particle counter was used to calculate the fragmentation fractal dimensions of particle size, but it can only measure the number of particles located in certain particle size ranges.

According to a study by Nan Jun [30,39], there is no clear linear relationship between the logarithm of the number of flocs in the corresponding particle size range and the logarithm of the particle size during the coagulation process. Equation (2) is not suitable for research on the fractal characteristics of PSD in flocculation processes.

Nan Jun [30,39] attempted to introduce fractal theory into the study of floc size distribution by using a particle counter to investigate the fractal characteristics of floc size distribution in the flocculation process, starting from the size distribution function of flocs.

In order to apply Equation (2) to the coagulation and sedimentation process, the following transformation is required:

$$p\left(r\right)=\frac{N\left(r\right)}{N}=\frac{{\displaystyle {\int }_0^{r}dN}}{N}=\frac{{\displaystyle {\int }_0^{r}n}\left(d_p\right)d\left(d_p\right)}{N}\propto \frac{1}{N}{r}^{D_f}$$

(3)

Equation (3) is taken from the natural logarithm at both ends.

$$D_f=\frac{\ln p\left(r\right)}{\ln r}$$

(4)

where p(r) is the probability density of particle size; r is the diameter of the particle; and D_f is the fragmentation fractal dimensions of particle size distribution. D_f has been used to evaluate the dispersion in water [30,39].

The first derivative of D_f is the ratio of change of the fractal dimension. The independent variable is the number of sampling points. The greater the change in the value of D_f, the greater the value of the first derivative of D_f. As the value of D_f increases, the corresponding value of the first derivative of D_f is positive. Conversely, when the value of D_f decreases, the corresponding value of the first order derivatives of D_f is negative.

3. Results and Discussion

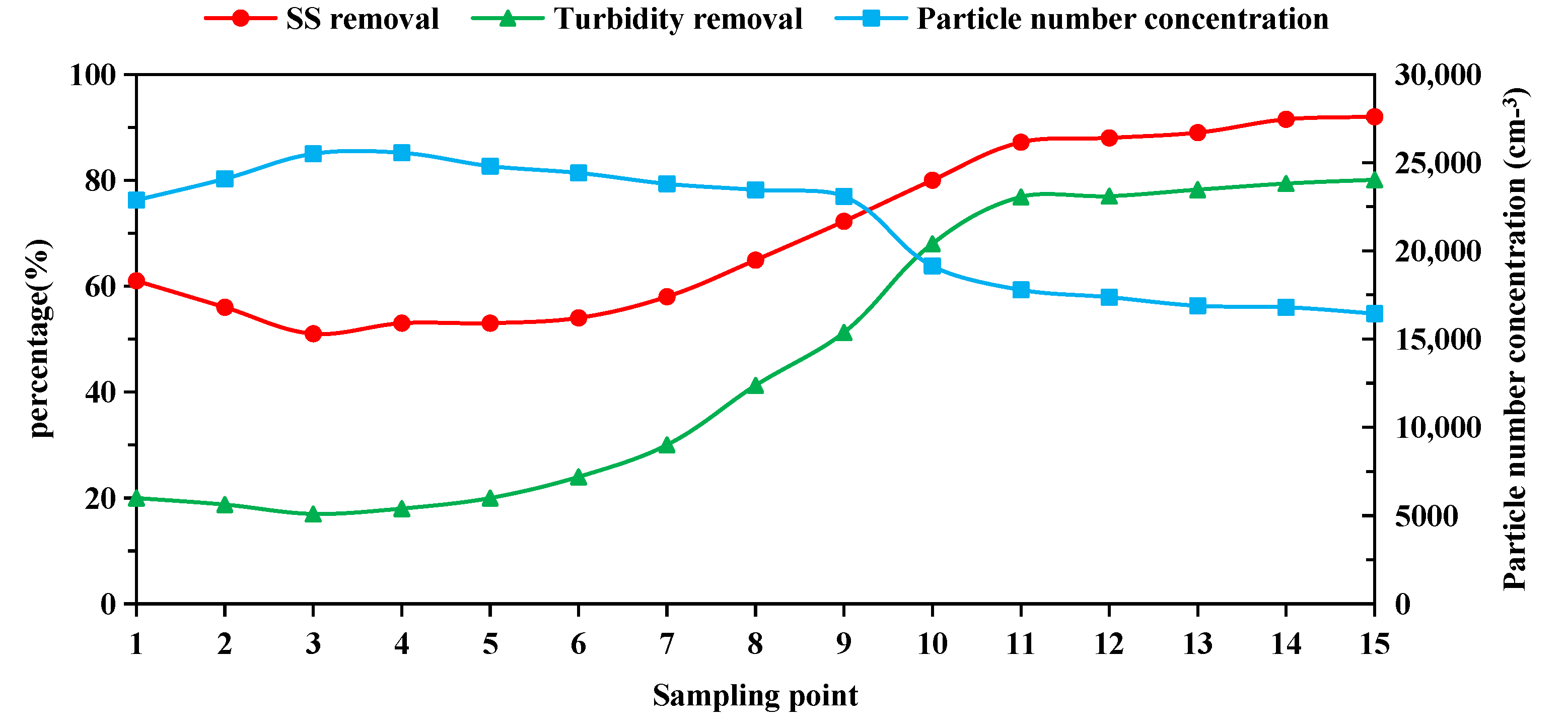
3.1. Water Quality Status in Different Flocculation States

In order to study the flocculation, growth, separation, and settling mechanism of flocs, samples were collected from 15 sampling points in the cone-clarifier for analysis. The particles move to the next sampling point with the increase in flocculation time in the cone-plate clarifier, that is, the flocculation time of the measured sample increases with the increase in sampling point number. The flocculation time of particles at sampling point 1 was the shortest, and that of particles at sampling point 15 was the longest. The SS, turbidity, and particle number of each sampling point in the cone-clarifier were studied, and the flocculation mechanism discussed.

As shown in Figure 4, the SS and turbidity removal efficiency first decreased and then increased with the increase in sampling point number. In contrast, the number of particles first increased and then decreased. At sampling point 1, the mine water in the pipeline mixer mixed with the reagent and entered the cone-plate clarifier tangentially at a high speed. At this time, it is in the primary stage of flocculation. The removal efficiency of SS and turbidity were 61% and 20%, respectively, and the particle number was 21,872 mL⁻¹. At sampling points 2 and 3, the shear speed was relatively fast, and a large number of smaller particles were generated. At this stage, the flocs grew rapidly. Therefore, the removal efficiency of SS and turbidity decreased, and the number of particles increased to 25,552 mL⁻¹.

At sampling points 4–6, the particle size of flocs grew to a larger size, at the same time, the tangential velocity decreased greatly. A smaller shear force can reduce the fragmentation of the large flocs that have been formed; on the contrary, the large flocs will further grow slowly under the action of lower shear speed. A large number of smaller particles grew into a small number of larger particles. The total number of particles decreased, and the removal efficiency of SS and turbidity increased. At sampling points 7–9, the mine water was fully flocculated. The floc reached the maximum size and settled rapidly under the action of gravity. Therefore, the removal efficiency of SS and turbidity increased rapidly, and the number of particles decreased. At sampling points 10–15, the removal efficiency of SS and turbidity increased, while the number of particles decreased, which was mainly due to the further flocculation and sedimentation of a large number of particles at the cone-plates. At sampling point 15, the water quality reached the optimal value. The removal efficiency of SS and turbidity reached 92% and 80.1%, respectively, and the number of particles was reduced to 16,445 mL⁻¹.

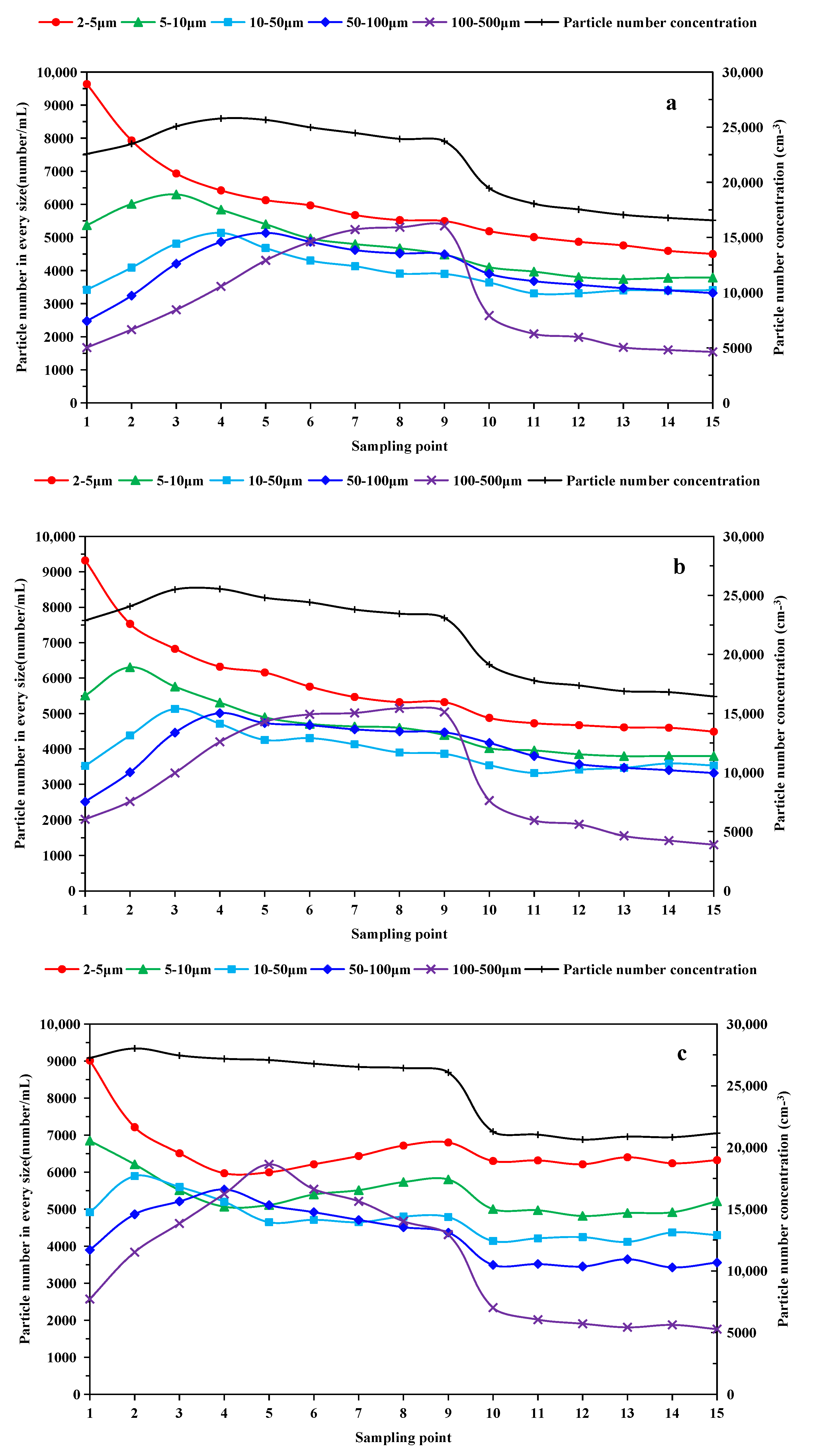
3.2. Particle Size Distribution in Different Flocculation States

In order to further study the mechanism of different flocculation states, the evolution of different particle sizes was studied. As shown in Figure 5, the total number of particles increased first and then decreased. Finally, the total number of particles at 15 sampling points was the least, which indicates that the flocculation process can effectively remove particles in the water phase.

At an inlet velocity of 1.63 m/s (Figure 5b), the number of particles in each channel changed sharply in the initial stage of flocculation. At sampling points 1–9, the number of 2–5 μm particles decreased rapidly, the number of 5–100 μm particles first increased and then decreased, and the number of 100–500 μm particles increased and then tended to stabilize. Finally, the number of particles in all channels reached a steady state, which indicates that the smaller particles grow into larger particles in this period. It should be noted that the variation of particle curves in different size ranges is not synchronous. The peak value of the particle number of the small size particles appeared earlier. With the increase in particle size, the peak of particle number appeared later, which indicates that the primary particles are prone to form micro-flocs rather than aggregate into large flocs directly. The larger particles were gradually formed by the combination of smaller micro flocs and monomer particles. At sampling point 10–15, the particles in all channels were reduced, which indicates that the cone-plate can effectively remove particles of various sizes.

Comparing the three images in Figure 5a–c, all the curves in Figure 5a were similar to those in Figure 5b in shape. The differences were that the peak of all curves at v = 0.46 m/s were delayed compared with that at v = 1.63 m/s because of the low energy input. When the shear velocity was 3.47 m/s, all curves changed rapidly and reached steady stage quickly. Then, the number of particles smaller than 10 μm began to increase, and the number of particles larger than 100 μm began to decrease, which indicates that a large number of flocs with a larger particle size were broken due to high energy dissipation.

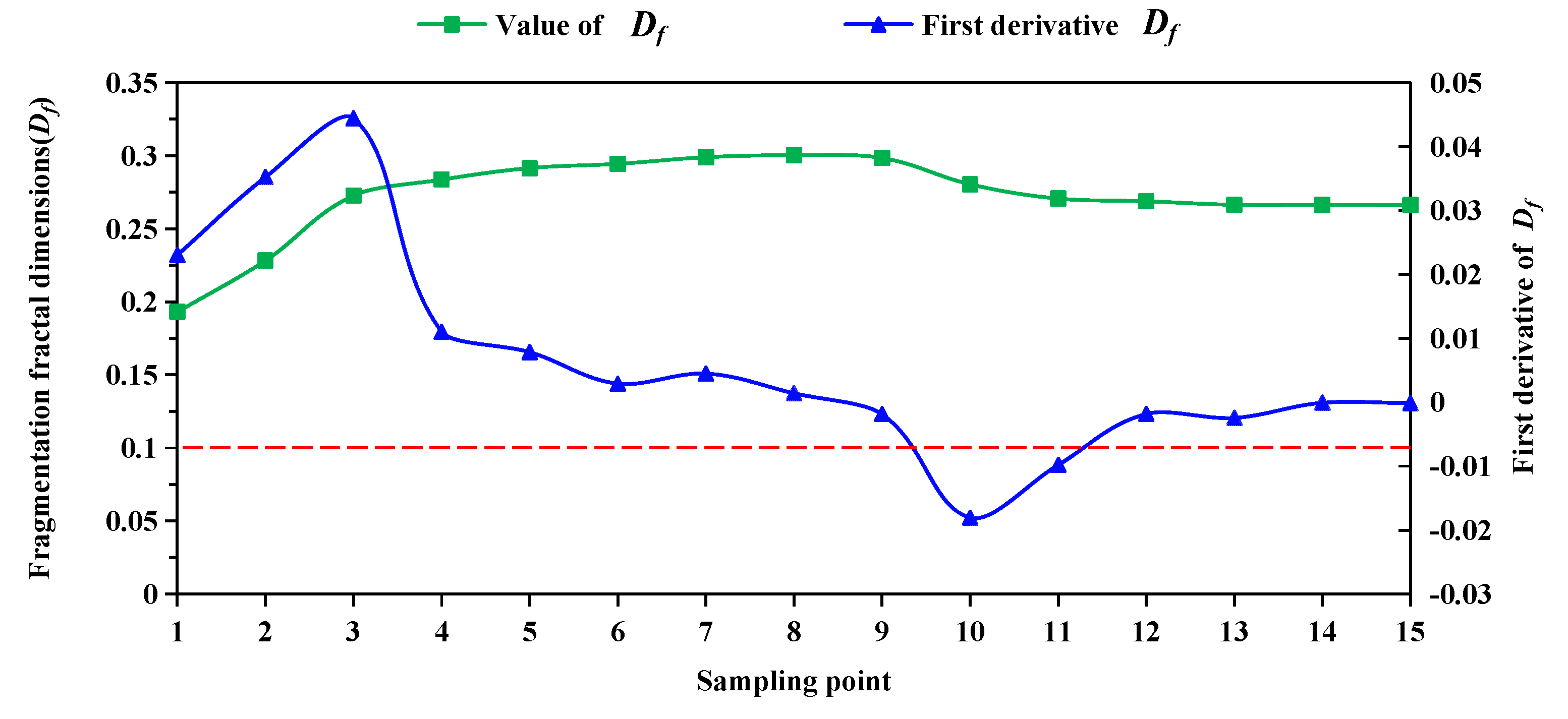
3.3. Evolution of Particle Size Distribution Fragmentation Fractal Dimensions

Fractal theory has been widely used to study the structure and dispersion of flocs in the flocculation process. In previous study, it has been confirmed that the fragmentation fractal dimensions of particle size distribution (D_f) can represent the change in particle size distribution in water [30]. The higher values of D_f reflect there are much larger particles and flocs in water, and lower values indicate that the small flocs are dominant in water [40,41]. In addition, the fractal features of particle size distribution can well represent the description details that PSD cannot express. Therefore, D_f can be used as a supplement to characterize the particle size growth. In order to study D_f better, the first derivative of D_f was used. The rate of change of D_f can be expressed by the first derivative of D_f. The inlet speed of 1.63 m/s was chosen as an example to discuss the variation of D_f and its first derivative at different sampling points (shown in Figure 6).

At the inlet speed of 1.63 m/s, the fragmentation fractal dimensions first increased rapidly to a stable value, and then decreased slightly to a stable value. At the first nine sampling points, the first derivative of D_f curves were at a positive value, which increased rapidly between sampling points 1 to 3, then decreased to around zero from point 3 to point 4, finally fluctuated in a narrow range between sampling points 4 to 9. This indicated that the growth rate of D_f increased in the early stage, and then the growth rate decreased to a stable period.

The first derivative of the D_f curve were negative at sampling points 9–12. At sampling points 9–10, the first derivative of D_f decreased rapidly because a large number of particles settled in the underflow. At sampling points 10–12, the first derivative of D_f increased gradually and remained stable around 0, which was due to the flocculation and adsorption of flocs by the cone-plate and the further settlement of large particles. Finally, D_f fluctuated in a narrow range around 0 at sampling points 12–15.

At sampling point 3, the first derivative of D_f reached the peak value, which indicated that the floc growth rate here was the fastest, and a large number of small flocs grew into larger flocs. At sampling point 10, the first derivative of the fragmentation fractal dimensions reached the minimum value, and the value was negative, which indicates that the generated large-particle flocs mainly settle and separate here. Finally, the number of particles decreased and the effluent quality improved.

3.4. Segment the Flocculation Process in the Cone-Plate Clarifier

In this section, we analyzed the particle size distribution and D_f of 15 sampling points in the cone-plate clarifier to study the evolution of flocculation. According to the progressive shear force, Wang Dongsheng divided the flocculation time into three stages. Particle size distribution and floc structure have a great influence on flocculation effect, but shear velocity alone cannot evaluate the properties of particles. Therefore, the cone-plate clarifier was divided into four zones by the change of PSD and D_f to further study the flocculation process. The inlet speed of 1.63 m/s was selected for regional division. The zone division of the flocculation process is shown in Figure 7.

In the pipe mixer, the reagent was mixed rapidly under the action of strong shear force. The particles become unstable due to the change of surface charge. In this zone, the colloidal particles have enough chance to collide with each other under the action of water flow, which provides conditions for the next zone of condensation. Therefore, this zone was called preparation.

In the cone-plate clarifier, the flocs collided and grew rapidly. Sampling point 1 was close to the inlet of cone-plate clarifier. At this sampling point, the number of 2–5 μm particles was the largest, and the larger the diameter of the particles, the fewer the number of particles. In this position, small flocs were dominant. From sampling point 1 to 3, with the increase of flocculation time, the particle size of 2–5 μm decreased rapidly, and the average particle size and the fragmentation fractal dimensions increased. At this time, flocs were mainly composed of small particles, and the particles grew rapidly, so this zone was called micro flocculation.

Between sampling point 4 and sampling point 6, the quantity of flocs with particle size less than 100 μm decreased slightly and the floc quantity with particle size between 100–500 μm still increased rapidly. The fragmentation fractal dimensions increased slowly. At this time, it mainly generated large size particles including flocs larger than 500 μm, so this zone was called floc growing.

At sampling point 7–9, the particle size of each channel tended to be stable, and the fragmentation fractal dimensions was basically stable. At this position, the flocculation and fragmentation of flocs reached dynamic equilibrium. At this time, the floc particle size reached the maximum, and it settled to the underflow settlement area under the action of gravity, so this zone was called settlement.

Between sampling points 10–15, the particles in each channel were further reduced due to the cone-plate and gravity, especially the large particles. Finally, the particle number and fragmentation fractal dimensions of all channels fluctuated near the minimum value and reached a stable state, so this zone was called exit pipe.

During the micro flocculation zone, the number of particles smaller than 100 μm grew rapidly and the fragmentation fractal dimensions increased rapidly from 0.17 to 0.27. During the floc growing zone, the number of particles smaller than 100 μm basically reached stability, while the number of particles larger than 100 μm grows faster. The fragmentation fractal dimensions increased slightly from 0.27 to 0.29. During the settlement zone, the number of particles of all sizes reached equilibrium and the fragmentation fractal dimensions stabilized at around 0.3. During the exit pipe zone, the number of particles of each size decreased after the settlement zone, where the larger the particle size, the more particles settled, the average particle size in the flow field decreased, and the fragmentation fractal dimensions decreased from 0.3 to about 0.26.

3.5. The Average Size and Fragmentation Fractal Dimensions under Different Speed at Different Zones

Initial floc, growing floc, and final floc are the floc at the end of the micro flocculation zone (point 3), floc growing zone (point 6), and exit pipe zone (point 15), respectively.

Figure 8 and Figure 9 show the average size of flocs and fragmentation fractal dimensions under different speeds at the end of the micro flocculation zone (initial floc), floc growing zone (growing floc), and exit pipe zone (final floc) at different inlet velocities. Figure 10 shows the percentage of particles larger than 50 μm in the final floc during the exit pipe zone.

The average particle size and fragmentation fractal dimensions of the initial floc increased with the increase in inlet velocity, and the average particle size of the flocs finally reached 47.5 μm. Because of the small size of the initial floc in the micro flocculation zone, the increase in shear velocity increased the number of particle collisions, which promoted flocculation without shear breakage, so it is easy to promote the growth of flocs. Meanwhile, the flocs generated at a faster speed are denser.

When the velocity was less than 1.63 m/s, the average particle size of the growing floc remained unchanged and the fragmentation fractal dimensions increased slightly with the increase in velocity. The effect of shear velocity on particle collision flocculation is greater than that of shear fragmentation at tangential velocities. This showed that with the increase in velocity, the flocs were denser, less likely to break, and more likely to settle with the same average particle size. Therefore, the number of the final floc larger than 50 μm decreased due to the faster settling speed.

When the inlet velocity was faster than 1.63 m/s, the particle size and fragmentation fractal dimensions of the growing floc decreased. At this time, particle shear fragmentation became dominant. The floc size was larger than that of the initial floc in the micro flocculation zone, and too fast a shear speed could cause the shear damage of flocs. Therefore, the number of particles larger than 50 μm reduced because of the fragmentation of particles.

The average particle size and the fragmentation fractal dimensions of the final floc decreased slightly with the increase in velocity, and the number of particles larger than 50 μm in Figure 10 decreased. This is mainly due to the fact that particles larger than 50 µm and with fragmentation fractal dimensions greater than 0.27 settled mostly in the settling zone. At greater than 1.63 m/s, the average particle size and the fragmentation fractal dimension decreased. This is mainly due to the fact that the larger shear velocities break up the larger flocs, resulting in an increase in the number of particles larger than 50 µm. In all speed ranges, with the increase in velocity, the percentage of particles larger than 50 μm decreased in the final floc during the exit pipe zone.

3.6. The Optimal Effluent Quality after Flocculation

According to the experiments, the optimal inlet velocity was chosen as 1.63 m/s. The quality of mine water before and after treatment is shown in

Table 3. Comparison of water quality parameters before and after treatment.

Property Indexes	Value before Treatment	Value after Treatment	Removal Efficiency
SS	355.32 mg/L	12.23 mg/L	92.01%
Turbidity	61.44 NTU	28.39 NTU	80.09%

.

As shown in Table 3, at this inlet velocity, SS was reduced from 355.32 mg/L to 28.39 mg/L and the SS removal efficiency reached 92.01%. Turbidity was reduced from 61.44 NTU to 12.23 NTU, with a efficiency removal efficiency of 80.09%.

The SS and turbidity removal efficiency were experimentally investigated at different tangential velocities. To further explore the effect of tangential velocity, the fitting equations were performed at different tangential velocities. The experimental results and the fitting equations are shown in Figure 11.

As shown in Figure 11, the removal of SS and turbidity increased first and then decreased as the tangential velocity increased. The removal efficiency of SS and turbidity at different tangential velocities was investigated by fitting the equations. The correlation coefficients were R = 0.9699 (SS removal efficiency) and R = 0.9968 (turbidity removal efficiency), respectively. These results showed that the equations had a high prediction accuracy.

Table 4. Values of experimental and predicted SS and turbidity removal efficiency.

Inlet Velocity (m/s)	SS Removal Efficiency (%)			Turbidity Removal Efficiency (%)
	Exp.	Pred.	Err.	Exp.	Pred.	Err.
0.46	82.85	83.41	0.56	72.74	73.01	0.27
0.93	89.07	88.24	0.83	77.64	77.21	0.43
1.63	92.01	91.81	0.20	80.09	80.09	0.00
2.31	90.46	91.12	0.66	78.77	79.01	0.24
3.47	80.69	80.49	0.20	68.46	68.38	0.08

revealed a good agreement between the values predicted by the equations and the actual experimental results.

According to the solution of the equation, the maximum removal efficiency of SS is 92.04% when the tangential velocity is 1.86 m/s, and the maximum removal efficiency of turbidity is 80.18% when the tangential velocity is 1.77 m/s.

4. Conclusions

The main conclusions of this study are listed as follows:

• A cone-plate clarifier was proposed to remove the particles with small size and low density in mine water. The particle collision efficiency was improved by the swirl field combined with gravity field. The larger particles formed by flocculation settled to the underflow under the action of gravity. The small particles that cannot settle to the underflow can be removed by the cone-plate to improve the particle removal efficiency. This has implications for our efforts to improve the efficiency of mine water treatment.
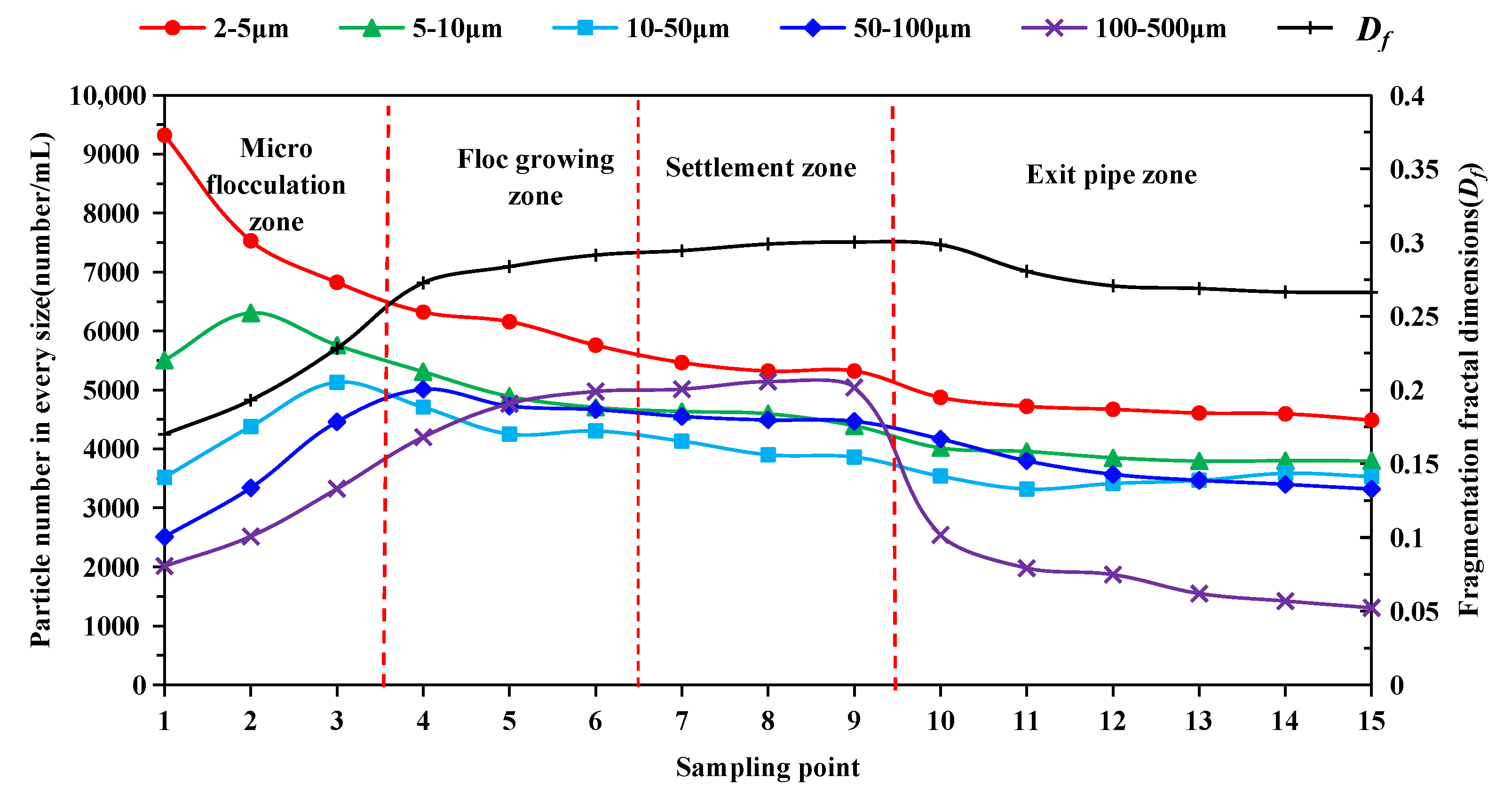
• The number of particles with different sizes at 15 sampling points measured by an on-line particle counter was used to study the growth law of particles in the cone-plate clarifier. In the early stage of flocculation, the particle size was small and the growth rate was fast. In the later stage of flocculation, the particle size was large and the growth rate was slow. Larger particles were gradually formed by the combination of smaller micro flocs and monomer particles, rather than directly aggregated into large flocs. This conclusion is consistent with Nanjun’s research [30,39].
• The particle size distribution of different sampling points was characterized by the fragmentation fractal dimensions. In this study, the first derivative of D_f was used as an effective method to analyze PSD and D_f. At sampling point 3, the first derivative value of D_f was the largest, which means that the growth rate was the fastest. At sampling point 10, the first derivative value of D_f was the lowest and negative, which indicated that the particle sedimentation rate was the fastest at this point.
• The number and fragmentation fractal dimensions of particles with different sizes were dynamically analyzed by the on-line particle counter. Based on the variation of PSD and D_f at different sampling points, the cone-plate clarifier was divided into four zones: micro flocculation, floc growing, settlement, and exit pipe. The change in particle characteristics in each zone can be detected in real time by using the D_f value.
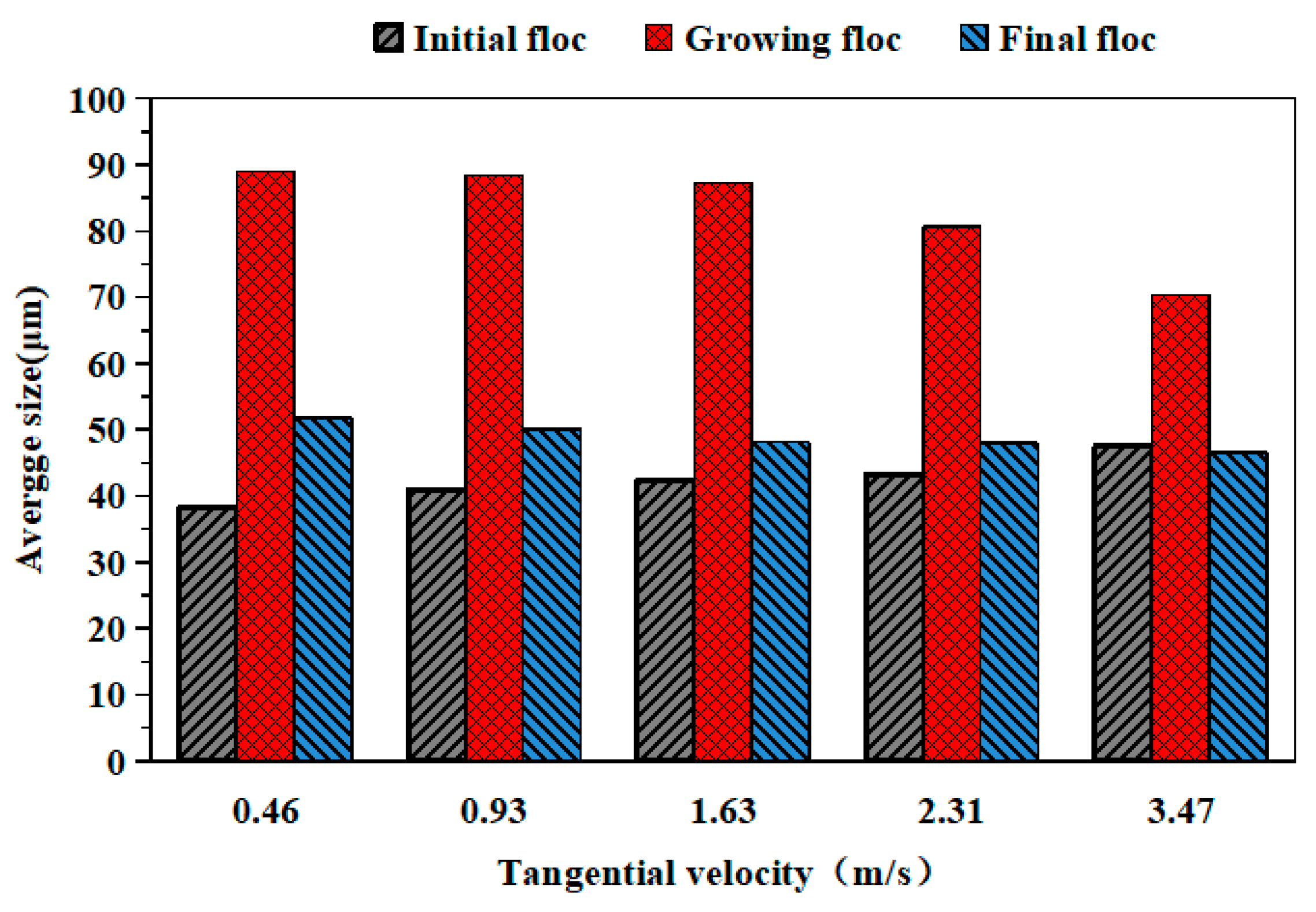
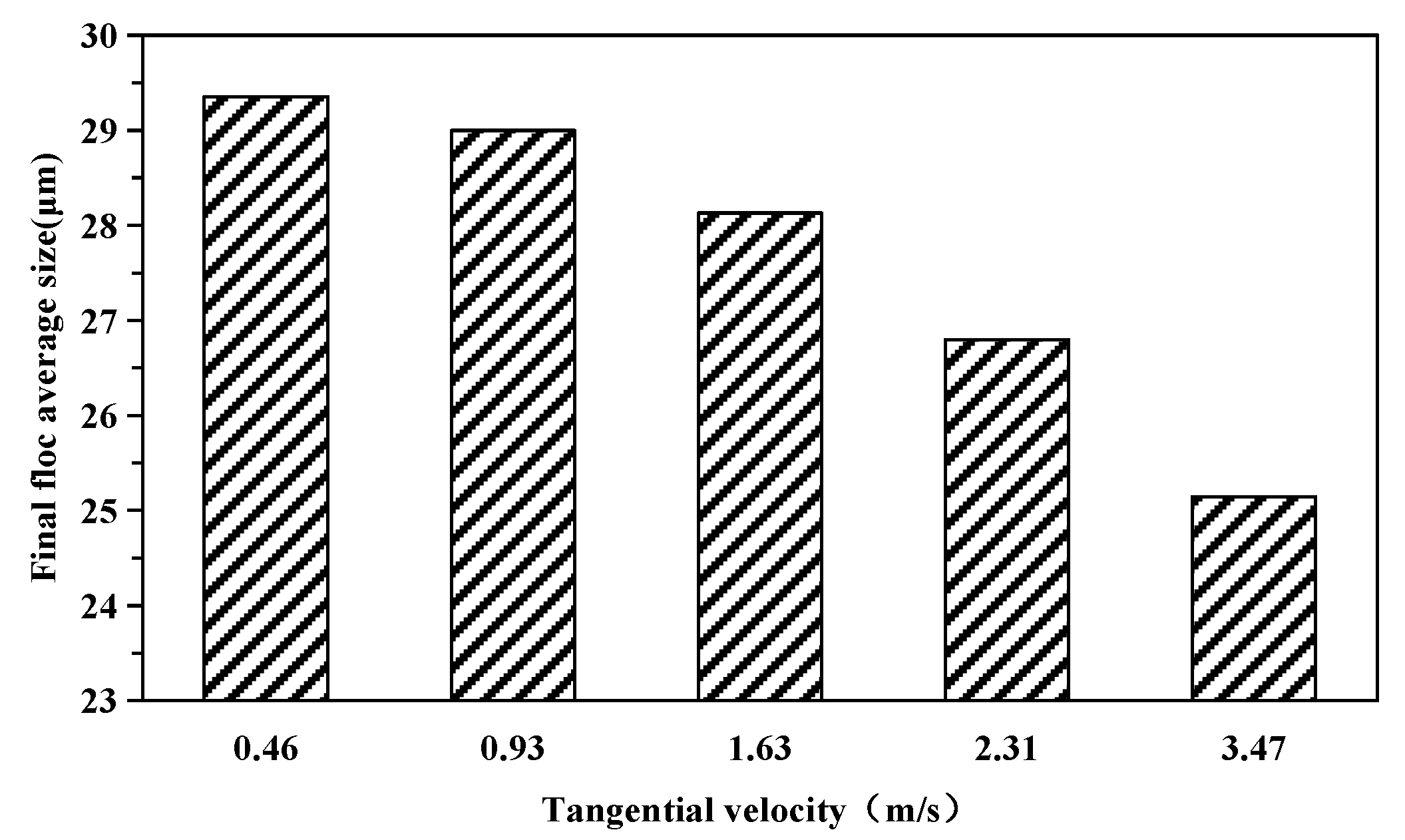
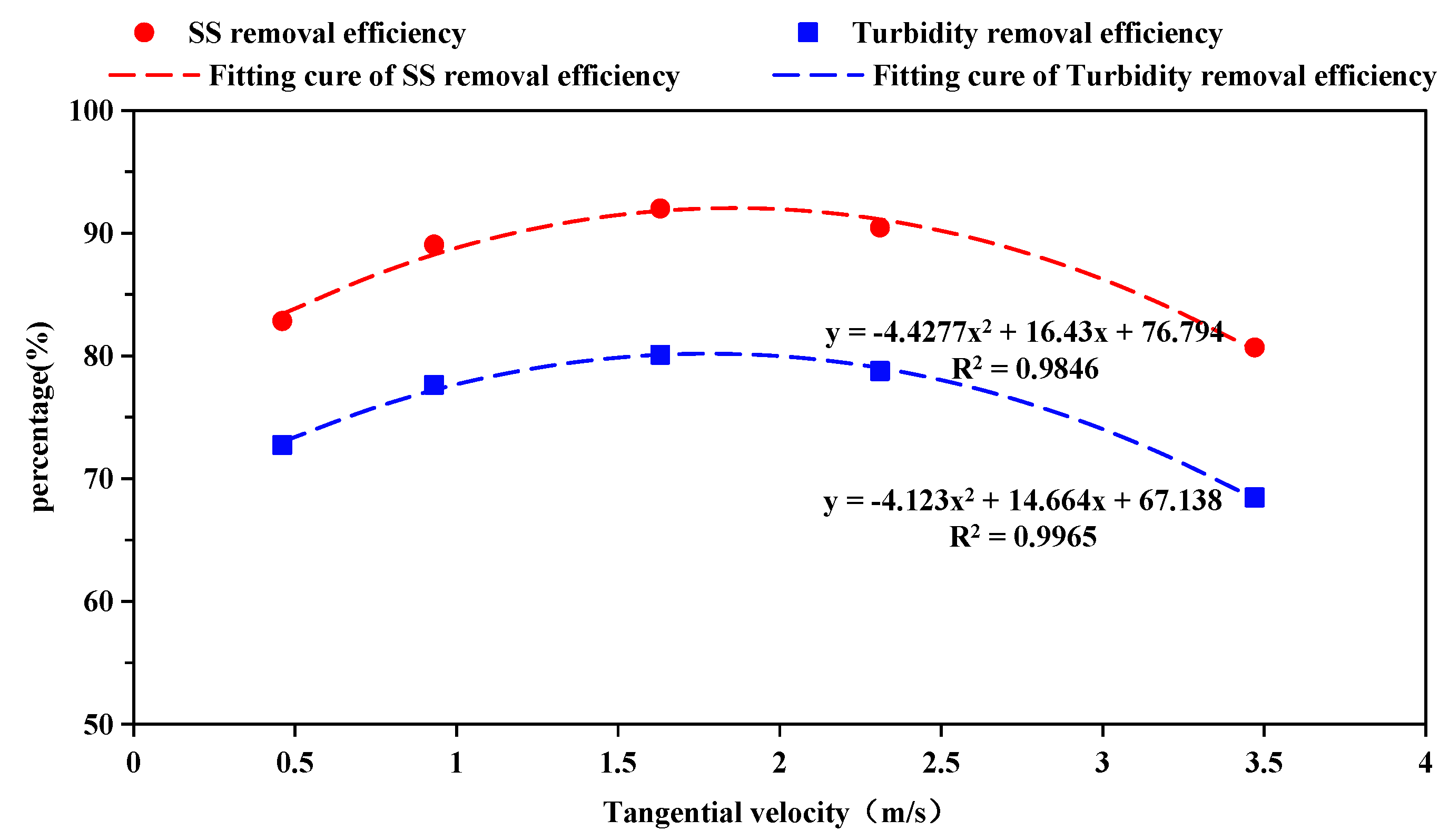
• The average size, fragmentation fractal dimensions of particles in different zones and the particle number of the final floc size larger than 50 μm at different speeds were studied. The optimal inlet velocity of the cone-plate clarifier was 1.63 m/s. The removal efficiency of SS and turbidity reached 92.01% and 80.0.9%, respectively. The particle number was reduced to 16,445 mL⁻¹.
• The fitting equations of SS and turbidity removal efficiency were performed at different tangential velocities. According to the solution of the equation, the maximum removal rate of ss was 92.04% when the tangential velocity was 1.86 m/s, and the maximum removal rate of turbidity was 80.18% when the tangential velocity was 1.77 m/s.
• Most water plants use particle counters to check the particle count. Therefore, measuring the number of particles and fragmentation fractal dimensions by means of a particle counter allows for the water quality to be checked in real time and effectively guides the water treatment in water plants.