A Method of Bending Shrinkage Groove on Vortex Suppression and Energy Improvement for a Hydrofoil with Tip Gap
Abstract
1. Introduction
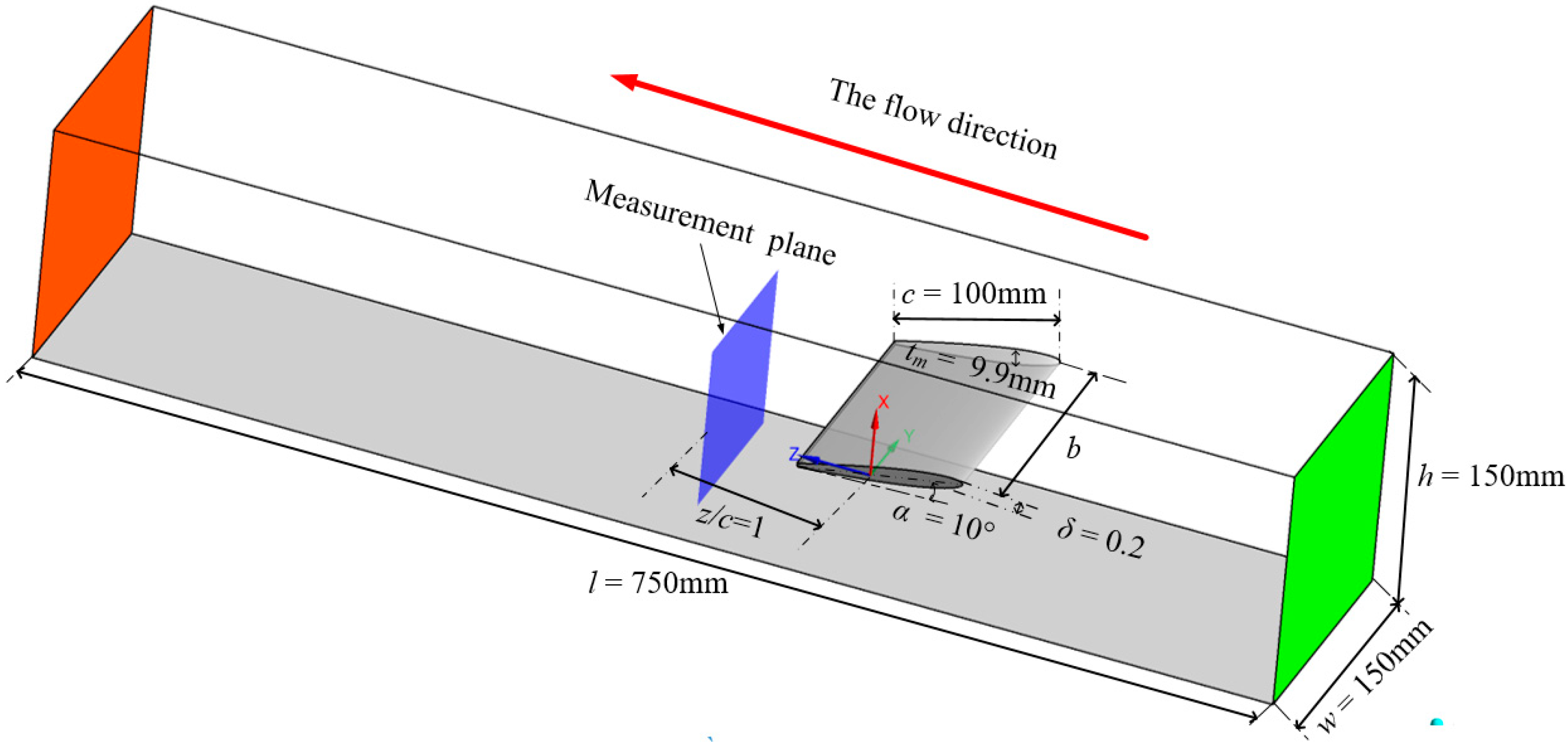
2. Research Object
2.1. Basic Physical Model of NACA0009 Hydrofoil
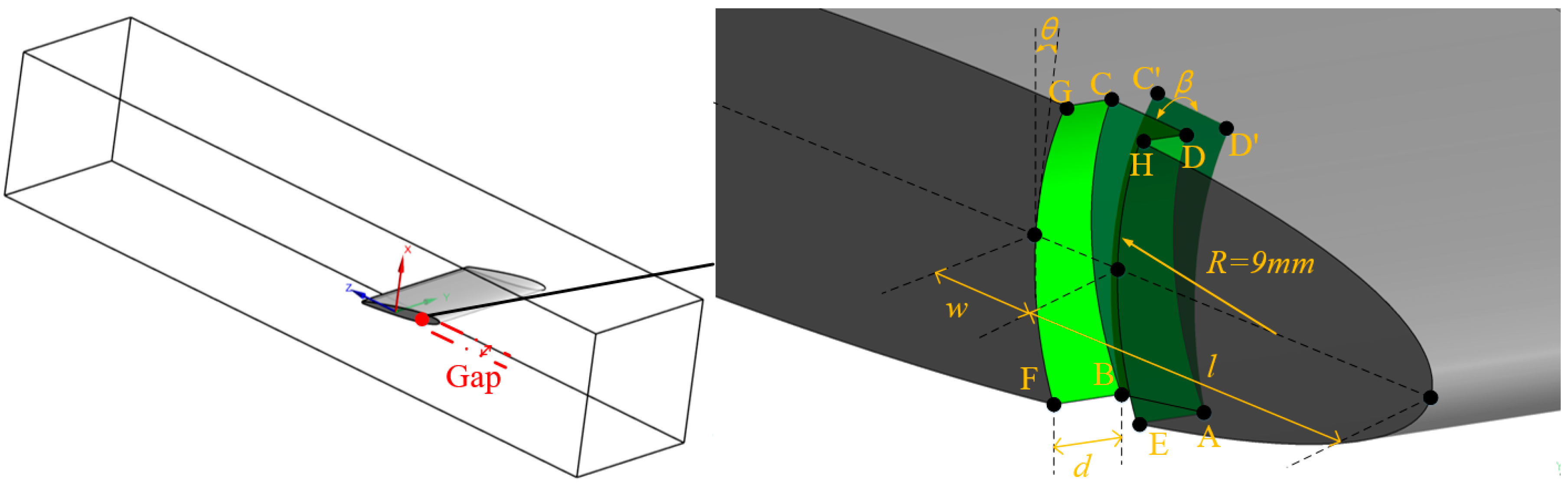
2.2. Parametric BSG
3. Optimization Design
3.1. Numerical Method
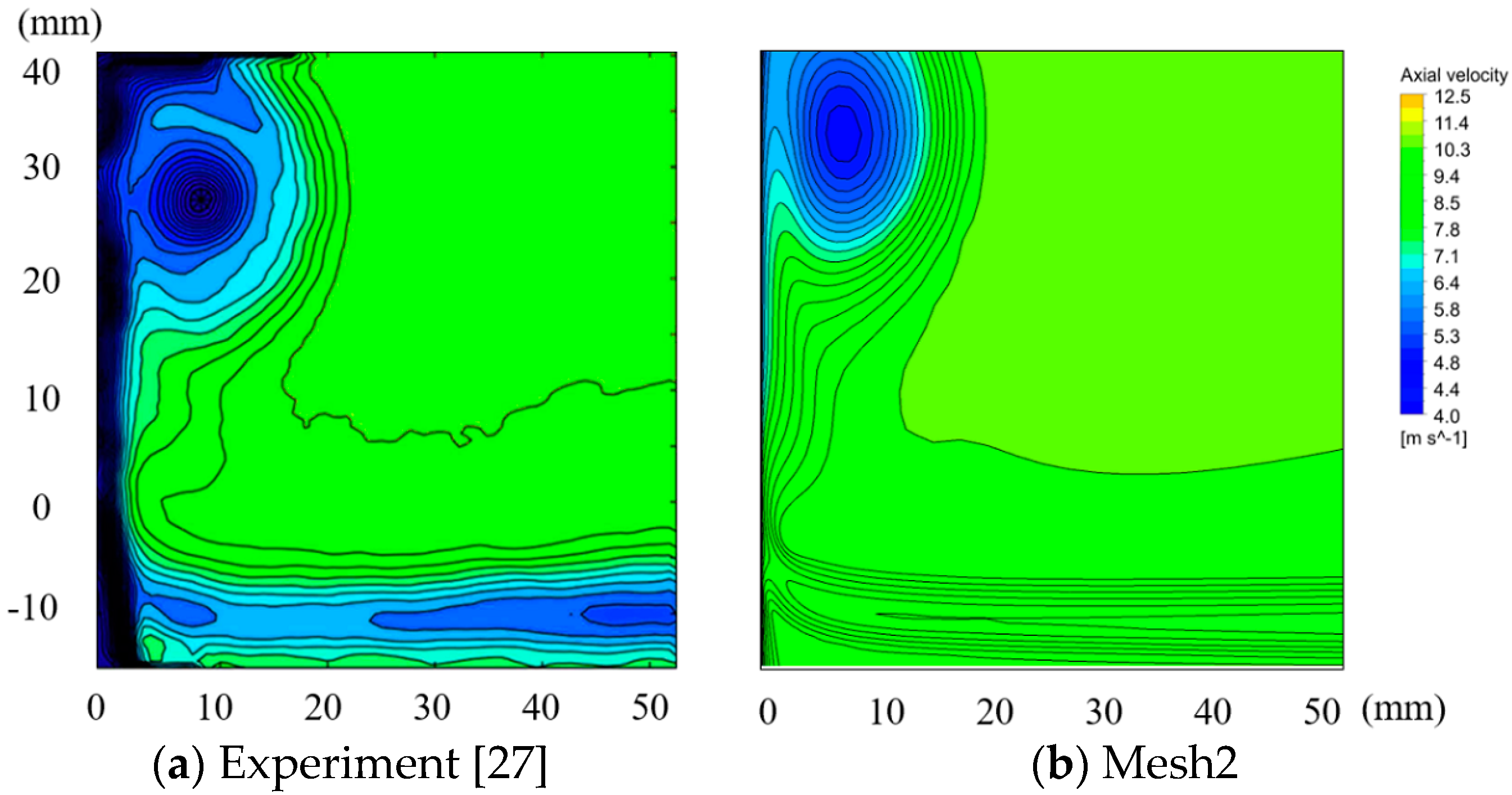
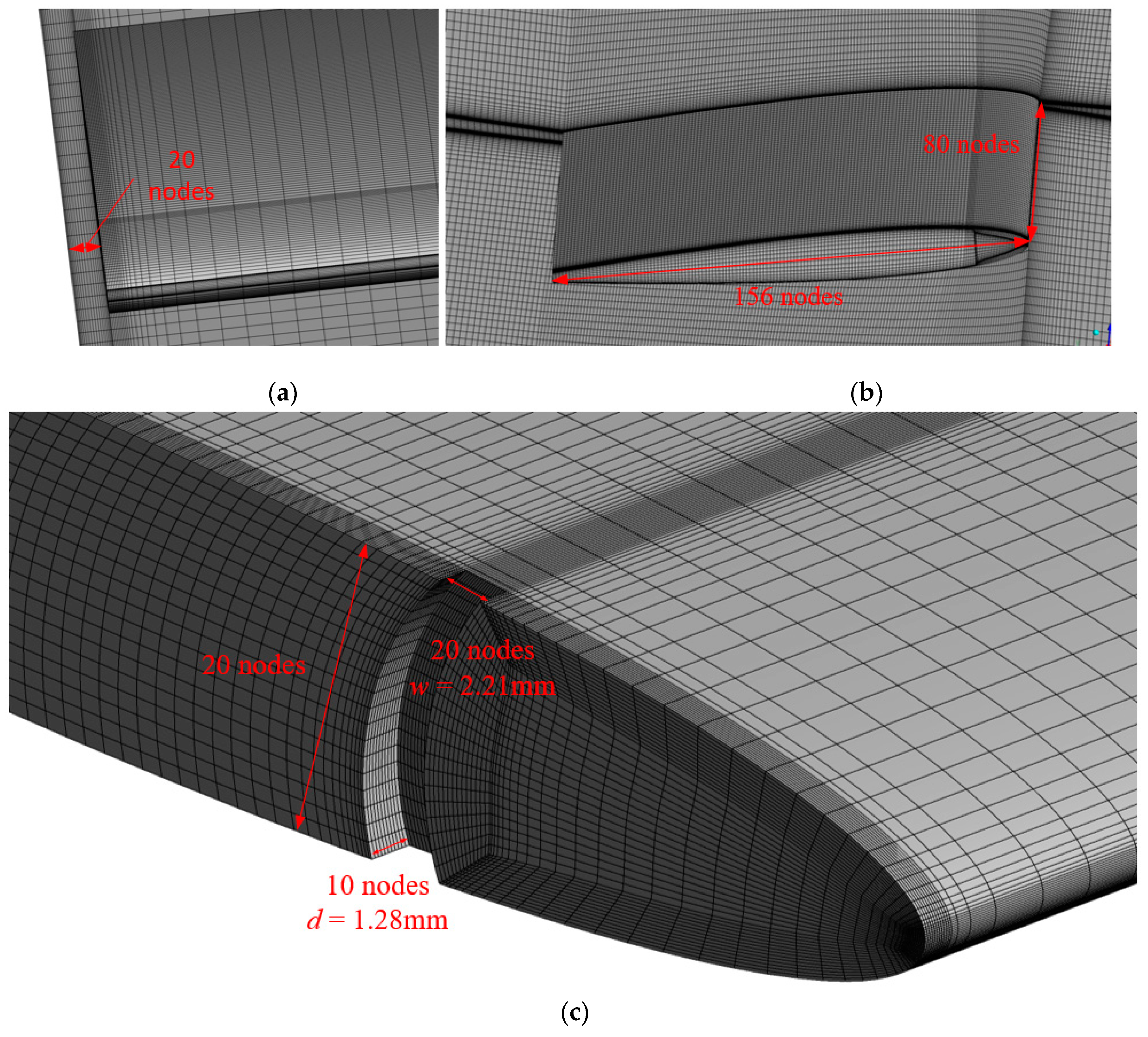
3.2. Grids Independence
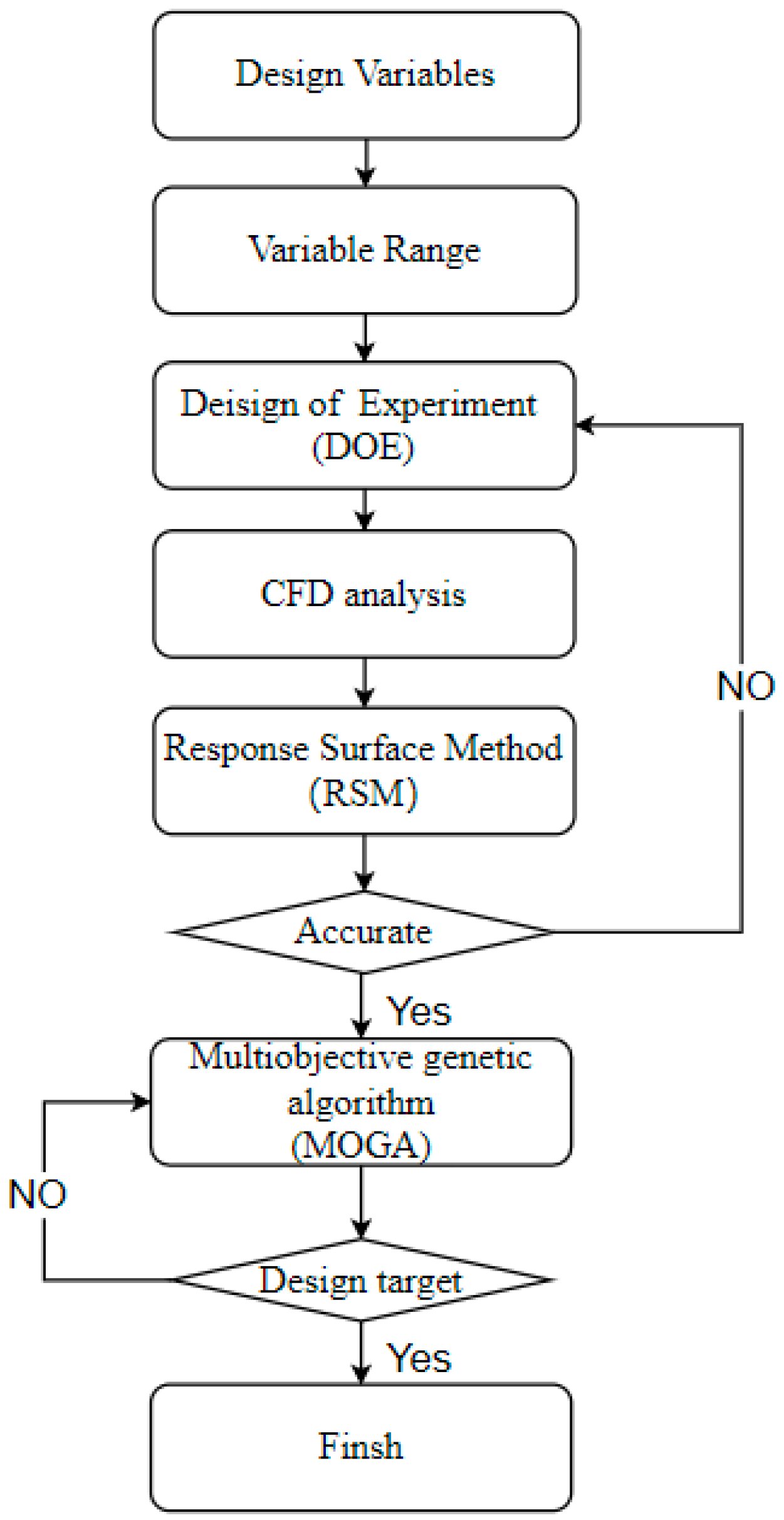
3.3. Optimization Design Method
4. Result and Discussion
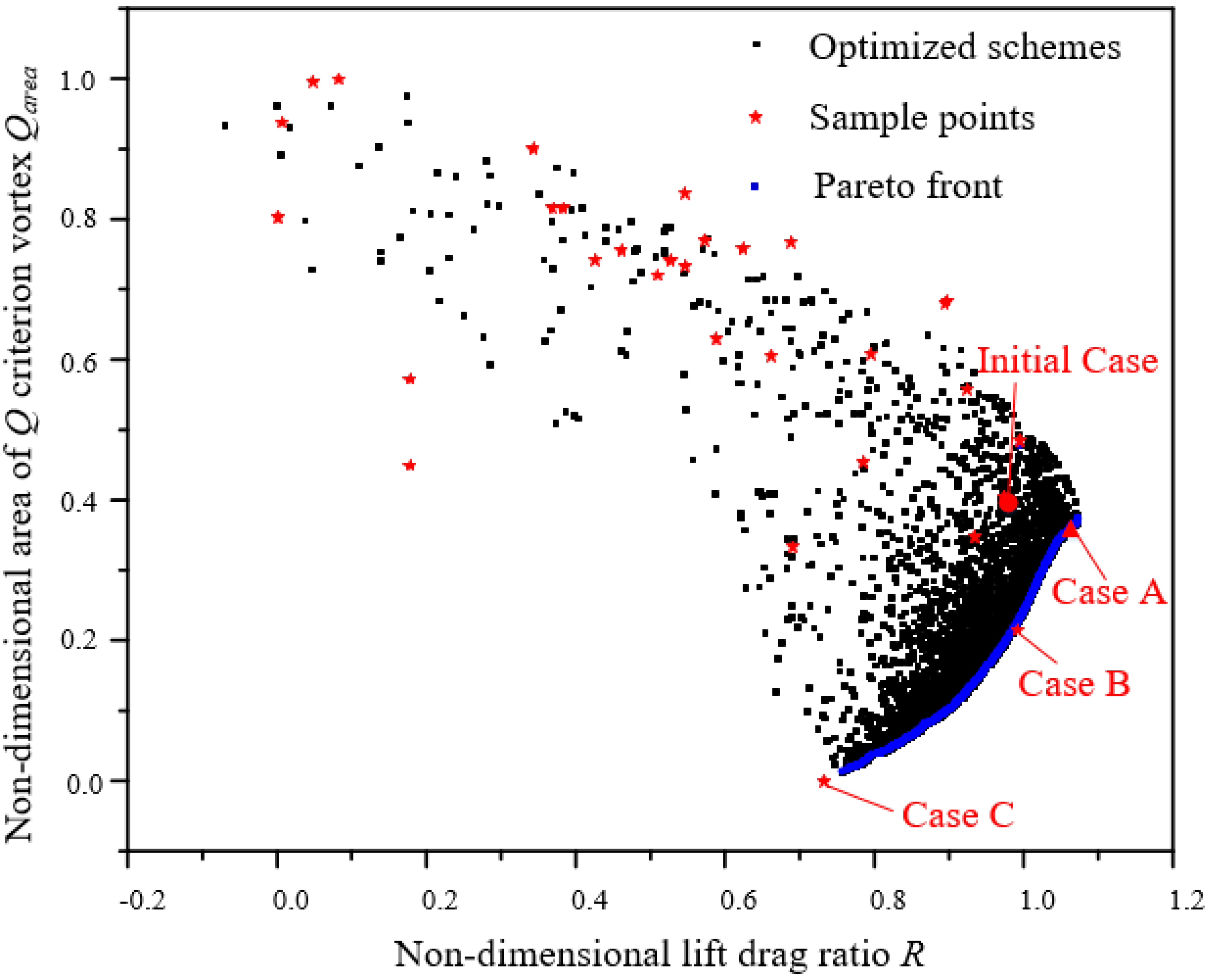
4.1. Optimization Results
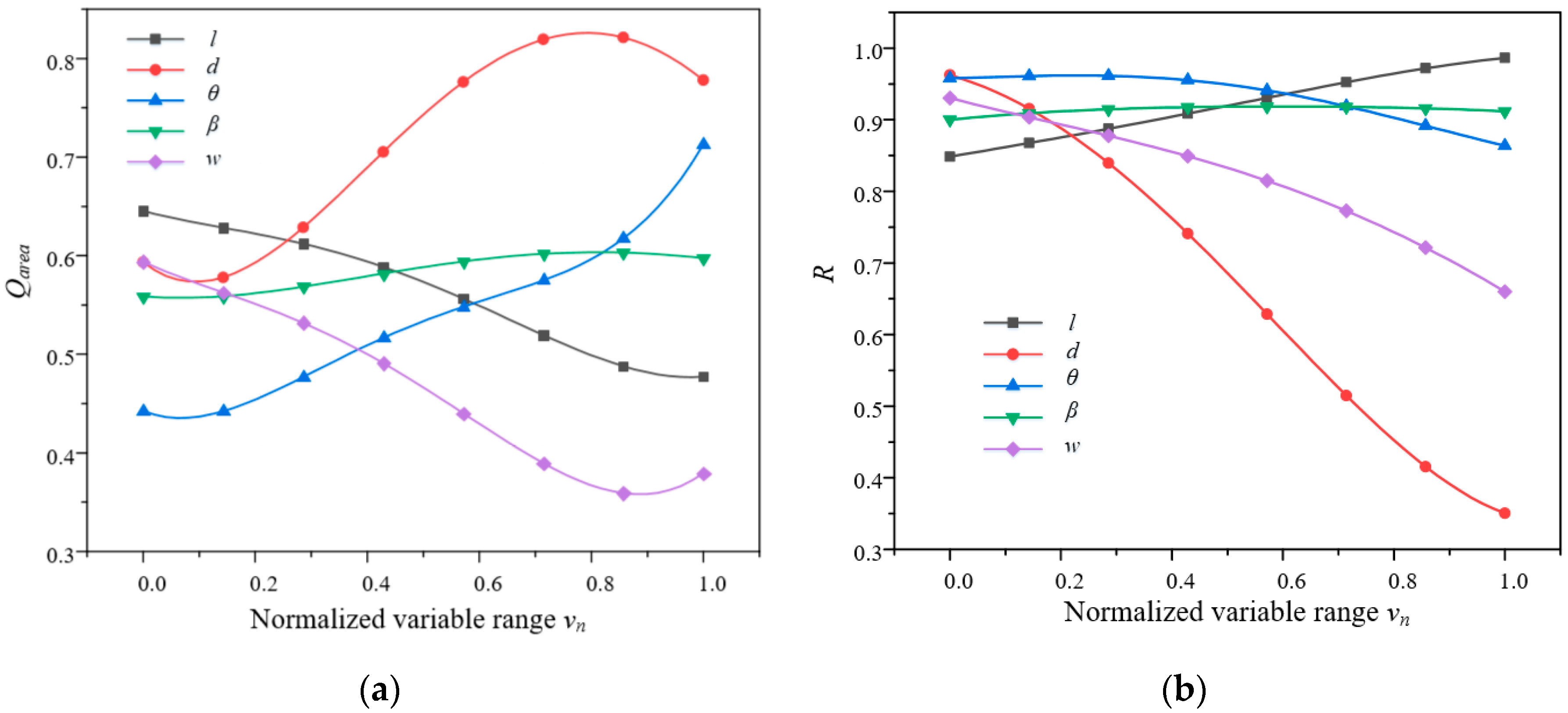
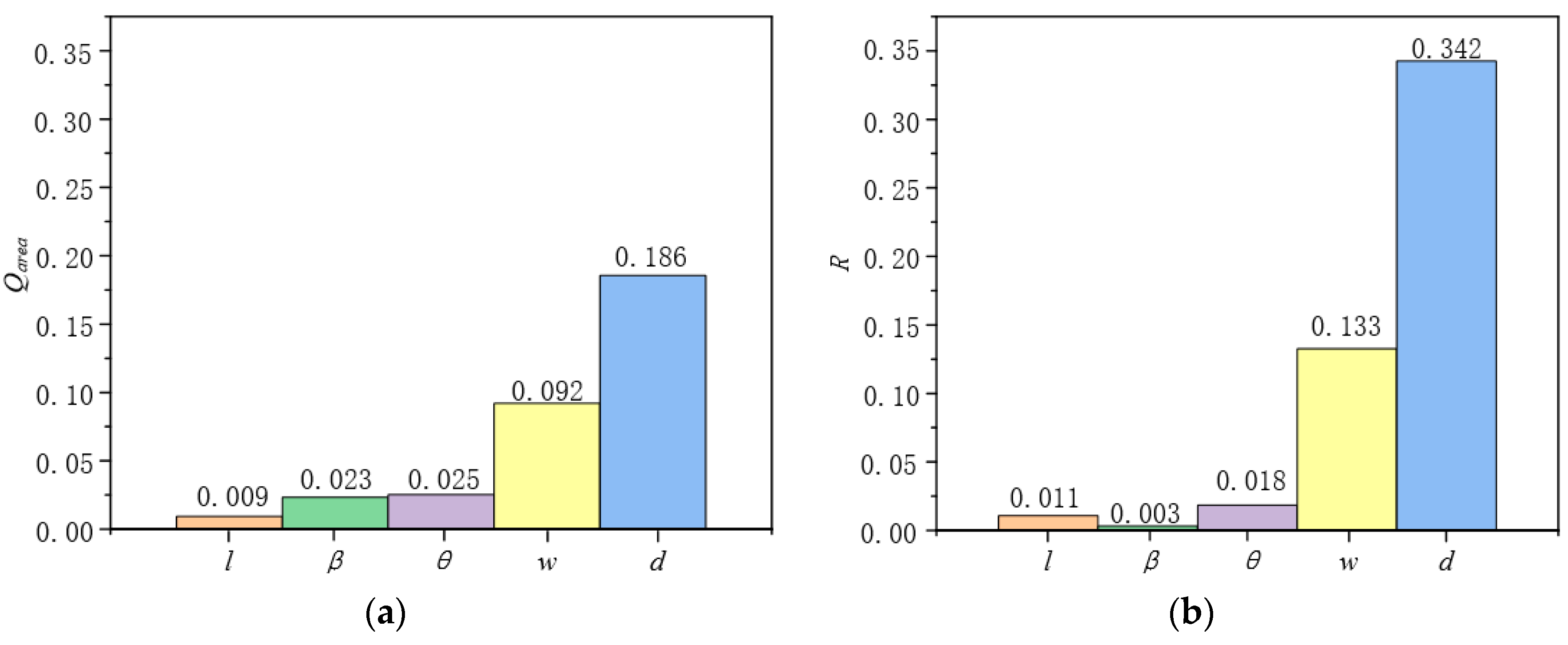
4.2. Sensitivity Analysis of Variables
4.3. Groove Suppress on TLV
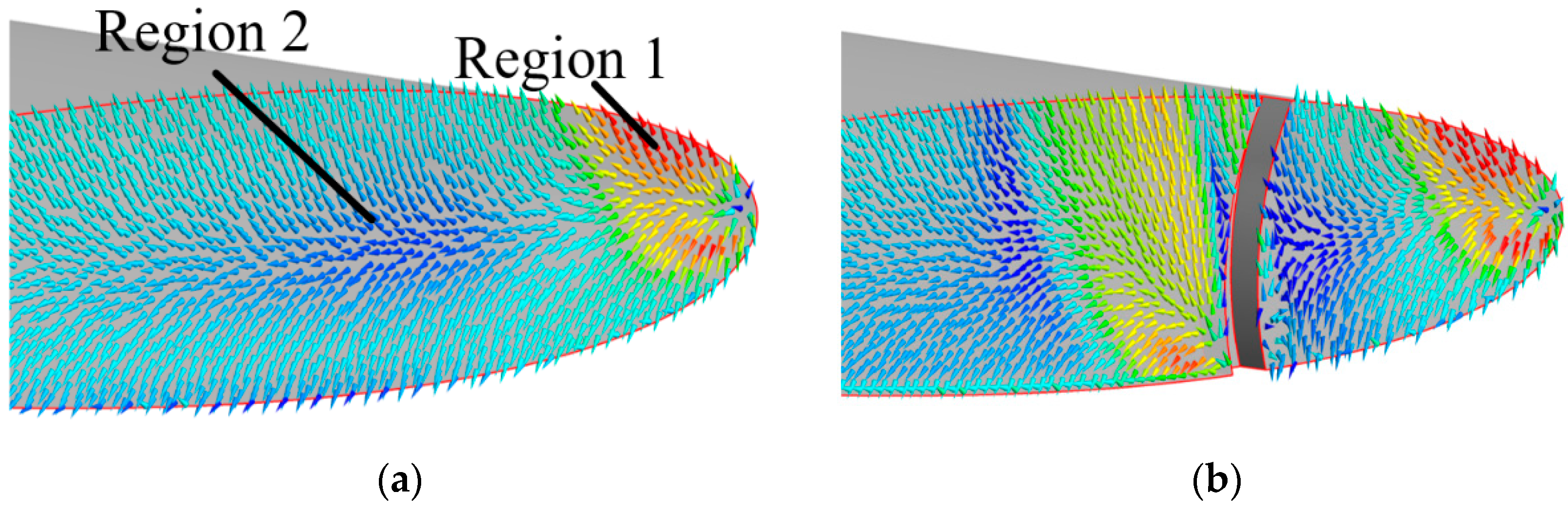
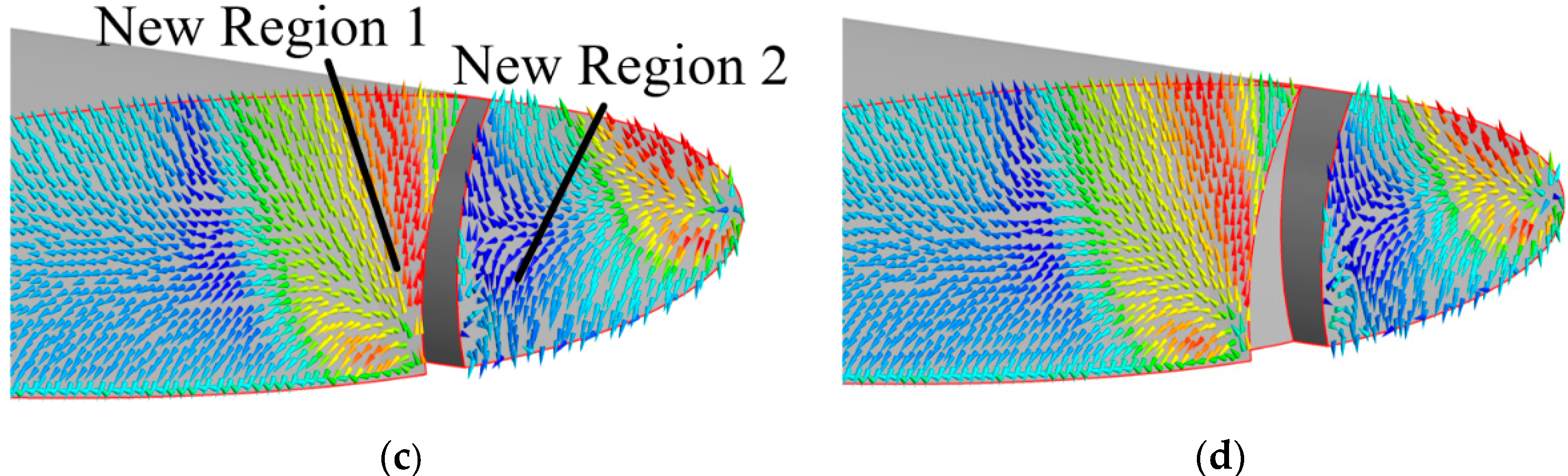
4.4. Groove Impact on the Flow Pattern
5. Conclusions
- (1)
- The preferred BSG foil, Case A, reduces the non-dimensional Q vortex isosurfaces area (Qarea = 2 × 107) by 5.14% and increases the lift drag ratio by 17.02%, compared to the initial case, proving that the present design strategy can be adapted to determine the preferred BSG configuration. However, some of the BSG configurations may cause performance deterioration. Therefore, the present BSG method should be adopted with caution.
- (2)
- According to the optimization result, when the present method is applied, a groove with a location far from the foil head, a tiny ration angle of θ, β, a 0.5 times gap size depth and an approximately gap size width is more contribute to improving the performance of the foil, as shown in Table 4.
- (3)
- Among the optimized parameters of BSG, groove depth d and groove width w have much more impact on foil performance. To match the optimization targets, the smaller groove depth is recommended. Besides, a wider groove width will significantly improve the lift drag ratio, but with energy loss.
- (4)
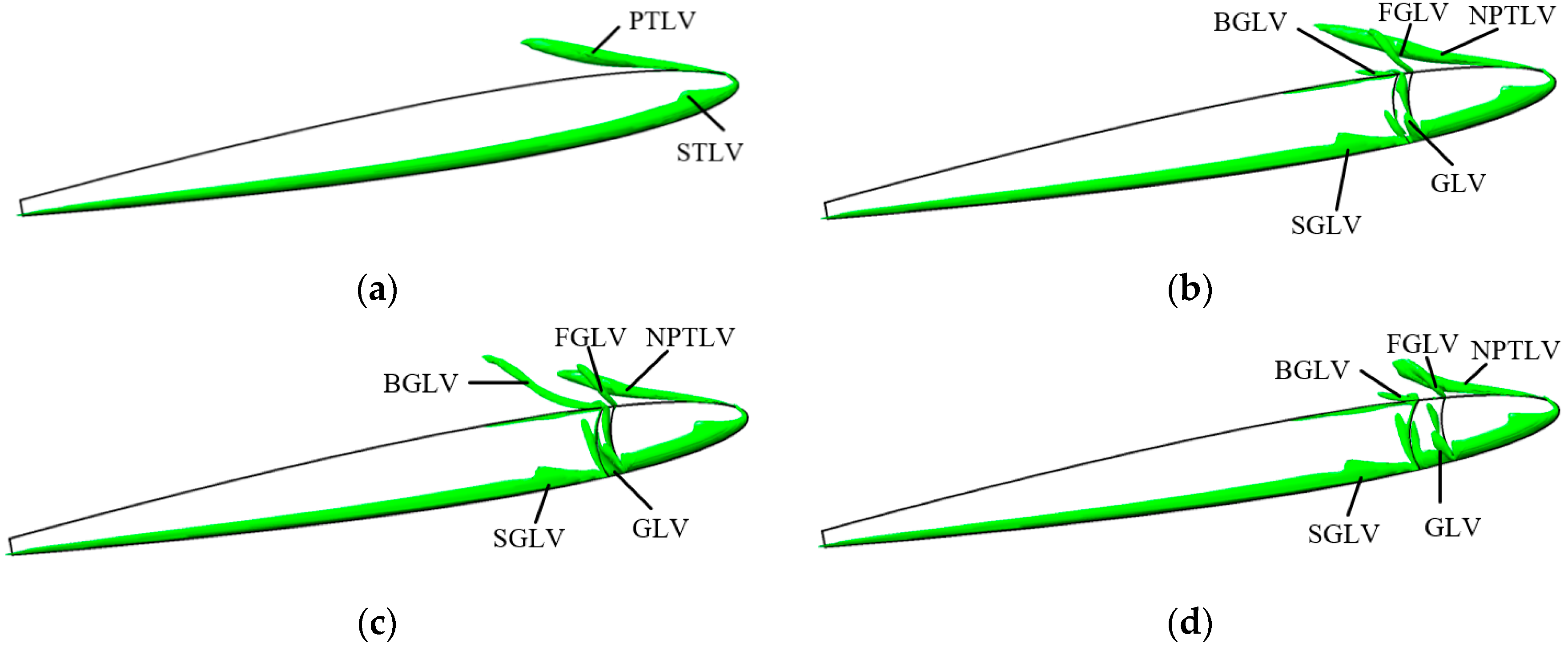
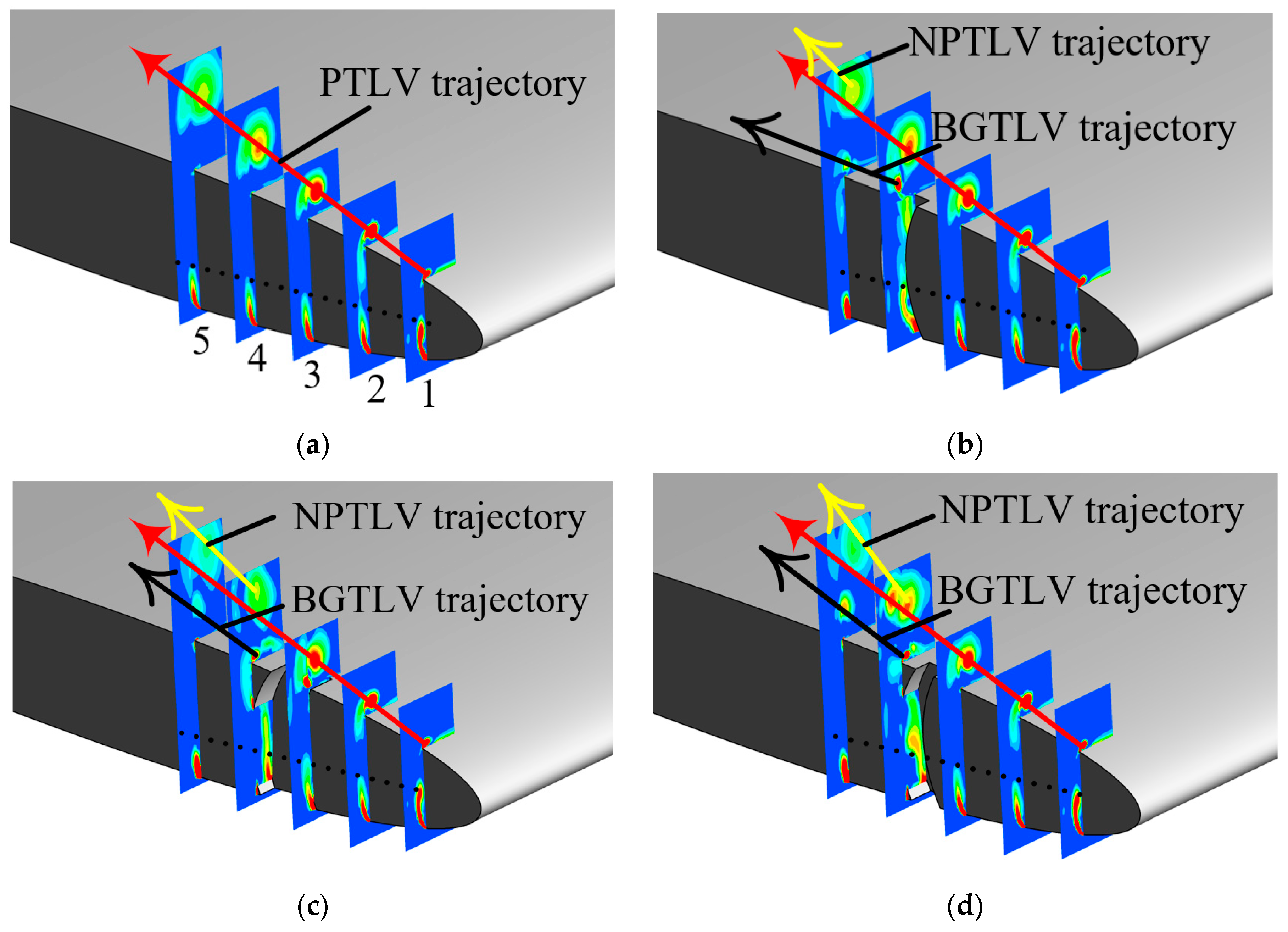
- The BSG can reduce the Q vortex area and slightly change the PTLV trajectory at the downstream. However, more vortex structure is produced, named GLV, BGLV, and FGLV, as shown in Figure 9.
- (5)
- The tip leakage flow is significantly affected by the BSG. Part of the leakage flow deposits in the groove first and then is divided into two parts. Part of the fluid overflows from the groove, and through the downstream blade tip into the primary stream. Another part of the fluid flows along the groove channel which further induces BGTV.
Author Contributions
Funding
Conflicts of Interest
References
- Taha, Z.; Sugiyono, T.M.Y.S.T.; Ya, T. Sawada, Numerical investigation on the performance of Wells turbine with non-uniform tip clearance for wave energy conversion. Appl. Ocean. Res. 2011, 33, 321–331. [Google Scholar] [CrossRef]
- Lu, Y.; Wang, Z.; Liu, X.; Xiao, Y.; Chen, C.; Wang, H.; Yan, J. Numerical prediction of pressure pulsation for a low head bidirectional tidal bulb turbine. Energy 2015, 89, 730–738. [Google Scholar] [CrossRef]
- Heo, M.W.; Kim, K.Y.; Kim, J.H.; Choi, Y.S. High-efficiency design of a mixed-flow pump using a surrogate model. J. Mech. Sci. Technol. 2016, 30, 541–547. [Google Scholar] [CrossRef]
- Zhang, W.W.; Yu, Z.Y.; Zhu, B.S. Characteristic analysis on the pressure fluctuation in the impeller of a low specific speed mixed flow pump. IOP Conf. Ser. Mater. Sci. Eng. 2016, 129, 12035. [Google Scholar] [CrossRef]
- Zhang, W.; Yu, Z.; Zhu, B. Influence of Tip Clearance on Pressure Fluctuation in Low Specific Speed Mixed-Flow Pump Passage. Energies 2017, 10, 148. [Google Scholar] [CrossRef]
- You, D.; Mittal, R.; Wang, M.; Moin, P. Large-eddy simulation of a rotor tip-clearance flow. Aiaa J. 2013. [Google Scholar] [CrossRef]
- Decaix, J.; Dreyer, M.; Balarac, G.; Farhat, M.; Münch, C. RANS computations of a confined cavitating tip-leakage vortex. Eur. J. Mech. B Fluids 2018, 67, 198–210. [Google Scholar] [CrossRef]
- Guo, T.; Zhao, S.; Liu, C. Study on flow characteristics and flow ripple reduction schemes of spool valves distributed radial piston pump. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2017, 231, 2291–2301. [Google Scholar] [CrossRef]
- Zhang, W.; Zhu, B.; Yu, Z.; Yang, C. Numerical study of pressure fluctuation in the whole flow passage of a low specific speed mixed-flow pump. Adv. Mech. Eng. 2017, 9, 168781401770765. [Google Scholar] [CrossRef]
- Experimental Study of the Effect of Blade Tip Clearance and Blade Angle Error on the Performance of Mixed-Flow Pump. SpringerLink. Available online: https://link.springer.com/article/10.1007/s11431-012-5080-6 (accessed on 7 August 2020).
- Hao, B.; Cao, S.L.; Chenglian, H.E.; Li, L.U. Experimental study of the effect of blade tip clearance and blade angle error on the performance of mixed-flow pump. Sci. China Technol. Sci. 2013, 56, 293–298. [Google Scholar] [CrossRef]
- Thakker, A.; Dhanasekaran, S.T. Computed effects of tip clearance on performance of impulse turbine for wave energy conversion. Renew. Energy 2004. [Google Scholar] [CrossRef]
- Kim, M.C.; Chun, H.H. Experimental Investigation into the performance of the Axial-Flow-Type Waterjet according to the Variation of Impeller Tip Clearance. Ocean Eng. 2007, 34, 275–283. [Google Scholar] [CrossRef]
- Inoue, M.; Kuroumaru, M.; Fukuhara, M. Behavior of Tip Leakage Flow behind an Axial Compressor Rotor. J. Eng. Gas. Turbines Power 1986, 108. [Google Scholar] [CrossRef]
- Lakshminarayana, B. Methods of Predicting the Tip Clearance Effects in Axial Flow Turbomachinery. J. Basic Eng. 1970, 92. [Google Scholar] [CrossRef]
- Miorini, R.L.; Wu, H.; Katz, J. The internal structure of the tip leakage vortex within the rotor of an axial waterjet pump. J. Turbomach. 2012, 134. [Google Scholar] [CrossRef]
- You, D.; Wang, M.; Moin, P.; Mittal, R. Study of tip-clearance flow in turbomachines using large-eddy simulation. Comput. Sci. Reng. 2004, 6. [Google Scholar] [CrossRef]
- You, D.; Wang, M.; Moin, P.; Mittal, R. Large-eddy simulation analysis of mechanisms for viscous losses in a turbomachinery tip-clearance flow. J. Fluid Mech. 2007, 586. [Google Scholar] [CrossRef]
- Guo, Q.; Zhou, L.; Wang, Z. Numerical evaluation of the clearance geometries effect on the flow field and performance of a hydrofoil. Renew. Energy 2016, 99. [Google Scholar] [CrossRef]
- Nho, Y.C.; Park, J.S.; Yong, J.S.; Kwak, J.S. Effects of turbine blade tip shape on total pressure loss and secondary flow of a linear turbine cascade. Int. J. Heat Fluid Flow 2012, 33. [Google Scholar] [CrossRef]
- Ye, X.; Zhang, J.; Li, C. Effect of blade tip pattern on performance of a twin-stage variable-pitch axial fan. Energy 2017. [Google Scholar] [CrossRef]
- Jung, Y.J.; Jeon, H.; Jung, Y.; Lee, K.J.; Choi, M. Effects of recessed blade tips on stall margin in a transonic axial compressor. Aerosp. Sci. Technol. 2016, 54. [Google Scholar] [CrossRef]
- Jung, Y.; Choi, M.; Park, J.Y.; Baek, J.H. Effects of recessed blade tips on the performance and flow field in a centrifugal compressor. J. Power Energy 2013, 227. [Google Scholar] [CrossRef]
- Xuemin, Y.; Pengmin, L.; Chunxi, L.; Xueliang, D. Numerical investigation of blade tip grooving effect on performance and dynamics of an axial flow fan. Energy 2015. [Google Scholar] [CrossRef]
- Zhao, J.L.; Wang, H.J.; Gong, R.Z.; Xuan, T.; Li, X.Y. Vortex structure research method based on Q-criterion in a high-speed centrifugal pump. In Proceedings of the 2014 ISFMFE-6th International Symposium on Fluid Machinery and Fluid Engineering, Wuhan, China, 22 October 2014. [Google Scholar] [CrossRef]
- Kolá, V.; Istek, J. Corotational and Compressibility Aspects Leading to a Modification of the Vortex-Identification Q-Criterion. Aiaa J. 2015, 53. [Google Scholar] [CrossRef]
- Dreyer, M.; Decaix, J.; Münch-Alligné, C.; Farhat, M. Mind the gap: A new insight into the tip leakage vortex using stereo-PIV. Exp. Fluids 2014, 55. [Google Scholar] [CrossRef]
- Li, D.; Wang, H.; Li, Z.; Nielsen, T.K.; Goyal, R.; Wei, X.; Qin, D. Transient characteristics during the closure of guide vanes in a pump-turbine in pump mode. Renew. Energy 2018, 118. [Google Scholar] [CrossRef]
- Huang, Z.; Huang, Z.; Fan, H. Influence of C groove on energy performance and noise source of a NACA0009 hydrofoil with tip clearance. Renew. Energy 2020, 159. [Google Scholar] [CrossRef]
- Ismail, C.; Urmila, B. Procedure for Estimation and Reporting of Uncertainty Due to Discretization in CFD Applications. J. Fluids Eng. 2008. [Google Scholar] [CrossRef]
- Liu, L.; Zhu, B.; Li, B.; Liu, X.; Zhao, Y. Parametric Design of an Ultrahigh-Head Pump-Turbine Runner Based on Multiobjective Optimization. Energies 2017, 10, 169. [Google Scholar] [CrossRef]

| Mesh | Mesh Nodes | CL | CD |
|---|---|---|---|
| Mesh1 | 3890724 | 1.232 | 0.0454 |
| Mesh2 | 4911942 | 1.236 | 0.0456 |
| Mesh3 | 5824564 | 1.241 | 0.0458 |
| Mesh | Mesh Nodes | CL | CD |
|---|---|---|---|
| G-Mesh1 | 5613162 | 1.2396 | 0.0459 |
| G-Mesh2 | 5683407 | 1.2395 | 0.0459 |
| G-Mesh3 | 5754192 | 1.2392 | 0.0459 |
| Range | d [mm] | l [mm] | θ [°] | β [°] | w [mm] |
|---|---|---|---|---|---|
| Lower bound | 1 (0.5δ) | 5 (0.05c) | 0 | −5 | 2 |
| Upper bound | 3 (1.5δ) | 20 (0.2c) | 10 | 5 | 5 |
| Case | Design Parameters | Qarea | R | ||||||
|---|---|---|---|---|---|---|---|---|---|
| d [mm] | l [mm] | θ [°] | β [°] | w [mm] | RSM | CFD | RSM | CFD | |
| A | 1.00 | 19.91 | 1.08 | −0.93 | 2.13 | 0.36 | 0.37 | 1.06 | 1.10 |
| B | 1.76 | 18.45 | 5.17 | 3.28 | 2.10 | - | 0.22 | - | 1.00 |
| C | 1.35 | 15.86 | 8.28 | −4.31 | 4.48 | - | 0.00 | - | 0.73 |
| Initial Case | - | - | - | - | - | - | 0.39 | - | 0.94 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hu, Z.; Huang, C.; Huang, Z.; Zhang, J. A Method of Bending Shrinkage Groove on Vortex Suppression and Energy Improvement for a Hydrofoil with Tip Gap. Processes 2020, 8, 1299. https://doi.org/10.3390/pr8101299
Hu Z, Huang C, Huang Z, Zhang J. A Method of Bending Shrinkage Groove on Vortex Suppression and Energy Improvement for a Hydrofoil with Tip Gap. Processes. 2020; 8(10):1299. https://doi.org/10.3390/pr8101299
Chicago/Turabian StyleHu, Zanao, Chuibing Huang, Zhenwei Huang, and Jinsong Zhang. 2020. "A Method of Bending Shrinkage Groove on Vortex Suppression and Energy Improvement for a Hydrofoil with Tip Gap" Processes 8, no. 10: 1299. https://doi.org/10.3390/pr8101299
APA StyleHu, Z., Huang, C., Huang, Z., & Zhang, J. (2020). A Method of Bending Shrinkage Groove on Vortex Suppression and Energy Improvement for a Hydrofoil with Tip Gap. Processes, 8(10), 1299. https://doi.org/10.3390/pr8101299





