Principal Component Analysis of Blast Furnace Drainage Patterns
Abstract
1. Introduction
2. Principal Component Analysis
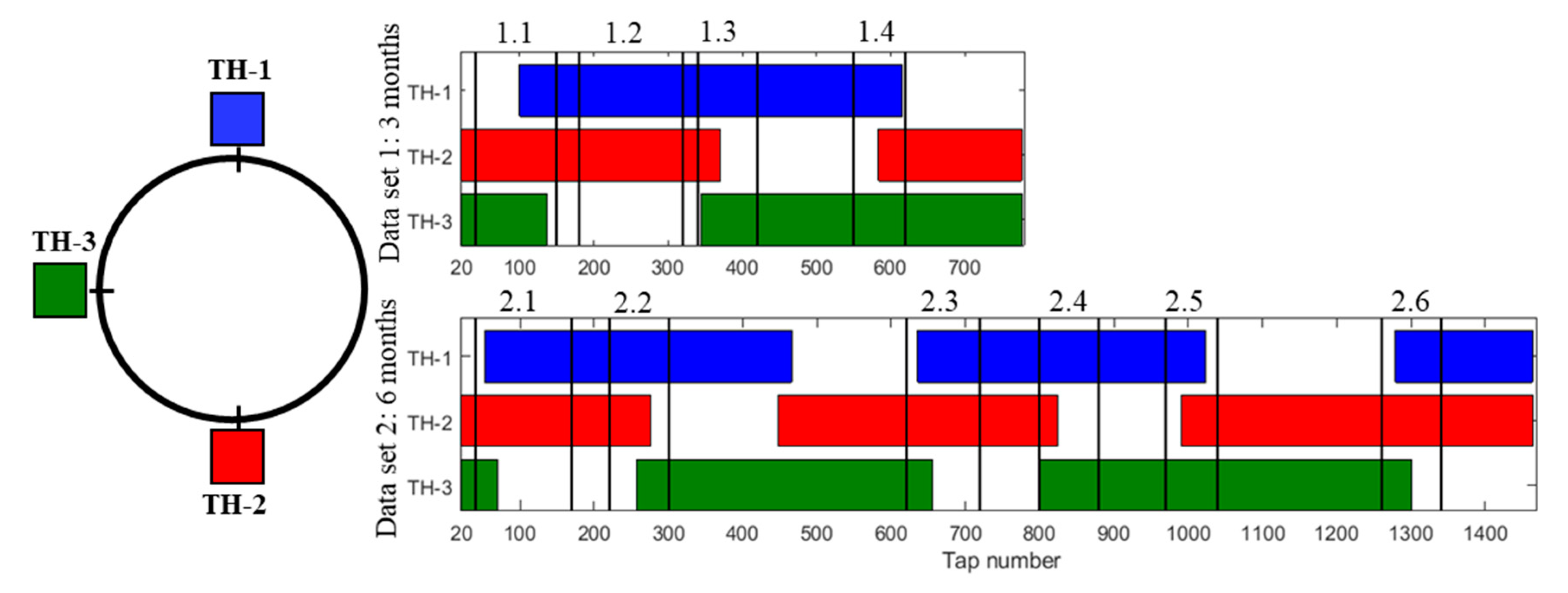
3. Method and Data Sets
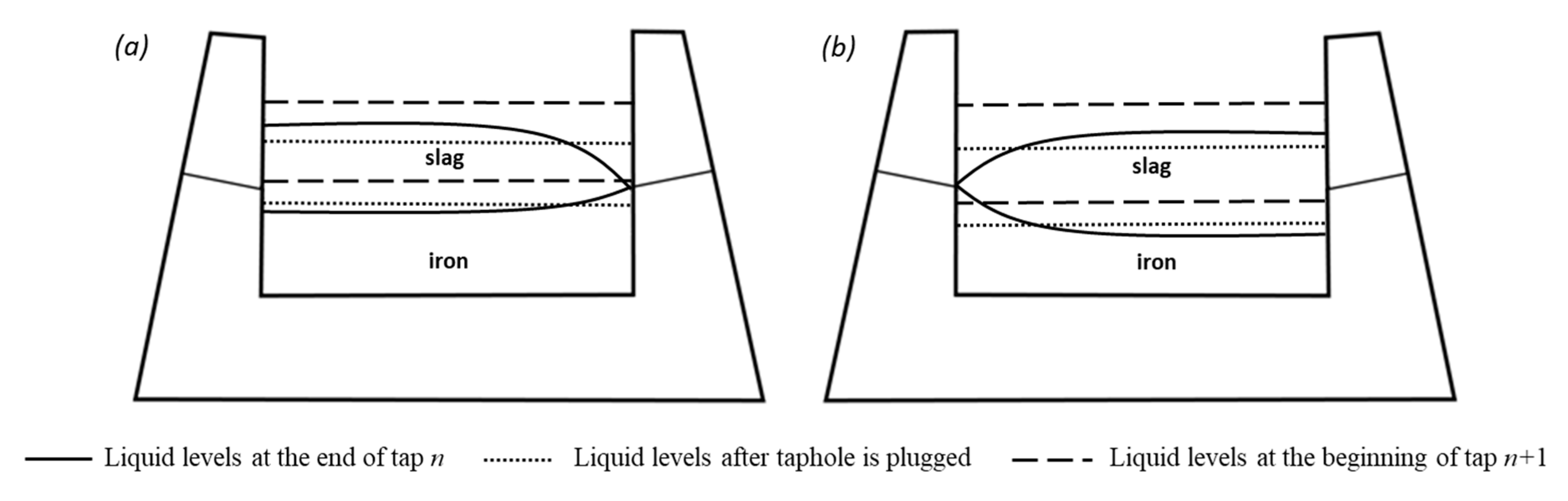
3.1. Pre-Processing of the Data Set
- outflow near zero for any of the phases;
- taps shorter than 40 min that may occur as a result of disturbances, stoppages, or contingencies;
- any of the phases shows an outflow rate above 2% of the production rate at all times (i.e., also during the intercast period, where there should be no liquid outflows); and
- the total outflow abruptly goes near zero as a result of measurement errors or possibly taphole clogging.
3.2. Post-Processing of the Results
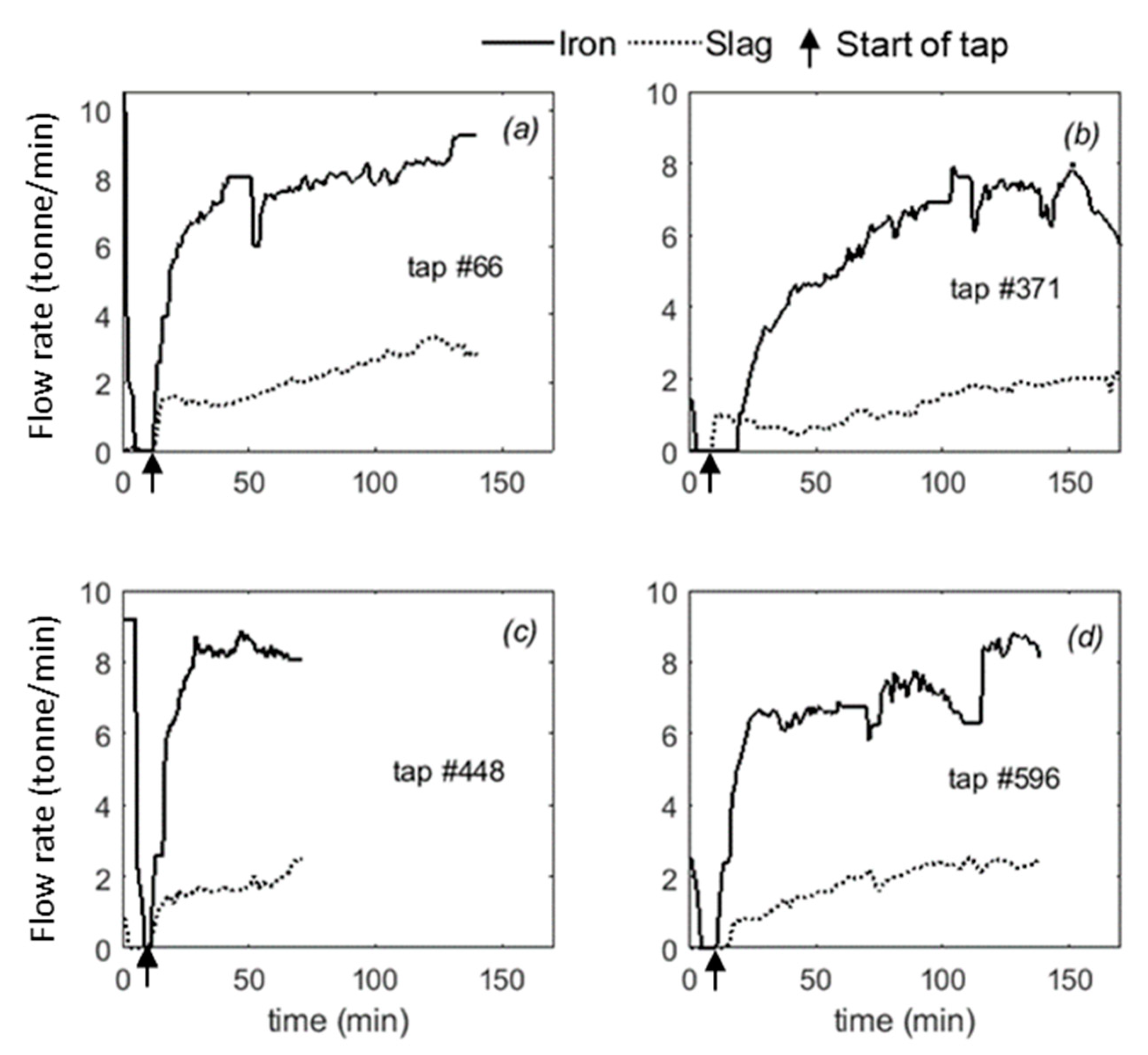
4. Results
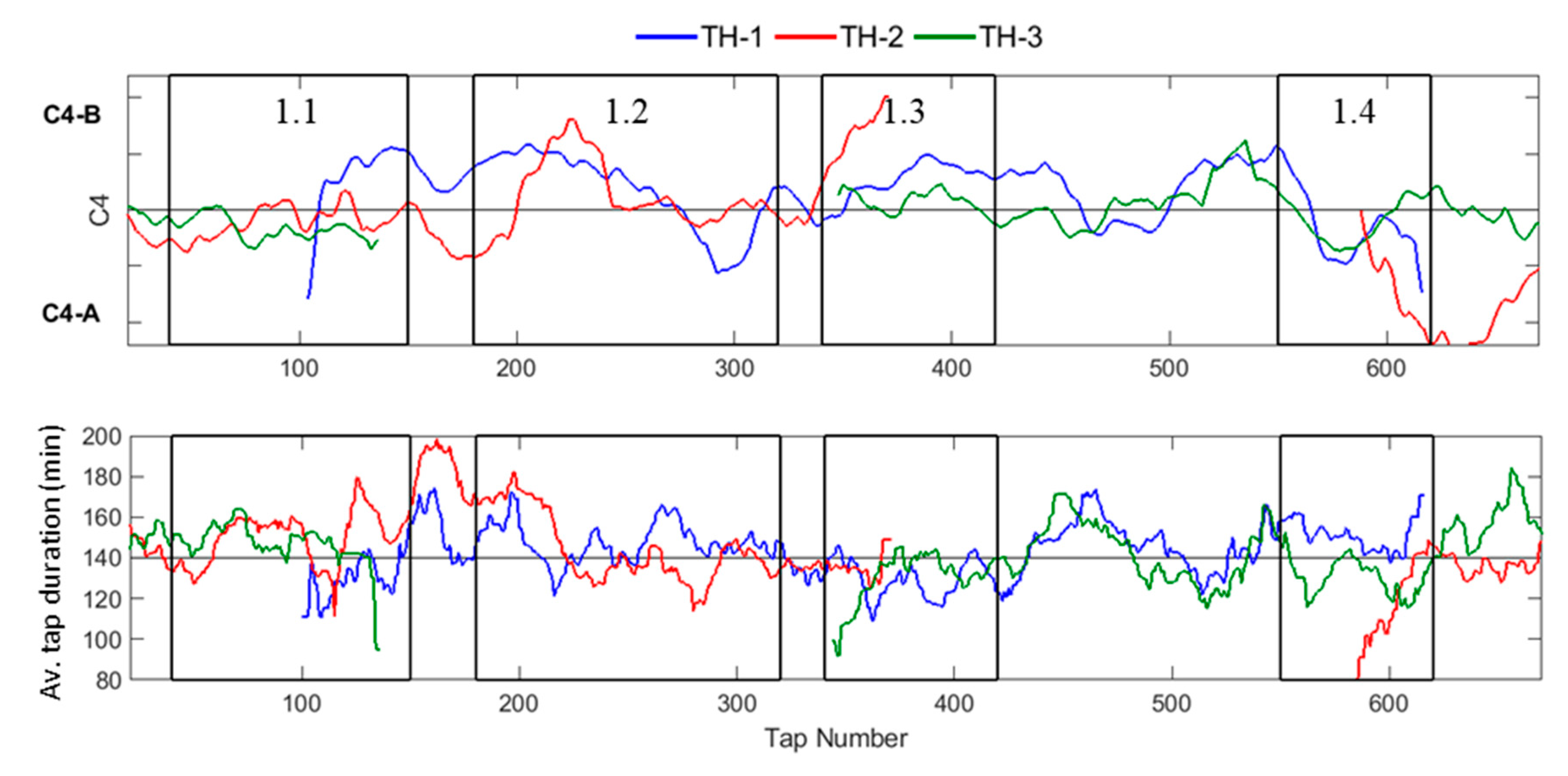
4.1. Data Set 1
4.1.1. Period 1.1
4.1.2. Period 1.2
4.1.3. Period 1.3
4.1.4. Period 1.4
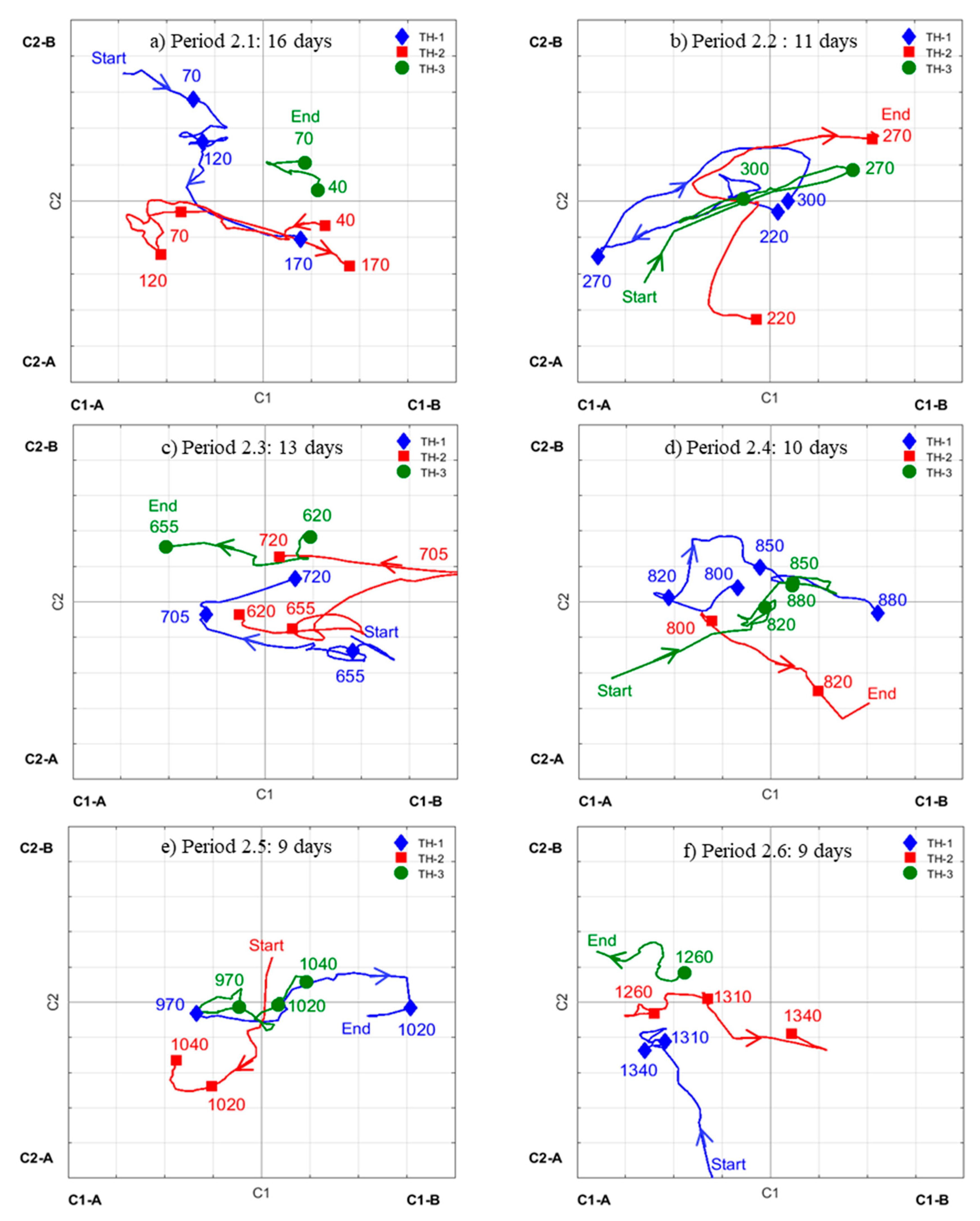
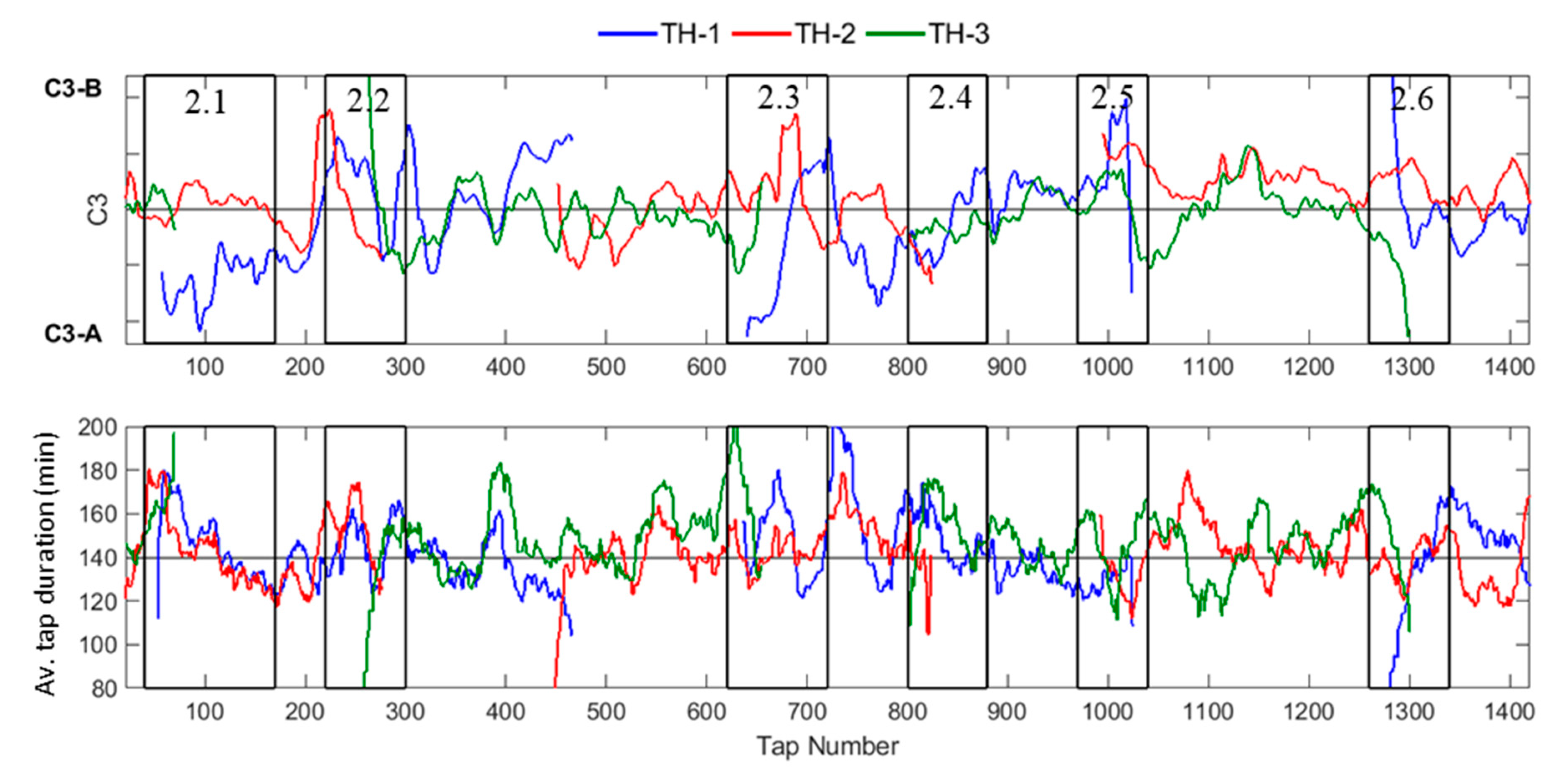
4.2. Data Set 2
4.2.1. Period 2.1
4.2.2. Period 2.2
4.2.3. Period 2.3
4.2.4. Period 2.4
4.2.5. Periods 2.5 and 2.6
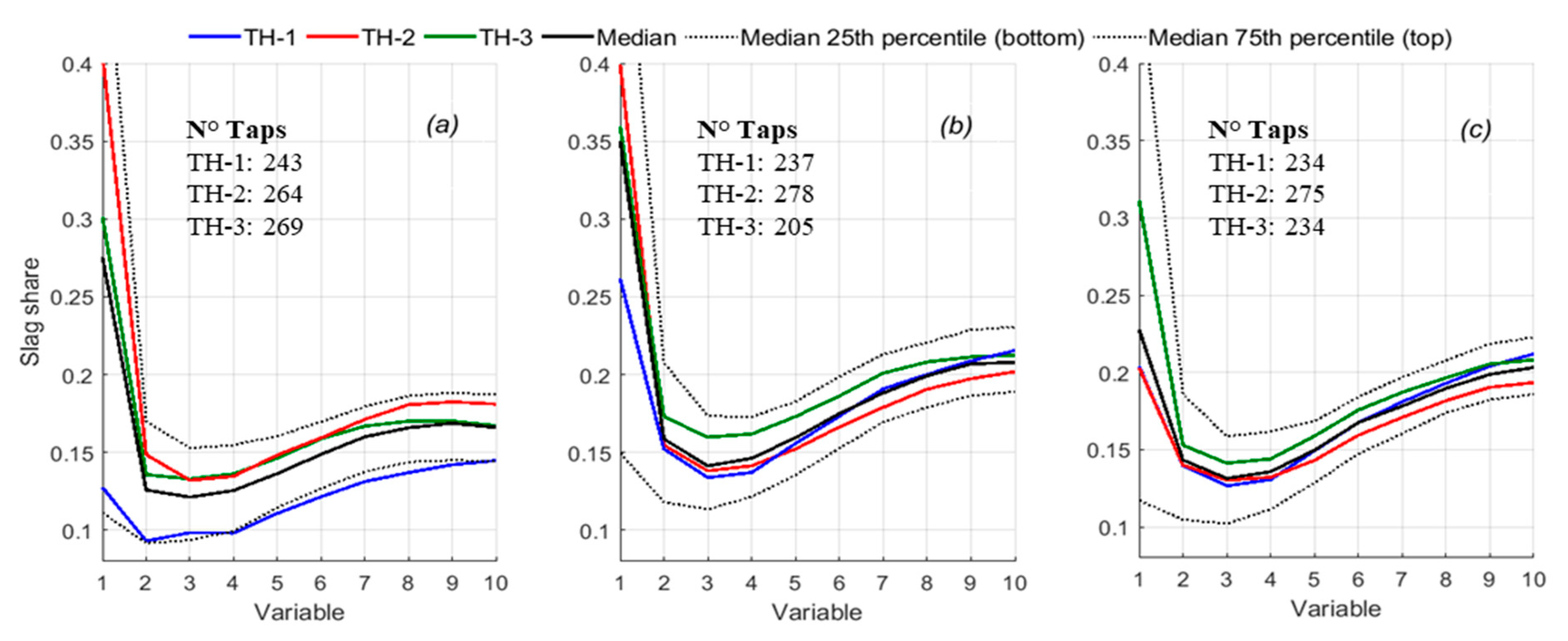
5. Results of PCA and Liquid Level Model
6. Summary of the Analysis
7. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Iida, M.; Ogura, K.; Hakone, T. Numerical Study on Metal/Slag Drainage Rate Deviation during Blast Furnace Tapping. ISIJ Int. 2009, 49, 1123–1132. [Google Scholar] [CrossRef]
- Iida, M.; Ogura, K.; Hakone, T. Analysis of Drainage Rate Variation of Molten Iron and Slag from Blast Furnace during Tapping. ISIJ Int. 2008, 48, 412–419. [Google Scholar] [CrossRef]
- Nouchi, T.; Sato, M.; Takeda, K.; Ariyama, T. Effects of Operation Condition and Casting Strategy on Drainage Efficiency of the Blast Furnace Hearth. ISIJ Int. 2005, 45, 1515–1520. [Google Scholar] [CrossRef][Green Version]
- Roche, M.; Helle, M.; van der Stel, J.; Louwerse, G.; Shao, L.; Saxén, H. On-Line Estimation of Liquid Levels in the Blast Furnace Hearth. Steel Res. Int. 2019, 90, 1800420. [Google Scholar] [CrossRef]
- Tsuchiya, N.; Fukutake, T.; Yamauchi, Y.; Matsumoto, T. In-furnace Conditions as Prerequisites for Proper Use and Design of Mud to Control Blast Furnace Taphole Length. ISIJ Int. 1998, 38, 116–125. [Google Scholar] [CrossRef]
- Zhao, Y.; Fu, D.; Lherbier, L.W.; Chen, Y.; Zhou, C.Q.; Grindey, J.G. Investigation of Skull Formation in a Blast Furnace Hearth. Steel Res. Int. 2014, 85, 891–901. [Google Scholar] [CrossRef]
- Takatani, K.; Inada, T.; Takata, K. Mathematical Model for Transient Erosion Process of Blast Furnace Hearth. ISIJ Int. 2001, 41, 1139–1145. [Google Scholar] [CrossRef]
- Panjkovic, V.; Truelove, J.S.; Zulli, P. Numerical modelling of iron flow and heat transfer in blast furnace hearth. Ironmak. Steelmak. 2002, 29, 390–400. [Google Scholar] [CrossRef]
- Tanzil, W.B.U.; Zulli, P.; Burgess, J.M.; Pinczewski, W.V. Experimental Model Study of the Physical Mechanisms Governing Blast Furnace Hearth Drainage. Trans. Iron Steel Inst. Jpn. 1984, 24, 197–205. [Google Scholar] [CrossRef]
- Nishioka, K.; Maeda, T.; Shimizu, M. A Three-dimensional Mathematical Modelling of Drainage Behavior in Blast Furnace Hearth. ISIJ Int. 2005, 45, 669–676. [Google Scholar] [CrossRef]
- Agrawal, A.; Kor, S.C.; Nandy, U.; Choudhary, A.R.; Tripathi, V.R. Real-time blast furnace hearth liquid level monitoring system. Ironmak. Steelmak. 2016, 43, 550–558. [Google Scholar] [CrossRef]
- Saxén, H. Model of Draining of the Blast Furnace Hearth with an Impermeable Zone. Metall. Mater. Trans. B 2015, 46, 421–431. [Google Scholar] [CrossRef]
- Saxén, H.; Brännbacka, J. Dynamic model of liquid levels in the blast furnace hearth. Scand. J. Metall. 2005, 34, 116–121. [Google Scholar] [CrossRef]
- Roche, M.; Helle, M.; van der Stel, J.; Louwerse, G.; Shao, L.; Saxén, H. Off-line Model of Blast Furnace Liquid Levels. ISIJ Int. 2018, 58, 2236–2245. [Google Scholar] [CrossRef]
- Bartholomew, D.J. Principal Components Analysis. Int. Encycl. Educ. (Third Ed.) 2010, 374–377. [Google Scholar] [CrossRef]
- Kourti, T. 4.02 Multivariate Statistical Process Control and Process Control, Using Latent Variables. Compr. Chemom. 2009, 21–54. [Google Scholar] [CrossRef]
- Helle, M.; Roche, M.; Saxén, H. On-line estimation of liquid levels and local drainage characteristics in the blast furnace. In Proceedings of the SteelSim, Toronto, ON, Canada, 13–15 August 2019. paper 24. [Google Scholar]
- Hu, X.; Sundqvist Ökvist, L.; Ölund, M. Materials Properties and Liquid Flow in the Hearth of the Experimental Blast Furnace. Metals 2019, 9, 527. [Google Scholar] [CrossRef]
- Nishioka, K.; Maeda, T.; Shimizu, M. Effect of Various In-furnace Conditions on Blast Furnace Hearth Drainage. ISIJ Int. 2005, 45, 1496–1505. [Google Scholar] [CrossRef]
- Fukutake, T.; Okabe, K. Experimental Studies of Slag Flow in the Blast Furnace Hearth During Tapping Operation. Trans. Iron Steel Inst. Jpn. 1976, 16, 309–316. [Google Scholar]
- Fukutake, T.; Okabe, K. Influences of Slag Tapping Conditions on the Amount of Residual Slag in the Blast Furnace Hearth. Trans. Iron Steel Inst. Jpn. 1976, 16, 317–323. [Google Scholar]
- Zulli, P. Blast Furnace Hearth Drainage with and without a Coke Free Layer. Doctoral Dissertation, Faculty of Engineering, UNSW, Sydney, Australia, 1991. [Google Scholar]



© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Roche, M.; Helle, M.; Saxén, H. Principal Component Analysis of Blast Furnace Drainage Patterns. Processes 2019, 7, 519. https://doi.org/10.3390/pr7080519
Roche M, Helle M, Saxén H. Principal Component Analysis of Blast Furnace Drainage Patterns. Processes. 2019; 7(8):519. https://doi.org/10.3390/pr7080519
Chicago/Turabian StyleRoche, Mauricio, Mikko Helle, and Henrik Saxén. 2019. "Principal Component Analysis of Blast Furnace Drainage Patterns" Processes 7, no. 8: 519. https://doi.org/10.3390/pr7080519
APA StyleRoche, M., Helle, M., & Saxén, H. (2019). Principal Component Analysis of Blast Furnace Drainage Patterns. Processes, 7(8), 519. https://doi.org/10.3390/pr7080519





