Next Article in Journal
Study on Risk Mitigation Measures for Atmospheric Storage Tank of Acrylic Acid Due to Abnormal Weather Conditions
Previous Article in Journal
Comparative Analysis of Sulfuric Acid Alkylation Technologies Based on a Reaction Kinetic Model
Previous Article in Special Issue
Effects of the PMMA Molecular Weight on the Thermal and Thermo-Oxidative Decomposition as the First Chemical Stage of Flaming Ignition
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Processes
Volume 13
Issue 5
10.3390/pr13051606
processes-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

Thermal Characterization of Structured Porous Materials and Phase Change Composites for Heat Sink Applications

by
Abhishek Agarwal
1,*,
Michel Kalenga Wa Kalenga
2 and
Masengo Ilunga
3
1
Department of Mechanical Engineering, College of Science and Technology, Royal University of Bhutan, Phuentsholing 21101, Bhutan
2
Department of Metallurgy, School of Mining, Metallurgy and Chemical Engineering, Faculty of Engineering and the Built Environment, University of Johannesburg, P.O. Box 17001, Johannesburg 2028, South Africa
3
Department of Civil Engineering, University of South Africa, Pretoria 1709, South Africa
*
Author to whom correspondence should be addressed.
Processes 2025, 13(5), 1606; https://doi.org/10.3390/pr13051606
Submission received: 2 April 2025 / Revised: 15 May 2025 / Accepted: 19 May 2025 / Published: 21 May 2025
(This article belongs to the Special Issue High-Temperature Behavior of Polymers and Composites)
Browse Figures
Versions Notes

Abstract

:
Heat sinks are commonly used in electronic devices to dissipate heat from electronic circuits. Phase change materials (PCMs) offer a viable solution for storing thermal energy during peak loads, helping to delay temperature spikes and maintain the heat sink within safe operating limits. The objective of the current study is to evaluate the energy storage and thermal characteristics of the PCMs used in the heat sink. The heat sink comprises a structured porous material (SPM), and the PCMs used in the analysis are Paraffin wax and Erythritol. The thermal analysis conducted on the heat sink composed of SPMs integrated with PCMs enabled the determination of thermal characteristics. The thermal characteristics evaluated from FEA analysis have shown superior heat absorption properties of Erythritol as compared to Paraffin wax during the initial phases. At 50 s after the simulation, the heat absorbed by Erythritol is 89% higher than Paraffin wax, whereas at higher stages, Paraffin wax exhibited higher heat absorption characteristics. At higher time intervals, i.e., 250 s after running the simulation, the Paraffin wax exhibited 49% higher heat absorption capacity as compared to Erythritol. This behavior of both PCM materials can be attributed to different specific heat capacities and latent heat of fusion at different temperatures. The higher thermal conductivity of Erythritol enables it to absorb higher heat initially, which makes it highly effective for short-duration thermal applications. The Paraffin wax has a higher latent heat of fusion and, therefore, stores more thermal energy for prolonged periods, which makes it suitable for applications demanding sustained thermal management. The study findings have suggested that for applications demanding rapid heat absorption, the Erythritol PCM is the best option, whereas the Paraffin wax is suited for applications demanding a longer duration of heat storage.

1. Introduction

Modern electronic devices require effective heat management solutions because of their fast technological progress [1]. Electronic components such as Central Processing Units (CPUs) and Graphics Processing Units (GPUs), along with power electronics and memory chips, produce extensive heat output when working [2]. The lack of proper cooling methods leads components to suffer a drop in performance as well as system instability before experiencing total failure from overheating [3,4]. Electronic devices require proper heat dissipation management during their design phase since optimal thermal strategies ensure both system performance and reliability [5]. Heat sinks serve as the primary thermal management solution that maintains wide application across various electronic systems [6,7]. The heat transfer mechanism in heat sinks relies on thermal energy movement between electronic components and surrounding air through the three processes of conduction, convection, and radiation. The operating performance of heat sinks relies on three primary dimensions, which are material characteristics along with shape elements such as fin designs and total contact area between devices and heat sinks [8]. The development of more powerful and compact electronic devices increases the difficulties for effective heat sink design. Advanced materials and structures have been combined to make innovative solutions to create smaller, lighter, more effective thermal management systems that offer better heat dissipation technologies [9].

1.1. Role of Thermal Evaluation in Heat Sink Design

The design improvement of heat sinks depends on complete thermal evaluations. The evaluations require testing different thermal parameters through simulation methods that examine temperature patterns, heat flow measures, and thermal path effectiveness [10]. Eliminating bottlenecks prior to mass production happens through operational scenario testing, which enables engineers to determine heat sink performance levels and guide the improvement of heat dissipation methods [11]. Thermal behavior of heat sinks is usually analyzed by using Computational Fluid Dynamics (CFDs) simulations, which can provide an accurate evaluation and design optimization of their design [12].

1.2. Integration of PCMs in Heat Sinks

PCMs have shown great potential as thermal management solutions for heat sinks over recent years [4,13]. They function as substances that consume and emit significant amounts through phase transition events from solid to liquid and liquid to gas states [14]. The ability of PCMs to absorb heat energy during heating and then provide thermal storage for cooling applications maintains steady temperatures in electronic components. Heat sinks that embed PCMs achieve superior thermal performance because they enable improved heat transfer combined with superior thermal energy storage capabilities, especially when thermal loads exhibit constant change or cyclic patterns [15]. Two primary methods exist for PCM heat sink integration, where materials are either embedded into heat sink substrates or used as interface layers between heat sink materials and components [16]. Multiple studies have investigated different PCMs, which include Paraffin wax as well as Erythritol and other materials encompassing organic and inorganic categories [17,18]. Most PCMs exhibit poor thermal conductivity, which impedes their effectiveness; therefore, researchers investigate ways to improve this property by incorporating nanoparticles as well as microstructures and different composite materials [19,20,21]. Multiple research investigations have focused on exploring PCM-based heat sinks and their resulting thermal outcomes [22,23]. The thermal behavior of PCM-based heat sinks with different fin configurations and volume fractions was researched by Rajesh Akula et al. [24]. The heat sink containing 8% fin volume fraction and Eicosane PCM showed the top performance under cyclic heat loads while delivering 6.1 times increased capability when compared to traditional heat sinks. This research emphasized the significance of choosing optimum fin shapes along with PCM substances for achieving better thermal control systems. The research of Pradunmya P. Dutta et al. [25] examined how fin designs, either individually or combined with PCM-based heat sinks, affect their operational performance.
The research showed that PEG-6000 served as the PCM when it was coupled with four fins, which maximized thermal efficiency. The research established that the extensive duration during which the heat sink maintained reduced temperatures proved that fin geometry stays crucial for thermal efficiency performance improvements. Taha Ghouchi et al. [26] developed a novel fin design system for microchannel cooling structures, which derived its inspiration from shark plate geometries. The authors conducted CFDs simulations to investigate the cooling effects of different nanofluids, such as MoS2 + H2O and GO + Ag/water. The investigation demonstrated that the newly designed fin structure produced superior performance when operating with nanofluids since these fluids enhanced both thermal transfer capabilities and operational effectiveness. The integration of PCMs in Thermal Energy Storage (TES) systems was examined by Miķelis Dzikēvičs et al. [27]. Their study evaluated thermal conductivity as a necessary factor to boost heat transport functions in PCM-based systems. Further research on TES applications requires additional factors for PCM performance improvement because heat transfer efficiency remains limited by low Rayleigh numbers. David Cabaleiro et al. [28] examined the thermal, along with physical characteristics of PCMs based on polyethylene glycol (PEG) and their response to carbon-based nanoparticles. Nano-diamond powders in PEG-based PCMs increased their thermal conductivity levels, so they offered better performance in heat dissipation applications. Ongoing efforts to enhance PCM performance correlate precisely with this research discovery about thermal conductivity improvement. The research by Andres Vargas et al. [29] involved producing two-tiered PCM-coupled heat sinks with Direct Metal Laser Sintering–Additive Manufacturing techniques. The heat sinks constructed from AlSi10Mg alloy contained topology-optimized fins that simultaneously dissipated heat to PCM and enabled the evacuation of excess energy through air circulation. Experimental testing showed that the multimode cooling structures proved highly efficient for short power outbursts and could be applied to advanced electronic package systems. Yan Zhang et al. [30] executed research that evaluated the thermal conductivity enhancement of Paraffin wax PCMs by integrating carbon nanomaterials along with metal components.
The numerical models showed that metal bubbles in the PCMs produced superior thermal conductivity results than carbon nanoparticles. This study further emphasizes the need to enhance PCM properties as it directly improves heat dissipation effectiveness. Although PCM-enhanced heat sink designs have made great advancements, the improvement of PCMs’ thermal conductivity and the integrity and stability of PCMs in the heat sink structure remain difficult to achieve [31,32,33,34].

1.3. SPM for Thermal Management

The current research interest in thermal management applications focuses on SPMs because these materials provide unique enhancements to PCM-based heat sinks’ performance [35]. The microstructural design of SPMs enhances both surface contact area and thermal conductance and heat dissipation effectiveness by their porous nature [36]. The use of metal foams or other porous structures makes these materials effective at distributing heat, which reduces the temperature gradient commonly found in heat sinks [37]. A combination of SPMs and PCMs in recent studies demonstrates superior performance regarding thermal storage alongside improved transfer features in the system. Research by Zhao et al. [38] showed that metal foam insertion into PCMs facilitates heat transfer through established pathways, which enhance thermal conduction levels. Qureshi et al. [39] studied the integration of PCMs and metallic foams, which resulted in better thermal conductivity and storage capability for managing elevated thermal loads with efficiency. The combined system of PCMs and solid porous media presents substantial potential for cooling systems needing strong heat absorption and efficient heat dispersal processes in electronics and high-performance devices.
Alongside PCM-integrated solutions, geometrical enhancements such as microchannel structures and porous materials have shown great promise in improving heat dissipation. For instance, pin-finned microchannel heat sinks (PF-MCHS) have demonstrated up to 94.8% higher Nusselt numbers compared to conventional flat microchannels, with geometries like rectangular and backward triangular fins offering superior heat transfer at the cost of increased flow resistance [40]. Similarly, tree-like microchannel heat sinks (TLMHS) designed using modified Murray’s law enable high-level branching, resulting in reduced thermal resistance and improved temperature uniformity, which are critical for cooling localized high heat fluxes [41]. While these techniques enhance convective transport, the integration of SPMs with PCMs introduces a hybrid conduction-storage mechanism, offering unique advantages in transient thermal scenarios.
Due to low thermal conductivity, most PCMs have limited effectiveness in high-performance applications. Nanoparticle incorporation or the use of advanced composites has shown promising results [42,43]; however, a coherent strategy to realize the best strategies to improve heat transfer and the capability of thermal storage is required. The combination of SPMs with PCMs produces an elevated solution beyond pure PCM systems because it enhances both thermal response speed and extended energy storage capabilities. This material combination enables uniform heat regulation while solving the slowness of thermal dissipation that usually affects PCM-based cooling methods. The method plays an essential role in improving heat sink performance for applications requiring continuous and rapid thermal control. This study investigates the combination of SPMs with PCM-based heat sinks by studying Paraffin wax and Erythritol to fulfill this research gap. We hypothesize that the combination of SPMs with PCMs should produce substantial heat transfer performance advancement in heat sinks because it improves heat transmutability while enlarging the surface area for heat dissipation. The primary research questions guiding this study are as follows:
  • How does the integration of SPM into PCM-based heat sinks affect their overall thermal performance?
  • How do Paraffin wax and Erythritol compare as PCMs in terms of heat storage and transfer efficiency?
  • Can the synergistic integration of SPMs with PCMs significantly enhance the heat dissipation capability of heat sinks under varying thermal loading conditions?
This investigation aims to evaluate the thermal behavior and energy storage capabilities of heat sinks integrated with PCMs and SPMs. By analyzing their performance under varying thermal loading conditions, the study provides valuable insights into their combined effectiveness for enhancing thermal management in electronic devices. A novel heat sink configuration—featuring SPMs bonded with PCMs—is proposed to further improve thermal performance. The research offers new understanding of the synergistic interaction between these materials, particularly in enhancing heat transfer and thermal storage. These findings are expected to contribute to the development of compact, energy-efficient thermal management systems, especially suited for high-performance and miniaturized electronic applications where space and efficiency are critical.

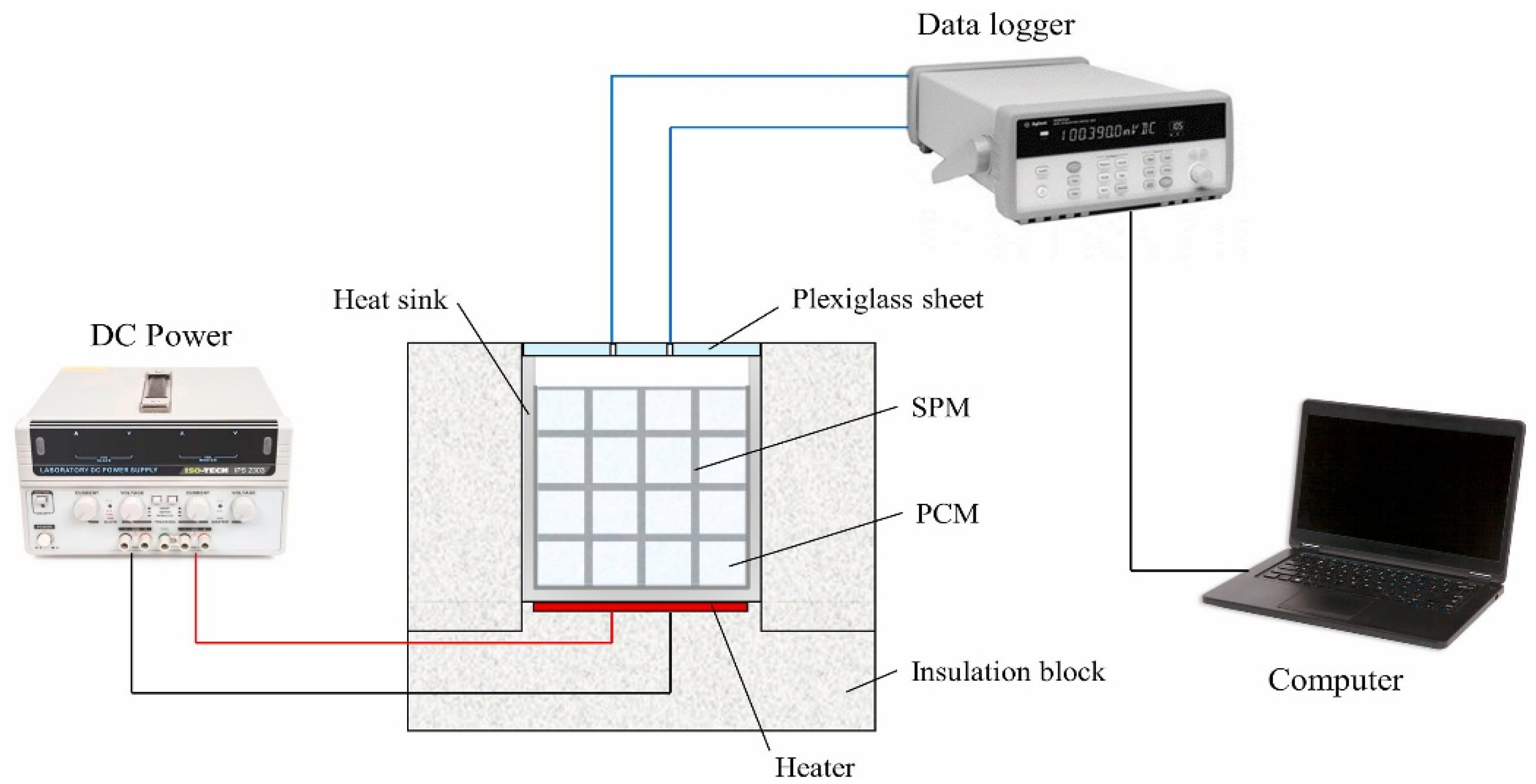
2. Materials and Methods

This study’s methodology is focused on a thermal analysis of the heat sink filled with SPMs integrated with PCMs. This section describes the procedure adopted for 3D modeling, meshing, thermal boundary conditions, and the simulation setup in ANSYS 2024 R1 to analyze the thermal performance of the proposed heat sink design.

2.1. Material Properties

The properties of the materials used in the design of heat sinks significantly impact their thermal performance. Table 1 presents the detailed thermal properties of the materials used in this study: aluminum (heat sink), Paraffin wax, Erythritol (PCM), and SPMs.
Table 1. Material properties.
Table 1. Material properties.
PropertyAluminum (Heat Sink) [44,45]Paraffin Wax (PCM) [46,47]Erythritol (PCM) [48]SPM [49,50]
Density (kg/m3)2700800–8501250850–950
Specific Heat (J/kg·K)900220023501500
Thermal Conductivity (W/m·K)2050.20.30.4
Latent Heat (J/kg)-150–250270–320-
Melting Point (°C)-46–68118–122-
Viscosity (Pa·s)-0.02–0.050.08–0.12-
Porosity---60–80% (typical range)
The heat sink material was chosen to be aluminum due to its favorable combination of thermal and mechanical properties. A thermal conductivity value of 205 W/m·K was used based on standard references such as [45,51] and widely accepted industrial data for general-purpose aluminum used in heat sink applications [52,53,54]. While the thermal conductivity of aluminum can vary depending on alloy and processing conditions (e.g., 117–227 W/m·K), the selected value represents a reasonable and commonly adopted average for commercial-grade aluminum (e.g., 6061-T6), ensuring both practicality and modeling consistency. Additionally, aluminum’s low density, corrosion resistance, and cost-effectiveness make it a widely preferred material for thermal management in electronic systems. In this study, Paraffin wax and erythritol were selected as the PCMs due to their favorable thermal properties. Paraffin wax, with a melting point range of 46–68 °C, is a widely used PCM in electronic cooling systems due to its reliable performance and stability during phase change. Erythritol was selected for its relatively high melting point (118–122 °C) and substantial latent heat, making it particularly effective for high-temperature thermal management applications that demand efficient heat storage and controlled release over extended durations. To further improve the thermal performance of the heat sink, SPMs were incorporated.
These materials, characterized by a moderate-to-high porosity range of 60–80%, offer an optimal balance between void volume for PCM infiltration and structural stability for sustained operation [55,56,57]. While higher porosities (up to 98%) have been reported in certain foam structures [57], the chosen range ensures effective thermal conductivity enhancement and mechanical integrity. Modeled as aluminum-based metallic foams, the SPMs improve the effective thermal response of the heat sink system by promoting uniform heat distribution throughout the PCM domain and ensuring compatibility with the primary heat sink material.
The foam structure is designed as a periodic lattice, with ligament dimensions of 10 mm, which ensures a balance between mechanical stability and optimal thermal performance. This open-cell structure maximizes the surface area available for heat exchange, allowing the PCM to infiltrate the porous material effectively and reducing the thermal resistance that is typically a challenge in pure PCM systems. By creating continuous conductive pathways, the SPM helps to accelerate heat transfer through the system, particularly during transient heating conditions, ensuring faster thermal response and reducing thermal lag.
The integration of SPM in this study is informed by extensive research that highlights its advantages in enhancing thermal conductivity and improving the efficiency of PCM-based thermal management systems. Porous materials, such as metallic foams, have been shown in previous studies to significantly enhance heat transfer by facilitating convective heat flow through the PCM, which is crucial during the phase change process. This enhanced thermal performance allows for a more stable and controlled temperature distribution across the heat sink, improving the overall thermal management of electronic systems. The selection of SPMs in this study thus draws on their proven effectiveness in overcoming the limitations of traditional PCM systems, offering a promising solution for high-performance thermal applications. These materials are chosen to comply with the motive of maximizing heat dissipation in modern electronic systems, particularly under transient thermal conditions.

2.2. Design and Modeling of Heat Sink with PCM and SPM

The proposed heat sink uses SPM in conjunction with PCM for its design. Figure 1 illustrates the heat sink design schematic. The heat sink possesses an overall dimension plan of 44 mm × 44 mm × 52 mm that aligns with standard specifications for electronic components within the small to medium classification [49]. Specific dimensions of 10 mm apply to both the SPM ligament length and thickness to achieve adequate heat transfer performance without compromising stability. The simulation model of the heat sink incorporating SPM was designed through Creo software (v10), which Parametric Technology Corporation (PTC) develops [58]. The precision design features of Creo enable users to regulate the heat sink component dimensions along with its structure [59]. The schematic diagram in Figure 1 demonstrates how PCM interacts with the SPM structure inside the heat sink. Figure 2 displays the 3D CAD model of the heat sink. The CAD design presents the precise layout of SPM and PCM elements for the heat sink structure.

2.3. Model Conversion and Importation to ANSYS

The CAD model developed in Creo gets converted into a Parasolid file format because ANSYS requires this format for its thermal analysis. Parasolid functions as a popular 3D solid modeling file format that allows efficient design-data migration between CAD software and analysis programs [60]. The imported design of the heat sink with SPM into ANSYS is shown in Figure 3.

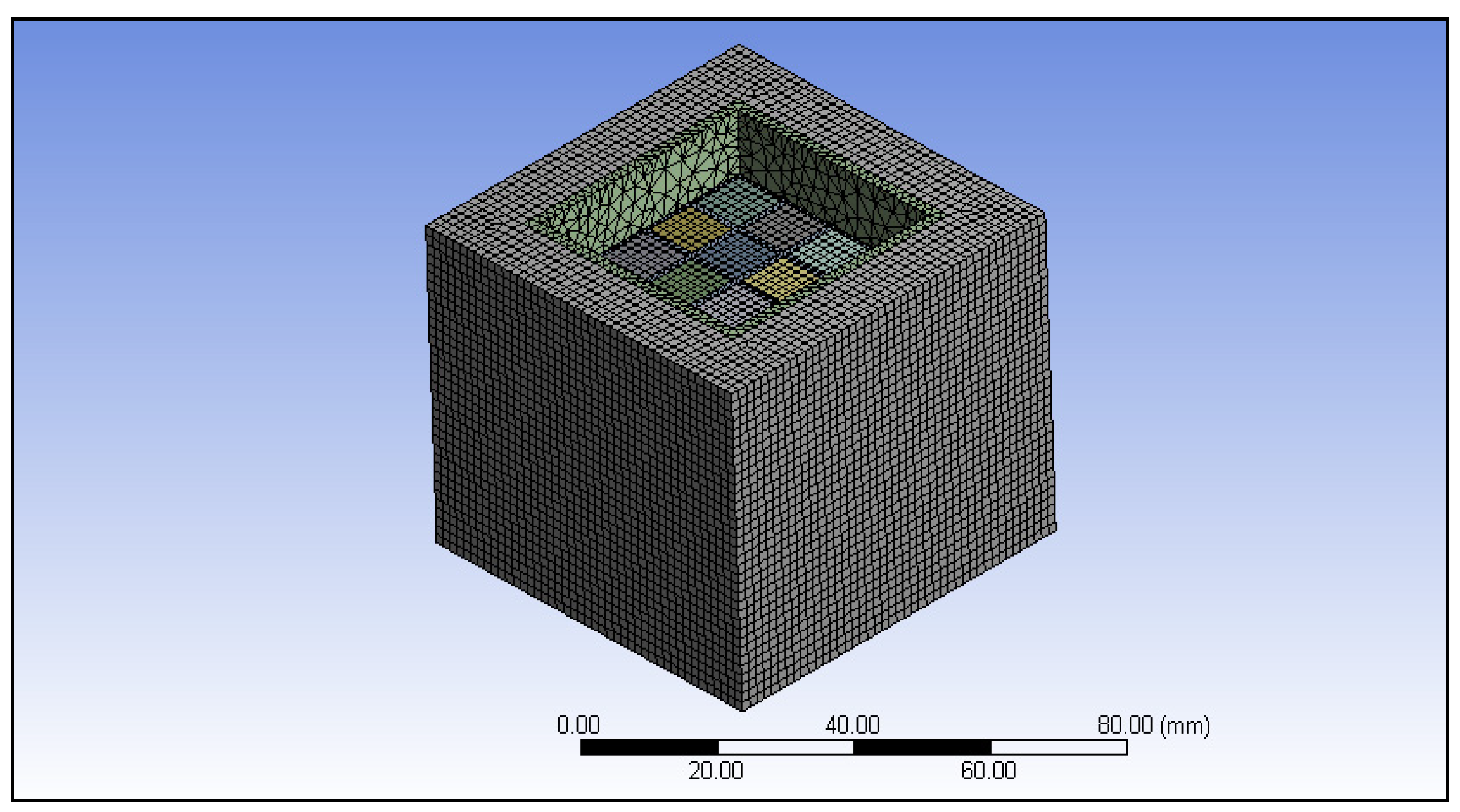
2.4. Meshing of the Model

To enable numerical analysis, the model must first be discretized into smaller elements using meshing. Meshing is a critical step as it divides the geometry into manageable parts for analysis, and the quality of the mesh directly affects the accuracy of the simulation.
  • Size Function: set to adaptive to ensure the mesh refines in areas with higher thermal gradients.
  • Relevance Center: set to fine to improve the resolution of the mesh.
  • Element Size: set to 0.680 mm, a value chosen to balance accuracy and computational cost.

Mesh Output and Grid Independence Evaluation

The total number of elements generated is 69,551, and the total number of nodes generated is 275,449. This level of meshing ensures high accuracy in capturing the thermal gradients and flow through the heat sink material. The grid independence test is presented in Table 3 of Section 3.6.
  • Meshed Model: the meshed model of the heat sink is presented in Figure 4, where each element is shown, and the discretization process is highlighted.
A grid independence study is performed to achieve accuracy and reliability in the results. The mesh quality needs to be validated by this test, and results should not have significantly changed further with mesh refinement. In Table 2, the grid independence test results are summarized.
The table shows that after a minimal temperature variation between meshes of different element counts at approximately 69,551 elements, the variation is again minimized. This indicates that the mesh has been refined enough and that further mesh refinement would not significantly change the solution.

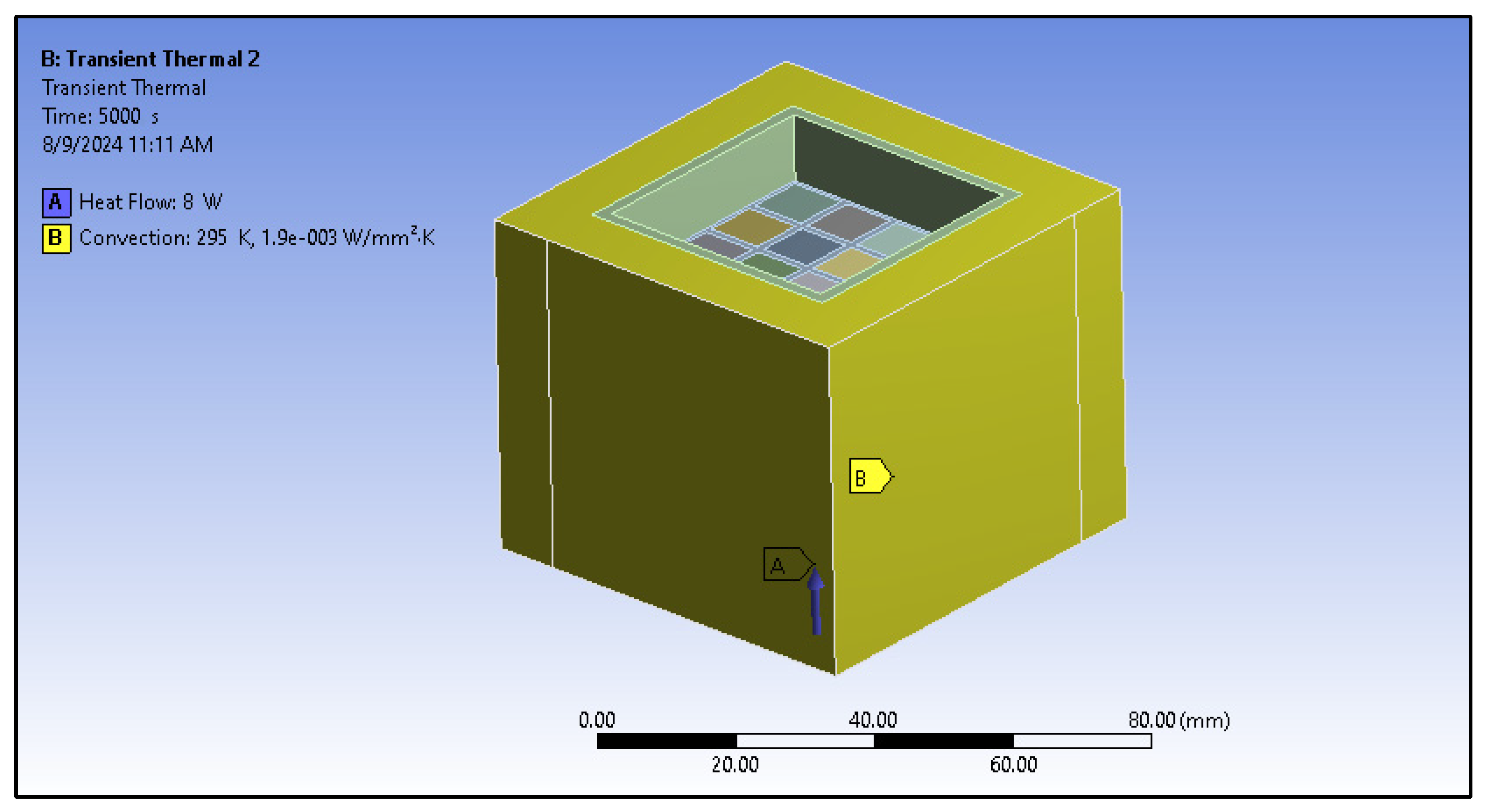
2.5. Application of Thermal Boundary Conditions

Boundary conditions for thermal analysis need to be applied next after the meshing process completes. The set boundary conditions determine how heat enters or leaves the heat sink during its interaction with external surroundings.
  • Heat Flow: A constant heat flux of 8 W is applied at the base of the heat sink. This value is selected based on typical power dissipation in small electronic devices.
  • Convection Boundary Condition: The convection heat transfer coefficient is taken to be 1900 W/m2·K, which is the rate of heat transfer between the heat sink and the surrounding air. The temperature of the ambient is set to typical room temperature, i.e., 295 K. Figure 5 shows the thermal boundary conditions on the heat sink model, where the heat flux and convection are applied to the model.

2.6. Simulation Setup

After that, the thermal boundary conditions can be applied. The next step will be to define the simulation settings. These are parameters for use in performing the transient thermal simulation within ANSYS. The end time for the simulation is set to 5000 s to model the heat sink’s thermal behavior over a sufficient period. The simulation is divided into 100 sub-steps to ensure accurate time–step resolution during transient heat flow analysis. Heat Convergence was monitored to bring the temperature solution to convergence [61].
Temperature convergence: the distribution of the temperature must reach a steady state during the simulation.
Line Convergence: it ensures that the analysis converges upon a solution over its course.
Matrix formulation, inversion, and multiplications control are left program-controlled to allow the solver to make automatic adjustments on these activities according to the current solution process. Under transient thermal conditions, the simulation is run using the model over time to obtain the dynamic heat dissipation and storage processes of PCMs and SPMs.

2.7. Solver Operations & Nodal Calculations

The ANSYS solver then performs a series of matrix operations, i.e., matrix formulation, matrix inversion, and matrix multiplication in the solution stage. These operations allow the solver to compute the temperature and heat flux values in the model for each node [62]. The heat flux and temperature distribution for each node in the mesh are calculated by the solver. These thermal behavior results are then interpolated across the element edges to provide a detailed picture of the thermal behavior of the heat sink. The thermal performance of the heat sink in terms of the temperature distribution, heat flux, and phase change behavior of the PCM is analyzed after the simulation has been run. The thermal conductivity, heat transfer efficiency, and energy storage capability of the unconventional designs are compared to conventional heat sink designs for improvement.
This study adopted a methodology integrating advanced design tools, accurate meshing techniques, and thermal boundary conditions for thermal performance analysis of PCM and SPM-based heat sinks. Using Creo for CAD modeling alongside ANSYS 2024 R1 for thermal simulations leads to precise and accurate thermal dynamics modeling of the system. An 8 W heat flux combined with a 1900 W/m2·K convection coefficient emerges from standard operating requirements for electronic components. The simulation executes properly because adaptive mesh refinement, when combined with a fine element size, enables the model to detect minute thermal gradients that exist in the heat sink structure, together with PCM’s phase transition behaviors. The reason for implementing transient thermal simulations is their ability to capture dynamic thermal system behavior when operational thermal loads change throughout time [63]. The methodology is able to give insights not only about the thermal efficiency of the design but also a reliable foundation for further optimization in future works by using this robust and comprehensive approach. That experience changes thermal requirements throughout use. Moreover, this ensures that the developed research will be used to aid in the creation of more efficient and effective heat dissipation for high-performance electronic devices.

3. Results and Discussion

The thermal performance analysis of heat sinks containing SPM with PCM is presented in this subsection. Several thermal parameters, such as heat flux distribution and temperature distribution, and heat absorption behavior, are assessed through time–interval analysis. The study examines heat sink behavior during transients by comparing all results to published literature and delivers a full explanation of its performance.

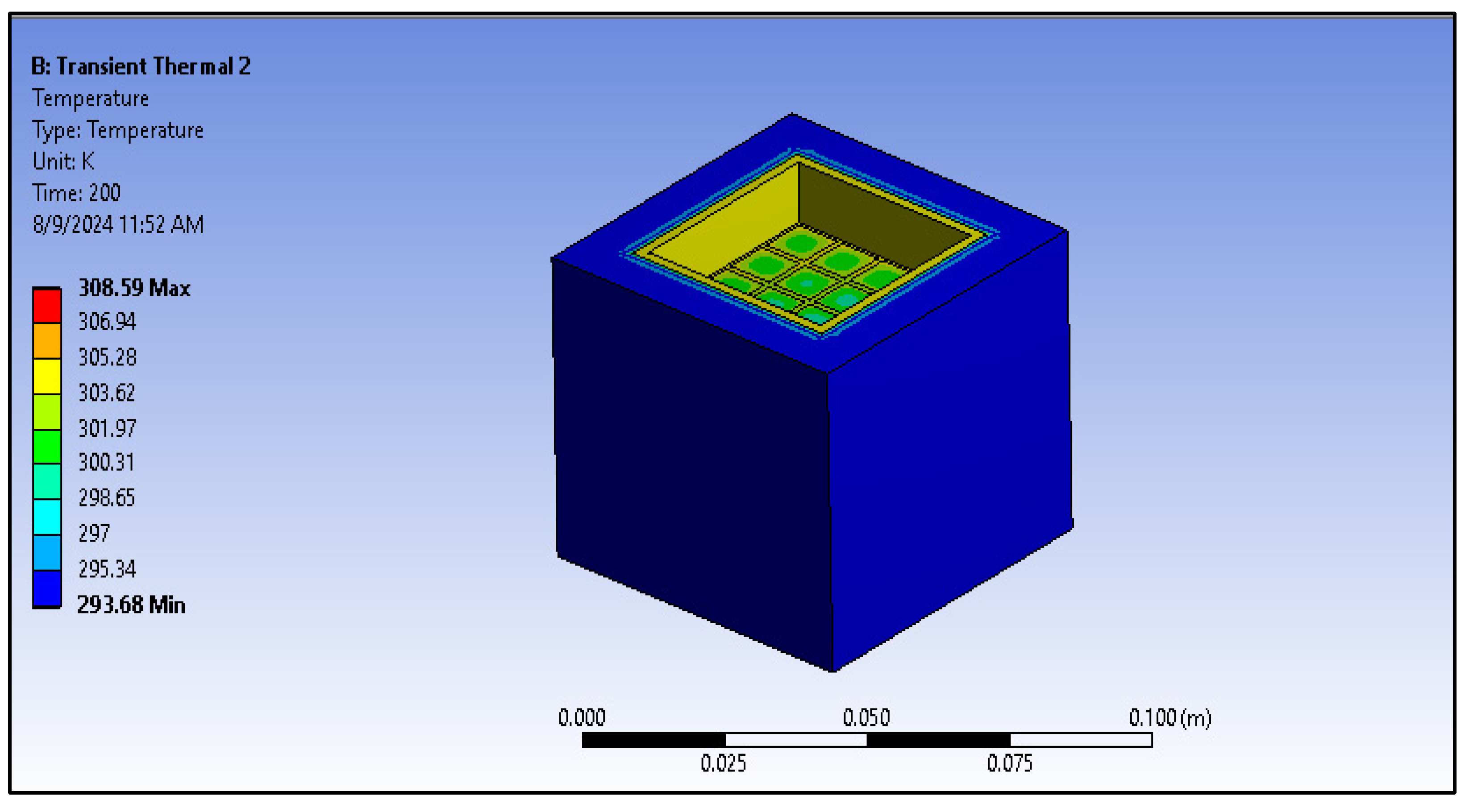
3.1. Temperature Distribution

Temperature distribution is an important parameter used to understand the heat dissipation capability of the heat sink with PCM and SPM. The temperature distribution figures below display the temperature at different time intervals, such as 100 s (Figure 6), where the temperature of the heat sink reaches 303.06 K, and the PCM temperature reaches 299 K. Such values follow the values in the literature [49].
  • At 200 s (Figure 7), the temperature of the heat sink reaches 306.94 K, and the PCM temperature reaches 301.97 K. We can see that as the PCM absorbs heat, the temperature continues to rise until the PCM melts slowly.
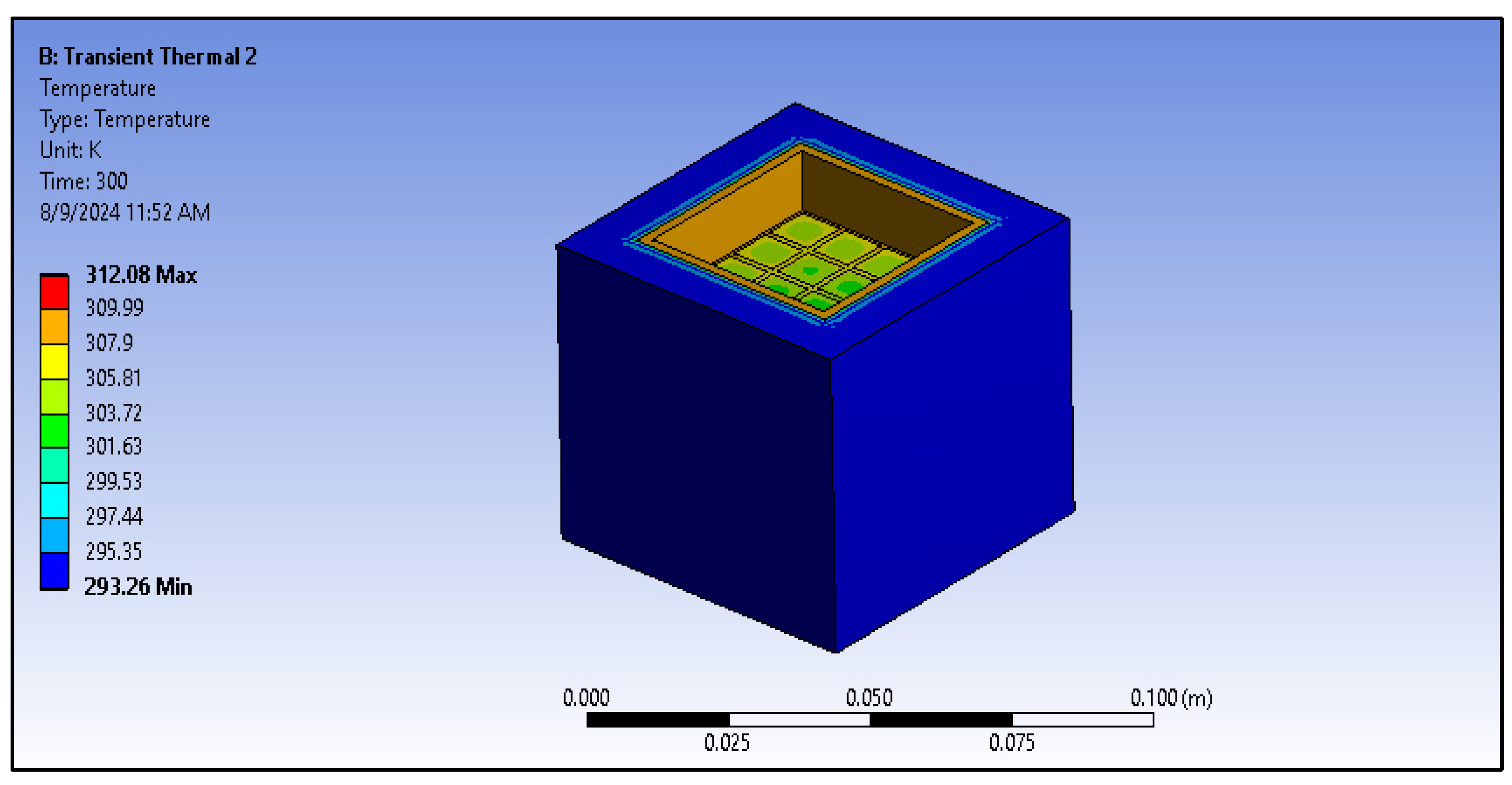
  • At 300 s, the temperature of the heat sink becomes 309.9 K, and the temperature of the PCM comes to 303.72 K as shown in Figure 8. The increasing trend is induced due to the continuous heat absorption by the PCM.
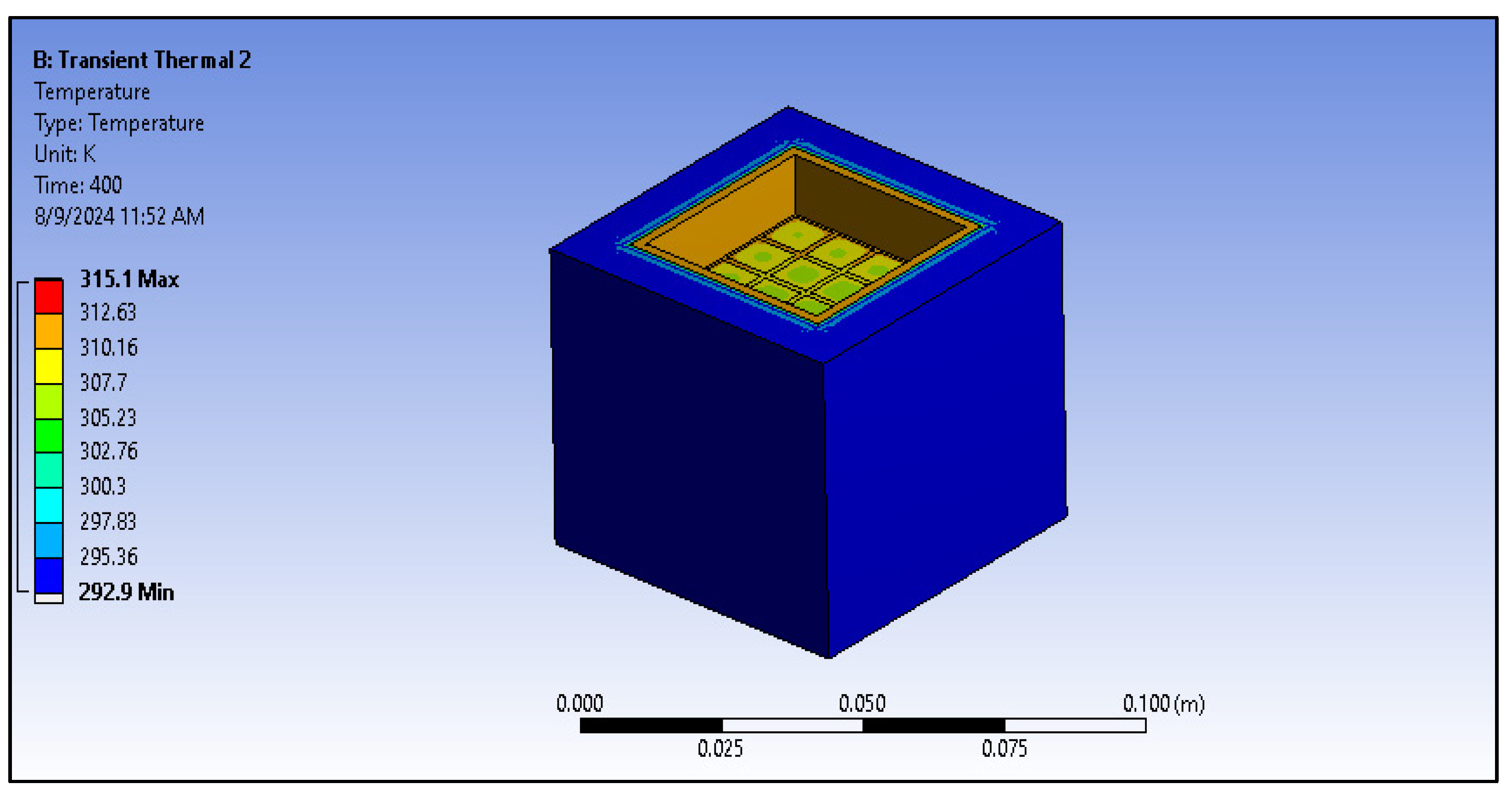
  • At 400 s, the heat sink temperature reaches 312 K, with the PCM material maintaining a temperature of 303.72 K. These values are indicative of the efficient heat transfer process and PCM performance, as shown in Figure 9.
The increase in temperature over time is consistent with the nature of heat dissipation from electronics, where the heat sink progressively absorbs and distributes the heat from the component. The gradual temperature rise also highlights the role of PCM in delaying temperature increase, maintaining thermal stability.

3.2. Temperature vs. Time Curve

The temperature vs. time curve for the heat sink with PCM is generated for a probe point located at the second layer lattice of the heat sink (as shown in Figure 10). The curve demonstrates an exponential increase in temperature over time. Initially, the temperature rise is relatively slow, but it accelerates as time progresses, ultimately reaching a maximum value of 357 K. This exponential temperature rise is characteristic of systems where heat accumulation is not instantaneous and is influenced by material properties and phase change behavior.

3.3. Heat Flux Distribution

Heat flux is a critical parameter for understanding how efficiently the heat sink disperses the thermal energy. The heat flux distribution across the heat sink and PCM material is examined in the following figures.
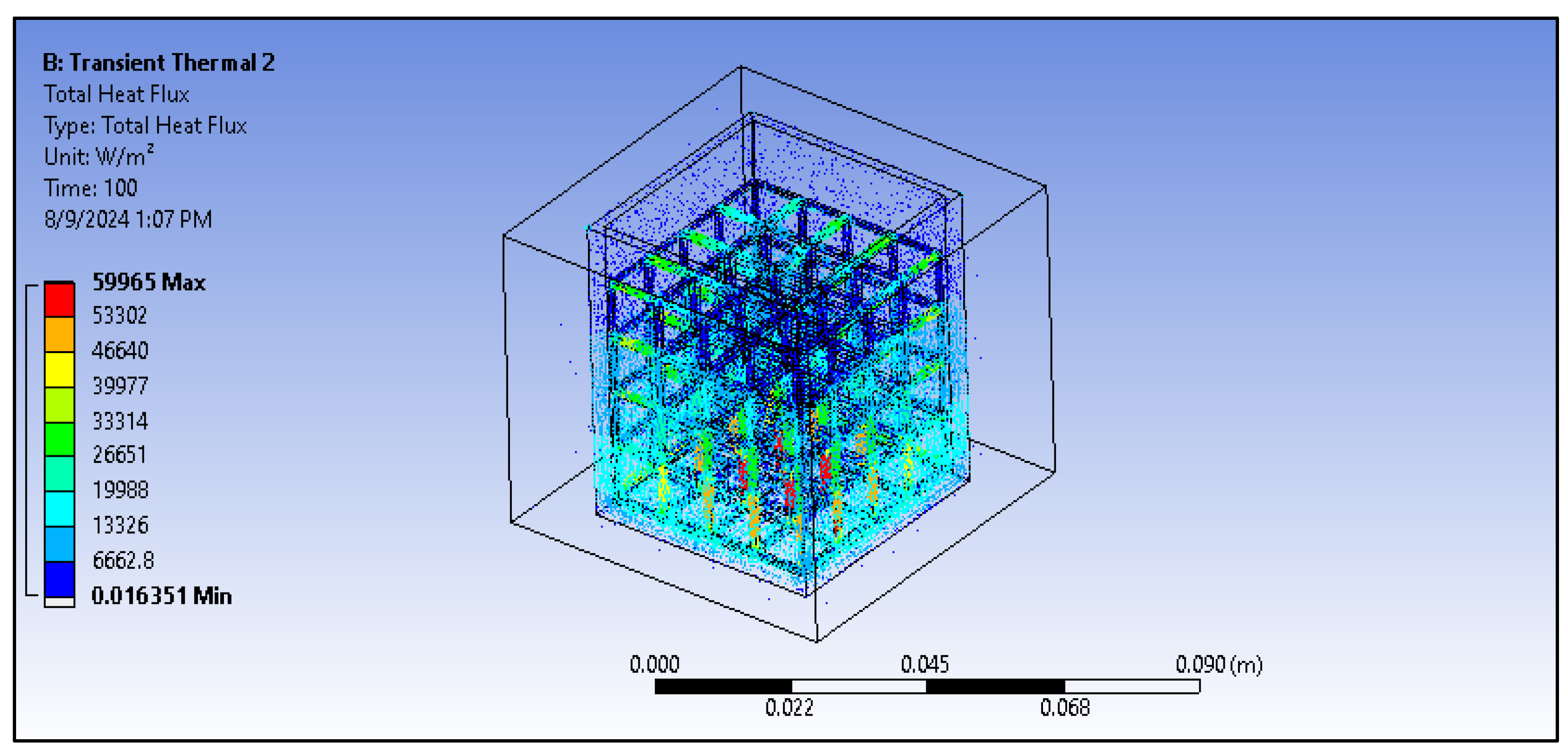
  • Heat Sink Domain: The heat flux vector plot for the entire geometry of the heat sink is shown in Figure 11. The highest magnitude of heat flux is observed at the bottom lattices of the heat sink, with values exceeding 59,965 W/m2. The heat flux is notably higher at the center lattices compared to the edge lattices, which experience a lower heat flux of approximately 26,655 W/m2.
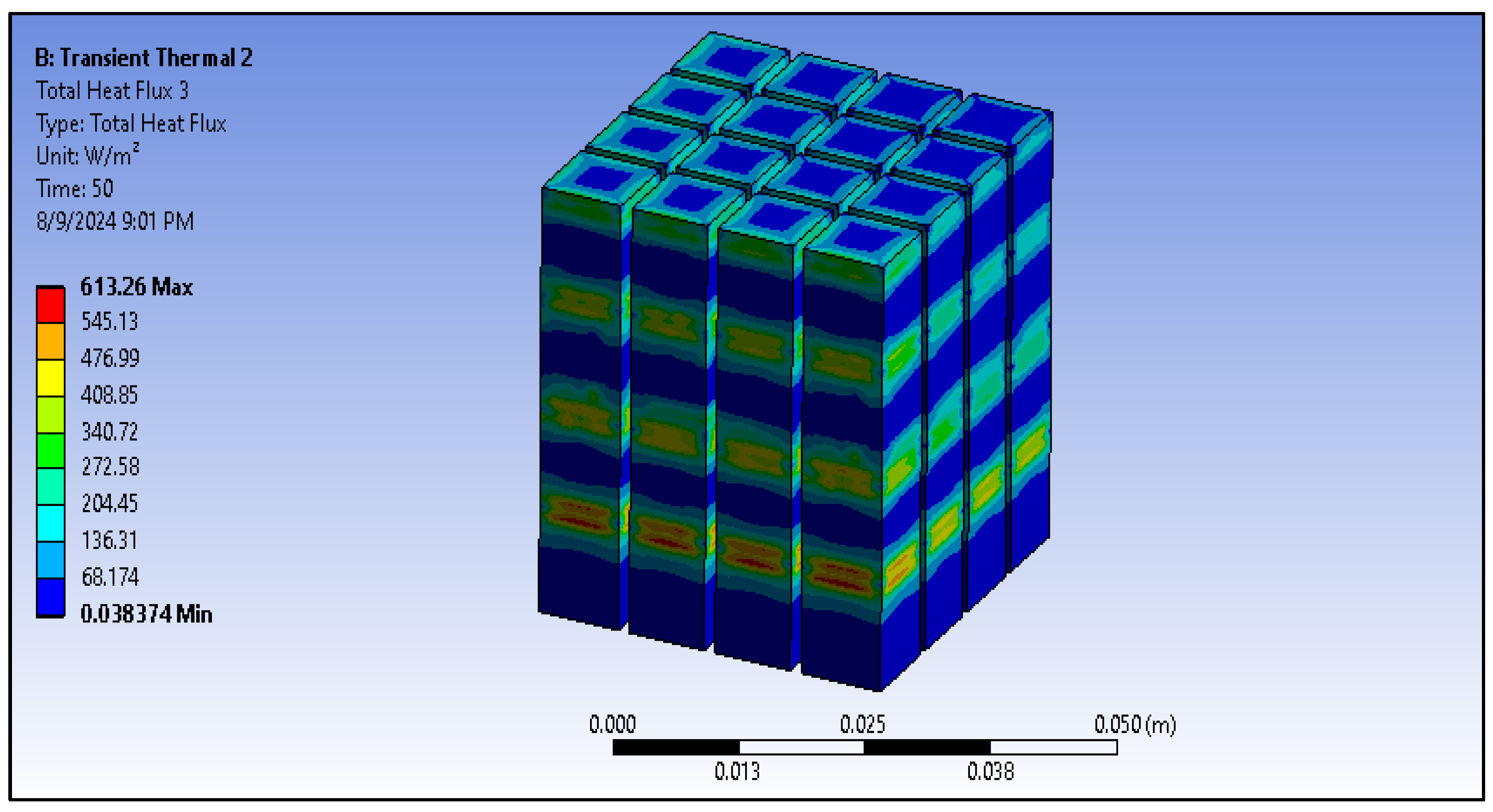
  • PCM Domain: The heat flux distribution within the PCM domain is shown in Figure 12. The plot reveals that the highest heat flux occurs at the lower zones of the PCM (near the base), with values reaching up to 613 W/m2. The heat flux decreases as the material moves away from the base, which corresponds to the natural heat distribution expected in PCMs.

3.4. Temperature Distribution in PCM Domain

The temperature distribution in the PCM domain shows a non-uniform pattern, as seen in Figure 13. The maximum temperature attained in the PCM domain is 358.8 K, while the minimum temperature is 355.6 K. These values reflect the thermal stratification of the PCM, with the lower zones of the material absorbing more heat due to their proximity to the heat sink. The non-uniform temperature distribution is typical for PCM systems, especially during the initial stages of phase change.

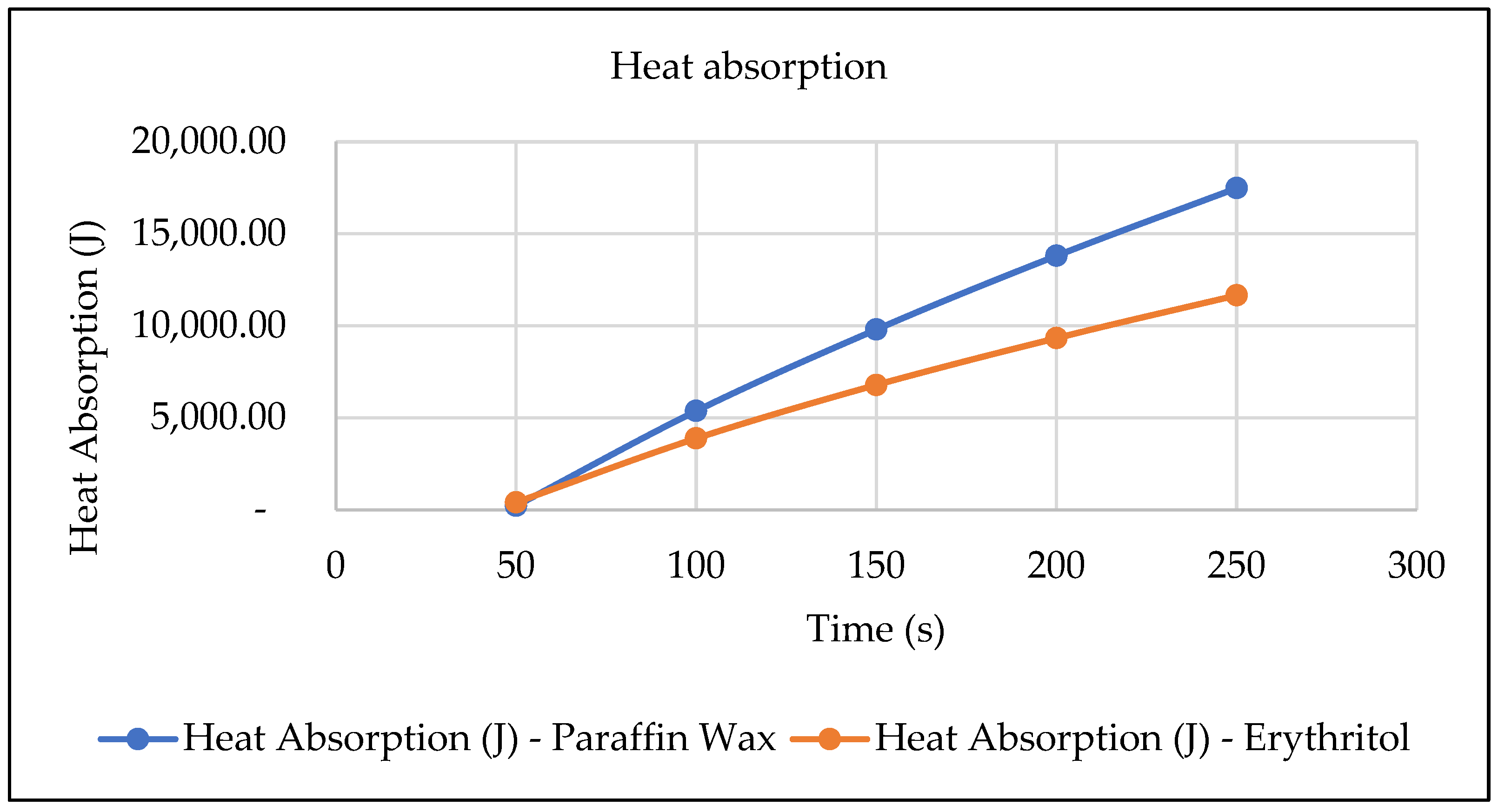
3.5. Heat Absorption by PCM Materials

The heat absorption characteristics of two PCMs—Paraffin wax and Erythritol—are compared in terms of their ability to absorb heat over time before and during the melting phase. Figure 14 shows the temperature vs. time curve for both PCMs.
The heat absorption is calculated using the following formulas [64]:
Before Melting: Q = m ⋅ c ⋅ ΔT
where m is the mass of the PCM, c is the specific heat capacity, and ΔT is the temperature change.
During Melting: Q = m ⋅ L
where L is the latent heat of fusion.
The heat absorption comparison for both materials is shown in Table 3 below.
Table 3. Heat absorption comparison.
Table 3. Heat absorption comparison.
Time (s)Heat Absorption (J)—Paraffin WaxHeat Absorption (J)—Erythritol
50215406
1005372.43886
1509805.26786
20013,8129338
25017,485.811,658
The results show that Erythritol absorbs more heat in the early stages of the heating process, but Paraffin wax demonstrates a more gradual and consistent increase in heat absorption, making it a more stable PCM over longer durations. The distinct thermal behaviors observed for Erythritol and Paraffin wax can be attributed to their different thermo-physical properties. Erythritol, with its higher thermal conductivity (~0.3 W/m·K) and specific heat capacity, absorbs heat more rapidly in the early stages of heating, leading to a quicker rise in temperature. In contrast, Paraffin wax, with a lower thermal conductivity (~0.2 W/m·K) and higher latent heat of fusion, absorbs heat more gradually but demonstrates superior thermal storage at later stages. This is evidenced by Paraffin wax’s ability to absorb 49% more heat at 250 s compared to Erythritol, as it efficiently stores energy through phase change without a significant increase in temperature.

3.6. Validation

To validate the accuracy and consistency of the findings from this study, we compared the maximum temperature results with those reported in the literature. As shown in Table 4, the maximum temperature obtained in our study for the heat sink with PCM integration was 315.12 K. In contrast, the temperature observed in the literature [49] was 314.15 K, with a small difference of +0.97 K. This close agreement validates the thermal model and simulation parameters adopted in this work. The negligible difference underscores the reliability of the numerical framework used and supports the suitability of the SPM and PCM integration approach for enhanced heat dissipation.
This small variation can be attributed to several factors, including differences in material properties (such as specific heat, thermal conductivity, and latent heat), simulation models, and boundary conditions. For instance, the use of Erythritol as the PCM in this study could explain the observed variation, as different PCMs possess unique thermal properties. Furthermore, the mesh settings, simulation software, and boundary conditions might have varied between our study and the referenced work, leading to slight differences in temperature distribution. Despite these minor variations, the temperature values obtained in our study are in reasonable agreement with those in the literature, thus supporting the validity of our simulation results.
The obtained results from the simulation match the expected behavior of the heat sink and the PCM system. The temperature distributions over time present the expected phenomena of temperature ramping as seen in other thermal management systems where heat dissipation is gradual. The results additionally show that PCMs can help maintain temperature by absorbing heat and slowing the rate at which temperature rises. The heat transfer distribution inside the PCM and heat sink domains indicates that the highest distribution of the thermal activity takes place at the system base, where the heat is applied first. This behavior is anticipated and helps facilitate the design of an efficient heat sink with PCM integration. Results from the temperature vs. time curve and the heat absorption follow the expected PCM behavior. Among these compounds, Paraffin wax shows very good heat absorption and is more stable for longer-term heat absorption compared with the other compounds studied; therefore, it is an ideal material for use in the electronic cooling system. The overall simulation results show that the proposed heat sink with PCM and SPM manages heat dissipation effectively, and the performance is satisfactory or even better than desired.
The observed thermal performance of the heat sink integrated with PCM and SPM can be attributed to coupled mechanisms of sensible heat absorption, latent heat storage, and enhanced conduction. Initially, Erythritol exhibits faster heat uptake due to its higher thermal conductivity (0.3 W/m·K) relative to Paraffin wax (0.2 W/m·K), enabling rapid heat diffusion and steeper temperature gradients. This results in higher early-stage heat absorption, as reflected in the simulation at 50 s. As heating progresses, Paraffin wax outperforms Erythritol in thermal storage due to its higher latent heat of fusion (~200–250 J/g), allowing it to absorb more energy without significant temperature rise. The SPM structure amplifies these effects by providing an interconnected solid matrix that promotes efficient thermal bridging and increased interfacial contact with the PCM. These pathways enhance internal conduction, reduce thermal resistance, and facilitate uniform melting, minimizing hot spots. Thus, the integrated mechanism—combining sensible heating, latent storage, and SPM-enhanced conduction—underpins the improved thermal regulation observed in the composite system. Further optimization of the geometry and PCM material properties can improve the efficiency of the heat sink to be applied for heat dissipation of high-performance electronic applications.

4. Conclusions

Thermal analysis of the heat sink with and without SPM and the PCM has been carried out to study the heat absorption and dissipation behaviors of the materials under transient thermal conditions. Finite Element Analysis (FEA) thermal properties evaluation showed significant differences between the performance of Erythritol and Paraffin wax as PCMs. During the initial phase of heating, erythritol showed a better heat absorption, 89% higher than Paraffin wax in absorbing heat at 50 s. However, as the simulation continued over longer time intervals, Paraffin wax was found to take in more heat at 250 s, by an amount of 49% more than Erythritol. Different specific heat capacities and latent heat of fusion values of the two materials lead to different heat storage and release capacities at different temperatures, which is the reason for this observed behavior. Paraffin wax, on the other hand, excels in applications that need long periods of thermal management with latent heat of fusion, as a higher value compared to Erythritol, which is better suited for short-duration rapid heat absorption applications since it has a higher thermal conductivity. The results answer the main research questions on the effectiveness of these PCMs to improve the thermal behavior of heat sinks and confirm the hypothesis that different PCMs provide different performance for different applications.
While significant insight is provided in the study, there are limitations. The simulation is done using idealized models, and real-world complications like variable environmental conditions or material degradation over time may not be considered explicitly. Moreover, the emphasis was limited to two PCMs, and more work could comprise a wider spectrum of PCMs and their combinations with various types of heat sinks. This research benefits from the fact that it can potentially lead to applications of electronic cooling, thermal energy storage, or more generally, any systems where thermal management is required. The results can be used to select appropriate PCM for particular applications where rapid heat absorption or long thermal regulation is needed. Future work needs to examine the performance of additional PCMs, especially novel thermal properties, together with the development of hybrid PCM systems. Further studies could also incorporate experimental validation to enhance the accuracy of the findings and extend the scope of this research to more diverse thermal management applications.

Author Contributions

Conceptualization, A.A. and M.K.W.K.; methodology, M.I.; software, A.A.; validation, M.I., A.A. and M.K.W.K.; formal analysis, A.A.; investigation, A.A.; resources, M.K.W.K.; data curation, M.I., A.A. and M.K.W.K.; writing—original draft preparation, A.A.; writing—review and editing, M.I. and M.K.W.K.; visualization, M.I.; supervision, A.A.; project administration, M.I.; funding acquisition, M.K.W.K. All authors have read and agreed to the published version of the manuscript.

Funding

The APC was funded by the University of Johannesburg.

Data Availability Statement

The original contributions presented in this study are included in the article. Further inquiries can be directed to the corresponding author.

Conflicts of Interest

The authors declare no conflicts of interest.

References

  1. Ghadim, H.B.; Godin, A.; Veillere, A.; Duquesne, M.; Haillot, D. Review of Thermal Management of Electronics and Phase Change Materials. Renew. Sustain. Energy Rev. 2025, 208, 115039. [Google Scholar] [CrossRef]
  2. Siahchehrehghadikolaei, S.; Gholinia, M.; Ghadikolaei, S.S.; Lin, C.-X. A CFD Modeling of CPU Cooling by Eco-Friendly Nanofluid and Fin Heat Sink Passive Cooling Techniques. Adv. Powder Technol. 2022, 33, 103813. [Google Scholar] [CrossRef]
  3. Rasool, G.; Xinhua, W.; Sun, T.; Hayat, T.; Sheremet, M.; Uddin, A.; Shahzad, H.; Abbas, K.; Razzaq, I.; Yuexin, W. Recent Advancements in Battery Thermal Management System (BTMS): A Review of Performance Enhancement Techniques with an Emphasis on Nano-Enhanced Phase Change Materials. Heliyon 2024, 10, e36950. [Google Scholar] [CrossRef]
  4. Agarwal, A.; Molwane, O.B.; Pitso, I. Thermal Analysis & Modelling of Phase Change Material Used in Low Temperature Industrial Wastewater Applications. Mater. Today Proc. 2021, 39, 596–605. [Google Scholar] [CrossRef]
  5. Bianco, V.; De Rosa, M.; Vafai, K. Phase-Change Materials for Thermal Management of Electronic Devices. Appl. Therm. Eng. 2022, 214, 118839. [Google Scholar] [CrossRef]
  6. Agarwal, A.; Letsatsi, M.T.; Pitso, I. Response Surface Optimization of Heat Sink Used in Electronic Cooling Applications. In Recent Advances in Materials and Modern Manufacturing: Select Proceedings of ICAMMM 2021; Springer Nature: Singapore, 2022; pp. 121–129. [Google Scholar]
  7. Kumar, H.; Agarwal, A.; Kalenga, M.K.W.; Prasad, R.; Kumar, P.; Chilakamarri, L.A.; Yelamasetti, B. Fabrication of Cu/SiC Surface Composite via Thermo-Mechanical Process (Friction Stir Processing) for Heat Sink Application. Materials 2025, 18, 1179. [Google Scholar] [CrossRef]
  8. Khan, W.A.; Culham, J.R.; Yovanovich, M.M. Performance of Shrouded Pin-Fin Heat Sinks for Electronic Cooling. J. Thermophys. Heat. Trans. 2006, 20, 408–414. [Google Scholar] [CrossRef]
  9. Rahman, M.A.; Hasnain, S.M.M.; Paramasivam, P.; Ayanie, A.G. Advancing Thermal Management in Electronics: A Review of Innovative Heat Sink Designs and Optimization Techniques. RSC Adv. 2024, 14, 31291–31319. [Google Scholar] [CrossRef]
  10. Gaikwad, A.; Sathe, A.; Sanap, S. A Design Approach for Thermal Enhancement in Heat Sinks Using Different Types of Fins: A Review. Front. Therm. Eng. 2023, 2, 980985. [Google Scholar] [CrossRef]
  11. Ahmad, E.Z.; Fazlizan, A.; Jarimi, H.; Sopian, K.; Ibrahim, A. Enhanced Heat Dissipation of Truncated Multi-Level Fin Heat Sink (MLFHS) in Case of Natural Convection for Photovoltaic Cooling. Case Stud. Therm. Eng. 2021, 28, 101578. [Google Scholar] [CrossRef]
  12. Sun, P.; Ismail, M.A.; Mustaffa, A.F. Metamodel-Based Design Optimization for Heat Transfer Enhancement of Finned Heat Sinks. Int. J. Therm. Sci. 2025, 214, 109896. [Google Scholar] [CrossRef]
  13. Agarwal, A. Computational Investigation of Vertical Axis Wind Turbine in Hydrogen Gas Generation Using PEM Electrolysis. J. New Mater. Electrochem. Syst. 2022, 25, 172–178. [Google Scholar] [CrossRef]
  14. Das, A.; Apu, M.M.H.; Akter, A.; Reza, M.M.A.; Mia, R. An Overview of Phase Change Materials, Their Production, and Applications in Textiles. Results Eng. 2025, 25, 103603. [Google Scholar] [CrossRef]
  15. Morciano, M.; Fasano, M.; Chiavazzo, E.; Mongibello, L. Trending Applications of Phase Change Materials in Sustainable Thermal Engineering: An up-to-Date Review. Energy Convers. Manag. X 2025, 25, 100862. [Google Scholar] [CrossRef]
  16. Nayak, K.C.; Saha, S.K.; Srinivasan, K.; Dutta, P. A Numerical Model for Heat Sinks with Phase Change Materials and Thermal Conductivity Enhancers. Int. J. Heat. Mass. Transf. 2006, 49, 1833–1844. [Google Scholar] [CrossRef]
  17. Yadav, A.; Pandey, A.; Samykano, M.; Kalidasan, B.; Said, Z. A Review of Organic Phase Change Materials and Their Adaptation for Thermal Energy Storage. Int. Mater. Rev. 2024, 69, 380–446. [Google Scholar] [CrossRef]
  18. Hou, X.; Xing, Y.; Xu, Z.; Du, Y.; Gao, Y.; Yin, J.; Wang, S. Design and Experimental Analysis of a Cooling System with Erythritol/Xylitol PCM Thermal Energy Storage. J. Energy Storage 2024, 87, 111452. [Google Scholar] [CrossRef]
  19. Trigui, A.; Abdelmouleh, M. Improving the Heat Transfer of Phase Change Composites for Thermal Energy Storage by Adding Copper: Preparation and Thermal Properties. Sustainability 2023, 15, 1957. [Google Scholar] [CrossRef]
  20. Yazdani McCord, M.R.; Seppälä, A.; Pourakbari-Kasmaei, M.; Zimmerman, J.B.; Rojas, O.J. From Low Conductivity to High Energy Efficiency: The Role of Conductive Polymers in Phase Change Materials. Chem. Eng. J. 2025, 508, 160804. [Google Scholar] [CrossRef]
  21. Rajendran, R.C.; Anto, T.; Agarwal, A.; Ilunga, M.; Jayamani, E.; Natarajan, V.D. Materials Synthesis and Characterization of Nano-Titanium Carbide-Filled Acrylonitrile Butadiene-Styrene Polymer Composites for Electromagnetic Interference Shielding. Rev. Compos. Matériaux Avancés 2024, 34, 673–679. [Google Scholar] [CrossRef]
  22. Al Siyabi, I.; Khanna, S.; Mallick, T.; Sundaram, S. Multiple Phase Change Material (PCM) Configuration for PCM-Based Heat Sinks—An Experimental Study. Energies 2018, 11, 1629. [Google Scholar] [CrossRef]
  23. Kothari, R.; Mahalkar, P.; Sahu, S.K.; Kundalwal, S.I. Experimental Investigations on Thermal Performance of PCM Based Heat Sink for Passive Cooling of Electronic Components. In Proceedings of the ASME 2018 16th International Conference on Nanochannels Microchannels, and Minichannels, Dubrovnik, Croatia, 10–13 June 2018; American Society of Mechanical Engineers: New York, NY, USA, 2018. [Google Scholar]
  24. Akula, R.; Balaji, C. Characterization of Phase Change Material-Based Heat Sinks for Cyclic Heat Loads. Heat Transf. Eng. 2025, 46, 51–71. [Google Scholar] [CrossRef]
  25. Dutta, P.P.; Saxena, V.; Sahu, S.K. Thermal Performance Analysis of Phase Change Material-Based Plate Finned Heat Sinks for Thermal Management Applications. In International Conference on Advances in Energy Research; Springer Nature: Singapore, 2023; pp. 485–495. [Google Scholar]
  26. Ghouchi, T.; Pourfallah, M.; Gholinia, M. The Thermal and Hydrodynamic Analysis in a Heat Sink with Different Configurations of Shark Scales-Based Fins. Appl. Therm. Eng. 2024, 250, 123492. [Google Scholar] [CrossRef]
  27. Dzikevics, M.; Ansone, A.; Blumberga, D. Modelling of Phase Change in Spheres for Applications in Solar Thermal Heat Storage Systems. Energy Procedia 2016, 95, 112–118. [Google Scholar] [CrossRef]
  28. Cabaleiro, D.; Hamze, S.; Fal, J.; Marcos, M.A.; Estellé, P.; Żyła, G. Thermal and Physical Characterization of PEG Phase Change Materials Enhanced by Carbon-Based Nanoparticles. Nanomaterials 2020, 10, 1168. [Google Scholar] [CrossRef] [PubMed]
  29. Vargas, A.; Huitink, D.; Iradukunda, A.-C.; Eddy, C. Topology Optimized Phase Change Material Integrated Heat Sinks and Validation. In Proceedings of the 2020 19th IEEE Intersociety Conference on Thermal and Thermomechanical Phenomena in Electronic Systems (ITherm), Orlando, FL, USA, 21–23 July 2020; pp. 703–707. [Google Scholar]
  30. Zhang, Y.; Wang, H.; Lu, P.; Fan, J.; Tu, Q.; Liu, J. Heat Transfer Analysis of Phase Change Materials with Metal Foams. In Proceedings of the 2021 22nd International Conference on Electronic Packaging Technology (ICEPT), Xiamen, China, 14–17 September 2021; pp. 1–5. [Google Scholar]
  31. Kant, K.; Biwole, P.H.; Shamseddine, I.; Tlaiji, G.; Pennec, F.; Fardoun, F. Recent Advances in Thermophysical Properties Enhancement of Phase Change Materials for Thermal Energy Storage. Sol. Energy Mater. Sol. Cells 2021, 231, 111309. [Google Scholar] [CrossRef]
  32. Raheem Junaidi, M.A. Influence of Advanced Composite Phase Change Materials on Thermal Energy Storage and Thermal Energy Conversion. J. Therm. Anal. Calorim. 2025. [Google Scholar] [CrossRef]
  33. Wu, Y.; Tang, Y.; Li, Z.; Ding, X.; Yuan, W.; Zhao, X.; Yu, B. Experimental Investigation of a PCM-HP Heat Sink on Its Thermal Performance and Anti-Thermal-Shock Capacity for High-Power LEDs. Appl. Therm. Eng. 2016, 108, 192–203. [Google Scholar] [CrossRef]
  34. Islam, A.; Pandey, A.K.; Saidur, R.; Aljafari, B.; Tyagi, V.V. Advancements in Foam-Based Phase Change Materials: Unveiling Leakage Control, Enhanced Thermal Conductivity, and Promising Applications. J. Energy Storage 2023, 74, 109380. [Google Scholar] [CrossRef]
  35. Hu, X.; Yuan, W.; Zhang, X.; Gong, X.; Shuai, Y.; He, S.; Xing, X. Comparative Studies on Thermal Management Performance of PCM-Based Heat Sinks Filled with Various Height Structured Porous Materials. Appl. Therm. Eng. 2025, 263, 125376. [Google Scholar] [CrossRef]
  36. Boucher, E.A. Porous Materials: Structure, Properties and Capillary Phenomena. J. Mater. Sci. 1976, 11, 1734–1750. [Google Scholar] [CrossRef]
  37. Bianco, N.; Busiello, S.; Iasiello, M.; Mauro, G.M. Finned Heat Sinks with Phase Change Materials and Metal Foams: Pareto Optimization to Address Cost and Operation Time. Appl. Therm. Eng. 2021, 197, 117436. [Google Scholar] [CrossRef]
  38. Zhao, C.Y.; Lu, W.; Tian, Y. Heat Transfer Enhancement for Thermal Energy Storage Using Metal Foams Embedded within Phase Change Materials (PCMs). Sol. Energy 2010, 84, 1402–1412. [Google Scholar] [CrossRef]
  39. Qureshi, Z.A.; Ali, H.M.; Khushnood, S. Recent Advances on Thermal Conductivity Enhancement of Phase Change Materials for Energy Storage System: A Review. Int. J. Heat Mass. Transf. 2018, 127, 838–856. [Google Scholar] [CrossRef]
  40. Wang, G.; Wang, Z.; Lai, L.; Xie, D.; Zhu, Y.; Ding, G.; Xu, Q. Experimental and Numerical Investigation of Hydrothermal Performance of a Microchannel Heat Sink with Pin Fins. Case Stud. Therm. Eng. 2024, 60, 104631. [Google Scholar] [CrossRef]
  41. Wang, G.; Sheng, K.; Wang, Y.; Ding, G.; Xie, D. Thermal Hydraulic Performance of Tree-like Microchannel Heat Sink with High Branching Level Based on the Improved Murray’s Law. Int. J. Heat Mass. Transf. 2024, 231, 125875. [Google Scholar] [CrossRef]
  42. Gohar, O.; Zubair Khan, M.; Bibi, I.; Bashir, N.; Tariq, U.; Bakhtiar, M.; Ramzan Abdul Karim, M.; Ali, F.; Bilal Hanif, M.; Motola, M. Nanomaterials for Advanced Energy Applications: Recent Advancements and Future Trends. Mater. Des. 2024, 241, 112930. [Google Scholar] [CrossRef]
  43. Kazaz, O.; Abu-Nada, E. Thermal Performance of Nano-Architected Phase Change Energetic Materials for a next-Generation Solar Harvesting System. Energy Convers. Manag. 2025, 327, 119541. [Google Scholar] [CrossRef]
  44. Shackelford, J.F.; Han, Y.-H.; Kim, S.; Kwon, S.-H. CRC Materials Science and Engineering Handbook; CRC Press: Boca Raton, FL, USA, 2016; ISBN 9780429130687. [Google Scholar]
  45. Drofenik, U.; Kolar, J.W. A Thermal Model of a Forced-Cooled Heat Sink for Transient Temperature Calculations Employing a Circuit Simulator. IEEJ Trans. Ind. Appl. 2006, 126, 841–851. [Google Scholar] [CrossRef]
  46. Kenisarin, M.; Mahkamov, K. Solar Energy Storage Using Phase Change Materials. Renew. Sustain. Energy Rev. 2007, 11, 1913–1965. [Google Scholar] [CrossRef]
  47. Moeini Sedeh, M.; Khodadadi, J.M. Thermal Conductivity Improvement of Phase Change Materials/Graphite Foam Composites. Carbon 2013, 60, 117–128. [Google Scholar] [CrossRef]
  48. Ma, C.; Wang, J.; Wu, Y.; Wang, Y.; Ji, Z.; Xie, S. Characterization and Thermophysical Properties of Erythritol/Expanded Graphite as Phase Change Material for Thermal Energy Storage. J. Energy Storage 2022, 46, 103864. [Google Scholar] [CrossRef]
  49. Hu, X.; Gong, X. Experimental Study on the Thermal Response of PCM-Based Heat Sink Using Structured Porous Material Fabricated by 3D Printing. Case Stud. Therm. Eng. 2021, 24, 100844. [Google Scholar] [CrossRef]
  50. Ahmed, S.S.; Huang, G.; Gegel, H.; Barker, D. Process Modeling of Ti-6Al-4V SPM Materials. MRS Proc. 1998, 521, 249. [Google Scholar] [CrossRef]
  51. Theodore, L.; Bergman, A.S.L.; Frank, P. Incropera Fundamentals of Heat and Mass Transfer, 7th ed.; John Wiley & Sons: Hoboken, NJ, USA, 2011; ISBN 9781118137253. [Google Scholar]
  52. Selection of Heat Sink Materials. Available online: https://www.ppi-uk.com/news/selection-of-heat-sink-materials/ (accessed on 10 April 2023).
  53. Optimizing Thermal Management with Advanced Heat Sink Solutions. Available online: https://www.jamcorcorp.com/news-and-views/optimizing-thermal-management-with-advanced-heat-sink-solutions.php (accessed on 12 April 2025).
  54. Li, J. Fundamentals of Heat Sink Design: Principles and Best Practices. Available online: https://firstmold.com/tips/heat-sink-design/ (accessed on 15 January 2025).
  55. Anagnostopoulos, A.; Navarro, M.E.; Jiang, Z.; Ding, Y. Enhancing Composite Phase Change Material Thermal Performance by Tuning Phase Change Materials Properties with Nanoparticles. Sol. Energy Mater. Sol. Cells 2025, 288, 113615. [Google Scholar] [CrossRef]
  56. Shodiev, A.; Chouchane, M.; Gaberscek, M.; Arcelus, O.; Xu, J.; Oularbi, H.; Yu, J.; Li, J.; Morcrette, M.; Franco, A.A. Deconvoluting the Benefits of Porosity Distribution in Layered Electrodes on the Electrochemical Performance of Li-Ion Batteries. Energy Storage Mater. 2022, 47, 462–471. [Google Scholar] [CrossRef]
  57. Hu, C.; Jiang, Y.; Chen, S.; Wang, L.; Li, H.; Li, Y.; Tang, D. Copper Foam Reinforced Polymer-Based Phase Change Material Composites for More Efficient Thermal Management of Lithium-Ion Batteries. J. Energy Storage 2023, 73, 108918. [Google Scholar] [CrossRef]
  58. Agarwal, A.; Dintwa, E.; Moorad, K.; Noko, L. Design and Investigation of a Low Cost & Portable Thermal Energy Storage System for Domestic Applications. In Proceedings of the Environment and Water Resource Management/837: Health Informatics/838: Modelling and Simulation/839: Power and Energy Systems; ACTAPRESS: Calgary, AB, Canada, 2016. [Google Scholar]
  59. Agarwal, A.; Kalenga, M.K.W.; Ilunga, M. CFD Simulation of Fluid Flow and Combustion Characteristics in Aero-Engine Combustion Chambers with Single and Double Fuel Inlets. Processes 2025, 13, 124. [Google Scholar] [CrossRef]
  60. Chang, K.-H. Solid Modeling. In Product Design Modeling Using CAD/CAE; Elsevier: Amsterdam, The Netherlands, 2014; pp. 125–167. [Google Scholar]
  61. Agarwal, A.; Rimal, A.; Ilunga, M. Non-Linear Contact Analysis and Response Surface Optimization of Railway Wheel Using ANSYS. Math. Model. Eng. Probl. 2024, 11, 2039–2047. [Google Scholar] [CrossRef]
  62. Agarwal, A.; Dinka, M.O.; Ilunga, M. Enhancing Engine Cylinder Heat Dissipation Capacity Through Direct Optimization (DO) Techniques. Processes 2024, 12, 2659. [Google Scholar] [CrossRef]
  63. Agarwal, A. Numerical Analysis of Solar Collector Absorber Tubes with C- Shaped Roughness for Enhanced Heat Transfer. E3S Web Conf. 2024, 547, 03019. [Google Scholar] [CrossRef]
  64. Carvill, J. Thermodynamics and Heat Transfer. In Mechanical Engineer’s Data Handbook; Elsevier: Amsterdam, The Netherlands, 1993; pp. 102–145. [Google Scholar]
Processes 13 01606 g001
Figure 1. Conceptual schematic illustrating PCM embedded in SPM structure within the heat sink (for explanatory purposes only) [49].
Figure 1. Conceptual schematic illustrating PCM embedded in SPM structure within the heat sink (for explanatory purposes only) [49].
Processes 13 01606 g001
Processes 13 01606 g002
Figure 2. 3D CAD model of heat sink with SPM.
Figure 2. 3D CAD model of heat sink with SPM.
Processes 13 01606 g002
Processes 13 01606 g003
Figure 3. Imported model of heat sink with SPM.
Figure 3. Imported model of heat sink with SPM.
Processes 13 01606 g003
Processes 13 01606 g004
Figure 4. Meshed model of heat sink.
Figure 4. Meshed model of heat sink.
Processes 13 01606 g004
Processes 13 01606 g005
Figure 5. Applied thermal boundary conditions on the heat sink.
Figure 5. Applied thermal boundary conditions on the heat sink.
Processes 13 01606 g005
Processes 13 01606 g006
Figure 6. Temperature distribution plot at 100 s.
Figure 6. Temperature distribution plot at 100 s.
Processes 13 01606 g006
Processes 13 01606 g007
Figure 7. Temperature distribution plot at 200 s.
Figure 7. Temperature distribution plot at 200 s.
Processes 13 01606 g007
Processes 13 01606 g008
Figure 8. Temperature distribution plot at 300 s.
Figure 8. Temperature distribution plot at 300 s.
Processes 13 01606 g008
Processes 13 01606 g009
Figure 9. Temperature distribution plot at 400 s.
Figure 9. Temperature distribution plot at 400 s.
Processes 13 01606 g009
Processes 13 01606 g010
Figure 10. Temperature vs. time curve.
Figure 10. Temperature vs. time curve.
Processes 13 01606 g010
Processes 13 01606 g011
Figure 11. Heat flux vector for entire geometry.
Figure 11. Heat flux vector for entire geometry.
Processes 13 01606 g011
Processes 13 01606 g012
Figure 12. Heat flux contour plot for PCM domain.
Figure 12. Heat flux contour plot for PCM domain.
Processes 13 01606 g012
Processes 13 01606 g013
Figure 13. Temperature distribution plot for PCM domain.
Figure 13. Temperature distribution plot for PCM domain.
Processes 13 01606 g013
Processes 13 01606 g014
Figure 14. Temperature vs. time curve for both PCMs.
Figure 14. Temperature vs. time curve for both PCMs.
Processes 13 01606 g014
Table 2. Grid independence test.
Table 2. Grid independence test.
Number of ElementsTemperature (K)Error Percentage
64,890312.542.25
65,309313.782.21
67,619314.081.81
69,497315.111.19
69,551315.120.89
Table 4. Comparison of maximum temperature results.
Table 4. Comparison of maximum temperature results.
SourceMaximum Temperature (K)Difference (K)
Present Work315.12-
Literature [49]314.15+0.97
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

Share and Cite

MDPI and ACS Style

Agarwal, A.; Kalenga, M.K.W.; Ilunga, M. Thermal Characterization of Structured Porous Materials and Phase Change Composites for Heat Sink Applications. Processes 2025, 13, 1606. https://doi.org/10.3390/pr13051606

AMA Style

Agarwal A, Kalenga MKW, Ilunga M. Thermal Characterization of Structured Porous Materials and Phase Change Composites for Heat Sink Applications. Processes. 2025; 13(5):1606. https://doi.org/10.3390/pr13051606

Chicago/Turabian Style

Agarwal, Abhishek, Michel Kalenga Wa Kalenga, and Masengo Ilunga. 2025. "Thermal Characterization of Structured Porous Materials and Phase Change Composites for Heat Sink Applications" Processes 13, no. 5: 1606. https://doi.org/10.3390/pr13051606

APA Style

Agarwal, A., Kalenga, M. K. W., & Ilunga, M. (2025). Thermal Characterization of Structured Porous Materials and Phase Change Composites for Heat Sink Applications. Processes, 13(5), 1606. https://doi.org/10.3390/pr13051606

Note that from the first issue of 2016, this journal uses article numbers instead of page numbers. See further details here.

Article Metrics

Back to TopTop