Optimization and Engineering Application of In-Seam Borehole Predrainage Technology for Coalbed Methane Based on Response Surface Methodology
Abstract
1. Introduction
2. Fluid–Solid Coupling Extraction Model of Gas-Bearing Coal Body
2.1. Model Assumptions
2.2. Stress Field Control Equation
2.3. Permeability Evolution Equation
2.4. Matrix Gas Diffusion Control Equation
2.5. Fracture Gas Seepage Control Equation
3. Simulation Scheme and Simulation Results
3.1. Simulation Scheme
3.1.1. Geometric Model and Boundary Conditions
3.1.2. RSM Simulation Scheme
3.1.3. Response Target
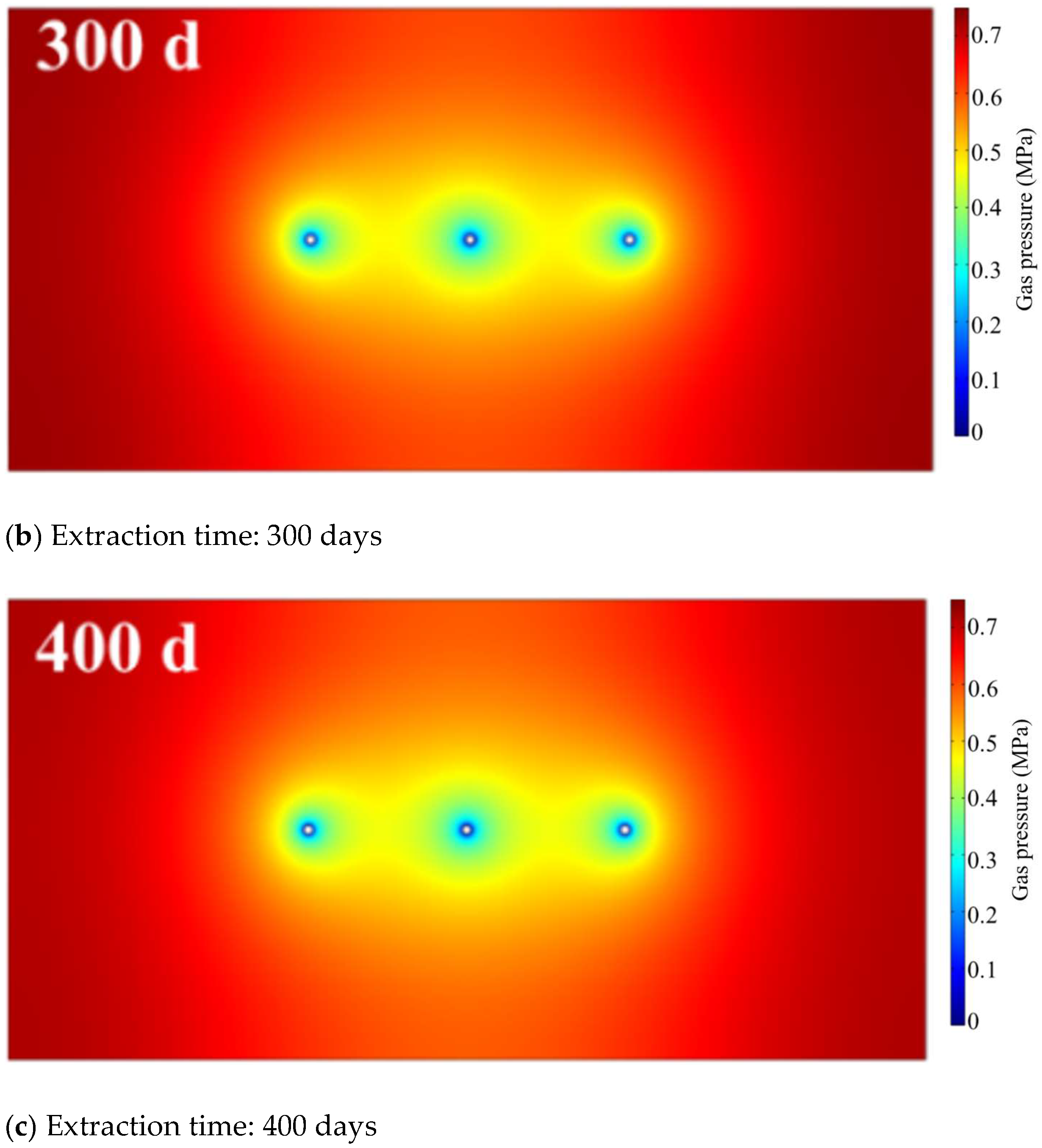
3.2. Simulation Results
3.3. Analysis of Influencing Factors of Coal Seam Gas Pre-Drainage
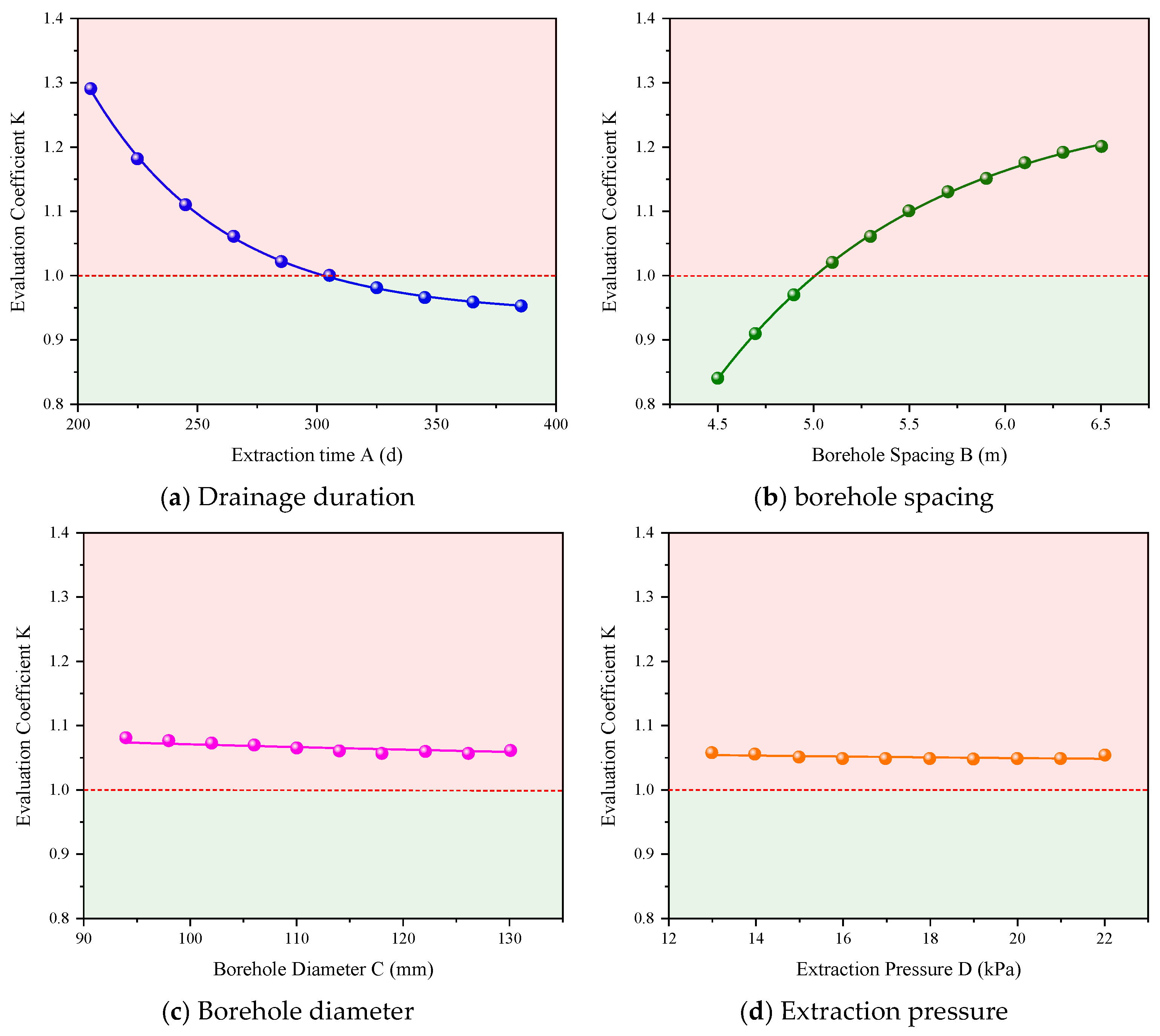
3.3.1. Influence of Single Factors on Extraction Effect
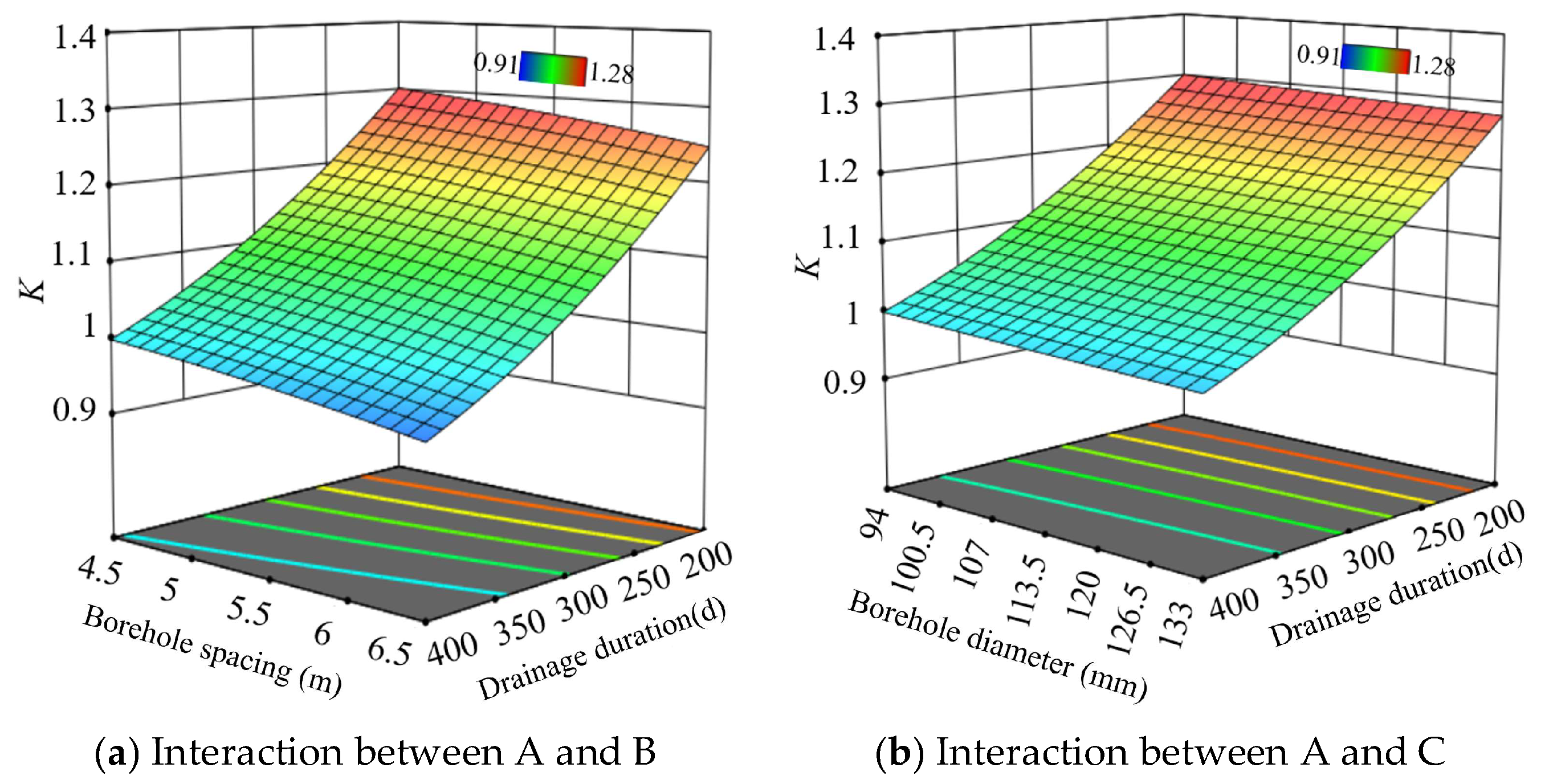
3.3.2. Influence of Interaction Effects on Gas Predrainage Efficiency
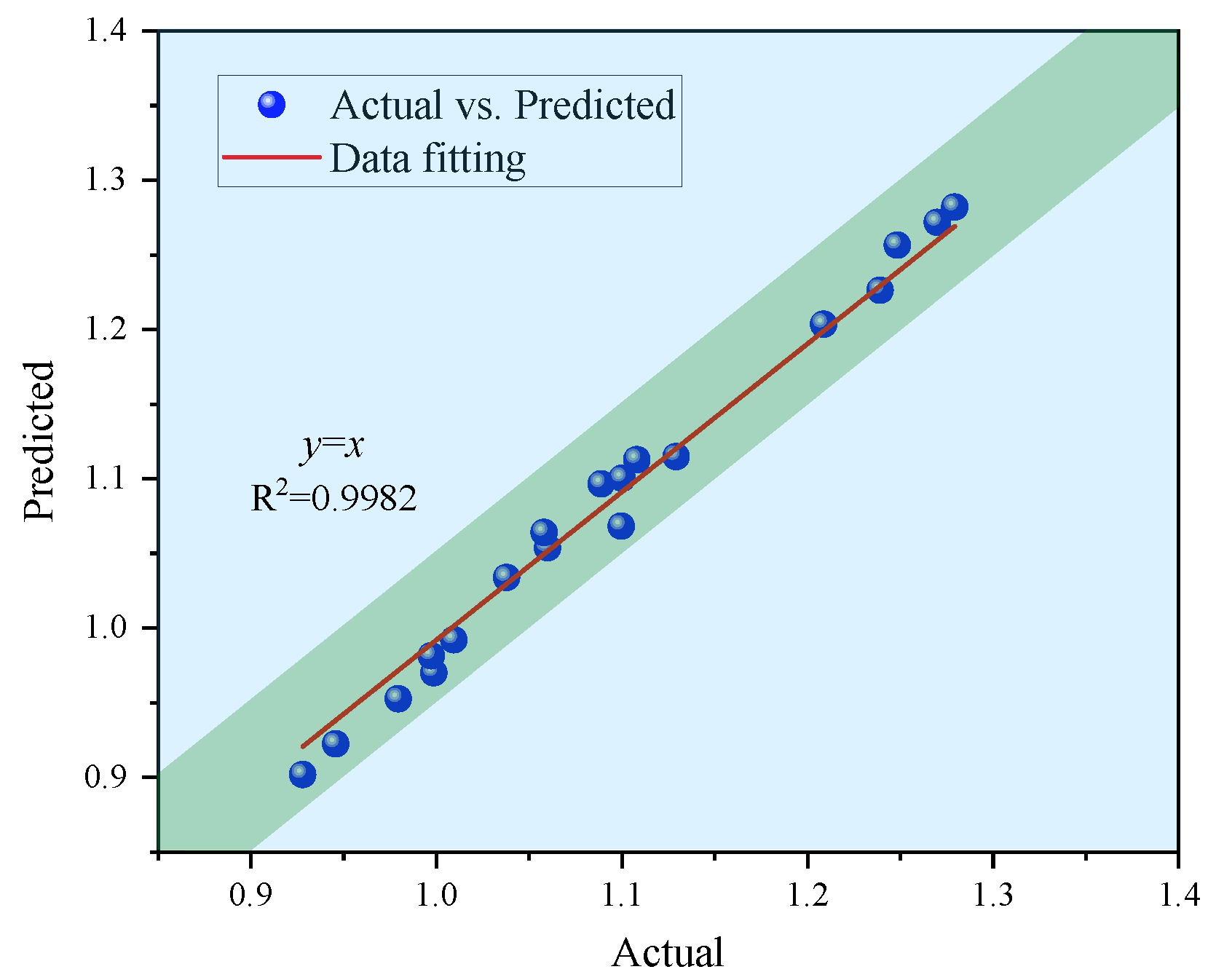
3.3.3. Regression Model and Parameter Optimization
4. Engineering Practice
4.1. Construction Parameters
4.2. Field Application
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Yue, J.; Xu, J.; Zhang, J.; Shi, B.; Zhang, M.; Li, Y.; Wang, C. Gas Displacement Characteristics During the Water Wetting Process of Gas-Bearing Coal and Microscopic Influence Mechanism. Sci. Total Environ. 2024, 949, 175034. [Google Scholar] [CrossRef]
- Shen, S.; Wang, H.; Ren, T.; Wang, Z. Research on the Pore Structure and Gas Adsorption/Desorption Characteristics of Tectonic Coal in Minor Fault Zone: Implications for Coal and Gas Outbursts. Powder Technol. 2025, 456, 120846. [Google Scholar] [CrossRef]
- Guo, J.; Chen, C.; Liu, Y.; Wen, H.; Quan, Y. Response Characteristics of Molecular Active Structure of Coal of Different Metamorphic Degree to Extractant. Energy 2025, 320, 135372. [Google Scholar] [CrossRef]
- Anani, A.; Adewuyi, S.O.; Risso, N.; Nyaaba, W. Advancements in Machine Learning Techniques for Coal and Gas Outburst Prediction in Underground Mines. Int. J. Coal Geol. 2024, 285, 104471. [Google Scholar] [CrossRef]
- Wang, C.; Liu, L.; Li, X.; Xu, C.; Li, K. Mechanism of Gas Pressure Action During the Initial Failure of Coal Containing Gas and its Application for an Outburst Inoculation. Int. J. Min. Sci. Technol. 2023, 33, 1511–1525. [Google Scholar] [CrossRef]
- Yang, G.; Song, D.; Wang, M.; Qiu, L.; He, X.; Khan, M.; Qian, S. New Insights Into Dynamic Disaster Monitoring through Asynchronous Deformation Induced Coal-Gas Outburst Mechanism of Tectonic and Raw Coal Seams. Energy 2024, 295, 131063. [Google Scholar] [CrossRef]
- Li, M.; Lu, S.; Wei, C.; Li, Z.; Ye, Q.; Zhang, Y. Study on Energy Instability Mechanism of Composite Coal Seam Based on the Coupling Effect of Damage and Unsteady Diffusion. Fuel 2025, 379, 133051. [Google Scholar] [CrossRef]
- Song, D.; Liu, Q.; Qiu, L.; Zhang, J.; Majid, K.; Peng, Y.; Zhao, Y.; Wang, M.; Guo, M.; Guo, T. Experimental Study on Resistivity Evolution Law and Precursory Signals in the Damage Process of Gas-Bearing Coal. Fuel 2024, 362, 130798. [Google Scholar]
- Lei, W.; Chai, J.; Zhang, Y.; Ding, G.; Yao, R.; Chen, Y.; Wang, Z.; Ma, C. Study on Pressure Relief Effect of Upper Protective Coal Seam Mining Based on Distributed Optical Fiber Sensing Monitoring. Opt. Fiber Technol. 2022, 68, 102830. [Google Scholar] [CrossRef]
- Jin, Y.; Tong, X.; Zheng, X.; Li, Y.; Dong, B. Risk Assessment of Coal and Gas Outbursts Driven by the Theory of Three Types of Hazards Coupled with 80 Accident Cases. Safety Sci. 2025, 184, 106771. [Google Scholar] [CrossRef]
- Lou, Z.; Wang, K.; Yao, H.; Zhao, W.; Qin, H.; Wu, Z.; Wei, G. A Novel Dynamic Filling Material for Plugging Fractures Around Underground Gas Extraction Boreholes: Experimental and Engineering Performances. Energy 2025, 314, 134202. [Google Scholar] [CrossRef]
- Zha, W.; Lin, B.; Liu, T.; Liu, T.; Yang, W.; Wang, W. Microscopic Mechanism of Gas-Water Two-Phase Flow Based on Low-Field Nuclear Magnetic Resonance. Gas Sci. Eng. 2025, 134, 205538. [Google Scholar] [CrossRef]
- Yuan, M.; Sun, S.; Liu, Y.; Yang, T.; Li, C.; Niu, Y.; Wen, X. Modeling and Control Method of Coal Instability Around Cavity Under Water Jet Impact Loading. Tunn. Undergr. Sp. Tech. 2025, 161, 106574. [Google Scholar] [CrossRef]
- Johnson, R.L. The Implementation of Subterranean Barriers with Mine Pre-Drainage to Reduce Coal Mine Methane Emissions from Open-Cut and Underground Metallurgical-Coal Mines. Int. J. Coal Geol. 2024, 283, 104402. [Google Scholar] [CrossRef]
- Lu, C.; Wang, H.; Tuo, P.; Zhang, X.; Hu, Q. An Evaluation Model of Distribution Uniformity of Sector Directional Boreholes: Application in Coalbed Gas Extraction. Measurement 2025, 253, 117626. [Google Scholar] [CrossRef]
- Li, Q.; Xu, J.; Yan, F.; Peng, S.; Zhang, C.; Zhang, X. Evolution Characteristics of Reservoir Parameters During Coalbed Methane Drainage Via in-Seam Horizontal Boreholes. Powder Technol. 2020, 362, 591–603. [Google Scholar] [CrossRef]
- Liu, W.; Han, D.; Xu, H.; Chu, X.; Qin, Y. Modeling of Gas Migration in a Dual-Porosity Coal Seam Around a Borehole: The Effects of Three Types of Driving Forces in Coal Matrix. Energy 2023, 264, 126181. [Google Scholar] [CrossRef]
- Xing, Y.; Zhang, F. Optimizing Borehole Spacing for Coal Seam Gas Pre-Drainage. J. Geophys. Eng. 2019, 16, 399–410. [Google Scholar] [CrossRef]
- Yan, L.; Wen, H.; Wang, Z.; Jin, Y.; Guo, J.; Liu, Y.; Fan, S. Prediction and Evaluation of Key Parameters in Coalbed Methane Pre-Extraction Based on Transformer and Inversion Model. Eng. Appl. Artif. Intel. 2025, 139, 109661. [Google Scholar] [CrossRef]
- Dong, J.; Cheng, Y.; Jin, K.; Zhang, H.; Liu, Q.; Jiang, J.; Hu, B. Effects of Diffusion and Suction Negative Pressure on Coalbed Methane Extraction and a New Measure to Increase the Methane Utilization Rate. Fuel 2017, 197, 70–81. [Google Scholar] [CrossRef]
- Shang, Y.; Wu, G.; Kong, D.; Wang, Y.; Li, F. Research on Influencing Factors of Effective Gas Extraction Radius in Coal Mine Based on Multiple Linear Regression. Adv. Mater. Sci. Eng. 2022, 2022, 3013647. [Google Scholar] [CrossRef]
- Zhang, C.; Xu, J.; Peng, S.; Li, Q.; Yan, F. Experimental Study of Drainage Radius Considering Borehole Interaction Based on 3D Monitoring of Gas Pressure in Coal. Fuel 2019, 239, 955–963. [Google Scholar] [CrossRef]
- Yuan, M.; Jing, Y.; Lanetc, Z.; Zhuravljov, A.; Soleimani, F.; Si, G.; Armstrong, R.T.; Mostaghimi, P. Modelling Multicomponent Gas Diffusion and Predicting the Concentration-Dependent Effective Diffusion Coefficient of Coal with Application to Carbon Geo-Sequestration. Fuel 2023, 339, 127255. [Google Scholar] [CrossRef]
- Wang, K.; Wang, Y.; Xu, C.; Guo, H.; Xu, Z.; Liu, Y.; Dong, H.; Ju, Y. Modeling of Multi-Field Gas Desorption-Diffusion in Coal: A New Insight Into the Bidisperse Model. Energy 2023, 267, 126534. [Google Scholar] [CrossRef]
- Fan, D.; Yang, C.; Sun, H.; Yao, J.; Zhang, L.; Jia, C.; Fu, S.; Sang, Q. A Framework of Parallel Physics-Informed Neural Network with Laplace Transform for Well Testing Interpretation. Phys. Fluids 2025, 37, 14114. [Google Scholar] [CrossRef]
- Liu, T.; Wang, J.; Lin, B.; Wang, X.; Huang, Q.; Li, J. Numerical Simulation on Enhanced Coalbed Methane Extraction by Hot Flue Gas Based on the Ecm-Dfm Model. Comput. Geotech. 2025, 184, 107253. [Google Scholar] [CrossRef]
- Ahamed, M.A.A.; Perera, M.S.A.; Ranjith, P.G. Implementation of an Elastoplastic Constitutive Model to Study the Proppant Embedment in Coal Under Different Pore Fluid Saturation Conditions: A Numerical and Experimental Study. Fuel 2022, 317, 123488. [Google Scholar] [CrossRef]
- Liu, T.; Lin, B.; Fu, X.; Zhao, Y.; Gao, Y.; Yang, W. Modeling Coupled Gas Flow and Geomechanics Process in Stimulated Coal Seam by Hydraulic Flushing. Int. J. Rock Mech. Min. 2021, 142, 104769. [Google Scholar] [CrossRef]
- Sharma, G.; Guria, C. An Improved Klinkenberg Permeability Model for Tight Reservoir Cores: Effects of Non-Linear Gas Slippage to Real Gases. Geoenergy Sci. Eng. 2024, 233, 212477. [Google Scholar] [CrossRef]
- Zhang, J.; Hou, X.; Liu, S.; Zhu, Y.; Liu, A.; Fan, L.; Wang, Y.; Zhang, H. Quantifying and Modeling of Coal Permeability Spatiotemporal Response: Implications for Gas Recovery and Co2 Sequestration. Fuel 2025, 388, 134561. [Google Scholar] [CrossRef]
- Wang, K.; Wang, Y.; Xu, C.; Li, X.; Guo, H.; Yi, K. Influence of Gas Diffusion in Coal Matrix on Gas Extraction Process: Modeling, Definition, and Quantitative Analysis. Gas Sci. Eng. 2025, 134, 205545. [Google Scholar] [CrossRef]
- Liu, Z.; Han, J.; Yang, H.; Lv, J.; Dong, S. A New Model for Coal Gas Seepage Based on Fracture-Pore Fractal Structure Characteristics. Int. J. Rock Mech. Min. 2024, 173, 105626. [Google Scholar] [CrossRef]
- Mu, D.; Ma, Q.; Zhang, K.; Zhao, J. A Gas-Mechanical-Damage Coupling Model Based on the Tlf-Sph Method and its Application to Gas Seepage in Fractured Coal. Comput. Geotech. 2024, 171, 106352. [Google Scholar] [CrossRef]
- Saber, E.; Qu, Q.; Aminossadati, S.M.; Zhu, Y.; Chen, Z. Horizontal Borehole Azimuth Optimization for Enhanced Stability and Coal Seam Gas Production. Rock Mech. Bull. 2024, 3, 100100. [Google Scholar] [CrossRef]
- Ng, E.C.J.; Ooi, J.B.; Hung, Y.M. Bicriteria Optimization of Subcooled Flow Boiling in Graphene-Coated Microchannels Using Response Surface Methodology. Int. Commun. Heat Mass. 2025, 164, 108914. [Google Scholar] [CrossRef]
- Yang, X.; Ling Du Lu, X.; Zhang, W. Optimization Design of Hydrogen Storage Reactor Based on Response Surface Methodology and Variable Pressure Hydrogen Charging. Int. J. Hydrogen Energ. 2025, 114, 426–439. [Google Scholar] [CrossRef]
- Xu, S.; Luo, J.; Chen, G.; Zhang, H.; Jia, Z.; Lan, Y.; Jiang, C. Influence and Optimization of Urea Injection Parameters on Scr Characteristics Based on Response Surface Methodology. J. Environ. Chem. Eng. 2025, 13, 116170. [Google Scholar] [CrossRef]
- Davraz, M.; Isildar, N.; Kaplan, A.N. Investigation of the Effects of Steam Curing of Concrete at Different Temperatures on Cost and Compressive Strength by Response Surface Methodology. Constr. Build. Mater. 2025, 470, 140589. [Google Scholar] [CrossRef]
- Yang, H.; Miao, D.; Liu, Z.; Zhao, D.; Zhang, M. Study on the Radial Displacement Law and Permeability Enhancement Effect of Coal Body Around Hydraulic Flushing Drilling. Geofluids 2021, 2021, 5535624. [Google Scholar] [CrossRef]
- Zhang, S.; Wang, Z.; Zhao, Y.; Chen, D.; Qiu, Y.; Wei, J. Study on the Design of Gas Extraction Drilling Angle of Crossing Coal Layer Drilling in the Floor Rock Roadway Considering the Boreholes Superposition Effect. Fuel 2024, 378, 132981. [Google Scholar] [CrossRef]
- Fang, H.H.; Zheng, C.S.; Qi, N.; Xu, H.J.; Liu, H.H.; Huang, Y.H.; Wei, Q.; Hou, X.W.; Li, L.; Song, S.L. Coupling Mechanism of Thm Fields and Slg Phases During the Gas Extraction Process and its Application in Numerical Analysis of Gas Occurrence Regularity and Effective Extraction Radius. Petrol. Sci. 2022, 19, 990–1006. [Google Scholar] [CrossRef]
- Yang, F.; Li, P.; Su, W.; Zhang, J.; Wang, L.; Guo, T.; Wang, S. Study on Reasonable Gas Extraction Radius Based on Multi-Index and Multi-Method. Energy Rep. 2022, 8, 287–294. [Google Scholar] [CrossRef]
- Zhang, H.; Cheng, Y.; Liu, Q.; Yuan, L.; Dong, J.; Wang, L.; Qi, Y.; Wang, W. A Novel in-Seam Borehole Hydraulic Flushing Gas Extraction Technology in the Heading Face: Enhanced Permeability Mechanism, Gas Flow Characteristics, and Application. J. Nat. Gas Sci. Eng. 2017, 46, 498–514. [Google Scholar] [CrossRef]
- Wang, K.; Wang, Y.; Xu, C.; Xu, Z.; Guo, H.; Liu, Y.; Dong, H. Transition of Dominated Factors in Coal Seam Gas Migration: Thermo-Hydro-Mechanical Modeling and Analysis. Int. J. Heat Mass Tran. 2025, 236, 126239. [Google Scholar] [CrossRef]
- Zhang, J.; Liu, Y.; Ren, P.; Han, H.; Zhang, S. A Fully Multifield Coupling Model of Gas Extraction and Air Leakage for in-Seam Borehole. Energy Rep. 2021, 7, 1293–1305. [Google Scholar] [CrossRef]
- Liu, T.; Lin, B.; Fu, X.; Zhu, C. Modeling Air Leakage Around Gas Extraction Boreholes in Mining-Disturbed Coal Seams. Process Saf. Environ. 2020, 141, 202–214. [Google Scholar] [CrossRef]
- Wang, K.; Wang, L.; Ju, Y.; Dong, H.; Zhao, W.; Du, C.; Guo, Y.; Lou, Z.; Gao, H. Numerical Study on the Mechanism of Air Leakage in Drainage Boreholes: A Fully Coupled Gas-Air Flow Model Considering Elastic-Plastic Deformation of Coal and its Validation. Process Saf. Environ. 2022, 158, 134–145. [Google Scholar] [CrossRef]
- Zhao, Y.; Qi, Q.; Jia, X.; Liu, Y.; Zhang, J. Numerical Simulation of Coal Spontaneous Combustion Around a Borehole Induced by Negative Pressure Gas Drainage. Geofluids 2021, 2021, 3938243. [Google Scholar] [CrossRef]




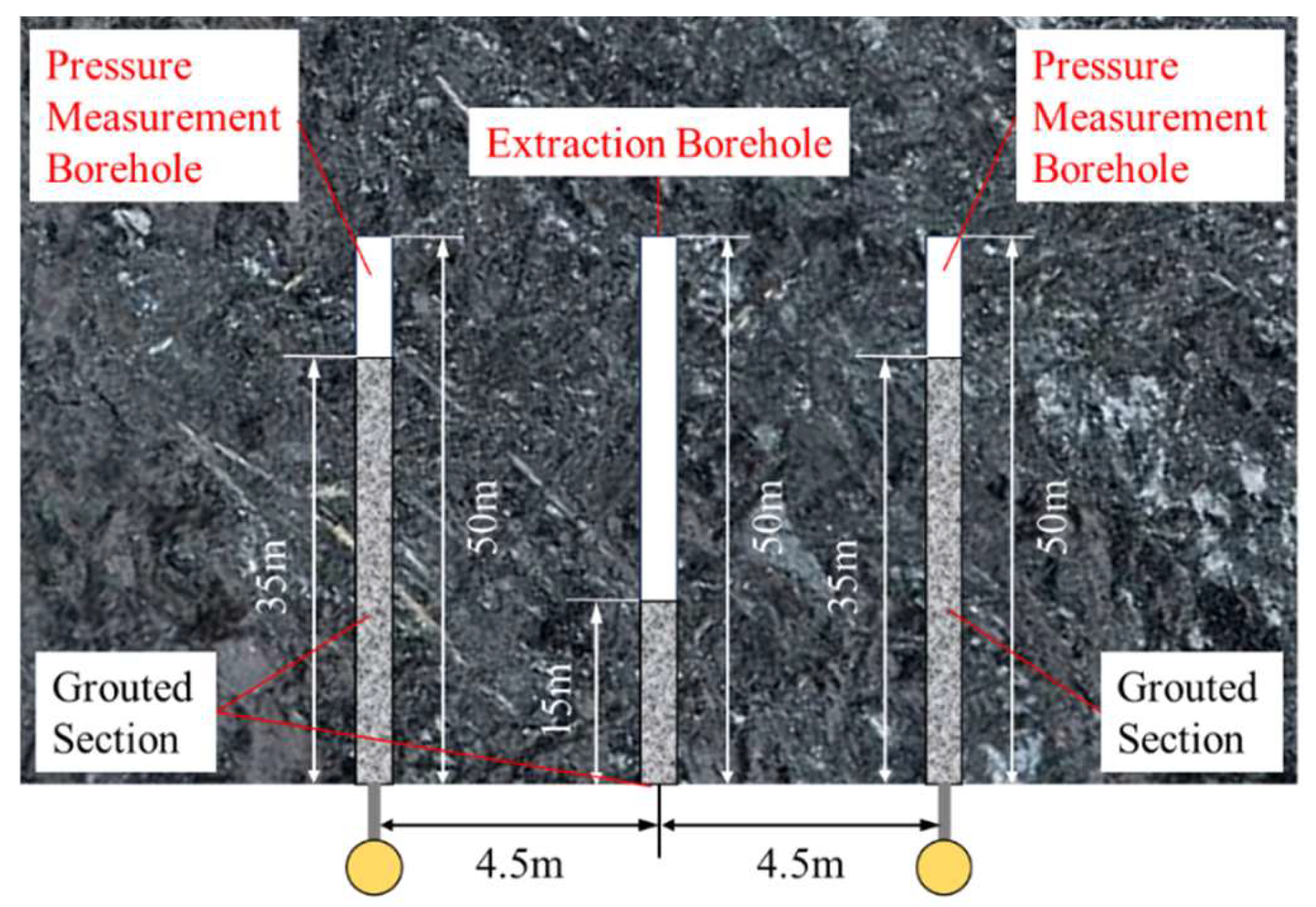

| Parameter | Value | Parameter | Value |
|---|---|---|---|
| Initial gas pressure of coal seam p0/MPa | 1.2 | Poisson’s ratio of coal seam vc | 0.33 |
| Initial permeability of coal seam k0/m2 | 7.6 × 10−16 | Internal friction angle φ/° | 20 |
| Initial fracture porosity of coal seam ϕf | 0.03 | Initial porosity of coal seam ϕm | 0.06 |
| Langmuir pressure PL/MPa | 0.78 | Density of coal ρc/(kg·m−3) | 1330 |
| Langmuir volume VL/(m3·kg−1) | 0.04 | Klinkenberg factor/MPa | 0.14 |
| Dynamic viscosity of methane μ/(Pa·s) | 1.08 × 10−5 | Temperature of coal seam T/K | 298 |
| Variables | Coded | Levels | ||
|---|---|---|---|---|
| −1 | 0 | +1 | ||
| Drainage duration/d | A | 200 | 300 | 400 |
| Borehole spacing/m | B | 5 | 6 | 7 |
| Borehole diameter/mm | C | 94 | 113 | 133 |
| Extraction negative pressure/kPa | D | 13 | 18 | 23 |
| Run | Influencing Factor | K | |||
|---|---|---|---|---|---|
| A | B | C | D | ||
| 1 | 400 | 5.5 | 133 | 18 | 0.93 |
| 2 | 300 | 5.5 | 94 | 13 | 1.10 |
| 3 | 200 | 5.5 | 133 | 18 | 1.24 |
| 4 | 300 | 6.5 | 113.5 | 13 | 1.04 |
| 5 | 400 | 6.5 | 113.5 | 18 | 0.91 |
| 6 | 300 | 5.5 | 133 | 23 | 1.06 |
| 7 | 300 | 5.5 | 113.5 | 18 | 1.07 |
| 8 | 200 | 5.5 | 113.5 | 23 | 1.25 |
| 9 | 300 | 5.5 | 94 | 23 | 1.10 |
| 10 | 300 | 5.5 | 133 | 13 | 1.06 |
| 11 | 200 | 5.5 | 94 | 18 | 1.27 |
| 12 | 300 | 4.5 | 113.5 | 23 | 1.11 |
| 13 | 300 | 4.5 | 94 | 18 | 1.13 |
| 14 | 300 | 5.5 | 113.5 | 18 | 1.07 |
| 15 | 300 | 6.5 | 113.5 | 23 | 1.04 |
| 16 | 300 | 5.5 | 113.5 | 18 | 1.07 |
| 17 | 300 | 5.5 | 113.5 | 18 | 1.07 |
| 18 | 300 | 4.5 | 133 | 18 | 1.09 |
| 19 | 400 | 4.5 | 113.5 | 18 | 0.98 |
| 20 | 300 | 6.5 | 133 | 18 | 0.97 |
| 21 | 200 | 5.5 | 113.5 | 18 | 1.28 |
| 22 | 300 | 6.5 | 94 | 18 | 1.06 |
| 23 | 400 | 5.5 | 94 | 18 | 0.96 |
| 24 | 200 | 6.5 | 113.5 | 18 | 1.21 |
| 25 | 400 | 5.5 | 113.5 | 13 | 0.95 |
| 26 | 300 | 4.5 | 113.5 | 13 | 1.11 |
| 27 | 400 | 6.5 | 113.5 | 23 | 0.95 |
| 28 | 200 | 6.5 | 113.5 | 13 | 1.25 |
| 29 | 300 | 6.5 | 113.5 | 18 | 1.07 |
| Response Model | |
| Coefficient of Determination R2 | 0.9956 |
| Predicted R2 | 0.9749 |
| Adjusted R2 | 0.9913 |
| Coefficient of Variation C. V/% | 0.9041 |
| Accuracy A.P | 54.2100 |
| Source | Sum of Squares | Mean Square | F | p |
|---|---|---|---|---|
| Model | 0.3066 | 0.0219 | 228.55 | <0.0001 |
| A | 0.2760 | 0.2760 | 2880.35 | <0.0001 |
| B | 0.1084 | 0.1084 | 192.09 | <0.0001 |
| C | 0.0061 | 0.0061 | 63.39 | <0.0001 |
| D | 0.0000 | 0.0000 | 0.0082 | 0.9391 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Y.; Liu, Q.; Zhu, C.; Wu, Y. Optimization and Engineering Application of In-Seam Borehole Predrainage Technology for Coalbed Methane Based on Response Surface Methodology. Processes 2025, 13, 1601. https://doi.org/10.3390/pr13051601
Li Y, Liu Q, Zhu C, Wu Y. Optimization and Engineering Application of In-Seam Borehole Predrainage Technology for Coalbed Methane Based on Response Surface Methodology. Processes. 2025; 13(5):1601. https://doi.org/10.3390/pr13051601
Chicago/Turabian StyleLi, Yanhui, Qian Liu, Chuanjie Zhu, and Yue’e Wu. 2025. "Optimization and Engineering Application of In-Seam Borehole Predrainage Technology for Coalbed Methane Based on Response Surface Methodology" Processes 13, no. 5: 1601. https://doi.org/10.3390/pr13051601
APA StyleLi, Y., Liu, Q., Zhu, C., & Wu, Y. (2025). Optimization and Engineering Application of In-Seam Borehole Predrainage Technology for Coalbed Methane Based on Response Surface Methodology. Processes, 13(5), 1601. https://doi.org/10.3390/pr13051601






