Study on Wellbore Stability of Shale–Sandstone Interbedded Shale Oil Reservoirs in the Chang 7 Member of the Ordos Basin
Abstract
1. Introduction
2. Characteristics Analysis of Shale Oil Reservoirs in the Changqing Area
3. Methodological Approach: Triaxial Stress Testing and Rock Failure Criteria
3.1. Triaxial Stress Test of Reservoir Rocks
3.2. Rock Strength Failure Criteria
3.2.1. M–C Criterion
3.2.2. Strength Criterion for Multiple Weak Surfaces in Shale Formation
4. Analysis of Stress State in the Wellbore Surrounding Rock
- (1)
- The formation is transversely isotropic and behaves as a linear elastic medium;
- (2)
- The plane strain condition is satisfied, which is used to calculate the stress distribution around the borehole;
- (3)
- The medium is uniform and continuous.
5. Discussion on Wellbore Stability in Shale–Sandstone Interbedded Reservoirs
5.1. Numerical Model of Shale–Sandstone Interlayer Reservoirs
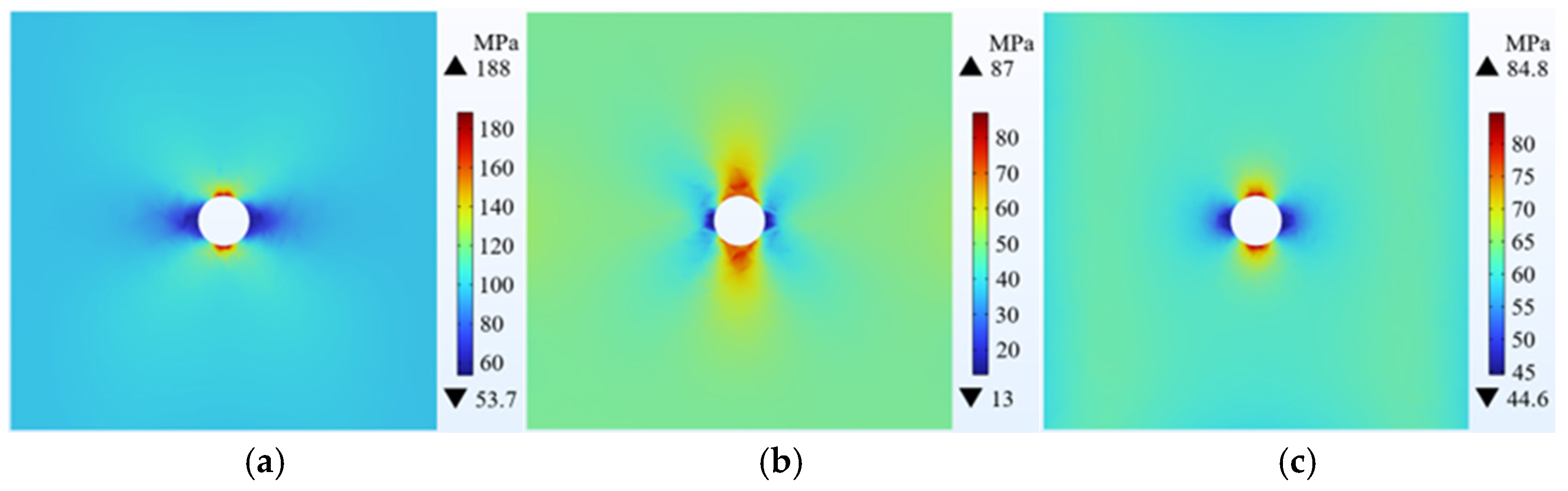
5.2. The Influence of Different Elastic Moduli of Shale–Sandstone on Wellbore Stability
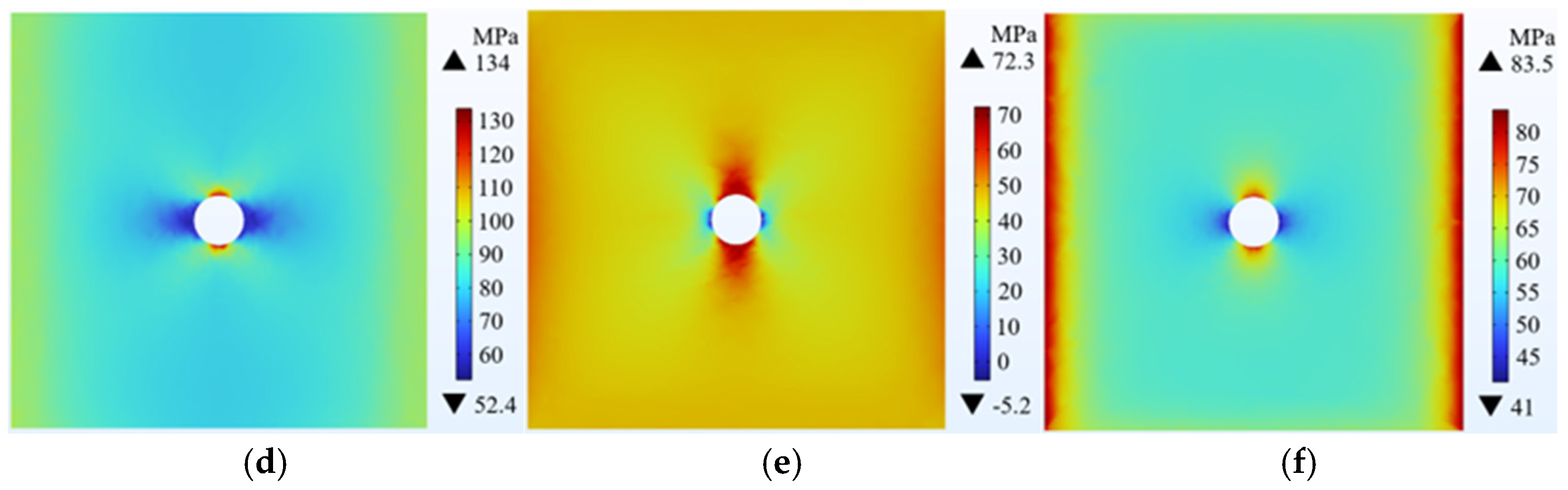
5.3. The Influence of Different Poisson’s Ratios of Shale–Sandstone on Wellbore Stability
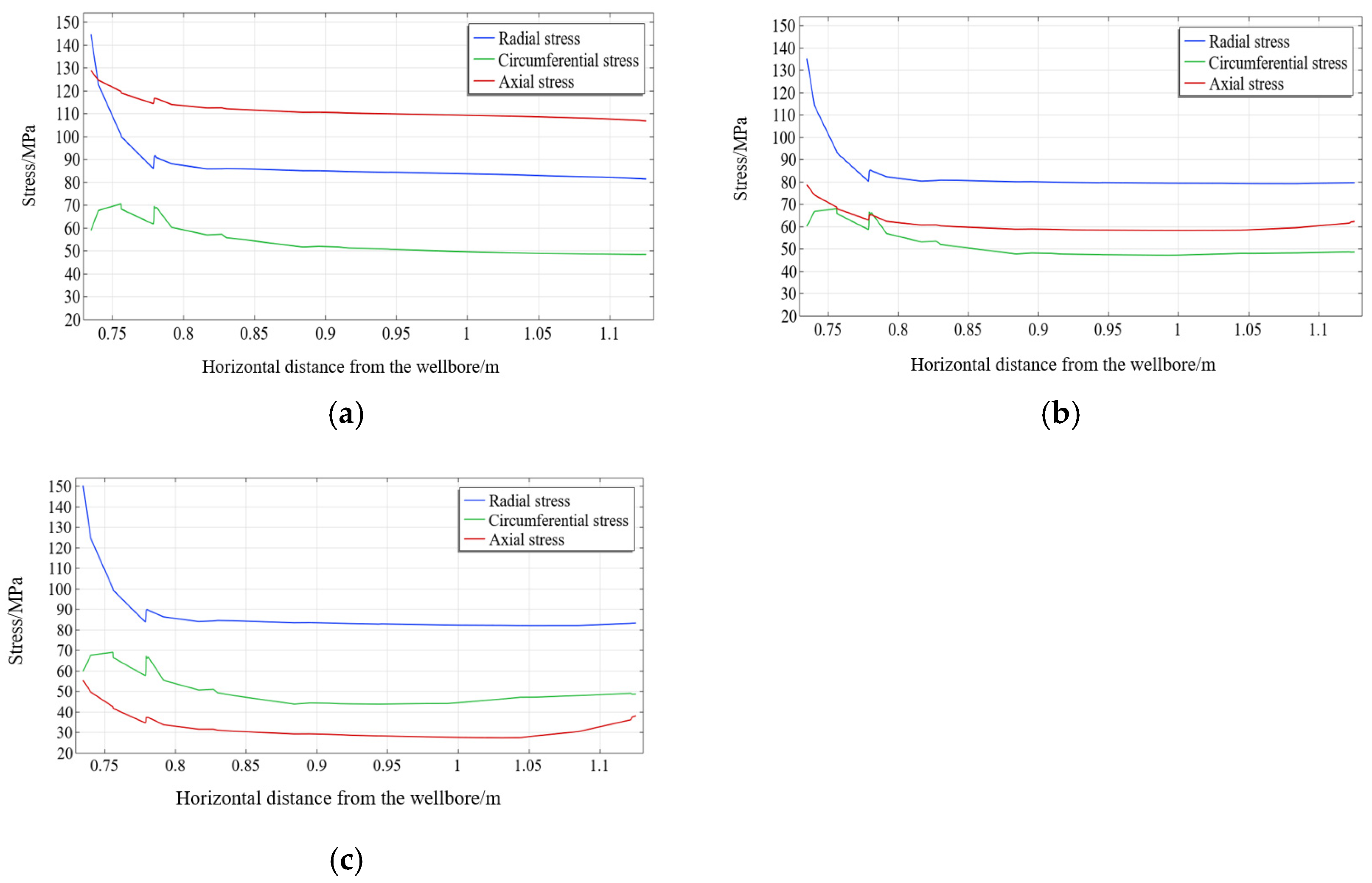
5.4. The Influence of Different Crustal Stresses on Wellbore Stability in Shale–Sandstone Reservoirs
5.5. Potential Applicability to Other Shale–Sandstone Interbedded Reservoirs
6. Conclusions
- (1)
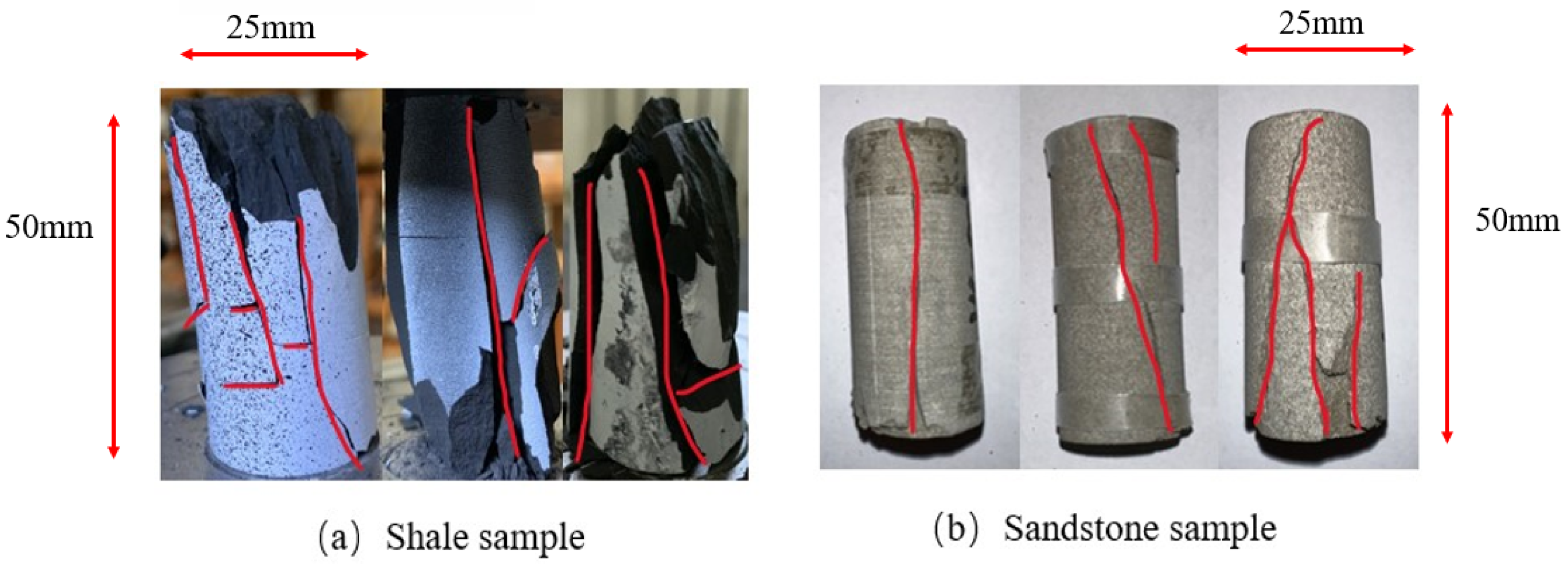
- Under triaxial compression conditions, an increase in confining pressure leads to a shift in the failure characteristics of sandstone specimens towards inclined plane shear failure. This failure mode is primarily characterized by a limited number of macroscopic cracks, exhibiting a uniform morphological configuration. In contrast, shale specimens exhibit a noticeable transition in failure mode, progressing from longitudinal splitting to inclined plane shear as confining pressure increases. The research results indicate these changes in failure behavior.
- (2)
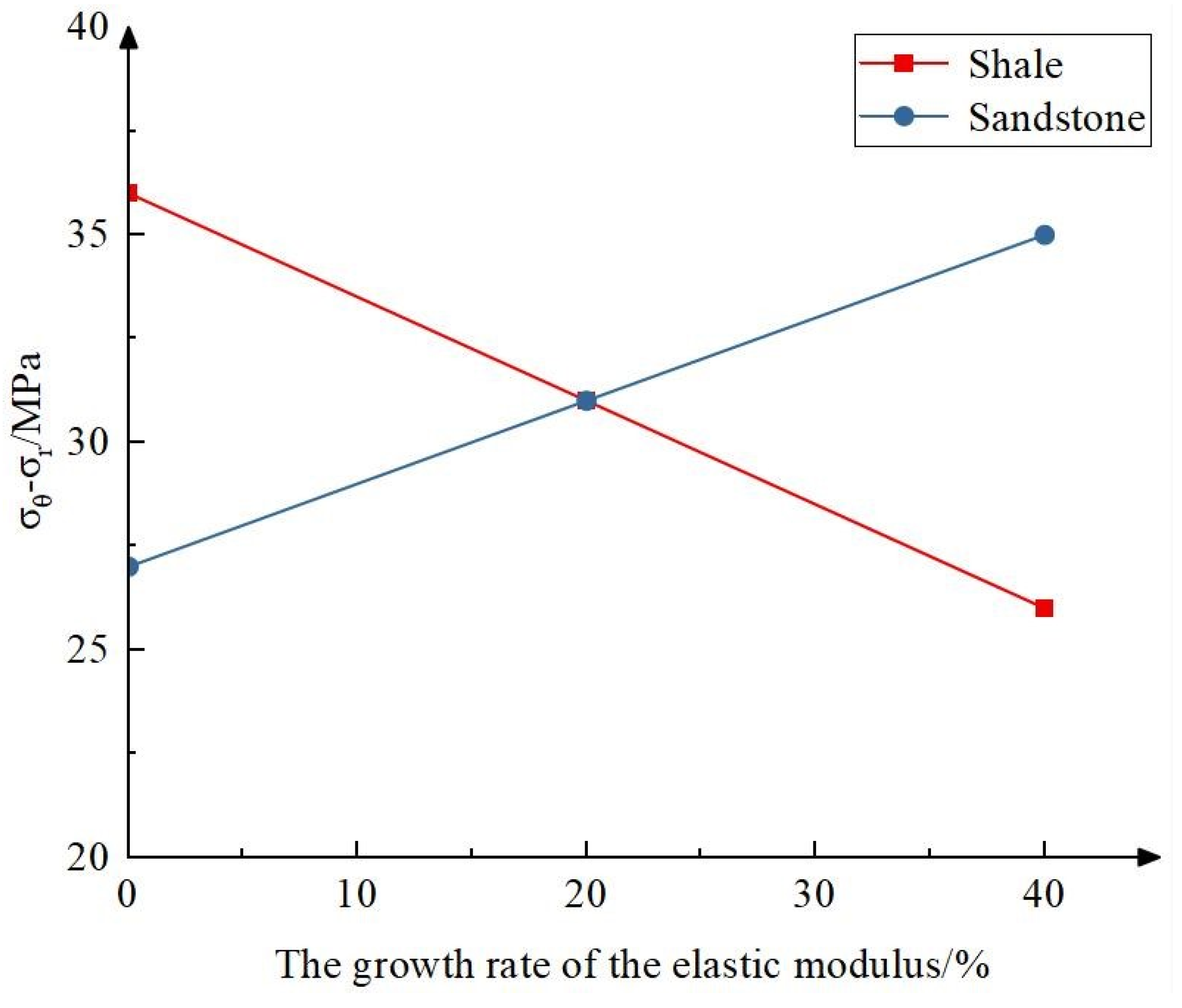
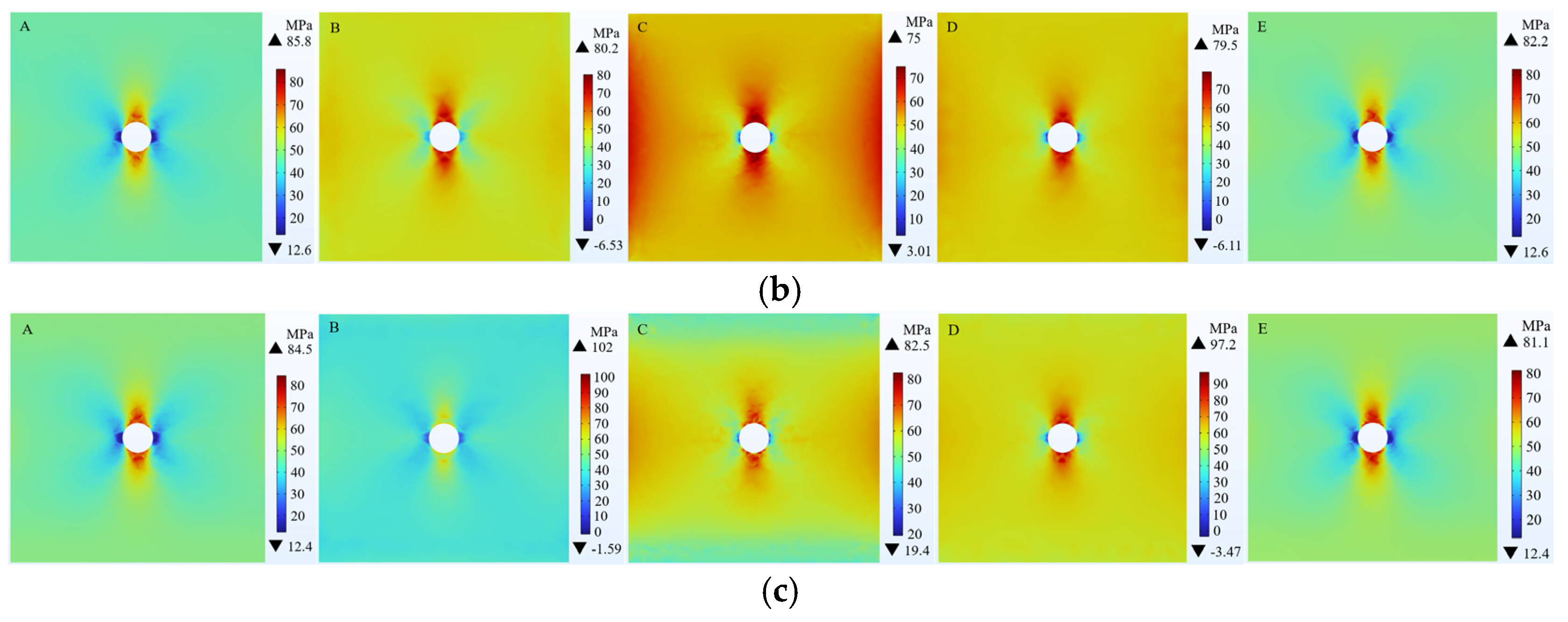
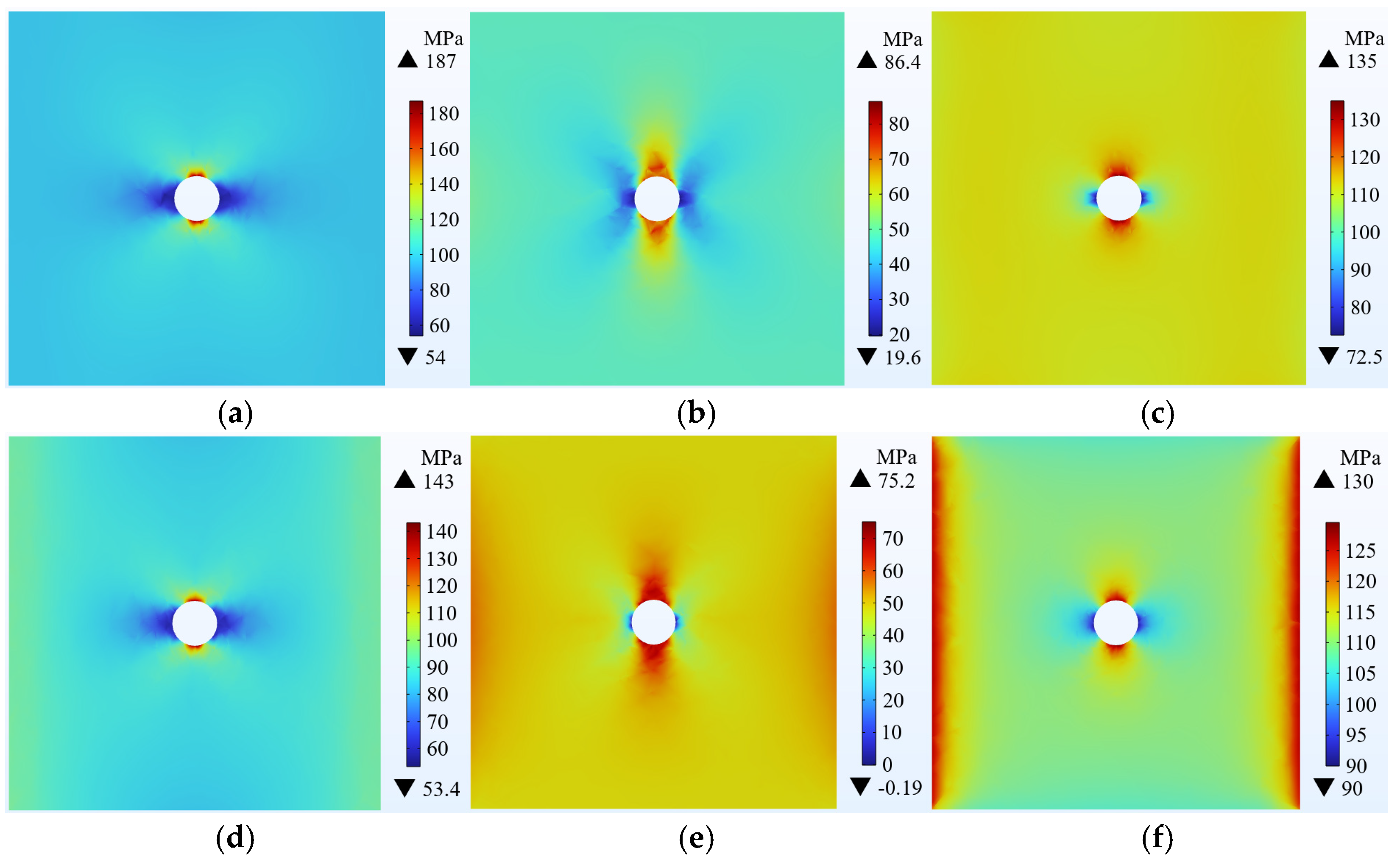
- The study investigated the effect of different elastic modulus between shale and sandstone on wellbore stability. As the elastic modulus of shale increased, the difference between and decreased from 36 MPa to 26 MPa. Conversely, when the elastic modulus of sandstone increased, the difference between and increased from 27 MPa to 35 MPa making the wellbore more prone to instability.
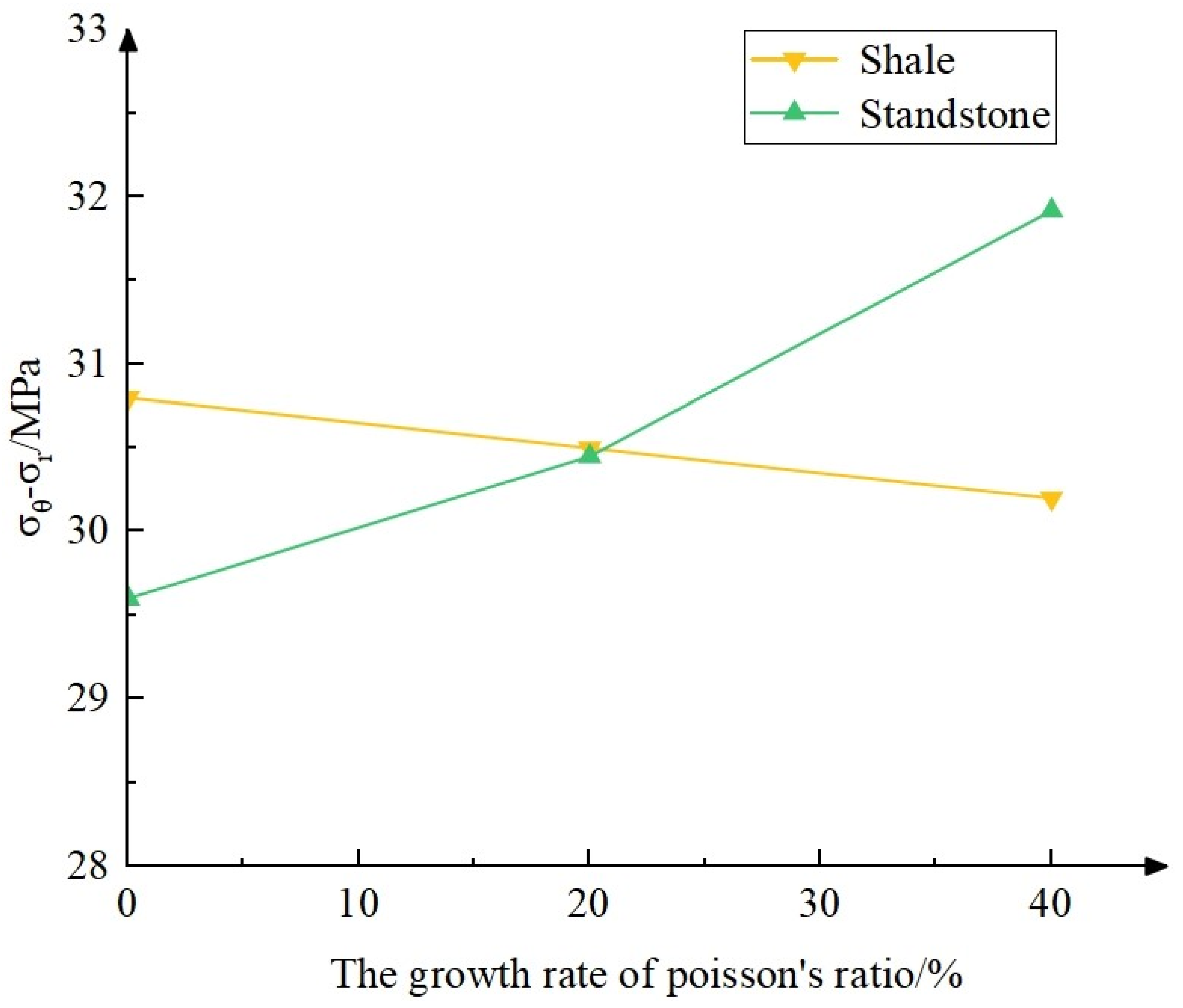
- (3)
- The analysis investigated the impact of varying Poisson’s ratios between shale and sandstone on wellbore stability. With an increase in the Poisson’s ratio of shale, the difference between and decreased from 30.8% to 30.2%. It was observed that changes in the Poisson’s ratio of shale had almost no impact on wellbore stability. However, when the Poisson’s ratio of sandstone increased, the difference between and increased from 29.6 MPa to 32 MPa. This observation suggests a direct correlation between an increase in the Poisson’s ratio of sandstone and an elevated risk of wellbore instability.
- (4)
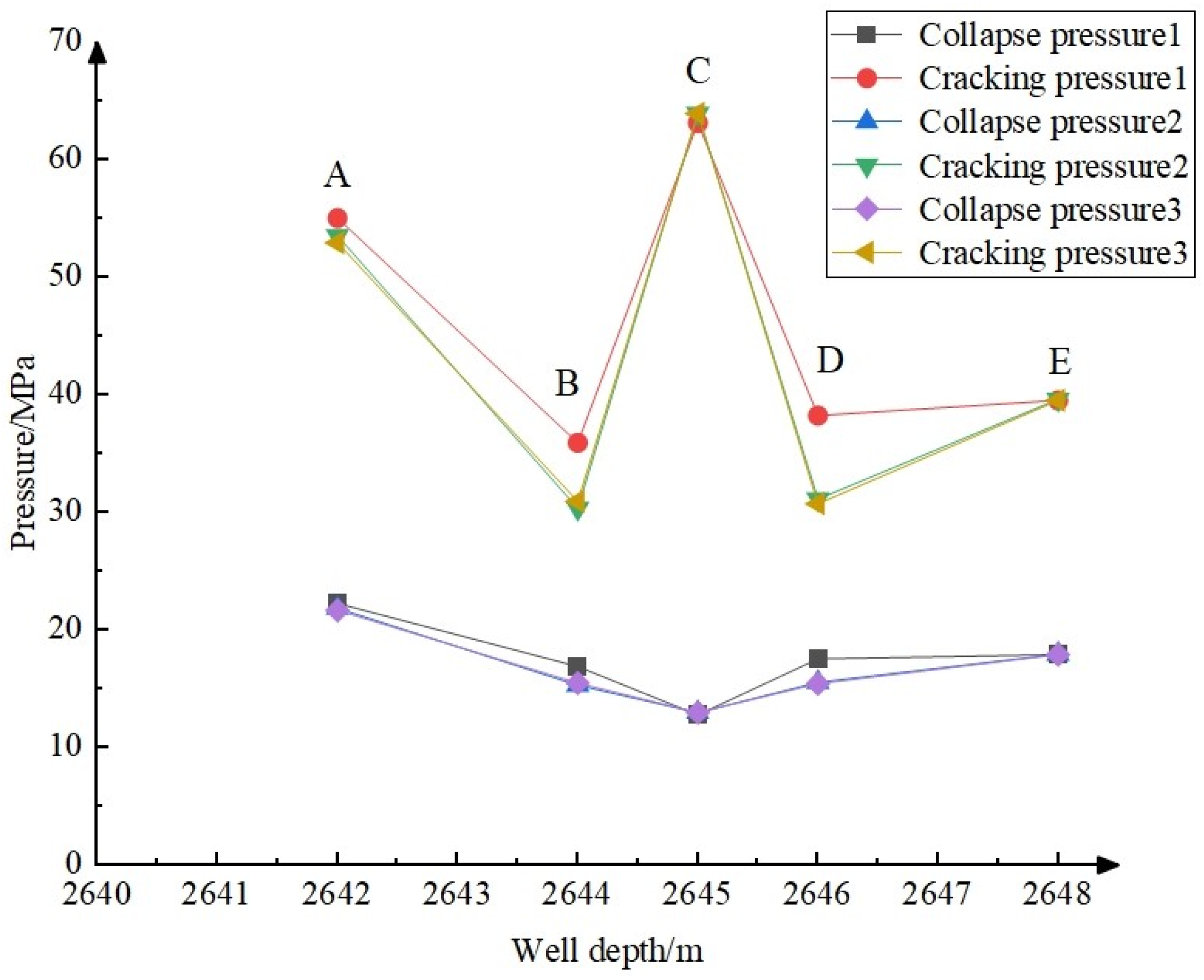
- The collapse pressure and fracture pressure for shale–sandstone were computed across varying elastic modulus and Poisson’s ratios to determine the safe density window. It can be seen that at points B and D, where the shale–sandstone interface is located, the safe density window is smaller to make it more prone to wellbore instability compared to points A, C, and E.
- (5)
- Furthermore, upon analyzing wellbore stability patterns under normal faulting, strike-slip faulting, and reverse faulting crustal stress mechanisms, the analysis indicates that the difference between radial and circumferential stresses becomes significantly larger under the reverse faulting crustal stress mechanism. This notable disparity underscores an increased risk of wellbore collapse and instability.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Fu, S.T.; Jin, Z.J.; Fu, J.H.; Li, S.X.; Yang, W.W. Transformation of understanding from tight oil to shale oil and significance of exploration and development in section 7 of Yanchang Group in Ordos Basin. J. Pet. 2021, 42, 561–569. [Google Scholar]
- Fu, J.; Li, S.; Niu, X.; Deng, X.; Zhou, X. Geological characteristics and progress of exploration and development of shale oil in the long 7 section of the Mesozoic Yanchang Formation in the Ordos Basin. China Pet. Explor. 2019, 24, 601–614. [Google Scholar]
- Yang, Y.; Zheng, X.; Xiao, Y.; Lei, Z.; Xing, H.; Xiong, T.; Liu, M. Progress in exploration and development of high-mature shale oil of PetroChina. China Pet. Explor. 2023, 28, 23. [Google Scholar]
- Cheng, Y.F.; Wang, J.Y.; Zhao, Y.Z.; Shen, M.C. Strength analysis of mud shale formation under multi-field coupling. J. Rock Mech. Eng. 2006, 25, 1912–1916. [Google Scholar]
- Wang, J.Y.; Cheng, Y.F.; Zhao, Y.Z.; Jiang, J.H.; Shen, M.C.; Guo, Y.F. Prediction of safe density window for drilling fluids in mud-shale formations under multi-field coupling. Drill. Complet. Fluids 2007, 24, 1–3. [Google Scholar]
- Niandou, H.; Shao, J.F.; Henry, J.P.; Fourmaintraux, D. Laboratory investigation of the mechanical behaviour of Tournemire shale. Int. J. Rock Mech. Min. Sci. 1997, 34, 3–16. [Google Scholar] [CrossRef]
- Masri, M.; Sibai, M.; Shao, J.F.; Mainguy, M. Experimental investigation of the effect of temperature on the mechanical behavior of Tournemire shale. Int. J. Rock Mech. Min. Sci. 2014, 70, 185–191. [Google Scholar] [CrossRef]
- Dewhurst, D.N.; Siggins, A.F.; Kuila, U.; Clennell, M.B.; Raven, M.D.; Nordgard-Bolas, H.M. Rock Physics, Geomechanics and Rock Properties in Shales—Where are the Links? In SHIRMS 2008: Proceedings of the First Southern Hemisphere International Rock Mechanics Symposium, Perth, Australia, 16–19 September 2008; Australian Centre for Geomechanics: Perth, Australia, 2008; pp. 461–474. [Google Scholar]
- Kuila, U.; Dewhurst, D.N.; Siggins, A.F.; Raven, M.D. Stress anisotropy and velocity anisotropy in low porosity shale. Tectonophysics 2011, 503, 34–44. [Google Scholar] [CrossRef]
- Chen, X.; Yang, Q.; He, M.C.; Li, K.F. Consider the wellbore stability analysis of the anisotropic strength of deep rock mass. J. Rock Mech. Eng. 2005, 16, 2882–2888. [Google Scholar]
- Li, J.; Liu, G.H.; Chen, M. Orthotropic anisotropic formation wellbore surrounding rock stress new model. J. Rock Mech. Eng. 2011, 30, 2481–2485. [Google Scholar]
- Chen, X.; Tan, C.P.; Detournay, C. A study on wellbore stability in fractured rock masses with impact of mud infiltration. J. Pet. Sci. Eng. 2003, 38, 145–154. [Google Scholar] [CrossRef]
- Wen, H.; Chen, M.; Jin, Y.; Wang, K.; Xia, Y.; Dong, J.N.; Niu, C.C. Research on the mechanical-chemical coupling of wellbore stability in brittle mud shale inclined segments. Pet. Explor. Dev. 2014, 41, 748–754. [Google Scholar] [CrossRef]
- Jin, Y.; Bo, K.H.; Zhang, Y.Z.; Lu, Y.H. Progress and considerations in the study of mechanical-chemical coupling for wellbore stability in deep, brittle mud shale. Oil Drill. Technol. 2023, 51, 159–169. [Google Scholar]
- Ding, Y.; Liu, X.J.; Luo, P.Y.; Liang, L.X. Study on the stability of wellbore in brittle mud shale formations. China Offshore Oil Gas 2018, 30, 142–149. [Google Scholar]
- Yu, B.; Yan, C.; Nie, Z. Chemical effect on wellbore instability of Nahr Umr shale. Sci. World J. 2013, 2013, 931034. [Google Scholar] [CrossRef]
- Liu, X.J.; Zeng, W.; Liang, L.X.; Xiong, J. Analysis of the impact of shale bedding on wellbore stability. China Saf. Prod. Sci. Technol. 2016, 12, 88–92. [Google Scholar]
- Ma, T.S.; Chen, P. Stability analysis of bedded shale in horizontal wells. J. Cent. South Univ. (Nat. Sci. Ed.) 2015, 46, 1375–1383. [Google Scholar]
- Lee, H.; Ong, S.H.; Azeemuddin, M.; Goodman, H. A wellbore stability model for formations with anisotropic rock strengths. J. Pet. Sci. Eng. 2012, 96, 109–119. [Google Scholar] [CrossRef]
- Ding, L.Q.; Wang, Z.Q.; Lu, J.G.; Sun, Y.D. Stability model of inclined wells in bedded formations based on the surrounding rock’s Mogi-Coulomb strength criterion. J. Rock Mech. Eng. 2017, 36, 622–632. [Google Scholar]
- Al-Bazali, T.M.; Zhang, J.; Wolfe, C.; Chenevert, M.E.; Sharma, M.M. Wellbore instability of directional wells in laminated and naturally fractured shales. J. Porous Media 2009, 12, 119–130. [Google Scholar] [CrossRef]
- Qiu, Y.; Ma, T.S.; Chen, Y.J.; Y, Y.; Deng, C.S. Evolutionary laws of wellbore stability in unbalanced horizontal wells in argillaceous siltstone reservoirs. J. Cent. South Univ. (Nat. Sci. Ed.) 2023, 54, 967–983. [Google Scholar]
- Zhang, W.; Liu, X.J.; Liang, L.X.; Xiong, J. Experimental study on the stability of boreholes in gas drilling in tight sandstone formations. Oil Drill. Technol. 2023, 51, 37–45. [Google Scholar]
- Wang, X.; Sterling, R.L. Stability analysis of a borehole wall during horizontal directional drilling. Tunn. Undergr. Space Technol. 2007, 22, 620–632. [Google Scholar] [CrossRef]
- Darvishpour, A.; Seifabad, M.C.; Wood, D.A.; Ghorbani, H. Wellbore stability analysis to determine the safe mud weight window for sandstone layers. Pet. Explor. Dev. 2019, 46, 1031–1038. [Google Scholar] [CrossRef]
- Cao, Y.; Wei, B.H.; Deng, J.G.; Yan, C.L.; Yuan, J.L. Analysis of wellbore stability in sandstone formations. Sci. Technol. Her. 2014, 32, 34–36. [Google Scholar]
- Zhao, B.; Hui, J.T.; Xu, Y.; Wang, J.; Sun, Y.Z.; Wang, J. Stress sensitivity of unconsolidated sandstone and its impact on borehole collapse. China Sci. Technol. Pap. 2020, 15, 980–986. [Google Scholar]
- Tan, Q.; Deng, J.G.; Yu, B.H. Study on Borehole Stability of Unconsolidated Sandstone in Depleted Reservior. Adv. Mater. Res. 2013, 616, 720–725. [Google Scholar] [CrossRef]
- Wang, B.Y.; Wei, B.H.; Dang, J.G. Study on the influence of temperature and seepage on the drilling fluid density window in unconsolidated sandstone reservoirs. Drill. Complet. Fluids 2005, 22, 40–42. [Google Scholar]
- Wang, Q.R.; Tao, S.Z.; Guan, P. Progress of Shale Oil research, exploration and development in Continental Basins of China. Nat. Gas Geosci. 2020, 31, 417–427. [Google Scholar]
- Zhou, Z.; Yan, Y.; Ren, S.; Qiao, D.; Guo, T.; Wang, H. Prospects and strategic recommendations for shale oil exploration in the Songliao Basin. China Min. Ind. 2017, 26, 171–174. [Google Scholar]
- Xie, J. Study on Borehole Stability of Horizontal Well in Unconsolidated Sandstone. Bachelor’s Thesis, Changjiang University, Jinzhou, China, 2021. [Google Scholar]






| Sweet Spot | Lithology Diagram | Lithology Combination | Sand Plot Ratio/% | Thickness of Single Sand Body/m |
|---|---|---|---|---|
| Sandwich type |  | The lithology combination of mud shale interbedded with multi-period thin-bedded siltstone. | >20 (general < 30) | <10 |
| Lamination type | 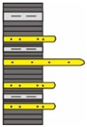 | Develops millimeter-to-centimeter-scale siltstone and mud shale complexes with lamellar structures. | 5~20 | 2~4 |
| Clay pattern | 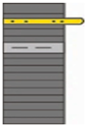 | Deep lake black shale is dominant, with medium to high maturity being the important area for current risk exploration, and medium to low maturity being the main target for in-situ conversion. | <5 | <2 |
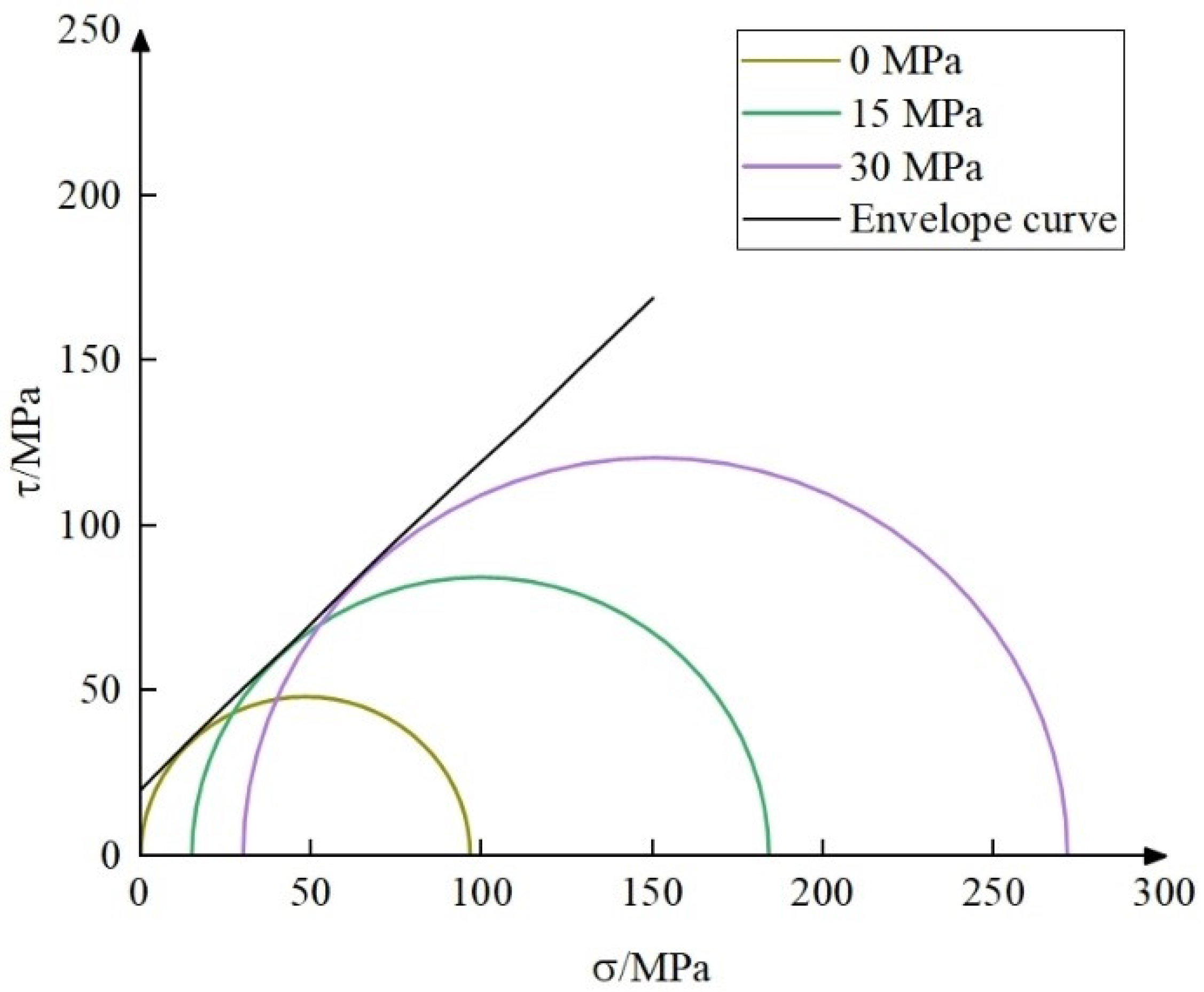
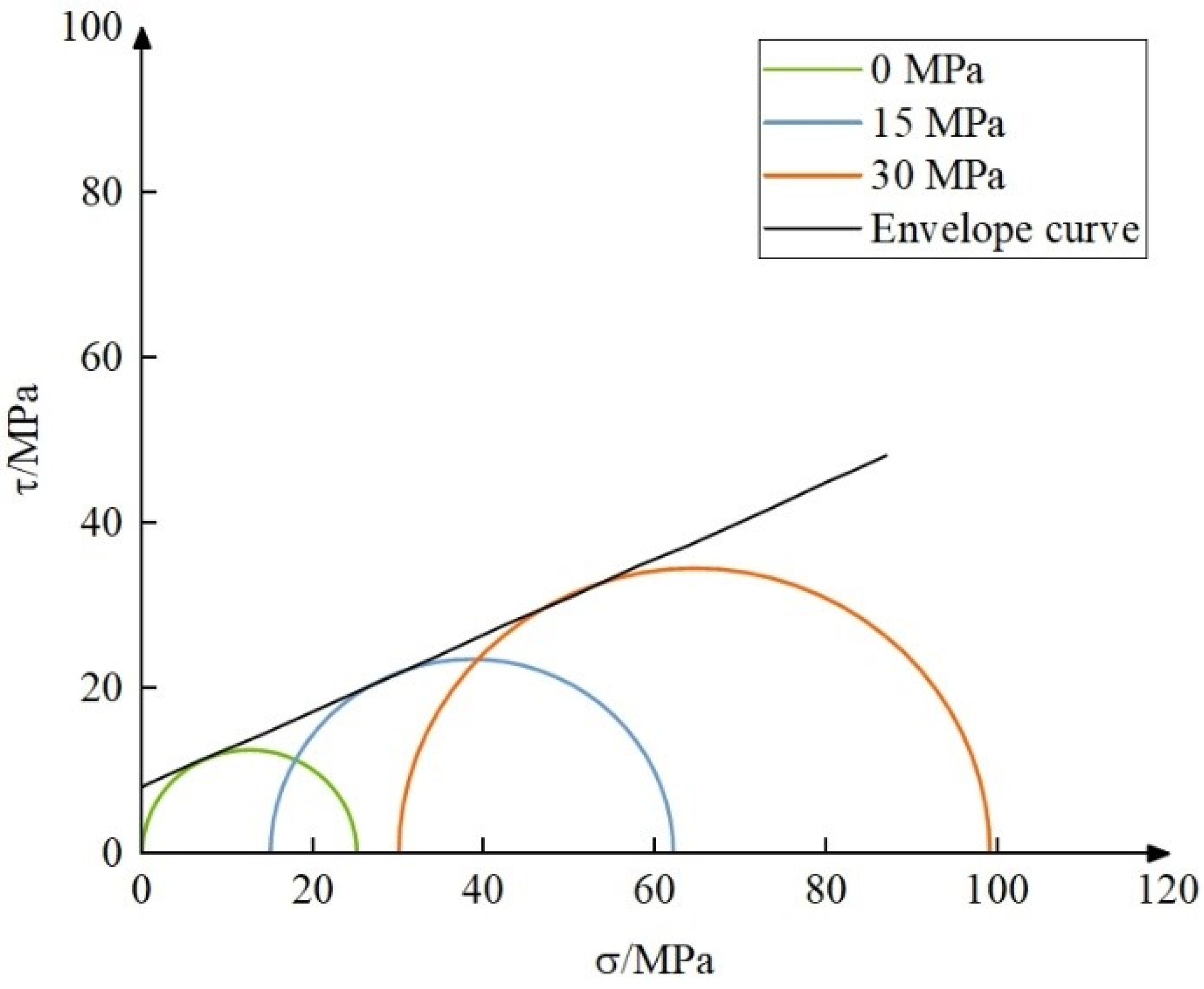
| Rock Types | Sample Number | Pressurization | Compressive Strength (MPa) | Average Value (MPa) | Elastic Modulus (GPa) | Average Value (GPa) | Poisson’s Ratio | Average Value |
|---|---|---|---|---|---|---|---|---|
| Shale | SH-0-1 | 0 | 23.14 | 25.12 | 21.67 | 23.06 | 0.17 | 0.2 |
| SH-0-2 | 24.66 | 22.64 | 0.23 | |||||
| SH-0-3 | 27.56 | 24.86 | 0.20 | |||||
| SH-15-1 | 15 | 59.31 | 62.11 | 26.21 | 24.99 | 0.21 | 0.21 | |
| SH-15-2 | 61.86 | 24.24 | 0.19 | |||||
| SH-15-3 | 65.16 | 24.53 | 0.22 | |||||
| SH-30-1 | 30 | 97.32 | 99.11 | 24.21 | 25.97 | 0.24 | 0.24 | |
| SH-30-2 | 98.68 | 26.27 | 0.23 | |||||
| SH-30-3 | 101.33 | 27.42 | 0.26 | |||||
| Sandstone | ST-0-1 | 0 | 94.56 | 96.52 | 13.12 | 14.14 | 0.23 | 0.22 |
| ST-0-2 | 93.21 | 15.08 | 0.21 | |||||
| ST-0-3 | 101.79 | 14.21 | 0.22 | |||||
| ST-15-1 | 15 | 181.33 | 183.91 | 15.26 | 16.61 | 0.24 | 0.25 | |
| ST-15-2 | 182.69 | 16.32 | 0.23 | |||||
| ST-15-3 | 187.71 | 18.25 | 0.28 | |||||
| ST-30-1 | 30 | 269.89 | 271.3 | 17.67 | 18.08 | 0.24 | 0.27 | |
| ST-30-2 | 270.56 | 18.35 | 0.29 | |||||
| ST-30-3 | 273.45 | 18.22 | 0.27 |
| Parameter Category | Value | Unit |
|---|---|---|
| Well depth | 2840 | m |
| Maximum horizontal stress | 95.06 | MPa |
| Minimum horizontal stress | 48.55 | MPa |
| Overburden pressure | 61.53 | MPa |
| Wellbore pressure | 62.5 | MPa |
| Shale density | 2.6 | g/cm3 |
| Shale cohesion | 8 | MPa |
| Shale friction angle | 25 | ° |
| Sandstone density | 2.5 | g/cm3 |
| Sandstone cohesion | 20 | MPa |
| Sandstone friction angle | 45 | ° |
| Types of Crustal Stress | /MPa | /MPa | /MPa |
|---|---|---|---|
| Normal fault stress | 95.06 | 48.55 | 111.53 |
| Strike-slip fault stress | 95.06 | 48.55 | 61.53 |
| Reverse fault stress | 95.06 | 48.55 | 31.53 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Suo, Y.; Kong, X.; Lyu, H.; Kong, C.; Wang, G.; Wang, X.; Zhou, L. Study on Wellbore Stability of Shale–Sandstone Interbedded Shale Oil Reservoirs in the Chang 7 Member of the Ordos Basin. Processes 2025, 13, 1361. https://doi.org/10.3390/pr13051361
Suo Y, Kong X, Lyu H, Kong C, Wang G, Wang X, Zhou L. Study on Wellbore Stability of Shale–Sandstone Interbedded Shale Oil Reservoirs in the Chang 7 Member of the Ordos Basin. Processes. 2025; 13(5):1361. https://doi.org/10.3390/pr13051361
Chicago/Turabian StyleSuo, Yu, Xuanwen Kong, Heng Lyu, Cuilong Kong, Guiquan Wang, Xiaoguang Wang, and Lingzhi Zhou. 2025. "Study on Wellbore Stability of Shale–Sandstone Interbedded Shale Oil Reservoirs in the Chang 7 Member of the Ordos Basin" Processes 13, no. 5: 1361. https://doi.org/10.3390/pr13051361
APA StyleSuo, Y., Kong, X., Lyu, H., Kong, C., Wang, G., Wang, X., & Zhou, L. (2025). Study on Wellbore Stability of Shale–Sandstone Interbedded Shale Oil Reservoirs in the Chang 7 Member of the Ordos Basin. Processes, 13(5), 1361. https://doi.org/10.3390/pr13051361






