Study on Mushy Zone Coefficient in Solidification Heat Transfer Mathematical Model of Thin Slab with High Casting Speed
Abstract
:1. Introduction
2. Experiment and Simulation
2.1. Basic Assumptions
- (1)
- The flow process of molten steel is a viscous incompressible fluid, and the effects of the phase change, vibration, protective slag and solidification shrinkage are not considered.
- (2)
- The effects of the fluctuation of the mold meniscus, taper, phase transformation of Fe-C alloy, solidified shell shrinkage and vibration on solidification heat transfer are ignored.
- (3)
- The continuous casting process is regarded as a steady state, and the turbulent effect of molten steel is simulated by the low Reynolds number standard k-ε model.
- (4)
- The mushy zone is regarded as a porous medium, and the flow in the mushy zone obeys Darcy’s law.
- (5)
- The movement speed of the solidified shell is consistent with the casting speed.
2.2. Governing Equation
2.3. Boundary Conditions and Physical Parameters
- (1)
- The superheat of molten steel is 20 K, and the inlet temperature of molten steel is 1823 K, and it is kept constant.
- (2)
- Due to the good heat preservation effect of the liquid surface mold slag, the free liquid surface is set as the adiabatic condition, and the submerged entry nozzle (SEN) wall is also set as the adiabatic wall.
- (3)
- The temperature gradient perpendicular to the symmetric plane is zero.
- (4)
- The moving speed of a narrow face and wide face is consistent with the casting speed, which is given by Patch. The boundary heat flux of the mold area is given according to the real-time monitoring data of the actual production computer; that is, the boundary condition of the mold is defined by the second type of boundary condition of heat transfer.
2.4. Grid and Computing Domain
2.5. Numerical Methods
2.6. Test Scheme
3. Results and Discussion
3.1. Description of the Effect of Amush on Heat Transfer and Solidification of Molten Steel
3.2. Amush Parameter Determination and Heat Transfer Solidification Model
3.2.1. Election of Amush Parameters
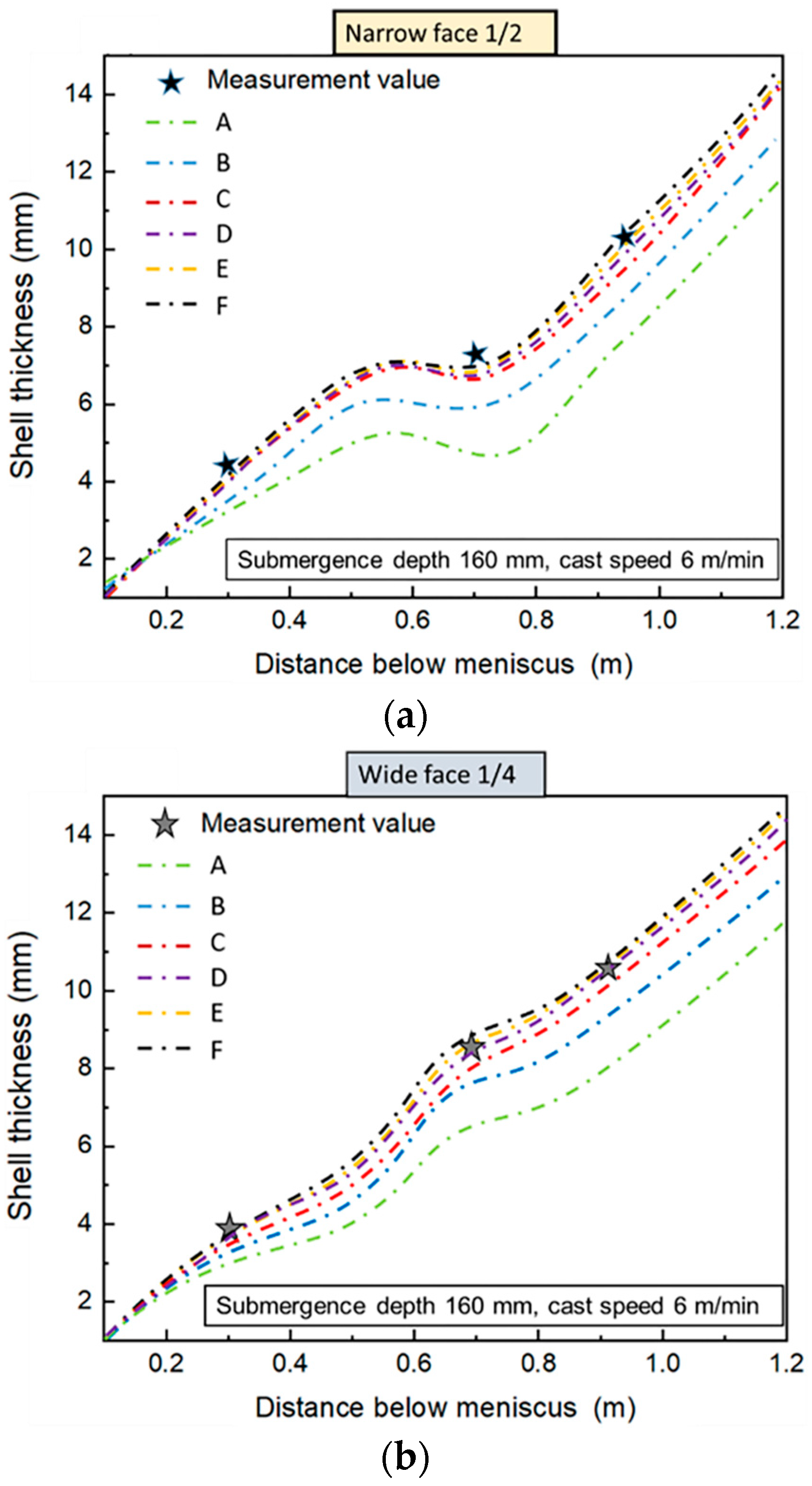
3.2.2. Verification of the Heat Transfer Solidification Model
4. Conclusions
- The smaller the mushy zone coefficient is, the higher the liquid–solid-phase ratio of steel near the liquid level, which is inconsistent with the actual situation. The larger the mushy zone between the solid and liquid in the front of the solidified shell, the thinner the solidified shell. The larger the mushy zone coefficient is, the lower the solid-phase ratio near the liquid surface, and the smaller the mushy zone area is, the thicker the solidified shell.
- The mushy zone coefficient of 3 × 108~9 × 108 kg/(m3⋅s) can reliably reveal the actual solidification phenomenon in the mold of thin slab continuous casting at high casting speed.
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Xuan, M.; Chen, M. Numerical investigation for the influence of turbulent heat transfer of mushy zone on shell growth in the slab mold: Casting and solidification. ISIJ Int. 2022, 62, 142–148. [Google Scholar] [CrossRef]
- Ghaemifar, S.; Mirzadeh, H. Refinement of banded structure via thermal cycling and its effects on mechanical properties of dual phase steel. Steel Res. Int. 2018, 89, 1700531. [Google Scholar] [CrossRef]
- Fujisaki, K. Magnetohydrodynamic solidification calculation in Darcy flow. IEEE Trans. Magn. 2003, 39, 3541. [Google Scholar] [CrossRef]
- Sun, H.B.; Zhang, J.Q. Study on the macrosegregation behavior for the bloom continuous casting: Model development and validation. Metall. Mater. Trans. 2014, 45, 1133. [Google Scholar] [CrossRef]
- Masud, A.; Hughes, T.J.R. A stabilized mixed finite element method for darcy flow. Comput. Methods Appl. Mech. Eng. 2002, 191, 4341–4370. [Google Scholar] [CrossRef]
- To, V.T.; To, Q.D.; Monchiet, V. On the Inertia Effects on the Darcy Law: Numerical Implementation and Confrontation of Micromechanics-Based Approaches. Transp. Porous Media 2016, 111, 171–191. [Google Scholar] [CrossRef]
- Bernardi, C.; Maarouf, S.; Yakoubi, D. Spectral discretization of darcy’s equations coupled with the heat equation. IMA J. Numer. Anal. 2015, 36, 1193–1216. [Google Scholar] [CrossRef]
- Li, H.M.; Liang, Y.F.; Chen, J.F.; Li, H.G.; Chen, X.Q. Relationship between pore structural characteristics and physical-mechanical properties of sandstone in shendong mining area. J. Henan Polytech. Univ. 2018, 37, 9–16. [Google Scholar]
- Kumar, S.S.; Rubio, E.J.; Noor-A-Alam, M.; Martinez, G.; Manandhar, S.; Shutthanandan, V. Structure, morphology, and optical properties of amorphous and nanocrystalline gallium oxide thin films. J. Phys. Chem. C 2013, 117, 4194–4200. [Google Scholar] [CrossRef]
- Pfeiler, C.; Thomas, B.G.; Wu, M. Solidification and particleentrapment during continuous casting of steel. Steel Res. Int. 2008, 79, 599–607. [Google Scholar] [CrossRef]
- Pacella, H.E.; Eash, H.J.; Frankowski, B.J.; Federspiel, W.J. Darcy permeability of hollow fiber bundles used in blood oxygenation devices. J. Membr. Sci. 2011, 382, 238–242. [Google Scholar] [CrossRef] [PubMed]
- Gu, J.P.; Beckermann, C. Simulation of convection and macroseg-regation in a large steel ingot. Metall. Mater. Trans. A 1999, 30, 1357–1366. [Google Scholar] [CrossRef]
- Aboutalebi, M.R.; Guthrie, R.I.L.; Seyedein, S.H. Mathematical modeling of coupled turbulent flotw and solidification in a single belt caster with electromagnetic brake. Appl. Math. Model. 2007, 31, 1671–1689. [Google Scholar] [CrossRef]
- Madhani, S.P.; D’Aloiso, B.D.; Frankowski, B.; Federspiel, W.J. Darcy permeability of hollow fiber membrane bundles made from membrana polymethylpentene fibers used in respiratory assist devices. Asaio J. 2016, 62, 329–331. [Google Scholar] [CrossRef]
- Valdes-Parada, F.J.; Ochoa-Tapia, J.A.; Alvarez-Ramirez, J. Validity of the permeability carman–kozeny equation: A volume averaging approach. Phys. A Stat. Mech. Appl. 2009, 388, 789–798. [Google Scholar] [CrossRef]
- Costa, A. Permeability-porosity relationship: A reexamination of the kozeny-carman equation based on a fractal pore-space geometry assumption. Geophys. Res. Lett. 2006, 33, 5134. [Google Scholar] [CrossRef]
- Ceretani, A.N.; Tarzia, D.A. Determination of one unknown thermal coefficient through a mushy zone model with a convective overspecified boundary condition. Math. Probl. Eng. 2015, 21, 637852. [Google Scholar] [CrossRef]
- Peng, P.; Lu, L.; Zheng, W.; Wang, J. Macrosegregation and channel segregation formation of faceted mushy zone in solidification of sn-ni hypereutectic alloy in a temperature gradient. Mater. Chem. Phys. 2021, 264, 124447. [Google Scholar] [CrossRef]
- Sun, H.B.; Zhang, J.Q. Effect of feeding modes of molten steel on the mould metallurgical behavior for round bloom casting. ISIJ Int. 2011, 51, 1657. [Google Scholar] [CrossRef]
- He, M.L.; Wang, N.; Chen, M.; Xuan, M. Physical and numerical simulation of the fluid flow and temperature distribution in bloom continuous casting mold. Steel Res. Int. 2017, 88, 1600447. [Google Scholar] [CrossRef]
- Trindade, L.B.; Nadalon, J.E.A.; Contini, A.C.; Barroso, R.C. Modeling of solidification in continuous casting round billet with mold electromagnetic stirring (M-EMS). Steel Res. Int. 2017, 88, 1600319. [Google Scholar] [CrossRef]
- Mohamed, F.; Eames, P.C. Numerical investigation of the influence of mushy zone parameter Amush on heat transfer characteristics in vertically and horizontally oriented thermal energy storage systems. Appl. Therm. Eng. 2019, 151, 90–99. [Google Scholar]
- Won, Y.M.; Thomas, B.G. Simple model of microsegregation during solidification of steels. Metall. Mater. Trans. A 2001, 32, 1755–1767. [Google Scholar] [CrossRef]
- Hietanen, P.T.; Louhenkilpi, S.; Yu, S. Investigation of solidification, heat transfer and fluid flow in continuous casting of steel using an advanced modeling approach. Steel Res. Int. 2017, 88, 1600355. [Google Scholar] [CrossRef]
- Ji, Y.; Tang, H.Y.; Lan, P.; Shang, C.; Zhang, J. Effect of dendritic morphology and central segregation of billet castings on the microstructure and mechanical property of hot-rolled wire rods. Steel Res. Int. 2017, 88, 1600426. [Google Scholar] [CrossRef]
- Wang, Q.Q. Stundy on the Multiphase Flow, Heat Transfer and Solidification, Motion and Entrapment of Inclusions During Continuous Casting; University of Science and Technology: Beijing, China, 2016. [Google Scholar]
- Yue, Q.; Zhou, L.; Wang, J.J. Numerical simulation of the coupling of molten Steel flow, heat transfer and solidification in a slab mold with Double transverse Stability Electromagnetic Braking. J. Process Eng. 2011, 11, 187–192. [Google Scholar]
- Zhang, L.M.; Xiao, P.C.; Wang, Y.; Liu, Z.X.; Zhu, L.G. Effect of EMBr on flow in slab continuous casting mold and industrial experiment of nail dipping measurement. Metals 2023, 13, 167. [Google Scholar] [CrossRef]
- Yang, C.L. Simulation Research on Solidification Heat Transfer and Film Formation of Protective Slag in Continuous Casting Mold; Chongqing University: Chongqing, China, 2018. [Google Scholar]
- Alizadeh, M. Correlation between the continuous casting parameters and secondary dendrite arm spacing in the mold region. Mater. Lett. 2013, 91, 146–149. [Google Scholar] [CrossRef]
- Minakawa, S.; Samarasekera, I.V.; Weinberg, F. Centerline porosityin plate castings. Metall. Trans. B 1985, 16, 823–829. [Google Scholar] [CrossRef]
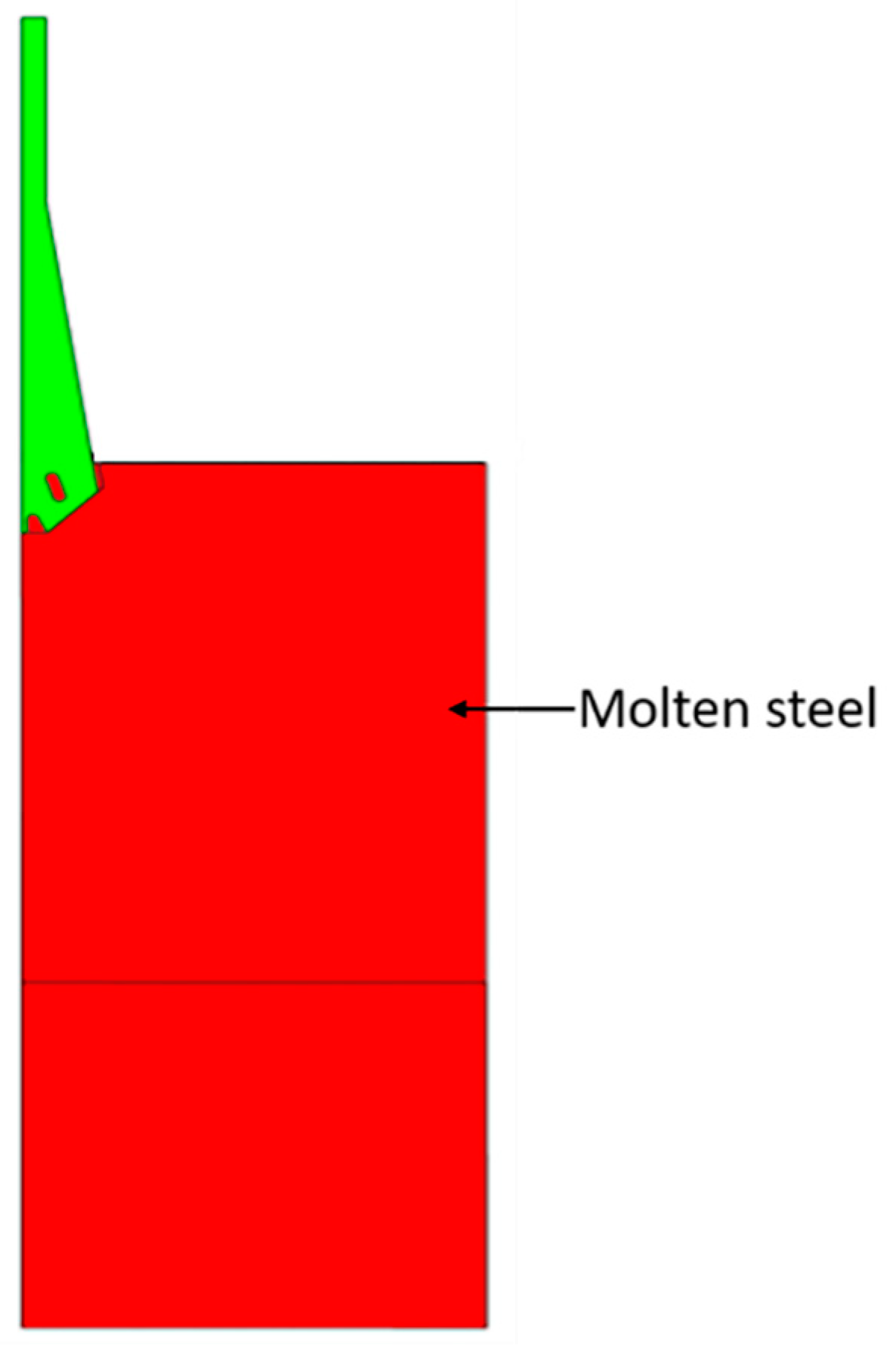
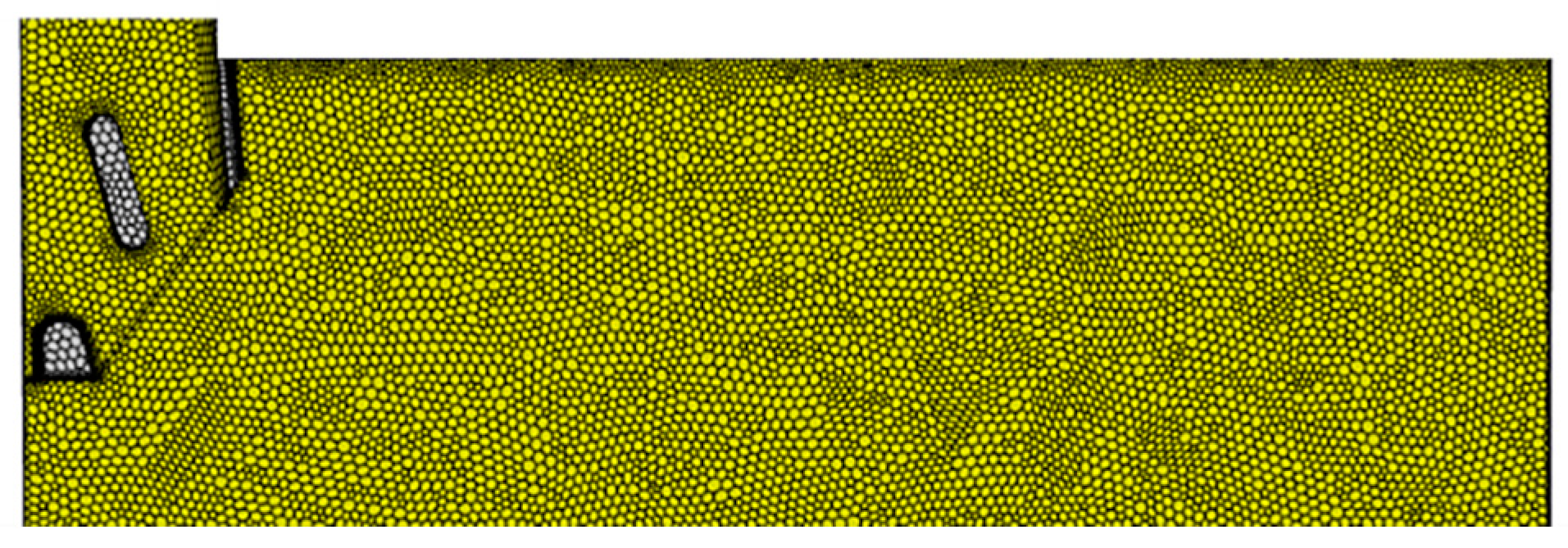

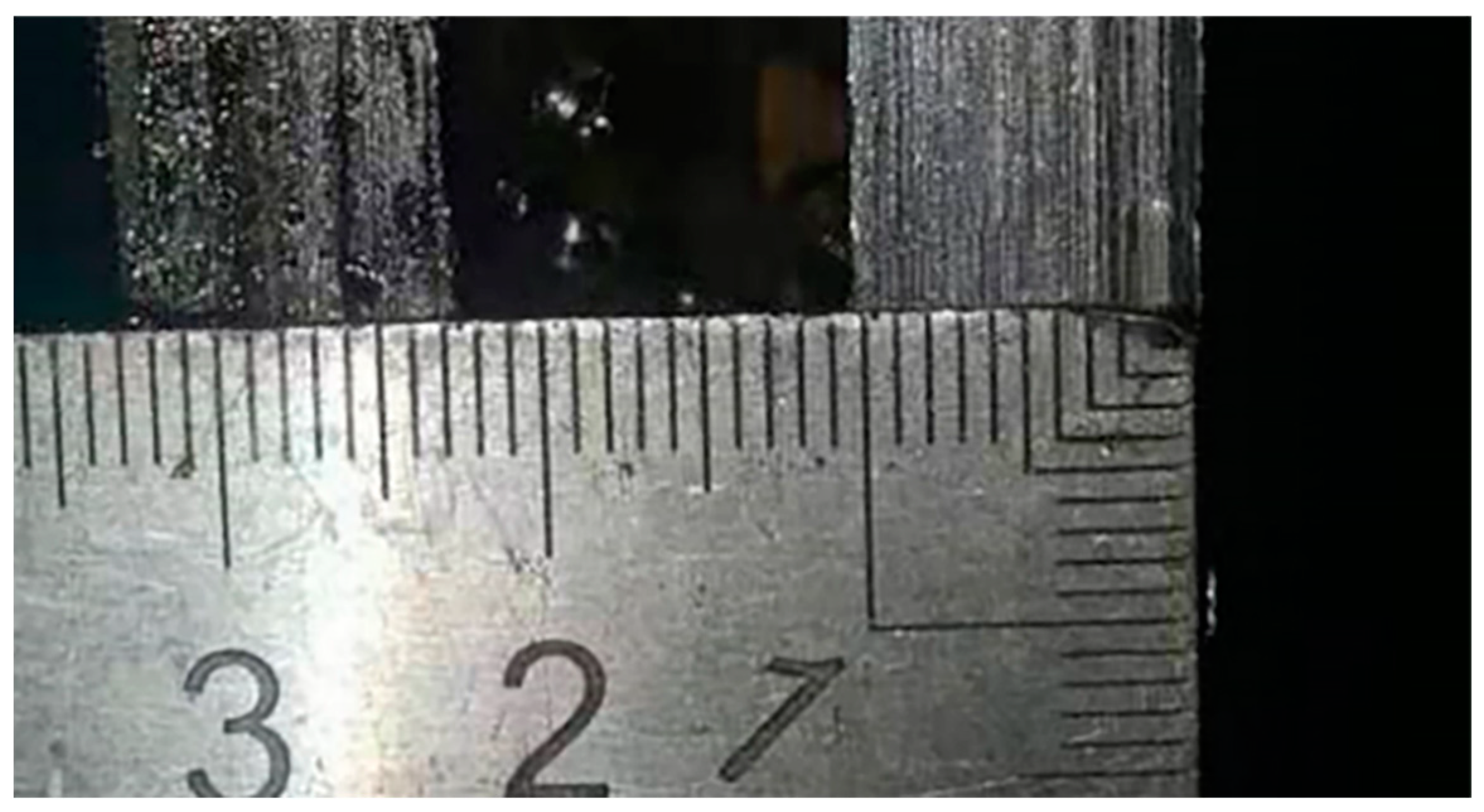
| Thermophysical Property Parameters | Value |
|---|---|
| Molten steel density | 7020 (kg/m3) |
| Molten steel viscosity | 0.0064 (Pa⋅s) |
| Casting speed | (4, 5, 6) m/min |
| Initial value of velocity (casting speed: (4, 5, 6) m/min) | (1.82, 2.27, 2.72) m/s |
| Turbulent kinetic energy (casting speed: (4, 5, 6) m/min) | (6.32, 9.31, 1.28) m2/s2 |
| Turbulent dissipation rate (casting speed: (4, 5, 6) m/min) | 1.48, 2.63, 4.24 |
| Superheat of molten steel | 20 K |
| Solidus temperature (TS) | 1763 K |
| Liquidus temperature (TL) | 1803 K |
| Specific Heat Capacity (Cp) | 680 J/(kgK) |
| Latent heat of solidification (LN) | 270,000 J/kg |
| Wide surface heat flux (4 m/min, 5 m/min, 6 m/min) | (2.0, 2.1, 2.2) × 106 W/m2 |
| Narrow surface heat flux (: 4 m/min, 5 m/min, 6 m/min) | (1.8, 1.9, 2.0) × 106 W/m2 |
| Specific heat capacity (CW) | 4.2 × 10³ J/(kgK) |
| Number | Amush (kg/(s)) |
|---|---|
| A | 1 × 105 |
| B | 1 × 107 |
| C | 1 × 108 |
| D | 3 × 108 |
| E | 6 × 108 |
| F | 9 × 108 |
| A: 1 × 105 | B: 1 × 107 | C: 1 × 108 |  | |
| Picture of solid- and liquid-phase distribution |  |  |  | |
| D: 3 × 108 | E: 6 × 108 | F: 9 × 108 | ||
| Picture of solid and liquid phase distribution |  |  |  |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ding, Z.; Xue, Y.; Zhang, L.; Li, C.; Wang, S.; Ni, G. Study on Mushy Zone Coefficient in Solidification Heat Transfer Mathematical Model of Thin Slab with High Casting Speed. Processes 2023, 11, 3108. https://doi.org/10.3390/pr11113108
Ding Z, Xue Y, Zhang L, Li C, Wang S, Ni G. Study on Mushy Zone Coefficient in Solidification Heat Transfer Mathematical Model of Thin Slab with High Casting Speed. Processes. 2023; 11(11):3108. https://doi.org/10.3390/pr11113108
Chicago/Turabian StyleDing, Zhijun, Yuekai Xue, Limin Zhang, Chenxiao Li, Shuhuan Wang, and Guolong Ni. 2023. "Study on Mushy Zone Coefficient in Solidification Heat Transfer Mathematical Model of Thin Slab with High Casting Speed" Processes 11, no. 11: 3108. https://doi.org/10.3390/pr11113108
APA StyleDing, Z., Xue, Y., Zhang, L., Li, C., Wang, S., & Ni, G. (2023). Study on Mushy Zone Coefficient in Solidification Heat Transfer Mathematical Model of Thin Slab with High Casting Speed. Processes, 11(11), 3108. https://doi.org/10.3390/pr11113108





