Novel Analytical Approach for the Space-Time Fractional (2+1)-Dimensional Breaking Soliton Equation via Mathematical Methods
Abstract
:1. Introduction
2. Preliminaries and Basic Definitions
3. Description of the Proposed Methods
3.1. Improved Simple Equation Method
3.2. Modified F-Expansion Method
4. Applications
4.1. Applications of Improved Simple Equation Method

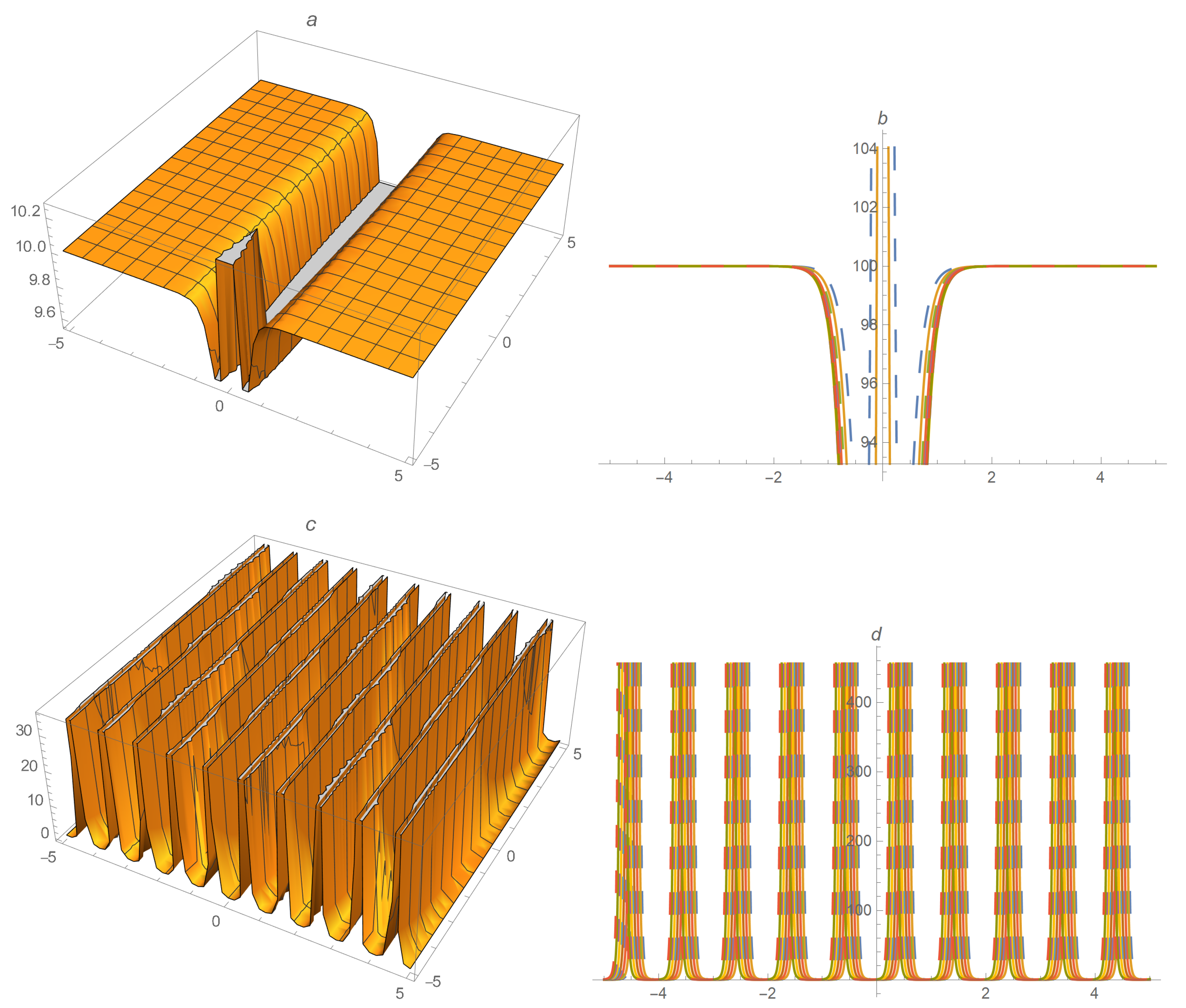
4.2. Applications of Modified F-Expansion Method
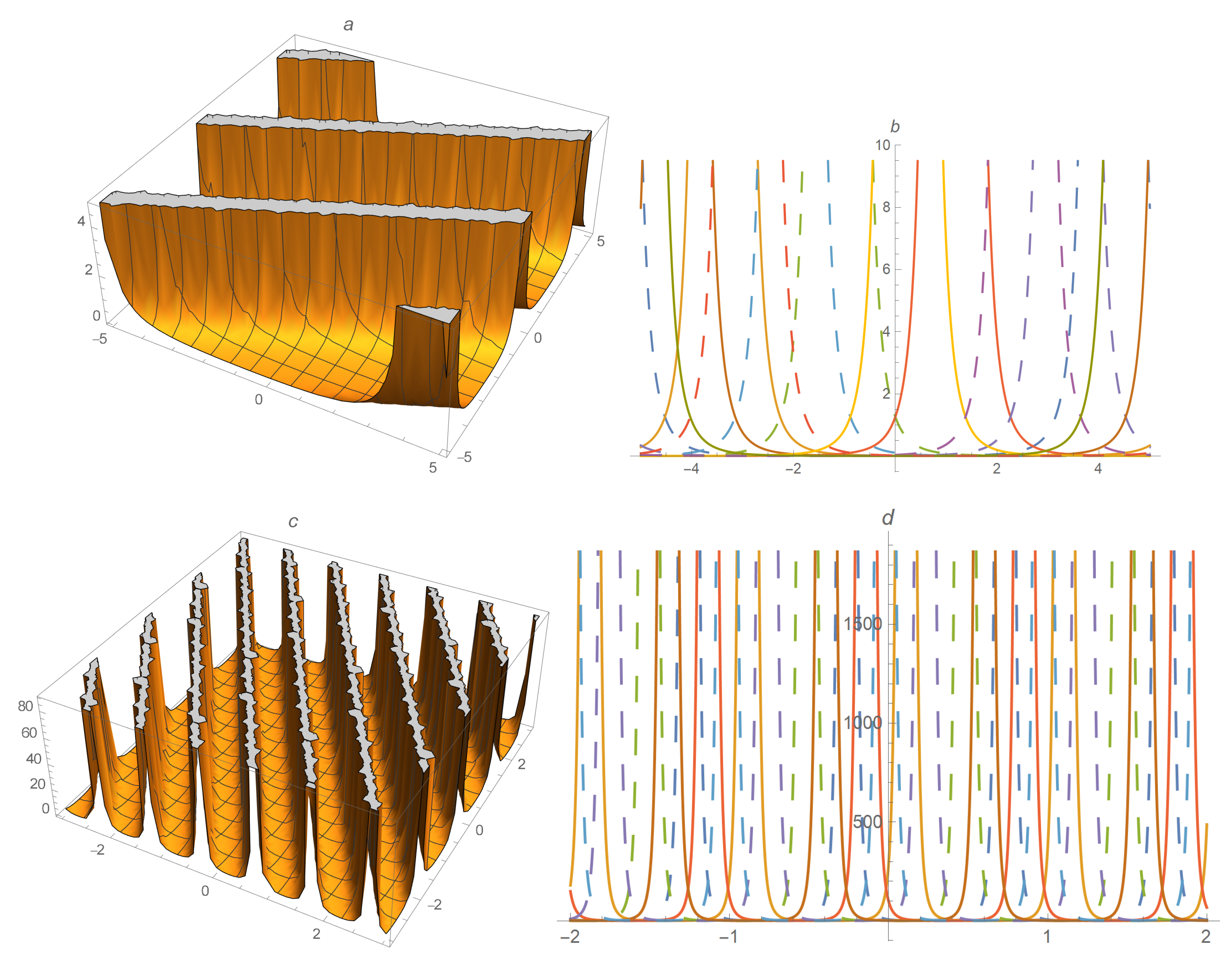
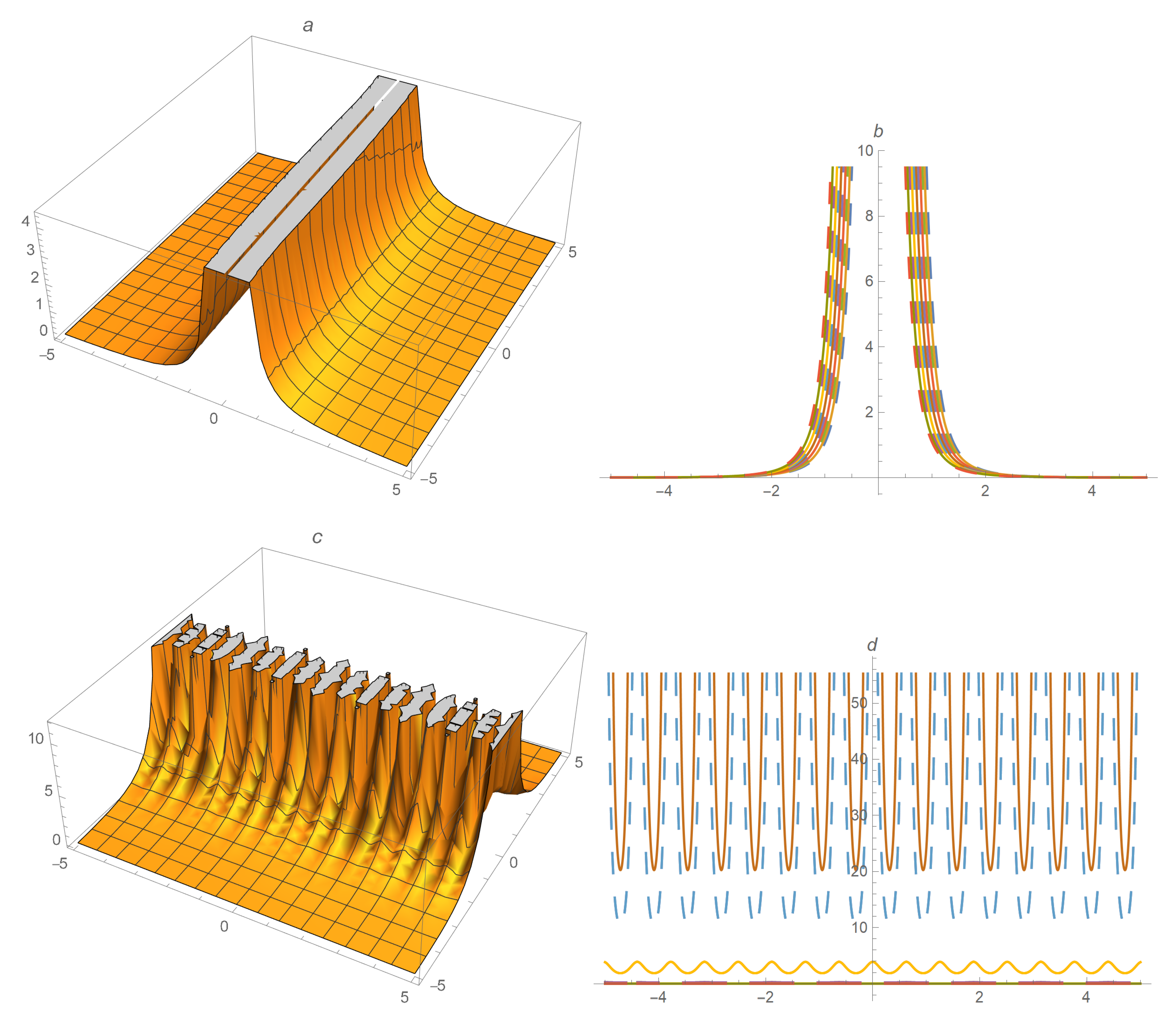


5. Results and Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Dalir, M.; Bashour, M. Application of fractional calculus. Appl. Math. Sci. 2010, 21, 1021–1032. [Google Scholar]
- Herrmann, R. Fractional Calculus: An Introduction for Physicists; World Scientific: Singapore, 2011. [Google Scholar]
- Javeed, S.; Saif, S.; Waheed, A.D. Baleanu, Exact solutions of the fractional mBBM equation and coupled system of fractional Boussinesq-Burgers. Respir. Physiol. 2018, 9, 1275–1281. [Google Scholar]
- Ekici, M.; Mirzazadeh, M.; Eslami, M.; Zhou, Q.; Moshokoa, S.P.; Biswas, A. Optical soliton perturbation with fractional temporal evolution by the first integral method with conformable fractional derivatives. Opt.-Int. J. Light Elect. Opt. 2016, 127, 10659–10669. [Google Scholar] [CrossRef]
- Younis, M. The first integral method for time-space fractional differential equations. J. Adv. Phys. 2013, 2, 220–223. [Google Scholar] [CrossRef]
- Özkan, Y.S.; Seadawy, A.R.; Yaşar, E. Multi-wave, breather and interaction solutions to (3+1) dimensional Vakhnenko–Parkes equation arising at propagation of high-frequency waves in a relaxing medium. J. Taibah Univ. Sci. 2021, 15, 666–678. [Google Scholar] [CrossRef]
- Younas, U.; Seadawy, A.R.; Younis, M.; Rizvi, S.T.R. Dispersive of propagation wave structures to the Dullin-Gottwald-Holm dynamical equation in a shallow water waves. Chin. J. Phys. 2020, 68, 348–364. [Google Scholar] [CrossRef]
- Seadawy, A.R.; Iqbal, M.; Lu, D. Application of mathematical methods on the ion sound and Langmuir waves dynamical systems. Pramana J. Phys. 2019, 93, 10. [Google Scholar] [CrossRef]
- Meng, F.; Feng, Q. Exact solution with variable coefficient function forms for conformable fractional partial differential equations by an Auxiliary equation method. Adv. Math. Phys. 2018, 2018, 4596506. [Google Scholar] [CrossRef] [Green Version]
- Guo, S.; Zhou, Y. Auxiliary equation method for the mKdV equation with variable coefficients. Appl. Math. Comput. 2010, 217, 1476–1483. [Google Scholar] [CrossRef]
- Zhang, S.; Zhang, H.Q. Fractional sub-equation method and its application to nonlinear fractional PDEs. Phys. Lett. 2011, 375, 1069–1073. [Google Scholar] [CrossRef]
- Tang, B.; He, Y.; Wu, L.; Zhang, X. A generalized fractional sub-equation method for a fractional differential equation with variable coefficients. Phys. Lett. 2012, 376, 2588–2590. [Google Scholar] [CrossRef]
- Althobaiti, A.; Althobaiti, S.; El-Rashidy, K.; Seadawy, A.R. Exact solutions for the nonlinear extended KdV equation in a stratified shear flow using modified exponential rational method. Results Phys. 2021, 29, 104723. [Google Scholar] [CrossRef]
- Kadkhoda, N.; Jafari, H. Application of the fractional sub-equation method to the space-time fractional differential equations. Int. J. Adv. Math. Mech. 2017, 4, 1–6. [Google Scholar]
- Zheng, B. (G′/G)-expansion method for solving fractional partial differential equations in the theory of mathematical physics. Commun. Theor. Phys. 2012, 58, 623–630. [Google Scholar] [CrossRef]
- Ahmed, I.; Seadawy, A.R.; Lu, D. M-shaped rational solitons and their interaction with kink waves in the Fokas-lenells equation. Phys. Scr. 2019, 94, 055205. [Google Scholar] [CrossRef]
- Lu, D.; Seadawy, A.R.; Iqbal, M. Mathematical physics via construction of traveling and solitary wave solutions of three coupled system of nonlinear partial differential equations and their applications. Results Phys. 2018, 11, 1161–1171. [Google Scholar] [CrossRef]
- Simbanefayi, I.; Khalique, C.M. Travelling wave solutions and conservation laws for the Korteweg-de Vries-Benjamin-Bona-Mahony equation. Respir. Physiol. 2018, 8, 57–63. [Google Scholar]
- Rahmatullah, R.; Ellahi; Mohyud-Din, S.T.; Khan, U. Exact traveling wave solutions of fractional order Boussinesq-like equations by applying the Exp-function method. Respir. Physiol. 2018, 8, 114–120. [Google Scholar] [CrossRef]
- Zheng, B. Exp-function method for solving fractional partial differential equations. Sci. World J. 2013, 2013, 465723. [Google Scholar] [CrossRef] [Green Version]
- Ozkan, Y.G.; Yaşar, E.; Seadawy, A. On the multi-waves, interaction and Peregrine-like rational solutions of perturbed Radhakrishnan–Kundu–Lakshmanan equation. Phys. Scr. 2020, 8, 085205. [Google Scholar] [CrossRef]
- Seadawy, A.R.; Ali, A.; Albarakati, W.A. Analytical wave solutions of the (2 + 1)-dimensional first integro-differential Kadomtsev-Petviashivili hierarchy equation by using modified mathematical methods. Results Phys. 2019, 15, 102775. [Google Scholar] [CrossRef]
- Ray, S.S.; Sahoo, S. Two efficient reliable methods for solving fractional fifth-order modified Sawada-Kotera equation appearing in mathematical physics. J. Ocean Eng. Sci. 2016, 1, 219–225. [Google Scholar]
- Gaber, A.A.; Aljohani, A.F.; Ebaid, A.; Machado, J.T. The generalized Kudryashov method for nonlinear space-time fractional partial differential equations of Burger’s type. Nonlinear Dynam. 2019, 95, 361–368. [Google Scholar] [CrossRef]
- Demiray, S.T.; Bulut, H. Generalized Kudryashov method for the nonlinear fractional double Sinh-Poisson equation. J. Nonlinear Sci. Appl. 2016, 9, 1349–1355. [Google Scholar] [CrossRef] [Green Version]
- Seadawy, A.R.; Yasmeen, A.; Raza, N.; Althobaiti, S. Novel solitary waves for fractional (2+1)-dimensional Heisenberg ferromagnetic model via new extended generalized Kudryashov method. Phys. Scr. 2021, 96, 125240. [Google Scholar] [CrossRef]
- Kumar, D.; Seadawy, A.R.; Joardar, A.K. Modified Kudryashov method via new exact solutions for some conformable fractional differential equations arising in mathematical biology. Chin. J. Phys. 2018, 56, 75–85. [Google Scholar] [CrossRef]
- Rahman, M.M.; Habib, M.A.; Ali, H.M.S.; Miah, M.M. The generalized Kudryashov method: A renewed mechanism for performing exact solitary wave solutions of some NLEEs. J. Mech. Cont. Math. Sci. 2019, 14, 323–339. [Google Scholar] [CrossRef]
- Kumar, S.; Kumar, D.; Kharbanda, H. Lie symmetry analysis, abundant exact solutions and dynamics of multisolitons to the (2 + 1)-dimensional KP-BBM equation. Pramana 2021, 95, 33. [Google Scholar] [CrossRef]
- Kumar, S.; Kumar, D.; Kumar, A. Lie symmetry analysis for obtaining the abundant exact solutions, optimal system and dynamics of solitons for a higher-dimensional Fokas equation. Chaos Solitons Fractals 2021, 142, 110507. [Google Scholar] [CrossRef]
- Kumar, S.; Kumar, D. Lie symmetry analysis and dynamical structures of soliton solutions for the -dimensional modified CBS equation. Int. J. Mod. Phys. 2020, 34, 25. [Google Scholar] [CrossRef]
- Kumar, S.; Kumar, A. Lie symmetry reductions and group Invariant Solutions of (2+1)-dimensional modified Veronese web equation. Nonlinear Dyn. 2019, 98, 1891–1903. [Google Scholar] [CrossRef]
- Kumar, S.; Niwas, M.; Wazwaz, A. Lie symmetry analysis, exact analytical solutions and dynamics of solitons for (2+1)-dimensional NNV equations. Phys. Scr. 2020, 95, 095204. [Google Scholar] [CrossRef]
- Kumar, S.; Rani, S. Lie symmetry reductions and dynamics of soliton solutions of (2+1)-dimensional Pavlov equation. Pramana 2020, 94, 116. [Google Scholar] [CrossRef]
- Ege, S.M.; Misirli, E. Extended Kudryashov method for fractional nonlinear differential equations. Math. Sci. Appl. E-NOTE 2018, 6, 19–28. [Google Scholar] [CrossRef]
- Akram, U.; Seadawy, A.R.; Rizvi, S.T.R.; Younis, M.; Althobaiti, S.; Sayed, S. Traveling wave solutions for the fractional Wazwaz–Benjamin–Bona–Mahony model in arising shallow water waves. Results Phys. 2021, 20, 103725. [Google Scholar] [CrossRef]
- Gomez, C.A.; Garzon, H.G.; Hernandez, J.C.R. On exact solutions for (4þ1)-dimensional Fokas equation with variable coefficients. Adv. Stud. Theor. Phys. 2017, 11, 765–771. [Google Scholar] [CrossRef]
- Seadawy, A.R.; Cheemaa, N. Some new families of spiky solitary waves of one-dimensional higher-order K-dV equation with power law nonlinearity in plasma physics. Indian J. Phys. 2020, 94, 117–126. [Google Scholar] [CrossRef]
- Guner, O. Singular and non-topological soliton solutions for nonlinear fractional differential equations. Chin. Phys. B 2015, 24, 100201. [Google Scholar] [CrossRef]
- Yildirim, Y.; Yasar, E. A (2+1)-dimensional breaking soliton equation: Solutions and conservation laws. Chaos Solit. Fractals 2018, 107, 146–155. [Google Scholar] [CrossRef]
- Rizvi, S.T.R.; Seadawy, A.R.; Ali, I.; Bibi, I.; Younis, M. Chirp-free optical dromions for the presence of higher order spatio-temporal dispersions and absence of self-phase modulation in birefringent fibers. Mod. Phys. Lett. B 2020, 35, 2050399. [Google Scholar] [CrossRef]
- Wazwaz, A.M. Breaking soliton equations and negative-order breaking soliton equations of typical and higher orders. Pramana Phys. 2016, 87, 68. [Google Scholar] [CrossRef]
- Yildiz, G.; Daghan, D. Solutions of the (2+1)-dimensional breaking soliton equation by using two different methods. J. Eng. Tech. Appl. Sci. 2016, 1, 13–18. [Google Scholar]
- Wang, S.F. Analytical multi-soliton solutions of a (2+1)-dimensional breaking soliton equation. SpringerPlus 2016, 5, 891. [Google Scholar] [CrossRef] [Green Version]
- Chen, Y.; Ma, S. Non-traveling wave solutions for the (2+1)-dimensional breaking soliton system. Appl. Math. 2012, 3, 813–818. [Google Scholar] [CrossRef] [Green Version]
- Zhang, J.F.; Heng, C.L. New multi-soliton solutions of the (2+1)-dimensional breaking soliton equations. Int. J. Mod. Phys. B 2003, 17, 4376–4381. [Google Scholar] [CrossRef]
- Li, Y.S.; Zhang, Y.J. Symmetrics of a (2þ1)-dimensional breaking soliton equation: Three-wave methods. J. Phys. Math. Gen. 2012, 26, 24. [Google Scholar]
- Darvishi, M.T.; Najafi, M. Some exact solutions of the (2+1)-dimensional breaking soliton equation using three-wave method. Int. J. Math. Comput. Sci. 2011, 5, 7. [Google Scholar]
- Seadawy, A.R.; Ali, A.; Zahed, H.; Baleanu, D. The Klein–Fock–Gordon and Tzitzeica dynamical equations with advanced analytical wave solutions. Results Phys. 2020, 19, 103565. [Google Scholar] [CrossRef]
- Ali, A.; Seadawy, A.R.; Baleanu, D. Computational solutions of conformable space-time derivatives dynamical wave equations: Analytical mathematical techniques. Results Phys. 2020, 19, 103419. [Google Scholar] [CrossRef]
- Seadawy, A.; Ali, A.; Baleanu, D. Transmission of high-frequency waves in a tranquil medium with general form of the Vakhnenko dynamical equation. Phys. Scr. 2020, 95, 095208. [Google Scholar] [CrossRef]
- Jumarie, G. Modified Riemann-Liouville derivative and fractional Taylor series of non-differentiables functions further results. Comput. Math. Appl. 2006, 51, 1367–1376. [Google Scholar] [CrossRef] [Green Version]
- Jumarie, G. From self-similarity to the fractional derivative of non-differentiable function via Mittag-Leffler functions. Appl. Math. Sci. 2008, 2, 1949–1962. [Google Scholar]
- Seadawy, A.R.; Cheemaa, N. Propagation of nonlinear complex waves for the coupled nonlinear Schrödinger Equations in two core optical fibers. Phys. A Stat. Mech. Appl. 2019, 529, 121330. [Google Scholar] [CrossRef]
- Hossain, A.K.S.; Akbar, M.A.; Azad, M.A.K. The closed form solutions of simplified MCH equation and third extended fifth order nonlinear equation. Propul. Power Res. 2019, 8, 163–172. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alruwaili, A.D.; Seadawy, A.R.; Ali, A.; Beinane, S.A.O. Novel Analytical Approach for the Space-Time Fractional (2+1)-Dimensional Breaking Soliton Equation via Mathematical Methods. Mathematics 2021, 9, 3253. https://doi.org/10.3390/math9243253
Alruwaili AD, Seadawy AR, Ali A, Beinane SAO. Novel Analytical Approach for the Space-Time Fractional (2+1)-Dimensional Breaking Soliton Equation via Mathematical Methods. Mathematics. 2021; 9(24):3253. https://doi.org/10.3390/math9243253
Chicago/Turabian StyleAlruwaili, Abdulmohsen D., Aly R. Seadawy, Asghar Ali, and Sid Ahmed O. Beinane. 2021. "Novel Analytical Approach for the Space-Time Fractional (2+1)-Dimensional Breaking Soliton Equation via Mathematical Methods" Mathematics 9, no. 24: 3253. https://doi.org/10.3390/math9243253
APA StyleAlruwaili, A. D., Seadawy, A. R., Ali, A., & Beinane, S. A. O. (2021). Novel Analytical Approach for the Space-Time Fractional (2+1)-Dimensional Breaking Soliton Equation via Mathematical Methods. Mathematics, 9(24), 3253. https://doi.org/10.3390/math9243253






