G3 Shape Adjustable GHT-Bézier Developable Surfaces and Their Applications
Abstract
1. Introduction
- The representation of a family of GHT-Bernstein basis functions and GHT-Bézier curves with geometric properties.
- The designing of Enveloping developable GHT-Bézier surfaces, surfaces interpolation geodesic developable GHT-Bézier curve with shape control parameters, Spine curve developable GHT-Bézier surfaces, and developable GHT-Bézier Canal surfaces based on computer technology.
- continuity and continuity of developable GHT-Bézier surfaces are also presented. Moreover, an algorithm is given to explain how to enforce these continuities in practice.
- This approach becomes more convenient because of the exclusion of difficult calculations which come from the nonlinearity of characteristic equations.
2. A Family of GHT-Bernstein Basis with Geometric Properties
3. GHT-Bézier Curves with Geometric Properties
4. Composition of GHT-Bézier Developable Surfaces
4.1. Dual Representation of One-Parameter Family of Planes
4.2. Enveloping Developable GHT-Bézier Surfaces
4.3. Spine Curve Developable GHT-Bézier Surfaces
4.4. Developable Surface Interpolating Geodesic GHT-Bézier Curve
4.5. Developable GHT-Bézier Canal Surface
5. Analysis Properties of Developable GHT-Bézier Surfaces
6. Continuity Conditions of Developable GHT-Bézier Surfaces
6.1. Axioms of Continuity between Developable GHT-Bézier Surfaces
6.2. Axioms of Farin-Boehm Continuity between Developable GHT-Bézier Surfaces
6.3. Axioms of Beta Continuity between Developable GHT-Bézier Surfaces
6.4. Axioms of Continuity between Developable GHT-Bézier Surfaces
6.5. Algorithm to Explain Axioms of Continuity for Developable GHT-Bézier Surfaces
- Consider the shape parameters and control planes of initial developable GHT-Bézier surface
- For continuity, the developable GHT-Bézier surfaces and have a common control plane.
- The second control plane of developable GHT-Bézier surface can be computed by using the second condition of Equation (31). Here the values of shape parameters, chosen freely from their domain while scale factors, can be any real constant.
- For continuity, the third control plane of surface can be obtained by using Farin-Boehm and Beta continuity condition between two adjacent developable GHT-Bézier surfaces.
- The last control plane of developable GHT-Bézier surface can be obtained by using the last condition of Equation (35), i.e., the continuity between two adjacent developable GHT-Bézier surfaces.
7. Designing of Developable GHT-Bézier Surfaces
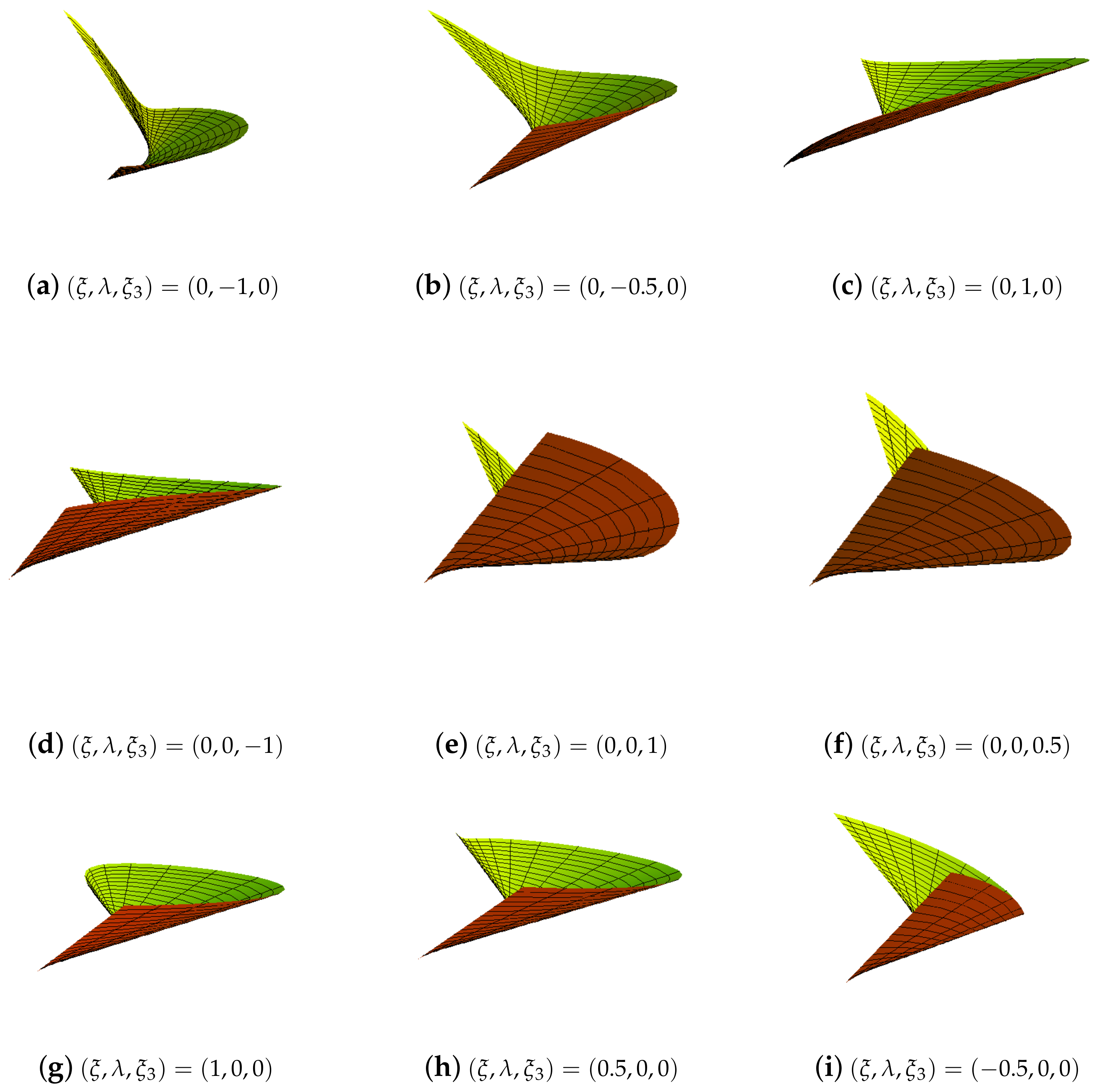
7.1. Examples for the Construction of Enveloping Developable GHT-Bézier Surfaces
- When all the shape parameters varies equally, then length of starting generator and ending generator will remain the same, but at the same time the enveloping developable surface as shown in Figure 3 will expand (or shrink).
- When two shape parameters are fixed to 1 and varies, then length of starting generator and ending generator are closely related (are same) and enveloping developable GHT-Bézier surface will shrink.
- When shape parameters are fixed to 1 and varies, then length of starting generator is greater then the length of ending generator as shown in Figure 4d–f.
- When shape parameters are fixed to 1 and varies, then length of ending generator is greater then the length of starting generator and enveloping developable GHT-Bézier surface will expand (or shrink) gradually as shown in Figure 4g–i.
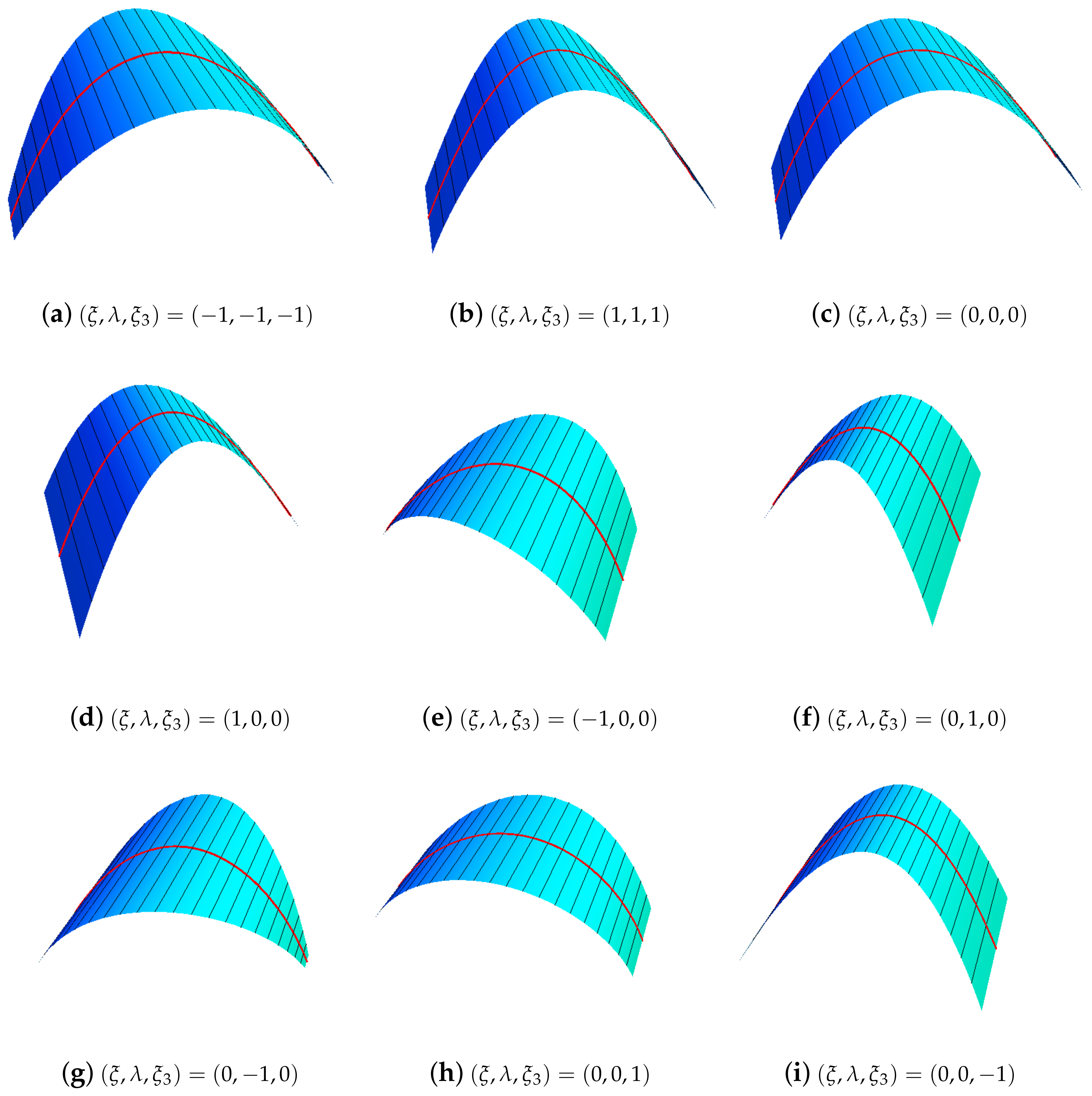
7.2. Spine Curve Developable GHT-Bézier Surface Construction
- When we consider all the shape parameters, varies equally with negative values, i.e., then length of starting generator increases with length of ending generator but when we have positive values of shape parameters, the length of starting generator decreases with length of ending generator. The behavior of shape parameters will shrink/expand, the shape of the surface as well.
- When two shape parameters are fixed to 0 and varies, then length of ending generator is larger then the length of starting generator However, as we increase the values of the surface will shrink and the length of starting generator will also increase.
- When are fixed to 0 and values of increase, then the length of starting generator decreases and length of ending generator increases. However, at the same time the spine curve developable GHT-Bézier surface will expand.
- When the shape parameters are fixed to and varies, then the length of starting generator increases and length of ending generator remains same. The surface will shrink by decreasing the values of shape parameters.
7.3. Designing of Developable Surface Interpolating Geodesic GHT-Bézier Curve with Parameters
- When shape control parameters vary simultaneously, then smooth behavior of surface interpolation geodesic developable surfaces is shown in Figure 7a–c.
- When we fix two shape parameters and vary the remaining shape parameters one by one, then the behavior of developable surfaces also varies and is obvious in Figure 7d–i. The values of shape parameters are mentioned under each figure.
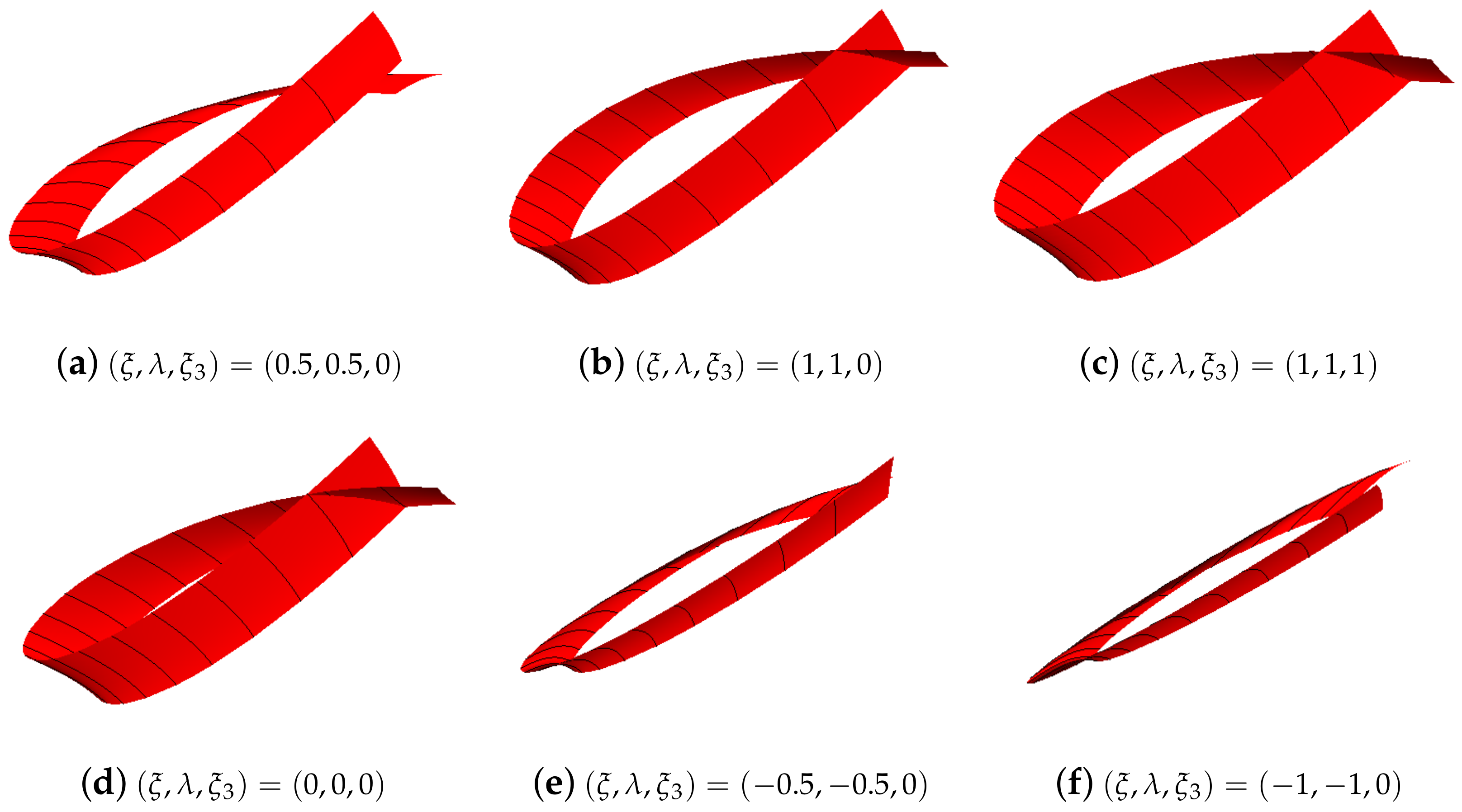
7.4. Construction of Developable GHT-Bézier Canal Surface with Shape Parameters
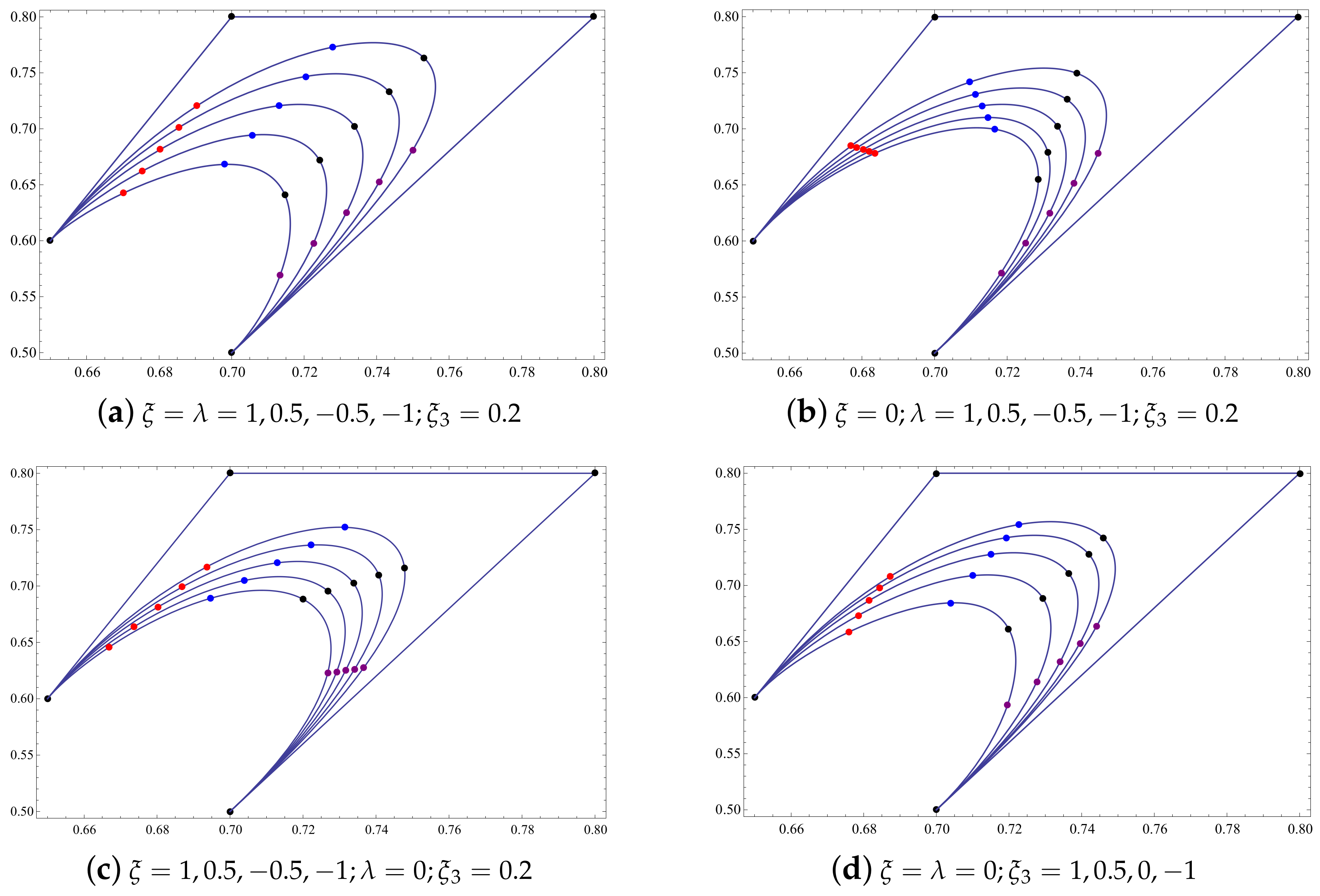
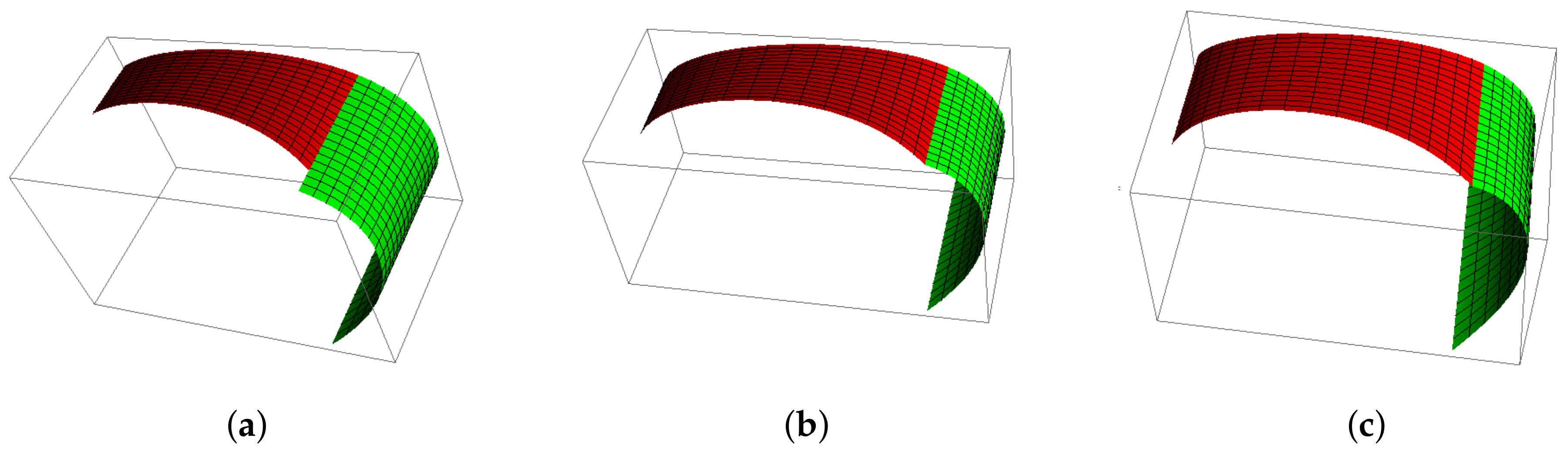
7.5. Examples of Smooth Continuity between Two Adjacent Developable GHT-Bézier Surfaces
8. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Chu, C.H.; Chen, J.T. Geometric design of developable composite Bézier surfaces. Comput.-Aided Des. Appl. 2004, 1, 531–539. [Google Scholar] [CrossRef][Green Version]
- Li, C.Y.; Wang, R.H.; Zhu, C.G. Design and G1 connection of developable surfaces through Bézier geodesics. Appl. Math. Comput. 2011, 218, 3199–3208. [Google Scholar] [CrossRef]
- Hu, G.; Cao, X.H.; Qin, X.Q. Geometric design and continuty of developable λ - Bézier surfaces. Adv. Eng. Soft. 2017, 114, 235–245. [Google Scholar] [CrossRef]
- Ammad, M.; Misro, M.Y.; Abbas, M.; Majeed, A. Generalized Developable Cubic Trigonometric Bézier Surfaces. Mathematics 2020, 9, 283. [Google Scholar]
- Zhang, X.W.; Wang, G.J. A new algorithm for designing developable GBT-Bézier surfaces. J. Zhejiang Univ. Sci. 2006, 7, 20506. [Google Scholar] [CrossRef]
- Hu, G.; Junli, W.; Xinqiang, Q. A new approach in designing of local controlled developable H-Bézier surfaces. Adv. Eng. Softw. 2018, 121, 26–38. [Google Scholar] [CrossRef]
- Chen, D.R.; Wang, G.J. Developable GBT-Bézier parametric surfaces. J. Comput. Aided Des. Comput. Graph. 2003, 15, 5705. [Google Scholar]
- Hu, G.; Cao, H.; Wu, J.; Wei, J. Construction of developable surfaces using generalized C-Bézier basis with shape parameters. Comput. Appl. Math. 2020, 39, 1–32. [Google Scholar] [CrossRef]
- Pottmann, H.; Farin, G. Developable rational Bézier and B-spline surfaces. Comput. Aided Geom. Des. 1995, 12, 51331. [Google Scholar] [CrossRef]
- Aumann, G. A simple algorithm for designing developable Bézier surfaces. Comput. Aided Geom. Des. 2003, 20, 601–619. [Google Scholar] [CrossRef]
- Aumann, G. Degree elevation and developable Bézier surfaces. Comput. Aided Geom. Des. 2004, 21, 661–670. [Google Scholar] [CrossRef]
- BiBi, S.; Abbas, M.; Misro, M.Y.; Hu, G. A novel approach of hybrid trigonometric Bézier curve to the modeling of symmetric revolutionary curves and symmetric rotation surfaces. IEEE Access 2019, 7, 165779–165792. [Google Scholar] [CrossRef]
- Qin, X.; Hu, G.; Zhang, N.; Shen, X.; Yang, Y. A novel extension to the polynomial basis functions describing Bézier curve and surfaces of degree n with multiple shape parameters. Appl. Math. Comput. 2013, 223, 1–16. [Google Scholar] [CrossRef]
- González, C.; Albrecht, G.; Paluszny, M.; Lentini, M. Design of C2 algebraic trigonometric Pythagoreans hodograph splies with shape paramneters. Comput. Appl. Math. 2016, 378, 1472–1495. [Google Scholar]
- Wu, X.; Han, X.; Luo, S. Quadratic trigonometric spline curve with shape parameters. In Proceedings of the 2007 10th IEEE International Conference on Computer-Aided Design and Computer Graphics, Beijing, China, 15–18 October 2007; pp. 1–16. [Google Scholar]
- BiBi, S.; Abbas, M.; Miura, K.T.; Misro, M.Y. Geometric Modelling of novel Generalized hybrid Trigonometric Bézier-like curve with Shape Parameters and its Applications. Mathematics 2020, 8, 967. [Google Scholar] [CrossRef]
- BiBi, S.; Abbas, M.; Misro, M.Y.; Majeed, A.; Nazir, T. Construction of Generalized Hybrid Trigonometric Bézier Surfaces with Shape Parameters and Their Applications. J. Math. Imaging Vis. 2021, 1–25. [Google Scholar] [CrossRef]
- Shi, F.Z. Computer Aided Geometric Design and NURBS; China Higher Education Press: Beijing, China, 2001. [Google Scholar]
- Han, X.A.; Ma, Y.; Huang, X. A novel generalization of Bézier curve and surface. J. Comput. Appl. Math. 2008, 217, 180–193. [Google Scholar] [CrossRef]
- Dube, M.; Yadav, B. The quintic trigonometric Bézier curve with single shape parameter. Int. J. Sci. Res. Pub. 2014, 4, 2250–3153. [Google Scholar]
- Ammad, M.; Misro, M.Y. Construction of Local Shape Adjustable Surfaces Using Quintic Trigonometric Bézier Curve. Symmetry 2020, 12, 1205. [Google Scholar] [CrossRef]
- Farin, G. Curves and Surfaces for CAGD. A Practical Guide, 5th ed.; Academic Press: San Diego, CA, USA, 2002. [Google Scholar]
- Juttler, B.; Sampoli, M.L. Hermite interpolation by piecewise polynomial surfaces with rational offsets. Comput. Aided Geom. Des. 2000, 17, 36185. [Google Scholar] [CrossRef]
- Tang, K.; Chen, M. Quasi-developable mesh surface interpolation via mesh deformation. IEEE Trans. Visual. Comput. Graph. 2009, 15, 51828. [Google Scholar]
- Zhou, M.; Peng, G.H.; Ye, Z.L. Design of developable surfaces by using duality between plane and point geometries. J. Comput. Aided Des. Comput. Graph. 2004, 16, 1401–1406. [Google Scholar]
- Zhao, H.Y.; Wang, G.J. A new method for designing a developable surface utilizing the surface pencil through a given curve. Prog. Nat. Sci. 2008, 18, 105–110. [Google Scholar] [CrossRef]
- Chen, M.; Tang, K. G2 quasi-developable GBT-Bézier surface interpolation of two space curves. Comput. Aided Des. 2013, 45, 136577. [Google Scholar] [CrossRef]
- Hwang, H.D.; Yoon, S.H. Constructing developable surfaces by wrapping cones and cylinders. Comput. Aided Des. 2015, 58, 230–235. [Google Scholar] [CrossRef]
- Chung, W.; Kim, S.H.; Shin, K.H. A method for planar development of 3D surfaces in shoe pattern design. J. Mech. Sci. Technol. 2008, 22, 1510–1519. [Google Scholar] [CrossRef]
- Veltkamp, R.C. Survey of continuities of curves and surfgaces. Comput. Graph. Forum. 1992, 11, 93–112. [Google Scholar] [CrossRef][Green Version]
- Rabinovich, M.; Hoffmann, E.Z.T.; Zurich, E. Discrete Geodesic Nets for Modeling Developable Surfaces. ACM Trans. Graph. 2017, 37, 1–17. [Google Scholar] [CrossRef]
- Qian, J.; Su, M.; Fu, M.; Juan, S.D. Geometric Characterizations of Canal Surfaces in Minkowski 3-Space. Mathematics 2019, 7, 703. [Google Scholar] [CrossRef]
- Tuncer, Y. Canal Surfaces in Galilean 3-Spaces. Kyungpook Math. J. 2017, 57, 319–326. [Google Scholar]
- Valdés, J.N.; Almagro, I.O. Canal surfaces and its application to the CAGD. Am. J. Eng. Res. 2021, 10, 19–31. [Google Scholar]
- Farin, G.E. Curves and Surfaces for CAGD; Morgan Kaufmann Publishers: San Francisco, CA, USA, 2002. [Google Scholar]
- Fu, X.; Jung, S.D.; Qian, J.; Su, M. Gometric characterization of canal surfaces in Minkowski 3-space. I. Bull. Korean Math. Soc. 2019, 56, 867–883. [Google Scholar]
- Xu, Z.Q.; Feng, R.Z.; Sun, J.G. Analytic and algebraic properties of canal surfaces. Appl. Math. Comput. 2016, 195, 220–228. [Google Scholar] [CrossRef]
- Cho, H.C.; Choi, H.I.; Kwon, S.H.; Lee, D.S.; Wee, N.S. Clifford algebra, Lorentzian geometry, and rational parametrization of canal surfaces. Comput. Aided Geom. 2004, 21, 327–339. [Google Scholar] [CrossRef]






Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
BiBi, S.; Misro, M.Y.; Abbas, M.; Majeed, A.; Nazir, T. G3 Shape Adjustable GHT-Bézier Developable Surfaces and Their Applications. Mathematics 2021, 9, 2350. https://doi.org/10.3390/math9192350
BiBi S, Misro MY, Abbas M, Majeed A, Nazir T. G3 Shape Adjustable GHT-Bézier Developable Surfaces and Their Applications. Mathematics. 2021; 9(19):2350. https://doi.org/10.3390/math9192350
Chicago/Turabian StyleBiBi, Samia, Md Yushalify Misro, Muhammad Abbas, Abdul Majeed, and Tahir Nazir. 2021. "G3 Shape Adjustable GHT-Bézier Developable Surfaces and Their Applications" Mathematics 9, no. 19: 2350. https://doi.org/10.3390/math9192350
APA StyleBiBi, S., Misro, M. Y., Abbas, M., Majeed, A., & Nazir, T. (2021). G3 Shape Adjustable GHT-Bézier Developable Surfaces and Their Applications. Mathematics, 9(19), 2350. https://doi.org/10.3390/math9192350







