A Novel Generalization of Bézier-like Curves and Surfaces with Shape Parameters
Abstract
:1. Introduction
- 1.
- The construction of a new gBBF with two shape parameters;
- 2.
- The construction of a new gBC with two shape parameters;
- 3.
- The construction of some geometric properties of the new gBBF and gBC and the conclusion that they are similar to the classical one;
- 4.
- Some free form curves are modeled by the proposed gBC;
- 5.
- Some Bézier-like surfaces with shape parameters are constructed;
- 6.
- The effects of the shape parameters on Bézier-like curves and surfaces are discussed.
2. Preliminaries
2.1. Bernstein Polynomials
2.2. Bézier Curve
2.3. Bézier Surfaces
3. Generalized Bézier-like Curves and Surfaces
3.1. Construction of Generalized Bernstein-like Basis Functions
- 1.
- Let , for , then the following functions:are called the Bernstein-like basis functions of degree .
3.2. Properties of the Bernstein-like Basis Functions
- 1.
- Degeneracy: If . That is, the Bernstein-like basis functions of the degree s with the parameters are only the standard Bernstein basis functions of the degree s.
- 2.
- Non-negativity: When , .Proof.By induction for s. If , we will rewrite the Bernstein-like basis functions as:Obviously, when , we have , . In fact, let us recognize that the cubic Bernstein-like basis functions are non-negative. The Bernstein-like basis functions of degree 2 are therefore non-negative.Now, suppose that the Bernstein-like basis functions of degree t are non-negative. We obtain from Equation (6):By our inductive hypothesis and the fact that , , we can conclude that the Bernstein-like basis functions of degree are non-negative. □
- 3.
- 4.
- Symmetry:Proof.We assume that Bernstein-like basis functions of degree 3 are symmetrical.Now, assume that the Bernstein-like basis functions of order t are symmetrical. Then, from the inductive hypothesis and recursive Formula (6), we have:□
- 5.
- Property at the end points: For :
- 6.
- 7.
- Linear Independence: iff .Proof.Sufficient condition is clear, and we shall show the requirement by induction as follows:First of all, we find the linear combination to be trivial:By comparing coefficients, we obtain:Upon any simplification, we receive from the linear freedom of the cubic Bernstein basis function: .It ensures that the Bernstein-like basis functions of the degree 2 is linearly independent.Assume the Bernstein-like basis functions of degree t are linearly independent. After that, we are going to prove that Bernstein-like basis functions are linearly independent of degree .Let us consider the linear combination:where . Substituting recursive Formula (6) in the above equation and rearranging it, we obtain:Because is an arbitrary value in the interval from the above equation, we obtain:and
3.3. Construction and Properties of the Bézier-like Curve
- 1.
- Convex hull property: The whole Bézier-like curve has to lie within the convex hull of its control polygon. This is implemented as the Bernstein-like basis functions being greater than zero and having a sum towards one.
- 2.
- Geometric invariance: However, since is an affine mixture of the control points, the Bézier-like curve geometry is distinct of coordinate system selection.
- 3.
- Symmetry: Control points of the Bézier curve can be marked as or without altering the structure of the curve. They are changed just in that they are reversed. When we do not understand the path of the curve, we have a curve:.
- 4.
- Geometric property at the endpoints From the property and the derivative at the endpoints of the Bernstein-like basis functions, we obtain:, , ,These imply that the Bézier-like curve interpolates at the endpoints and tangents at the end edges.
- 5.
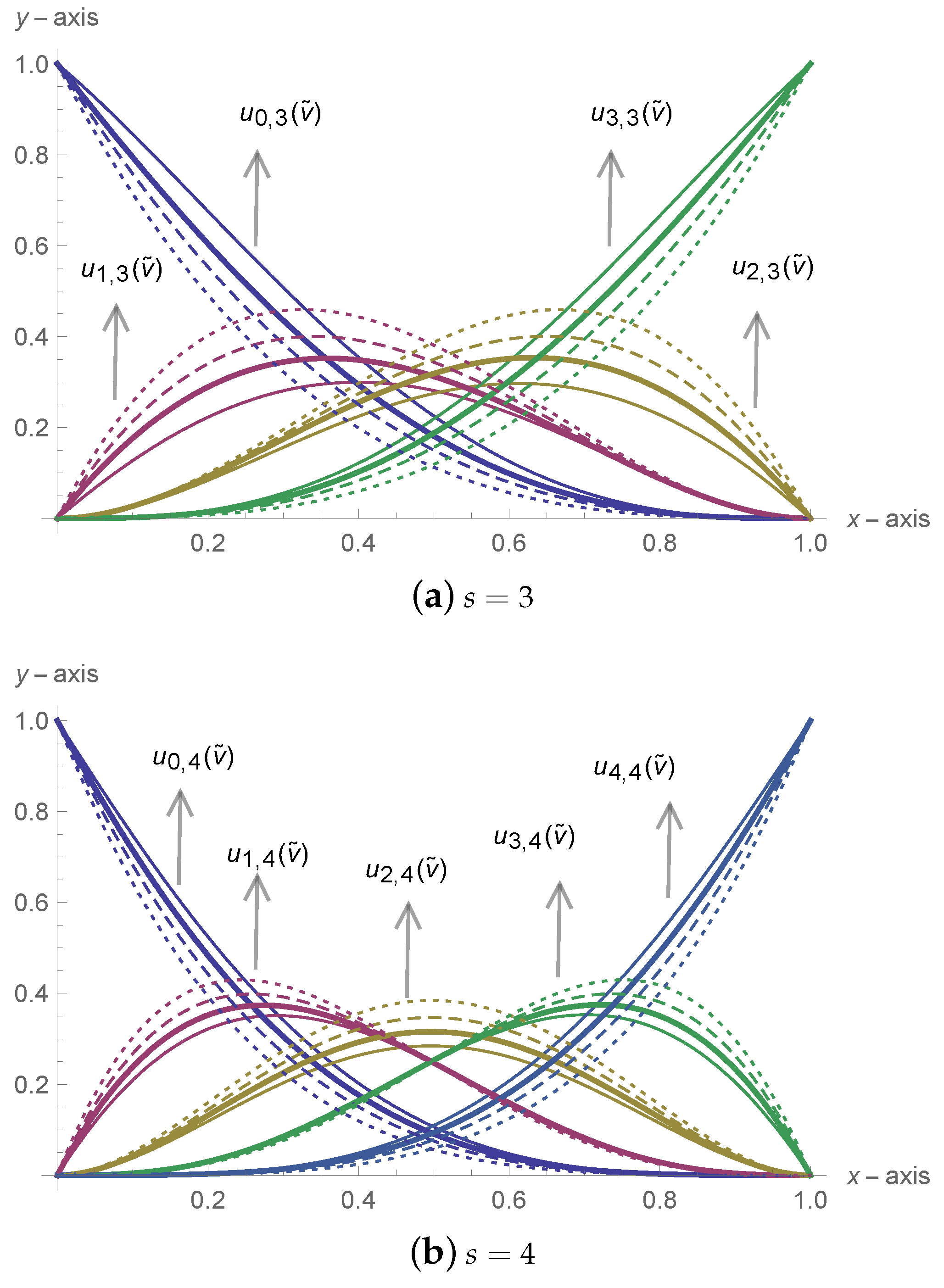
- Shape adjustable property: With the control polygon, the form of the famous Bézier curve can be fully decided. Yet, this is not the case for the Bézier curve. Promising to fix the control polygon, the form of the Bézier-like curve can also be changed by adjusting the shape parameters.Figure 3 displays four Bézier-like curve of degree 3 with similar control polygons with different shape parameters. From the figure, we can see that the Bézier-like curve introduces the control polygon by increasing the shape parameter. Besides that, since the Bézier-like curve seems to be just the classical Bézier curve when .
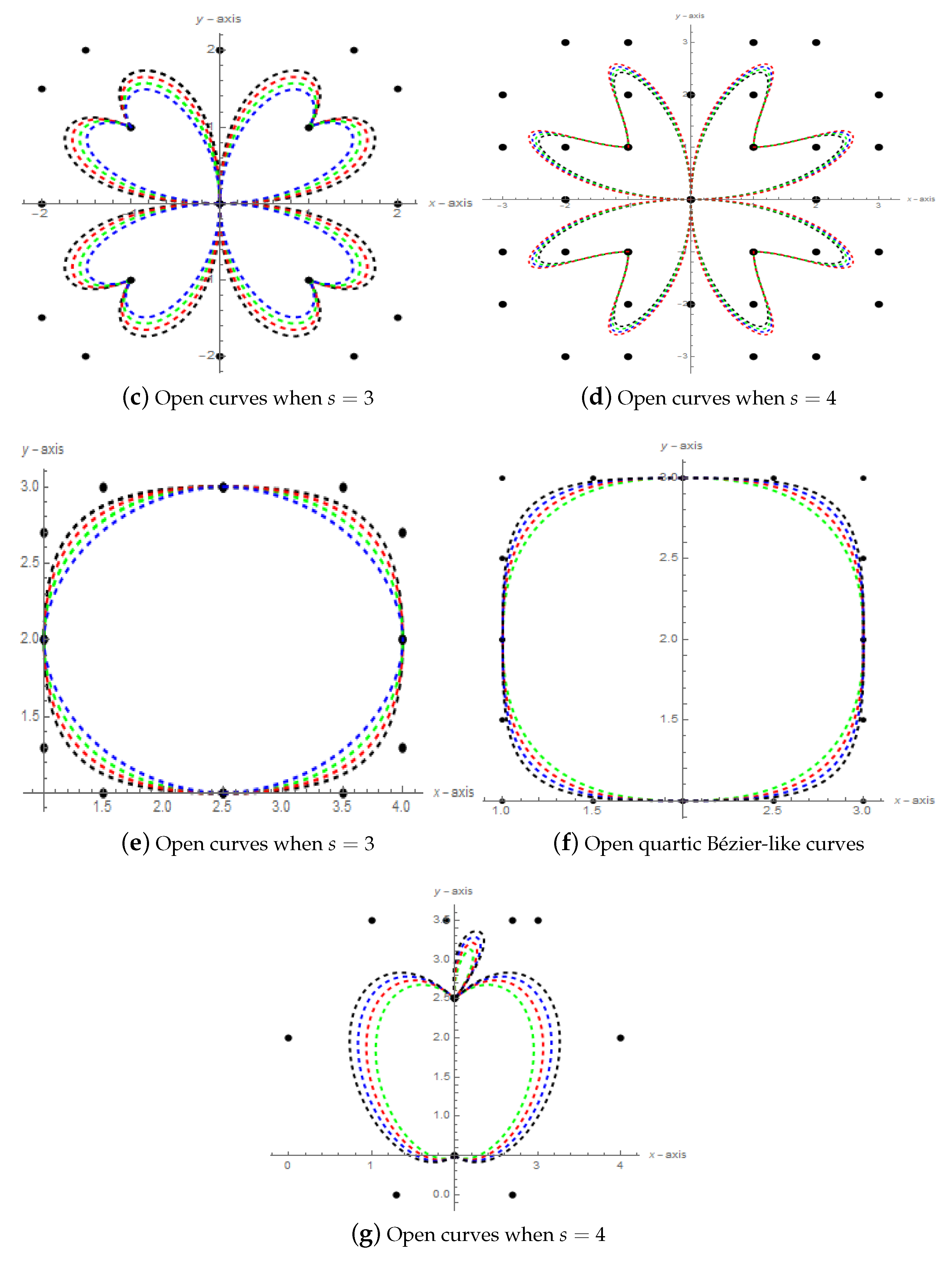
4. Open and Closed Curves
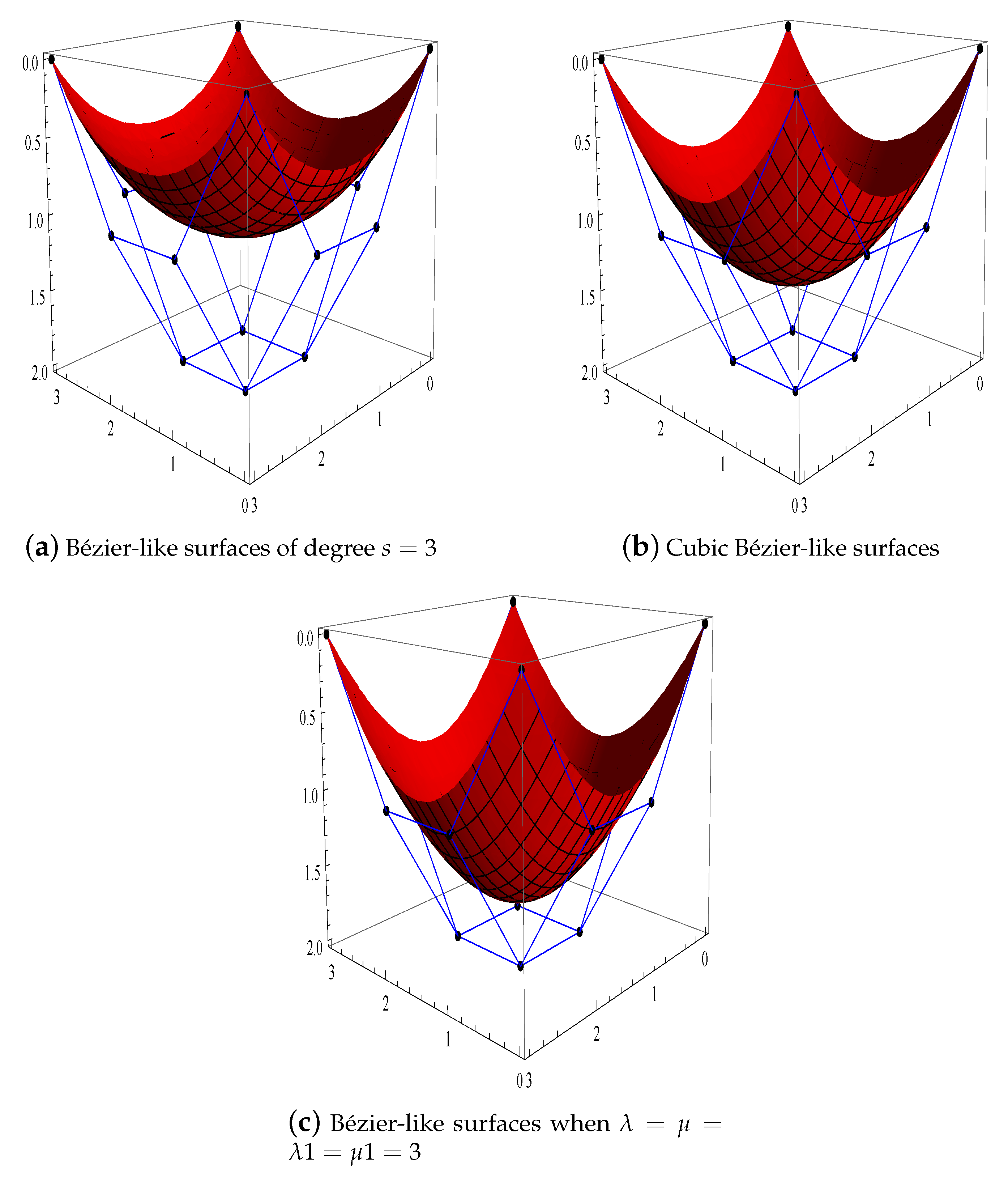
5. Development of Bézier-like Surfaces with Shape Parameters
Impact of Shape Parameters on Bézier-like Surfaces
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Farin, G.E. Curves and Surfaces for Computer-Aided Geometric Design: A Practical Code; Academic Press Inc.: Cambridge, MA, USA, 1996. [Google Scholar]
- Chen, Q.; Wang, G. A class of Bézier-like curves. Comput. Aided Geom. Des. 2003, 20, 29–39. [Google Scholar] [CrossRef]
- Han, X.; Ma, Y.; Huang, X. A novel generalization of Bézier curve and surface. J. Comput. Appl. Math. 2008, 217, 180–193. [Google Scholar] [CrossRef] [Green Version]
- Zhang, J. C-curves: An extension of cubic curves. Comput. Aided Geom. Des. 1996, 13, 199–217. [Google Scholar] [CrossRef]
- Farin, G. Triangular Bernstein Bézier patches. Comput. Aided Geom. Des. 1986, 3, 83–127. [Google Scholar] [CrossRef]
- Barnhill, R.E.; Gregory, J.A. Compatible smooth interpolation in triangles. J. Approx. Theory 1975, 15, 214–225. [Google Scholar] [CrossRef] [Green Version]
- Powell MJ, D.; Sabin, M.A. Piecewise quadratic approximations on triangles. ACM Trans. Math. Softw. 1977, 3, 316–332. [Google Scholar] [CrossRef]
- Gregory, J.A.; Charrot, P. A C1 triangular interpolation patch for computer-aided geometric design. Comput. Graph. Image Process. 1980, 13, 80–87. [Google Scholar] [CrossRef]
- Farin, G. Designing C1 surfaces consisting of triangular cubic patches. Comput.-Aided Des. 1982, 14, 253–256. [Google Scholar] [CrossRef]
- Chang, G.; Feng, Y. An improved condition for the convexity of Bernstein Bézier surfaces over triangles. Comput. Aided Geom. Des. 1984, 1, 279–283. [Google Scholar] [CrossRef]
- Rababah, A. Distances with rational triangular Bézier surfaces. Appl. Math. Comput. 2005, 160, 379–386. [Google Scholar] [CrossRef]
- Goodman, T.; Said, H.B. Properties of generalized Ball curves and surfaces. Comput.-Aided Des. 1991, 23, 554–560. [Google Scholar] [CrossRef]
- Hu, S.M.; Wang, G.J.; Sun, J.G. A type of triangular Ball-surface and its properties. J. Comput. Sci. Technol. 1998, 13, 63–72. [Google Scholar] [CrossRef]
- Zhang, C.; Cheng, F. Triangular patch modeling using combination method. Comput. Aided Geom. Des. 2002, 19, 645–662. [Google Scholar] [CrossRef]
- Chen, J.; Wang, G. Construction of triangular DP surface and its application. J. Comput. Appl. Math. 2008, 219, 312–326. [Google Scholar] [CrossRef] [Green Version]
- Juan, C.; Guozhao, W. An extension of Bernstein Bézier surface over the triangular domain. Prog. Nat. Sci. 2007, 17, 352–357. [Google Scholar] [CrossRef]
- Yang, L.; Zeng, X. Bézier curves and surfaces with shape parameters. Int. J. Comput. Math. 2009, 86, 1253–1263. [Google Scholar] [CrossRef]
- Ali, J.M. An Alternative Derivation of Said Basic Function. SainsMalaysiana 1994, 23, 16–25. [Google Scholar]
- Yan, L.; Liang, J. An extension of the Bézier model. Appl. Math. Comput. 2011, 218, 2863–2879. [Google Scholar] [CrossRef]
- Ahmad, A.; Amat, A.H.; MAli, J. A generalization of a Bézier-like curve. Educ.-J. Sci. Math. Technol. 2014, 1, 56–68. [Google Scholar]
- BiBi, S.; Abbas, M.; Misro, M.Y.; Hu, G. A Novel Approach of Hybrid Trigonometric Bezier Curve to the Modeling of SymmetricRevolutionary Curves and Symmetric Rotation Surfaces. IEEE Access 2019, 7, 165779–165792. [Google Scholar] [CrossRef]
- Qin, X.; Hu, G.; Zhang, N.; Shen, X.; Yang, Y. A novel extension to the polynomial basis functions describing Bezier curves and surfaces of degree n with multiple shape parameters. Appl. Math. Comput. 2013, 223, 1–16. [Google Scholar] [CrossRef]
- Hu, G.; Wei, G.; Wu, J. Shape Adjustable Generalized Bézier Rotation with the multiple shape parameters. Results Math. 2017, 72, 1281–1313. [Google Scholar] [CrossRef]
- Maqsood, S.; Abbas, M.; Hu, G.; Ramli, A.; Miura, K.T. A novel generalization of trigonometric Bézier curve and surface with shape parameters and its applications. Math. Probl. Eng. 2020, 2020, 4036434. [Google Scholar] [CrossRef]
- Maqsood, S.; Abbas, M.; Miura, K.T.; Majeed, A.; Hu, G.; Nazir, T. Shape-adjustable developable generalized blended trigonometric Bézier surfaces and their applications. Adv. Differ. Equ. 2021, 1, 459. [Google Scholar] [CrossRef]
- Maqsood, S.; Abbas, M.; Miura, K.T.; Majeed, A.; Iqbal, A. Geometric modeling and applications of generalized blended trigonometric Bézier curves with shape parameters. Adv. Differ. Equ. 2020, 1, 550. [Google Scholar] [CrossRef]
- Maqsood, S.; Abbas, M.; Miura, K.T.; Majeed, A.; BiBi, S.; Nazir, T. Geometric modeling of some engineering GBT-Bézier surfaces with shape parameters and their applications. Adv. Differ. Equ. 2021, 1, 490. [Google Scholar] [CrossRef]
- Chen, X.; Tan, J.; Liu, Z.; Xie, J. Approximation of functions by a new family of generalized Bernstein operators. J. Math. Anal. Appl. 2017, 450, 244–261. [Google Scholar] [CrossRef]
- Srivastava, H.M.; Ansari, K.J.; Özger, F.; Ödemis Özger, Z. A Link between Approximation Theory and Summability Methods via Four-Dimensional Infinite Matrices. Mathematics 2021, 9, 1895. [Google Scholar] [CrossRef]
- Cai, Q.B.; Aslan, R. On a New Construction of Generalized q-Bernstein Polynomials Based on Shape Parameter λ. Symmetry 2021, 13, 1919. [Google Scholar] [CrossRef]
- Hiemstra, R.R.; Hughes, T.J.; Manni, C.; Speleers, H.; Toshniwal, D. A Tchebycheffian Extension of Multidegree B-Splines: Algorithmic Computation and Properties. Siam J. Numer. Anal. 2020, 58, 1138–1163. [Google Scholar] [CrossRef]
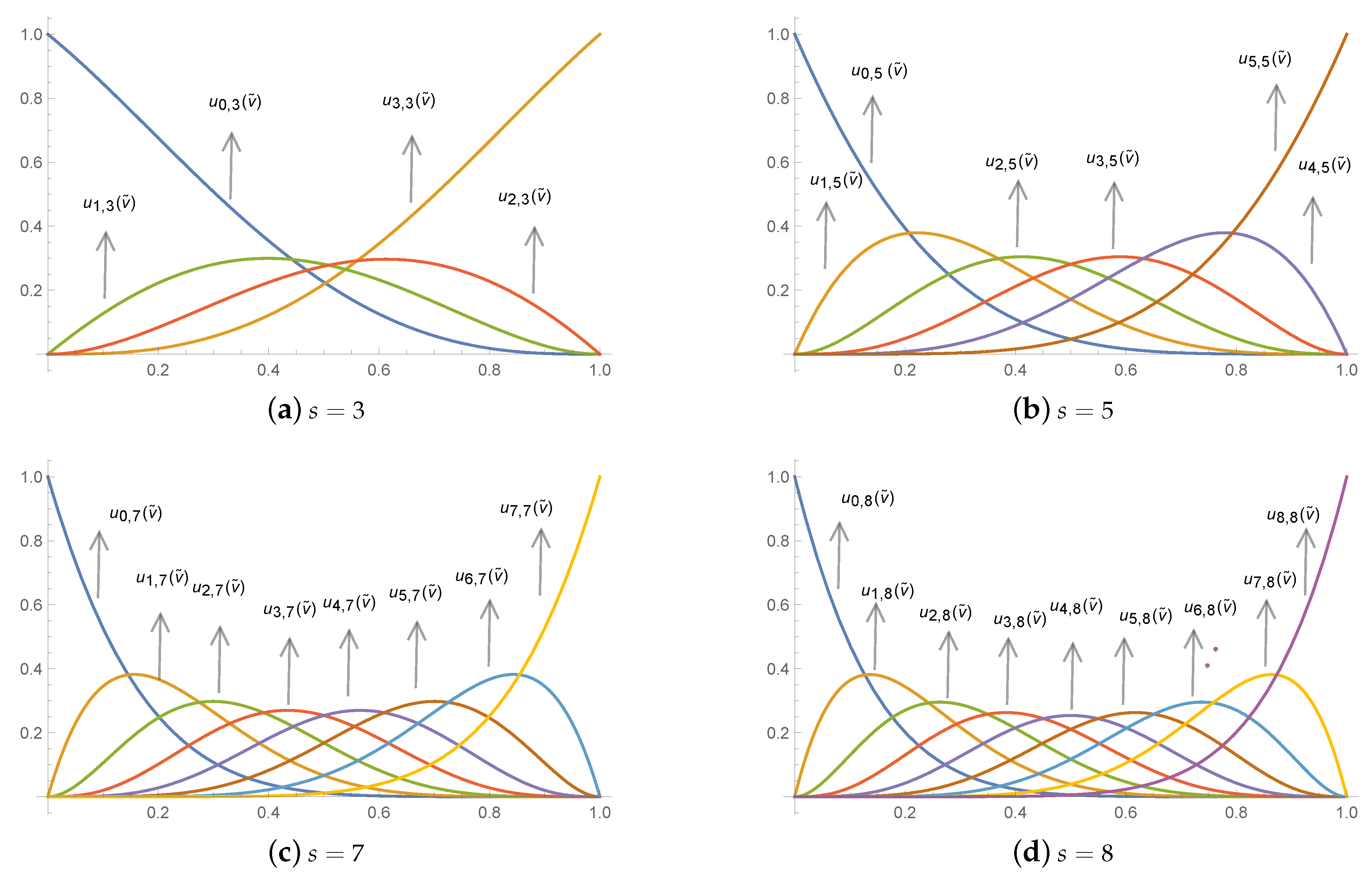


| Sr. No | Existing Method | Proposed Method |
|---|---|---|
| 1 | Qin et al. [22] presented the basis functions of order n with shape parameters. | The proposed basis functions are constructed with only two shape parameters. |
| 2 | The degeneracy of basis functions are difficult to prove such as in [21,24]. | We can easily degenerate the classical Bernstein basis function from proposed basis functions. |
| 3 | The linearity of GHT-Bernstein basis functions are not easy to prove, such as in [21]. | The proposed basis function are linearly independent. |
| 4 | Azhar et al. [20] generalized the A-Bézier for degree . | We generalized the proposed basis function for degree . |
| 5 | In [21], they used four different shape parameters with different intervals. | We used two parameters with the same interval. |
| Degree s | Proposed gBBF | Samia et al. [21] | Sidra et al. [24] | Sidra et al. [25] |
|---|---|---|---|---|
| 3 | 0.062500 | 0.203125 | 0.312500 | 0.15625 |
| 7 | 0.000000 | 0.015625 | 0.015625 | 0.015625 |
| 9 | 0.015625 | 0.046875 | 0.015625 | 0.046875 |
| 11 | 0.031250 | 0.156250 | 0.125000 | 0.062500 |
| 13 | 0.156250 | 0.546875 | 0.546875 | 0.328125 |
| 15 | 0.781250 | 1.937500 | 2.046880 | 1.859380 |
| Degree s | Proposed gBBF | Samia et al. [21] | Sidra et al. [24] | Sidra et al. [25] |
|---|---|---|---|---|
| 3 | 0.062500 | 0.203125 | 0.312500 | 0.15625 |
| 5 | 0.140625 | 0.265600 | 0.265600 | 0.50000 |
| 7 | 0.281250 | 0.843750 | 0.869375 | 1.04688 |
| 9 | 1.437500 | 3.347500 | 3.390630 | 3.20313 |
| 11 | 6.156250 | 14.32810 | 14.04690 | 13.1875 |
| 13 | 26.70130 | 62.15630 | 58.92190 | 49.8750 |
| 15 | 124.5000 | 269.4690 | 263.4380 | 210.578 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ameer, M.; Abbas, M.; Abdeljawad, T.; Nazir, T. A Novel Generalization of Bézier-like Curves and Surfaces with Shape Parameters. Mathematics 2022, 10, 376. https://doi.org/10.3390/math10030376
Ameer M, Abbas M, Abdeljawad T, Nazir T. A Novel Generalization of Bézier-like Curves and Surfaces with Shape Parameters. Mathematics. 2022; 10(3):376. https://doi.org/10.3390/math10030376
Chicago/Turabian StyleAmeer, Moavia, Muhammad Abbas, Thabet Abdeljawad, and Tahir Nazir. 2022. "A Novel Generalization of Bézier-like Curves and Surfaces with Shape Parameters" Mathematics 10, no. 3: 376. https://doi.org/10.3390/math10030376
APA StyleAmeer, M., Abbas, M., Abdeljawad, T., & Nazir, T. (2022). A Novel Generalization of Bézier-like Curves and Surfaces with Shape Parameters. Mathematics, 10(3), 376. https://doi.org/10.3390/math10030376







