Norms That Regulate the Theorem Construction Process in an Inquiry Classroom of 3D Geometry: Teacher’s Management to Promote Them
Abstract
:1. Introduction
2. Conceptual Framework
2.1. Argument and Argumentation
2.2. Theorem Construction Process
2.3. Norm
2.4. Teacher’s Professional Actions
3. Methodology
3.1. Context and Participants
3.2. Data Collection
3.3. Analysis
4. Results
4.1. Episode A. Actions of Armando–Juan–Valentín Group to Solve the Problem
4.2. Episode B. Professional Actions by Teacher
4.3. Episode C. Proof of Conjectures
4.4. Episode D. Actions Became Norms
5. Discussion and Final Remarks
Author Contributions
Funding
Informed Consent Statement
Conflicts of Interest
References
- Yackel, E.; Cobb, P. Sociomathematical Norms, Argumentation, and Autonomy in Mathematics. J. Res. Math. Educ. 1996, 27, 458–477. [Google Scholar] [CrossRef]
- Makar, K.; Bakker, A.; Ben-Zvi, D. Scaffolding norms of argumentation-based inquiry in a primary mathematics classroom. ZDM 2015, 47, 1107–1120. [Google Scholar] [CrossRef] [Green Version]
- Assis, A.; Godino, J.D.; Frade, C. As dimensões normativa e metanormativa em um contexto de aulas exploratório-investigativas. Rev. Latinoam. Investig. Matemática Educ. 2012, 15, 171–198. [Google Scholar]
- Herbst, P.; Chen, C.; Weiss, M.; González, G.; Nachlieli, T.; Hamlin, M.; Brach, C. “Doing proofs” in Geometry Classrooms. In Teaching and Learning of Proof across the Grades: A K-16 Perspective; Blanton, M., Stylianou, D., Knuth, E., Eds.; Routledge: New York, NY, USA, 2009; pp. 250–268. [Google Scholar]
- Van Zoest, L.; Stockero, S.; Taylor, C. The durability of professional and sociomathematical norms intentionally fostered in an early pedagogy course. J. Math. Teach. Educ. 2012, 15, 293–315. [Google Scholar] [CrossRef]
- Conner, A.; Singletary, L.; Smith, R.; Francisco, R. Teacher support for collective argumentation: A framework for examining how teachers support students’ engagement in mathematical activities. Educ. Stud. Math. 2014, 86, 401–429. [Google Scholar] [CrossRef]
- Makar, K.; Fielding-Wells, J. Shifting more than the goal posts: Developing classroom norms of inquiry-based learning in mathematics. Math. Educ. Res. J. 2018, 30, 53–63. [Google Scholar] [CrossRef] [Green Version]
- Yackel, E. What we can learn from analyzing the teacher’s role in collective argumentation. J. Math. Behav. 2002, 21, 423–440. [Google Scholar] [CrossRef]
- Godino, J.; Batanero, C.; Font, V. The onto-semiotic approach to research in mathematics education. Z. Didaktik der Math. 2007, 39, 1–2, 127–135. [Google Scholar] [CrossRef] [Green Version]
- Font, V.; Godino, J.; Gallardo, J. The emergence of objects from mathematical practices. Educ. Stud. Math. 2013, 82, 97–124. [Google Scholar] [CrossRef]
- Krummheuer, G. The ethnography of argumentation. In The Emergence of Mathematical Meaning: Interaction in Classroom Cultures; Cobb, P., Bauersfeld, H., Eds.; Lawrence Erlbaum Associates: Hillsdale, NJ, USA, 1995; pp. 229–269. [Google Scholar]
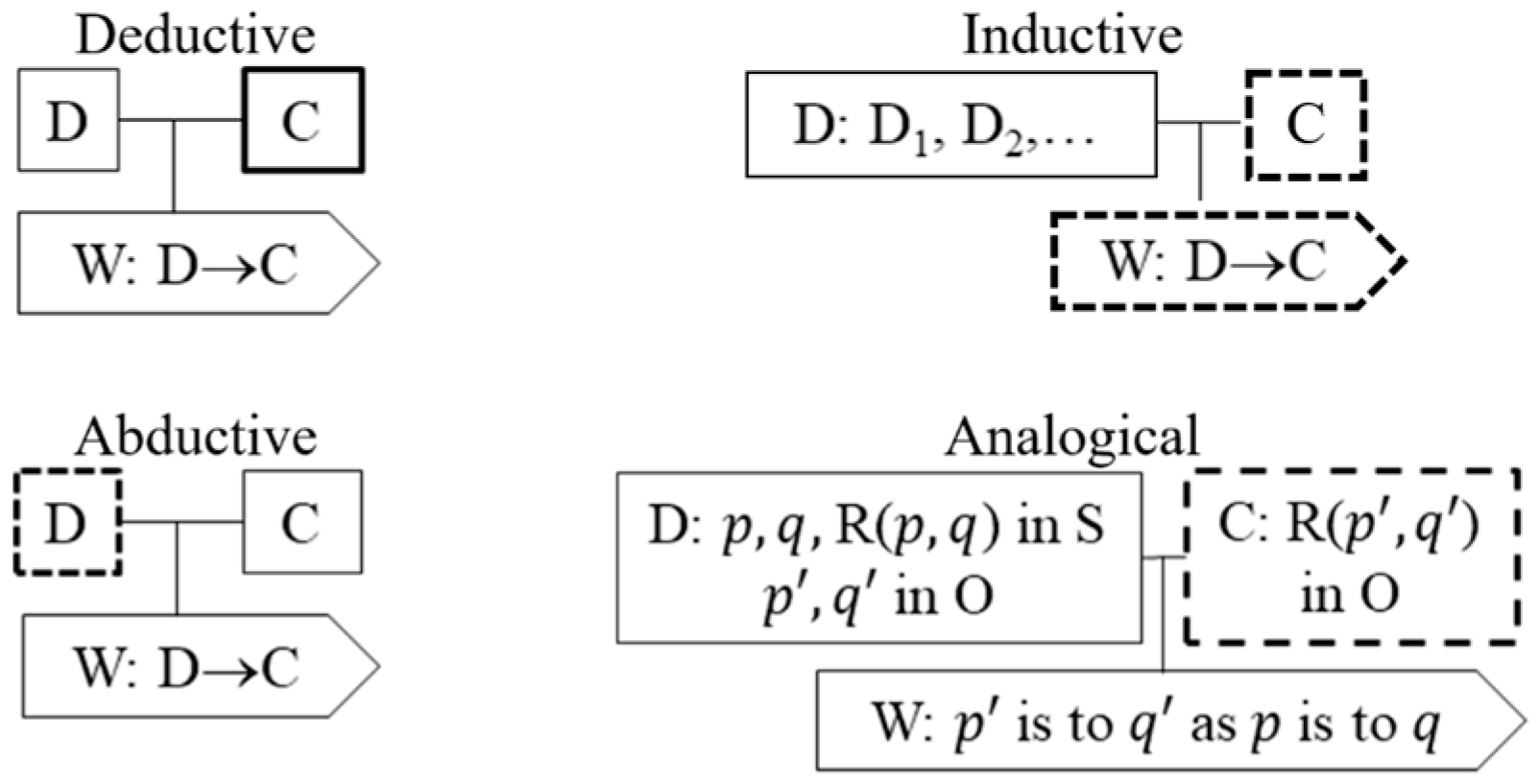
- Molina, O.; Pino-Fan, L.; Font, V. Estructura y dinámica de argumentos analógicos, abductivos y deductivos: Un curso de geometría del espacio como contexto de reflexión. Enseñanza de las Ciencias 2019, 37, 93–116. [Google Scholar]
- Toulmin, S. The Uses of Arguments, 1st ed.; Cambridge University Press: Cambridge, UK, 2003. [Google Scholar]
- Pedemonte, B. How can the relationship between argumentation and proof be analysed. Educ. Stud. Math. 2007, 66, 23–41. [Google Scholar] [CrossRef]
- Juthe, A. Argument by analogy. Argumentation 2005, 19, 1–27. [Google Scholar] [CrossRef]
- Stylianides, A.; Bieda, K.; Morselli, F. Proof and Argumentation in Mathematics Education Research. In The Second Handbook of Research on the Psychology of Mathematics Education; Guitérrez, A., Leder, G., Boero, P., Eds.; Sense Publishers: Rotterdam, The Netherlands, 2016; pp. 315–352. [Google Scholar]
- Becker, J.P.; Shimada, S. The Open-Ended Approach: A New Proposal for Teaching Mathematics; National Council of Teachers of Mathematics: Reston, VA, USA, 1997. [Google Scholar]
- Baccaglini-Frank, A.; Mariotti, M. Generating Conjectures in Dynamic Geometry: The Maintaining Dragging Model. Int. J. Comput. Math. Learn. 2010, 15, 225–253. [Google Scholar] [CrossRef]
- Mariotti, M.; Bartolini Bussi, M.; Boero, P.; Ferri, F.; Garuti, R. Approaching geometry theorems in contexts: From history and epistemology to cognition. In Proceedings of the 21st Conference of the International Group for the Psychology of Mathematics Education, 1; PME: Lahti, Finland, 1997; pp. 180–195. [Google Scholar]
- Godino, J.; Font, V.; Wilhelmi, M.; Castro, C. Aproximación a la dimensión normativa en Didáctica de la Matemática desde un enfoque ontosemiótico. Enseñanza de las Ciencia 2009, 27, 59–76. [Google Scholar]
- Brousseau, G. The Didactical Contract: The Teacher, the Student and the Milieu. In Theory of Didactical Situations in Mathematics. Mathematics Education Library; Springer: Dordrecht, The Netherlands, 2002; pp. 226–249. [Google Scholar]
- Voigt, J. Patterns and routines in classroom interaction. Rech. Didact. Mathématiques 1985, 6, 69–118. [Google Scholar]
- Pino-Fan, L.; Godino, J.; Font, V. Assessing key epistemic features of didactic-mathematical knowledge of prospective teachers: The case of the derivative. J. Math. Teach. Educ. 2018, 21, 63–94. [Google Scholar] [CrossRef]
- Arzarello, F.; Olivero, F.; Paola, D.; Robutti, O. A cognitive analysis of dragging practises in Cabri environments. Z. Didakt. Der Math. 2002, 34, 66–72. [Google Scholar] [CrossRef]
- Breda, A.; Hummes, V.; Sychocki, R.; Sánchez, A. El papel de la fase de observación de la implementación en la metodología estudio de clases. Bolema 2021, 35, 263–288. [Google Scholar] [CrossRef]
- Nathan, M.; Knuth, E. A Study of Whole Classroom Mathematical Discourse and Teacher Change. Cogn. Instr. 2003, 21, 175–207. [Google Scholar] [CrossRef]
- Mammana, M.F.; Micale, B.; Pennisi, M. Analogy and dynamic geometry system used to introduce three-dimensional geometry. Int. J. Math. Educ. Sci. Technol. 2012, 43, 818–830. [Google Scholar] [CrossRef]
- Conner, A.; Singletary, L.; Smith, R.; Wagner, P.; Francisco, R. Identifying kinds of reasoning in collective argumentation. Math. Think. Learn. 2014, 16, 181–200. [Google Scholar] [CrossRef]
- Stylianides, G.; Stylianides, A.; Weber, K. Research on the teaching and learning of proof: Taking stock and moving forward. In The First Compendium for Research in Mathematics Education; Cai, J., Ed.; National Council of Teachers of Mathematics: Reston, VA, USA, 2017; pp. 237–266. [Google Scholar]
- Sala, G.; Barquero, B.; Font, V. Inquiry and Modeling for Teaching Mathematics in Interdisciplinary Contexts: How Are They Interrelated? Mathematics 2021, 9, 1714. [Google Scholar] [CrossRef]

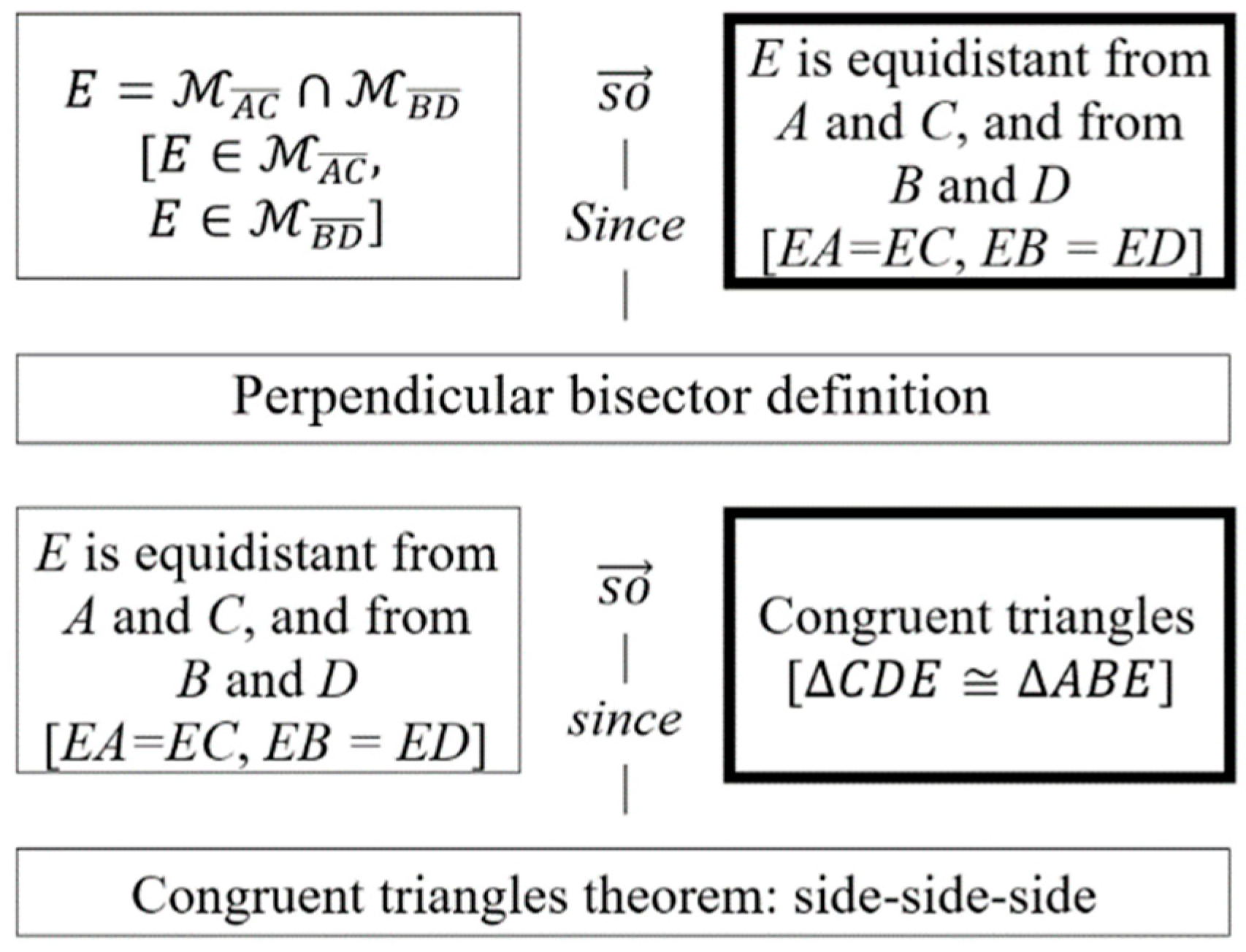

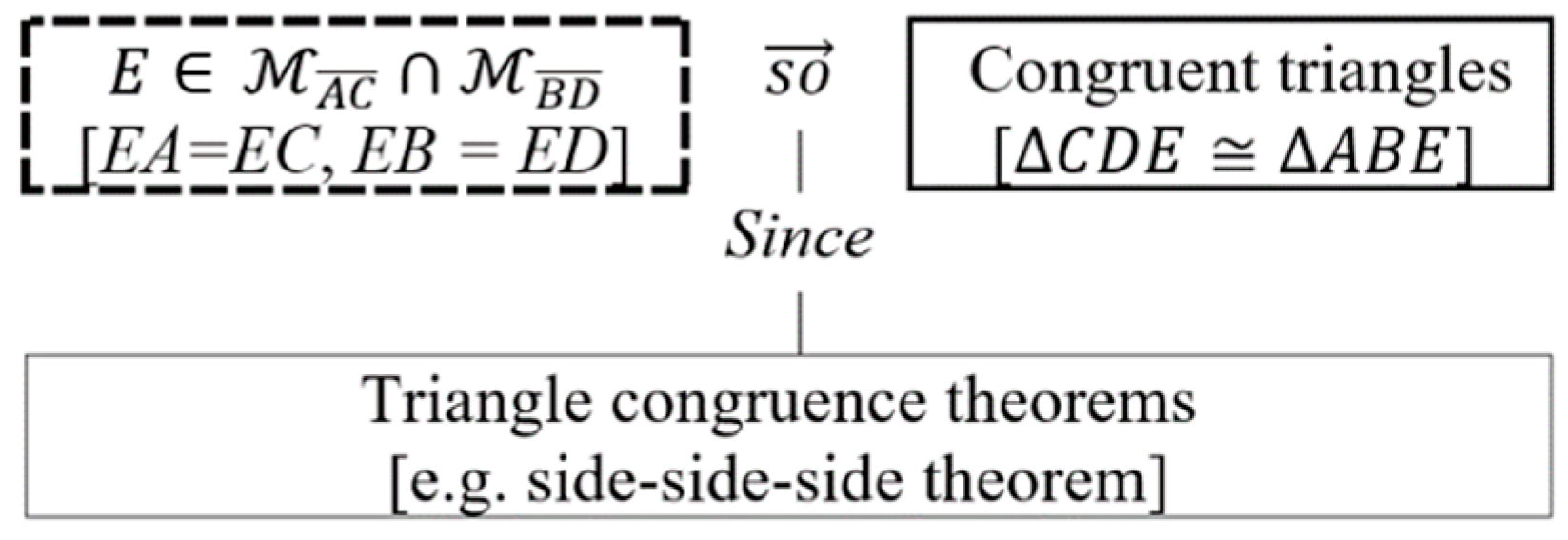
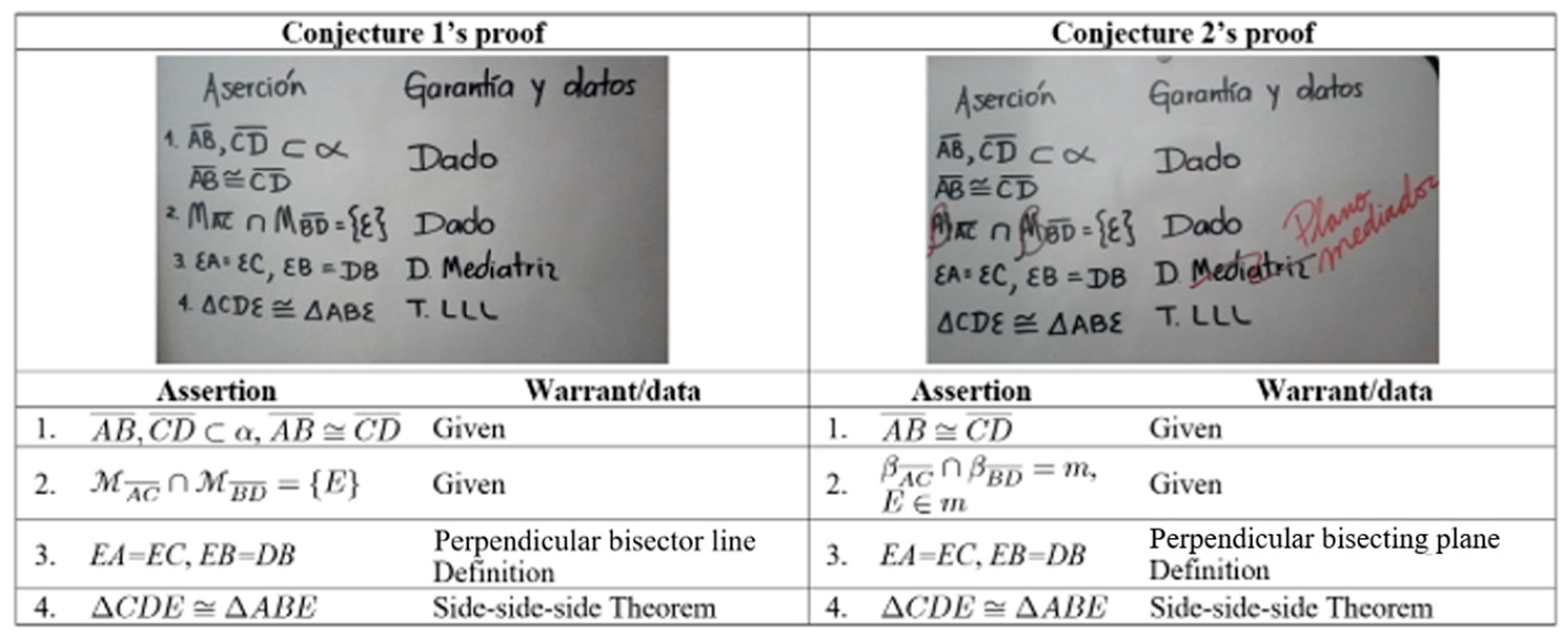
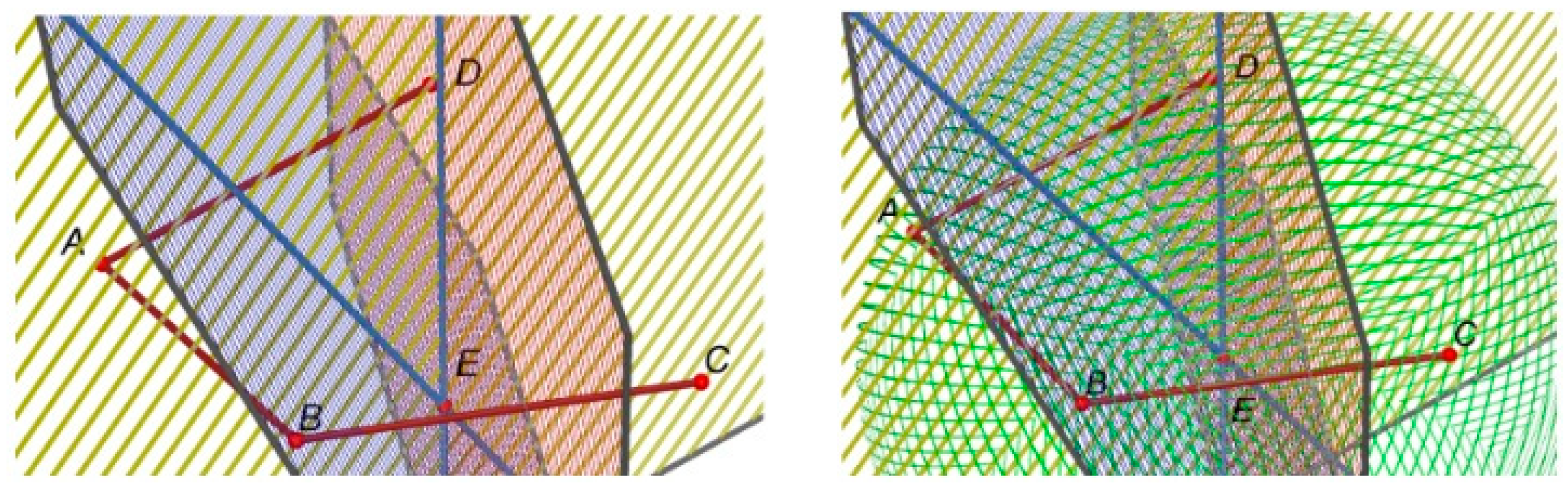
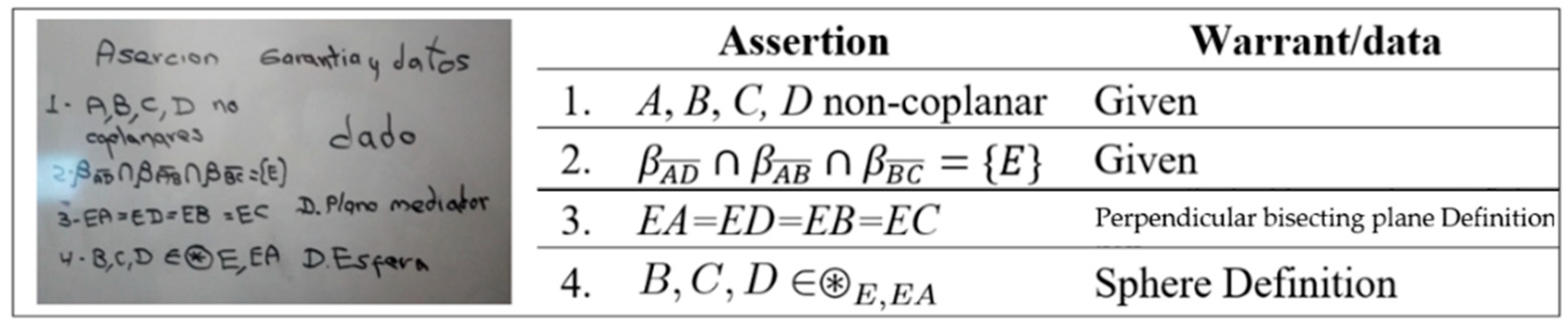
| Practices: Solving Open-Ended Problems and Formulating Conjectures | |
|---|---|
| Meta-norm (epistemic) | To solve an open-ended problem, it is necessary to: |
| Procedure |
|
| Practice: Proving A Conjecture | |
| Norm (epistemic) | To elaborate a proof of a conditional proposition, it is necessary to: |
| Procedure |
|
| Elements | Tools |
|---|---|
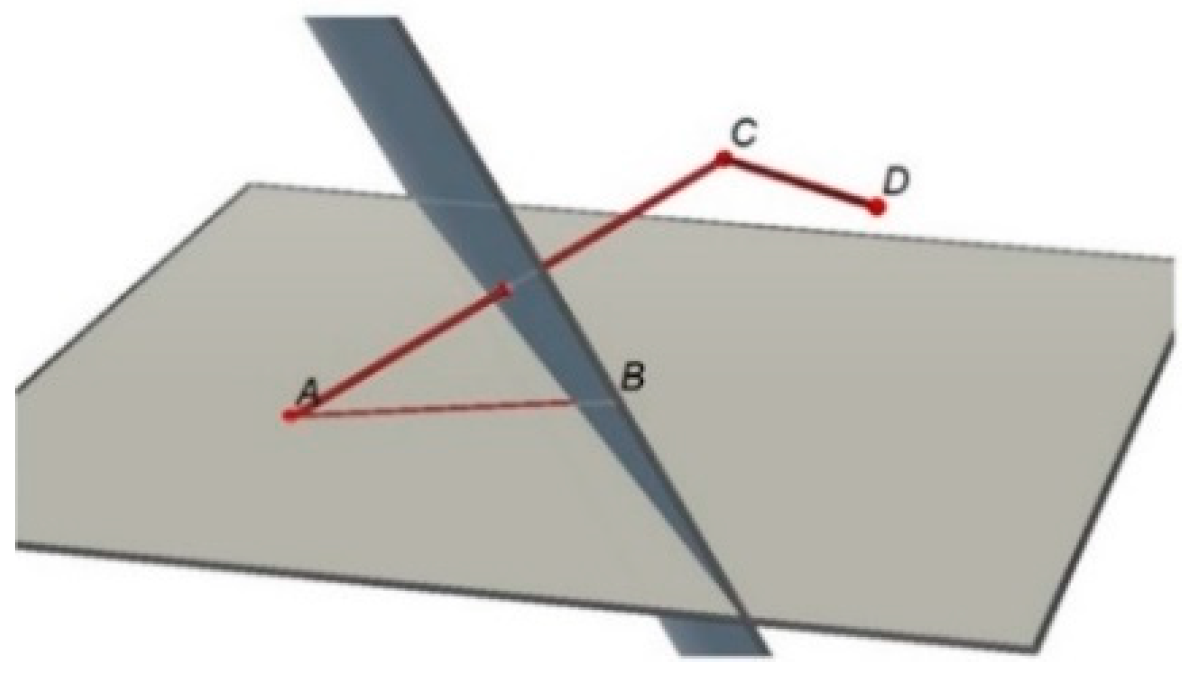
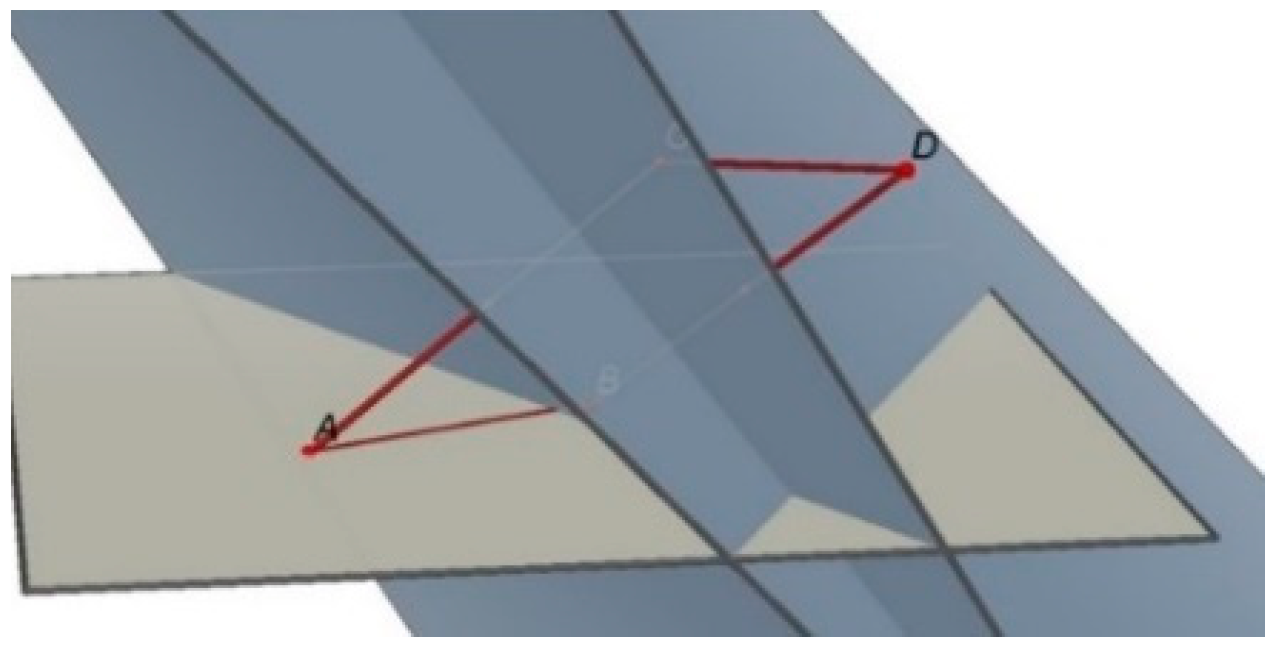
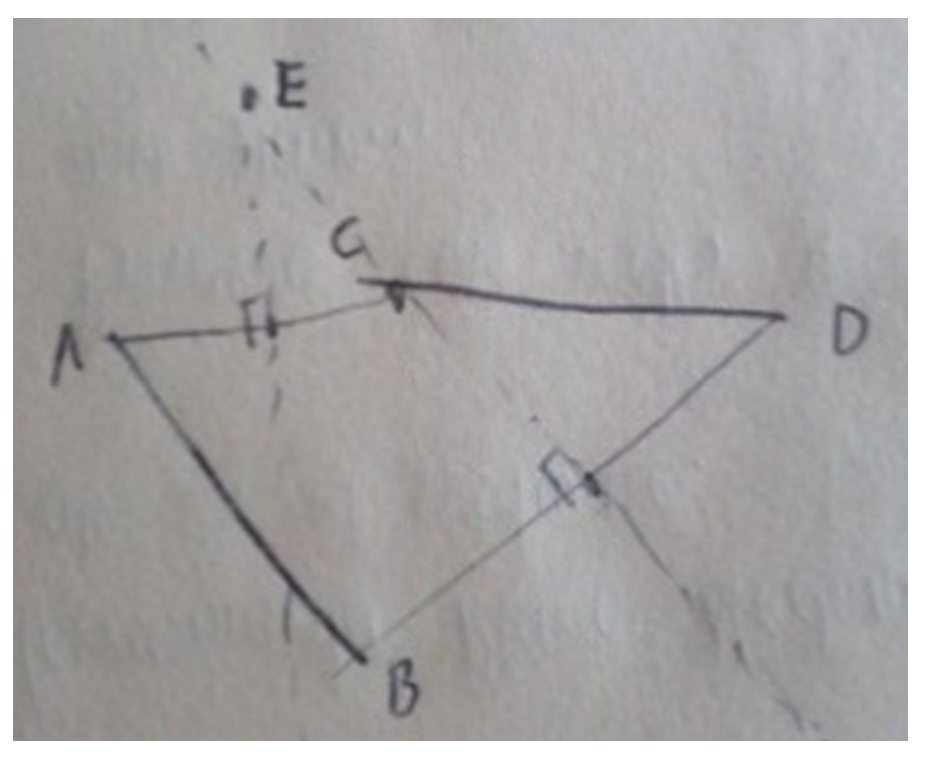
| Identification of practices and norms: the analysis of practices was focused on the identification and description of mathematical practices such as solving open-ended problems, formulating conjectures, and proving them. Taking these practices as a reference, we focus on determining norms that involve issues about the legitimacy of arguments. | We used the OSA proposal about normative dimension [20] to enunciate and typify the norms identified. In the section “Results”, we use codes to indicate actions that could be reiterative and potentially become norms. For actions referring to solving open-ended problems, formulating a conjecture, and proving the conjecture practices, we use, respectively, the codes Solve, Formulate, and Prove followed by a number to indicate a specific norm. At the end of the analysis of each episode, we present tables describing the possible norms identified (i.e., coded actions suggesting norms), indicating the type of the possible norm. To illustrate the codification of actions, we present the following examples. (1) The action “Armando told Juan which objects he should build. Juan had already built the congruent non-coplanar segments” was typified as Solve 1. This is because it alludes to the norm “Use the DGS to construct the objects given in the statement” of the solving open-ended problems practice. (2) The action “the conjectures that emerged after using abductive and analogical arguments, once legitimized, were as follows. In both cases, what is inferred from the argument is the antecedent of the conjecture; the given property is the consequent” was typified as Formulate 1. This is because it alludes to the norm “The data inferred from the abductive argument are placed as the antecedent of the conjecture; the given property is set as the consequent” of the formulating a conjecture practice. (3) The action “determining the data ( are coplanar) and the thesis of the conditional to be proved (∆CDE ≅ ∆ABE)” was typified as Prove 1. This is due to the fact that it alludes to the norm “Determine the data and the thesis of the conditional to be proved” of the proving a conjecture practice. |
| Identification of primary objects: this analysis focuses on specifying the primary objects that emerge from a specific practice. For this case, we focus on the arguments that emerge from the indicated practices. | We used Toulmin’s basic model to typified arguments as deductive, inductive, analogical, or abductive. |
| Description of interactions: this analysis focuses on specifying the teacher’s professional actions to promote student learning; in this case, we concentrate on determining professional actions that encouraged the establishment of norms. | Although we had in mind the actions proposed by Makar et al. [2], Assis et al. [3], Yackel and Cobb [1], Yackel [8], and Conner et al. [6], our intention was to identify more detailed actions that specify actions as the following ones: remembering the norms frequently, highlighting exemplary student actions, modeling expectations with their actions, posing open-ended problems, asking students to share their ideas with the whole class, and asking students opinions about others’ ideas (conjectures, arguments). To present the results, we used the code TA and a number to indicate a specific professional action (e.g., TA1 indicates the first specific professional action identified). |
| Practices | Students’ Action | Type of Possible Norm |
|---|---|---|
| Solving open-ended problems | Solve 1. Identify the conditions and questions given in the task statement. Solve 2. Use the DGS to construct the objects given in the statement. Solve 4. Consider an analogy that relates 2D and 3D domains and infer a solution in the 3D domain by means of an analogical argument. Of course, a solution in the 2D domain is known and validated. Solve 3. Write a report of the productions; in this case, of the construction procedure. | Epistemic meta-norm: this set of actions could be a meta-norm that alludes to how to perform mathematics; in this case, to how to approach the solution of a problem with a DGS (meta-level aspect). In particular, Solve 2 could be a mediational norm that encourages the use of a DGS to construct objects involved in a problem. |
| Proving a statement/conjecture | Prove 1. Determine the data (antecedent) and the thesis (consequent) of the conditional to be proved. Prove 2. Determine the elements of the corresponding theoretical system that are used as a warrant for each argumentative step. Prove 3. Configure a deductive chain of arguments that responds to a specific way of proceeding (direct proof in this case). | Epistemic norm: this set of actions regulates the proving a conjecture practice. |
| General Professional Actions | Specify Professional Actions | Actions Which Could Be Norms | Practice |
|---|---|---|---|
| Highlighting exemplary student actions | TA1. Assess the students’ actions as exemplary by explaining the usefulness of an analogical argument and when it is legitimate. | Solve 4—for problems that ask for enough conditions to be able to conclude a given property, considering an analogy that relates 2D and 3D domains and inferring a solution in the 3D domain by means of an analogical argument. Of course, a solution in the 2D domain is known and validated. If the inference effectively solves the problem, the analogical argument is legitimized. | Solving open-ended problems |
| Formulate 1. The data inferred from the abductive argument are set as the antecedent of the conjecture; the given property is placed as the consequent. | Formulating a conjecture | ||
| Modeling expectations with their actions | TA2. Model expectations with her actions, showing the usefulness of an abductive argument, and highlighting when it is legitimate. | Solve 5—for problems that ask for enough conditions to be able to conclude a given property, considering an abductive argument whose warrant is a proposition belonging to the theoretical system available, the assertion is the property given in the statement, and the inference (data) could be the conditions to solve the problem. If the data effectively solve the problem, the abductive argument is legitimized. | Solving open-ended problem |
| Formulate 2. The relation inferred from the analogical argument is placed as the antecedent of the conjecture; the given property is set as the consequent. | Formulating a conjecture |
| General Professional Actions | Specify Professional Actions | Actions Which Could Be Norms |
|---|---|---|
| All Practices | ||
| Posing open-ended problems | TA3. Posing open-ended problems to provoke the need to introduce new objects into the theoretical system. | Prove 4. Defining an object and proving its existence is necessary to be able to use it legitimately in mathematical practices. |
| Proving a Conjecture Practice | ||
| Highlighting exemplary student actions | TA1. Assess the students’ actions as exemplary by explaining the usefulness of an analogical argument and when it is legitimate. | Prove 7. Regarding 3D geometry: if an analogical argument was used in the solution of a problem and in proofs relative to the 2D domain, then analogous objects can be used in the proof in a 3D domain in a similar deductive chain. |
| Modeling expectations with their actions | TA2. Modeling expectations with her actions, showing the usefulness of an abductive argument and highlighting when it is legitimate. | Prove 5. The warrants of abductive arguments arising in the solution of a problem can be used to determine the warrants of deductive arguments in a proof. |
| Solving Open-Ended Problems Practice |
|---|
| Epistemic Meta-Norm 1: To solve an open problem, it is necessary to: Solve 1. Identify the conditions and questions given in the task statement. Solve 2. Use the DGS to construct the objects given in the statement. Solve 4. For problems that ask for conditions to conclude a given property, (i) consider an analogy that relates 2D and 3D domains and infer a solution in the 3D domain by means of an analogical argument. Of course, a solution in the 2D domain must be known and validated. If the inference effectively solves the problem, the analogical argument is legitimized. (ii) Make a construction in a DGS that involves the objects that solve the problem to verify their effectiveness. Or Solve 5. For problems that ask for conditions to conclude a given property, (i) consider an abductive argument whose warrant is a proposition belonging to the theoretical system available, the assertion is the property given in the statement, and the inference (data) are the sufficient conditions needed to solve the problem. If the data effectively solves the problem, the abductive argument is legitimized. (ii) Make a construction in DGS that involves the objects that solve the problem to verify their effectiveness. Solve 3. Present a written report of their productions, in this case, of the construction procedure. |
| Formulating A Conjecture Practice |
| Epistemic Meta-Norm 2: To formulate a conjecture, it is necessary to: Formulate 1. The data inferred from the abductive argument must be set as the antecedent of the conjecture, and the given property must be placed as the consequent. This fact legitimizes the abductive argument. Or Formulate 2. The relation inferred from the analogical argument must be placed as the antecedent of the conjecture and the given property set as the consequent. These facts legitimize the analogical argument. |
| Proving A Conjecture Practice |
| Epistemic Norm 3: To elaborate a proof of a conditional proposition, it is necessary to: Prove 1. Determine the data and the thesis of the conditional to be proved. Prove 2. Determine the elements of the corresponding theoretical system that can be used as a warrant for each argumentative step. Prove 3. Configure a deductive chain of arguments that responds to a specific way of proceeding (direct in this case). Prove 4. Define an object and prove its existence to be able to use it legitimately in mathematical practices. |
| Epistemic Meta-Norm 4: If an abductive argument was used in the solution of a conjecture problem, then this abductive argument can be used to: Prove 5. Provide the warrants of the deductive arguments in a proof. Prove 6. Elaborate a deductive chain of a proof, but in a “different direction” (i.e., the deductive chain starts with the inference (data) of the abductive argument, while assertion and warrants remain the same). These facts legitimize the abductive argument. Regarding 3D geometry: Prove 7. If an analogical argument was used in the solution of a problem and in proofs relative to 2D domain, then analogous objects can be used in the proof in a 3D domain in a similar deductive chain. This fact legitimizes the analogical argument. |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Molina, O.; Font, V.; Pino-Fan, L. Norms That Regulate the Theorem Construction Process in an Inquiry Classroom of 3D Geometry: Teacher’s Management to Promote Them. Mathematics 2021, 9, 2296. https://doi.org/10.3390/math9182296
Molina O, Font V, Pino-Fan L. Norms That Regulate the Theorem Construction Process in an Inquiry Classroom of 3D Geometry: Teacher’s Management to Promote Them. Mathematics. 2021; 9(18):2296. https://doi.org/10.3390/math9182296
Chicago/Turabian StyleMolina, Oscar, Vicenç Font, and Luis Pino-Fan. 2021. "Norms That Regulate the Theorem Construction Process in an Inquiry Classroom of 3D Geometry: Teacher’s Management to Promote Them" Mathematics 9, no. 18: 2296. https://doi.org/10.3390/math9182296
APA StyleMolina, O., Font, V., & Pino-Fan, L. (2021). Norms That Regulate the Theorem Construction Process in an Inquiry Classroom of 3D Geometry: Teacher’s Management to Promote Them. Mathematics, 9(18), 2296. https://doi.org/10.3390/math9182296







