New Approaches to the General Linearization Problem of Jacobi Polynomials Based on Moments and Connection Formulas
Abstract
:1. Introduction
- An approach based on deriving a new formula of the moments of the shifted normalized Jacobi polynomials in terms of their original shifted Jacobi polynomials but with different parameters;
- An approach based on making use of the connection formulas between two different normalized Jacobi polynomials.
- In the articles [28,31,34], the linearization formulas were established by reducing some exiting ones in the literature with the aid of some celebrated reduction formulas or via some symbolic algorithms; however, in the current article, we establish two new approaches for deriving some linearization formulas, and after that reduce these linearization formulas by symbolic computation.
- The articles [9,29,30] deal with some special linearization formulas. In fact, the approaches followed were based on expressing products of hypergeometric functions in terms of a single generalized hypergeometric function using some suitable transformation formulas; however, the current article deals with some general linearization formulas.
- We do believe that the approach based on the moments formulas can be followed to establish linearization formulas of different orthogonal polynomials and not restricted to Jacobi polynomials.
2. Some Elementary Properties of the Classical Jacobi Polynomials and Their Shifted Ones
3. New Moments Formulas of the Shifted Normalized Jacobi Polynomials
4. A New Approach for Solving Jacobi Linearization Problem via Moments Formulas
5. Some New Linearization Formulas of Chebyshev Polynomials Using the Connection Coefficients Approach
6. Numerical Application on the Non-Linear Riccati Equation
6.1. Tau Algorithm for the Non-Linear Riccati Differential Equation
6.2. Numerical Tests
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Quintana, Y.; Ramírez, W.; Urieles, A. On an operational matrix method based on generalized Bernoulli polynomials of level m. Calcolo 2018, 55, 1–29. [Google Scholar] [CrossRef]
- Fitri, S.; Thomas, D.K.; Wibowo, R.B.E. Coefficient inequalities for a subclass of Bazilevič functions. Demonstratio Math. 2020, 53, 27–37. [Google Scholar] [CrossRef]
- Urieles, A.; Ortega, M.J.; Ramírez, W.; Vega, S. New results on the q-generalized Bernoulli polynomials of level m. Demonstratio Math. 2019, 52, 511–522. [Google Scholar] [CrossRef]
- Doha, E.H.; Abd-Elhameed, W.M.; Bassuony, M.A. On the coefficients of differentiated expansions and derivatives of Chebyshev polynomials of the third and fourth kinds. Acta Math. Sci. 2015, 35, 326–338. [Google Scholar] [CrossRef]
- Doha, E.H.; Abd-Elhameed, W.M. On the coefficients of integrated expansions and integrals of Chebyshev polynomials of third and fourth kinds. Bull. Malays. Math. Sci. Soc. 2014, 37, 383–398. [Google Scholar]
- Abd-Elhameed, W.M.; Alkenedri, A.M. Spectral solutions of linear and nonlinear BVPs using certain Jacobi polynomials generalizing third-and fourth-kinds of Chebyshev polynomials. CMES Comput. Model. Eng. Sci. 2021, 126, 955–989. [Google Scholar]
- Sánchez-Ruiz, J. Logarithmic potential of Hermite polynomials and information entropies of the harmonic oscillator eigenstates. J. Math. Phys. 1997, 38, 5031–5043. [Google Scholar] [CrossRef] [Green Version]
- Dehesa, J.S.; Finkelshtdein, A.M.; Sánchez-Ruiz, J. Quantum information entropies and orthogonal polynomials. J. Comput. Appl. Math. 2001, 133, 23–46. [Google Scholar] [CrossRef]
- Abd-Elhameed, W.M. New formulae between Jacobi polynomials and some fractional Jacobi functions generalizing some connection formulae. Anal. Math. Phys. 2019, 9, 73–98. [Google Scholar] [CrossRef]
- Abd-Elhameed, W.M. Novel expressions for the derivatives of sixth-kind Chebyshev polynomials: Spectral solution of the non-linear one-dimensional Burgers’ equation. Fractal Fract. 2021, 5, 74. [Google Scholar] [CrossRef]
- Askey, R.; Gasper, G. Linearization of the product of Jacobi polynomials. III. Canad. J. Math. 1971, 23, 332–338. [Google Scholar] [CrossRef]
- Gasper, G. Linearization of the product of Jacobi polynomials. I. Canad. J. Math. 1970, 22, 171–175. [Google Scholar] [CrossRef]
- Gasper, G. Linearization of the product of Jacobi polynomials. II. Canad. J. Math. 1970, 22, 582–593. [Google Scholar] [CrossRef]
- Hylleraas, E.A. Linearization of products of Jacobi polynomials. Math. Scan. 1962, 10, 189–200. [Google Scholar] [CrossRef] [Green Version]
- Rahman, M. A non-negative representation of the linearization coefficients of the product of Jacobi polynomials. Canad. J. Math. 1981, 33, 915–928. [Google Scholar] [CrossRef]
- Sánchez-Ruiz, J.; Dehesa, J.S. Some connection and linearization problems for polynomials in and beyond the Askey scheme. J. Comput. Appl. Math. 2001, 133, 579–591. [Google Scholar] [CrossRef]
- Chaggara, H.; Koepf, W. On linearization coefficients of Jacobi polynomials. Appl. Math. Lett. 2010, 23, 609–614. [Google Scholar] [CrossRef] [Green Version]
- Srivastava, H.M. A unified theory of polynomial expansions and their applications involving Clebsch-Gordan type linearization relations and Neumann series. Astrophys. Space Sci. 1988, 150, 251–266. [Google Scholar] [CrossRef]
- Srivastava, H.M.; Niukkanen, A.W. Some Clebsch-Gordan type linearization relations and associated families of Dirichlet integrals. Math. Comput. Model. 2003, 37, 245–250. [Google Scholar] [CrossRef]
- Niukkanen, A.W. Clebsch-Gordan-type linearisation relations for the products of Laguerre polynomials and hydrogen-like functions. J. Phy. A Math. Gen. 1985, 18, 1399. [Google Scholar] [CrossRef]
- Kim, T.; Kim, D.S.; Lee, H.; Kwon, J. Studies in sums of finite products of the second, third, and fourth kind Chebyshev polynomials. Mathematics 2020, 8, 210. [Google Scholar] [CrossRef] [Green Version]
- Dolgy, D.V.; Kim, D.S.; Kim, T.; Kwon, J. Connection problem for sums of finite products of Chebyshev polynomials of the third and fourth kinds. Symmetry 2018, 10, 617. [Google Scholar] [CrossRef] [Green Version]
- Kim, T.; Kim, D.S.; Dolgy, D.V.; Park, J.W. Sums of finite products of Legendre and Laguerre polynomials. Adv. Differ. Equ. 2018, 2018, 277. [Google Scholar] [CrossRef]
- Kim, T.; Kim, D.S.; Jang, L.C.; Dolgy, D.V. Representing by several orthogonal polynomials for sums of finite products of Chebyshev polynomials of the first kind and Lucas polynomials. Adv. Difference Equ. 2019, 2019, 162. [Google Scholar] [CrossRef]
- Kim, T.; Kim, D.S.; Jang, L.C.; Jang, G.W. Fourier series for functions related to Chebyshev polynomials of the first kind and Lucas polynomials. Mathematics 2018, 6, 276. [Google Scholar] [CrossRef] [Green Version]
- Kim, D.S.; Dolgy, D.V.; Kim, D.; Kim, T. Representing by orthogonal polynomials for sums of finite products of Fubini polynomials. Mathematics 2019, 7, 319. [Google Scholar] [CrossRef] [Green Version]
- Kim, D.S.; Kim, T. On sums of finite products of balancing polynomials. J. Comput. Appl. Math. 2020, 377, 112913. [Google Scholar] [CrossRef]
- Doha, E.H.; Abd-Elhameed, W.M. New linearization formulae for the products of Chebyshev polynomials of third and fourth kinds. Rocky Mountain J. Math. 2016, 46, 443–460. [Google Scholar] [CrossRef]
- Abd-Elhameed, W.M.; Doha, E.H.; Ahmed, H.M. Linearization formulae for certain Jacobi polynomials. Ramanujan J. 2016, 39, 155–168. [Google Scholar] [CrossRef]
- Abd-Elhameed, W.M. New product and linearization formulae of Jacobi polynomials of certain parameters. Integral Transforms Spec. Funct. 2015, 26, 586–599. [Google Scholar] [CrossRef]
- Abd-Elhameed, W.M. New formulas for the linearization coefficients of some nonsymmetric Jacobi polynomials. Adv. Differ. Equ. 2015, 2015, 168. [Google Scholar] [CrossRef] [Green Version]
- Sánchez-Ruiz, J. Linearization and connection formulae involving squares of Gegenbauer polynomials. Appl. Math. Lett. 2001, 14, 261–267. [Google Scholar] [CrossRef] [Green Version]
- Abd-Elhameed, W.M. New formulae of squares of some Jacobi polynomials via hypergeometric functions. Hacet. J. Math. Stat. 2017, 46, 165–176. [Google Scholar] [CrossRef]
- Abd-Elhameed, W.M.; Badah, B.M. New specific and general linearization formulas of some classes of Jacobi polynomials. Mathematics 2021, 9, 74. [Google Scholar] [CrossRef]
- Srivastava, H.M. Some Clebsch-Gordan type linearisation relations and other polynomial expansions associated with a class of generalised multiple hypergeometric series arising in physical and quantum chemical applications. J. Phys. A Math. Gen. 1988, 21, 4463. [Google Scholar] [CrossRef]
- Ahmed, H.M. Computing expansions coefficients for Laguerre polynomials. Integral Transform Spec. Funct. 2020. [Google Scholar] [CrossRef]
- Abd-Elhameed, W.M.; Youssri, Y.H. Neoteric formulas of the monic orthogonal Chebyshev polynomials of the sixth-kind involving moments and linearization formulas. Adv. Difference Equ. 2021, 2021, 84. [Google Scholar] [CrossRef]
- Markett, C. Linearization of the product of symmetric orthogonal polynomials. Constr. Approx. 1994, 10, 317–338. [Google Scholar] [CrossRef]
- Popov, B.S.; Srivastava, H.M. Linearization of a product of two polynomials of different orthogonal systems. Facta Univ. Ser. Math. Inform 2003, 18, 1–8. [Google Scholar]
- Olver, F.W.; Lozier, D.W.; Boisvert, R.F.; Clark, C.W. NIST Handbook of Mathematical Functions Hardback and CD-ROM; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Andrews, G.E.; Askey, R.; Roy, R. Special Functions; Cambridge University Press: Cambridge, UK, 1999. [Google Scholar]
- Rainville, E.D. Special Functions; The Maximalan Company: New York, NY, USA, 1960. [Google Scholar]
- Doha, E.H.; Abd-Elhameed, W.M.; Ahmed, H.M. The coefficients of differentiated expansions of double and triple Jacobi polynomials. Bull. Iranian Math. Soc. 2012, 38, 739–765. [Google Scholar]
- Mason, J.C.; Handscomb, D.C. Chebyshev Polynomials; Chapman and Hall: New York, NY, USA; CRC: Boca Raton, FL, USA, 2003. [Google Scholar]
- Koepf, W. Hypergeometric Summation: An Algorithmic Approach to Summation and Special Function Identities, 2nd ed.; Springer Universitext; Springer: London, UK, 2014. [Google Scholar]
- Van Hoeij, M. Finite singularities and hypergeometric solutions of linear recurrence equations. J. Pure Appl. Algebra 1999, 139, 109–131. [Google Scholar] [CrossRef] [Green Version]
- Doha, E.H.; Abd-Elhameed, W.M. Efficient spectral-Galerkin algorithms for direct solution of second-order equations using ultraspherical polynomials. SIAM J. Sci. Comput. 2002, 24, 548–571. [Google Scholar] [CrossRef]
- Mabood, F.; Ismail, A.; Hashim, I. Application of optimal homotopy asymptotic method for the approximate solution of Riccati equation. Sains Malays. 2013, 42, 863–867. [Google Scholar]
- Odibat, Z.; Momani, S. Modified homotopy perturbation method: Application to quadratic Riccati differential equation of fractional order. Chaos Solitons Fractals 2008, 36, 167–174. [Google Scholar] [CrossRef]
- Batiha, B.; Noorani, M.S.M.; Hashim, I. Application of variational iteration method to a generalRiccati equation. Int. Math. Forum 2007, 2, 2759–2770. [Google Scholar] [CrossRef] [Green Version]
- Sakar, M. Iterative reproducing kernel Hilbert spaces method for Riccati differential equations. J. Comput. Appl. Math. 2017, 309, 163–174. [Google Scholar] [CrossRef]
- Lakestani, M.; Dehghan, M. Numerical solution of Riccati equation using the cubic B-spline scaling functions and Chebyshev cardinal functions. Comput. Phys. Commun. 2010, 181, 957–966. [Google Scholar] [CrossRef]
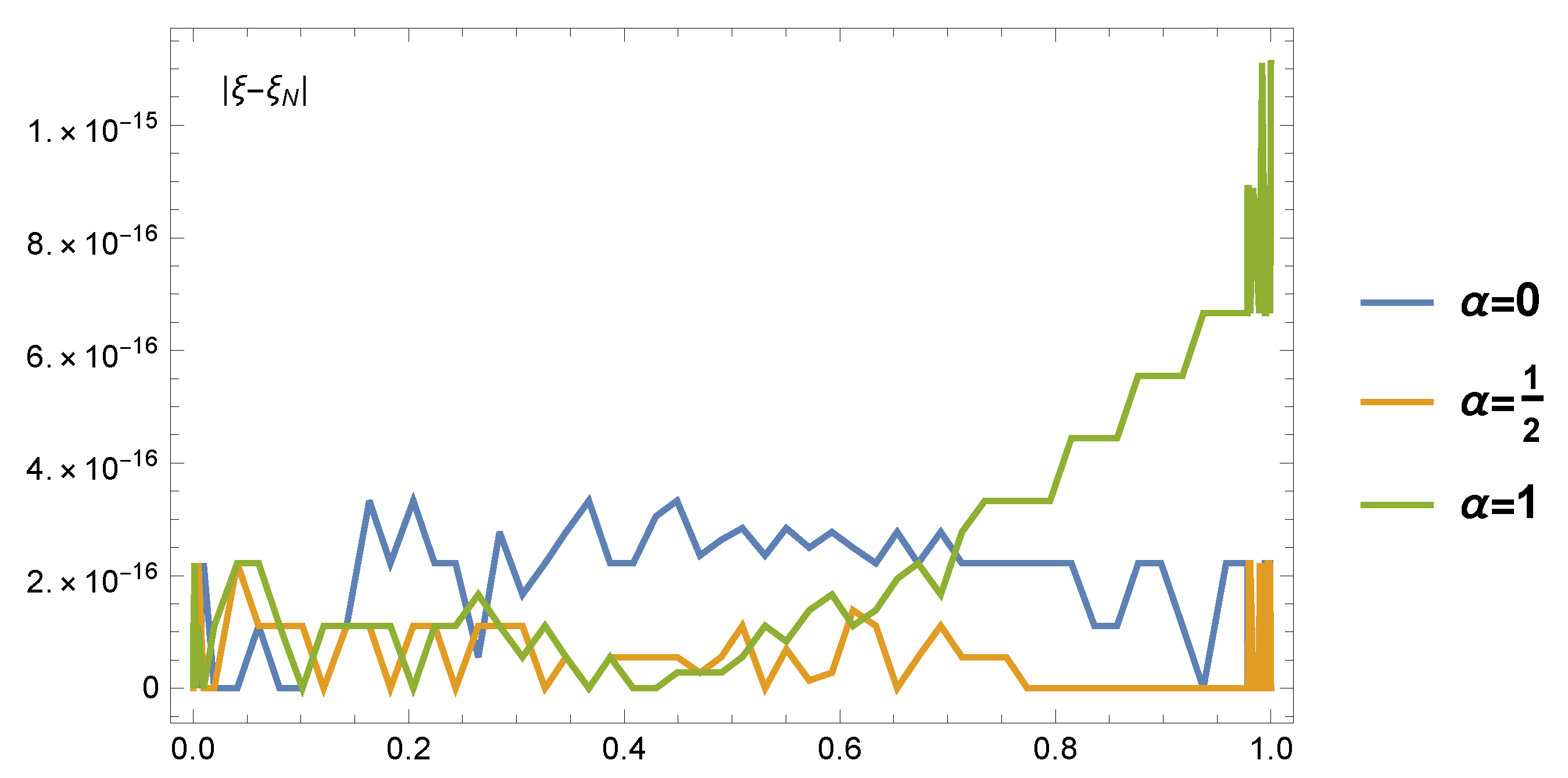
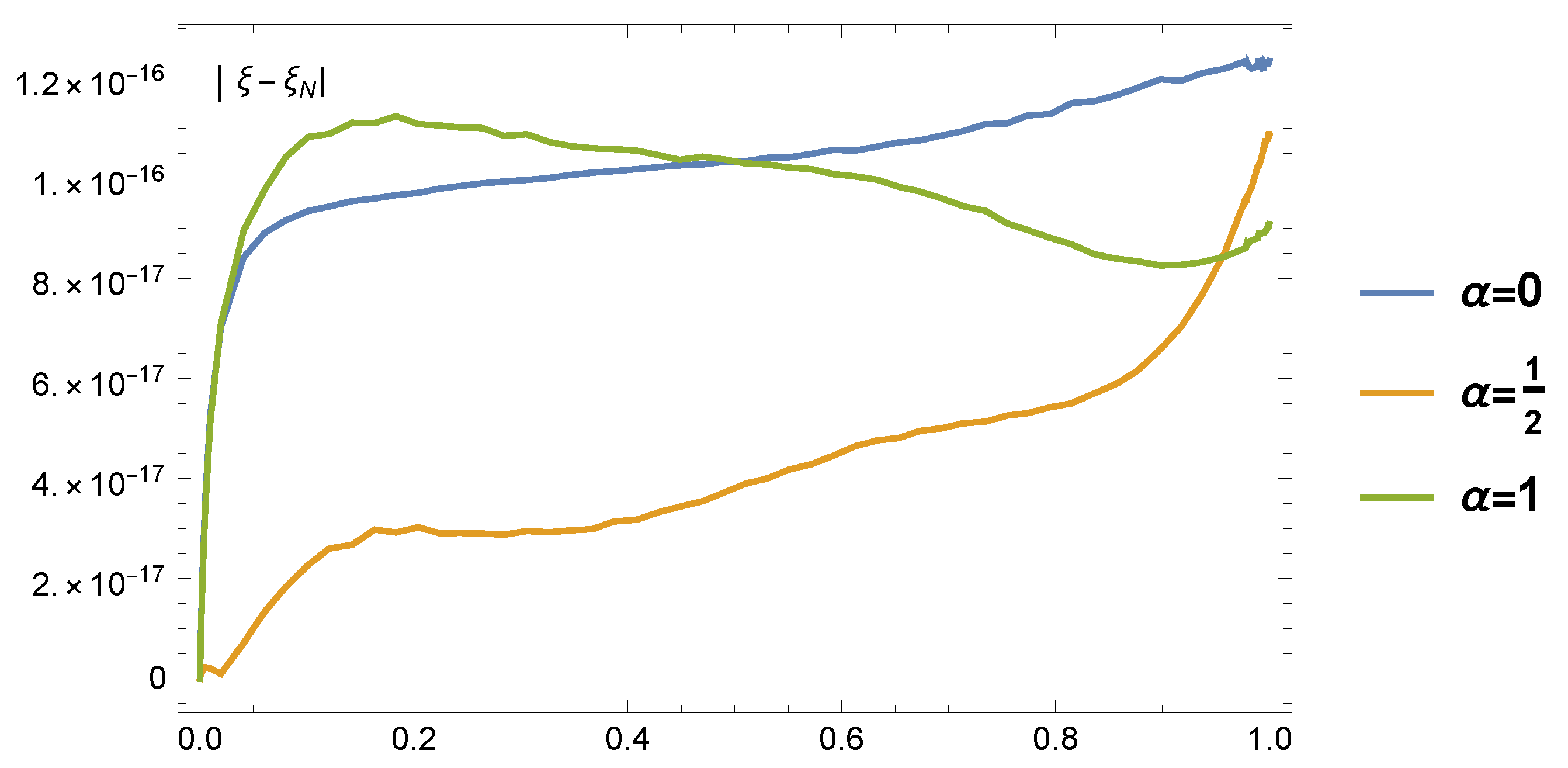
| N | 6 | 8 | 10 | 12 | 14 | 16 |
|---|---|---|---|---|---|---|
| E | 2.358 × | 3.264 × | 6.382 × | 5.943 × | 2.975 × | 6.241 × |
| x | OHAM [48] | MHPM [49] | VIM [50] | IRKHSM [51] | The Method in [9] | |
|---|---|---|---|---|---|---|
| 0 | 0 | 0 | 0 | 0 | 0 | 0 |
| 0.1 | 3.20 | 1.00 | 1.98 | 3.58 | 1.52 | 1.27 |
| 0.2 | 2.90 | 1.20 | 1.03 | 7.58 | 1.27 | 2.23 |
| 0.3 | 1.10 | 1.00 | 8.85 | 1.20 | 2.57 | 1.34 |
| 0.4 | 2.50 | 3.03 | 3.33 | 1.66 | 3.27 | 2.31 |
| 0.5 | 4.40 | 1.55 | 7.26 | 2.12 | 3.57 | 3.68 |
| 0.6 | 5.50 | 4.69 | 9.98 | 2.52 | 4.15 | 4.32 |
| 0.7 | 5.50 | 1.05 | 8.84 | 2.87 | 4.21 | 4.95 |
| 0.8 | 3.80 | 1.88 | 1.54 | 3.40 | 4.31 | 5.62 |
| 0.9 | 3.20 | 2.80 | 4.99 | 4.90 | 4.35 | 5.94 |
| 1.0 | 3.40 | 3.43 | 3.47 | 9.22 | 4.42 | 6.24 |
| N | 8 | 10 | 12 | 14 | 16 |
|---|---|---|---|---|---|
| 3.51 | 2.45 | 2.39 | 5.94 | 5.37 | |
| 1.38 | 3.27 | 8.36 | 4.62 | 3.74 | |
| 4.58 | 3.19 | 4.94 | 6.84 | 2.22 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abd-Elhameed, W.M.; Badah, B.M. New Approaches to the General Linearization Problem of Jacobi Polynomials Based on Moments and Connection Formulas. Mathematics 2021, 9, 1573. https://doi.org/10.3390/math9131573
Abd-Elhameed WM, Badah BM. New Approaches to the General Linearization Problem of Jacobi Polynomials Based on Moments and Connection Formulas. Mathematics. 2021; 9(13):1573. https://doi.org/10.3390/math9131573
Chicago/Turabian StyleAbd-Elhameed, Waleed Mohamed, and Badah Mohamed Badah. 2021. "New Approaches to the General Linearization Problem of Jacobi Polynomials Based on Moments and Connection Formulas" Mathematics 9, no. 13: 1573. https://doi.org/10.3390/math9131573
APA StyleAbd-Elhameed, W. M., & Badah, B. M. (2021). New Approaches to the General Linearization Problem of Jacobi Polynomials Based on Moments and Connection Formulas. Mathematics, 9(13), 1573. https://doi.org/10.3390/math9131573






