Numerical Simulation of Gas–Liquid Two-Phase Flow Characteristics of Centrifugal Pump Based on the CFD–PBM
Abstract
1. Introduction
2. Mathematic Models
2.1. Governing Equations
2.2. Solution Methods for the PBM
3. Numerical Simulation Procedures
3.1. Pump Model
3.2. Mesh Generation
3.3. Gas–Liquid Two-Phase Flow Calculation Settings
4. Calculation Results and Analysis
4.1. Validation of the CFD–PBM
4.1.1. Comparison of Hydraulic Performance Using Different Multiphase Models
4.1.2. Comparison of Pressure under Different Inlet Gas Volume Fractions (IGVFs)
4.1.3. Comparison of Velocity under Different IGVFs
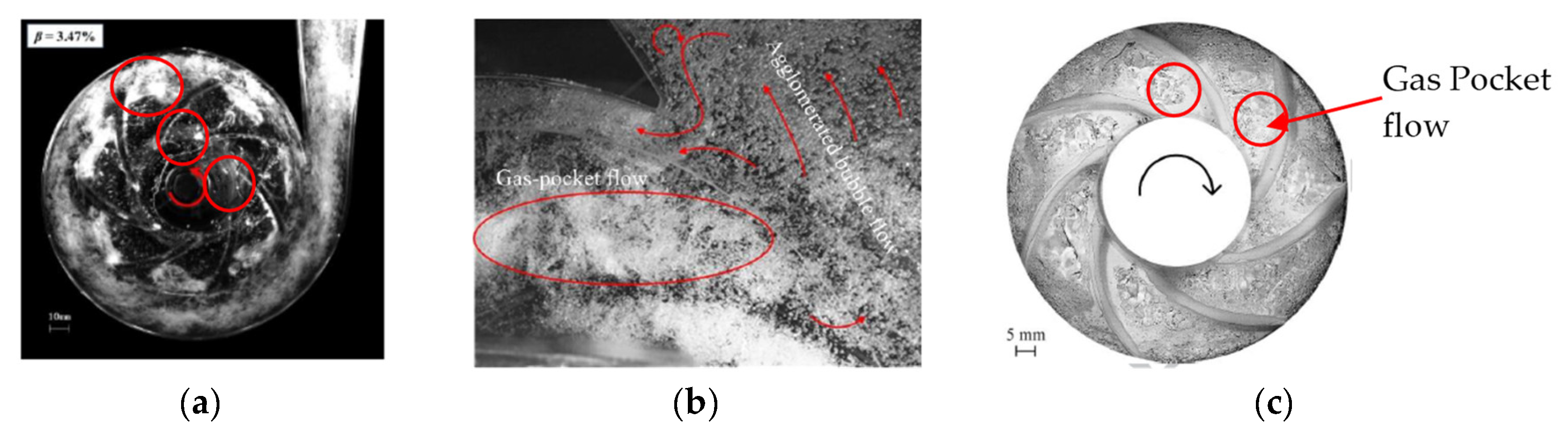
4.2. Transient Inner Flow Characteristics under Low IGVF
4.2.1. Transient Velocity Distribution at Different Timesteps
4.2.2. Transient Mean Bubble Diameter Distribution at Different Timesteps
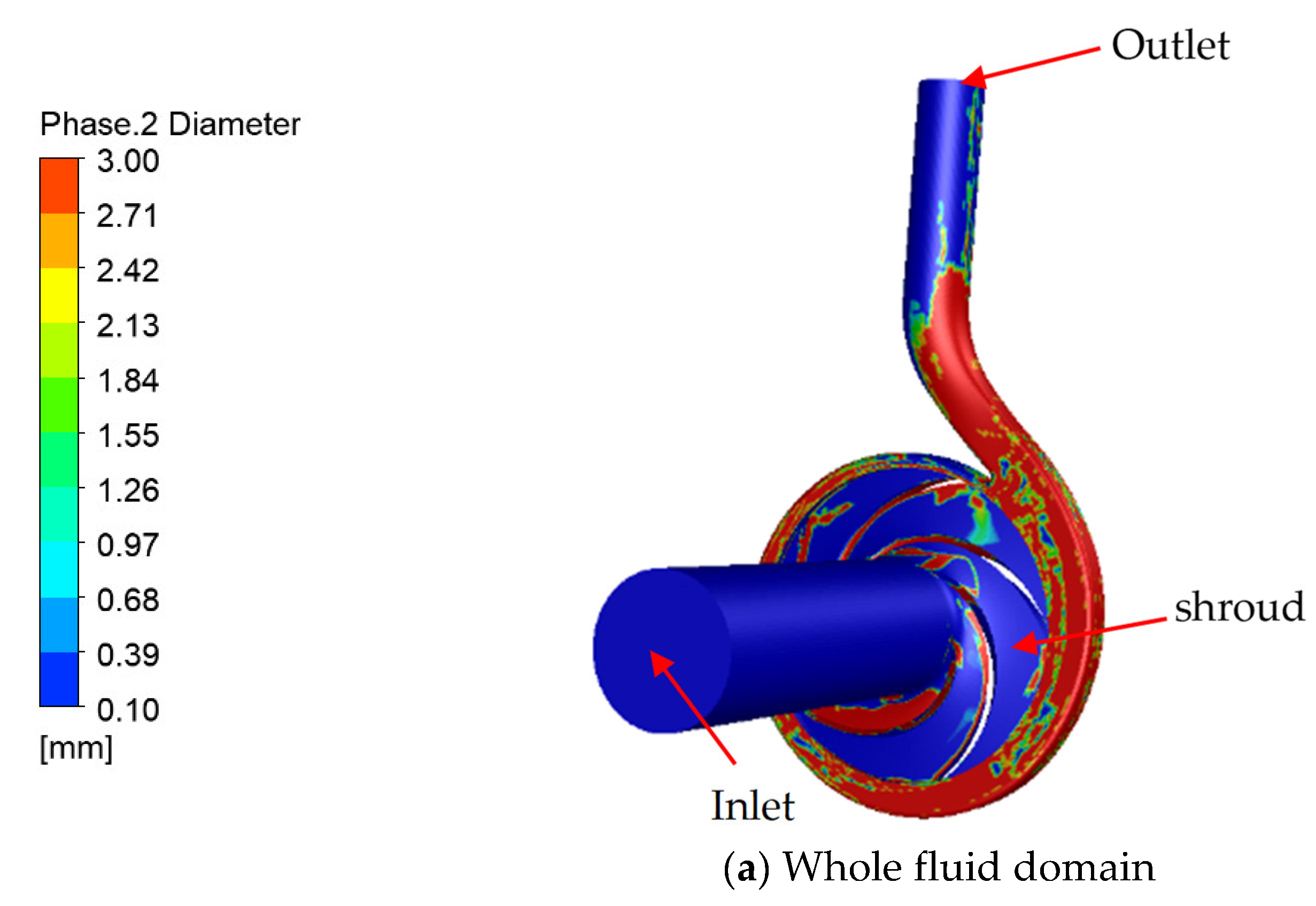
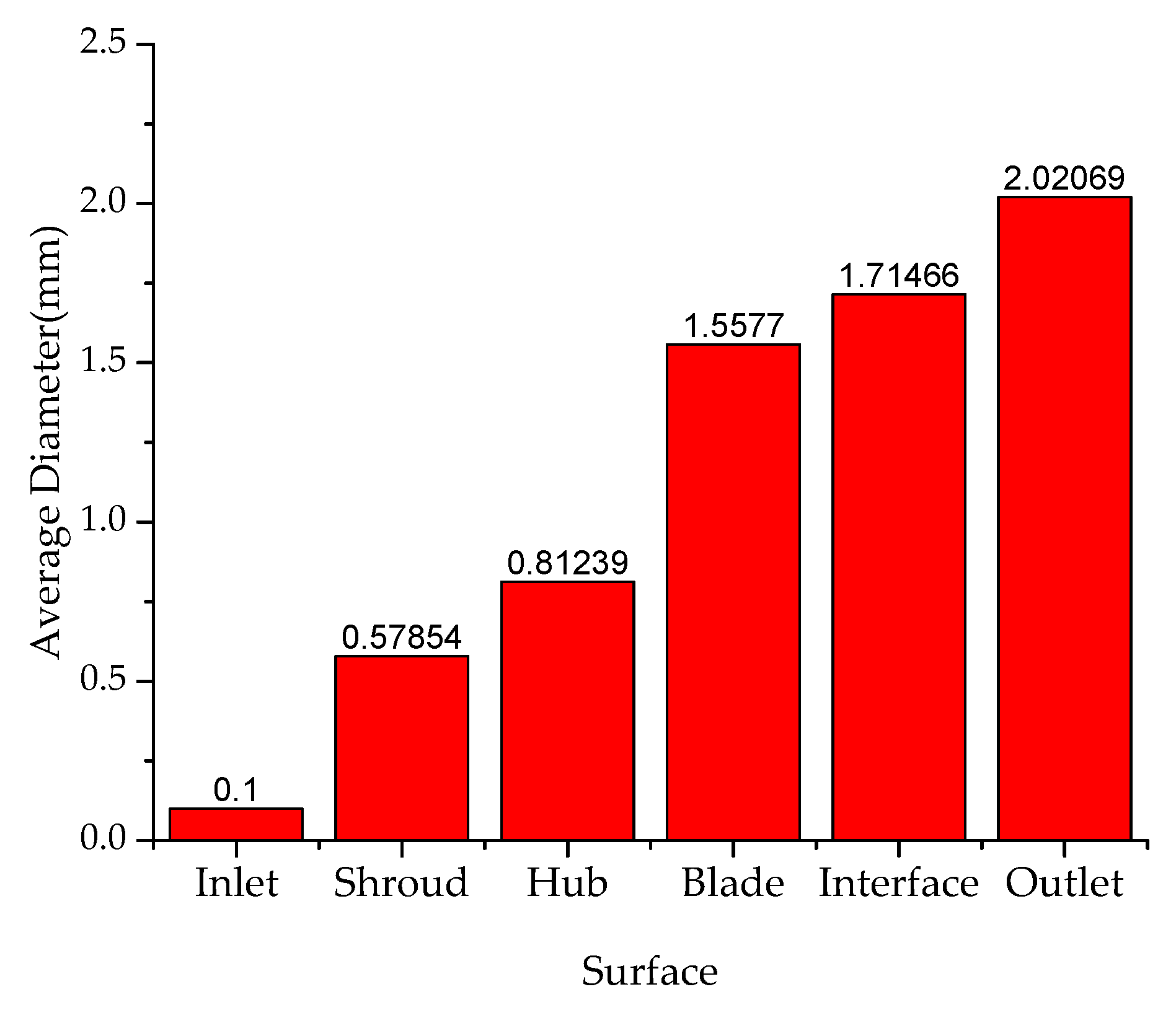
4.3. The Mean Bubble Diameter Distribution in the Whole Computing Domain
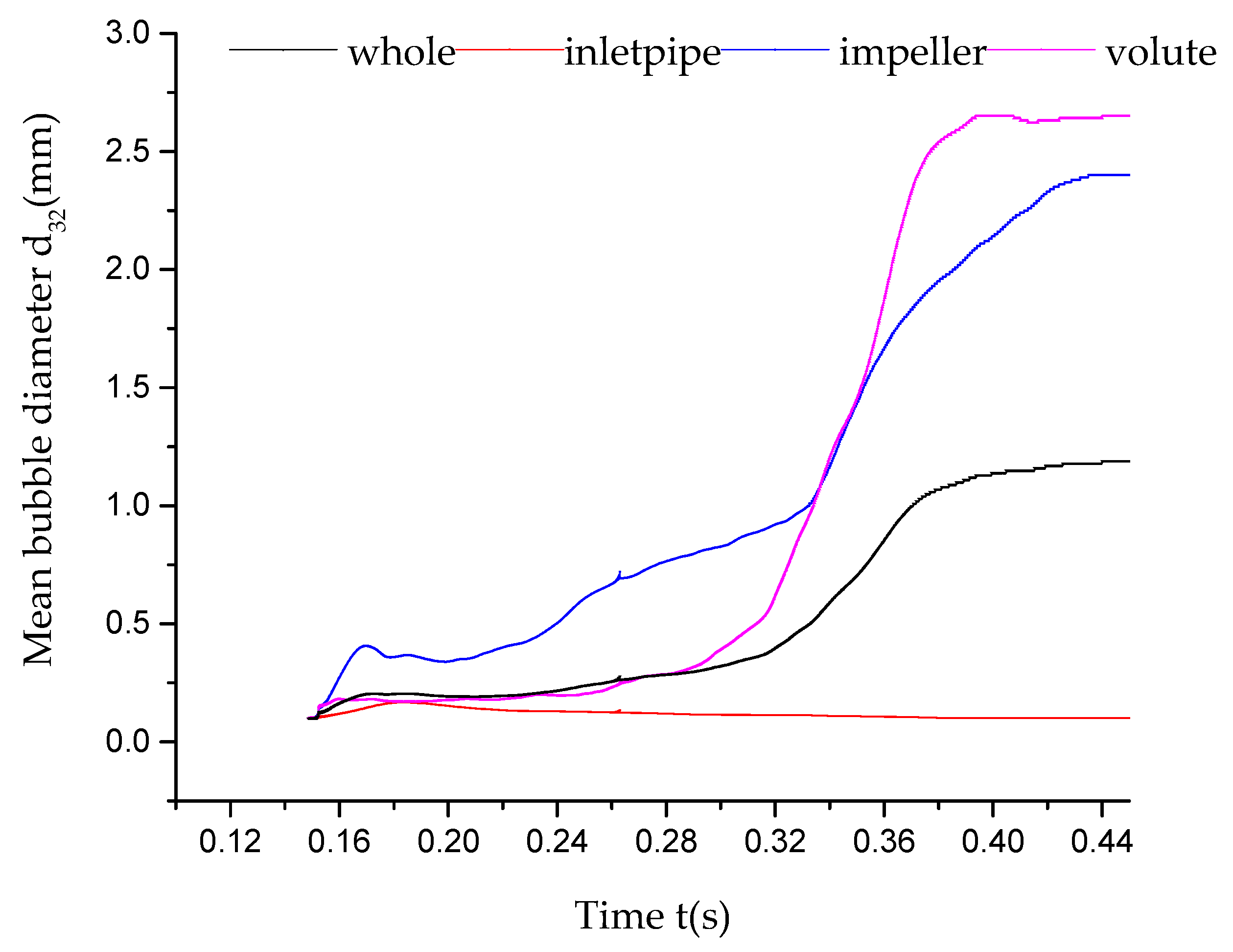
4.4. Variation of the Mean Bubble Diameter with Time
5. Conclusions
- (1)
- The entire study showed the capability of the CFD–PBM in the numerical simulation of gas–liquid two-phase flow in a vane pump using the law of bubble coalescence and breakage.
- (2)
- Where the centrifugal pump flow was unstable, bubble concentration was more likely to be produced, forming large-diameter bubbles concentration area to the working face of the impeller. The proportion of large-diameter bubbles in volute was larger than the impeller, and most of them were concentrated in the cavity at the junction of volute and impeller which was related to the rotor–stator interaction between impeller and volute.
- (3)
- The CFD–PBM could capture the variation of bubble diameter each time. The general rule was that the bubble coalescence rate was much faster than the bubble breakage rate which caused the bubble diameter grow rapidly and enter a steady rising stage. When it finally entered to the equilibrium state, the coalescence rate equaled the breakage rate and the mean diameter of bubbles became stable, which did not fluctuate with time.
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| g | Acceleration due to gravity, m/s2 |
| ρ | Density, kg/m3 |
| H | Head, m |
| M | Torque, N·m |
| w | Angular speed, rad/s |
| k | Kinetic energy of turbulence, m2/s2 |
| ϵ | Dissipation of kinetic energy of turbulence, m2/s2 |
| ω | Specific dissipation of turbulence kinetic energy, s−1 |
| t | Time, s |
| x, y, z | Coordinates in stationary frame |
| xi | Cartesian coordinates: x, y, z |
| i, j | Components in different directions |
| μ | Dynamic viscosity, Pa·s |
| β’, γ | Turbulence—model coefficients |
| μT | Turbulent viscosity, m2/s |
Abbreviations
| IGVF | Inlet gas volume fraction |
| PBM | Population Balance Model |
| 3-D | Three dimensional |
| CFD | Computational fluid dynamics |
| SST | Shear stress transport |
References
- Johann, F.G. Centrifugal Pumps; Springer: Berlin/Heidelberg, Germany; New York, NY, USA, 2008. [Google Scholar]
- Pei, J.; Osman, M.; Wang, W.J. Unsteady flow characteristics and cavitation prediction in the double-suction centrifugal pump using a novel approach. Proc. Inst. Mech. Eng. Part A J. Power Energy 2019, 234, 283–299. [Google Scholar] [CrossRef]
- Tang, S.N.; Yuan, S.Q.; Zhu, Y. Deep learning-based intelligent fault diagnosis methods towards rotating machinery. IEEE Access 2020, 8, 9335–9346. [Google Scholar] [CrossRef]
- Li, X.J.; Chen, B.; Luo, X.W.; Zhu, Z.C. Effects of flow pattern on hydraulic performance and energy conversion characterisation in a centrifugal pump. Renew. Energy 2020, 151, 475–487. [Google Scholar] [CrossRef]
- Minemura, K.; Murakami, M. Effects of entrained air on the performance of a centrifugal pump (first report, performance and flow conditions). Trans. Jpn. Soc. Mech. Eng. 1974, 17, 1047–1055. [Google Scholar]
- Minemura, K.; Murakami, M. Effects of entrained air on the performance of a centrifugal pump (second report, effects of number of blades). Trans. Jpn. Soc. Mech. Eng. 1974, 17, 1286–1295. [Google Scholar]
- Patel, B.R.; Runstadler, J.P. Investigations into the two-phase flow behavior of centrifugal pumps. Proc Polyphase Flow in Turbomachinery; ASME: New York, NY, USA, 1978; pp. 79–100. [Google Scholar]
- Verde, W.; Biazussi, J.L.; Sassim, N.A. Experimental study of gas-liquid two-phase flow patterns within centrifugal pumps impellers. Exp. Therm. Fluid Sci. 2017, 85, 37–51. [Google Scholar] [CrossRef]
- Barrios, L.; Prado, M.G. Modeling two phase flow inside an electrical submersible pump stage. In Proceedings of the ASME 2009 28th International Conference on Ocean, Offshore and Arctic Engineering, Honolulu, HI, USA, 31 May–5 June 2009; pp. 227–231. [Google Scholar]
- Burrascano, P.; Callegari, S.; Montisci, A.; Ricci, M.; Versaci, M. Ultrasonic Nondestructive Evaluation Systems; Springer International Publishing: Cham, Switzerland, 2015. [Google Scholar] [CrossRef]
- Caridad, J.; Asuaje, M.; Kenyery, F. Characterization of a centrifugal pump impeller under two-phase flow conditions. J. Pet. Sci. Eng. 2008, 63, 18–22. [Google Scholar] [CrossRef]
- Marin, M.; Rahmat, E. On solutions of Saint-Venant’s problem for elastic dipolar bodies with voids. Carpathian J. Math. 2017, 33, 219–232. [Google Scholar]
- Kim, J.H.; Lee, H.C. Hydrodynamic of experiment performance of a multiphase Improvement of pump using design techniques. J. Fluids Eng. 2015, 137, 081301. [Google Scholar] [CrossRef]
- Wu, Y.L. Three-dimension calculation of oil-bubble flows through a centrifugal Pump impeller. In Proceedings of the Third Internal Conference on Pump and Fan, Beijing, China, 13–16 October 1998; Tsinghua University Press: Beijing, China, 1998; pp. 526–532. [Google Scholar]
- Zhao, W.G.; He, X.X.; Wang, X.Y. Numerical Simulation of Cavitation Flow in a Centrifugal Pump. Appl. Mech. Mater. 2013, 444, 509–516. [Google Scholar] [CrossRef]
- Wang, T.; Wang, J. Numerical simulations of gas—Liquid mass transfer in bubble columns with a CFD–PBM coupled model. Chem. Eng. Sci. 2007, 62, 7107–7118. [Google Scholar] [CrossRef]
- Zhang, B.; Kong, L.T.; Jin, H.B. CFD simulation of gas—Liquid flow in a high-pressure bubble column with a modified population balance model. Chin. J. Chem. Eng. 2018, 26, 1350–1358. [Google Scholar] [CrossRef]
- Crowley, T.J.; Meadows, E.S.; Kostoulas, E. Control of particle size distribution described by a population balance model of semibatch emulsion polymerization. J. Process Control 2000, 10, 419–432. [Google Scholar] [CrossRef]
- Chen, X.Z.; Luo, Z.H.; Yan, W.C. Three-dimensional CFD-PBM coupled model of the temperature fields in fluidized-bed polymerization reactors. Aiche J. 2011, 57, 3351–3366. [Google Scholar] [CrossRef]
- Yan, W.C.; Luo, Z.H.; Guo, A.Y. Coupling of CFD with PBM for a pilot-plant tubular loop polymerization reactor. Chem. Eng. Sci. 2011, 66, 5148–5163. [Google Scholar] [CrossRef]
- Gu, Z.; Su, J.; Li, Y. Behaviors of the Dispersed Phase in the Multiphase System and Population Balance Model. Chem. React. Eng. Technol. 2007, 23, 162–167. [Google Scholar]
- Wu, C.F. Study on Distribution and Motion Feature of Salt Out Crystal Particles in Impeller of Centrifugal Pump. Ph.D. Thesis, Jiangsu University, Zhenjiang, China, 2016. (In Chinese with English Abstract). [Google Scholar]
- Wang, T.; Wang, J.; Jin, Y. Population balance model for gas-liquid flows: Influence of bubble coalescence and breakup models. Ind. Eng. Chem. Res. 2005, 44, 7540–7549. [Google Scholar] [CrossRef]
- Patruno, L.E.; Dorao, C.A.; Dupuy, P.M. Identification of droplet breakage kernel for population balance modelling. Chem. Eng. Sci. 2009, 64, 638–645. [Google Scholar] [CrossRef]
- Chen, Y.M.; Patil, A.; Bai, C.R.; Wang, Y.T. Numerical Study on the First Stage Head Degradation in an Electrical Submersible Pump with Population Balance Model. J. Energy Resour. Technol. 2019, 141, 022003. [Google Scholar] [CrossRef]
- Yan, S.; Sun, S.; Luo, X.; Chen, S.; Li, C.; Feng, J. Numerical Investigation on Bubble Distribution of a Multistage Centrifugal Pump Based on a Population Balance Model. Energies 2020, 13, 908. [Google Scholar] [CrossRef]
- Menter, F.R. Two-equation eddy-viscosity turbulence models for engineer applications. Aiaa J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- Zhang, F.; Appiah, D.; Hong, F. Energy loss evaluation in a side channel pump under different wrapping angles using entropy production method. Int. Commun. Heat Mass Transf. 2020, 113, 104526. [Google Scholar] [CrossRef]
- Wang, Y.F.; Zhang, F.; Yuan, S.Q. Effect of Unrans and Hybrid Rans-Les Turbulence Models on Unsteady Turbulent Flows Inside a Side Channel Pump. ASME J. Fluids Eng. 2020, 142, 061503. [Google Scholar] [CrossRef]
- Hasse, C.; Sohm, V.; Durst, B. Detached eddy simulation of cyclic large scale fluctuations in a simplified engine setup. Int. J. Heat Fluid Flow 2009, 30, 32–43. [Google Scholar] [CrossRef]
- Liu, Y.; Hinrichsen, O. Study on CFD–PBM turbulence closures based on k–ε and Reynolds stress models for heterogeneous bubble column flows. Comput. Fluids 2014, 105, 91–100. [Google Scholar] [CrossRef]
- ANSYS. ANSYS, Release 15.0 ANSYS Documentation; ANSYS, Inc.: Canonsburg, PA, USA, 2013. [Google Scholar]
- Randolph, A.D. , Larson, M.A. Theory of Particulate Processes; Academic Press Inc.: London, UK, 1988. [Google Scholar]
- Marchisio, D.L.; Vigil, R.D.; Fox, R.O. Quadrature method of moments for aggregation-breakage processes. J. Colloid Interface Sci. 2003, 258, 322–334. [Google Scholar] [CrossRef]
- McGraw, R. Description of aerosol dynamics by the quadrature Method of Moments. Aerosol Sci. Technol. 1997, 27, 255–265. [Google Scholar] [CrossRef]
- Si, Q.R.; Zhang, H.Y.; Gerard, B. Experimental Investigations on the Inner Flow Behavior of Centrifugal Pumps under Inlet Air-Water Two-Phase Conditions. Energies 2019, 12, 4377. [Google Scholar] [CrossRef]
- Zhu, J.; Zhang, H. CFD Simulation of ESP Performance and Bubble Size Estimation under Gassy Conditions. In Proceedings of the SPE Annual Technical Conference and Exhibition, Amsterdam, The Netherlands, 27–29 October 2014. [Google Scholar]
- Kosmowski, I. Behavior of centrifugal pump when conveying gas entrained liquids. In Proceedings of the 7th Technical Conference of BPMA, Albany, NY, USA, 15–17 September 1982; pp. 283–291. [Google Scholar]
- Zhang, Z.D. Experimental study on gas-liquid flow characteristics and pump performance of centrifugal pump impeller under bubble inflow. Master’s Thesis, Xi’an University of Technology, Xi’an, China, 2019. [Google Scholar]
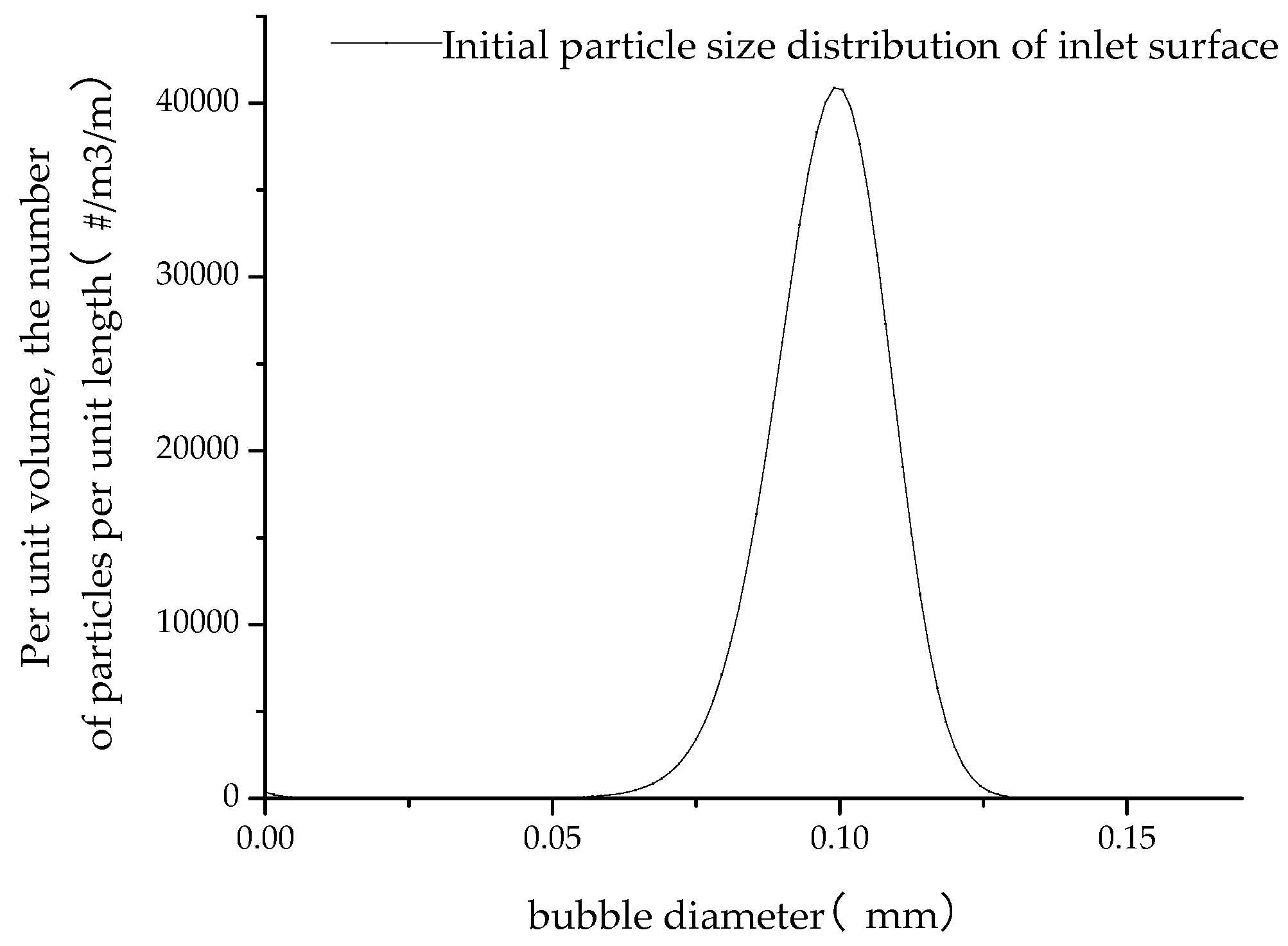
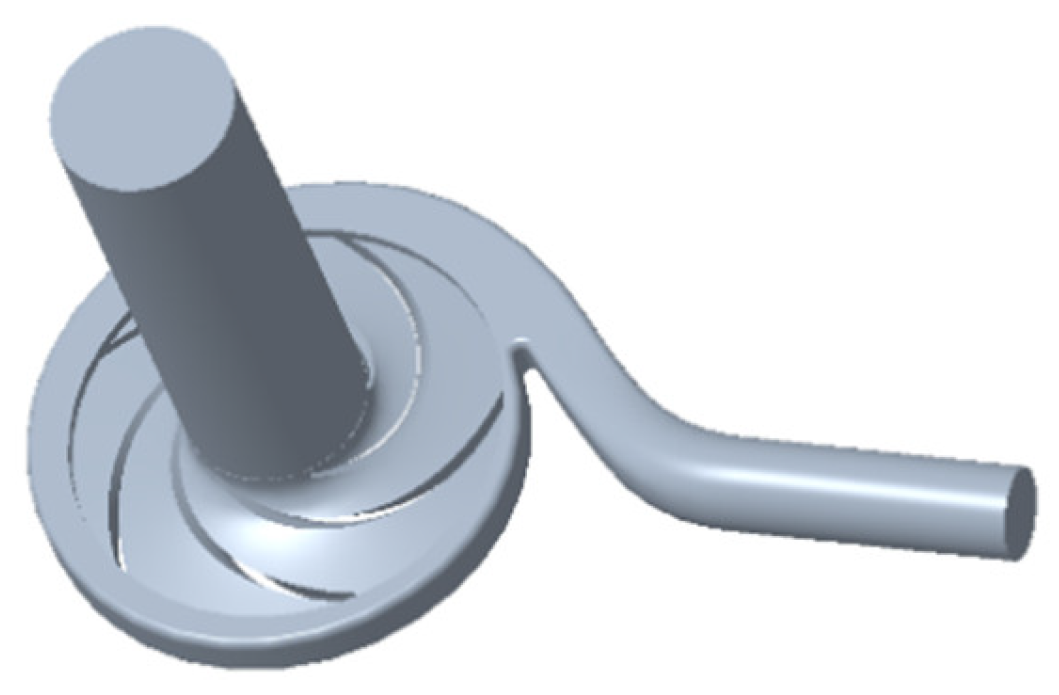



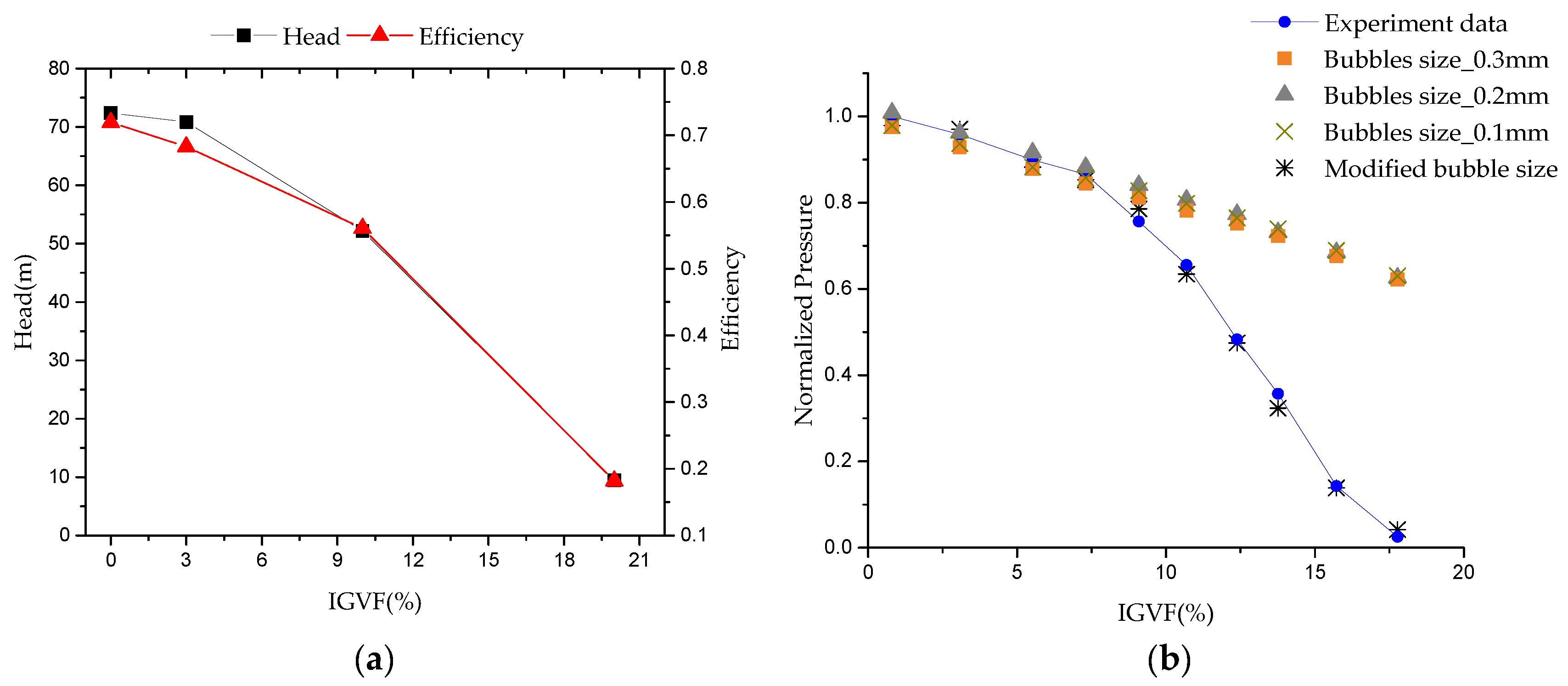





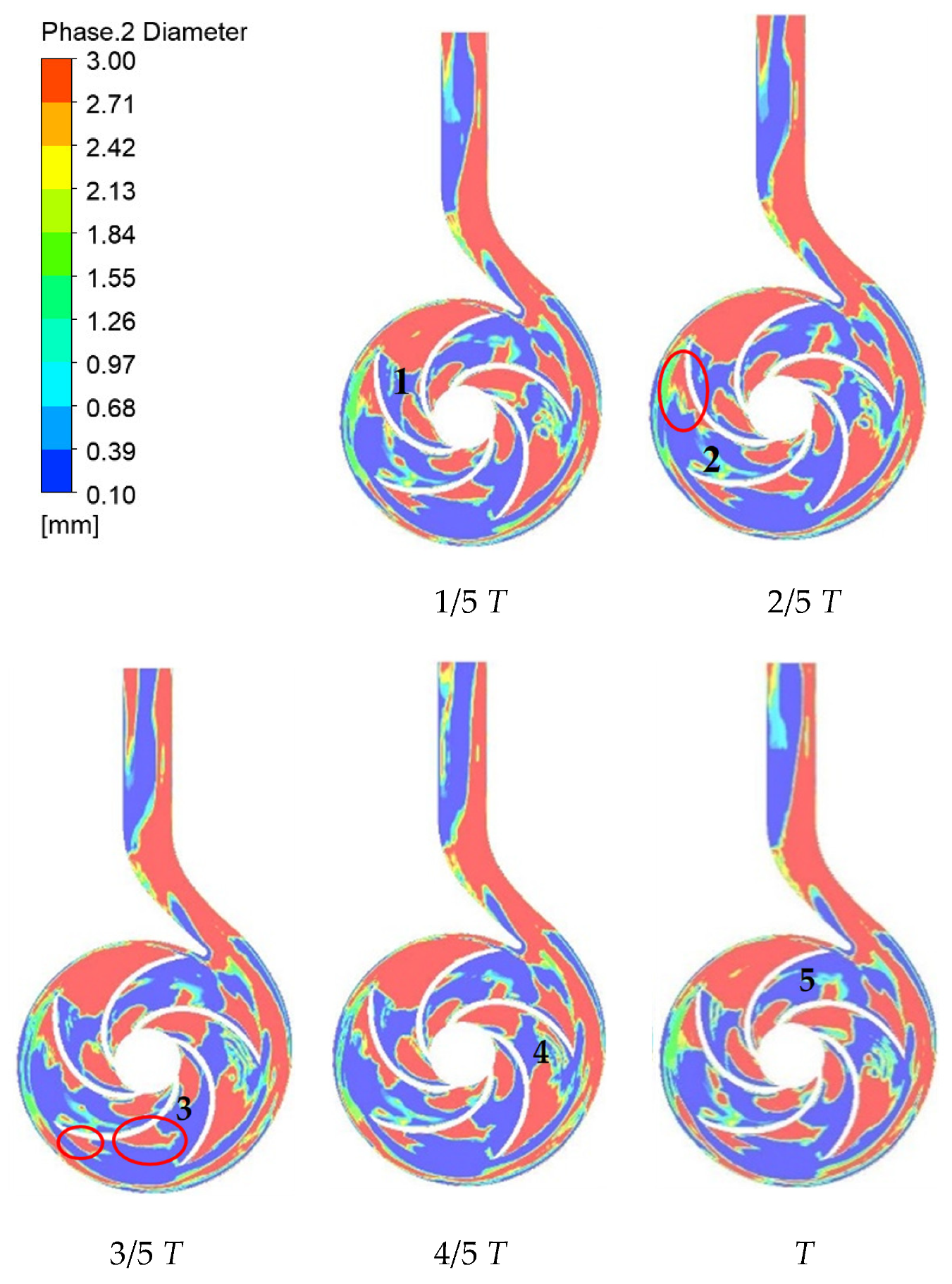


| Boundary Conditions | ||
|---|---|---|
| Location | Boundary type | Mass and momentum |
| Inlet of inlet pipe | Velocity–inlet | Velocity |
| Outlet of volute | Pressure–outlet | Static pressure |
| Physical surfaces | Wall | No-slip |
| Rotor–stator interfaces | ||
| Transient state | Transient Rotor–stator | |
| Turbulence model | ||
| SST k-ω | ||
| Solver control for transient simulation | ||
| Timestep | 1.7182 × 10−4 s (14 cycles) | |
| Total time | 0.288 s | |
| RMS residual | 10−5 | |
| Grid Number/106 | Head (m) | Efficiency | Outlet Velocity (m/s) |
|---|---|---|---|
| 2.66 | 71.7645 | 0.715834 | 8.662 |
| 3.35 | 72.3672 | 0.719333 | 8.608 |
| 4.00 | 72.2497 | 0.718956 | 8.632 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, F.; Zhu, L.; Chen, K.; Yan, W.; Appiah, D.; Hu, B. Numerical Simulation of Gas–Liquid Two-Phase Flow Characteristics of Centrifugal Pump Based on the CFD–PBM. Mathematics 2020, 8, 769. https://doi.org/10.3390/math8050769
Zhang F, Zhu L, Chen K, Yan W, Appiah D, Hu B. Numerical Simulation of Gas–Liquid Two-Phase Flow Characteristics of Centrifugal Pump Based on the CFD–PBM. Mathematics. 2020; 8(5):769. https://doi.org/10.3390/math8050769
Chicago/Turabian StyleZhang, Fan, Lufeng Zhu, Ke Chen, Weicheng Yan, Desmond Appiah, and Bo Hu. 2020. "Numerical Simulation of Gas–Liquid Two-Phase Flow Characteristics of Centrifugal Pump Based on the CFD–PBM" Mathematics 8, no. 5: 769. https://doi.org/10.3390/math8050769
APA StyleZhang, F., Zhu, L., Chen, K., Yan, W., Appiah, D., & Hu, B. (2020). Numerical Simulation of Gas–Liquid Two-Phase Flow Characteristics of Centrifugal Pump Based on the CFD–PBM. Mathematics, 8(5), 769. https://doi.org/10.3390/math8050769







