Novel Face Index for Benzenoid Hydrocarbons
Abstract
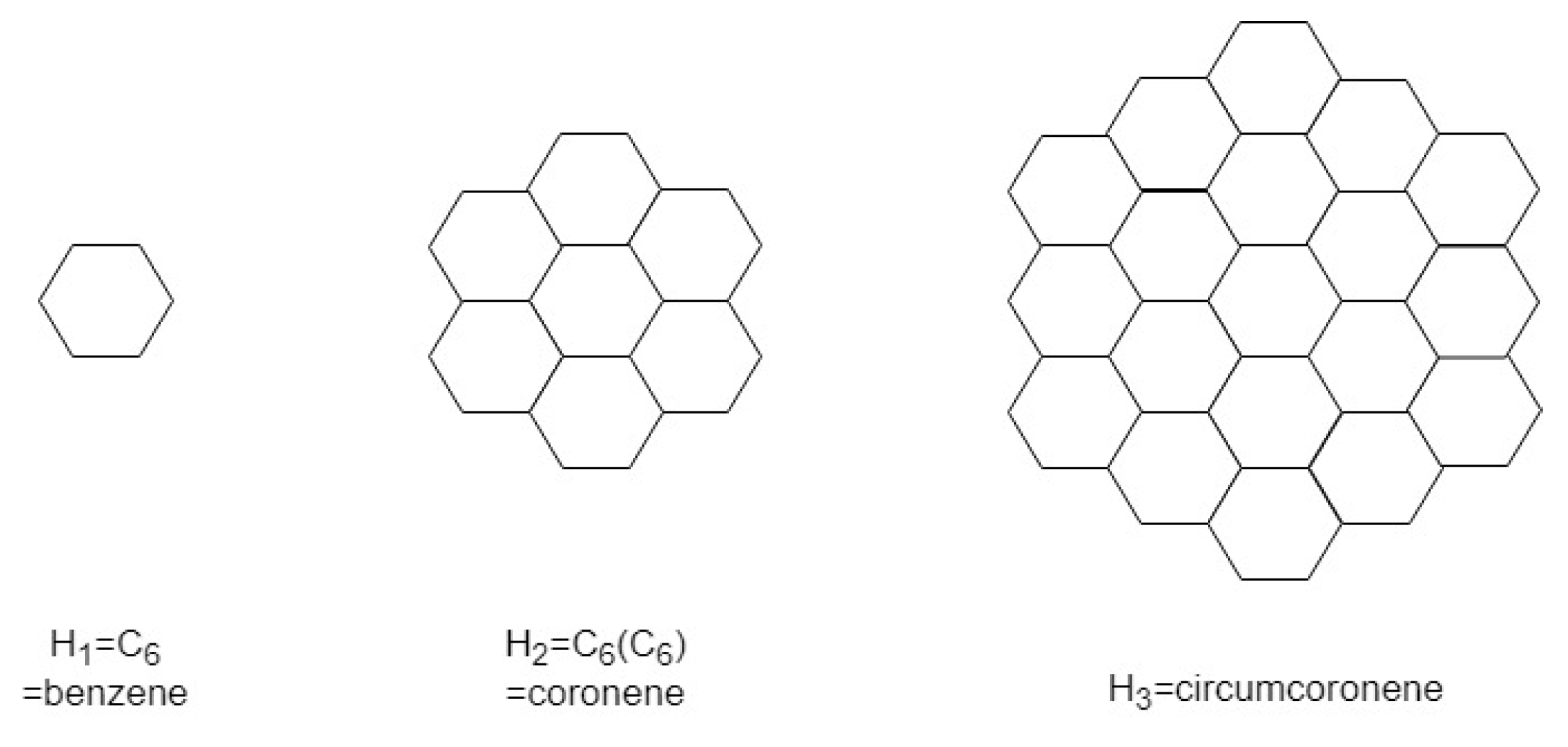
1. Introduction
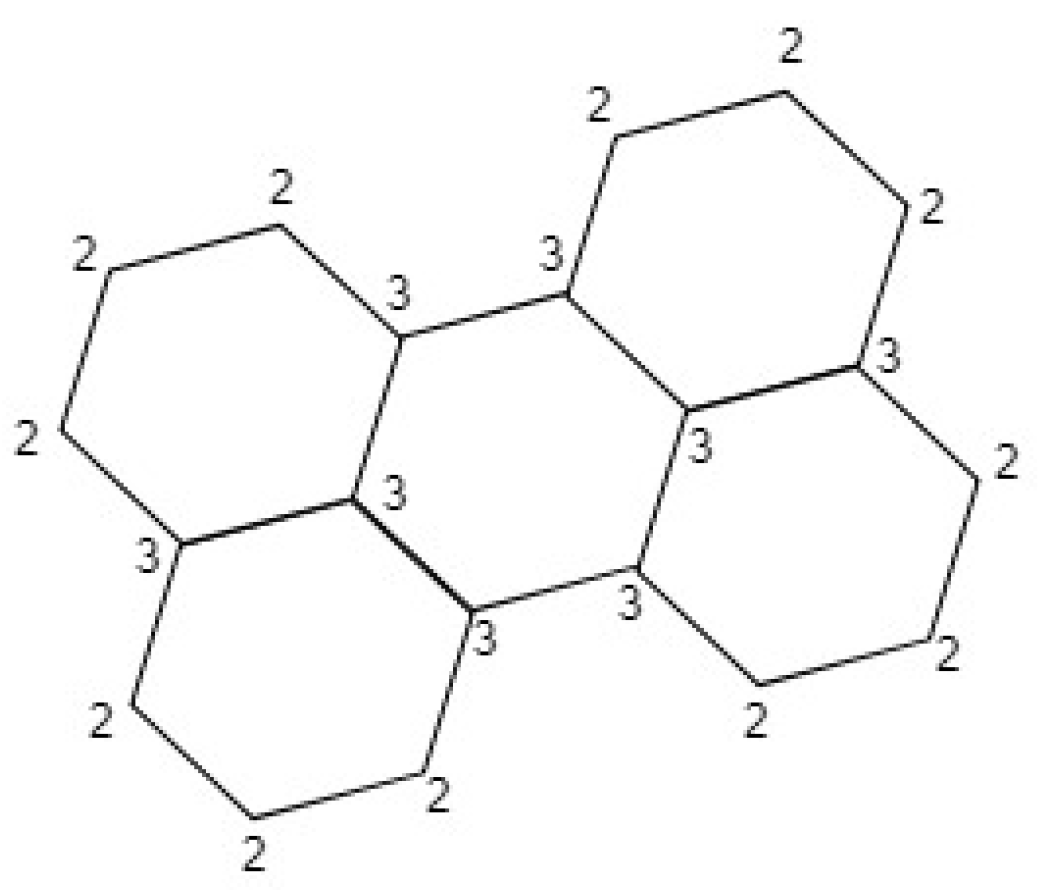
Definition of the Face Index
2. Discussion
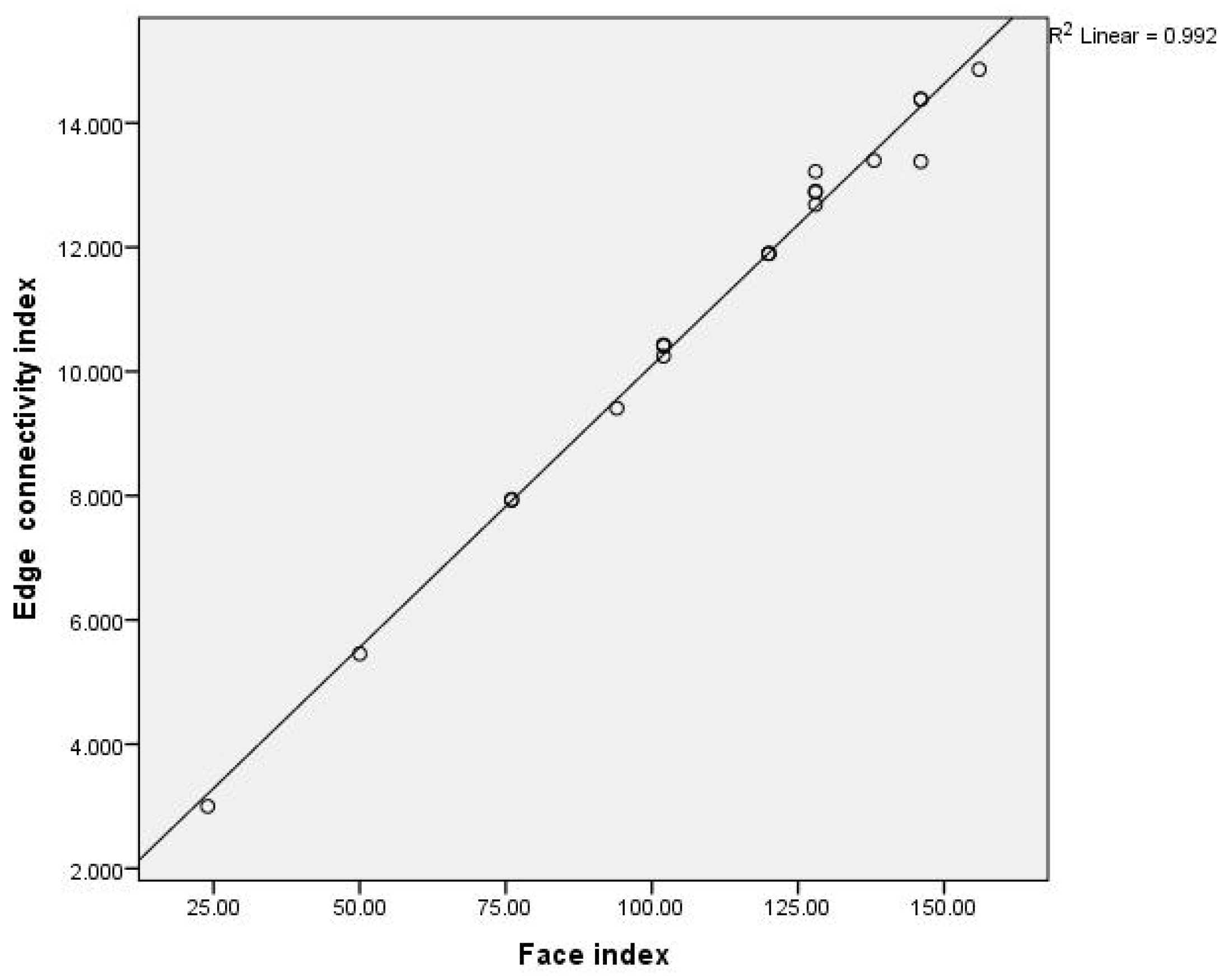
2.1. Relationships between the Face Index and Vertex and Edge Connectivity Indices for Benzenoid Hydrocarbons
2.2. Linear Mathematical Models of the Face Index () for the -Electron Energy (E)
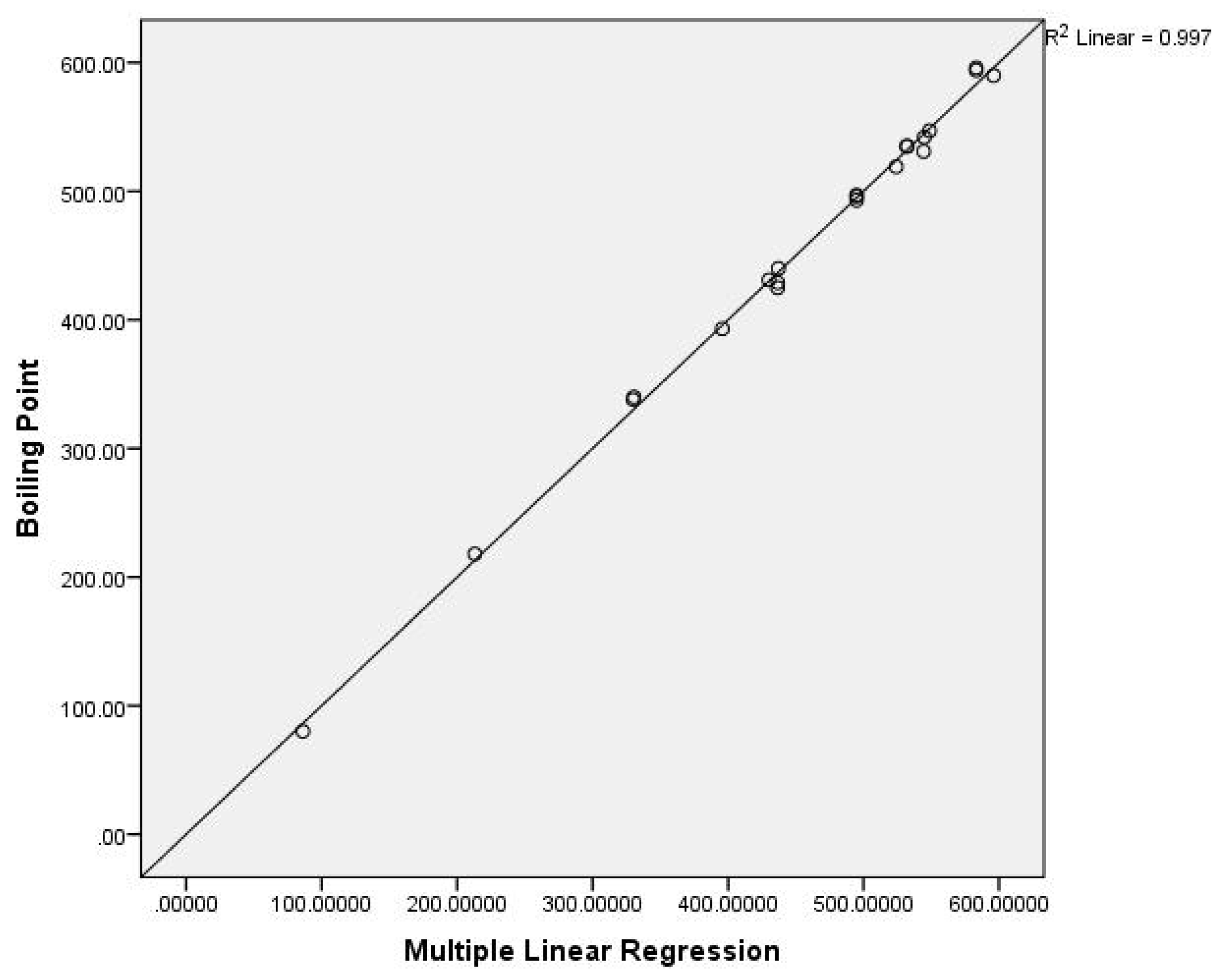
2.3. Linear Mathematical Models of the Face Index () for the Boiling Points ().
3. Computational Techniques for the Face Index
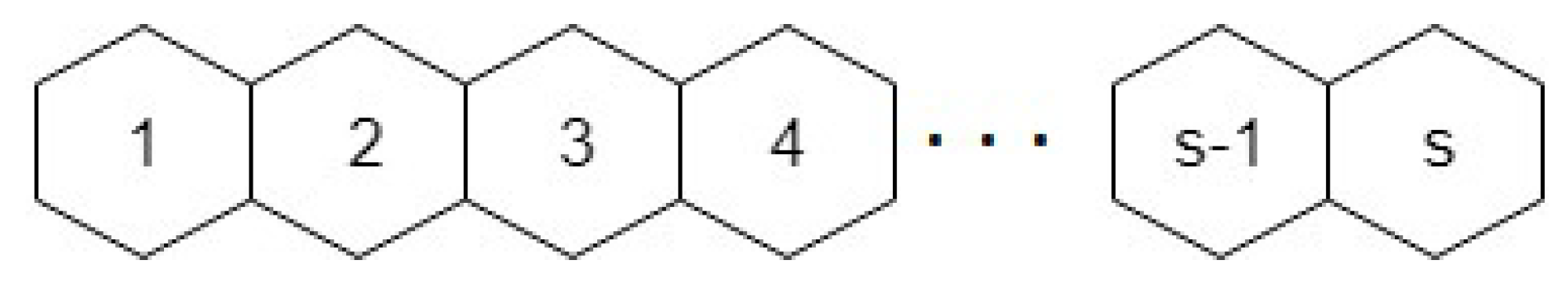
3.1. Face Index of Graphene
3.2. Face index of Triangular Benzenoid
3.3. Face Index of Circumcoronene Series of Benzeniod
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Balaban, A.T. Topological indices and their uses: A new approach for coding of alkanes. J. Mol. Struct. Theochem 1988, 165, 243–253. [Google Scholar] [CrossRef]
- Estrada, E.; Ivanciuc, O.; Gutman, I.; Gutierrez, A.; Rodrìguez, L. Extended Wiener indices. A new set of descriptors for quantitative structure-property studies. New J. Chem. 1998, 22, 819–823. [Google Scholar] [CrossRef]
- Taherpour, A.A.; Shafiei, F. The structural relationship between Randić indices, adjacency matrixes, distance matrixes and maximum wave length of linear simple conjugated polyene compounds. J. Mol. Struct. Theochem 2005, 726, 183–188. [Google Scholar] [CrossRef]
- Yao, X.J.; Fan, B.; Doucet, J.P.; Panaye, A.; Liu, M.; Zhang, R.; Zhang, X.; Hu, Z. Quantitative structure property relationship models for the prediction of liquid heat capacity. QSAR Comb. Sci. 2003, 22, 29–48. [Google Scholar] [CrossRef]
- Jäntschi, L. A test detecting the outliers for continuous distributions based on the cumulative distribution function of the data being tested. Symmetry 2019, 11, 835. [Google Scholar] [CrossRef]
- Cash, G.G. Correlation of physicochemical properties of alkylphenols with their graph-theoretical parameter. Chemosphere 1995, 31, 4307–4315. [Google Scholar] [CrossRef]
- Gupta, S.P.; Singh, P. The Relationship of π-binding energy with molecular connectivity in hydrocarbons. Bull. Chem. Soc. Jpn. 1979, 52, 2745–2746. [Google Scholar] [CrossRef]
- Gutman, I.; Trinajstić, N. Graph theory and molecular orbitals. Total π-electron energy of alternant hydrocarbons. Chem. Phys. Lett. 1972, 17, 535–538. [Google Scholar] [CrossRef]
- Hosseine, H.; Shafiei, F. Entropy prodection of benzene derivatives using topological indices. Stud. UBB Chem. 2017, LXII, 297–310. [Google Scholar] [CrossRef]
- Randić, M. Quantitative Structure-Property Relationship. Boiling Points of Planar Benzenoids. New J. Chem. 1996, 20, 1001–1009. [Google Scholar]
- Estrada, E. Edge Adjacency Relationships and a Novel Topological Index Related to Molecular Volume. J. Chem. Inf. Comput. Sci. 1995, 35, 31–33. [Google Scholar] [CrossRef]
- Randić, M. On Characterization of Molecular Branching. J. Am. Chem. Soc. 1975, 97, 6609–6615. [Google Scholar] [CrossRef]
- Nikolic, S.; Trinajstić, N. Comparison between the Vertex- and Edge-Connectivity Indices for Benzenoid Hydrocarbons. J. Chem. Inf. Comput. Sci. 1998, 38, 42–46. [Google Scholar] [CrossRef]
- Minami, T.; Ito, S.; Nakano, M. Theoretical study of singlet fission in oligorylenes. J. Phys. Chem. Lett. 2012, 3, 2719–2723. [Google Scholar] [CrossRef]
- Plavšić, D.; Trinajstić, N.; Amić, D.; Šoxsxkić, M. Comparison between the structure-boiling point relationships different descriptors for condensed benzenoids. New J. Chem. 1998, 22, 1075–1078. [Google Scholar] [CrossRef]
- Coulson, C.A.; Streitwieser, J. Dictionary of π-Electron Calculations; Freeman: San Francisco, CA, USA, 1965. [Google Scholar]
- Basak, S.C.; Grunwald, G.D.; Niemi, G.J. Use of Graph-Theoretic and Geometrical Molecular Descriptors in Structure-Activity Relationships. In From Chemical Topology to Three-Dimensional Geometry; Balaban, A.T., Ed.; Plenum: New York, NY, USA, 1997; pp. 73–116. [Google Scholar]
- Farahani, M.R.; Gao, W. Degree-based indices computation for special chemical molecular structures using edge dividing method. Appl. Math. Nonlinear Sci. 2016, 1, 94–117. [Google Scholar]
- Gao, W.; Farahani, M.R. Computing the reverse eccentric connectivity index for certain family of nanocone and fullerene structures. J. Nano. Tech. 2016. [Google Scholar] [CrossRef]
- Gao, W.; Siddiqui, M.K.; Imran, M.; Jamil, M.K.; Farahani, M.R. Forgotten topological index of chemical structure in drugs. Saudi Pharm. J. 2016. [Google Scholar] [CrossRef]
- Gao, W.; Shi, L. Szeged related indices of unilateral polyomino chain and unilateral hexagonal chain. IAENG Int. J. Appl. Math. 2015, 45, 138–150. [Google Scholar]
- Gao, W.; Wang, W.F.; Farahani, M.R. Topological indices study of molecular structure in anticancer drugs. J. Chem. 2016. [Google Scholar] [CrossRef]
- Wang, W.F.; Gao, W. Second atom-bond connectivity index of special chemical molecular structures. J. Chem. 2014. [Google Scholar] [CrossRef]
- Wang, W.F.; Gao, W. The vertex version of weighted wiener number for bicyclic molecular structures. Comput. Math. Meth. Med. 2015. [Google Scholar] [CrossRef]
- Yan, L.; Gao, W.; Li, J.S. General harmonic index and general sum connectivity index of polyomino chains and nanotubes. J. Comput. Theor. Nanosci. 2015, 12, 3940–3944. [Google Scholar] [CrossRef]
- Zhang, X.; Xin, J.; Ding, F. The edges of graphene. Nanoscale 2013, 5, 2556–2569. [Google Scholar] [CrossRef] [PubMed]
- Mishra, S.; Beyer, D.; Eimre, K.; Liu, J.; Berger, R.; Gröning, O.; Pignedoli, C.A.; Müllen, K.; Fasel, R.; Feng, X.; et al. Synthesis and Characterization of π-Extended Triangulene. J. Am. Chem. Soc. 2019, 141, 10621–10625. [Google Scholar] [CrossRef] [PubMed]








| Benzenoid Hydrocarbons | E | ||||
|---|---|---|---|---|---|
| benzene | 24 | 3 | 3 | 8 | 80.1 |
| naphthalene | 50 | 4.966 | 5.455 | 13.683 | 218.0 |
| phenanthrene | 76 | 6.95 | 7.926 | 19.448 | 338.0 |
| anthracene | 76 | 6.933 | 7.942 | 19.314 | 340.0 |
| chrysene | 102 | 8.933 | 10.247 | 25.192 | 431.0 |
| benzanthracene | 102 | 8.916 | 10.414 | 25.101 | 425.0 |
| triphenylene | 102 | 8.95 | 10.414 | 25.275 | 429.0 |
| tetracene | 102 | 8.899 | 10.43 | 25.188 | 440.0 |
| benzo(a)pyrene | 120 | 9.916 | 11.897 | 28.22 | 496.0 |
| benzo(e)pyrene | 120 | 9.933 | 11.897 | 28.336 | 493.0 |
| perylene | 120 | 9.933 | 11.897 | 28.245 | 497.0 |
| anthanthrene | 138 | 10.899 | 13.397 | 31.253 | 547.0 |
| benzoperylene | 146 | 10.916 | 13.379 | 31.425 | 542.0 |
| dibenzo(a,c)anthracene | 128 | 10.916 | 12.902 | 30.942 | 535.0 |
| dibenzo(a,h)anthracene | 128 | 10.899 | 12.885 | 30.881 | 535.0 |
| dibenzo(a,i)anthracene | 128 | 10.899 | 13.218 | 30.88 | 531.0 |
| picene | 128 | 10.915 | 12.686 | 30.943 | 519.0 |
| coronene | 156 | 11.899 | 14.863 | 34.572 | 590.0 |
| dibenzo(a,h)pyrene | 146 | 11.566 | 14.385 | 33.928 | 596.0 |
| dibenzo(a,g)pyrene | 146 | 11.491 | 14.385 | 33.954 | 594.0 |
| pyrene | 94 | 7.933 | 9.408 | 22.506 | 393.0 |
| Rows | |||||
|---|---|---|---|---|---|
| 1 | 2 | - | s − 2 | - | - |
| 2 | - | 2 | 2 | 2(s − 2) | - |
| 3 | - | 2 | 3 | 2(s − 2) | (s − 1) |
| 4 | - | 2 | 4 | 2(s − 2) | 2(s − 1) |
| . | . | . | . | . | . |
| . | . | . | . | . | . |
| . | . | . | . | . | . |
| n | - | 2 | n | 2(s − 2) | (n − 2)(s − 1) |
| Rows | ||||
|---|---|---|---|---|
| 1 | 1 | - | - | - |
| 2 | - | 3 | - | - |
| 3 | - | 3 | 3 | - |
| 4 | - | 3 | 6 | 1 |
| 5 | - | 3 | 9 | 3 |
| 6 | - | 3 | 12 | 6 |
| . | . | . | . | . |
| . | . | . | . | . |
| . | . | . | . | . |
| n | - | 3 | 3(n − 2) |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jamil, M.K.; Imran, M.; Abdul Sattar, K. Novel Face Index for Benzenoid Hydrocarbons. Mathematics 2020, 8, 312. https://doi.org/10.3390/math8030312
Jamil MK, Imran M, Abdul Sattar K. Novel Face Index for Benzenoid Hydrocarbons. Mathematics. 2020; 8(3):312. https://doi.org/10.3390/math8030312
Chicago/Turabian StyleJamil, Muhammad Kamran, Muhammad Imran, and Kanza Abdul Sattar. 2020. "Novel Face Index for Benzenoid Hydrocarbons" Mathematics 8, no. 3: 312. https://doi.org/10.3390/math8030312
APA StyleJamil, M. K., Imran, M., & Abdul Sattar, K. (2020). Novel Face Index for Benzenoid Hydrocarbons. Mathematics, 8(3), 312. https://doi.org/10.3390/math8030312







