The Effect of a Hyperbolic Two-Temperature Model with and without Energy Dissipation in a Semiconductor Material
Abstract
1. Introduction
2. Basic Equations
3. Application
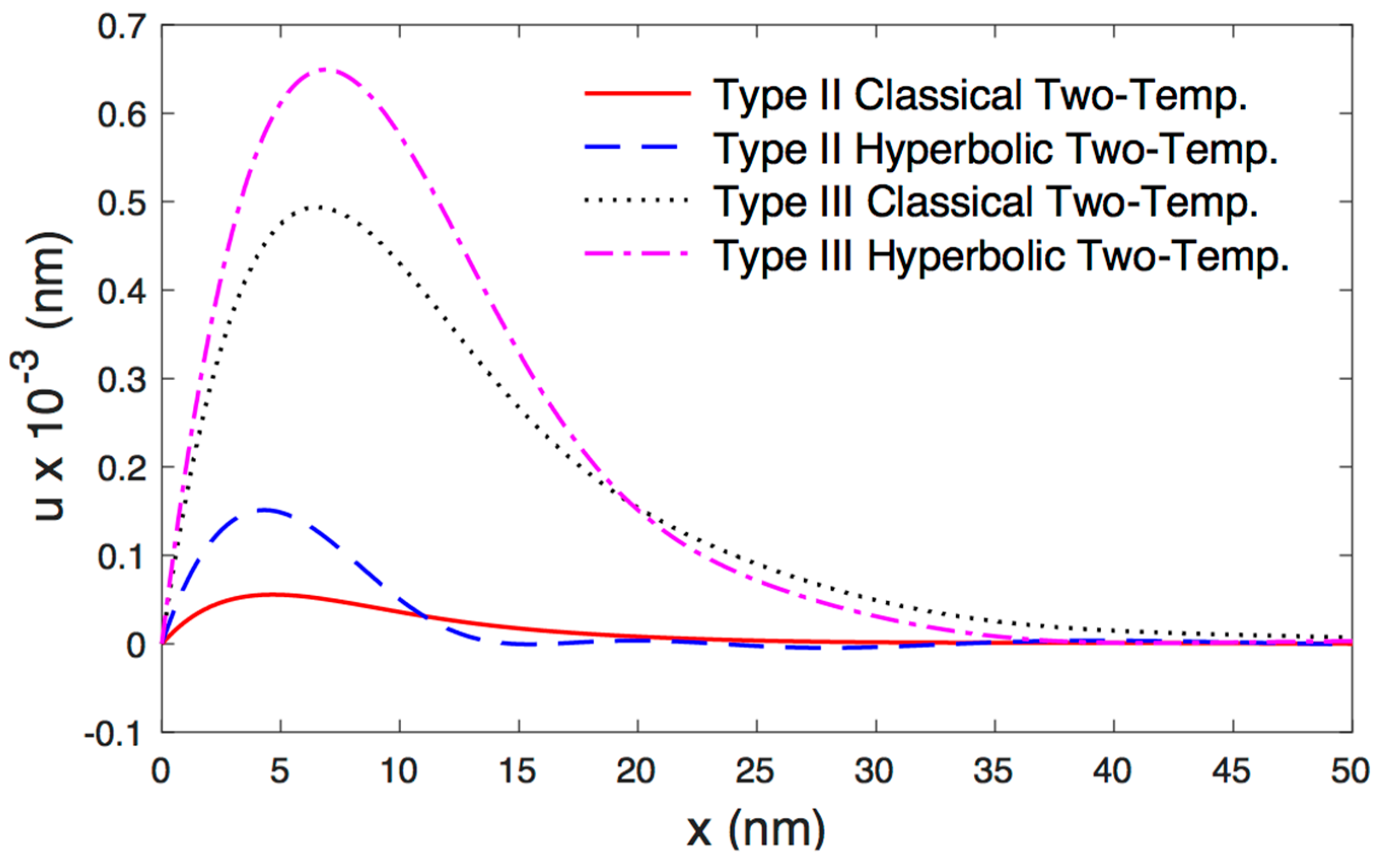
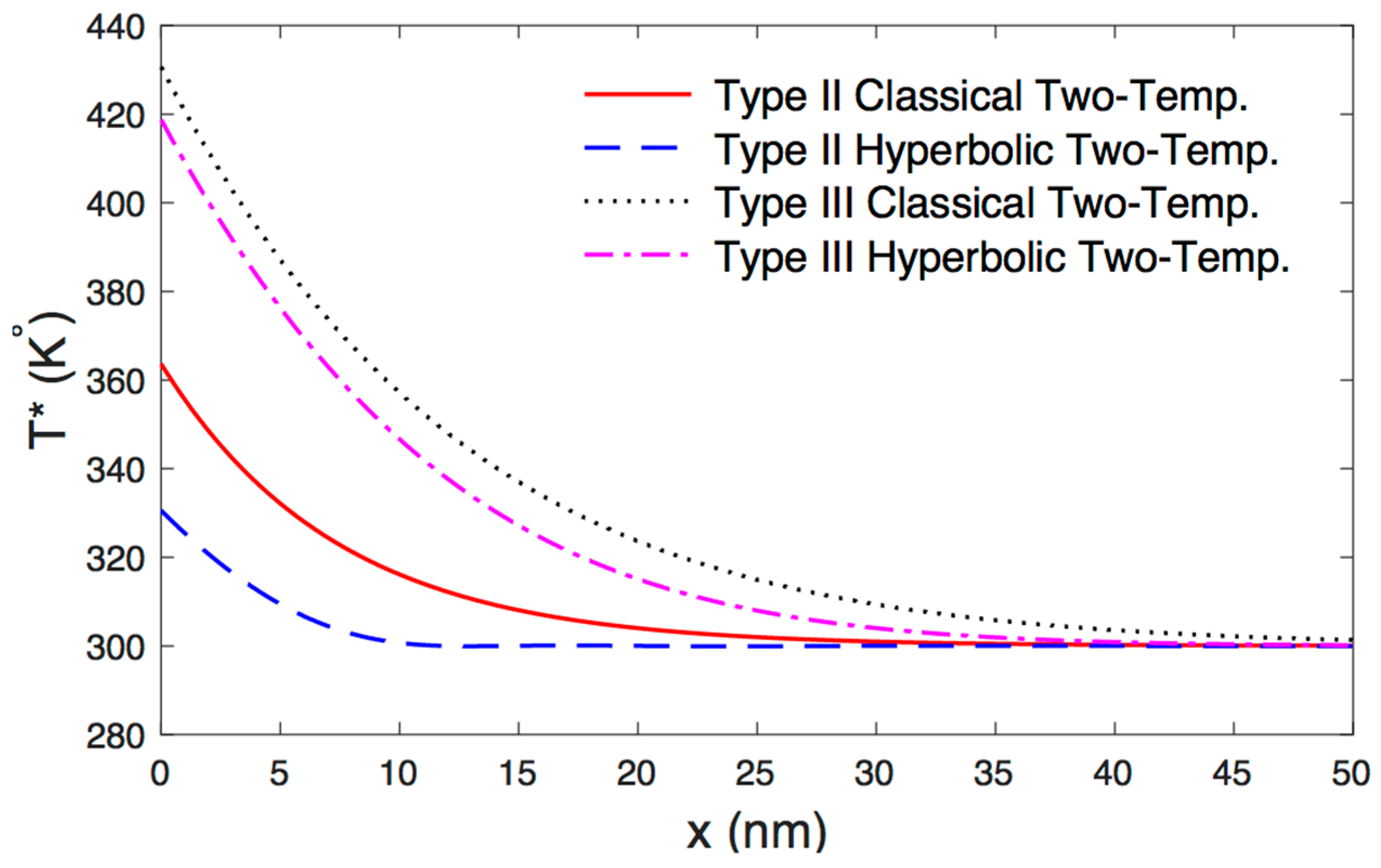
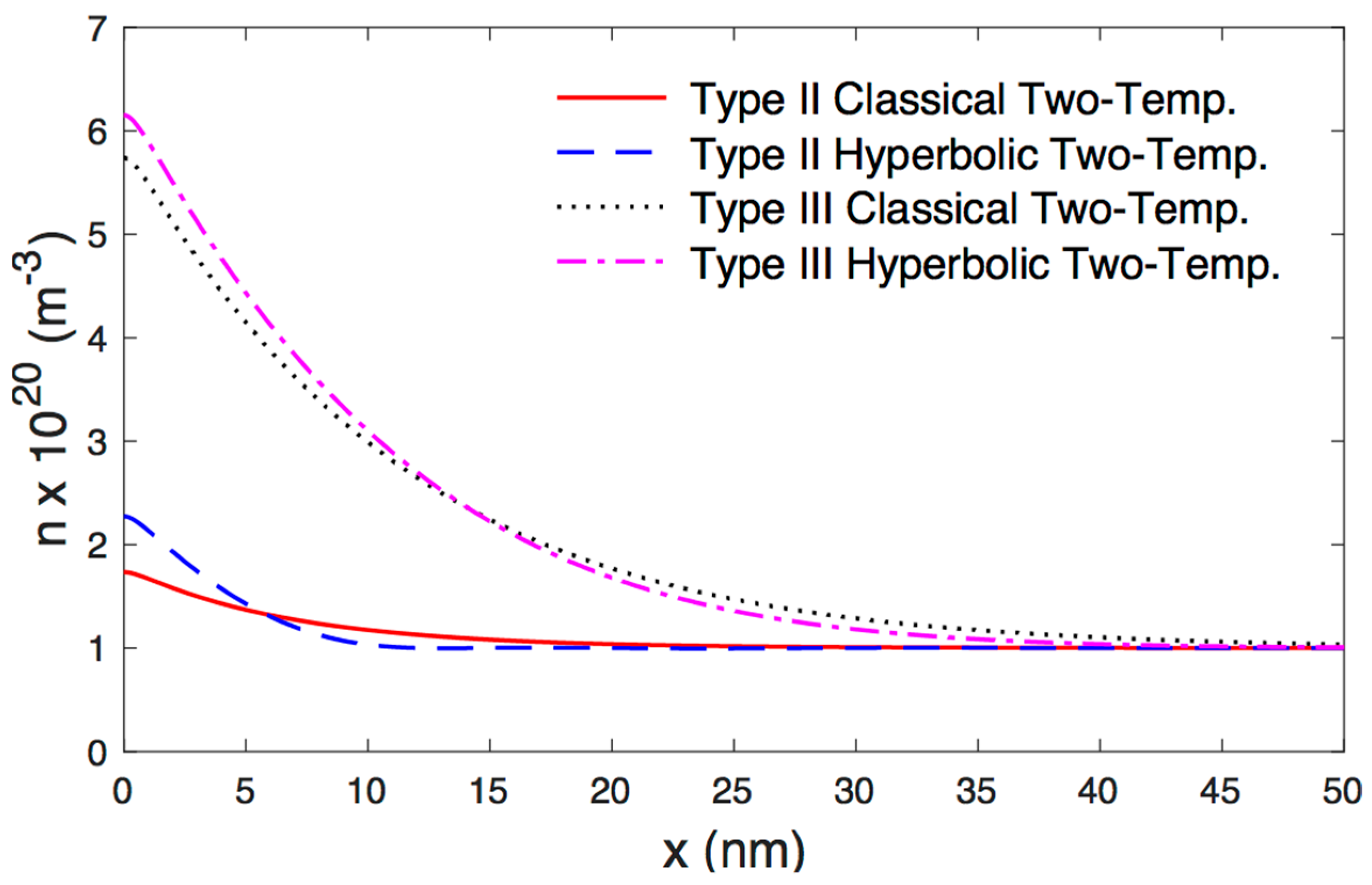
4. Numerical Result and Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Todorović, D. Plasma, thermal, and elastic waves in semiconductors. Rev. Sci. Instrum. 2003, 74, 582–585. [Google Scholar] [CrossRef]
- Todorović, D. Photothermal and electronic elastic effects in microelectromechanical structures. Rev. Sci. Instrum. 2003, 74, 578–581. [Google Scholar] [CrossRef]
- Song, Y.; Todorovic, D.M.; Cretin, B.; Vairac, P. Study on the generalized thermoelastic vibration of the optically excited semiconducting microcantilevers. Int. J. Solids Struct. 2010, 47, 1871–1875. [Google Scholar] [CrossRef]
- Song, Y.; Todorovic, D.M.; Cretin, B.; Vairac, P.; Xu, J.; Bai, J. Bending of Semiconducting Cantilevers under Photothermal Excitation. Int. J. Thermophys. 2014, 35, 305–319. [Google Scholar] [CrossRef]
- Song, Y.; Bai, J.; Ren, Z. Reflection of Plane Waves in a Semiconducting Medium under Photothermal Theory. Int. J. Thermophys. 2012, 33, 1270–1287. [Google Scholar] [CrossRef]
- Song, Y.; Bai, J.; Ren, Z. Study on the reflection of photothermal waves in a semiconducting medium under generalized thermoelastic theory. Acta Mech. 2012, 223, 1545–1557. [Google Scholar] [CrossRef]
- Lotfy, K. The elastic wave motions for a photothermal medium of a dual-phase-lag model with an internal heat source and gravitational field. Can. J. Phys. 2016, 94, 400–409. [Google Scholar] [CrossRef]
- Hobiny, A.D.; Abbas, I.A. A study on photothermal waves in an unbounded semiconductor medium with cylindrical cavity. Mech. Time-Depend. Mater. 2017, 21, 61–72. [Google Scholar] [CrossRef]
- Chen, P.J.; Gurtin, M.E. On a theory of heat conduction involving two temperatures. Z. Angew. Math. Phys. 1968, 19, 614–627. [Google Scholar] [CrossRef]
- Chen, P.J.; Gurtin, M.E.; Williams, W.O. On the thermodynamics of non-simple elastic materials with two temperatures. Z. Angew. Math. Phys. 1969, 20, 107–112. [Google Scholar] [CrossRef]
- Gurtin, M.E.; Williams, W.O. An axiomatic foundation for continuum thermodynamics. Arch. Ration. Mech. Anal. 1967, 26, 83–117. [Google Scholar] [CrossRef]
- Ezzat, M.A.; El-Karamany, A.S.; Ezzat, S.M. Two-temperature theory in magneto-thermoelasticity with fractional order dual-phase-lag heat transfer. Nucl. Eng. Des. 2012, 252, 267–277. [Google Scholar] [CrossRef]
- Abbas, I.A.; Youssef, H.M. Two-temperature generalized thermoelasticity under ramp-type heating by finite element method. Meccanica 2013, 48, 331–339. [Google Scholar] [CrossRef]
- Youssef, H.M.; El-Bary, A.A. Theory of hyperbolic two-temperature generalized thermoelasticity. Mater. Phys. Mech. 2018, 40, 158–171. [Google Scholar]
- Kumar, R.; Prasad, R.; Kumar, R. Thermoelastic interactions on hyperbolic two-temperature generalized thermoelasticity in an infinite medium with a cylindrical cavity. Eur. J. Mech.-A/Solids 2020, 82, 104007. [Google Scholar] [CrossRef]
- Prasad, R.; Kumar, R. On the characterization and stability of plane waves under hyperbolic two-temperature generalized thermoelasticity. J. Therm. Stress. 2020, 1–18. [Google Scholar] [CrossRef]
- Ezzat, M.A. Hyperbolic thermal-plasma wave propagation in semiconductor of organic material. Waves Random Complex Media 2020, 1–25. [Google Scholar] [CrossRef]
- Alzahrani, F.S.; Abbas, I.A. Photo-thermo-elastic interactions without energy dissipation in a semiconductor half-space. Results Phys. 2019, 15, 102805. [Google Scholar] [CrossRef]
- Hobiny, A.; Abbas, I. A GN model on photothermal interactions in a two-dimensions semiconductor half space. Results Phys. 2019, 15, 102588. [Google Scholar] [CrossRef]
- Lotfy, K.; El-Bary, A.; Hassan, W.; Alharbi, A.; Almatrafi, M. Electromagnetic and Thomson effects during photothermal transport process of a rotator semiconductor medium under hydrostatic initial stress. Results Phys. 2020, 16, 102983. [Google Scholar] [CrossRef]
- Ali, H.; Jahangir, A.; Khan, A. Reflection of waves in a rotating semiconductor nanostructure medium through torsion-free boundary condition. Indian J. Phys. 2019, 1–9. [Google Scholar] [CrossRef]
- Alzahrani, F.S.; Abbas, I.A. Photo-Thermal Interactions in a Semiconducting Media with a Spherical Cavity under Hyperbolic Two-Temperature Model. Mathematics 2020, 8, 585. [Google Scholar] [CrossRef]
- Lotfy, K.; El-Bary, A.; Tantawi, R. Effects of variable thermal conductivity of a small semiconductor cavity through the fractional order heat-magneto-photothermal theory. Eur. Phys. J. Plus 2019, 134, 280. [Google Scholar] [CrossRef]
- Bera, M.B.; Das, N.C.; Lahiri, A. Eigenvalue approach to two-temperature generalized thermoelastic interactions in an annular disk. J. Therm. Stress. 2015, 38, 1310–1324. [Google Scholar] [CrossRef]
- Santra, S.; Lahiri, A.; Das, N.C. Eigenvalue approach on thermoelastic interactions in an infinite elastic solid with voids. J. Therm. Stress. 2014, 37, 440–454. [Google Scholar] [CrossRef]
- Kumar, R.; Abbas, I.A. Deformation due to thermal source in micropolar thermoelastic media with thermal and conductive temperatures. J. Comput. Theor. Nanosci. 2013, 10, 2241–2247. [Google Scholar] [CrossRef]
- Marin, M.; Nicaise, S. Existence and stability results for thermoelastic dipolar bodies with double porosity. Contin. Mech. Thermodyn. 2016, 28, 1645–1657. [Google Scholar] [CrossRef]
- Marin, M.; Ellahi, R.; Chirilă, A. On solutions of Saint-Venant’s problem for elastic dipolar bodies with voids. Carpath. J. Math. 2017, 33, 219–232. [Google Scholar]
- Mondal, S.; Sur, A. Photo-thermo-elastic wave propagation in an orthotropic semiconductor with a spherical cavity and memory responses. Waves Random Complex Media 2020, 1–24. [Google Scholar] [CrossRef]
- Marin, M.; Vlase, S.; Ellahi, R.; Bhatti, M. On the partition of energies for the backward in time problem of thermoelastic materials with a dipolar structure. Symmetry 2019, 11, 863. [Google Scholar] [CrossRef]
- Marin, M.; Craciun, E.-M.; Pop, N. Considerations on mixed initial-boundary value problems for micropolar porous bodies. Dyn. Syst. Appl. 2016, 25, 175–196. [Google Scholar]
- Hobiny, A. Effect of the hyperbolic two-temperature model without energy dissipation on Photo-thermal interaction in a semi-conducting medium. Results Phys. 2020, 18, 103167. [Google Scholar] [CrossRef]
- Abd-Elaziz, E.M.; Marin, M.; Othman, M.I. On the effect of Thomson and initial stress in a thermo-porous elastic solid under GN electromagnetic theory. Symmetry 2019, 11, 413. [Google Scholar] [CrossRef]
- Itu, C.; Öchsner, A.; Vlase, S.; Marin, M.I. Improved rigidity of composite circular plates through radial ribs. Proc. Inst. Mech. Eng. Part L J. Mater. Des. Appl. 2019, 233, 1585–1593. [Google Scholar] [CrossRef]
- Vlase, S.; Marin, M.; Öchsner, A.; Scutaru, M. Motion equation for a flexible one-dimensional element used in the dynamical analysis of a multibody system. Contin. Mech. Thermodyn. 2019, 31, 715–724. [Google Scholar] [CrossRef]
- Abbas, I.A.; Marin, M. Analytical solution of thermoelastic interaction in a half-space by pulsed laser heating. Phys. E Low-Dimens. Syst. Nanostruct. 2017, 87, 254–260. [Google Scholar] [CrossRef]
- Hobiny, A.; Abbas, I.A. Analytical solutions of photo-thermo-elastic waves in a nonhomogenous semiconducting material. Results Phys. 2018, 10, 385–390. [Google Scholar] [CrossRef]
- Mandelis, A.; Nestoros, M.; Christofides, C. Thermoelectronic-wave coupling in laser photothermal theory of semiconductors at elevated temperatures. Opt. Eng. 1997, 36, 459–468. [Google Scholar] [CrossRef]
- Abbas, I.A. A dual phase lag model on thermoelastic interaction in an infinite fiber-reinforced anisotropic medium with a circular hole. Mech. Based Des. Struct. Mach. 2015, 43, 501–513. [Google Scholar] [CrossRef]
- Abbas, I.A. The effects of relaxation times and a moving heat source on a two-temperature generalized thermoelastic thin slim strip. Can. J. Phys. 2015, 93, 585–590. [Google Scholar] [CrossRef]
- Stehfest, H. Algorithm 368: Numerical inversion of Laplace transforms [D5]. Commun. ACM 1970, 13, 47–49. [Google Scholar] [CrossRef]
- Abbas, I.A.; Hobiny, A. A two-temperature photothermal interaction in a semiconductor medium containing a cylindrical hole. Int. J. Thermophys. 2018, 39, 17. [Google Scholar] [CrossRef]



© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alzahrani, F.; Abbas, I. The Effect of a Hyperbolic Two-Temperature Model with and without Energy Dissipation in a Semiconductor Material. Mathematics 2020, 8, 1711. https://doi.org/10.3390/math8101711
Alzahrani F, Abbas I. The Effect of a Hyperbolic Two-Temperature Model with and without Energy Dissipation in a Semiconductor Material. Mathematics. 2020; 8(10):1711. https://doi.org/10.3390/math8101711
Chicago/Turabian StyleAlzahrani, Faris, and Ibrahim Abbas. 2020. "The Effect of a Hyperbolic Two-Temperature Model with and without Energy Dissipation in a Semiconductor Material" Mathematics 8, no. 10: 1711. https://doi.org/10.3390/math8101711
APA StyleAlzahrani, F., & Abbas, I. (2020). The Effect of a Hyperbolic Two-Temperature Model with and without Energy Dissipation in a Semiconductor Material. Mathematics, 8(10), 1711. https://doi.org/10.3390/math8101711





