Assessing the Performance of Green Mines via a Hesitant Fuzzy ORESTE–QUALIFLEX Method
Abstract
1. Introduction
2. Literature Review
3. Methodology
3.1. Hesitant Fuzzy Sets
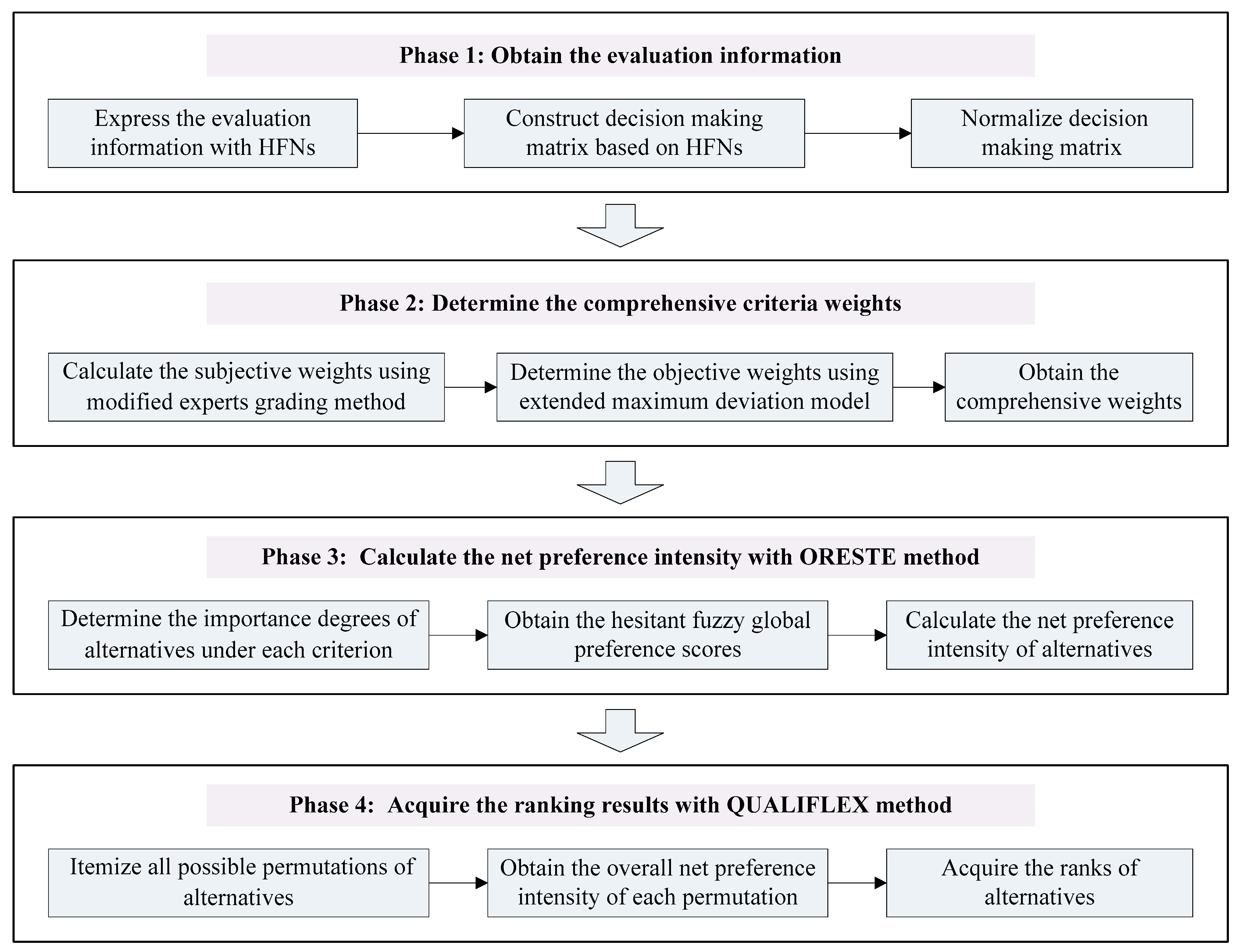
3.2. Hesitant Fuzzy ORESTE–QUALIFLEX Method
4. Case Study
4.1. Engineering Background Description
4.2. Assessment Criteria System of Green Mines
4.3. Performance Assessment of Green Mines
5. Discussions
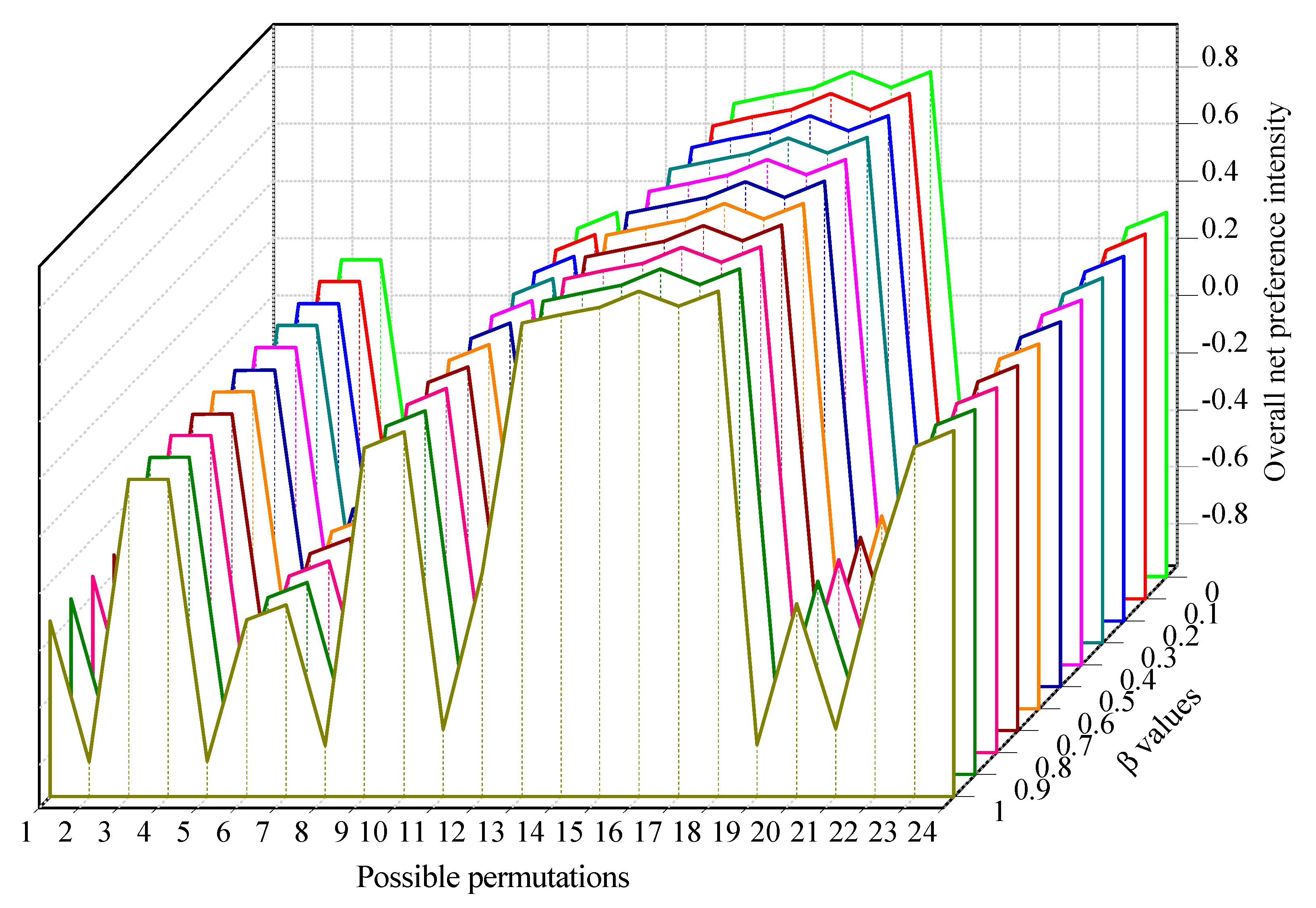
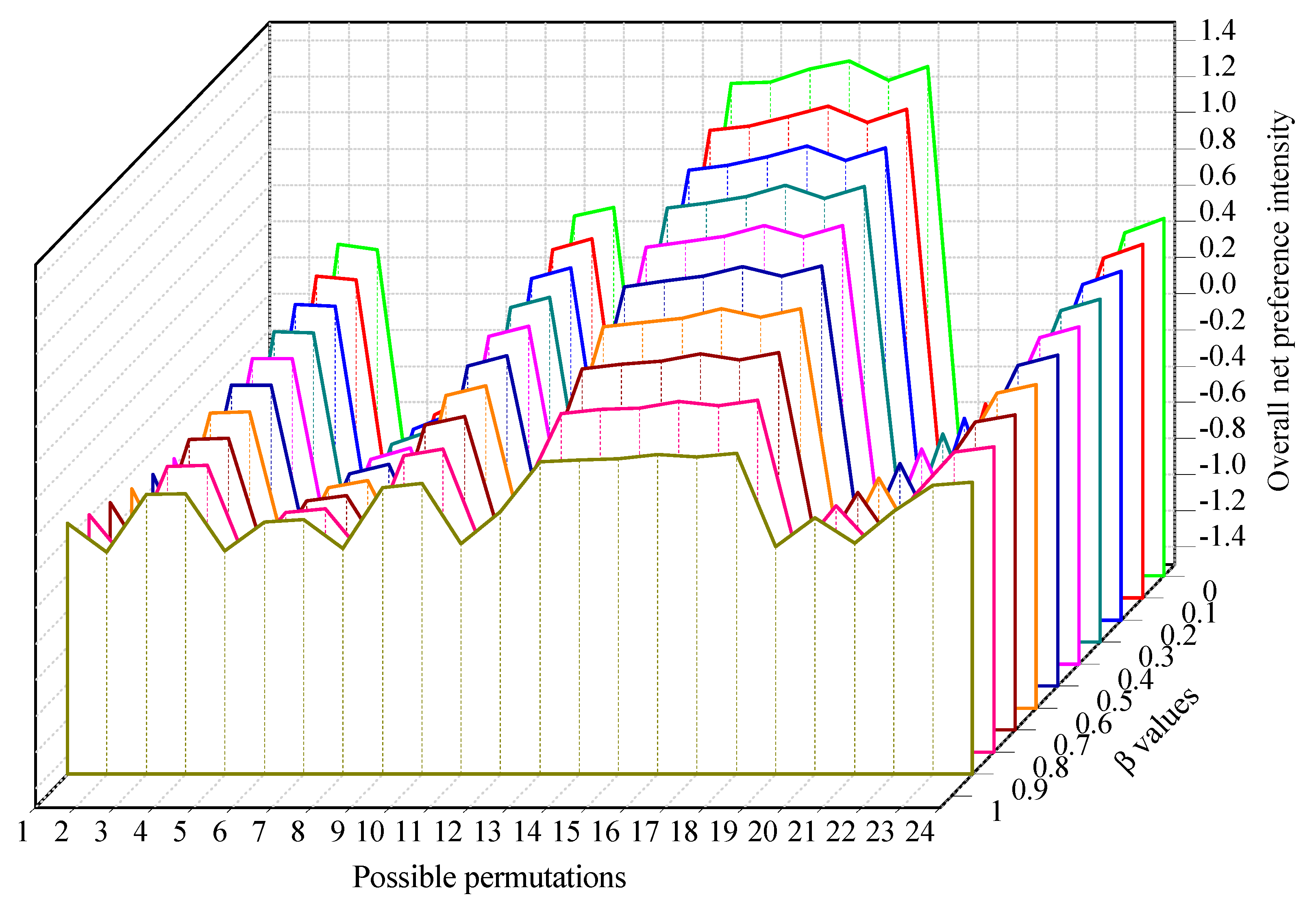
5.1. Sensitivity Analysis
5.2. Comparison Analysis
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Ali, S.H.; Giurco, D.; Arndt, N.; Nickless, E.; Brown, G.; Demetriades, A.; Durrheim, R.; Enriquez, M.A.; Kinnaird, J.; Littleboy, A.; et al. Mineral supply for sustainable development requires resource governance. Nature 2017, 543, 367–372. [Google Scholar] [CrossRef] [PubMed]
- Luo, S.Z.; Liang, W.Z.; Xing, L.N. Selection of mine development scheme based on similarity measure under fuzzy environment. Neural Comput. Appl. 2019, 1–12. [Google Scholar] [CrossRef]
- Sun, S.Y.; Sun, H.; Zhang, D.S.; Zhang, J.F.; Cai, Z.Y.; Qin, G.H.; Song, Y.M. Response of soil microbes to vegetation restoration in coal mining subsidence areas at Huaibei coal mine China. Int. J. Environ. Res. Public Health 2019, 16, 1757. [Google Scholar] [CrossRef] [PubMed]
- Liang, W.Z.; Zhao, G.Y.; Wu, H.; Dai, B. Risk assessment of rockburst via an extended MABAC method under fuzzy environment. Tunn. Undergr. Space Tech. 2019, 83, 533–544. [Google Scholar] [CrossRef]
- Timofeev, I.; Kosheleva, N.; Kasimov, N. Contamination of soils by potentially toxic elements in the impact zone of tungsten—Molybdenum ore mine in the Baikal region: A survey and risk assessment. Sci. Total. Environ. 2018, 642, 63–76. [Google Scholar] [CrossRef] [PubMed]
- Shang, D.L.; Yin, G.Z.; Li, X.S.; Li, Y.J.; Jiang, C.B.; Kang, X.T.; Liu, C.; Zhang, C. Analysis for green mine (phosphate) performance of China: An evaluation index system. Resour. Policy 2015, 46, 71–84. [Google Scholar] [CrossRef]
- Wang, W.S.; Zou, J.L. Efficiency evaluation and optimization of green mining construction in coal enterprises based on DEA. China Coal 2013, 39, 119–121. [Google Scholar]
- Xu, J.Q.; Yu, G.; He, D.Y. Multi-expert evaluation method of Green Mine: A case study on Xinwen mining group’s Huafeng mine. Resour. Ind. 2016, 18, 61–68. [Google Scholar]
- Hu, J.H.; Xiao, K.L.; Chen, X.H.; Liu, Y.M. Interval type-2 hesitant fuzzy set and its application in multi-criteria decision making. Comput. Ind. Eng. 2015, 87, 91–103. [Google Scholar] [CrossRef]
- Luo, S.Z.; Zhang, H.Y.; Wang, J.Q.; Li, L. Group decision-making approach for evaluating the sustainability of constructed wetlands with probabilistic linguistic preference relations. J. Oper. Res. Soc. 2019, 1–17. [Google Scholar] [CrossRef]
- Torra, V. Hesitant fuzzy sets. Int. J. Intell. Syst. 2010, 25, 529–539. [Google Scholar] [CrossRef]
- Alcantud, J.C.R.; Torra, V. Decomposition theorems and extension principles for hesitant fuzzy sets. Inform. Fusion 2018, 41, 48–56. [Google Scholar] [CrossRef]
- Torra, V.; Narukawa, Y. On hesitant fuzzy sets and decision. In Proceedings of the 18th IEEE International Conference on Fuzzy Systems, Jeju Island, Korea, 20–24 August 2009; pp. 1378–1382. [Google Scholar]
- Rodríguez, R.M.; Martínez, L.; Torra, V.; Xu, Z.S.; Herrera, F. Hesitant fuzzy sets: state of the art and future directions. Int. J. Intell. Syst. 2014, 29, 495–524. [Google Scholar] [CrossRef]
- Xu, Y.; Liu, L.Z.; Zhang, X.Y. Multilattices on typical hesitant fuzzy sets. Inform. Sci. 2019, 491, 63–73. [Google Scholar] [CrossRef]
- Deveci, M.; Özcan, E.; John, R.; Öner, S.C. Interval type-2 hesitant fuzzy set method for improving the service quality of domestic airlines in Turkey. J. Air Trans. Manag. 2018, 69, 83–98. [Google Scholar] [CrossRef]
- Iordache, M.; Schitea, D.; Deveci, M.; Akyurt, İ.Z.; Iordache, I. An integrated ARAS and interval type-2 hesitant fuzzy sets method for underground site selection: Seasonal hydrogen storage in salt caverns. J. Petrol. Sci. Eng. 2019, 175, 1088–1098. [Google Scholar] [CrossRef]
- Iiang, W.Z.; Zhao, G.Y.; Wang, X.; Zhao, J.; Ma, C.D. Assessing the rockburst risk for deep shafts via distance-based multi-criteria decision making approaches with hesitant fuzzy information. Eng. Geol. 2019, 105211. [Google Scholar] [CrossRef]
- Xu, Z.S.; Zhang, X.L. Hesitant fuzzy multi-attribute decision making based on TOPSIS with incomplete weight information. Knowl. Based Syst. 2013, 52, 53–64. [Google Scholar] [CrossRef]
- Zeng, S.; Baležentis, A.; Su, W. The multi-criteria hesitant fuzzy group decision making with MULTIMOORA method. Econ. Comput. Econ. Cybern. 2013, 47, 171–184. [Google Scholar]
- Zhang, N.; Wei, G.W. Extension of VIKOR method for decision making problem based on hesitant fuzzy set. Appl. Math. Model. 2013, 37, 4938–4947. [Google Scholar] [CrossRef]
- Zhang, X.L.; Xu, Z.S. The TODIM analysis approach based on novel measured functions under hesitant fuzzy environment. Knowl. Based Syst. 2014, 61, 48–58. [Google Scholar] [CrossRef]
- Zhang, X.L.; Xu, Z.S. Interval programming method for hesitant fuzzy multi-attribute group decision making with incomplete preference over alternatives. Comput. Ind. Eng. 2014, 75, 217–229. [Google Scholar] [CrossRef]
- Chen, N.; Xu, Z.S.; Xia, M.M. The ELECTRE I multi-criteria decision-making method based on hesitant fuzzy sets. Int. J. Inf. Tech. Decis. 2015, 14, 621–657. [Google Scholar] [CrossRef]
- Chen, N.; Xu, Z.S. Hesitant fuzzy ELECTRE II approach: A new way to handle multi-criteria decision making problems. Inform. Sci. 2015, 292, 175–197. [Google Scholar] [CrossRef]
- Zhang, X.L.; Xu, Z.S. Hesitant fuzzy qualiflex approach with a signed distance-based comparison method for multiple criteria decision analysis. Expert Syst. Appl. 2015, 42, 873–884. [Google Scholar] [CrossRef]
- Mahmoudi, A.; Sadi-Nezhad, S.; Makui, A.; Vakili, M.R. An extension on PROMETHEE based on the typical hesitant fuzzy sets to solve multi-attribute decision-making problem. Kybernetes 2016, 45, 1213–1231. [Google Scholar] [CrossRef]
- Acar, C.; Beskese, A.; Temur, G.T. Sustainability analysis of different hydrogen production options using hesitant fuzzy AHP. Int. J. Hydrogen Energy 2018, 43, 18059–18076. [Google Scholar] [CrossRef]
- Kutlu Gündoğdu, F.; Kahraman, C.; Civan, H.N. A novel hesitant fuzzy EDAS method and its application to hospital selection. J. Intell. Fuzzy Syst. 2018, 35, 6353–6365. [Google Scholar] [CrossRef]
- Galo, N.R.; Calache, L.D.D.R.; Carpinetti, L.C.R. A group decision approach for supplier categorization based on hesitant fuzzy and ELECTRI TRI. Int. J. Prod. Econ. 2018, 202, 182–196. [Google Scholar] [CrossRef]
- Peng, J.J.; Wang, J.Q.; Yang, W.E. A multi-valued neutrosophic qualitative flexible approach based on likelihood for multi-criteria decision-making problems. Int. J. Syst. Sci. 2017, 48, 425–435. [Google Scholar] [CrossRef]
- Dong, J.Y.; Chen, Y.; Wan, S.P. A cosine similarity based QUALIFLEX approach with hesitant fuzzy linguistic term sets for financial performance evaluation. Appl. Soft Comput. 2018, 69, 316–329. [Google Scholar] [CrossRef]
- Tian, Z.P.; Wang, J.; Wang, J.Q.; Zhang, H.Y. Simplified neutrosophic linguistic multi-criteria group decision-making approach to green product development. Group Decis. Negotiat. 2017, 26, 597–627. [Google Scholar] [CrossRef]
- Ji, P.; Zhang, H.Y.; Wang, J.Q. Fuzzy decision-making framework for treatment selection based on the combined QUALIFLEX–TODIM method. Int. J. Syst. Sci. 2017, 48, 3072–3086. [Google Scholar] [CrossRef]
- Liang, W.Z.; Zhao, G.Y.; Hong, C.S. Performance assessment of circular economy for phosphorus chemical firms based on VIKOR-QUALIFLEX method. J. Clean. Prod. 2018, 196, 1365–1378. [Google Scholar] [CrossRef]
- Zhang, C.; Wu, X.L.; Wu, D.; Liao, H.C.; Luo, L.; Herrera-Viedma, E. An intuitionistic multiplicative ORESTE method for patients’ prioritization of hospitalization. Int. J. Environ. Res. Public Health 2018, 15, 777. [Google Scholar] [CrossRef] [PubMed]
- Roubens, M. Preference relations an actions and criteria in multicriteria decision making. Eur. J. Oper. Res. 1982, 10, 51–55. [Google Scholar] [CrossRef]
- Yerlikaya, M.A.; Arikan, F. Constructing the performance effectiveness order of SME supports programmes via Promethee and Oreste techniques. J. Fac. Eng. Archit. Gaz. 2016, 31, 1007–1016. [Google Scholar]
- Kaya, T. Monitoring brand performance based on household panel indicators using a fuzzy rank-based ORESTE methodology. J. Mult. Valued Log. Soft 2018, 31, 443–467. [Google Scholar]
- Wu, X.L.; Liao, H.C. An approach to quality function deployment based on probabilistic linguistic term sets and ORESTE method for multi-expert multi-criteria decision making. Inform. Fusion 2018, 43, 13–26. [Google Scholar] [CrossRef]
- Tian, Z.P.; Nie, R.X.; Wang, J.Q.; Zhang, H.Y. Signed distance-based ORESTE for multicriteria group decision-making with multigranular unbalanced hesitant fuzzy linguistic information. Expert Syst. 2019, 36, e12350. [Google Scholar] [CrossRef]
- Li, J.; Luo, L.; Wu, X.L.; Liao, C.C.; Liao, H.C.; Shen, W.W. Prioritizing the elective surgery patient admission in a Chinese public tertiary hospital using the hesitant fuzzy linguistic ORESTE method. Appl. Soft Comput. 2019, 78, 407–419. [Google Scholar] [CrossRef]
- Liang, W.Z.; Luo, S.Z.; Zhao, G.Y. Evaluation of cleaner production for gold mines employing a hybrid multi-criteria decision making approach. Sustainability 2019, 11, 146. [Google Scholar] [CrossRef]
- Delgado, A.; Romero, I. Environmental conflict analysis using an integrated grey clustering and entropy-weight method: A case study of a mining project in Peru. Environ. Model. Softw. 2016, 77, 108–121. [Google Scholar] [CrossRef]
- Kokangül, A.; Polat, U.; Dağsuyu, C. A new approximation for risk assessment using the AHP and Fine Kinney methodologies. Saf. Sci. 2017, 91, 24–32. [Google Scholar]
- Wang, Y.M. Using the method of maximizing deviations to make decision for multi-indicies. J. Syst. Eng. Electron. 1997, 8, 21–26. [Google Scholar]
- Mardani, A.; Nilashi, M.; Zakuan, N.; Loganathan, N.; Soheilirad, S.; Saman, M.Z.M.; Ibrahim, O. A systematic review and meta-analysis of SWARA and WASPAS methods: Theory and applications with recent fuzzy developments. Appl. Soft Comput. 2017, 57, 265–292. [Google Scholar] [CrossRef]
- Luo, S.Z.; Liang, W.Z. Optimization of roadway support schemes with likelihood–based MABAC method. Appl. Soft Comput. 2019, 80, 80–92. [Google Scholar] [CrossRef]
- Xia, M.M.; Xu, Z.S. Hesitant fuzzy information aggregation in decision making. Int. J. Approx. Reason. 2011, 52, 395–407. [Google Scholar] [CrossRef]
- Xu, Z.S.; Xia, M.M. On distance and correlation measures of hesitant fuzzy information. Int. J. Intell. Syst. 2011, 26, 410–425. [Google Scholar] [CrossRef]
- Zhou, Z.X.; Dou, Y.J.; Liao, T.J.; Tan, Y.J. A preference model for supplier selection based on hesitant fuzzy sets. Sustainability 2018, 10, 659. [Google Scholar] [CrossRef]
- Farhadinia, B. A series of score functions for hesitant fuzzy sets. Inform. Sci. 2014, 277, 102–110. [Google Scholar] [CrossRef]
- Liang, W.Z.; Zhao, G.Y.; Wu, H.; Chen, Y. Assessing the risk degree of goafs by employing hybrid TODIM method under uncertainty. Bull. Eng. Geol. Environ. 2018, 78, 3767–3782. [Google Scholar] [CrossRef]
- Ministry of Natural Resources of the People’s Republic of China (MNRPRC). Announcement of the Nine Industry Standards such as Green Mine Construction Specification of Non-Metallic Minerals Industry Released by Ministry of Natural Resources of the People’s Republic of China. 2018. Available online: http://gi.mlr.gov.cn/201806/t20180628_1962186.html. (accessed on 5 July 2019).
| Author (Year) | MCDM Methods | Case Study |
|---|---|---|
| Xu and Zhang (2013) [19] | Technique for order performance by similarity to ideal solution (TOPSIS) | Energy policy selection |
| Zeng et al. (2013) [20] | Multiobjective optimization by ratio analysis plus the full multiplicative from (MULTIMOORA) | Manager selection |
| Zhang and Wei (2013) [21] | Visekriterijumsko kompromisno rangiranje (VIKOR) | Project selection |
| Zhang and Xu (2014) [22] | Traditional acronym in Portuguese of interactive and multicriteria decision-making (TODIM) | Evaluation of the service quality among domestic airlines |
| Zhang and Xu (2014) [23] | Linear programming technique for multidimensional analysis of preference (LINMAP) | Energy project selection |
| Chen et al. (2015) [24] | Elimination and choice translating reality (ELECTRE) I | Project selection |
| Chen and Xu (2015) [25] | ELECTRE II | Third-party reverse logistics provider selection |
| Zhang and Xu (2015) [26] | QUALIFLEX | Green supplier selection |
| Mahmoudi et al. (2016) [27] | Preference ranking organization method for enrichment evaluation (PROMETHEE) | Ranking of overseas outstanding teachers |
| Acar et al. (2018) [28] | Analytic hierarchy process (AHP) | Sustainability evaluation of hydrogen production options |
| Kutlu Gündoğdu et al. (2018) [29] | Evaluation based on distance from average solution (EDAS) | Hospital selection |
| Galo et al. (2018) [30] | ELECTRE TRI | Supplier categorization |
| Evaluation Criteria | Descriptions |
|---|---|
| Mining area environment | This refers to the environment of the mining area and mainly includes appearance of the mining area, layout of function, and greening of the mining area. |
| Resource development approaches | This refers to the superiority of development approaches and mainly includes mining technology, environmental monitoring, and environmental restoration. |
| Comprehensive utilization of resources | This refers to the comprehensive utilization of resources and mainly includes the utilization of solid waste, wastewater, and associated resources. |
| Energy conservation and emission reduction | This refers to the saving of energy and emission of various pollutants and mainly includes energy conservation, discharge of solid waste, wastewater, exhaust gas, and dust. |
| Technological innovation | This refers to the level of technical innovation and mainly includes innovation ability, automation performance, and digital mine. |
| Management level | This refers to the management level of enterprise and mainly includes the culture, management, and credit of enterprise, social stability, and responsibility. |
| {0.5,0.7,0.6,0.6} | {0.8,0.7,0.7,0.7} | {0.9,0.8,0.9,0.7} | {0.7,0.6,0.6,0.7} | {0.6,0.7,0.6,0.5} | {0.6,0.5,0.5,0.7} | |
| {0.9,0.7,0.9,0.8} | {0.7,0.7,0.6,0.6} | {0.6,0.7,0.8,0.6} | {0.7,0.6,0.5,0.5} | {0.5,0.6,0.6,0.5} | {0.7,0.8,0.8,0.7} | |
| {0.7,0.8,0.7,0.8} | {0.9,0.7,0.8,0.7} | {0.6,0.8,0.8,0.7} | {0.9,0.8,0.9,0.7} | {0.8,0.7,0.6,0.7} | {0.8,0.8,0.7,0.8} | |
| {0.7,0.6,0.6,0.5} | {0.7,0.5,0.6,0.6} | {0.5,0.6,0.5,0.7} | {0.8,0.7,0.8,0.9} | {0.7,0.9,0.7,0.7} | {0.6,0.7,0.7,0.8} |
| 0.6 | 0.8 | 0.8 | 0.7 | 0.8 | 0.7 | |
| 0.8 | 0.7 | 0.7 | 0.8 | 0.9 | 0.8 | |
| 0.7 | 0.8 | 0.9 | 0.7 | 0.9 | 0.8 | |
| 0.8 | 0.8 | 0.8 | 0.8 | 0.8 | 0.9 | |
| {0.6,0.8,0.7,0.8} | {0.8,0.7,0.8,0.8} | {0.8,0.7,0.9,0.8} | {0.7,0.8,0.7,0.8} | {0.8,0.9,0.9,0.8} | {0.7,0.8,0.8,0.9} | |
| 0.7200 | 0.7737 | 0.7969 | 0.7483 | 0.8485 | 0.7969 | |
| 0.1537 | 0.1652 | 0.1701 | 0.1597 | 0.1811 | 0.1701 |
| A1 | 0.2158 | 0.2643 | 0.2952 | 0.2286 | 0.2305 | 0.2042 |
| A2 | 0.2973 | 0.2367 | 0.2410 | 0.2008 | 0.2119 | 0.2684 |
| A3 | 0.2711 | 0.2814 | 0.2590 | 0.2895 | 0.2694 | 0.2776 |
| A4 | 0.2158 | 0.2176 | 0.2047 | 0.2811 | 0.2883 | 0.2498 |
| 0.0000 | 0.6517 | 1.0000 | 0.3245 | 0.3090 | 0.0000 | |
| 1.0000 | 0.3483 | 0.3874 | 0.0000 | 0.0000 | 0.7829 | |
| 0.6461 | 1.0000 | 0.6126 | 1.0000 | 0.6910 | 1.0000 | |
| 0.0000 | 0.0000 | 0.0000 | 0.8209 | 1.0000 | 0.6044 |
| 0.1192 | 0.4728 | 0.7177 | 0.2615 | 0.2503 | 0.1118 | |
| 0.7171 | 0.2681 | 0.3002 | 0.1254 | 0.1221 | 0.5647 | |
| 0.4722 | 0.7150 | 0.4502 | 0.7181 | 0.5036 | 0.7159 | |
| 0.1192 | 0.1058 | 0.1228 | 0.5938 | 0.7176 | 0.4417 |
| A1 | 0.0000 | −0.0274 | −0.2736 | −0.0279 |
| 0.0274 | 0.0000 | −0.2462 | −0.0005 | |
| 0.2736 | 0.2462 | 0.0000 | 0.2457 | |
| 0.0279 | 0.0005 | −0.2457 | 0.0000 |
| −0.3301 | −0.8214 | 0.1624 | 0.1635 | −0.8203 | −0.3279 | −0.2753 | −0.7666 |
| ∆10 | |||||||
| 0.2720 | 0.3279 | −0.7108 | −0.1635 | 0.7097 | 0.7381 | 0.7644 | 0.8203 |
| ∆24 | |||||||
| 0.7666 | 0.8214 | −0.7644 | −0.2720 | −0.7097 | −0.1624 | 0.2753 | 0.3301 |
| Rank | Rank | ||
|---|---|---|---|
| 0 | 0.6 | ||
| 0.1 | 0.7 | ||
| 0.2 | 0.8 | ||
| 0.3 | 0.9 | ||
| 0.4 | 1 | ||
| 0.5 |
| Rank | Rank | ||
|---|---|---|---|
| 0 | 0.6 | ||
| 0.1 | 0.7 | ||
| 0.2 | 0.8 | ||
| 0.3 | 0.9 | ||
| 0.4 | 1 | -- | |
| 0.5 |
| −0.1281 | −0.2959 | 0.0521 | 0.0646 | −0.2834 | −0.1032 | −0.1153 | −0.2830 |
| Θ12 | |||||||
| 0.0778 | 0.1032 | −0.2577 | −0.0646 | 0.2452 | 0.2641 | 0.2581 | 0.2834 |
| Θ22 | |||||||
| 0.2830 | 0.2959 | −0.2581 | −0.0778 | −0.2452 | −0.0521 | 0.1153 | 0.1281 |
| - | R | R | R | |
| P | - | R | I | |
| P | P | - | P | |
| A4 | P | I | R | - |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liang, W.; Dai, B.; Zhao, G.; Wu, H. Assessing the Performance of Green Mines via a Hesitant Fuzzy ORESTE–QUALIFLEX Method. Mathematics 2019, 7, 788. https://doi.org/10.3390/math7090788
Liang W, Dai B, Zhao G, Wu H. Assessing the Performance of Green Mines via a Hesitant Fuzzy ORESTE–QUALIFLEX Method. Mathematics. 2019; 7(9):788. https://doi.org/10.3390/math7090788
Chicago/Turabian StyleLiang, Weizhang, Bing Dai, Guoyan Zhao, and Hao Wu. 2019. "Assessing the Performance of Green Mines via a Hesitant Fuzzy ORESTE–QUALIFLEX Method" Mathematics 7, no. 9: 788. https://doi.org/10.3390/math7090788
APA StyleLiang, W., Dai, B., Zhao, G., & Wu, H. (2019). Assessing the Performance of Green Mines via a Hesitant Fuzzy ORESTE–QUALIFLEX Method. Mathematics, 7(9), 788. https://doi.org/10.3390/math7090788






