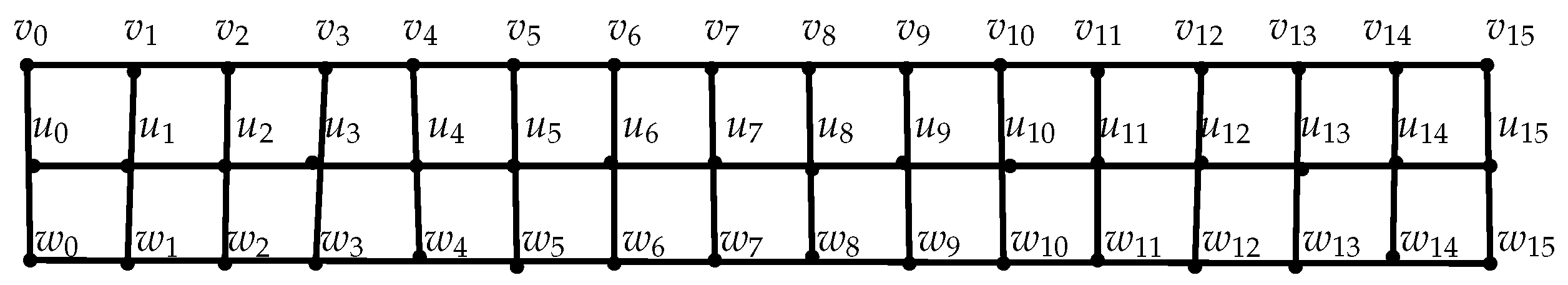
k-Rainbow Domination Number of P₃□P_n

Abstract

Let k be a positive integer, and set $\left[k\right]:=\{1,2,\dots ,k\}$. For a graph G, a k-rainbow dominating function (or kRDF) of G is a mapping $f:$$V\left(G\right)\to 2^{\left[k\right]}$ in such a way that, for any vertex $v\in V\left(G\right)$ with the empty set under f, the condition ${\bigcup }_{u\in N_G\left(v\right)}f\left(u\right)=\left[k\right]$ always holds, where $N_G\left(v\right)$ is the open neighborhood of v. The weight of kRDF f of G is the summation of values of all vertices under f. The k-rainbow domination number of G, denoted by ${\gamma }_{rk}\left(G\right)$, is the minimum weight of a kRDF of G. In this paper, we obtain the k-rainbow domination number of grid $P_3\square P_n$ for $k\in \{2,3,4\}$.

1. Introduction

For a graph G, we denote by $V\left(G\right)$ and $E\left(G\right)$ the vertex set and the edge set of G, respectively. For a vertex $v\in V\left(G\right)$, the open neighborhood of v, denoted by $N_G\left(v\right)$, is the set $\{u\in V(G):uv\in E(G\left)\right\}$ and the closed neighborhood of v, denoted by $N_G\left[v\right]$, is the set $N_G\left(v\right)\cup \left\{v\right\}$. The degree of a vertex $v\in V\left(G\right)$, denoted by $d_G\left(v\right)$, is defined by $d_G\left(v\right)=\left|N_G\left(v\right)\right|$. We let $\delta \left(G\right)$ and $\Delta \left(G\right)$ denote the minimum degree and maximum degree of a graph G, respectively.

Let k be a positive integer, and $\left[k\right]:=\{1,2,\dots ,k\}$. For a graph G, a k-rainbow dominating function (or kRDF) of G is a mapping $f:$$V\left(G\right)\to 2^{\left[k\right]}$ in such a way that for any vertex $v\in V\left(G\right)$ with the empty set under f, the condition ${\bigcup }_{u\in N_G\left(v\right)}f\left(u\right)=\left[k\right]$ always holds. The weight of a kRDF f of G is the value $\omega \left(f\right):={\sum }_{v\in V\left(G\right)}\left|f\left(v\right)\right|$. The k-rainbow domination number of G, denoted by ${\gamma }_{rk}\left(G\right)$, is the minimum weight of a kRDF of G. A kRDF f of G is a ${\gamma }_{rk}$-function if $\omega \left(f\right)={\gamma }_{rk}\left(G\right)$. The k-rainbow domination number was introduced by Brešar, Henning, and Rall [1] was studied by several authors (see, for example [2,3,4,5,6,7,8,9,10,11,12,13,14,15]).

For graphs F and G, we let $F\square G$ denote the Cartesian product of F and G. Vizing [16] conjectured that for arbitrary graphs F and G, $\gamma (F\square G)\ge \gamma \left(F\right)\gamma \left(G\right)$. This conjecture is still open, and the domination number or its related invariants of $F\square G$ are extensively studied with the motivation from Vizing’s conjecture.

Concerning the k-rainbow domination number of $F\square G$, one problem naturally arises: Given two graphs F and G under some conditions, determine ${\gamma }_{rk}(F\square G)$ for all k. In [3], the authors determined ${\gamma }_{rk}(P_2\square P_n)$ for $k=3,4,5$.

In this paper, we examine grid graphs $P_3\square P_n$, and determine the value ${\gamma }_{rk}(P_3\square P_n)$ for $k\in \{2,3,4\}$ and all n, where $P_m$ is the path of order m.

2. 2-Rainbow Domination Number of ${\mathit{P}}_{\mathbf{3}}\square {\mathit{P}}_{\mathit{n}}$

We write $V(P_3\square P_n)=\{v_i,u_i,w_i\mid 0\le i\le n-1\}$ and let $E(P_3\square P_n)=\{v_i{u}_i,u_i{w}_i\mid 0\le i\le n-1\}\cup \{v_i{v}_{i+1},u_i{u}_{i+1},w_i{w}_{i+1}\mid 0\le i\le n-1\}$ (see Figure 1). A 2RDF f is given in three lines, where in the first line there are values of the function f for vertices $\{v_0,v_1,\dots ,v_{n-1}\}$, in the second line of the vertices $\{u_0,u_1,\dots ,u_{n-1}\}$, and in the third line of the vertices $\{w_0,w_1,\dots ,w_{n-1}\}$ (see Figure 2). Furthermore, we use $0,1,2,3$ to encode the sets $\varnothing ,\left\{1\right\},\left\{2\right\},\{1,2\}$.

To provide a complete answer, we need the following fact that can easily be proved as an exercise.

Fact 1.

${\gamma }_{r2}(P_3\square P_3)=4,{\gamma }_{r2}(P_3\square P_4)=6,{\gamma }_{r2}(P_3\square P_5)=7,{\gamma }_{r2}(P_3\square P_6)=8,{\gamma }_{r2}(P_3\square P_7)=10.$

Theorem 1.

For $n\ge 8$, ${\gamma }_{r2}(P_3\square P_n)=⌈{\displaystyle \frac{5n+3}{4}}⌉$.

Proof. 

First, we present constructions of a 2RDF of $P_3\square P_n$ of the desired weight.

• $n\equiv 0\left(\mathrm{mod}8\right)$:
  $020030010200\cdots 300102003001$
  $101000200010\cdots 002000100020$
  $020201003002\cdots 010030020101$
• $n\equiv 1\left(\mathrm{mod}8\right)$:
  $020030010200\cdots 3001020030010$
  $101000200010\cdots 0020001000202$
  $020201003002\cdots 0100300201010$
• $n\equiv 2\left(\mathrm{mod}8\right)$:
  $020030010200\cdots 30010200300101$
  $101000200010\cdots 00200010002020$
  $020201003002\cdots 01003002010101$
• $n\equiv 3\left(\mathrm{mod}8\right)$:
  $020030010200\cdots 300102003001001$
  $101000200010\cdots 002000100020220$
  $020201003002\cdots 010030020101001$
• $n\equiv 4\left(\mathrm{mod}8\right)$:
  $020030010200\cdots 3001020030010010$
  $101000200010\cdots 0020001000202202$
  $020201003002\cdots 0100300201010010$
• $n\equiv 5\left(\mathrm{mod}8\right)$:
  $020030010200\cdots 30010200300102020$
  $101000200010\cdots 00200010002000101$
  $020201003002\cdots 01003002010030020$
• $n\equiv 6\left(\mathrm{mod}8\right)$:
  $020030010200\cdots 3001020030$
  $101000200010\cdots 0020001001$
  $020201003002\cdots 0100300201$
• $n\equiv 7\left(\mathrm{mod}8\right)$:
  $020030010200\cdots 30010200301$
  $101000200010\cdots 00200010002$
  $020201003002\cdots 01003002010$

To show that these are also lower bounds, we prove there is a ${\gamma }_{r2}(P_3\square P_n)$-function, f such that for every $0\le i\le n-1$, $\omega \left(f_i\right)=|f\left(v_i\right)|+|f\left(u_i\right)|+|f\left(w_i\right)|\ge 1$. Let $n\ge 8$ and f be a ${\gamma }_{r2}(P_3\square P_n)$-function such that the cardinality of $S=\{i\mid 0\le i\le n-1\mathrm{and}\omega \left(f_i\right)=0\}$ is as small as possible. We claim that $\left|S\right|=0$. Suppose, to the contrary, that $\left|S\right|\ge 1$ and let s be the smallest positive integer for which $\omega \left(f_s\right)=0$. Then, $\omega \left(f_{s-1}\right)+\omega \left(f_{s+1}\right)\ge 6$. Then, we consider the following cases.

Case 1.

$s=1$ (the case $s=n-1$ is similar).

Then, we have $f\left(v_1\right)=f\left(u_1\right)=f\left(w_1\right)=\{1,2\}$ and the function g defined by $g\left(u_0\right)=\left\{1\right\}$, $g\left(v_1\right)=g\left(w_1\right)=\left\{2\right\}$, $g\left(u_2\right)=f\left(u_2\right)\cup \left\{1\right\}$, $g\left(v_0\right)=g\left(w_0\right)=g\left(u_1\right)=\varnothing$ and $g\left(x\right)=f\left(x\right)$ otherwise, is a 2RDF of $P_3\square P_n$ of weight at most $\omega \left(f\right)$, which contradicts the choice of f.

Case 2.

$s=1$ ($s=n-2$ is similar).

Then, $\omega \left(f_0\right)+\omega \left(f_2\right)\ge 6$ and the function g defined by $g\left(u_0\right)=g\left(u_2\right)=\left\{1\right\}$, $g\left(v_1\right)=g\left(w_1\right)=\left\{2\right\}$, $g\left(v_3\right)=f\left(v_3\right)\cup \left\{2\right\}$, $g\left(w_3\right)=f\left(w_3\right)\cup \left\{2\right\}$, $g\left(v_0\right)=g\left(w_0\right)=g\left(u_1\right)=g\left(v_2\right)=g\left(w_2\right)=\varnothing$ and $g\left(x\right)=f\left(x\right)$ otherwise, is an 2RDF of $P_3\square P_n$ of weight at most $\omega \left(f\right)$, which contradicts the choice of f.

Case 3.

$2\le s\le n-3$.

Since $\omega \left(f_{s-2}\right)\ge 1$, then $|f\left(v_{s-2}\right)|+|f\left(u_{s-2}\right)|+|f\left(w_{s-2}\right)|\ge 1$. First, let $\left|f\right(u_{s-2}\left)\right|\ge 1$. We may assume that $\left\{1\right\}\subseteq f\left(u_{s-2}\right)$. It is easy to see that the function g defined by $g\left(v_{s-1}\right)=g\left(v_{s+1}\right)=g\left(w_{s-1}\right)=g\left(w_{s+1}\right)=\left\{2\right\}$, $g\left(u_s\right)=\left\{1\right\}$, $g\left(u_{s+2}\right)=f\left(u_{s+2}\right)\cup \left\{1\right\}$, $g\left(u_{s-1}\right)=g\left(v_s\right)=g\left(w_s\right)=g\left(u_{s+1}\right)=\varnothing$ and $g\left(x\right)=f\left(x\right)$ otherwise, is an 2RDF of $P_3\square P_n$ of weight at most $\omega \left(f\right)$, which contradicts the choice of f. Now, let $\left|f\right(w_{s-2}\left)\right|\ge 1$ ($\left|f\right(v_{s-2}\left)\right|\ge 1$ is similar). We may assume that $\left\{1\right\}\subseteq f\left(w_{s-2}\right)$. Hence, the function g defined by $g\left(v_{s-2}\right)=f\left(v_{s-2}\right)\cup \left\{1\right\}$, $g\left(v_{s+1}\right)=g\left(u_{s-1}\right)=g\left(w_{s+1}\right)=\left\{2\right\}$, $g\left(u_s\right)=\left\{1\right\}$, $g\left(u_{s+2}\right)=f\left(u_{s+2}\right)\cup \left\{1\right\}$, $g\left(u_{s-1}\right)=g\left(v_s\right)=g\left(w_s\right)=g\left(w_{s-1}\right)=g\left(u_{s+1}\right)=\varnothing$ and $g\left(x\right)=f\left(x\right)$ otherwise, is an 2RDF of $P_3\square P_n$ of weight $\omega \left(f\right)$, which is contradicting the choice of f. Therefore, $\left|S\right|=0$.

We can see that for every $0\le i\le n-2$, if $\omega \left(f_i\right)=\omega \left(f_{i+1}\right)=\omega \left(f_{i+2}\right)=1$, then $\omega \left(f_{i-1}\right),\omega \left(f_{i+3}\right)>1$. In addition, there is the function f such that, if $\omega \left(f_0\right)=1$ ($\omega \left(f_{n-1}\right)=1$ is similar), then $\omega \left(f_1\right)>1$ and $\omega \left(f_1\right)+\omega \left(f_2\right)+\omega \left(f_3\right)+\omega \left(f_4\right)\ge 6$ and if $\omega \left(f_0\right)=2$ ($\omega \left(f_{n-1}\right)=2$ is similar), then $\omega \left(f_0\right)+\omega \left(f_1\right)+\omega \left(f_2\right)+\omega \left(f_3\right)\ge 6$.

If $\omega \left(f_0\right)=1$ and $\omega \left(f_{n-1}\right)=1$, then

$$\begin{array}{ccc}\hfill 4\omega \left(f\right)& =& 4\sum _{0\le i\le n-1}\omega \left(f_i\right)\hfill \\ & =& [3\omega \left(f_0\right)+2\omega \left(f_1\right)+\omega \left(f_2\right)]+[3\omega \left(f_{n-1}\right)+2\omega \left(f_{n-2}\right)+\omega \left(f_{n-3}\right)]\hfill \\ & & +\sum _{i\in \{0,\dots ,n-4\}-\{1,n-5\}}\left(\omega \left(f_i\right)+\omega \left(f_{i+1}\right)+\omega \left(f_{i+2}\right)+\omega \left(f_{i+3}\right)\right)\hfill \\ & & +[\omega \left(f_1\right)+\omega \left(f_2\right)+\omega \left(f_3\right)+\omega \left(f_4\right)]+[\omega \left(f_{n-5}\right)+\omega \left(f_{n-4}\right)+\omega \left(f_{n-3}\right)+\omega \left(f_{n-2}\right)]\hfill \\ & \ge & 8+8+5(n-5)+12\hfill \\ & =& 5(n-3)+18.\hfill \end{array}$$

If $\omega \left(f_0\right)=1$ and $\omega \left(f_{n-1}\right)=2$, then

$$\begin{array}{ccc}\hfill 4\omega \left(f\right)& =& 4\sum _{0\le i\le n-1}\omega \left(f_i\right)\hfill \\ & =& [3\omega \left(f_0\right)+2\omega \left(f_1\right)+\omega \left(f_2\right)]+[3\omega \left(f_{n-1}\right)+2\omega \left(f_{n-2}\right)+\omega \left(f_{n-3}\right)]\hfill \\ & & +\sum _{i\in \{0,\dots ,n-4\}-\left\{1\right\}}\left(\omega \left(f_i\right)+\omega \left(f_{i+1}\right)+\omega \left(f_{i+2}\right)+\omega \left(f_{i+3}\right)\right)\hfill \\ & & +[\omega \left(f_1\right)+\omega \left(f_2\right)+\omega \left(f_3\right)+\omega \left(f_4\right)]\hfill \\ & \ge & 8+9+5(n-4)+6\hfill \\ & =& 5(n-3)+18.\hfill \end{array}$$

If $\omega \left(f_0\right)=2$ and $\omega \left(f_{n-1}\right)=2$, then

$$\begin{array}{ccc}\hfill 4\omega \left(f\right)& =& 4\sum _{0\le i\le n-1}\omega \left(f_i\right)\hfill \\ & =& [3\omega \left(f_0\right)+2\omega \left(f_1\right)+\omega \left(f_2\right)]+[3\omega \left(f_{n-1}\right)+2\omega \left(f_{n-2}\right)+\omega \left(f_{n-3}\right)]\hfill \\ & & +\sum _{i\in \{0,\dots ,n-4\}-\left\{1\right\}}[\omega \left(f_i\right)+\omega \left(f_{i+1}\right)+\omega \left(f_{i+2}\right)+\omega \left(f_{i+3}\right)]\hfill \\ & & +[\omega \left(f_1\right)+\omega \left(f_2\right)+\omega \left(f_3\right)+\omega \left(f_4\right)]\hfill \\ & \ge & 9+9+5(n-3)\hfill \\ & =& 5(n-3)+18.\hfill \end{array}$$

Thus, $\omega \left(f\right)=⌈\frac{5n+3}{4}⌉$. □

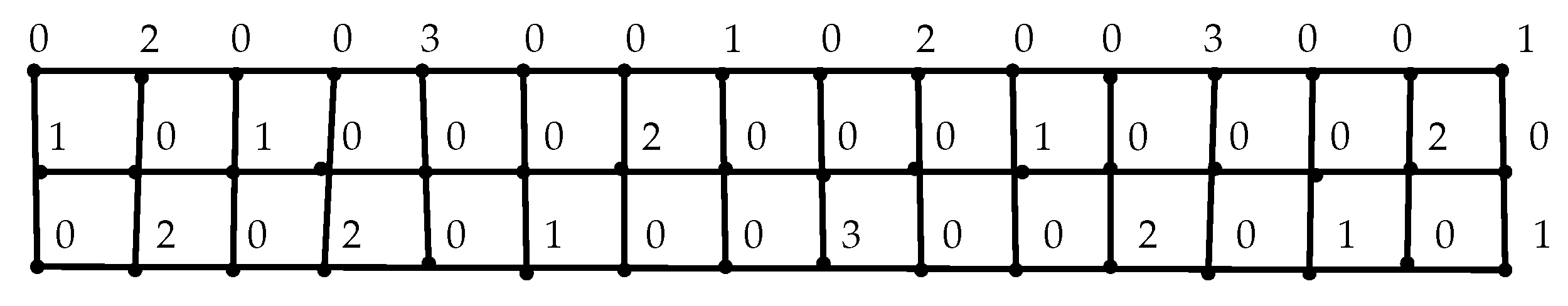
3. 3-Rainbow Domination Number of ${\mathit{P}}_{\mathbf{3}}\square {\mathit{P}}_{\mathit{n}}$

As in the previous section, a 3RDF is given in three lines and we use $0,1,2,3$ to encode the sets $\varnothing ,\left\{1\right\},\left\{2\right\},\left\{3\right\}$.

To provide a complete answer, we need the following fact.

Fact 2.

${\gamma }_{r3}(P_3\square P_3)=5,{\gamma }_{r3}(P_3\square P_4)=8.$

Theorem 2.

For $n\ge 5$,

$${\gamma }_{r3}(P_3\square P_n)=\left\{\begin{array}{ccc}(3n+1)/2& \mathrm{if}& n\equiv 1\left(\mathrm{mode}2\right),\hfill \\ (3n+2)/2& \mathrm{if}& n\equiv 0\left(\mathrm{mode}2\right),\hfill \end{array}\right.$$

Proof. 

First, we present constructions of a 3RDF of $P_3\square P_n$ of the desired weight.

• $n\equiv 0\left(\mathrm{mod}4\right)$:
  $2010\cdots 20102201$
  $0303\cdots 03030030$
  $1020\cdots 10201102$
• $n\equiv 1\left(\mathrm{mod}4\right)$:
  $2010\cdots 20102$
  $0303\cdots 03030$
  $1020\cdots 10201$
• $n\equiv 2\left(\mathrm{mod}4\right)$:
  $2010\cdots 2010201201$
  $0303\cdots 0303030030$
  $1020\cdots 1020102102$
• $n\equiv 3\left(\mathrm{mod}4\right)$:
  $2010\cdots 2010201$
  $0303\cdots 0303030$
  $1020\cdots 1020102$

To show that these are also lower bounds, we prove there is a ${\gamma }_{r3}(P_3\square P_n)$-function, f that satisfies the following conditions:

• For every $0\le i\le n-1$, $\omega \left(f_i\right)=|f\left(v_i\right)|+|f\left(u_i\right)|+|f\left(w_i\right)|\ge 1$,
• For every $1\le i\le n-2$, if $\omega \left(f_i\right)=1$, then $\omega \left(f_{i-1}\right)+\omega \left(f_{i+1}\right)\ge 4$. In particular, if $\omega \left(f_i\right)=1$, then $(\omega \left(f_{i-1}\right)+\omega \left(f_i\right))+(\omega \left(f_i\right)+\omega \left(f_{i+1}\right))\ge 6$,
• $\omega \left(f_0\right)\ge 2$ and $\omega \left(f_{n-1}\right)\ge 2$.

First, we show that for every ${\gamma }_{r3}(P_3\square P_n)$-function f, $\omega \left(f_i\right)=|f\left(v_i\right)|+|f\left(u_i\right)|+|f\left(w_i\right)|\ge 1$ when $0\le i\le n-1$. Let $n\ge 5$ and f be a ${\gamma }_{r3}(P_3\square P_n)$-function and $S=\{i\mid 0\le i\le n-1\mathrm{and}\omega \left(f_i\right)=0\}$. We claim that $\left|S\right|=0$. Assume to the contrary that $\left|S\right|\ge 1$. Then, we consider the following cases.

Case 1.

$0\in S$ (the case $n-1\in S$ is similar).

Then, we have $f\left(v_1\right)=f\left(u_1\right)=f\left(w_1\right)=\{1,2,3\}$ and it is easy to see that the function g defined by $g\left(v_0\right)=\left\{1\right\}$, $g\left(u_1\right)=\left\{3\right\}$, $g\left(w_0\right)=\left\{2\right\}$, $g\left(v_2\right)=f\left(v_2\right)\cup \left\{2\right\}$, $g\left(w_2\right)=f\left(w_2\right)\cup \left\{1\right\}$, $g\left(u_0\right)=g\left(v_1\right)=g\left(w_1\right)=\varnothing$ and $g\left(x\right)=f\left(x\right)$ otherwise, is an 3RDF of $P_3\square P_n$ of weight less than $\omega \left(f\right)$, which is a contradiction.

Let s be the smallest positive integer for which $\omega \left(f_s\right)=0$. Then, $s\ge 1$ and $\omega \left(f_{s-1}\right)+\omega \left(f_{s+1}\right)\ge 9$.

Case 2.

$s=1$ ($s=n-2$ is similar).

Then, the function g defined by $g\left(v_0\right)=g\left(u_0\right)=g\left(w_0\right)=\left\{1\right\}$, $g\left(v_1\right)=\left\{2\right\}$, $g\left(w_1\right)=\left\{1\right\}$, $g\left(u_2\right)=\left\{3\right\}$, $g\left(v_3\right)=f\left(v_3\right)\cup \left\{1\right\}$, $g\left(w_3\right)=f\left(w_3\right)\cup \left\{2\right\}$, $g\left(u_1\right)=g\left(v_2\right)=g\left(w_2\right)=\varnothing$ and $g\left(x\right)=f\left(x\right)$ otherwise, is an 3RDF of $P_3\square P_n$ of weight less than $\omega \left(f\right)$, which is a contradiction.

Case 3.

$2\le s\le n-3$.

The function g defined by $g\left(u_{s-1}\right)=g\left(u_{s+1}\right)=\left\{3\right\}$, $g\left(v_s\right)=\left\{2\right\}$, $g\left(w_s\right)=\left\{1\right\}$, $g\left(v_{s-2}\right)=f\left(v_{s-2}\right)\cup \left\{1\right\}$, $g\left(w_{s-2}\right)=f\left(w_{s-2}\right)\cup \left\{2\right\}$, $g\left(v_{s+2}\right)=f\left(v_{s+2}\right)\cup \left\{1\right\}$, $g\left(w_{s+2}\right)=f\left(w_{s+2}\right)\cup \left\{2\right\}$, $g\left(v_{s-1}\right)=g\left(v_{s+1}\right)=g\left(u_s\right)=g\left(w_{s-1}\right)=g\left(w_{s+1}\right)=\varnothing$ and $g\left(x\right)=f\left(x\right)$ otherwise, is an 3RDF of $P_3\square P_n$ of weight less than $\omega \left(f\right)$, which is a contradiction. Therefore, $\left|S\right|=0$.

Now, let f be a ${\gamma }_{r3}(P_3\square P_n)$-function. It is easy to see that, if $\omega \left(f_i\right)=1$, then $\omega \left(f_{i-1}\right)+\omega \left(f_{i+1}\right)\ge 4$ when $1\le i\le n-2$.

Finally, we show that there is ${\gamma }_{r3}(P_3\square P_n)$-function f such that $\omega \left(f_0\right)\ge 2$ ( $\omega \left(f_{n-1}\right)\ge 2$ is similar). Let f be a ${\gamma }_{r3}(P_3\square P_n)$-function such that $\omega \left(f_0\right)=1$. If $\left|f\right(v_0\left)\right|=1$ ($\left|f\right(w_0\left)\right|=1$ is similar), then $|f\left(w_0\right)|=|f\left(u_0\right)|=0$, $\left|f\right(u_1\left)\right|\ge 2$ and $\left|f\right(w_1\left)\right|=3$. We may assume that $\{1,2\}\subseteq f\left(u_1\right)$. It is easy to see that the function g defined by $g\left(w_0\right)=\left\{3\right\}$, $g\left(w_2\right)=\left\{3\right\}$, $g\left(w_1\right)=\varnothing$ and $g\left(x\right)=f\left(x\right)$ otherwise, is an 3RDF of $P_3\square P_n$ of weight less than $\omega \left(f\right)$, which is a contradiction. Now, let $\left|f\right(u_0\left)\right|=1$. Then, $|f\left(w_0\right)|=|f\left(v_0\right)|=0$, $\left|f\right(v_1\left)\right|\ge 2$ and $\left|f\right(w_1\left)\right|\ge 2$. It is easy to see that the function g defined by $g\left(w_0\right)=\left\{1\right\}$, $g\left(w_2\right)=\left\{2\right\}$, $g\left(u_1\right)=\left\{3\right\}$, $g\left(v_2\right)=f\left(v_2\right)\cup \left\{1\right\}$, $g\left(w_2\right)=f\left(w_2\right)\cup \left\{2\right\}$, $g\left(u_1\right)=g\left(u_2\right)=\varnothing$ and $g\left(x\right)=f\left(x\right)$ otherwise, is an 3RDF of $P_3\square P_n$ of weight $\omega \left(f\right)$.

Hence, there is a ${\gamma }_{r3}(P_3\square P_n)$-function, f that satisfies the following conditions:

• For every $0\le i\le n-1$, $\omega \left(f_i\right)\ge 1$;
• For every $1\le i\le n-2$, if $\omega \left(f_i\right)=1$, then $\omega \left(f_{i-1}\right)+\omega \left(f_{i+1}\right)\ge 4$; and
• $\omega \left(f_0\right)\ge 2$ and $\omega \left(f_{n-1}\right)\ge 2$.

If n is odd, then

$$\begin{array}{ccc}\hfill 2\omega \left(f\right)& =& 2\sum _{0\le i\le n-1}\omega \left(f_i\right)\hfill \\ & =& \omega \left(f_0\right)+\omega \left(f_{n-1}\right)+\sum _{0\le i\le n-2}(\omega \left(f_i\right)+\omega \left(f_{i+1}\right))\hfill \\ & \ge & 4+3(n-1).\hfill \end{array}$$

Then, $\omega \left(f\right)=\frac{3n+1}{2}$ when n is odd. Now, let n is even. Then, there is $s\ne n-1$ such that $\omega \left(f_s\right)+\omega \left(f_{s+1}\right)\ge 4$. Hence,

$$\begin{array}{ccc}\hfill 2\omega \left(f\right)& =& 2\sum _{0\le i\le n-1}\omega \left(f_i\right)\hfill \\ & =& \omega \left(f_s\right)+\omega \left(f_{s+1}\right)+\omega \left(f_0\right)+\omega \left(f_{n-1}\right)+\sum _{0\le i\le n-2,i\ne s}(\omega \left(f_i\right)+\omega \left(f_{i+1}\right))\hfill \\ & \ge & 8+3(n-2).\hfill \end{array}$$

Therefore, $\omega \left(f\right)=\frac{3n+2}{2}$ when n is even. □

4. 4-Rainbow Domination Number of ${\mathit{P}}_{\mathbf{3}}\square {\mathit{P}}_{\mathit{n}}$

As above, a 4RDF is given in three lines and we use $0,1,2,5$ to encode the sets $\varnothing ,\left\{1\right\},\left\{2\right\},\{3,4\}$.

To provide a complete answer, we need the following fact.

Fact 3.

${\gamma }_{r4}(P_3\square P_3)=6,{\gamma }_{r4}(P_3\square P_4)=9.$

Theorem 3.

For $n\ge 5$, ${\gamma }_{r4}(P_3\square P_n)=2n$.

Proof. 

First, we show that ${\gamma }_{r4}(P_3\square P_n)\le 2n$. To do this, we present constructions of a 4RDF of $P_3\square P_n$ of the desired weight.

• $n\equiv 0\left(\mathrm{mod}4\right)$:
  $2010\cdots 20102201$
  $0505\cdots 05050050$
  $1020\cdots 10201102$
• $n\equiv 1\left(\mathrm{mod}4\right)$:
  $2010\cdots 20102$
  $0505\cdots 05050$
  $1020\cdots 10201$
• $n\equiv 2\left(\mathrm{mod}4\right)$:
  $2010\cdots 2010201201$
  $0505\cdots 0505050050$
  $1020\cdots 1020102102$
• $n\equiv 3\left(\mathrm{mod}4\right)$:
  $2010\cdots 2010201$
  $0505\cdots 0505050$
  $1020\cdots 1020102$

To prove the inverse inequality, we show that every ${\gamma }_{r4}(P_3\square P_n)$-function f satisfies the following conditions:

• For every $0\le i\le n-1$, $\omega \left(f_i\right)=|f\left(v_i\right)|+|f\left(u_i\right)|+|f\left(w_i\right)|\ge 1$;
• For every $1\le i\le n-2$, if $\omega \left(f_i\right)=1$, then $\omega \left(f_{i-1}\right)+\omega \left(f_{i+1}\right)\ge 6$; and
• $\omega \left(f_0\right)\ge 2$ and $\omega \left(f_{n-1}\right)\ge 2$.

First, we show that for every ${\gamma }_{r4}(P_3\square P_n)$-function f, $\omega \left(f_i\right)=|f\left(v_i\right)|+|f\left(u_i\right)|+|f\left(w_i\right)|\ge 1$ when $0\le i\le n-1$. Let $n\ge 5$ and f be a ${\gamma }_{r4}(P_3\square P_n)$-function and $S=\{i\mid 0\le i\le n-1\mathrm{and}\omega \left(f_i\right)=0\}$. We claim that $\left|S\right|=0$. Assume to the contrary that $\left|S\right|\ge 1$. Then, we consider the following cases.

Case 1.

$0\in S$ (the case $n-1\in S$ is similar).

Then, we have $f\left(v_1\right)=f\left(u_1\right)=f\left(w_1\right)=\{1,2,3,4\}$ and the function g defined by $g\left(v_0\right)=\left\{1\right\}$, $g\left(u_1\right)=\{3,4\}$, $g\left(w_0\right)=\left\{2\right\}$, $g\left(v_2\right)=f\left(v_2\right)\cup \left\{2\right\}$, $g\left(w_2\right)=f\left(w_2\right)\cup \left\{1\right\}$, $g\left(u_0\right)=g\left(v_1\right)=g\left(w_1\right)=\varnothing$ and $g\left(x\right)=f\left(x\right)$ otherwise, is an 4RDF of $P_3\square P_n$ of weight less than $\omega \left(f\right)$, which is a contradiction.

Let $\omega \left(f_s\right)=0$. Then, $s\ge 1$ and $\omega \left(f_{s-1}\right)+\omega \left(f_{s+1}\right)\ge 12$.

Case 2.

$s=1$ ($s=n-2$ is similar).

The function g defined by $g\left(v_0\right)=g\left(u_0\right)=g\left(w_0\right)=\left\{1\right\}$, $g\left(v_1\right)=\left\{2\right\}$, $g\left(w_1\right)=\left\{1\right\}$, $g\left(u_2\right)=\{3,4\}$, $g\left(v_3\right)=f\left(v_3\right)\cup \left\{1\right\}$, $g\left(w_3\right)=f\left(w_3\right)\cup \left\{2\right\}$, $g\left(u_1\right)=g\left(v_2\right)=g\left(w_2\right)=\varnothing$ and $g\left(x\right)=f\left(x\right)$ otherwise, is an 4RDF of $P_3\square P_n$ of weight less than $\omega \left(f\right)$, which is a contradiction.

Case 3.

$2\le s\le n-3$.

Then, it is easy to see that the function g defined by $g\left(u_{s-1}\right)=g\left(u_{s+1}\right)=\{3,4\}$, $g\left(v_s\right)=\left\{2\right\}$, $g\left(w_s\right)=\left\{1\right\}$, $g\left(v_{s-2}\right)=f\left(v_{s-2}\right)\cup \left\{1\right\}$, $g\left(w_{s-2}\right)=f\left(w_{s-2}\right)\cup \left\{2\right\}$, $g\left(v_{s+2}\right)=f\left(v_{s+2}\right)\cup \left\{1\right\}$, $g\left(w_{s+2}\right)=f\left(w_{s+2}\right)\cup \left\{2\right\}$, $g\left(v_{s-1}\right)=g\left(v_{s+1}\right)=g\left(u_s\right)=g\left(w_{s-1}\right)=g\left(w_{s+1}\right)=\varnothing$ and $g\left(x\right)=f\left(x\right)$ otherwise, is an 4RDF of $P_3\square P_n$ of weight less than $\omega \left(f\right)$, which is a contradiction. Therefore, $\left|S\right|=0$.

Now, let f be a ${\gamma }_{r4}(P_3\square P_n)$-function. It is easy to see that, if $\omega \left(f_i\right)=1$, then $\omega \left(f_{i-1}\right)+\omega \left(f_{i+1}\right)\ge 6$ when $1\le i\le n-2$.

We show that for every ${\gamma }_{r4}(P_3\square P_n)$-function f $\omega \left(f_0\right)\ge 2$ ($\omega \left(f_{n-1}\right)\ge 2$ is similar). Let f be a ${\gamma }_{r4}(P_3\square P_n)$-function such that $\omega \left(f_0\right)=1$. If $\left|f\right(v_0\left)\right|=1$ ($\left|f\right(w_0\left)\right|=1$ is similar), then $|f\left(w_0\right)|=|f\left(u_0\right)|=0$, $\left|f\right(u_1\left)\right|\ge 3$ and $\left|f\right(w_1\left)\right|=4$. We may assume that $\{1,2,3\}\subseteq f\left(u_1\right)$. The function g defined by $g\left(w_0\right)=\left\{4\right\}$, $g\left(w_2\right)=\left\{4\right\}$, $g\left(w_1\right)=\varnothing$ and $g\left(x\right)=f\left(x\right)$ otherwise, is an 4RDF of $P_3\square P_n$ of weight less than $\omega \left(f\right)$, which is a contradiction. Now, let $\left|f\right(u_0\left)\right|=1$. Then, $|f\left(w_0\right)|=|f\left(v_0\right)|=0$, $\left|f\right(v_1\left)\right|\ge 3$ and $\left|f\right(w_1\left)\right|\ge 3$. The function g defined by $g\left(w_0\right)=\left\{1\right\}$, $g\left(w_2\right)=\left\{2\right\}$, $g\left(u_1\right)=\{3,4\}$, $g\left(v_2\right)=f\left(v_2\right)\cup \left\{1\right\}$, $g\left(w_2\right)=f\left(w_2\right)\cup \left\{2\right\}$, $g\left(u_1\right)=g\left(u_2\right)=\varnothing$ and $g\left(x\right)=f\left(x\right)$ otherwise, is an 4RDF of $P_3\square P_n$ of weight less than $\omega \left(f\right)$, which is a contradiction.

Hence, every ${\gamma }_{r4}(P_3\square P_n)$-function f satisfies the following conditions:

• For every $0\le i\le n-1$, $\omega \left(f_i\right)\ge 1$;
• For every $1\le i\le n-2$, if $\omega \left(f_i\right)=1$, then $\omega \left(f_{i-1}\right)+\omega \left(f_{i+1}\right)\ge 6$. In particular $(\omega \left(f_{i-1}\right)+\omega \left(f_i\right))+(\omega \left(f_i\right)+\omega \left(f_{i+1}\right))\ge 8$; and
• $\omega \left(f_0\right)\ge 2$ and $\omega \left(f_{n-1}\right)\ge 2$.

Hence,

$$\begin{array}{ccc}\hfill 2\omega \left(f\right)& =& 2\sum _{0\le i\le n-1}\omega \left(f_i\right)\hfill \\ & =& \sum _{0\le i\le n-2}(\omega \left(f_i\right)+\omega \left(f_{i+1}\right))+\omega \left(f_0\right)+\omega \left(f_{n-1}\right)\hfill \\ & \ge & 4(n-1)+4.\hfill \end{array}$$

Hence, $\omega \left(f\right)=2n$. □