Precision Fixed-Time Formation Control for Multi-AUV Systems with Full State Constraints

Abstract

The trajectory tracking the control of autonomous underwater vehicle (AUV) systems faces considerable challenges due to strong inter-axis coupling and complex time-varying external disturbances. This paper proposes a novel fixed-time control scheme incorporating a switching threshold-based event-driven strategy to address critical issues in multi-AUV formation control, including full-state constraints, unmeasurable states, model uncertainties, limited communication resources, and unknown time-varying disturbances. A rapid and stable dimensional augmented state observer (RSDASO) was first developed to achieve fixed-time convergence in estimating aggregated disturbances and unmeasurable states. Subsequently, a logarithmic barrier Lyapunov function was constructed to derive a fixed-time control law that guarantees bounded system errors within a predefined interval while strictly confining all states to specified constraints. The introduction of a switching threshold event-triggering mechanism (ETM) significantly reduced communication resource consumption. The simulation results demonstrate the effectiveness of the proposed method in improving control accuracy while substantially lowering communication overhead.

1. Introduction

Recently, the exploitation of marine resources has become the focus of global attention [1,2]. As an emerging tool for ocean exploration, autonomous underwater vehicles (AUVs) play an important role in many fields. With the continuous development of science and technology, AUVs not only have a wide range of uses such as for seabed survey, nautical charting, underwater archaeology, rescue, etc., it can also facilitate underwater diving and recreational programs [3,4,5,6]. The realization of the above applications relies on the autonomous motion control capability of AUV systems in marine environments, which is therefore a current research hotspot. The complex nonlinear dynamics of AUV systems, such as the strong coupling between model variables, the uncertainty of physical parameter variations, and the unknown complexity of external time-varying perturbations, make the trajectory tracking control of AUVs a challenging task [7,8].

To solve the above problems, scholars have proposed many control strategies. For example, neural network control and designed disturbance observers are more widely used in dealing with the problem of external disturbances and model uncertainty [9,10]. The authors in [11,12] utilized RBF neural networks to approximate the unknown term. The authors in [13] proposed a neural network weight adjustment method based on the neural network approach containing a saturation factor, which overcomes the effects of the uncertainty of the dynamic model on the modeling and control of the system. A perturbation observer was constructed in [14] to estimate the unknown time-varying perturbations present in the system, and the dynamic surface was further applied to design a robust time-varying formation control law. Wang et al. estimated the composite disturbance acting on an AUV by designing a nonlinear disturbance observer [15]. However, the above studies could only make the error converge asymptotically, that is, when the time tended to infinity, the system error could converge, which not ideal in attempting to meet the requirements of practical applications [16].

Compared with the above asymptotically stabilized systems, finite-time stabilized systems usually converge faster, so research on underwater robot control based on finite-time control theory was developed [17]. In [18], the authors used a finite-time control technique to design a new type of perturbation observer for high-order nonlinear systems, which realized the accurate estimation of external perturbations. However, the convergence time of a finite-time stabilized system is related to the initial value of the system, which somewhat diminishes the applicability of this scheme. With the development of fixed-time theory [19], one can make the tracking error of the system converge in a fixed time, which improves the convergence speed of the system, and the convergence time of the system is independent of the initial state. Wan et al. in [20] designed a fixed-time perturbation observer using an auxiliary system to accurately estimate the composite perturbation, taking into account the external perturbation and the model parameter invariance.

With the upgrading of the demand for marine operations, the execution capability of a single underwater robot is limited, and it is more difficult to complete complex tasks independently. In addition, the complexity of the operating environment of underwater robots often requires multiple AUVs to cooperate with each other and work in synergy in order to improve operational efficiency [21]. Therefore, the study of the formation control of multiple AUVs is of great significance and value for practical engineering applications. In [22], researchers applied adaptive neural networks to deal with uncertain dynamics and environmental perturbations in the system and combined this with the backstepping method to propose a 3D formation control strategy for AUV neural networks. In [23], the authors constructed a prediction-based fuzzy state observer to estimate the unknown dynamics and marine environment perturbations in AUV systems and proposed a multi-AUV formation control law by combining line-of-sight and backstepping methods.

However, the above studies are all based on the time triggering mechanism, which needs to update the control signals continuously, which may lead to problems of occupying system communication resources, increasing the pressure on system communication, and the waste of communication resources. Different from the traditional time-triggering mechanism, the event-triggering mechanism enables the system to update the control signal only when the triggering conditions are met, which solves the problem of resource waste well. This gives ETM a wide range of application prospects in networked control systems [24]. Deng Y et al. in [25] investigated the path tracking control of underdriven underwater robots with an event-triggering mechanism. However, none of them considered the problem that the state of the AUV system is constrained and that the speed of the AUV is often not accurately obtainable in practical engineering environments.

Following on from the above, this study takes the formation control of multi-AUVs as the research background and proposes a fixed-time control method based on the triggering mechanism of switching threshold events, considering the existence of a system with full-state constraints, unmeasurable speed, unknown external time-varying interference, and limited communication resources. The designed control method can keep the system in formation configuration, and the tracking error converges within a fixed time. This paper makes the following key contributions:

• The developed RSDASO possesses a streamlined architecture with minimal parametric requirements, enabling the precise fixed-time estimation of both composite disturbances and unmeasurable system states.
• The full-state constraints are satisfied by constructing logarithmic barrier Lyapunov functions. The developed switching threshold event-triggering mechanism further reduces the communication resource consumption of the system.

The structure of this article is outlined below. Section 2 introduces the 3D AUV model, including relevant terminology and preliminaries. Section 3 presents the construction of a rapid and stable observer, the follower control strategy is described, and the stability of the system is verified. The feasibility of the control strategies was verified through simulation experiments described in Section 4. In conclusion, Section 5 presents the findings of this study.

2. Notations and Preliminaries

2.1. Notations

• Denoting $a={[a_1,a_2,\dots ,a_n]}^{\mathrm{T}}\in {\mathbb{R}}^n$ and $si{g}^x\left(a\right)={[si{g}^x\left(a_1\right),si{g}^x\left(a_2\right),\dots ,si{g}^x\left(a_n\right)]}^{\mathrm{T}}$, where $si{g}^x\left(a_i\right)={\left|a_i\right|}^x\mathrm{sgn}\left(a_i\right)$, $(i=1,2,\dots ,n)$. $\mathrm{sgn}\left(a\right)$, is denoted as

  $$\begin{array}{cccc}& & \hfill \mathrm{sgn}\left(a\right)=\left\{\begin{array}{cc}\hfill 1,& a>0\hfill \\ \hfill 0,& a=0\hfill \\ \hfill -1,& a<0\hfill \end{array}\right.& \end{array}$$

  (1)
• Let ${\lambda }_{max}\left\{X\right\}$ and ${\lambda }_{min}\left\{X\right\}$ represent the maximum and minimum singular values of matrix X, respectively.
• Considering a given vector $x={[x_1,x_2,\dots ,x_n]}^{\mathrm{T}}$, $x^{\alpha }$ is defined as $x={[x_1,x_2,\dots ,x_n]}^{\mathrm{T}}$.

2.2. Preliminaries

Lemma 1

([26]). Consider the nonlinear dynamical system described by

$$\begin{array}{cccc}& & \hfill \dot{r}=f\left(r\left(t\right)\right),& \end{array}$$

(2)

where $r\in {\mathbb{R}}^n$ denotes the state vector and $f\left(r\right(t\left)\right)$ characterizes the nonlinear dynamics. A sufficient condition for the origin of system (2) to be practically fixed-time stable is the existence of a positive definite function $V\left(r\right)$ satisfying the following inequality:

$$\begin{array}{cccc}& & \hfill \dot{V}\left(r\right)\le -{\lambda }_1{V}^x\left(r\right)-{\lambda }_2{V}^y\left(r\right)+l& \end{array}$$

(3)

with ${\lambda }_1>0$, ${\lambda }_2>0$, $l>0$, $x>1$ and $0<y<1$. The upper bound of convergence time T is determined by the following inequality:

$$\begin{array}{cccc}& & \hfill T\le T_{max}={\displaystyle \frac{1}{{\lambda }_1\omega \left(x-1\right)}}+{\displaystyle \frac{1}{{\lambda }_2\omega \left(1-y\right)}}& \end{array}$$

(4)

where $0<\omega <1$. The system’s solution can converge to the following residual set:

$$\begin{array}{cccc}& & \hfill \Theta =\left\{r\left|V\left(r\right)\le min\left\{{\left({\displaystyle \frac{l}{(1-\omega ){\lambda }_1}}\right)}^{{\displaystyle \frac{1}{x}}},{\left({\displaystyle \frac{l}{(1-\omega ){\lambda }_2}}\right)}^{{\displaystyle \frac{1}{y}}}\right\}\right.\right\}& \end{array}$$

(5)

Lemma 2

([27]). For ∀$x_i>0$, $i=1,2,\dots ,n$, the following inequality is satisfied:

$$\begin{array}{cc}\hfill \left\{\begin{array}{c}{\left(\sum _{i=1}^n{y}_i\right)}^k⩽\sum _{i=1}^n{y}_i^k,0<k<1\hfill \\ {\left(\sum _{i=1}^n{y}_i\right)}^k⩽{\displaystyle \frac{1}{n^{1-k}}}\sum _{i=1}^n{y}_i^k,1<k<\infty .\hfill \end{array}\right.& \end{array}$$

(6)

Lemma 3

([28,29]). For all real numbers $x\in R$ and positive constants $y>0$, the following inequalities are satisfied:

$$\begin{array}{cccc}& & \hfill -xtanh\left({\displaystyle \frac{x}{y}}\right)⩽0,& \end{array}$$

(7)

$$\begin{array}{cccc}& & \hfill 0⩽\left|x\right|-xtanh\left({\displaystyle \frac{x}{y}}\right)⩽0.2785y.& \end{array}$$

(8)

Lemma 4

([30]). For any constant a, when the condition $\left|b\right|<a$ holds, the following inequality is satisfied:

$$\begin{array}{cccc}& & \hfill ln{\displaystyle \frac{a^2}{a^2-b^2}}⩽{\displaystyle \frac{b^2}{a^2-b^2}}.& \end{array}$$

(9)

2.3. Problem Formulation and Analysis

The coupled dynamics and kinematics of the 5-DOF AUV system are governed by the following nonlinear equations [31]:

$$\begin{array}{cc}\hfill \left\{\begin{array}{c}\dot{\mathit{x}}=\mathit{v}\hfill \\ \dot{\mathit{v}}=R{M}^{-1}\mathit{u}+\overline{\mathit{\psi }}\hfill \\ \overline{\mathit{\psi }}=\dot{R}\mathit{\sigma }+R{M}^{-1}(-C\mathit{\sigma }-D\mathit{\sigma }+\mathit{\psi }-g),\hfill \end{array}\right.& \end{array}$$

(10)

with $\mathit{x}={[x_1,x_2,x_3,x_4,x_5]}^{\mathrm{T}}\in {\mathbb{R}}^5$, denoting the generalized pose vector. The velocity vector in the body-fixed frame is defined as $\mathit{v}={[v_1,v_2,v_3,v_4,v_5]}^{\mathrm{T}}\in {\mathbb{R}}^5$, where $\mathit{\sigma }={[{\sigma }_1,{\sigma }_2,{\sigma }_3,{\sigma }_4,{\sigma }_5]}^{\mathrm{T}}\in {\mathbb{R}}^5$ comprises both linear and angular velocity components. The control input vector $\mathit{u}={[u_1,u_2,u_3,u_4,u_5]}^{\mathrm{T}}$ and time-varying disturbance vector $\mathit{\psi }={[{\psi }_1,{\psi }_2,{\psi }_3,{\psi }_4,{\psi }_5]}^{\mathrm{T}}$ represent the actuation forces and external perturbations, respectively. The restoring force vector is denoted by $\mathit{g}={[g_1,g_2,g_3,g_4,g_5]}^{\mathrm{T}}$. The transformation between the body frame and Earth frame is governed by the rotation matrix $R\left(\mathit{x}\right)$ The system inertia matrix M, Coriolis–centripetal matrix C, and damping matrix D complete the dynamic description (for detailed matrix structures, see [31]). The term $\overline{\mathit{\psi }}$ aggregates all unmodeled disturbances and parametric uncertainties.

Assumption A1

([32]). The combined effects of external disturbances and model uncertainties $\overline{\mathit{\psi }}$ admit a known variation bound: $\parallel \dot{\overline{\mathit{\psi }}}\parallel \le E$, where E quantifies the maximum energy rate of the disturbance input.

Assumption A2

([33]). The coupled Coriolis and damping terms (C and D) are unknown but bounded nonlinear functions of velocity. Furthermore, the velocity vector v remains unmeasurable in practice, necessitating state estimation techniques.

Remark 1.

Assumptions 1 and 2 align with standard practice in the marine vehicle control literature, as demonstrated in [20,34,35]. These premises are justified through both theoretical analysis and experimental validation in underwater robotic systems.

3. Lyapunov-Based Control Design and Stability Assessment

3.1. Construction of the RSDASO

To reconstruct the full state vector and lumped disturbances, we develop an accelerated convergent extended state observer with the following structure:

$$\begin{array}{cccc}& & \hfill \left\{\begin{array}{c}\dot{\widehat{\mathit{x}}}=\widehat{\mathit{v}}+{\mu }_{1}si{g}^{a_1}\left(\tilde{\mathit{x}}\right)+{ϵ}_{1}si{g}^{b_1}\left(\tilde{\mathit{x}}\right)\hfill \\ \dot{\widehat{\mathit{v}}}=\widehat{\overline{\mathit{\psi }}}+R{M}^{-1}\mathit{u}+{\mu }_{2}si{g}^{a_2}\left(\tilde{\mathit{x}}\right)+{ϵ}_{2}si{g}^{b_2}\left(\tilde{\mathit{x}}\right)\hfill \\ \dot{\widehat{\overline{\mathit{\psi }}}}={\mu }_{3}si{g}^{a_3}\left(\tilde{\mathit{x}}\right)+{ϵ}_{3}si{g}^{b_3}\left(\tilde{\mathit{x}}\right)+\gamma \mathrm{sgn}\left(\tilde{\mathit{x}}\right)\hfill \end{array}\right.& \end{array}$$

(11)

where $\widehat{\mathit{x}}$, $\widehat{\mathit{v}}$, and $\widehat{\overline{\mathit{\psi }}}$ represent the estimated values of $\mathit{x}$, $\mathit{v}$, and $\overline{\mathit{\psi }}$, respectively. The observer parameters are constrained as $a_i\in (0,1)$, $b_i>1$, $a_i=i\overline{a}-(i-1)$, $b_i=i{b}_i-(i-1)$, $i=1,2,3$; $\overline{a}\in (1-l_1,1)$, $\overline{b}\in (1,1+l_2)$, with ${\chi }_1>0$, ${\chi }_2>0$ being sufficiently small positive constants. The aggregate disturbance bound satisfies $E<\gamma$. The gain selection of RSDASO ensures the Hurwitz property of the following characteristic matrices:

$$\begin{array}{cccc}& & \hfill N_1=\left[\begin{array}{ccc}-{\mu }_1& 1& 0\\ -{\mu }_2& 0& 1\\ -{\mu }_3& 0& 0\end{array}\right],& \end{array}$$

(12)

$$\begin{array}{cccc}& & \hfill N_2=\left[\begin{array}{ccc}-{ϵ}_1& 1& 0\\ -{ϵ}_2& 0& 1\\ -{ϵ}_3& 0& 0\end{array}\right],& \end{array}$$

(13)

Theorem 1.

Under Assumptions 1 and 2, the proposed Rapid and Stable Dimensional Augmented State Observer (RSDASO) guarantees asymptotically exact estimation of the full state vector $\mathit{x}$, $\mathit{v}$, and aggregated disturbances $\overline{\mathit{\psi }}$. Moreover, the estimation errors exhibit fixed-time convergence to zero, with the convergence time upper-bounded by

$$\begin{array}{cccc}& & \hfill T_1\le {\displaystyle \frac{{\lambda }_{max}^{\vartheta }\left({\Xi }_1\right)}{{\chi }_1\vartheta }}+{\displaystyle \frac{1}{{\chi }_2\sigma {\omega }^{\sigma }}},& \end{array}$$

(14)

where ${\chi }_1={\lambda }_{min}\left(Q_1\right)/{\lambda }_{min}\left({\Xi }_1\right)$, ${\chi }_2={\lambda }_{min}\left(Q_2\right)/{\lambda }_{min}\left({\Xi }_2\right)$, $\vartheta =1-\overline{a}$, $\sigma =\overline{\beta }-1$. Let ω be a positive constant satisfying $\omega \le {\lambda }_{min}\left({\Xi }_2\right)$, where $Q_1,Q_2,{\Xi }_1$, and ${\Xi }_2$ are symmetric positive definite matrices with full rank. These matrices are constrained by the following Lyapunov equations: ${\Xi }_1{N}_1+N_1^{\mathrm{T}}{\Xi }_1=-Q_1$, ${\Xi }_2{N}_2+N_2^{\mathrm{T}}{\Xi }_2=-Q_2$.

Proof of Theorem 1. 

Define the state estimation errors of the RSDASO as follows:

$$\begin{array}{cccc}& & \hfill \left\{\begin{array}{c}\tilde{\mathit{x}}=\mathit{x}-\widehat{\mathit{x}}\hfill \\ \tilde{\mathit{v}}=\mathit{v}-\widehat{\mathit{v}}\hfill \\ \widetilde{\overline{\mathit{\psi }}}=\overline{\mathit{\psi }}-\widehat{\overline{\mathit{\psi }}}\hfill \end{array}\right.& \end{array}$$

(15)

The time derivative of the Lyapunov function candidate (11) yields

$$\begin{array}{cccc}& & \hfill \left\{\begin{array}{c}\dot{\tilde{\mathit{x}}}=\tilde{\mathit{v}}-{\mu }_{1}si{g}^{a_1}\left(\tilde{\mathit{x}}\right)-{ϵ}_{1}si{g}^{b_1}\left(\tilde{\mathit{x}}\right)\hfill \\ \dot{\tilde{\mathit{v}}}=\widetilde{\overline{\mathit{\psi }}}-{\mu }_{2}si{g}^{a_2}\left(\tilde{\mathit{x}}\right)-{ϵ}_{2}si{g}^{b_2}\left(\tilde{\mathit{x}}\right)\hfill \\ \dot{\widetilde{\overline{\mathit{\psi }}}}=\dot{\overline{\mathit{\psi }}}-{\mu }_{3}si{g}^{a_3}\left(\tilde{\mathit{x}}\right)-{ϵ}_{3}si{g}^{b_3}\left(\tilde{\mathit{x}}\right)-\gamma \mathrm{sgn}\left(\tilde{\mathit{x}}\right)\hfill \end{array}\right.& \end{array}$$

(16)

Following Theorem 2 in [36], the observer estimation error (15) exhibits fixed-time convergence to zero within settling time $T_1$, i.e.,

$$\begin{array}{cccc}& & \hfill \forall t\ge T_1:\parallel \tilde{\mathit{x}}\left(t\right)\parallel =\parallel \tilde{\mathit{v}}\left(t\right)\parallel =\parallel \tilde{\mathit{\psi }}\left(t\right)\parallel =0& \end{array}$$

(17)

This completes the proof of Theorem 1 regarding the closed-loop system’s fixed-time stability.    □

3.2. Lyapunov-Based Fixed-Time Controller Design for Follower AUV Formation

The system tracking error is formally defined as

$$\begin{array}{cccc}& & \hfill \left\{\begin{array}{c}{\mathit{e}}_{i,1}={\mathit{x}}_i-\mathit{x}+P_{i,1}\hfill \\ {\mathit{e}}_{i,2}={\widehat{\mathit{v}}}_i-{\mathit{v}}_{i,d}\hfill \end{array}\right.& \end{array}$$

(18)

Define the tracking error vectors for the ith follower AUV as ${\mathit{e}}_{i,1}={[e_{i,11},e_{i,12},e_{i,13},e_{i,14},e_{i,15}]}^{\mathrm{T}}$, ${\mathit{e}}_{i,2}={[e_{i,21},e_{i,22},\dots ,e_{i,25}]}^{\mathrm{T}}$, where ${\mathit{v}}_{i,d}={[v_{i,d1},v_{i,d2},\dots ,v_{i,d5}]}^{\mathrm{T}}$ represents the virtual control law to be designed. Let $\mathit{x}\in {\mathbb{R}}^5$ denote the virtual leader’s reference trajectory, where the subscript $i\in \{1,\dots ,N\}$ identifies the $i^{\mathrm{th}}$ follower AUV in the formation. The relative formation configuration is defined by the position offset vector $P_{i,1}\in {\mathbb{R}}^5$, which specifies the desired spatial relationship between the ith follower and the virtual leader.

The virtual control law for the $i^{\mathrm{th}}$ AUV is designed as

$$\begin{array}{cccc}& & \hfill v_{i,dj}={\dot{x}}_j-{\displaystyle \frac{b_{i,1j}{e}_{i,1j}^{\beta }}{{({\kappa }_{i,1j}^2-e_{i,1j}^2)}^{\frac{\beta -1}{2}}}}-{\displaystyle \frac{c_{i,1j}{e}_{i,1j}^{\alpha }}{{({\kappa }_{i,1j}^2-e_{i,1j}^2)}^{\frac{\alpha -1}{2}}}}& \end{array}$$

(19)

The controller parameters are constrained as follows: $b_{i,1j}>0$, $c_{i,1j}>0$, $\beta >1$, and $0<\alpha <1$. ${\kappa }_{i,1j}$ is the bounding boundary of the error and $j\in \{1,\dots ,5\}$ indexes the degree of freedom.

A switching threshold-based event-triggering mechanism is proposed to regulate communication transmission:

$$\begin{array}{cccc}& & \hfill t_{i,j,k+1}=\left\{\begin{array}{c}inf\left\{t\in \mathbb{R}|\left|m_{i,j}\left(t\right)\right|\ge s_{i,j1}+{\ell }_{i,j}\left|u_{i,eqj}\left(t\right)\right|\right\},\hfill \\ \left|u_{i,eqj}\left(t\right)\right|\le {\chi }_{i,j}\hfill \\ inf\left\{t\in \mathbb{R}|\left|m_{i,j}\left(t\right)\right|\ge s_{i,j2}\right\},\left|u_{i,eqj}\left(t\right)\right|>{\chi }_{i,j}\hfill \end{array}\right.& \end{array}$$

(20)

$$\begin{array}{cccc}& & \hfill {\varpi }_{i,j}\left(t\right)=\left\{\begin{array}{c}-{\overline{u}}_{i,eqj}tanh\left({\displaystyle \frac{e_{i,2j}{\overline{u}}_{i,eqj}}{{\delta }_{i,j}}}\right)(1+{\ell }_{i,j})-\hfill \\ {\displaystyle \frac{(1+{\ell }_{i,j})e_{i,2j}}{2{(1-{\ell }_{i,j})}^2}},\left|u_{i,eqj}\left(t\right)\right|\le {\chi }_{i,j}\hfill \\ -{\overline{u}}_{i,eqj}-{\displaystyle \frac{1}{2}}{e}_{i,2j},\left|u_{i,eqj}\left(t\right)\right|>{\chi }_{i,j}\hfill \end{array}\right.& \end{array}$$

(21)

$$\begin{array}{cccc}& & \hfill u_{i,eqj}\left(t\right)={\varpi }_{i,j}\left(t_{i,j,k}\right),\forall t\in \left[t_{i,j,k},t_{i,j,k+1}\right),& \end{array}$$

(22)

where ${\mathit{u}}_{i,eq}=R_i{M}_i^{-1}{\mathit{u}}_i={[u_{i,eq1},u_{i,eq2},u_{i,eq3}]}^{\mathrm{T}}$. The virtual control law is constructed as ${\overline{\mathit{u}}}_{i,eq}={[{\overline{u}}_{i,eq1},{\overline{u}}_{i,eq2},{\overline{u}}_{i,eq3}]}^{\mathrm{T}}$. $m_{i,j}\left(t\right)={\varpi }_{i,j}\left(t\right)-u_{i,eqj}\left(t\right)$. ${\delta }_{i,j}>0,0<l_{i,j}<1$, $s_{i,j1}>0$, $s_{i,j1}>0$, and ${\chi }_{i,j}>0$ are design constants. $t_{i,j,k+1}$ is the controller update time and $t_{i,j,1}$ represents the initial time $t_0$.

Leveraging the RSDASO from (11), the virtual control law ${\overline{\mathit{u}}}_{eq}$ is constructed as

$$\begin{array}{cc}\hfill {\overline{u}}_{i,eqj}& =-{\mu }_{2}si{g}^{a_2}\left({\tilde{x}}_{i,j}\right)-{ϵ}_{2}si{g}^{b_2}\left({\tilde{x}}_{i,j}\right)-{\widehat{\overline{\psi }}}_{i,j}+{\dot{v}}_{i,dj}-{\overline{e}}_{i,j}\hfill \\ & -{\displaystyle \frac{b_{i,2j}{e}_{i,2j}^{\beta }}{{({\kappa }_{i,2j}^2-e_{i,2j}^2)}^{\frac{\beta -1}{2}}}}-{\displaystyle \frac{c_{i,2j}{e}_{i,2j}^{\alpha }}{{({\kappa }_{i,2j}^2-e_{i,2j}^2)}^{\frac{\alpha -1}{2}}}},\hfill \end{array}$$

(23)

where ${\overline{\mathit{e}}}_i={[{\overline{e}}_{i,1},{\overline{e}}_{i,2},{\overline{e}}_{i,3},{\overline{e}}_{i,4},{\overline{e}}_{i,5}]}^{\mathrm{T}}$ and ${\overline{e}}_{i,j}=e_{i,1j}({\kappa }_{i,2j}^2-e_{i,2j}^2)/({\kappa }_{i,1j}^2-e_{i,1j}^2)$, $j=1,2,\dots ,5$.

Theorem 2.

Consider the AUV system (10) subject to state constraints and external disturbances. Under Assumptions 1 and 2, with the RSDASO (11), event-triggering mechanism (20)–(22), and virtual control laws (19) and (23), the tracking errors ${\mathit{e}}_{i,1}$ and ${\mathit{e}}_{i,2}$ exhibit practical fixed-time convergence with the following properties:

• The closed-loop system exhibits practical fixed-time stability, enabling all follower AUVs to establish the desired formation within a finite time $T_{i,2}$ that is independent of initial conditions;
• All system states remain strictly within the specified constraints during operation;
• The event-triggering mechanism maintains a strictly positive minimum inter-execution interval, thereby precluding Zeno behavior.

Proof of Theorem 2. 

The composite Lyapunov function candidate for the closed-loop system is constructed as

$$\begin{array}{cccc}& & \hfill V_i=\sum _{j=1}^5{\displaystyle \frac{1}{2}}ln{\displaystyle \frac{{\kappa }_{i,1j}^2}{{\kappa }_{i,1j}^2-e_{i,1j}^2}}+\sum _{j=1}^5{\displaystyle \frac{1}{2}}ln{\displaystyle \frac{{\kappa }_{i,2j}^2}{{\kappa }_{i,2j}^2-e_{i,2j}^2}}.& \end{array}$$

(24)

Differentiating the Lyapunov function (24) yields

$$\begin{array}{cccc}& & \hfill \begin{array}{cc}\hfill {\dot{V}}_i& =\sum _{j=1}^5{\displaystyle \frac{e_{i,1j}}{{\kappa }_{i,1j}^2-e_{i,1j}^2}}{\dot{e}}_{i,1j}+\sum _{j=1}^5{\displaystyle \frac{e_{i,2j}}{{\kappa }_{i,2j}^2-e_{i,2j}^2}}{\dot{e}}_{i,2j}\hfill \\ & =\sum _{j=1}^5{\displaystyle \frac{e_{i,1j}}{{\kappa }_{i,1j}^2-e_{i,1j}^2}}({\tilde{v}}_{i,j}+e_{i,2j}+v_{i,dj}-{\dot{x}}_j)+\sum _{j=1}^5{\displaystyle \frac{e_{i,2j}}{{\kappa }_{i,2j}^2-e_{i,2j}^2}}(u_{i,eqj}+{\Delta }_{i,j}-{\overline{e}}_{i,j}),\hfill \end{array}& \end{array}$$

(25)

where ${\mathbf{\Delta }}_i={[{\Delta }_{i,1},{\Delta }_{i,2},{\Delta }_{i,3},{\Delta }_{i,4},{\Delta }_{i,5}]}^{\mathrm{T}}={\mu }_{2}si{g}^{a_2}\left({\tilde{\mathit{x}}}_i\right)+{ϵ}_{2}si{g}^{b_2}\left({\tilde{\mathit{x}}}_i\right)+{\widehat{\overline{\mathit{\psi }}}}_i-{\dot{\mathit{v}}}_{i,d}+{\overline{\mathit{e}}}_i$, ${\mathit{u}}_{i,eq}=R{M}^{-1}{\mathit{u}}_i$.

Applying the virtual control law (19) in conjunction with Lemma 2 yields the following key properties:

$$\begin{array}{cccc}& & \hfill \begin{array}{cc}\hfill {\dot{V}}_i& \le -\sum _{j=1}^5{\displaystyle \frac{b_{i,1j}{e}_{i,1j}^{\beta +1}}{{({\kappa }_{i,1j}^2-e_{i,1j}^2)}^{\frac{\beta +1}{2}}}}-\sum _{j=1}^5{\displaystyle \frac{c_{i,1j}{e}_{i,1j}^{\alpha +1}}{{({\kappa }_{i,1j}^2-e_{i,1j}^2)}^{\frac{\alpha +1}{2}}}}+\sum _{j=1}^5{\displaystyle \frac{e_{i,2j}}{{\kappa }_{i,2j}^2-e_{i,2j}^2}}(u_{i,eqj}+{\Delta }_{i,j}-{\overline{e}}_{i,j})\hfill \\ & +\sum _{j=1}^5{\displaystyle \frac{e_{i,1j}{e}_{i,2j}}{{\kappa }_{i,1j}^2-e_{i,1j}^2}}-\sum _{j=1}^5{\displaystyle \frac{e_{i,2j}{\overline{e}}_{i,j}}{{\kappa }_{i,2j}^2-e_{i,2j}^2}}+\sum _{j=1}^5{\displaystyle \frac{e_{i,1j}{\tilde{v}}_{i,j}}{{\kappa }_{i,1j}^2-e_{i,1j}^2}}\hfill \\ & \le -\sum _{j=1}^5{\displaystyle \frac{b_{i,1j}{e}_{i,1j}^{\beta +1}}{{({\kappa }_{i,1j}^2-e_{i,1j}^2)}^{\frac{\beta +1}{2}}}}-\sum _{j=1}^5{\displaystyle \frac{c_{i,1j}{e}_{i,1j}^{\alpha +1}}{{({\kappa }_{i,1j}^2-e_{i,1j}^2)}^{\frac{\alpha +1}{2}}}}+\sum _{j=1}^5{\displaystyle \frac{e_{i,2j}}{{\kappa }_{i,2j}^2-e_{i,2j}^2}}(u_{i,eqj}+{\Delta }_{i,j})\hfill \\ & +\sum _{j=1}^5{\displaystyle \frac{e_{i,1j}{\tilde{v}}_{i,j}}{{\kappa }_{i,1j}^2-e_{i,1j}^2}}.\hfill \end{array}& \end{array}$$

(26)

Based on Cases 1–2 in Appendix C, the following inequality can be obtained:

$$\begin{array}{ccccc}& & \hfill e_{i,2j}{u}_{i,eqj}& ⩽e_{i,2j}{\overline{u}}_{i,eqj}+0.2785{\delta }_{i,j}+{\displaystyle \frac{1}{2}}{s}_{i,j1}^2+{\displaystyle \frac{1}{2}}{s}_{i,j2}^2.\hfill & \end{array}$$

(27)

Given the positive constants ${\kappa }_{i,j},{\delta }_{i,j}$, $s_{i,j1}$, and $s_{i,j2}$, there exists a uniform bounding constant ${\theta }_i>0$ satisfying

$$\begin{array}{cccc}& & \hfill \begin{array}{cc}\hfill {\dot{V}}_i& \le -\sum _{j=1}^5{\displaystyle \frac{b_{i,1j}{e}_{i,1j}^{\beta +1}}{{({\kappa }_{i,1j}^2-e_{i,1j}^2)}^{\frac{\beta +1}{2}}}}-\sum _{j=1}^5{\displaystyle \frac{c_{i,1j}{e}_{i,1j}^{\alpha +1}}{{({\kappa }_{i,1j}^2-e_{i,1j}^2)}^{\frac{\alpha +1}{2}}}}-\sum _{j=1}^5{\displaystyle \frac{b_{i,2j}{e}_{i,2j}^{\beta +1}}{{({\kappa }_{i,2j}^2-e_{i,2j}^2)}^{\frac{\beta +1}{2}}}}\hfill \\ & -\sum _{j=1}^5{\displaystyle \frac{c_{i,2j}{e}_{i,2j}^{\alpha +1}}{{({\kappa }_{i,2j}^2-e_{i,2j}^2)}^{\frac{\alpha +1}{2}}}}+\sum _{j=1}^5{\displaystyle \frac{e_{i,1j}{\tilde{v}}_i}{{\kappa }_{i,1j}^2-e_{i,1j}^2}}+{\theta }_i.\hfill \end{array}& \end{array}$$

(28)

According to Theorem 1, the estimation error of the observer converges in fixed time when $t\ge T_1$, so that we have

$$\begin{array}{cccc}& & \hfill \begin{array}{cc}\hfill {\dot{V}}_i& \le -\sum _{j=1}^5{\displaystyle \frac{b_{i,1j}{e}_{i,1j}^{\beta +1}}{{({\kappa }_{i,1j}^2-e_{i,1j}^2)}^{\frac{\beta +1}{2}}}}-\sum _{j=1}^5{\displaystyle \frac{c_{i,1j}{e}_{i,1j}^{\alpha +1}}{{({\kappa }_{i,1j}^2-e_{i,1j}^2)}^{\frac{\alpha +1}{2}}}}-\sum _{j=1}^5{\displaystyle \frac{b_{i,2j}{e}_{i,2j}^{\beta +1}}{{({\kappa }_{i,2j}^2-e_{i,2j}^2)}^{\frac{\beta +1}{2}}}}\hfill \\ & -\sum _{j=1}^5{\displaystyle \frac{c_{i,2j}{e}_{i,2j}^{\alpha +1}}{{({\kappa }_{i,2j}^2-e_{i,2j}^2)}^{\frac{\alpha +1}{2}}}}+{\theta }_i.\hfill \end{array}& \end{array}$$

(29)

By applying Lemma 2 and Lemma 4, the system dynamics in (29) can be reformulated as

$$\begin{array}{cccc}& & \hfill \begin{array}{cc}\hfill {\dot{V}}_i& \le -{\varphi }_{i,1}{(\sum _{j=1}^5{\displaystyle \frac{1}{2}}ln{\displaystyle \frac{{\kappa }_{i,1j}^2}{{\kappa }_{i,1j}^2-e_{i,1j}^2}})}^{{\displaystyle \frac{\alpha +1}{2}}}-{\varphi }_{i,2}{(\sum _{j=1}^5{\displaystyle \frac{1}{2}}ln{\displaystyle \frac{{\kappa }_{i,1j}^2}{{\kappa }_{i,1j}^2-e_{i,1j}^2}})}^{{\displaystyle \frac{\beta +1}{2}}}\hfill \\ & -{\phi }_{i,1}{(\sum _{j=1}^5{\displaystyle \frac{1}{2}}ln{\displaystyle \frac{{\kappa }_{i,2j}^2}{{\kappa }_{i,2j}^2-e_{i,2j}^2}})}^{{\displaystyle \frac{\alpha +1}{2}}}-{\phi }_{i,2}{(\sum _{j=1}^5{\displaystyle \frac{1}{2}}ln{\displaystyle \frac{{\kappa }_{i,2j}^2}{{\kappa }_{i,2j}^2-e_{i,2j}^2}})}^{{\displaystyle \frac{\beta +1}{2}}}+{\theta }_i\hfill \\ & \le -{\gamma }_{i,1}{V}^p-{\gamma }_{i,2}{V}^q+{\theta }_i,\hfill \end{array}& \end{array}$$

(30)

where ${\varphi }_{i,1}=3^{{\displaystyle \frac{1-\alpha }{2}}}\times 2^{{\displaystyle \frac{\alpha +1}{2}}}min\{c_{i,11},c_{i,12},c_{i,13}\}$, ${\varphi }_{i,2}=2^{{\displaystyle \frac{\beta +1}{2}}}min\{b_{i,11},b_{i,12},b_{i,13}\}$, ${\phi }_{i,1}=3^{{\displaystyle \frac{1-\alpha }{2}}}\times 2^{{\displaystyle \frac{\alpha +1}{2}}}min\{c_{i,21},c_{i,22},c_{i,23}\}$, ${\phi }_{i,2}=2^{{\displaystyle \frac{\beta +1}{2}}}min\{b_{i,21},b_{i,22},b_{i,23}\}$; ${\gamma }_{i,1}=min\{{\varphi }_{i,1},{\phi }_{i,1}\}$, ${\gamma }_{i,2}=3^{{\displaystyle \frac{1-\beta }{2}}}min\{{\varphi }_{i,2},{\phi }_{i,2}\}$.

Under the conditions of Lemma 1, the tracking errors ${\mathit{e}}_{i,1}$ and ${\mathit{e}}_{i,2}$ exhibit fixed-time convergence to the origin. The convergence time $T_{i,2}$ is explicitly bounded by

$$\begin{array}{cccc}& & \hfill T_{i,2}\le {\displaystyle \frac{1}{{\gamma }_{i,1}{\rho }_i(p-1)}}+{\displaystyle \frac{1}{{\gamma }_{i,2}{\rho }_i(1-q)}}.& \end{array}$$

(31)

The composite tracking error ${\mathit{E}}_i={[{\mathit{e}}_{i,1},{\mathit{e}}_{i,2}]}^{\mathrm{T}}$ converges to a compact residual set:

$$\begin{array}{cccc}& & \hfill {\Omega }_i=\left\{V_i\left({\mathit{E}}_i\right)⩽min\left\{{\left[{\displaystyle \frac{{\theta }_i}{{\gamma }_{i,1}(1-{\rho }_i)}}\right]}^{{\displaystyle \frac{1}{p}}},{\left[{\displaystyle \frac{{\theta }_i}{{\gamma }_{i,2}(1-{\rho }_i)}}\right]}^{{\displaystyle \frac{1}{q}}}\right\}\right\}.& \end{array}$$

(32)

Based on (32), the tracking errors $e_{i,1j}$ and $e_{i,2j}$ are uniformly bounded. Since $x_j$ is bounded, it follows that $v_{i,dj}$ is bounded, according to (19). Since $e_{i,2j}$, $v_{i,dj}$, and ${\widehat{\overline{\psi }}}_{i,j}$ are bounded, ${\overline{u}}_{eqi}$ is bounded. Finally, according to (21) and (22), ${\varpi }_{i,j}$ and $u_{i,eqj}$ are bounded, i.e., $u_{i,j}$ is bounded. The proposed control system guarantees that all closed-loop signals are ultimately uniformly bounded.

As ${\varpi }_{i,j}$ is a function of ${\overline{u}}_{eqi}$ and $e_{i,2j}$, ${\dot{\varpi }}_{i,j}$ is bounded, i.e., ${\dot{\varpi }}_{i,j}<{\overline{\varpi }}_{i,j}$ with ${\overline{\varpi }}_{i,j}>0$. In addition, when $\left|u_{i,eqj}\left(t\right)\right|\le {\chi }_{i,j}$ and $t\to t_{i,j,k+1}$, $m_{i,j}\left(t\right)=s_{i,j1}+{\ell }_{i,j}\left|u_{i,eqj}\left(t\right)\right|=0$. So, the time interval satisfies

$$\begin{array}{cccc}& & \hfill t_{i,j}^{\ast }⩾{\displaystyle \frac{{\ell }_{i,j}\left|u_{i,eqj}\right|+s_{i,j1}}{{\overline{\varpi }}_{i,j}}}.& \end{array}$$

(33)

Similarly, when $\left|u_{i,eqj}\left(t\right)\right|>{\chi }_{i,j}$, one has

$$\begin{array}{cccc}& & \hfill t_{i,j}^{\ast }⩾{\displaystyle \frac{s_{i,j2}}{{\overline{\varpi }}_{i,j}}}.& \end{array}$$

(34)

In conclusion, the Zeno behavior is successfully avoided.    □

Remark 2.

The developed control strategy guarantees fixed-time stabilization for AUV systems operating under state constraints and subject to unknown time-varying disturbances. As established in (31), the system achieves convergence within a finite time interval whose upper bound is completely determined by the controller design parameters, independent of initial conditions or external disturbances. This fixed-time convergence property is rigorously demonstrated through the Lyapunov-based analysis in (31), where the settling time bound is shown to depend solely on the selection of the control gains.

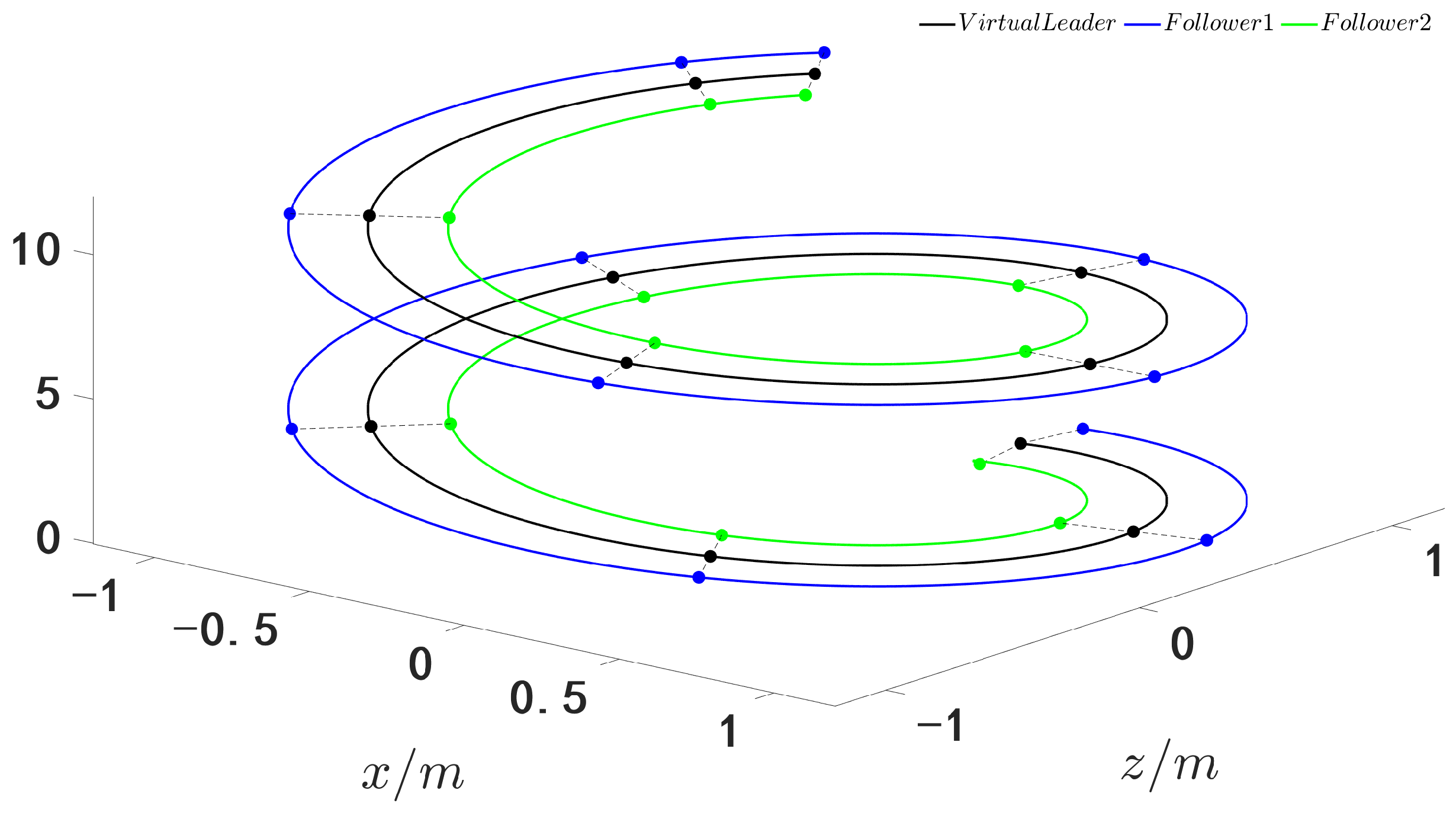
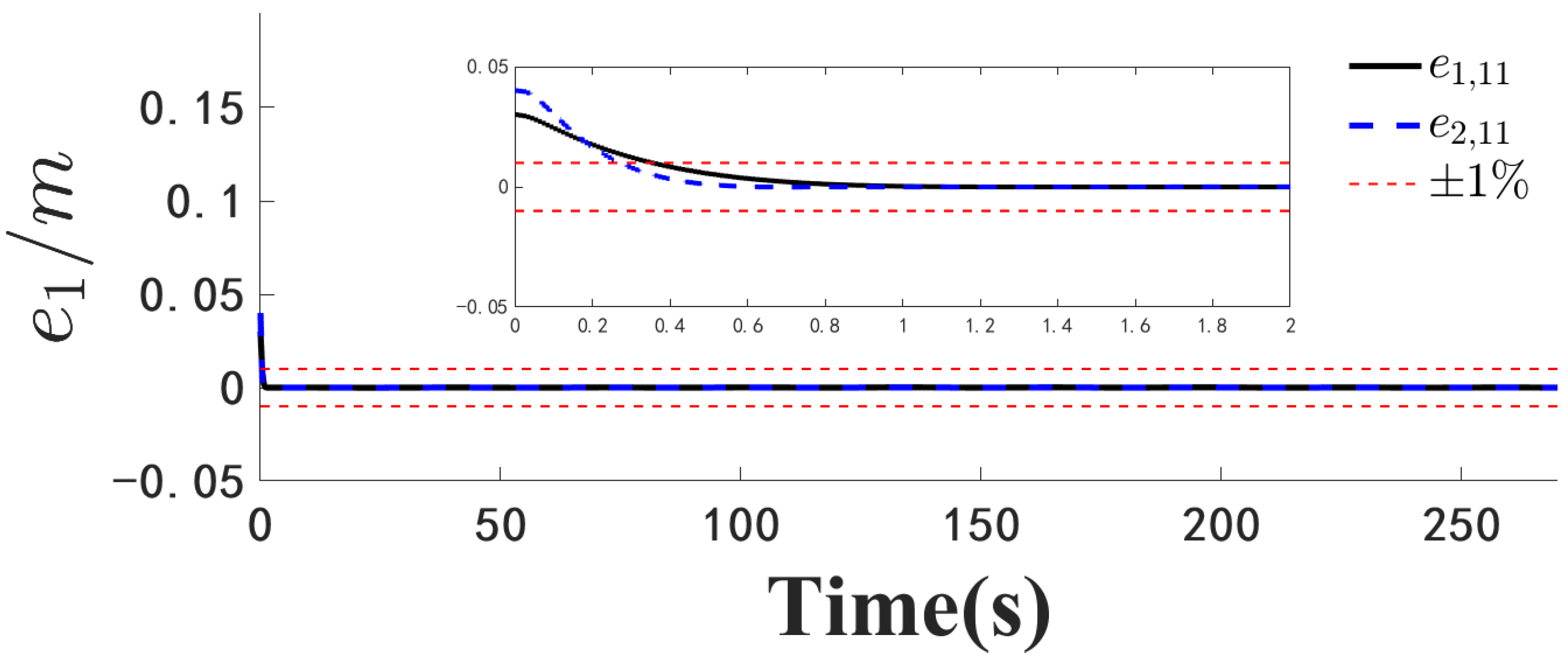
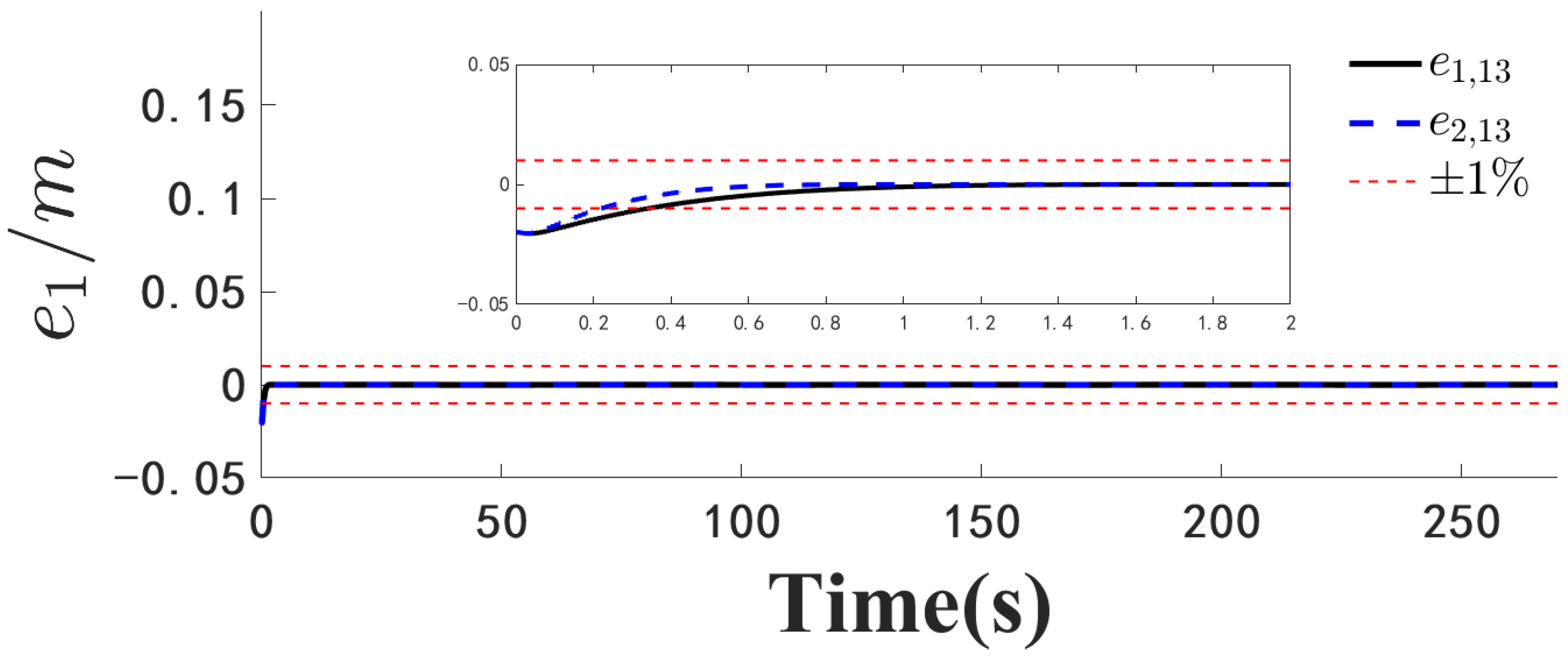
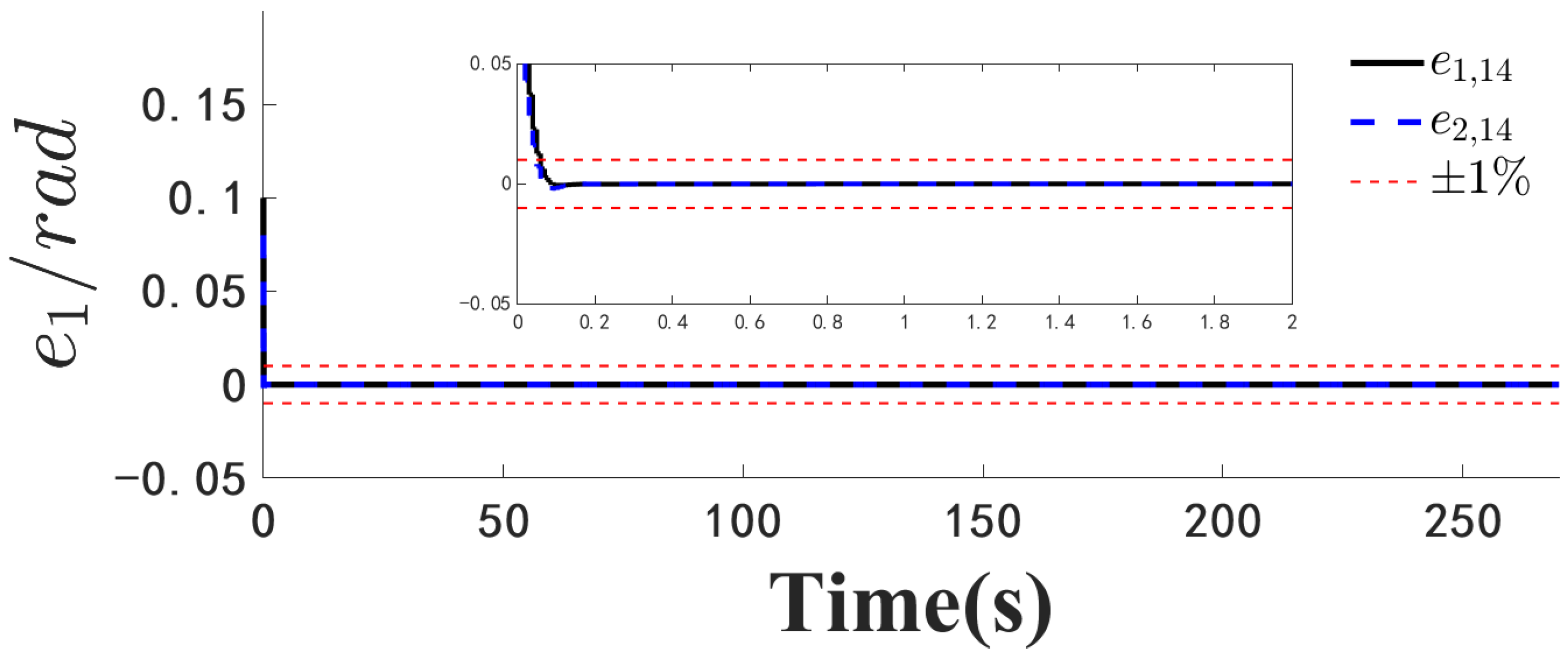
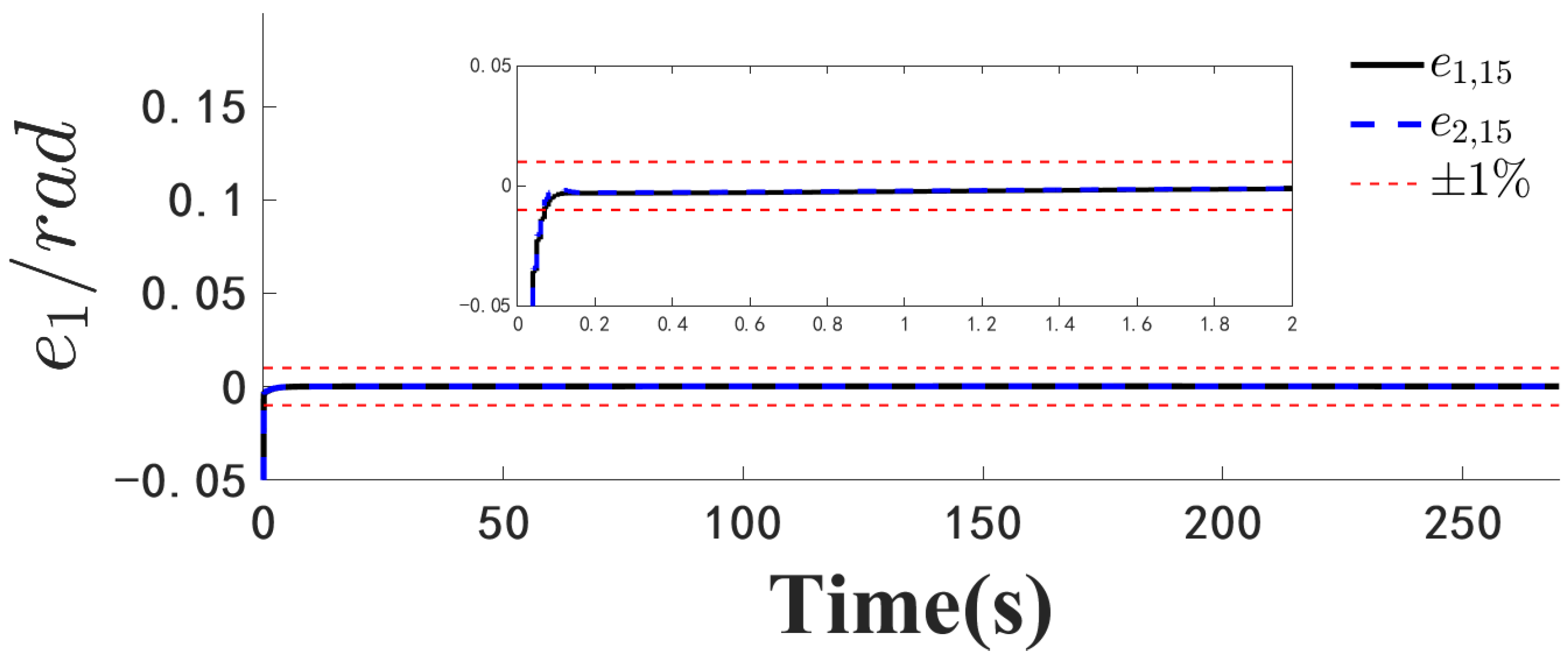
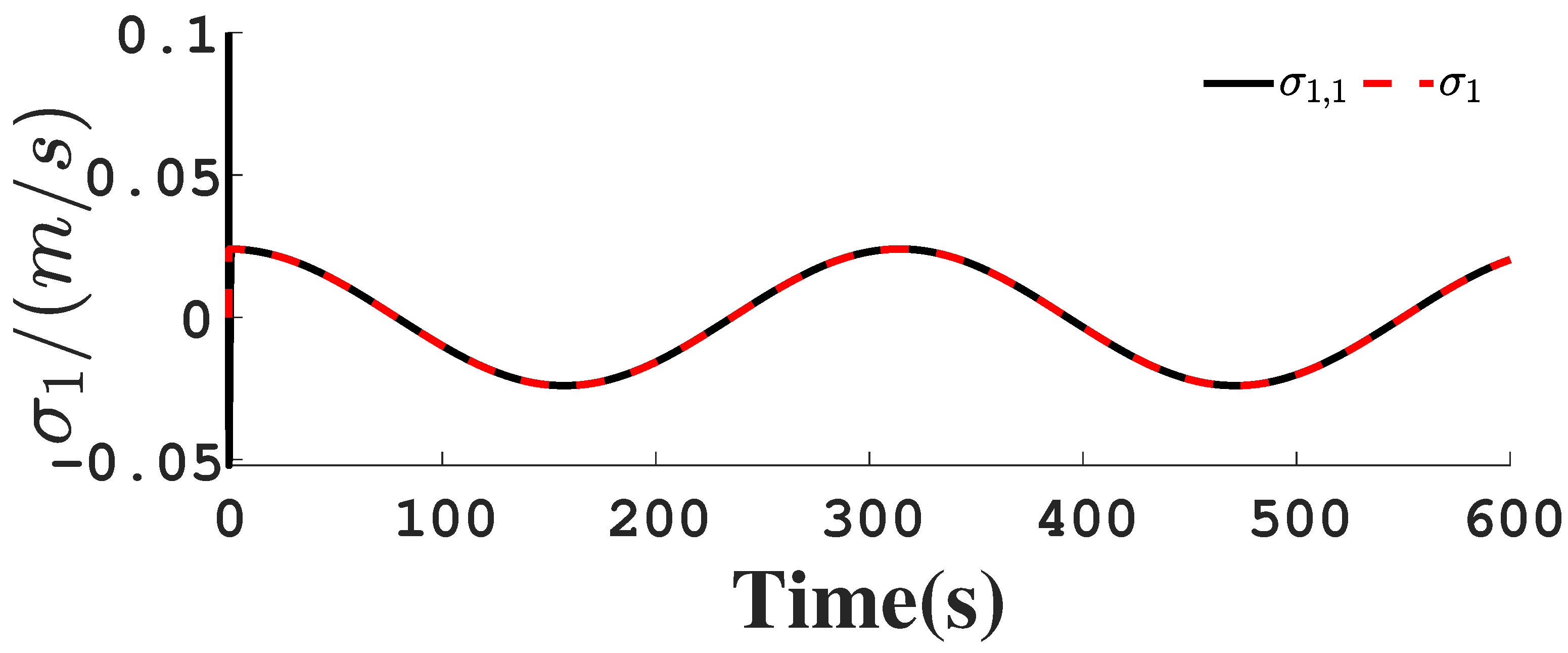
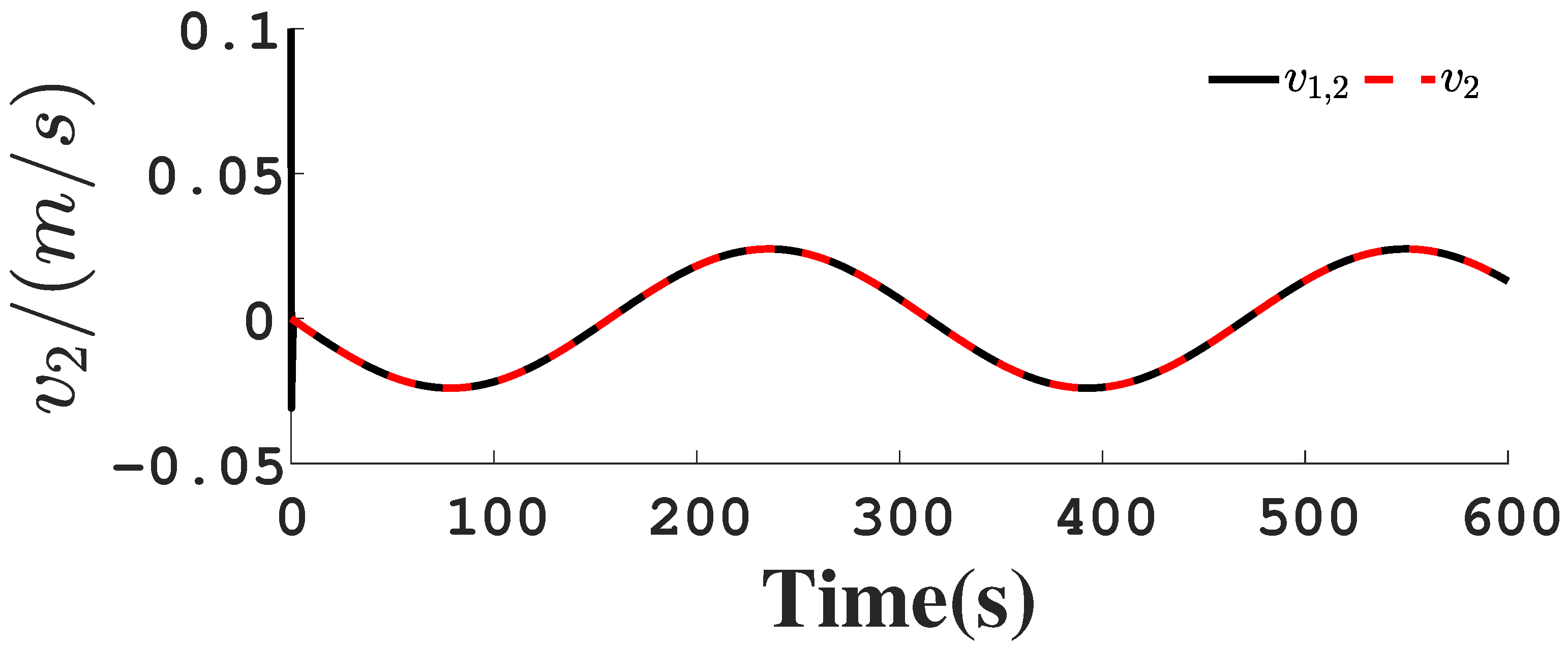
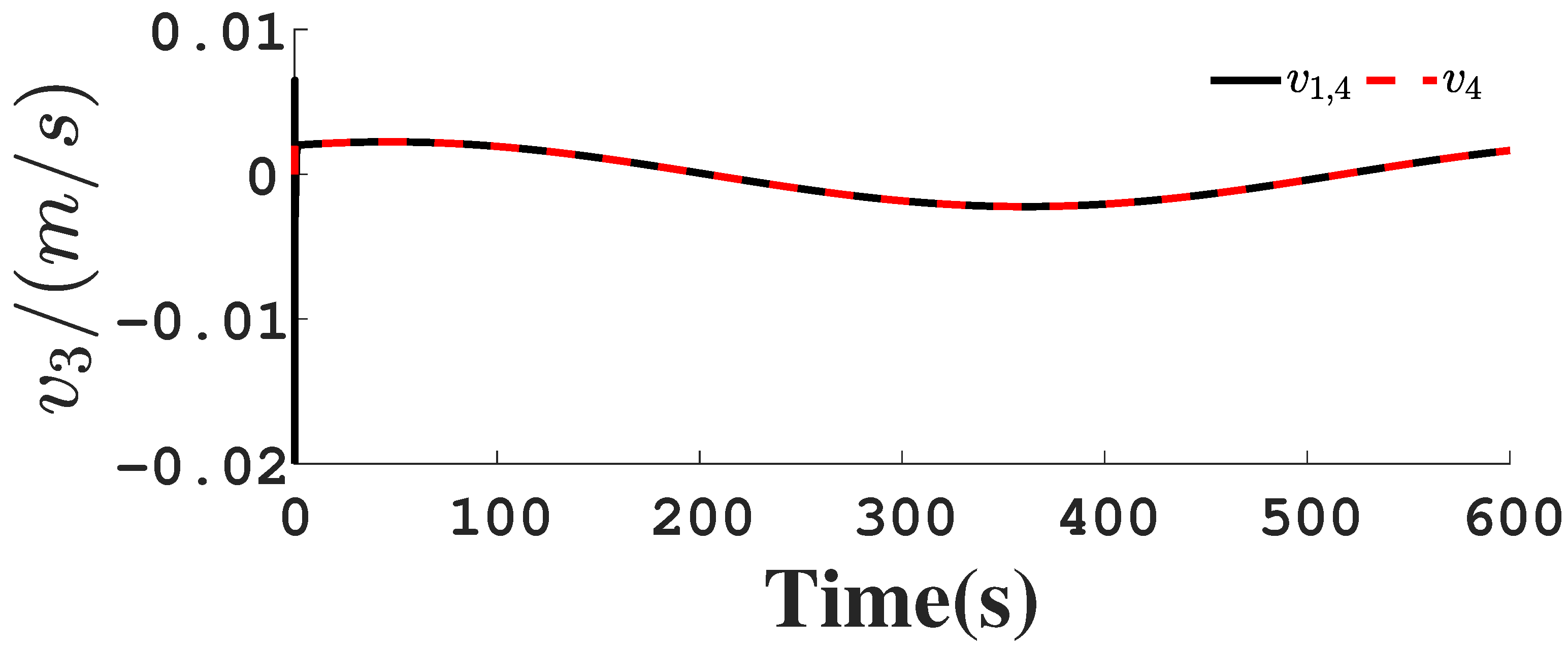
4. Simulation and Results

The efficacy of the proposed control approach is evaluated through a formation tracking scenario involving two follower AUVs pursuing a virtual leader AUV, with the experimental results confirming its performance.

The pseudocode for the proposed control algorithm simulation experiment is shown in Algorithm A1 in Appendix A.

Please refer to

Table A1. AUV physical model parameters.

Element	Value	Element	Value	Element	Value
m	390	$I_y$	305.67	$I_z$	305.67
$X_{\dot{u}}$	−49.12	$Y_{\dot{v}}$	−311.52	$Z_{\dot{\omega }}$	−311.52
$X_u$	−20	$Y_v$	−200	$Z_{\omega }$	−200
$X_{uu}$	−30	$Y_{vv}$	−300	$Z_{\omega \omega }$	−300
$N_r$	−100	$N_{\dot{r}}$	−87.63	$N_{rr}$	300
$M_q$	−200	$M_{\dot{q}}$	−87.63	$M_{qq}$	−300

in Appendix B for the parameters of the AUV dynamic model [31].

The following formulation is adopted for the time-varying external disturbances affecting the AUV:

$$\begin{array}{cc}\hfill \left\{\begin{array}{c}{\psi }_1=5.5sin\left(0.01t\right)+4.5sin\left(0.2t\right)\left(N\right)\hfill \\ {\psi }_2=4.5+3.0sin\left(0.02t\right)\left(N\right)\hfill \\ {\psi }_3=3.5sin\left(0.01t\right)+5.5sin\left(0.1t\right)\left(N\right)\hfill \\ {\psi }_4=5+2.0cos\left(0.01t\right)(N·m)\hfill \\ {\psi }_5=2.0sin\left(0.01t\right)+2(N·m).\hfill \end{array}\right.& \end{array}$$

(35)

The controller parameters are specified as follows: ${\kappa }_{1,1j}={\kappa }_{1,2j}=2$, ${\kappa }_{2,1j}={\kappa }_{2,2j}=1$, $b_{1,1j}=c_{1,1j}=1$, $b_{2,2j}=1$, $c_{2,2j}=2$, ${\delta }_{1,j}={\delta }_{2,j}=0.1$, $h_{1,j}=h_{2,j}=0.1$, $\alpha =0.8$, $\beta =1.2$, ${\ell }_1=0.01$, ${\ell }_2=0.02$, ${\ell }_3=0.05$, ${\ell }_4=0.03$, and ${\ell }_5=0.1$, where $j=1,2,...,5$.

The parameters associated with the RSDASO are $a_1=0.8$, $a_2=0.6$, $a_3=0.4$, $b_1=1.2$, $b_2=1.4$, $b_3=1.6$, ${\mu }_1=11$, ${\mu }_2=26$, ${\mu }_3=16$, ${\delta }_1=11$, ${\delta }_2=26$, ${\delta }_3=16$, and $\gamma =5$.

The simulation results are shown in Figure 1, Figure 2, Figure 3, Figure 4, Figure 5, Figure 6, Figure 7, Figure 8, Figure 9, Figure 10, Figure 11, Figure 12, Figure 13, Figure 14, Figure 15, Figure 16, Figure 17, Figure 18, Figure 19, Figure 20, Figure 21, Figure 22, Figure 23, Figure 24, Figure 25, Figure 26, Figure 27, Figure 28, Figure 29 and Figure 30. Figure 1 illustrates the trajectory of two AUVs following the virtual leader. The three components of the tracking error for position and attitude are shown in Figure 2, Figure 3, Figure 4, Figure 5 and Figure 6. The tracking of the linear and angular velocities of the AUVs is shown in Figure 7, Figure 8, Figure 9, Figure 10 and Figure 11. Figure 12, Figure 13, Figure 14, Figure 15 and Figure 16 illustrate the variation of the control input signal. Figure 17, Figure 18, Figure 19, Figure 20 and Figure 21 show the observation errors for position and attitude. The tracking of unmeasurable velocities by the observer is demonstrated in Figure 22, Figure 23 and Figure 24. Figure 25, Figure 26, Figure 27, Figure 28 and Figure 29 illustrate the update interval for the control signals. A comparison of the number of signal updates for each actuator is shown in Figure 30.

Figure 1, Figure 2, Figure 3, Figure 4, Figure 5, Figure 6, Figure 7, Figure 8, Figure 9, Figure 10, Figure 11, Figure 12, Figure 13, Figure 14, Figure 15, Figure 16, Figure 17, Figure 18, Figure 19, Figure 20, Figure 21, Figure 22, Figure 23, Figure 24, Figure 25, Figure 26, Figure 27, Figure 28 and Figure 29 confirm the boundedness of all system signals. Specifically, Figure 1 demonstrates close convergence between the AUV’s actual trajectory and the reference path, validating the tracking performance of the proposed controller. Figure 2, Figure 3, Figure 4, Figure 5 and Figure 6 further reveal that the state 1 tracking error remains confined within a 1% error margin, while Figure 7, Figure 8, Figure 9, Figure 10 and Figure 11 corroborate effective velocity tracking. The actuator inputs, depicted in Figure 12, Figure 13, Figure 14, Figure 15 and Figure 16, exhibit smooth and physically realizable profiles upon system stabilization. The observer’s efficacy is evidenced in Figure 17, Figure 18, Figure 19, Figure 20, Figure 21, Figure 22, Figure 23 and Figure 24. Figure 17, Figure 18, Figure 19, Figure 20 and Figure 21 show rapid convergence of position/attitude estimation errors to a near-zero residual band, whereas Figure 22, Figure 23 and Figure 24 confirm the accurate reconstruction of unmeasurable linear and angular velocities. The event-triggering mechanism (ETM) performance is quantified in Figure 30 and

Table 1. Table of event triggers for each AUV.

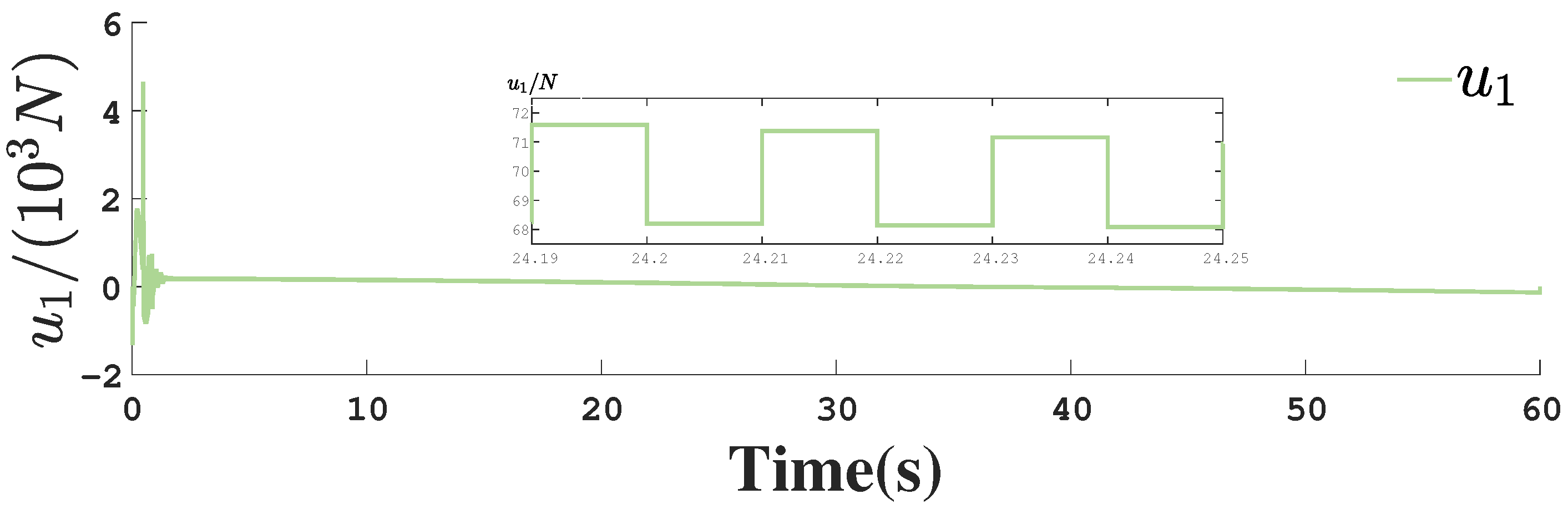
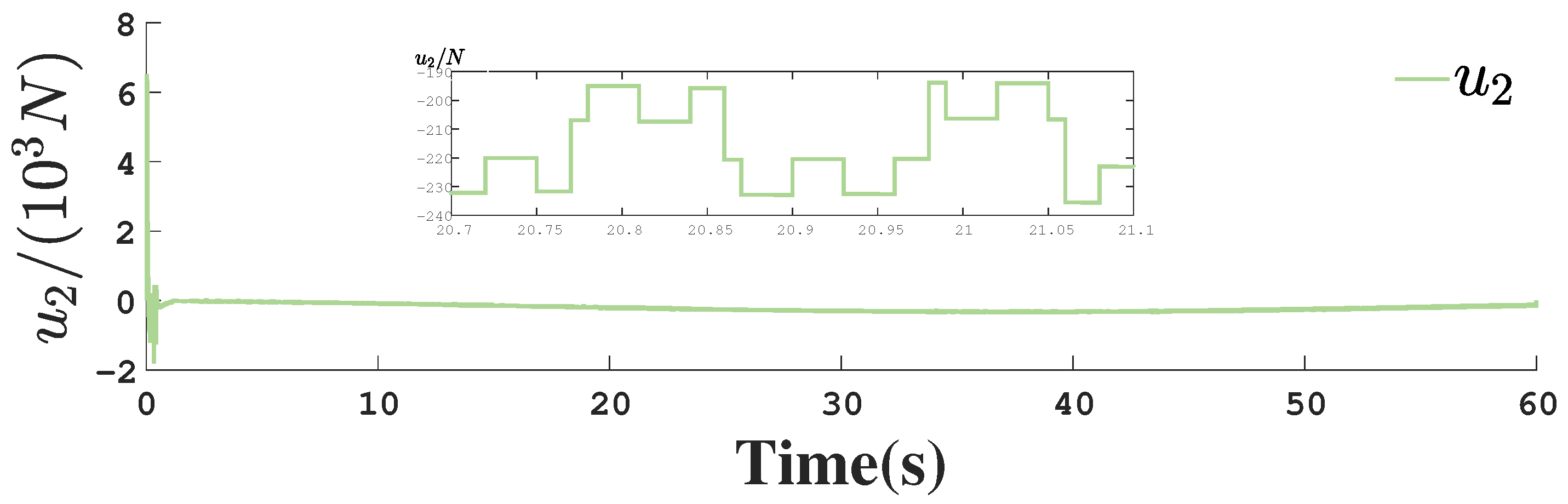
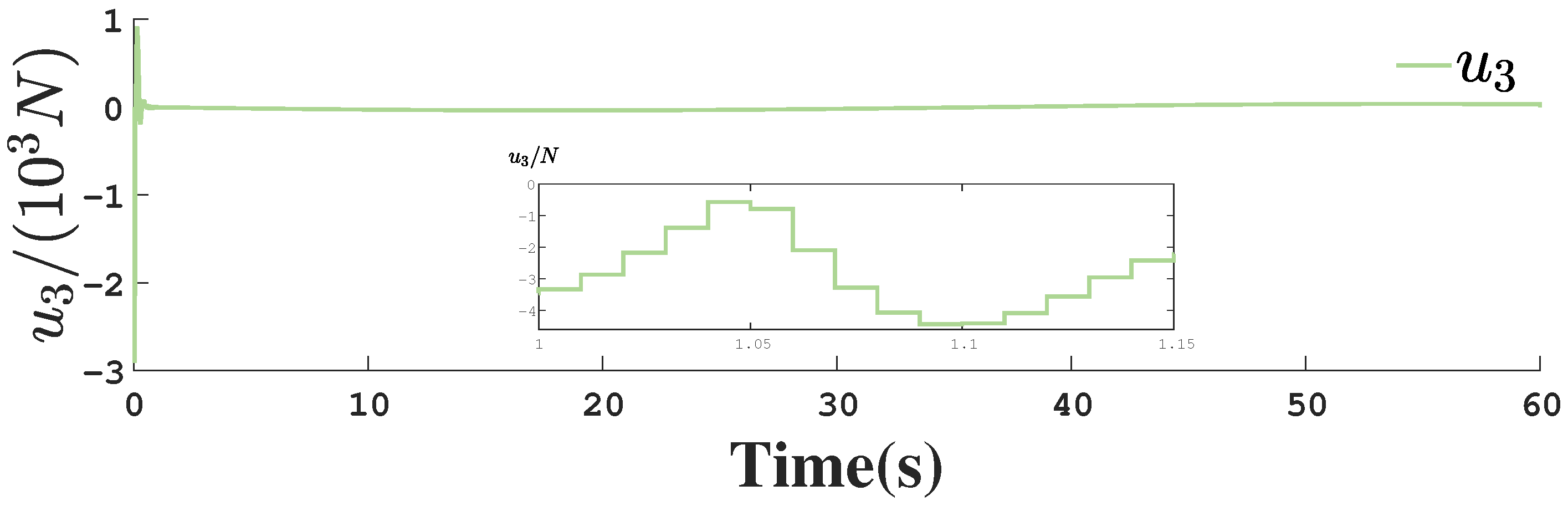
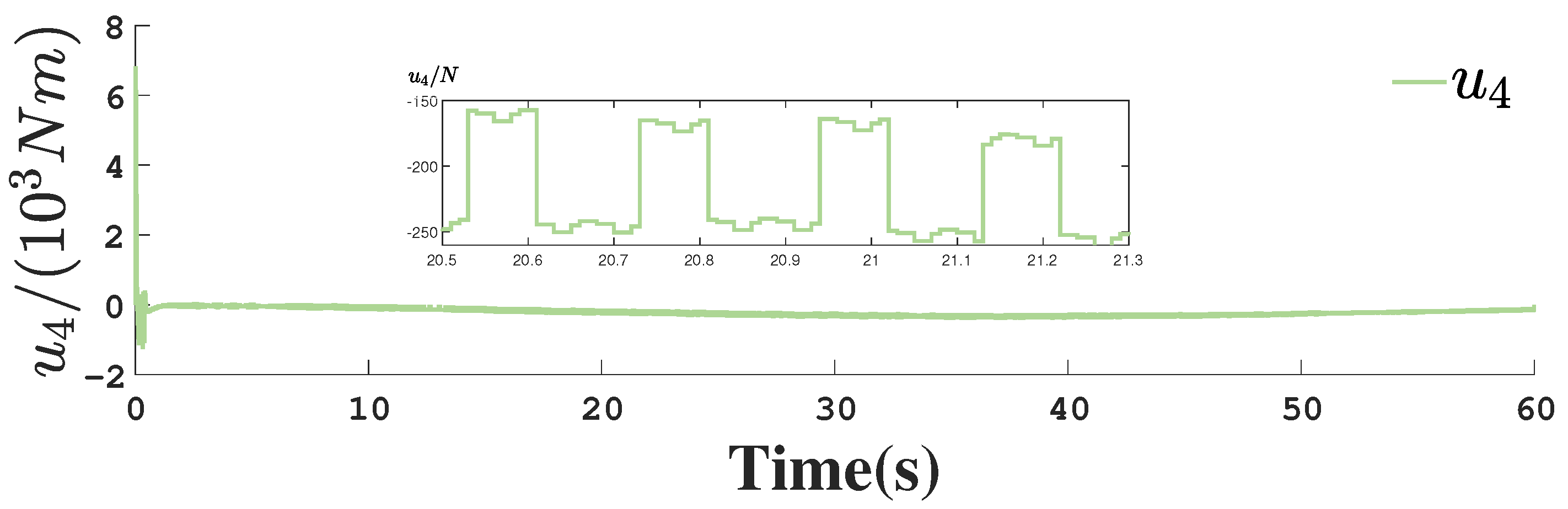
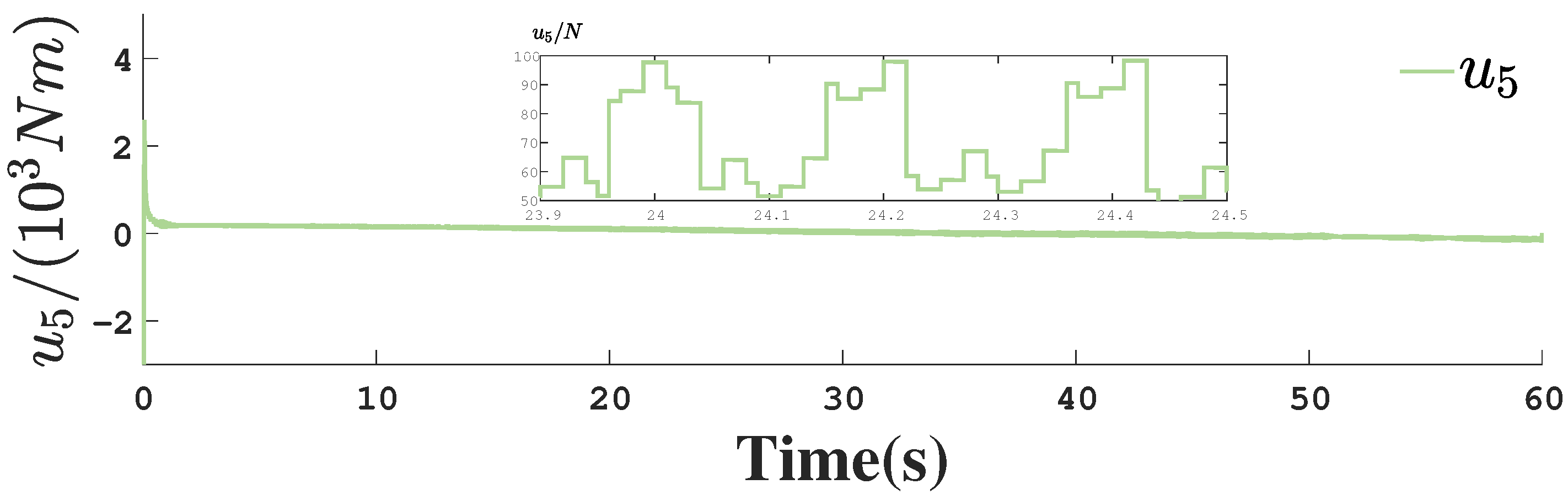
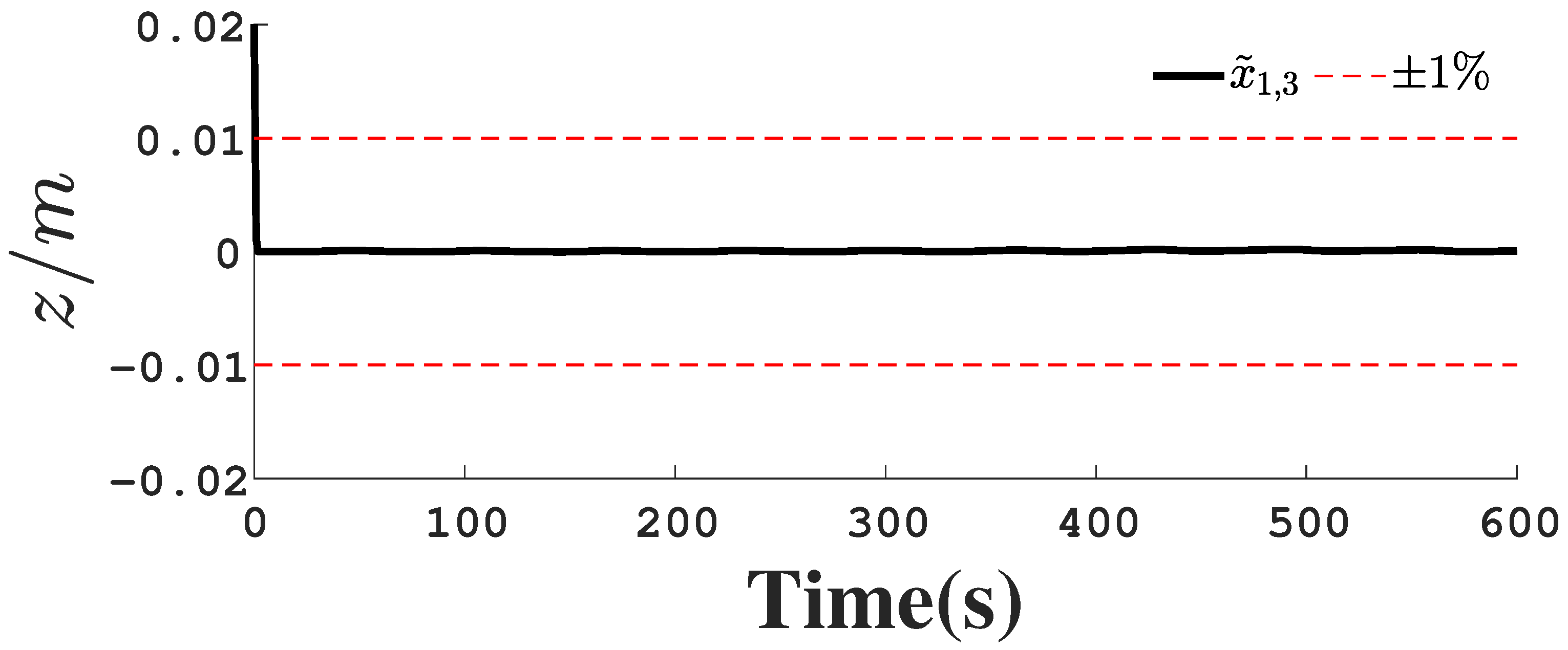
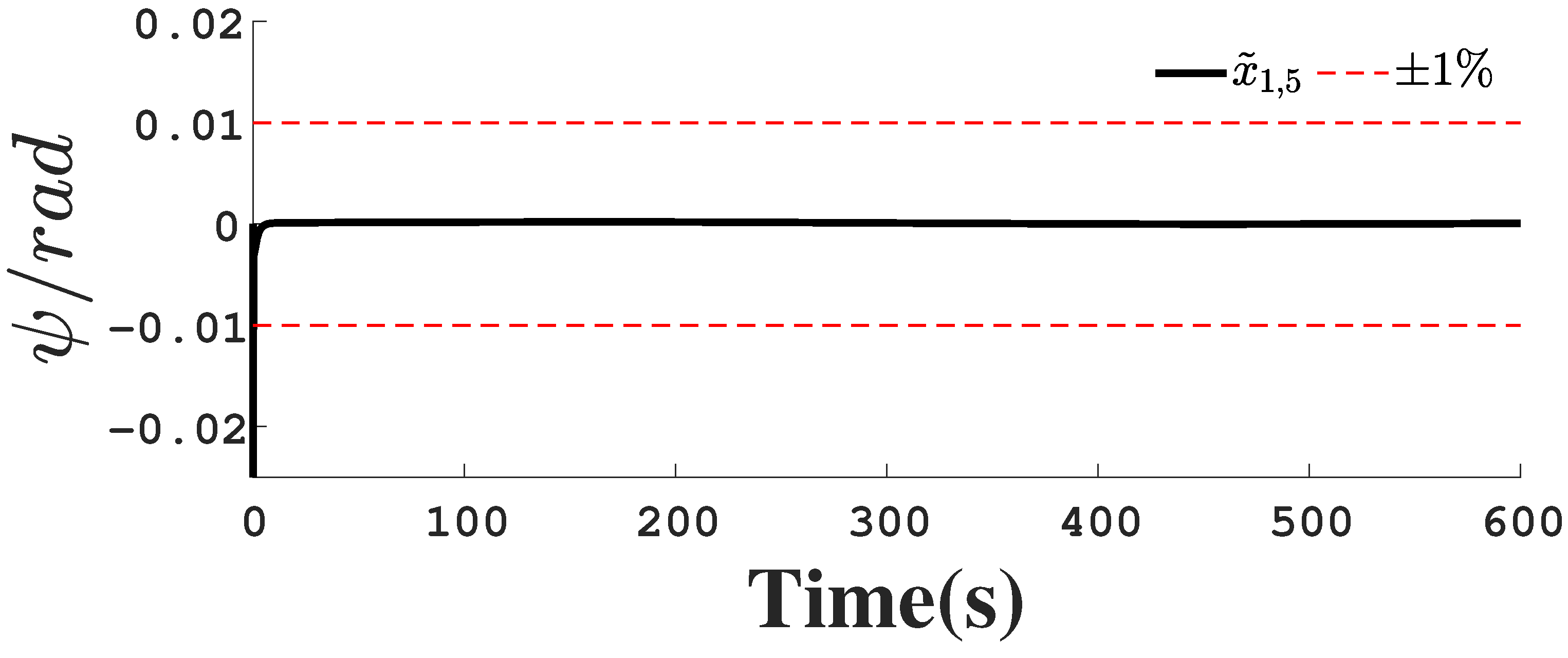
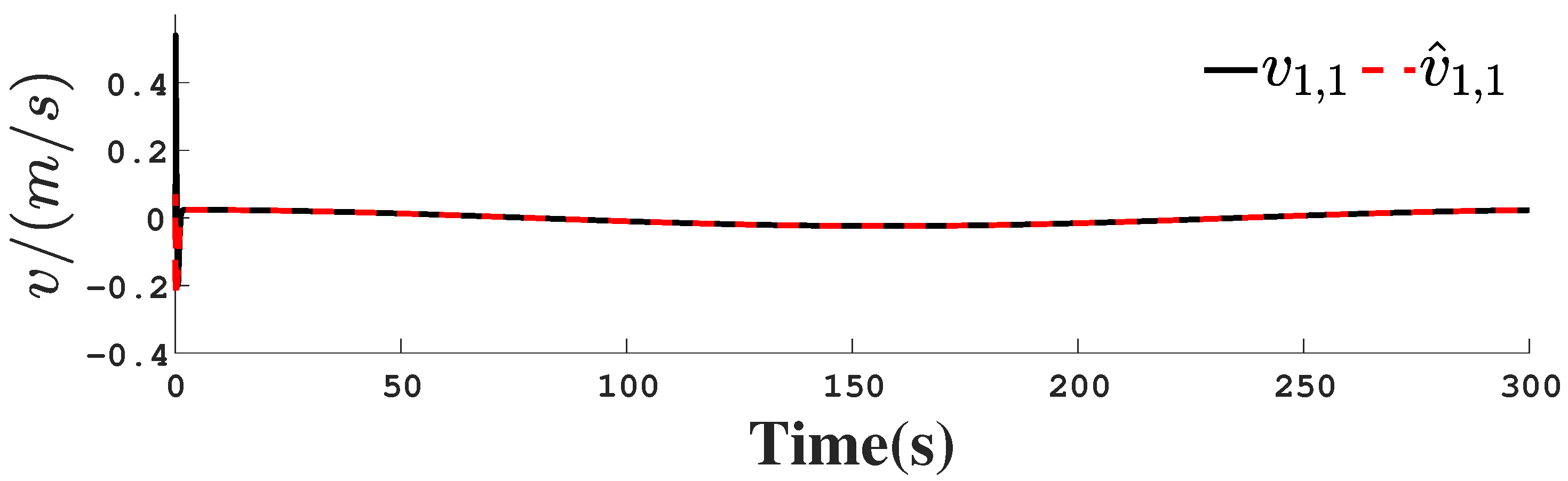
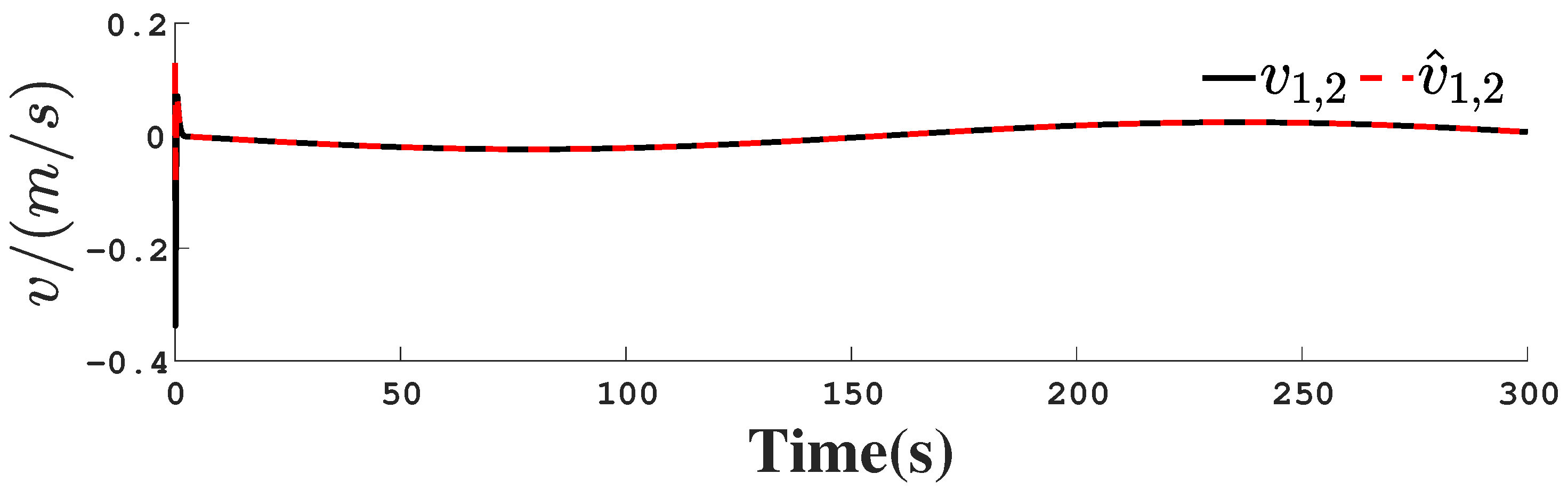
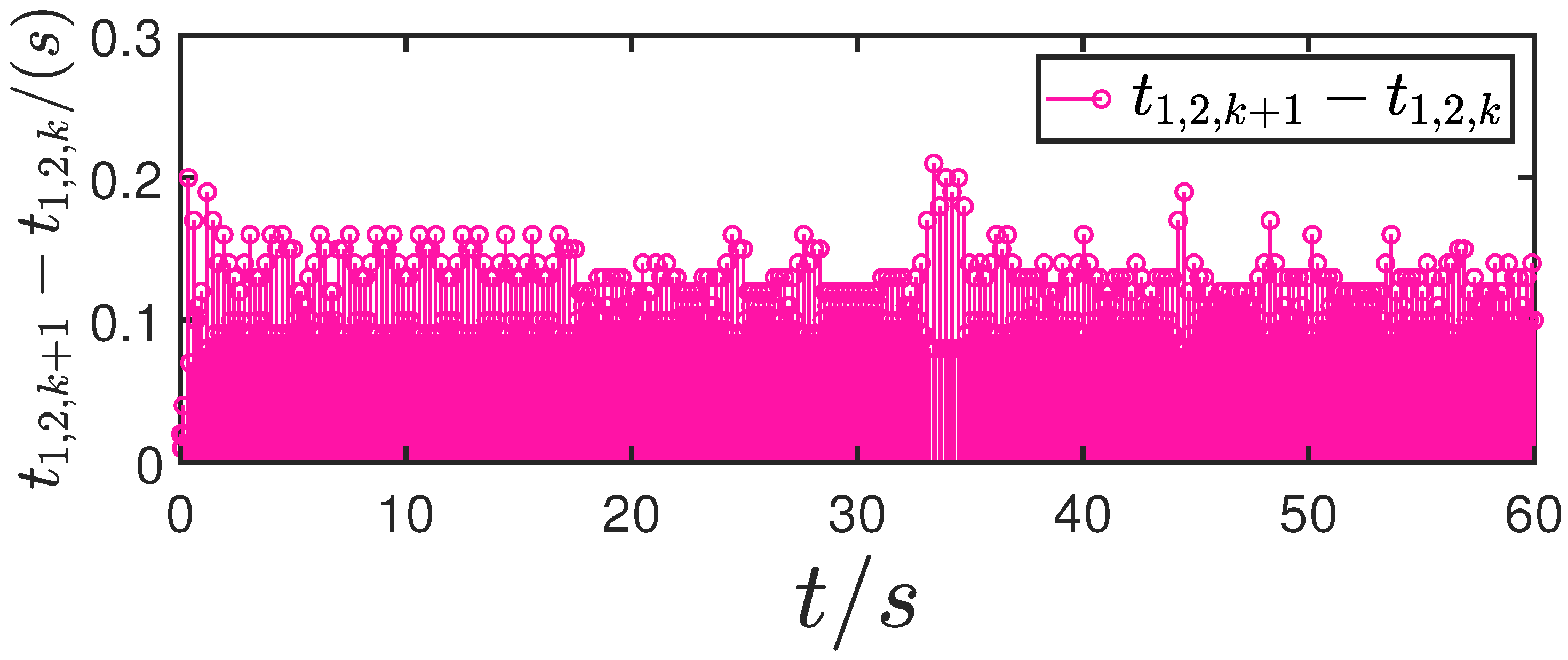
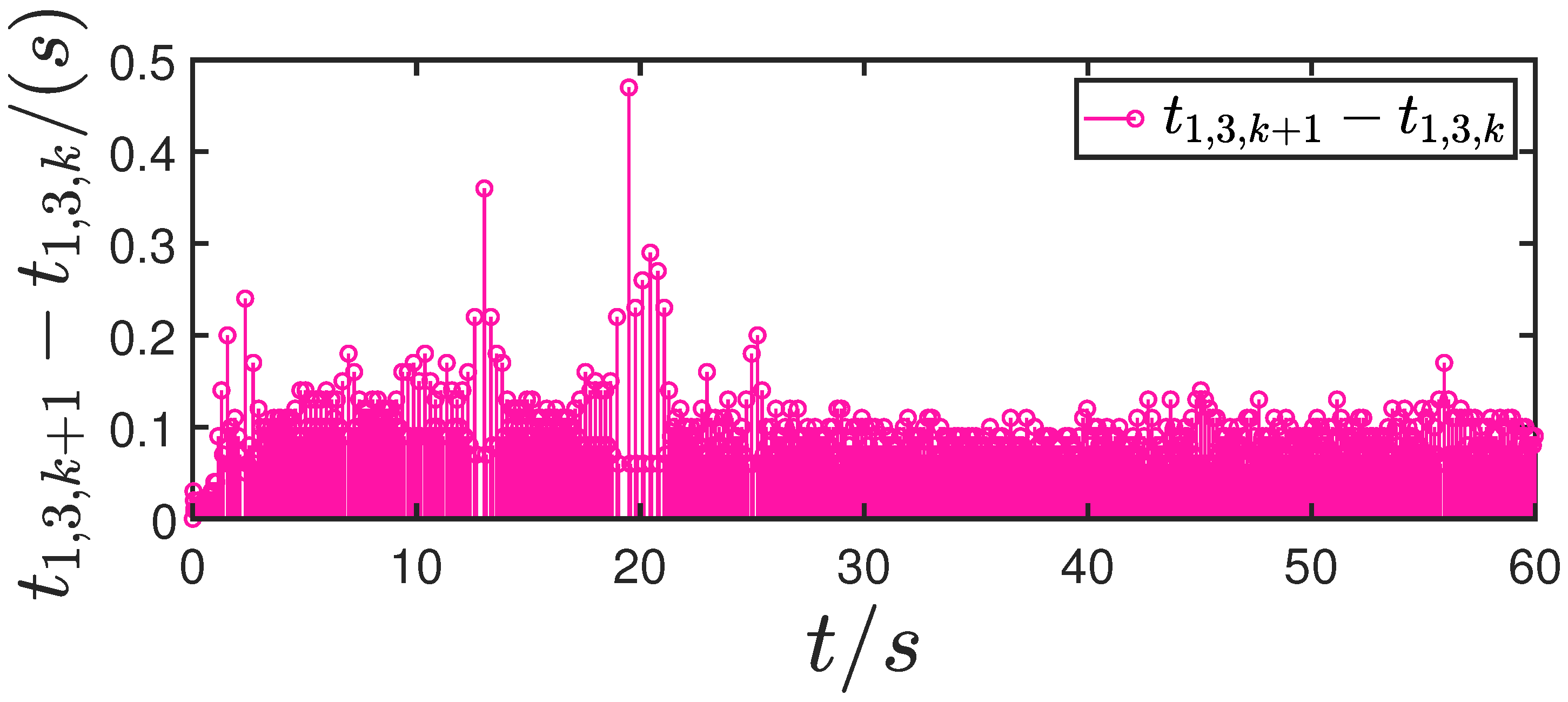
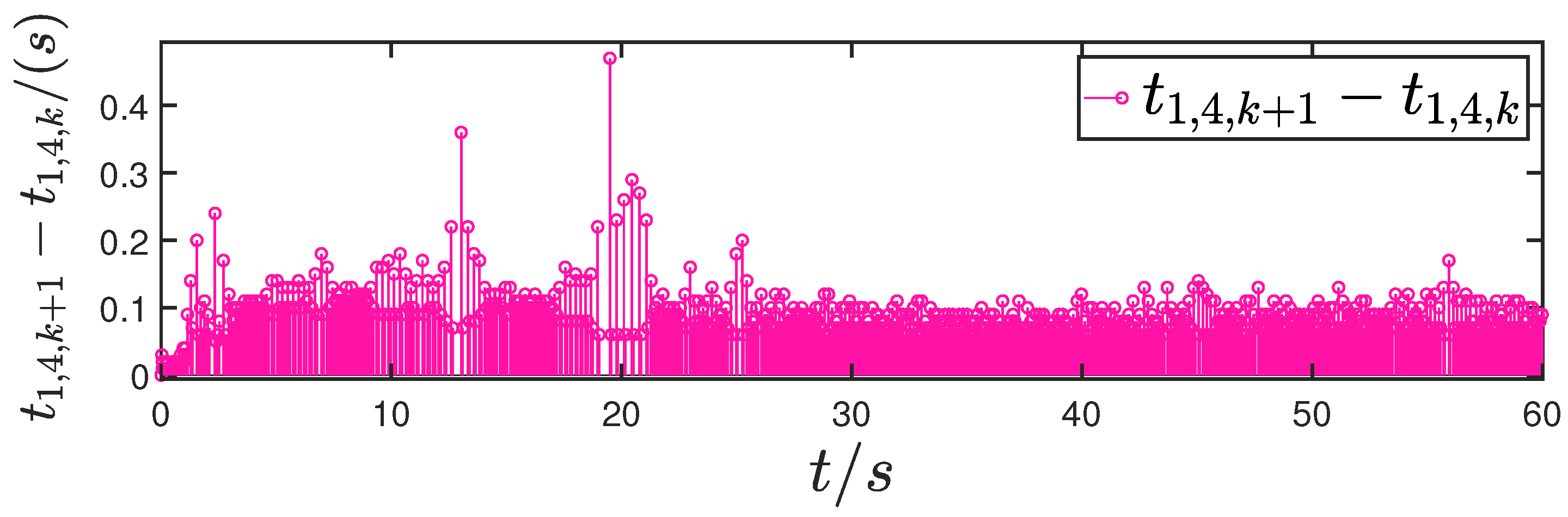
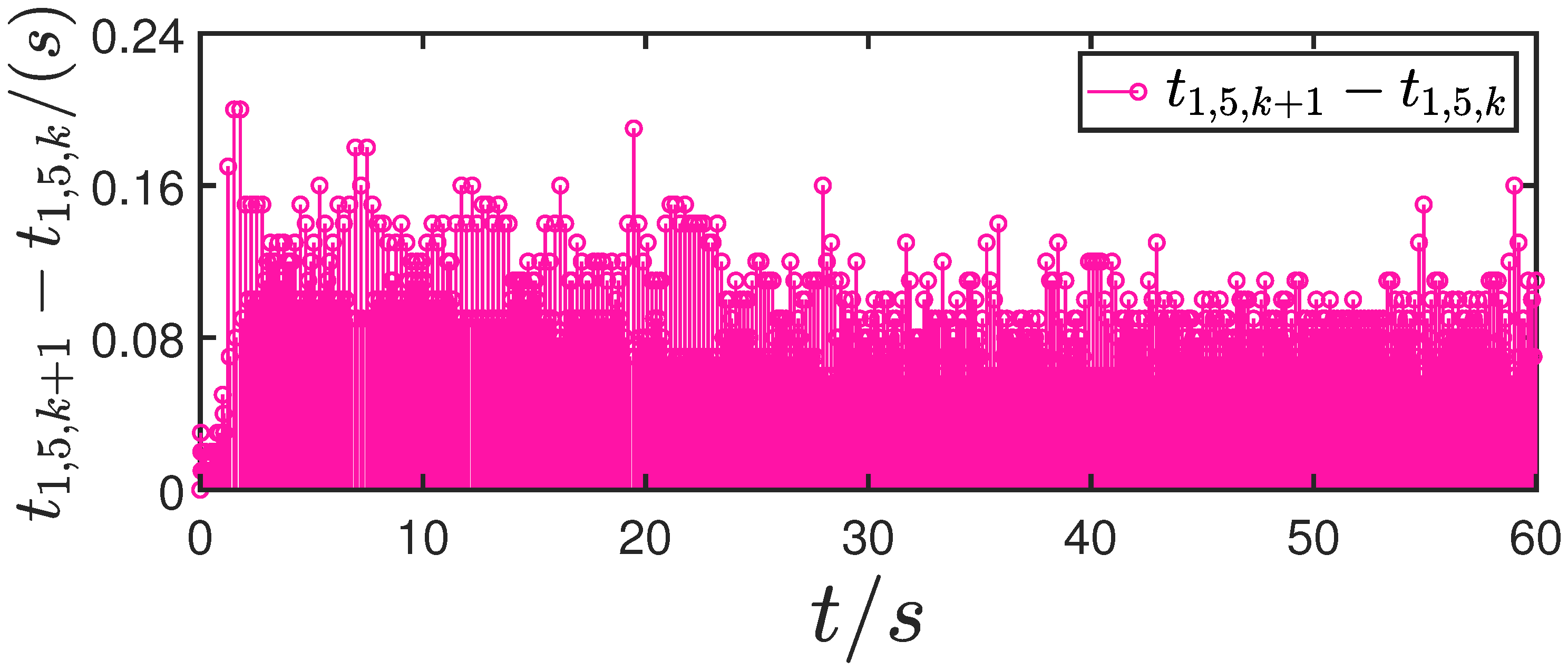
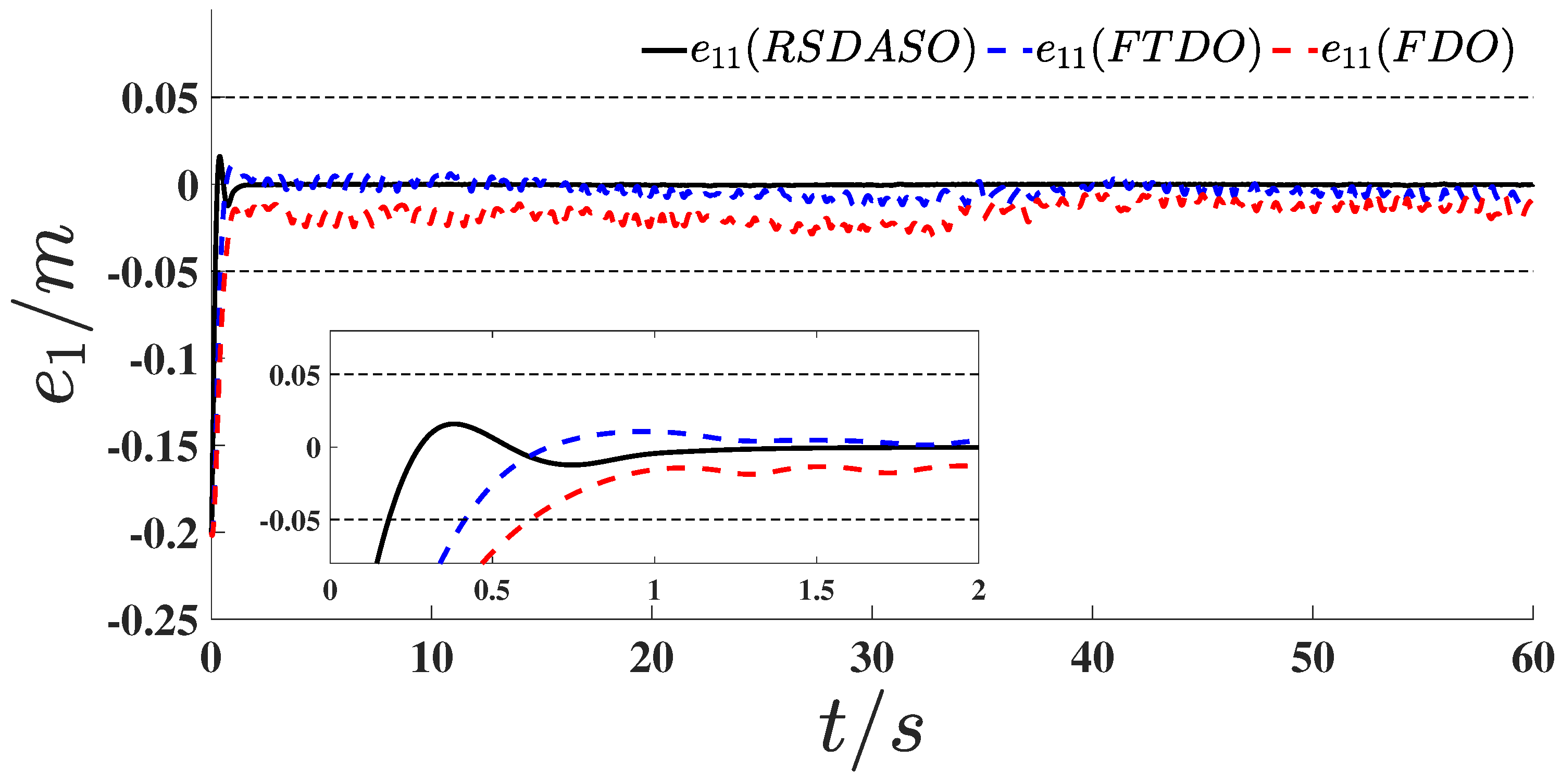
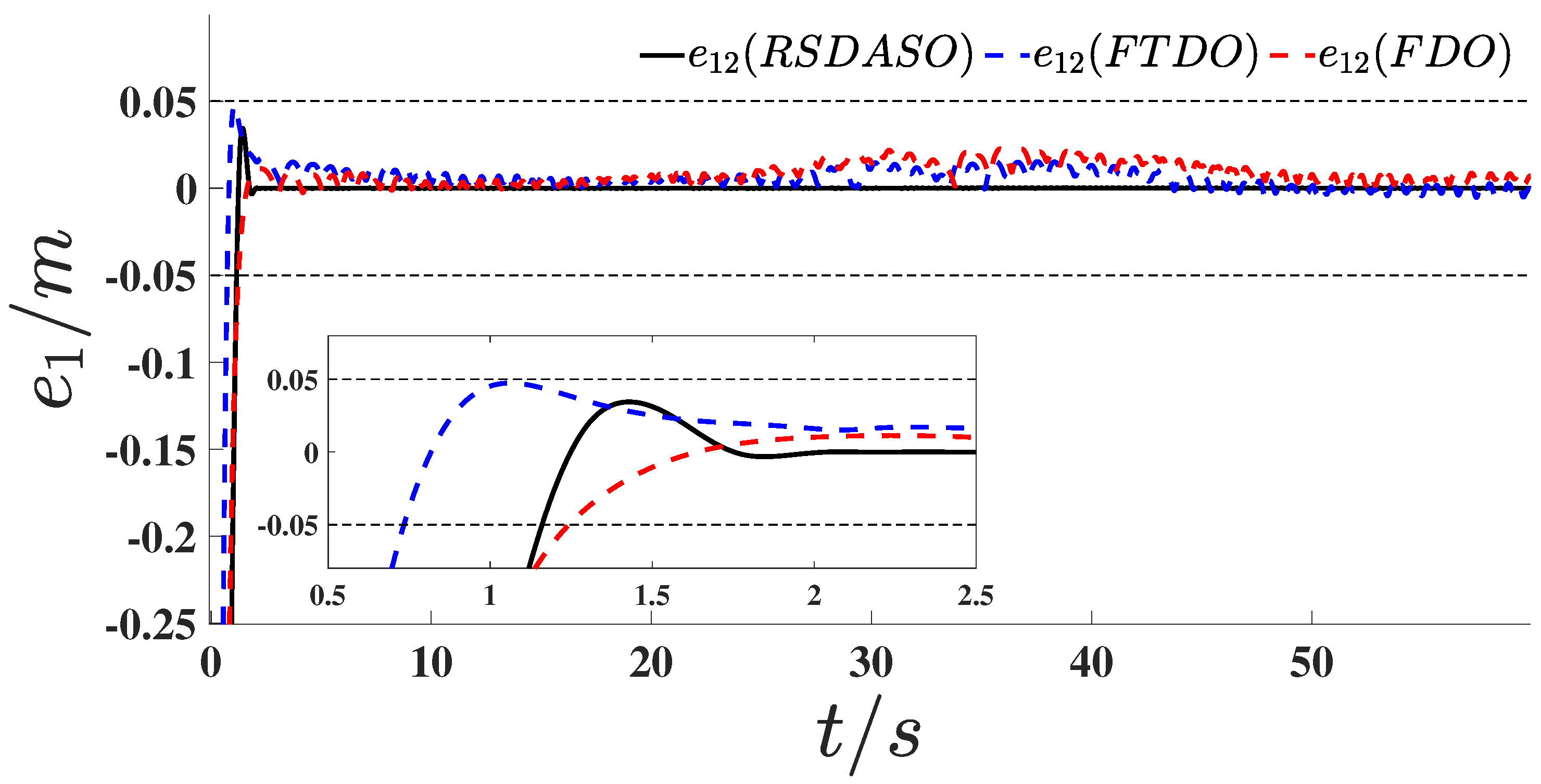
Item	Actuator 1	Actuator 2	Actuator 3	Actuator 4	Actuator 5
TTM	30,000	30,000	30,000	30,000	30,000
Percentage reduction	/	/	/	/	/
Follower 1	5701	7393	5292	6811	7066
Percentage reduction	80%	75%	82%	77%	76%
Follower 2	8122	7547	5287	6443	5411
Percentage reduction	73%	74%	82%	78%	82%

. Compared to time-triggered schemes (TTM), the ETM reduces trigger instances by 75–82% across actuators (e.g., 5701 triggers for Actuator 1 versus TTM’s fixed-interval triggering), significantly alleviating computational and communication loads. Crucially, Figure 25, Figure 26, Figure 27, Figure 28 and Figure 29 verify the absence of Zeno behavior. These results collectively substantiate the control scheme’s effectiveness in achieving precise tracking, robust observation, and resource-efficient implementation.

In order to demonstrate the superiority of the present method (RSDASO), it was compared with the Fixed Time Disturbance Observer (FTDO) [31] and Finite Time Disturbance Observer (FDO). The simulation results are shown in Figure 31, Figure 32 and Figure 33. The figures demonstrate a comparison of the AUV position and attitude tracking errors under different control strategies. As shown in Figure 31, the proposed RSDASO method (black curve) exhibited significant advantages over the two control strategies, FTDO (blue dashed line) and FDO (red dashed line), in terms of the position tracking error $e_{11}$. During the initial stage (near $t=0$), although the errors of all three methods showed notable changes, the RSDASO method demonstrated a faster error convergence rate, rapidly approaching zero error. From $t=0$ to 1 s, its error fluctuated with a smaller amplitude and stabilized quickly. After the system stabilized, the error curve of the RSDASO method remained within a narrow fluctuation range, maintaining a level close to zero, which highlights its high positional tracking stability. In contrast, while the error curves of FTDO and FDO also tended toward zero, their fluctuation amplitudes were larger than those of the RSDASO method, particularly for the FDO method (red dashed line), which exhibited more pronounced error fluctuations throughout the entire time period. Further analysis of the position tracking errors in the other two degrees of freedom, as shown in Figure 32 and Figure 33, revealed that the RSDASO method achieved faster error convergence in the initial stage, exhibited smoother error curves with significantly smaller fluctuation amplitudes during the intermediate stage, and stabilized errors at a level closer to zero with the smallest fluctuation range in the later stage. Collectively, the RSDASO method demonstrated superior performance in convergence speed, stability, and error control accuracy across all degrees of freedom, underscoring its excellent efficacy in trajectory tracking control.

5. Conclusions

This study effectively addressed the challenging issues associated with multi-AUV formation systems, including full-state constraints, unmeasurable states, model uncertainties, limited communication resources, and unknown time-varying external disturbances. It presented a fixed-time accurate formation control method for multi-AUVs based on a switching threshold event-triggering mechanism. The theoretical demonstration showed that (1) all system errors (including observer estimation errors) converged within a fixed time interval and (2) the communication burden was significantly reduced while rigorously avoiding Zeno behavior. The efficacy of the proposed control strategy was conclusively validated through comprehensive simulation examples. Future work will focus on addressing time-delay issues in multi-AUV formation control systems, which represent a critical challenge in practical underwater applications.