Numerical Solution of Nonlinear Quadratic Integral Equation of Hammerstein Type Based on Fixed-Point Scheme
Abstract
1. Introduction
2. Theoretical Background
- (i)
- The family ker is nonempty and ker ;
- (ii)
- ;
- (iii)
- ;
- (iv)
- for ;
- (v)
- If is a sequence of sets from such that , and if , then the intersection is nonempty;
- (vi)
- .
- (vii)
- for ;
- (viii)
- .
- (1)
- Function is continuous and as .
- (2)
- Function is continuous and the function belongs to the space .
- (3)
- There exists a continuous function such thatand .
- (4)
- The function is uniformly continuous on every rectangle of the form ; moreover, there exists a continuous function and a continuous and nondecreasing function such thatfor and
- (5)
- The function is continuous and there exist continuous functions such that functions and are integrable over and the following inequalityis satisfied for . Moreover, we assume that and function is bounded on the interval
- (6)
- The constants are defined as follows:;;;.These constants are finite.
- (7)
- The inequality has a positive solution .
3. Sinc Method
4. Convergence Analysis
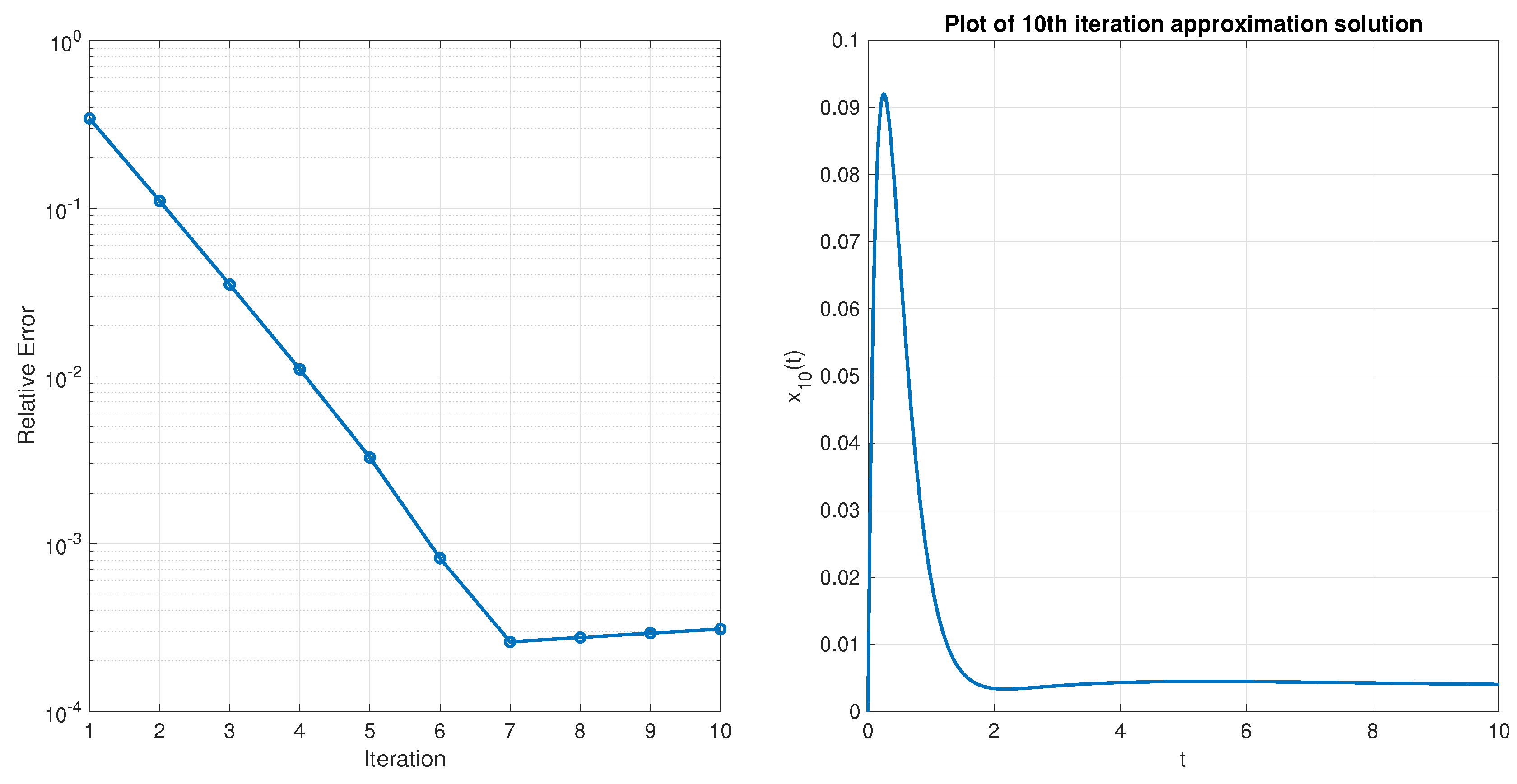
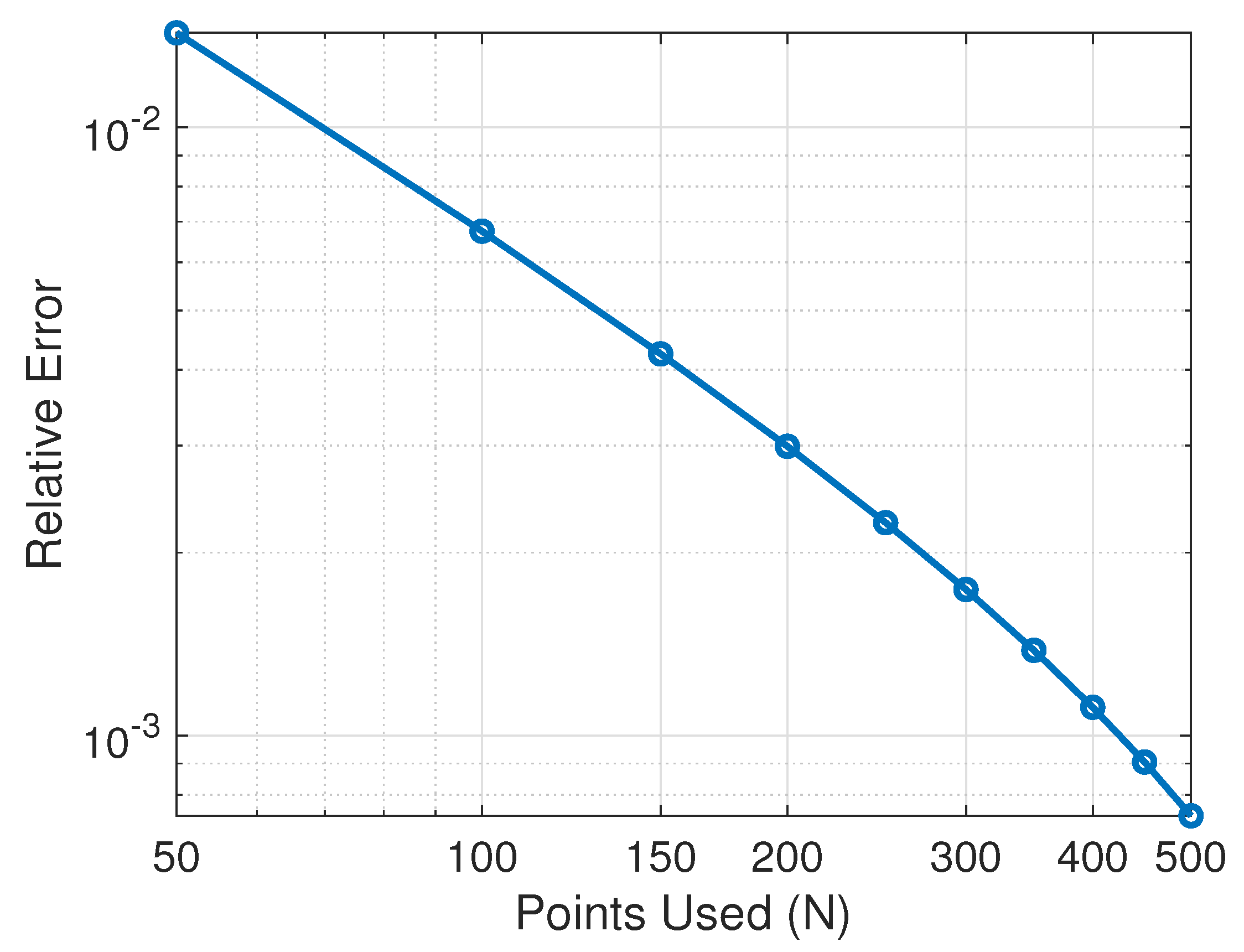
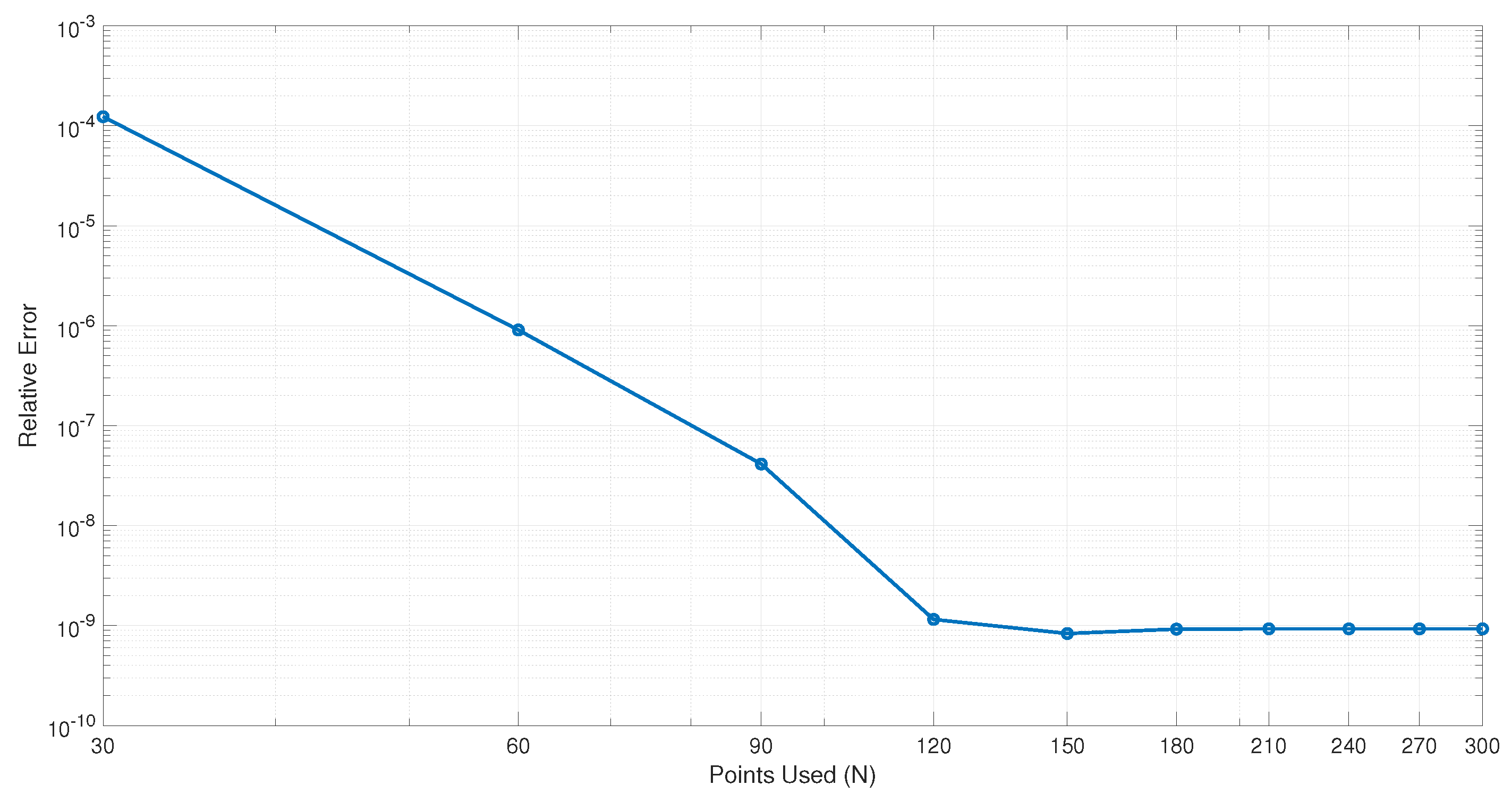
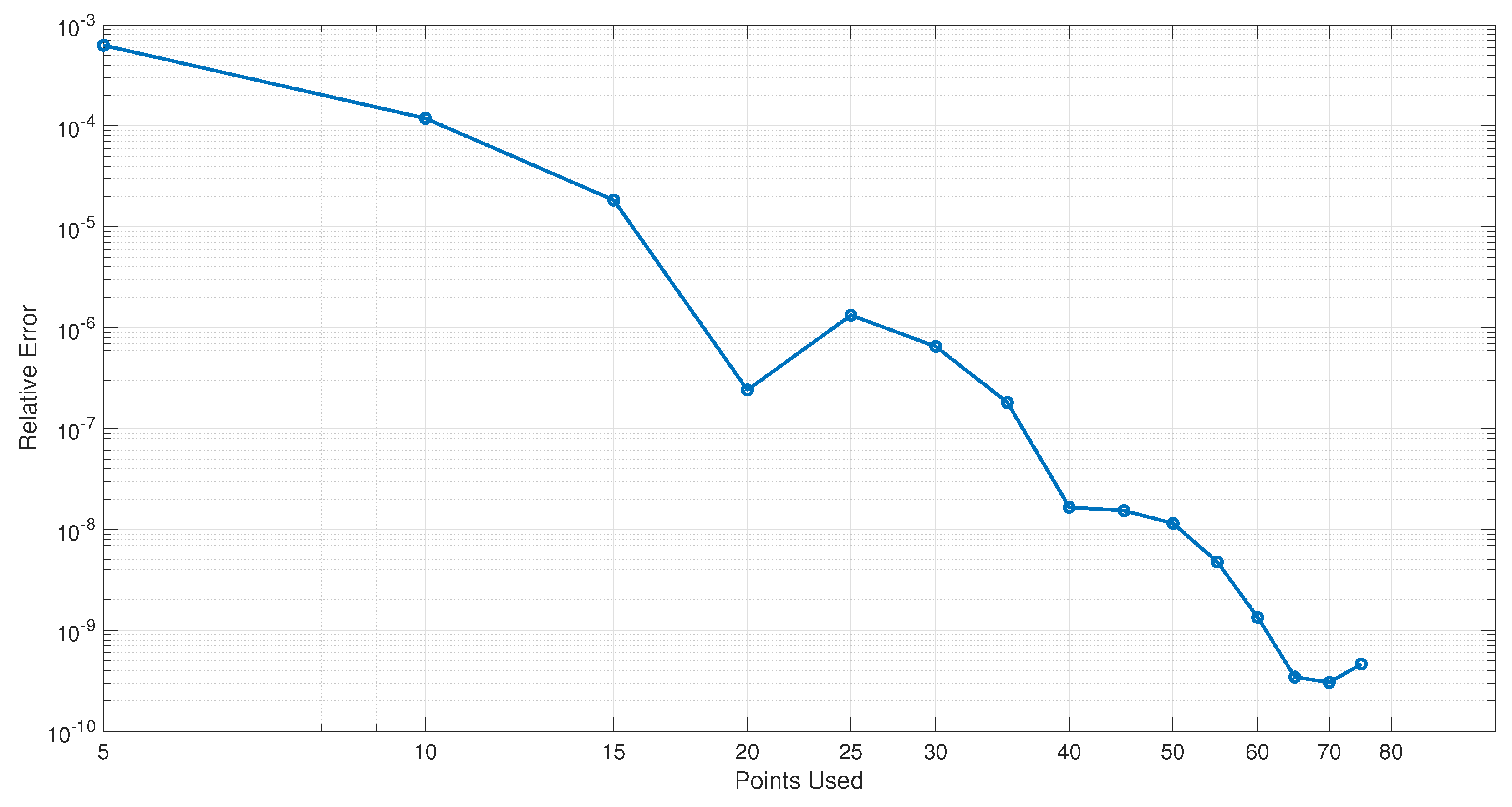
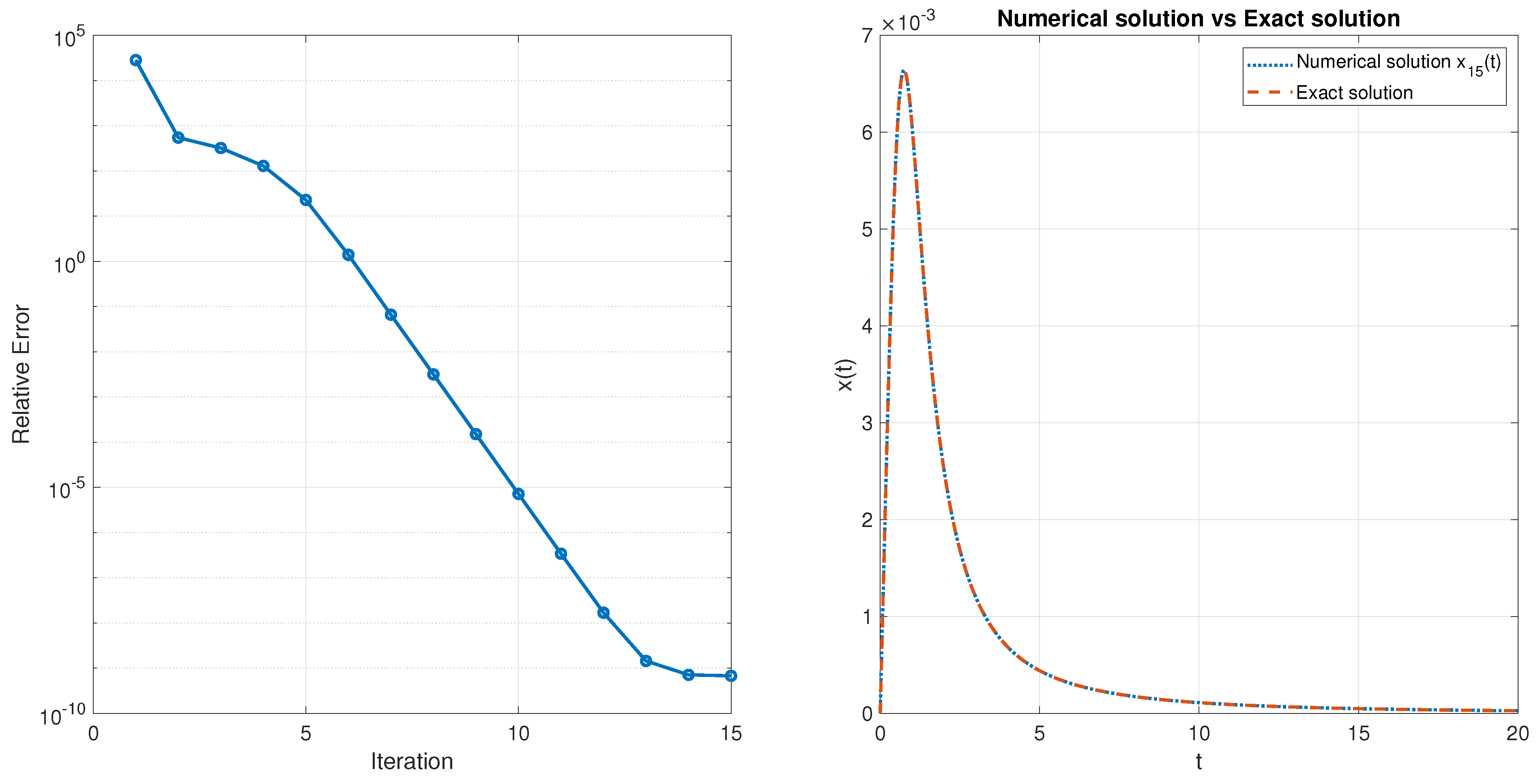
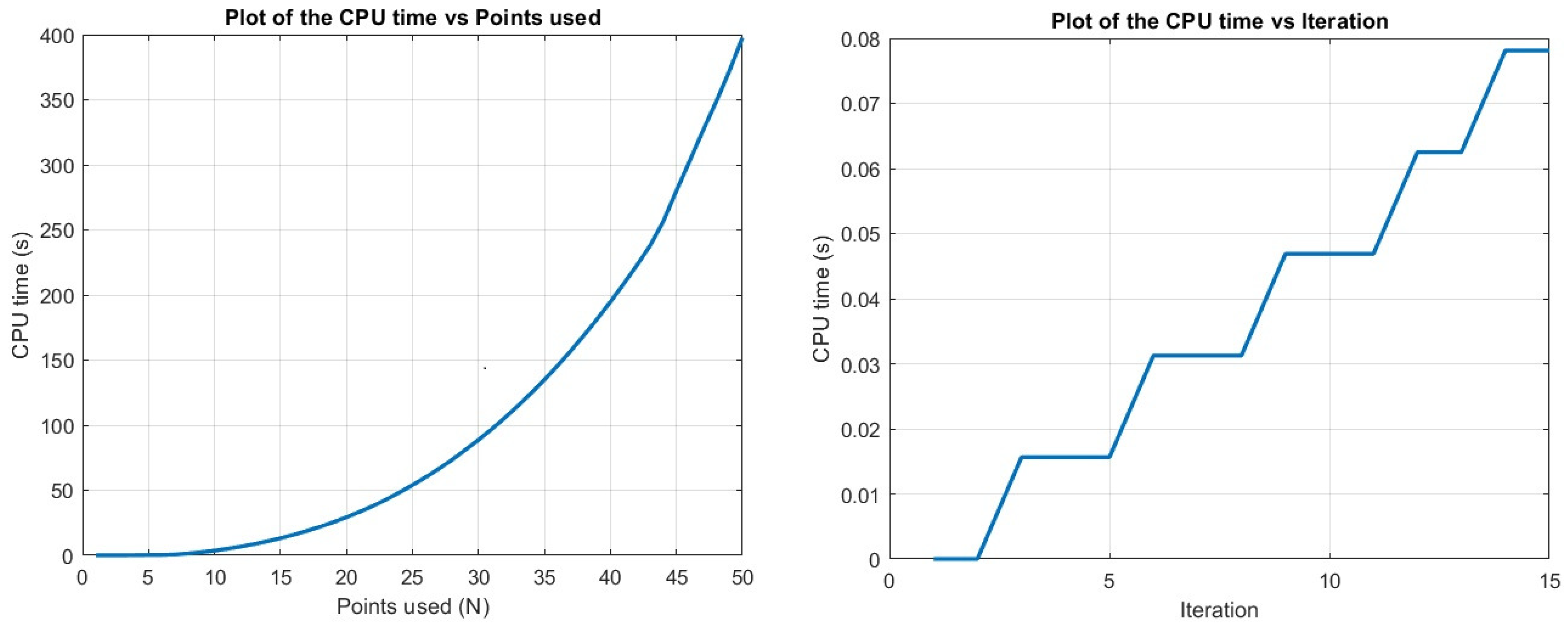
5. Numerical Examples
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Anselone, P.; Lee, J. Nonlinear integral equations on the half line. J. Integral Equations Appl. 1992, 4, 1–14. [Google Scholar]
- Deimling, K. Nonlinear Functional Analysis; Springer: Berlin/Heidelberg, Germany, 1985. [Google Scholar]
- Graham, I.G.; Mendes, W.R. Nystrom-product integration for Wiener-Hopf equations with applications to radiative transfer. IMA J. Numer. Anal. 1989, 9, 261–284. [Google Scholar] [CrossRef]
- Busbridge, W. The Mathematics of Radiative Transfer; Cambridge University Press: Cambridge, UK, 1960. [Google Scholar]
- Cahlon, B.; Eskin, M. Existence theorems for an integral equation of the chandrasekhar h-equation with perturbation. J. Math. Anal. Appl. 1981, 83, 159–171. [Google Scholar] [CrossRef]
- Chandrasekhar, S. Radiative Transfer; Oxford University Press: London, UK, 1950. [Google Scholar]
- Hu, S.; Khavanin, M.; Zhuang, W.A.N. Integral equations arising in the kinetic theory of gases. Appl. Anal. 1989, 34, 261–266. [Google Scholar] [CrossRef]
- Kelley, C.T. Approximation of solutions of some quadratic integral equations in transport theory. J. Integral Equ. 1982, 4, 221–237. [Google Scholar]
- Delves, L.M.; Mohamed, J.L. Computational Method for Integral Equations; Cambridge University Press: Cambridge, UK, 1985. [Google Scholar]
- Atkinson, K. The Numerical Solution of Integral Equations of Second Kind; Cambridge University Press: Cambridge, UK, 1997. [Google Scholar]
- Atkinson, K.E. The numerical solution of a non-linear boundary integral equation on smooth surfaces. IMA J. Numer. Anal. 1994, 14, 461–483. [Google Scholar] [CrossRef]
- Atkinson, K.E.; Chandler, G. Boundary integral equation methods for solving laplace’s equation with nonlinear boundary conditions: The smooth boundary case. Math. Comput. 1990, 55, 451–472. [Google Scholar]
- Finn, G.; Jefferies, J. Studies in spectral line formation: I. formulation and simple applications. J. Quant. Spectrosc. Radiat. Transf. 1968, 8, 1675–1703. [Google Scholar] [CrossRef]
- Nahid, N.; Das, P.; Nelakanti, G. Projection and multi projection methods for nonlinear integral equations on the half-line. J. Comput. Appl. Math. 2019, 359, 119–144. [Google Scholar] [CrossRef]
- Assari, P.; Dehghan, M. A meshless discrete galerkin method based on the free shape parameter radial basis functions for solving Hammerstein integral equations. Numer. Math. Theory Methods Appl. 2018, 11, 540–568. [Google Scholar]
- Kumar, S.; Sloan, I.H. A new collocation-type method for Hammerstein integral equations. Math. Comput. 1987, 48, 585–593. [Google Scholar] [CrossRef]
- Wang, X.; Lin, W. Id-wavelets method for Hammerstein integral equations. J. Comput. Math. 1998, 16, 499–508. [Google Scholar]
- Kaneko, H.; Noren, R.D.; Novaprateep, B. Wavelet applications to the Petrov-Galerkin method for Hammerstein equations. Appl. Numer. Math. 2003, 45, 255–273. [Google Scholar] [CrossRef]
- Allouch, C.; Sbibih, D.; Tahrichi, M. Superconvergent Nyström and degenerate kernel methods for hammerstein integral equations. J. Comput. Appl. Math. 2014, 258, 30–41. [Google Scholar] [CrossRef]
- Banas, J.; Martin, J.; Sadarangani, K. On solutions of a quadratic integral equation of Hammerstein type. Math. Comput. Model. 2006, 43, 97–104. [Google Scholar] [CrossRef]
- Banas, J.; Goebel, K. Measures of Noncompactness in Banach Spaces in Lecture Notes in Pure an Applied Mathematics; Marcel Dekker: New York, NY, USA, 1980. [Google Scholar]
- Agarwal, R.; Meehan, M.; O’Regan, D. Fixed Point Theory and Applications; Cambridge University Press: Cambridge, UK, 2004. [Google Scholar]
- Okayama, T. Error estimates with explicit constants for sinc quadrature and sinc indefinite integration over infinite intervals. Reliab. Comput. 2013, 19, 45–65. [Google Scholar]
- Stenger, F. Numerical Method Base on Sinc and Analytic Function; Springer: New York, NY, USA, 1993. [Google Scholar]
- Lund, J.; Bowers, K. Sinc Method for Quaderature and Diffrential Equation; SIAM: Philadelphia, PA, USA, 1992. [Google Scholar]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mollapourasl, R.; Siebor, J. Numerical Solution of Nonlinear Quadratic Integral Equation of Hammerstein Type Based on Fixed-Point Scheme. Mathematics 2025, 13, 1413. https://doi.org/10.3390/math13091413
Mollapourasl R, Siebor J. Numerical Solution of Nonlinear Quadratic Integral Equation of Hammerstein Type Based on Fixed-Point Scheme. Mathematics. 2025; 13(9):1413. https://doi.org/10.3390/math13091413
Chicago/Turabian StyleMollapourasl, Reza, and Joseph Siebor. 2025. "Numerical Solution of Nonlinear Quadratic Integral Equation of Hammerstein Type Based on Fixed-Point Scheme" Mathematics 13, no. 9: 1413. https://doi.org/10.3390/math13091413
APA StyleMollapourasl, R., & Siebor, J. (2025). Numerical Solution of Nonlinear Quadratic Integral Equation of Hammerstein Type Based on Fixed-Point Scheme. Mathematics, 13(9), 1413. https://doi.org/10.3390/math13091413





