A Characterization of Three-Dimensional Convex Polytopes with Five Pairwise Antipodal Vertices
Abstract
1. Introduction
2. The Convex Hull of the Union of a Simplex and a Singleton
- 1.
- ,
- 2.
- .
- 1.
- The points and are antipodal in .
- 2.
- .
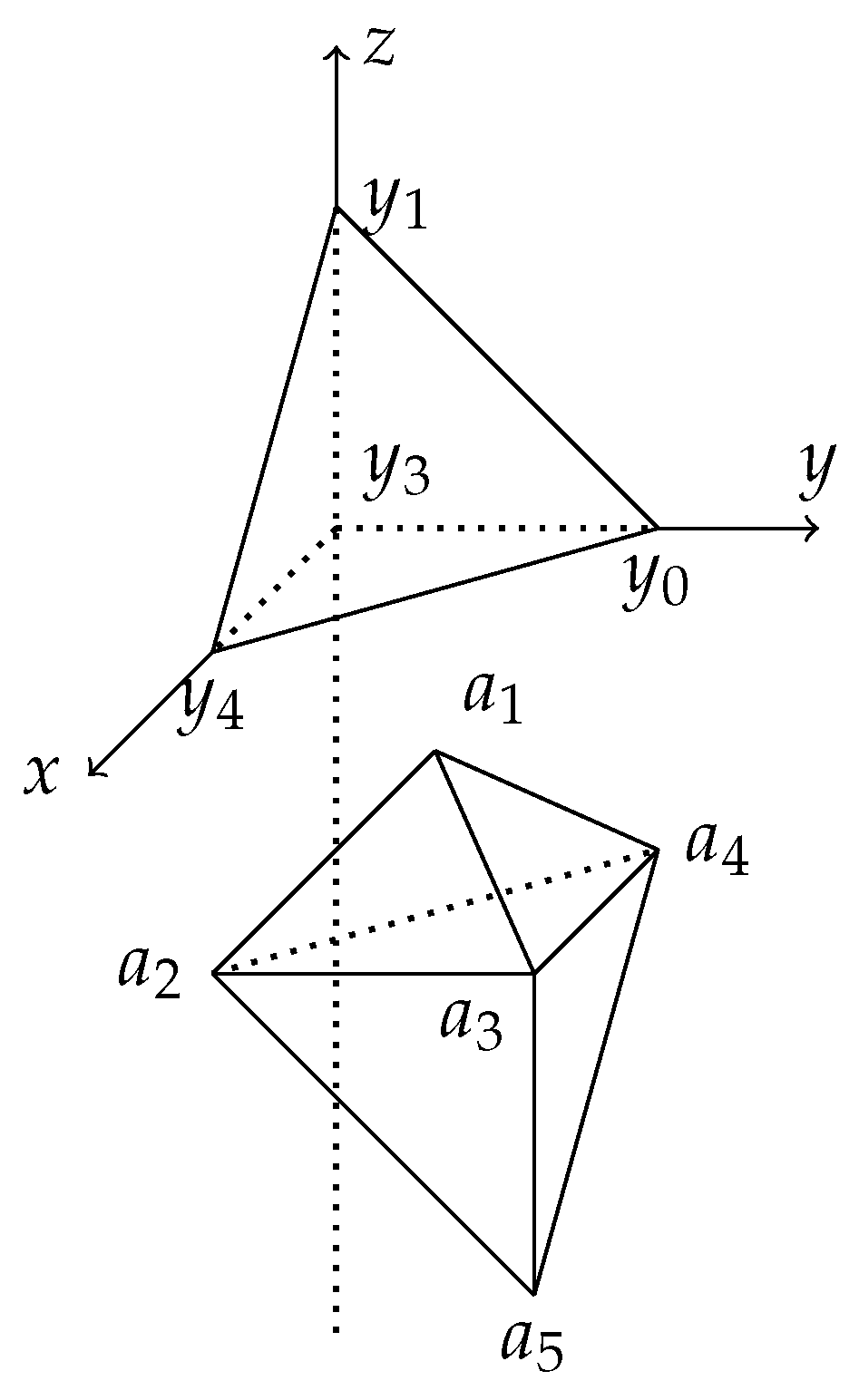
3. Main Results
- 1.
- K is the convex hull of the union of a singleton and a parallelogram.
- 2.
- K is affinely equivalent to a member of .
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Danzer, L.W.; Grünbaum, B. Über zwei Probleme bezüglich konvexer Körper von P. Erdos und von V. L. Klee. Math. Z. 1962, 79, 95–99. [Google Scholar] [CrossRef]
- Martini, H.; Soltan, V. Antipodality properties of finite sets in Euclidean space. Discret. Math. 2005, 290, 221–228. [Google Scholar] [CrossRef]
- Makai, E., Jr.; Martini, H. On the number of antipodal or strictly antipodal pairs of points in finite subsets of . In Applied Geometry and Discrete Mathematics, The “Victor Klee Festschrift”. DIMACS Ser. Discr. Math. Theor. Comp. Sci. 1991, 4, 457–470. [Google Scholar]
- Makai, E., Jr.; Martini, H. On the number of antipodal or strictly antipodal pairs of points in finite subsets of , II. Period. Math. Hung. 1993, 27, 185–198. [Google Scholar] [CrossRef]
- Makai, E., Jr.; Martini, H.; Nguyên, M.H.; Soltan, V.; Talata, I. On the number of antipodal or strictly antipodal pairs of points in finite subsets of , III. arXiv 2021, arXiv:2103.13182. [Google Scholar]
- Levi, F.W. Überdeckung eines Eibereiches durch Parallelverschiebung seines offenen Kerns. Arch. Math. 1955, 6, 369–370. [Google Scholar] [CrossRef]
- Bezdek, K. Classical Topics in Discrete Geometry, CMS Books in Mathematics/Ouvrages de Mathématiques de la SMC; Springer: New York, NY, USA, 2010. [Google Scholar]
- Boltyanski, V.; Martini, H.; Soltan, P.S. Excursions into Combinatorial Geometry; Springer: Berlin/Heidelberg, Germany, 1997. [Google Scholar]
- Bezdek, K. Hadwiger’s covering conjecture and its relatives. Am. Math. Mon. 1992, 99, 954–956. [Google Scholar] [CrossRef]
- Bezdek, K.; Khan, M.A. The geometry of homothetic covering and illumination. In Discrete Geometry and Symmetry; Springer Proceedings in Mathematics & Statistics Series; Springer: Cham, Switzerland, 2018; Volume 234, pp. 1–30. [Google Scholar]
- Boltyanski, V.; Gohberg, I.Z. Stories about covering and illuminating of convex bodies. Nieuw Arch. Wisk. 1995, 13, 1–26. [Google Scholar]
- Martini, H.; Soltan, V. Combinatorial problems on the illumination of convex bodies. Aequationes Math. 1999, 57, 121–152. [Google Scholar] [CrossRef]
- Wu, S.; He, C. Covering functionals of convex polytopes. Linear Algebra Appl. 2019, 577, 53–68. [Google Scholar] [CrossRef]
- Grünbaum, B. Convex Polytopes; Springer: New York, NY, USA, 2003. [Google Scholar]
- Wu, S.; Zhang, K.; He, C. Homothetic covering of convex hulls of compact convex sets. Contrib. Discrete Math. 2022, 17, 31–37. [Google Scholar] [CrossRef]
- Soltan, V. Affine diameters of convex-bodies—A survey. Expo. Math. 2005, 23, 47–63. [Google Scholar] [CrossRef]
- Gao, S.; Martini, H.; Wu, S.; Zhang, L. New covering and illumination results for a class of polytopes. Arch. Math. 2024, 122, 599–607. [Google Scholar] [CrossRef]
- Hiriart-Urruty, J.B.; Lemaréchal, C. Fundamentals of Convex Analysis; Springer: Berlin/Heidelberg, Germany, 2001. [Google Scholar]
- Soltan, V. Lectures on Convex Sets; World Scientific Publishing Co. Pte. Ltd.: Hackensack, NJ, USA, 2015. [Google Scholar]
- Webster, R. Convexity; Oxford Univ. Press: New York, NY, USA, 1994. [Google Scholar]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guo, R.; He, C.; Zhang, L.; Wu, S. A Characterization of Three-Dimensional Convex Polytopes with Five Pairwise Antipodal Vertices. Mathematics 2025, 13, 1412. https://doi.org/10.3390/math13091412
Guo R, He C, Zhang L, Wu S. A Characterization of Three-Dimensional Convex Polytopes with Five Pairwise Antipodal Vertices. Mathematics. 2025; 13(9):1412. https://doi.org/10.3390/math13091412
Chicago/Turabian StyleGuo, Rong, Chan He, Longzhen Zhang, and Senlin Wu. 2025. "A Characterization of Three-Dimensional Convex Polytopes with Five Pairwise Antipodal Vertices" Mathematics 13, no. 9: 1412. https://doi.org/10.3390/math13091412
APA StyleGuo, R., He, C., Zhang, L., & Wu, S. (2025). A Characterization of Three-Dimensional Convex Polytopes with Five Pairwise Antipodal Vertices. Mathematics, 13(9), 1412. https://doi.org/10.3390/math13091412






