Calculus of Long Rectangular Plates Embedded in Long Borders with Uniform Vertical Load on a Line Parallel to the Long Borders
Abstract
1. Introduction
2. Calculus Premises Using the Transfer Matrix Method for Long Rectangular Plates
2.1. The State Vectors and the Transfer Matrix for a Long Rectangular Plate
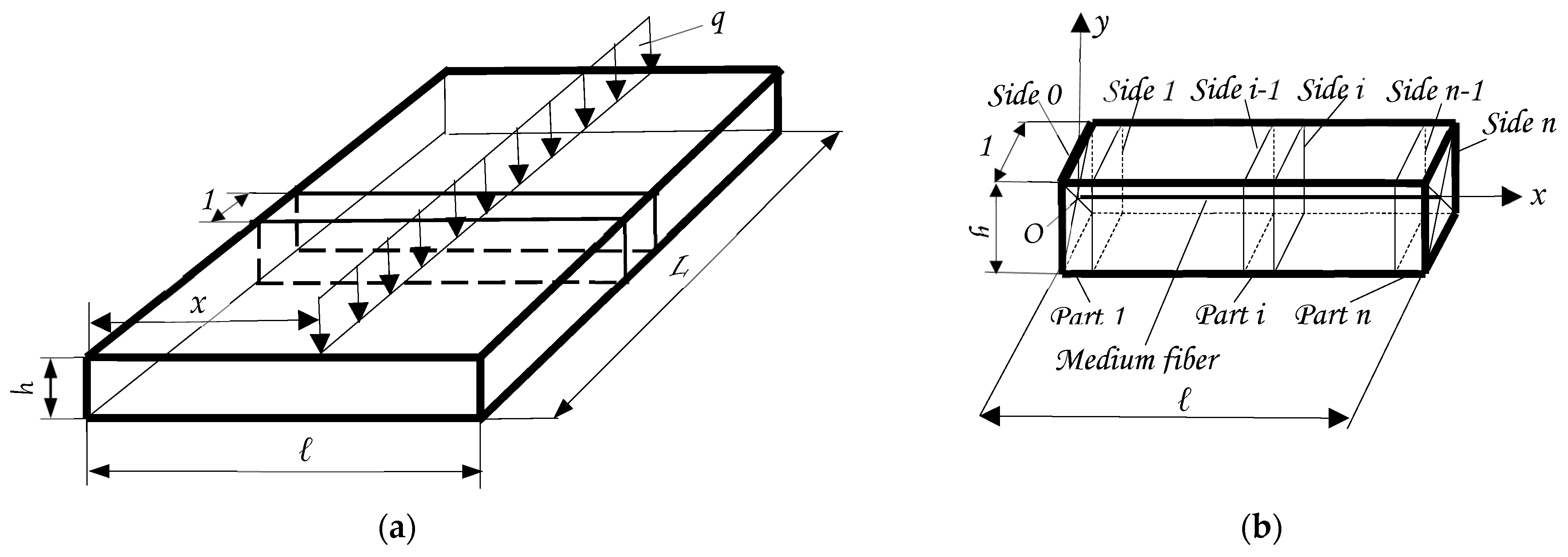
2.1.1. State Vectors
- -
- A state vector is associated with each side. For some side x, it is associated a state vector {V(x)} = {V}x with four elements (a(x), r(x), M(x), and C(x)). The state vector and its elements always have the index of the side it is on, (Figure 2b), as in the following:
- -
- {V(x)} = {V}x is state vector corresponding at the side x;
- -
- a(x) = ax is the arrow;
- -
- r(x) = rx is the rotation of the average fiber in the x section;
- -
- M(x) = Mx is the bending moment;
- -
- C(x) = Cx is the cutting force at the x-axis point.
2.1.2. Transfer Matrix
- -
- {Ve}1 is the state vector corresponding to the external forces acting on part 1.
2.2. Approach for Analytical Calculus of a Long Rectangular Plate
2.2.1. Study Hypotheses
- -
- The plate is subjected to an axial force along the long sides, with P being the side per unit (as in Figure 2b);
- -
- The charge density q(x) is expressed per unit of length;
- -
- The bending moment due to a single external load is denoted by m(x).
2.2.2. The Arrow Calculus for the Unit Beam
- E is the Young’s modulus;
- h is the thickness of the plate;
- ν is the Poisson’s coefficient.
2.3. Transfer Matrix for a Long Rectangular Plate
3. Applications and Results for the Calculus of a Long Rectangular Plate Embedded in the Two Long Borders Charged with Vertical Uniform Loads That Act on a Line Parallel Along the Long Borders
3.1. Unit Width Beam Embedded in the Two Edges Charged with a Concentrated Load (Figure 3a–d)

3.1.1. Unit Width Beam Embedded in the Two Edges Charged with a Concentrated Load (-P), Which Acts in a Certain Section x0 (As in Figure 3a)
3.1.2. Unit Width Beam Embedded in the Two Edges Charged with a Concentrated Load (-P), Which Acts in a Section x0 = l/2, (As in Figure 3b)
3.1.3. Unit Width Beam Embedded at the Two Edges Charged with a Concentrated Load P, Which Acts in a Certain Section x0 (As in Figure 3c)
3.1.4. Unit Width Beam Embedded at the Two Edges Charged with a Concentrated Load P, Which Acts in the Section x0 = l/2, (Figure 3d)
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Gery, P.-M.; Calgaro, J.-A. Les Matrices-Transfert Dans Le Calcul Des Structures; Éditions Eyrolles: Paris, France, 1973. [Google Scholar]
- Suciu, M.; Tripa, M.-S. Strength of Materials; UT Press: Cluj-Napoca, Romania, 2024. [Google Scholar]
- Warren, C.Y. ROARK’S Formulas for Stress & Strain, 6th ed.; McGraw Hill Book Company: New York, NY, USA, 1989. [Google Scholar]
- Codrea, L.; Tripa, M.-S.; Opruţa, D.; Gyorbiro, R.; Suciu, M. Transfer-Matrix Method for Calculus of Long Cylinder Tube with Industrial Applications. Mathematics 2023, 11, 3756. [Google Scholar] [CrossRef]
- Suciu, M. About an approach by Transfer-Matrix Method (TMM) for mandible body bone calculus. Mathematics 2023, 11, 450. [Google Scholar] [CrossRef]
- Cojocariu-Oltean, O.; Tripa, M.-S.; Baraian, I.; Rotaru, D.-I.; Suciu, M. About Calculus Through the Transfer Matrix Method of a Beam with Intermediate Support with Applications in Dental Restorations. Mathematics 2024, 12, 3861. [Google Scholar] [CrossRef]
- Rezaiee-Pajand, M.; Arabi, E.; Masoodi, A.R. A triangular shell element for geometrically nonlinear analysis. Acta Mech. 2018, 229, 323–342. [Google Scholar] [CrossRef]
- Suciu, M. Calculus of Long Rectangle Plate Articulated on Both Long Sides Charged with a Linear Load Uniformly Distributed on a Line Parallel to the Long Borders through the Transfer-Matrix Method. EMSJ 2024, 8, 157–167. [Google Scholar] [CrossRef]
- Viebahn, N.; Pimenta, P.M.; Schröder, J. A simple triangular finite element for nonlinear thin shells: Statics, dynamics and anisotropy. Comput. Mech. 2017, 59, 281–297. [Google Scholar] [CrossRef]
- Sanchez, M.L.; Pimenta, P.M.; Ibrahimbegovic, A. A simple geometrically exact finite element for thin shells—Part 1: Statics. Comput. Mech. 2023, 72, 1119–1139. [Google Scholar] [CrossRef]
- Costa, J.C.; Pimenta, P.M.; Wriggers, P. Meshless analysis of shear deformable shells: Boundary and interface constraints. Comput. Mech. 2016, 57, 679–700. [Google Scholar] [CrossRef]
- Cui, X.Y.; Wang, G.; Li, G.Y. A continuity re-relaxed thin shell formulation for static and dynamic analyses of linear problems. Arch. Appl. Mech. 2015, 85, 1847–1867. [Google Scholar] [CrossRef]
- Yessenbayeva, G.A.; Yesbayeva, D.N.; Syzdykova, N.K. On the finite element method for calculation of rectangular plates. Bul. Karaganda Univ. Math. 2019, 95, 128–135. [Google Scholar] [CrossRef]
- Yessenbayeva, G.A.; Yesbayeva, D.N.; Makazhanova, T.K. On the calculation of the rectangular finite element of the plate. Bul. Karaganda Univ. Mech. 2018, 90, 150–156. [Google Scholar] [CrossRef]
- Yuhong, B. Bending of Rectangular Plate with Each Edges Arbitrary Point Supported under a Concentrated Load. Appl. Math. Mech. 2000, 21, 591–596. [Google Scholar] [CrossRef]
- Ohga, M.; Shigematsu, T.; Kohigashi, S. Analysis of folded plate structures by a combined boundary element-transfer matrix method. Comput. Struct. 1991, 41, 739–744. [Google Scholar] [CrossRef]
- Ohga, M.; Shigematsu, T.; Hara, T. A Finite Element-Transfer Matrix Method For Dynamic Analysis Of Frame Structures. J. Sound Vib. 1993, 167, 401–411. [Google Scholar] [CrossRef]
- Papanikolaou, V.K.; Doudoumis, I.N. Elastic analysis and application tables of rectangular plates with unilateral contact support conditions. Comput. Struct. 2001, 79, 2559–2578. [Google Scholar] [CrossRef]
- Tuozzo, D.M.; Silva, O.M.; Kulakauskas, L.V.Q.; Vargas, J.G.; Lenzi, A. Time-harmonic analysis of acoustic pulsation in gas pipeline systems using the Finite Element Transfer Matrix Method: Theoretical aspects. Mech. Syst. Signal Process. 2023, 186, 109824. [Google Scholar] [CrossRef]
- Vijayasree, N.K.; Munjal, M.L. On an Integrated Transfer Matrix method for multiply connected mufflers. J. Sound Vib. 2012, 331, 1926–1938. [Google Scholar] [CrossRef]
- Delyavs’kyi, M.V.; Zdolbits’ka, N.V.; Onyshko, L.I.; Zdolbits’kyi, A.P. Determination of the stress-strain state in thin orthotropic plates on Winkler’s elastic foundations. Mater. Sci. 2015, 50, 782–791. [Google Scholar] [CrossRef]
- Lin, H.-C.; Lu, S.-C.; Huang, H.-H. Evaluation of a Hybrid Underwater Sound-Absorbing Metastructure by Using the Transfer Matrix Method. Materials 2023, 16, 1718. [Google Scholar] [CrossRef]
- Cretu, N.; Pop, M.-I.; Andia Prado, H.S. Some Theoretical and Experimental Extensions Based on the Properties of the Intrinsic Transfer Matrix. Materials 2022, 15, 519. [Google Scholar] [CrossRef]
- Verdière, K.; Panneton, R.; Elkoun, S.; Dupon, T.; Leclaire, P. Transfer matrix method applied to the parallel assembly of sound absorbing materials. J. Acoust. Soc. Am. 2013, 134, 4648–4658. [Google Scholar] [CrossRef] [PubMed]
- Rui, X.T.; Zhang, J.S.; Wang, X.; Rong, B.; He, B.; Jin, Z. Multibody system transfer matrix method: The past, the present, and the future. Int. J. Mech. Syst. Dyn. 2022, 2, 3–26. [Google Scholar] [CrossRef]
- Roi, X.T.; Wang, X.; Zhou, Q.B.; Zhang, J.S. Transfer matrix method for multibody systems (Rui method) and its applications. China Technol. Sci. 2019, 62, 712–720. [Google Scholar] [CrossRef]
- Gerges, S.N.Y.; Jordan, R.; Thieme, F.A.; Bento Coelho, J.L.; Arenas, J.P. Muffler Modeling by Transfer Matrix Method and Experimental Verification. J. Braz. Soc. Mech. Sci. Eng. 2005, 27, 132–140. [Google Scholar] [CrossRef]
- Revenko, V. Development of two-dimensional theory of thick plates bending on the basis of general solution of Lamé equations. Sci. J. Ternopil Natl. Tech. Univ. 2018, 1, 33–39. [Google Scholar] [CrossRef]
- Chevillotte, F.; Panneton, R. Coupling transfer matrix method to finite element method for analyzing the acoustics of complex hollow body networks. Appl. Acoust. 2011, 72, 962–968. [Google Scholar] [CrossRef]
- Fertis, D.; Lee, C. Equivalent systems for the analysis of rectangular plates of varying thickness. Dev. Theor. Appl. Mech. 1990, 15, 627–637. [Google Scholar]
- Volokh, K.Y. On the classical theory of plates. J. Appl. Math. Mech. 1994, 58, 1101–1110. [Google Scholar] [CrossRef]
- Meleshko, V.V.; Gomilko, A.M. On the bending of clamped rectangular plates. Mech. Res. Commun. 1994, 21, 19–24. [Google Scholar] [CrossRef]
- Revenko, V.P.; Revenko, A.V. Determination of Plane Stress-Strain States of the Plates on the Basis of the Three-Dimensional Theory of Elasticity. Mater. Sci. 2017, 52, 811–818. [Google Scholar] [CrossRef]
- Zveryayev, Y.M. Analysis of the hypotheses used when constructing the theory of beams and plates. J. Appl. Math. Mech. 2003, 67, 425–434. [Google Scholar] [CrossRef]
- Ahanova, A.S.; Yessenbayeva, G.A.; Tursyngaliev, N.K. On the calculation of plates by the series representation of the deflection function. Bull. Karaganda University. Math. Ser. 2016, 82, 15–22. [Google Scholar]
- Delyavskyy, M.; Rosinski, K. Solution of non-rectangular plates with macroelement method. AIP Conf. Proc. 2017, 1822, 020005. [Google Scholar]
- Kuliyev, S. Stress state of compound polygonal plate. Mech. Res. Commun. 2003, 30, 519–530. [Google Scholar] [CrossRef]
- Delyavskyy, M.; Sobczak-Piąstka, J.; Rosinski, K.; Buchaniec, D.; Famulyak, Y. Solution of thin rectangular plates with various boundary conditions. AIP Conf. Proc. 2023, 2949, 020023. [Google Scholar]
- Imrak, C.E.; Gerdemeli, I. An exact solution for the deflection of a clamped rectangular plate under uniform load. Appl. Math. Sci. 2007, 1, 2129–2137. [Google Scholar]
- Matrosov, A.V.; Suratov, V.A. Stress-strain state in the corner points of a clamped plate under uniformly distributed normal load. Mater. Phys. Mech. 2018, 36, 124–146. [Google Scholar]
- Moubayed, N.; Wahab, A.; Bernard, M.; El-Khatib, H.; Sayegh, A.; Alsaleh, F.; Dachouwalyf, Y.; Chehadeh, N. Static analysis of an orthotropic plate. Phys. Procedia 2014, 55, 367–372. [Google Scholar] [CrossRef]
- Bhavikatti, S.S. Theory of Plates and Shells; New Age International (P) Limited Publishers: New Delhi, India; Bangalore, India; Chennai, India; Cochin, India; Guwahati, India; Hyderabad, India; Kolkata, India; Lucknow, India; Mumbai, India, 2024; ISBN (13) 978-81-224-3492-7. Available online: http://117.202.29.23:8080/jspui/bitstream/123456789/1574/1/Theory%20of%20Plates%20and%20Shells-New%20Age%20International%20Ltd%20S.S.%20Bhavikatti.pdf (accessed on 10 August 2024).
- Grigorenko, Y.M.; Rozhok, L.S. Stress–strain analysis of rectangular plates with a variable thickness and constant weight. Int. Appl. Mech. 2002, 38, 167–173. [Google Scholar] [CrossRef]
- Altenbach, H. Analysis of homogeneous and non-homogeneous plates. Solid Mech. Its Appl. 2008, 154, 1–36. [Google Scholar] [CrossRef]
- Fetea, M.S. Theoretical and comparative study regarding the mechanical response under the static loading for different rectangular plates. Ann. Univ. Oradea Fascicle Environ. Prot. 2018, 31, 141–146. [Google Scholar]
- Goloskokov, D.P.; Matrosov, A.V. Approximate analytical solutions in the analysis of thin elastic plates. AIP Conf. Proc. 2018, 1959, 070012. [Google Scholar]
- Kutsenko, A.; Kutsenko, O.; Yaremenko, V.V. On some aspects of implementation of boundary elements method in plate theory. Mach. Energetics 2021, 12, 107–111. [Google Scholar] [CrossRef]
- Niyonyungu, F.; Karangwa, J. Convergence analysis of finite element approach to classical approach for analysis of plates in bending. Adv. Sci. Technol. Res. J. 2019, 13, 170–180. [Google Scholar] [CrossRef]
- Orynyak, I.; Danylenko, K. Method of matched sections as a beam-like approach for plate analysis. Finite Elem. Anal. Des. 2024, 230, 104103. [Google Scholar] [CrossRef]
- Nikolić Stanojević, V.; Dolićanin, Ć.; Radojković, M. Application of Numerical methods in Solving a Phenomenon of the Theory of thin Plates. Sci. Tech. Rev. 2010, 60, 61–65. [Google Scholar]
- Vijayakumar, K. Review of a few selected theories of plates in bending. Int. Sch. Res. Not. 2014, 1, 291478. [Google Scholar] [CrossRef]
- Surianinov, M.; Shyliaiev, O. Calculation of plate-beam systems by method of boundary elements. Int. J. Eng. Technol. UAE 2018, 7, 238–241. [Google Scholar] [CrossRef]
- Sprinţu, I.; Fuiorea, I. Analytical solutions of the mechanical answer of thin orthotropic plates. In Proceedings of the Romanian Academy; Series A. Romanian Academy: Bucharest, Romania, 2013; Volume 14, pp. 343–350. Available online: https://academiaromana.ro/sectii2002/proceedings/doc2013-4/11-Fuiorea.pdf (accessed on 1 August 2023).
- Pinto-Cruz, M.C. Optimized Transfer Matrix Approach for Global Buckling Analysis: Bypassing Zero Matrix Inversion. Period. Polytech. Civ. Eng. 2024, 69, 28–44. [Google Scholar] [CrossRef]
- ASTM E2611-09; Standard Test Method for Measurement of Normal Incidence Sound Transmission of Acoustical Materials Based on the Transfer Matrix Method, 2009. American Society for Testing and Materials: New York, NY, USA, 2009. Available online: https://webstore.ansi.org/standards/astm/astme261109?srsltid=AfmBOopQ-dF-nYZAadkDHf4iJO5UNQICSyTG4_h7E3qEdeXFiXAN8MsP (accessed on 8 November 2024).

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Opruţa, D.; Tripa, M.-S.; Codrea, L.; Boldor, C.; Dumea, D.; Gyorbiro, R.; Brisc, C.; Bărăian, I.; Opriţoiu, P.; Chereches, A.; et al. Calculus of Long Rectangular Plates Embedded in Long Borders with Uniform Vertical Load on a Line Parallel to the Long Borders. Mathematics 2025, 13, 993. https://doi.org/10.3390/math13060993
Opruţa D, Tripa M-S, Codrea L, Boldor C, Dumea D, Gyorbiro R, Brisc C, Bărăian I, Opriţoiu P, Chereches A, et al. Calculus of Long Rectangular Plates Embedded in Long Borders with Uniform Vertical Load on a Line Parallel to the Long Borders. Mathematics. 2025; 13(6):993. https://doi.org/10.3390/math13060993
Chicago/Turabian StyleOpruţa, Daniel, Mihai-Sorin Tripa, Luminiţa Codrea, Cristian Boldor, Dan Dumea, Robert Gyorbiro, Cosmin Brisc, Iulia Bărăian, Petre Opriţoiu, Aurel Chereches, and et al. 2025. "Calculus of Long Rectangular Plates Embedded in Long Borders with Uniform Vertical Load on a Line Parallel to the Long Borders" Mathematics 13, no. 6: 993. https://doi.org/10.3390/math13060993
APA StyleOpruţa, D., Tripa, M.-S., Codrea, L., Boldor, C., Dumea, D., Gyorbiro, R., Brisc, C., Bărăian, I., Opriţoiu, P., Chereches, A., & Suciu, M. (2025). Calculus of Long Rectangular Plates Embedded in Long Borders with Uniform Vertical Load on a Line Parallel to the Long Borders. Mathematics, 13(6), 993. https://doi.org/10.3390/math13060993





