An Improved Relationship between the Solution and Its Corresponding Function in Fourth-Order Neutral Differential Equations and Its Applications
Abstract
1. Introduction
- ()
- and belong to , and q belongs to ;
- ()
- , , , and ;
- ()
- , , , and .
- ()
- , whereand
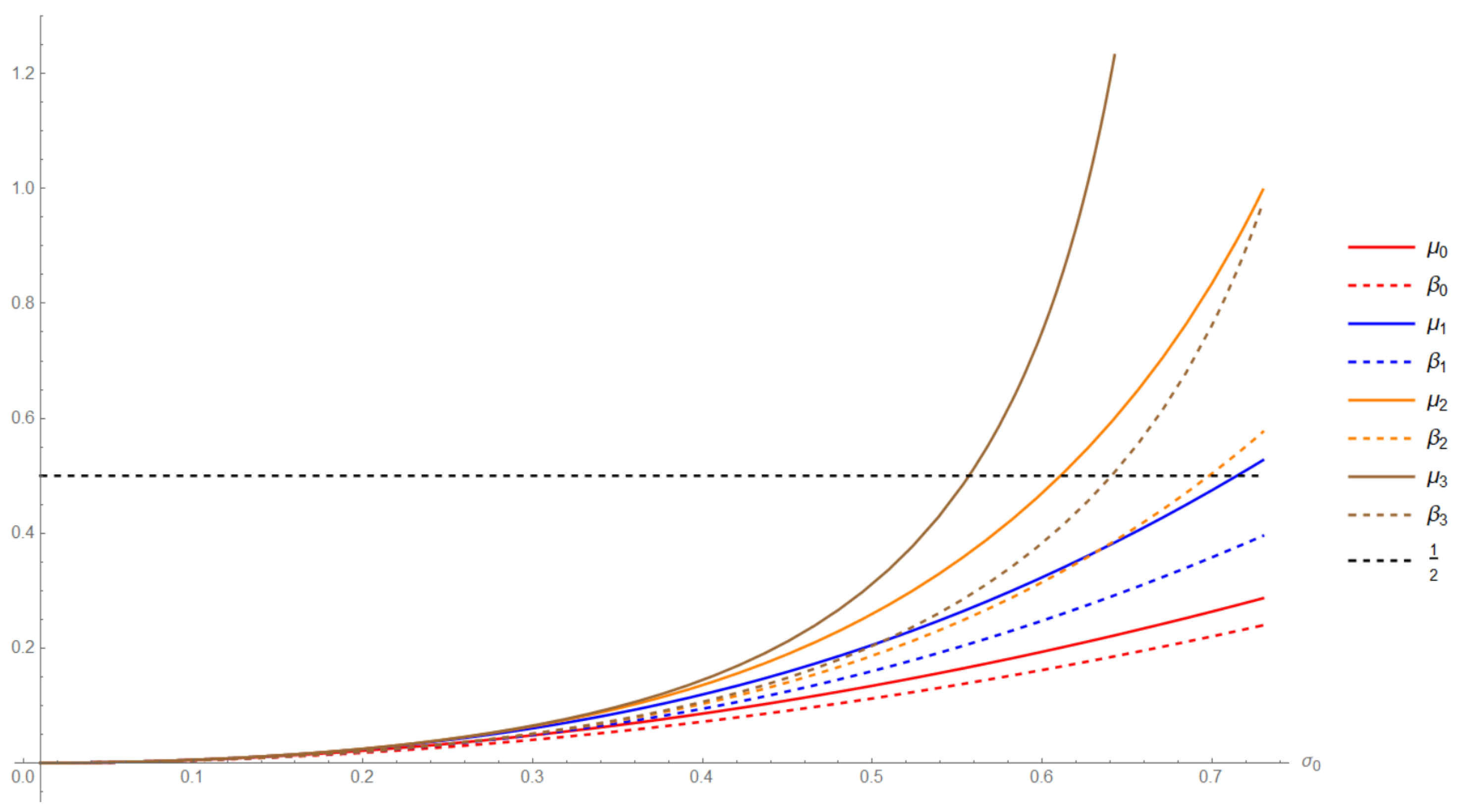
2. Asymptotic and Monotonic Properties
- ():
- ():
- ():
- (i)
- for
- (ii)
2.1. Category
- (A1-1)
- , for all ,
- (A1-2)
- ,
- (A1-3)
- .
- (A1-4)
- ,
- (A1-5)
- .
- (i)
- there is such that
- (ii)
- (iii)
- there exists a positive integer n such that , for , where , for any , andIf , then .
2.2. Category
- (A2-1)
- ,
- (A2-2)
- for .
- (A2-3)
- ,
- (A2-4)
- .
- (i)
- there is such that
- (ii)
- (iii)
- there exists a positive integer n such that , for , for any , and
- (A2-5)
- (A2-6)
- (A2-7)
- .
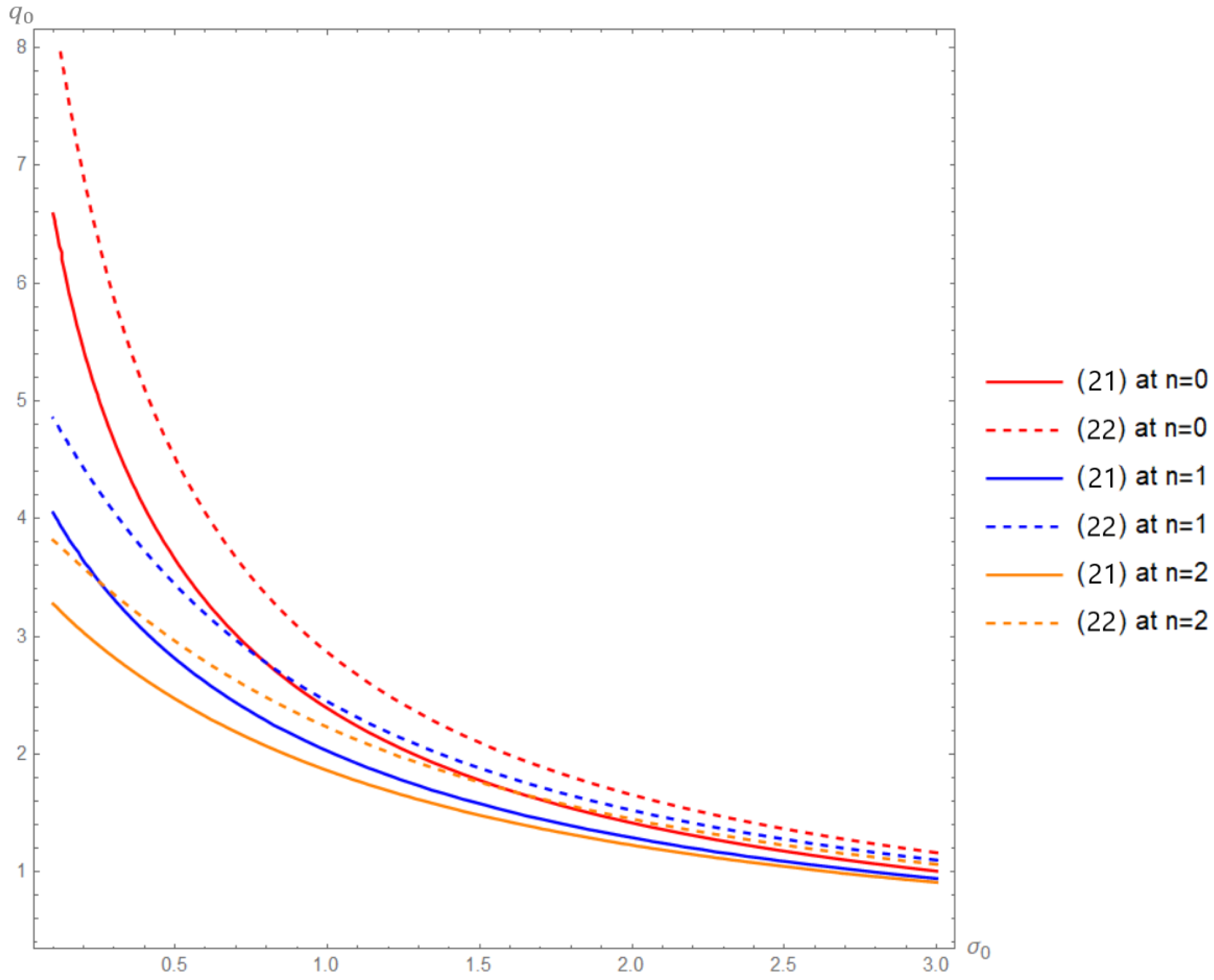
3. Oscillation Conditions
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Oldham, K.; Spanier, J. The Fractional Calculus Theory and Applications of Differentiation and Integration to Arbitrary Order; Elsevier: Amsterdam, The Netherlands, 1974. [Google Scholar]
- Samko, S.G.; Kilbas, A.A.; Marichev, O.I. Fractional Integrals and Derivatives; Gordon and Breach Science Publishers: Yverdon-les-Bains, Switzerland, 1993. [Google Scholar]
- Hale, J.K. Functional differential equations. In Oxford Applied Mathematical Sciences; Springer: New York, NY, USA, 1971; Volume 3. [Google Scholar]
- Rihan, F.A. Delay Differential Equations and Applications to Biology; Springer Nature Singapore Pte Ltd.: Singapore, 2021. [Google Scholar]
- Ladde, G.S.; Lakshmikantham, V.; Zhang, B.G. Oscillation Theory of Differential Equations with Deviating Arguments; Marcel Dekker: New York, NY, USA, 1987. [Google Scholar]
- Gyori, I.; Ladas, G. Oscillation Theory of Delay Differential Equations with Applications; Clarendon Press: Oxford, UK, 1991. [Google Scholar]
- Erbe, L.H.; Kong, Q.; Zhong, B.G. Oscillation Theory for Functional Differential Equations; Marcel Dekker: New York, NY, USA, 1995. [Google Scholar]
- Agarwal, R.P.; Grace, S.R.; O’Regan, D. Oscillation Theory for Second Order Linear, Half-Linear, Superlinear and Sublinear Dynamic Equations; Kluwer Academic Publishers: Dordrecht, The Netherlands, 2002. [Google Scholar]
- Džurina, J.; Jadlovská, I. A sharp oscillation result for second-order half-linear noncanonical delay differential equations. Electron. J. Qual. Theory Differ. Equ. 2020, 46, 1–14. [Google Scholar] [CrossRef]
- Džurina, J.; Jadlovská, I. Kneser-type oscillation criteria for second-order half-linear delay differential equations. Appl. Math. Comput. 2020, 380, 125289. [Google Scholar]
- Jadlovská, I. Oscillation criteria of Kneser-type for second-order half-linear advanced differential equations. Appl. Math. Lett. 2020, 106, 106354. [Google Scholar] [CrossRef]
- Jadlovská, I. New criteria for sharp oscillation of second-order neutral delay differential equations. Mathematics 2021, 9, 2089. [Google Scholar] [CrossRef]
- Džurina, J.; Grace, S.R.; Jadlovská, I.; Li, T. Oscillation criteria for second-order Emden-Fowler delay differential equations with a sublinear neutral term. Math. Nachr. 2020, 5, 910–922. [Google Scholar] [CrossRef]
- Bohner, M.; Grace, S.R.; Jadlovská, I. Oscillation criteria for second-order neutral delay differential equations. Electron. J. Qual. Theory Differ. Equ. 2017, 60, 1–12. [Google Scholar] [CrossRef]
- Bohner, M.; Grace, S.R.; Jadlovská, I. Sharp oscillation criteria for second-order neutral delay differential equations. Math. Methods Appl. Sci. 2020, 17, 10041–10053. [Google Scholar] [CrossRef]
- Moaaz, O.; Ramos, H.; Awrejcewicz, J. Second-order Emden–Fowler neutral differential equations: A new precise criterion for oscillation. Appl. Math. Lett. 2021, 118, 107172. [Google Scholar] [CrossRef]
- Almarri, B.; Moaaz, O.; Anis, M.; Qaraad, B. Third-Order Neutral Differential Equation with a Middle Term and Several Delays: Asymptotic Behavior of Solutions. Axioms 2023, 12, 166. [Google Scholar] [CrossRef]
- Gopal, T.; Ayyappan, G.; Graef, J.R.; Thandapani, E. Oscillatory and asymptotic behavior of solutions of third-order quasi-linear neutral difference equations. Math. Slovaca. 2022, 72, 411–418. [Google Scholar] [CrossRef]
- Jadlovská, I.; Chatzarakis, G.E.; Džurina, J.; Grace, S.R. On sharp oscillation criteria for general third-order delay differential equations. Mathematics 2021, 9, 1675. [Google Scholar] [CrossRef]
- Thandapani, E.; Göktürk, B.; Özdemir, O.; Tunç, E. Oscillatory behavior of semi-canonical nonlinear neutral differential equations of third-order via comparison principles. Qual. Theory Dyn. Syst. 2023, 22, 30. [Google Scholar] [CrossRef]
- Alzabut, J.; Agarwal, R.P.; Grace, S.R.; Jonnalagadda, J.M.; Selvam, A.G.M.; Wang, C. A survey on the oscillation of solutions for fractional difference equations. Mathematics 2022, 10, 894. [Google Scholar] [CrossRef]
- Santra, S.S.; Scapellato, A. Some conditions for the oscillation of second-order differential equations with several mixed delays. J. Fixed Point Theory Appl. 2022, 24, 18. [Google Scholar] [CrossRef]
- Santra, S.S.; El-Nabulsi, R.A.; Khedher, K.M. Oscillation of second-order differential equations with multiple and mixed delays under a canonical operator. Mathematics 2021, 9, 1323. [Google Scholar] [CrossRef]
- Santra, S.S.; Khedher, K.M.; Yao, S.W. New aspects for oscillation of differential systems with mixed delays and impulses. Symmetry 2021, 13, 780. [Google Scholar] [CrossRef]
- Tunç, E.; Özdemir, O. Comparison theorems on the oscillation of even order nonlinear mixed neutral differential equations. Math. Methods Appl. Sci. 2023, 46, 631–640. [Google Scholar] [CrossRef]
- Graef, J.R.; Özdemir, O.; Kaymaz, A.; Tunc, E. Oscillation of damped second-order linear mixed neutral differential equations. Mon. Math. 2021, 194, 85–104. [Google Scholar] [CrossRef]
- Yang, D.; Bai, C. On the oscillation criteria for fourth-order p-Laplacian differential equations with middle term. J. Funct. Spaces 2021, 2021, 1–10. [Google Scholar] [CrossRef]
- Zeng, Y.; Li, Y.; Luo, L.; Luo, Z. Oscillation of generalized neutral delay differential equations of Emden-Fowler type with with damping. J. Zhejiang Univ.-Sci. A 2016, 43, 394–400. [Google Scholar]
- Hassan, T.S.; Sun, Y.; Menaem, A.A. Improved oscillation results for functional nonlinear dynamic equations of second order. Mathematics 2020, 8, 1897. [Google Scholar] [CrossRef]
- Hassan, T.S.; Grace, S.R. Comparison criteria for nonlinear functional dynamic equations of higher order. Discret. Dyn. Nat. Soc. 2016, 2016, 6847956. [Google Scholar] [CrossRef]
- O’Regan, D.; Hassan, T.S. Oscillation criteria for solutions to nonlinear dynamic equations of higher order. Hacet. J. Math. Stat. 2016, 45, 417–427. [Google Scholar] [CrossRef]
- Hassan, A.M.; Ramos, H.; Moaaz, O. Second-Order Dynamic Equations with Noncanonical Operator: Oscillatory Behavior. Fractal Fract. 2023, 7, 134. [Google Scholar] [CrossRef]
- Agarwal, R.P.; Zhang, C.; Li, T. Some remarks on oscillation of second order neutral differential equations. Appl. Math. Comput. 2016, 274, 178–181. [Google Scholar] [CrossRef]
- Moaaz, O.; Muhib, A.; Owyed, S.; Mahmoud, E.E.; Abdelnaser, A. Second-order neutral differential equations: Improved criteria for testing the oscillation. Jpn. J. Math. 2021, 2021, 6665103. [Google Scholar] [CrossRef]
- Hassan, T.S.; Moaaz, O.; Nabih, A.; Mesmouli, M.B.; El-Sayed, A. New sufficient condi tions for oscillation of second-order neutral delay differential equations. Axioms 2021, 10, 281. [Google Scholar] [CrossRef]
- Bohner, M.; Grace, S.R.; Jadlovská, I. Sharp results for oscillation of second-order neutral delay differential equations. Electron. J. Qual. Theory Differ. Equ. 2023, 4, 1–23. [Google Scholar] [CrossRef]
- Moaaz, O.; Mahmoud, E.E.; Alharbi, W.R. Third-order neutral delay differential equations: New iterative criteria for oscillation. J. Funct. Spaces 2020, 2020, 1–8. [Google Scholar] [CrossRef]
- Jadlovská, I.; Džurina, J.; Graef, J.R.; Grace, S.R. Sharp oscillation theorem for fourth-order linear delay differential equations. J. Inequalities Appl. 2022, 2022, 122. [Google Scholar] [CrossRef]
- Almarri, B.; Ramos, H.; Moaaz, O. New Monotonic Properties of the Class of Positive Solutions of Even-Order Neutral Differential Equations. Mathematics 2022, 10, 1470. [Google Scholar] [CrossRef]
- Muhib, A.; Moaaz, O.; Cesarano, C.; Askar, S.S.; Elabbasy, E.M. Neutral Differential Equations of Fourth-Order: New Asymptotic Properties of Solutions. Axioms 2022, 11, 52. [Google Scholar] [CrossRef]
- Fowler, R.H. Emden’s equation: The solutions of Emden’s and similar differential equations. Mon. Not. R. Astron. Soc. 1930, 91, 63–91. [Google Scholar] [CrossRef]
- Wong, J.S.W. On the generalized Emden–Fowler equation. SIAM Rev. 1975, 17, 339–360. [Google Scholar] [CrossRef]
- Berkovich, L.M. The generalized Emden–Fowler equation. Sym. Nonlinear Math. Phys. 1997, 1, 155–163. [Google Scholar]
- Agarwal, R.P.; Grace, S.R.; O’Regan, D. Oscillation Theory for Difference and Functional Differential Equations; Kluwer Academic: Dordrecht, The Netherlands, 2000. [Google Scholar]
- Kiguradze, I.T.; Chanturia, T.A. Asymptotic Properties of Solutions of Nonautonomous Ordinary Diferential Equations. Math. Its Appl. 1993. [Google Scholar] [CrossRef]
- Elabbasy, E.M.; Moaaz, O.; Ramos, H.; Muhib, A. Improved criteria for oscillation of noncanonical neutral differential equations of even order. Adv. Differ. Equ. 2021, 2021, 412. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Moaaz, O.; Cesarano, C.; Almarri, B. An Improved Relationship between the Solution and Its Corresponding Function in Fourth-Order Neutral Differential Equations and Its Applications. Mathematics 2023, 11, 1708. https://doi.org/10.3390/math11071708
Moaaz O, Cesarano C, Almarri B. An Improved Relationship between the Solution and Its Corresponding Function in Fourth-Order Neutral Differential Equations and Its Applications. Mathematics. 2023; 11(7):1708. https://doi.org/10.3390/math11071708
Chicago/Turabian StyleMoaaz, Osama, Clemente Cesarano, and Barakah Almarri. 2023. "An Improved Relationship between the Solution and Its Corresponding Function in Fourth-Order Neutral Differential Equations and Its Applications" Mathematics 11, no. 7: 1708. https://doi.org/10.3390/math11071708
APA StyleMoaaz, O., Cesarano, C., & Almarri, B. (2023). An Improved Relationship between the Solution and Its Corresponding Function in Fourth-Order Neutral Differential Equations and Its Applications. Mathematics, 11(7), 1708. https://doi.org/10.3390/math11071708