Modulations of Stochastic Modeling in the Structural and Energy Aspects of the Kundu–Mukherjee–Naskar System
Abstract
:1. Introduction
2. The New Stochastic Solutions
3. Closed-Form Solutions
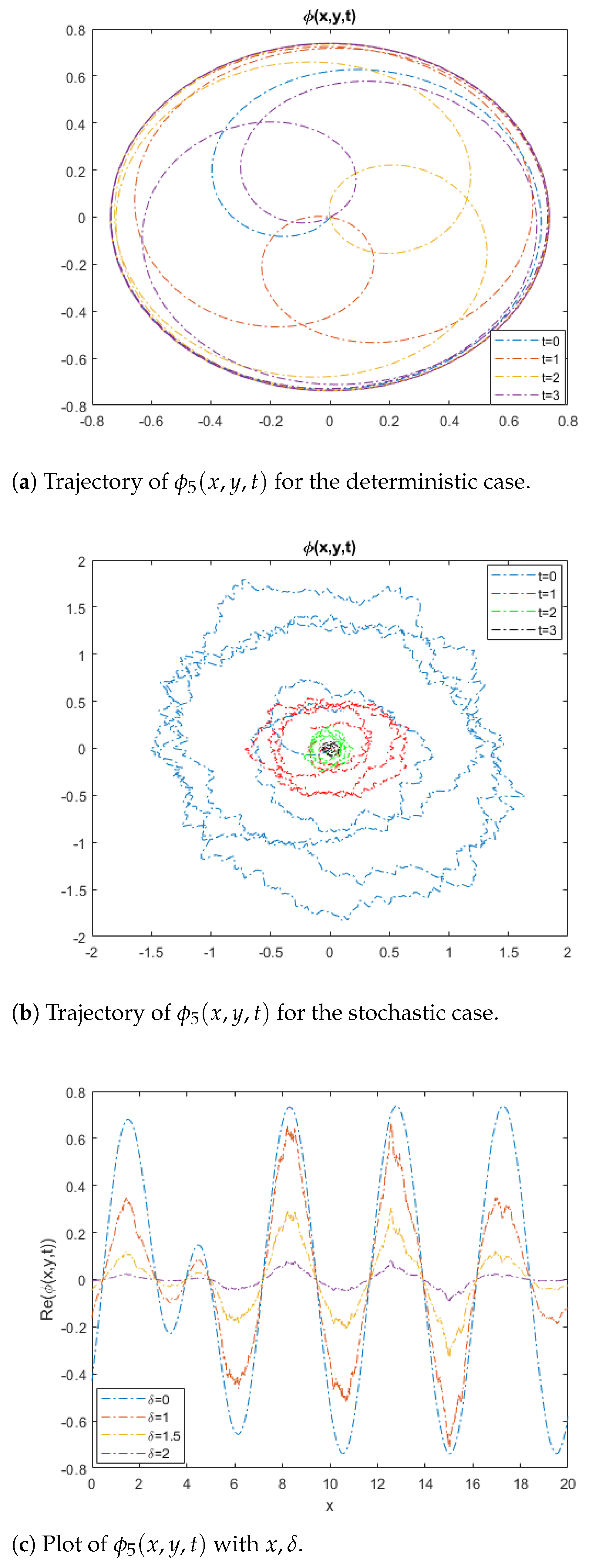
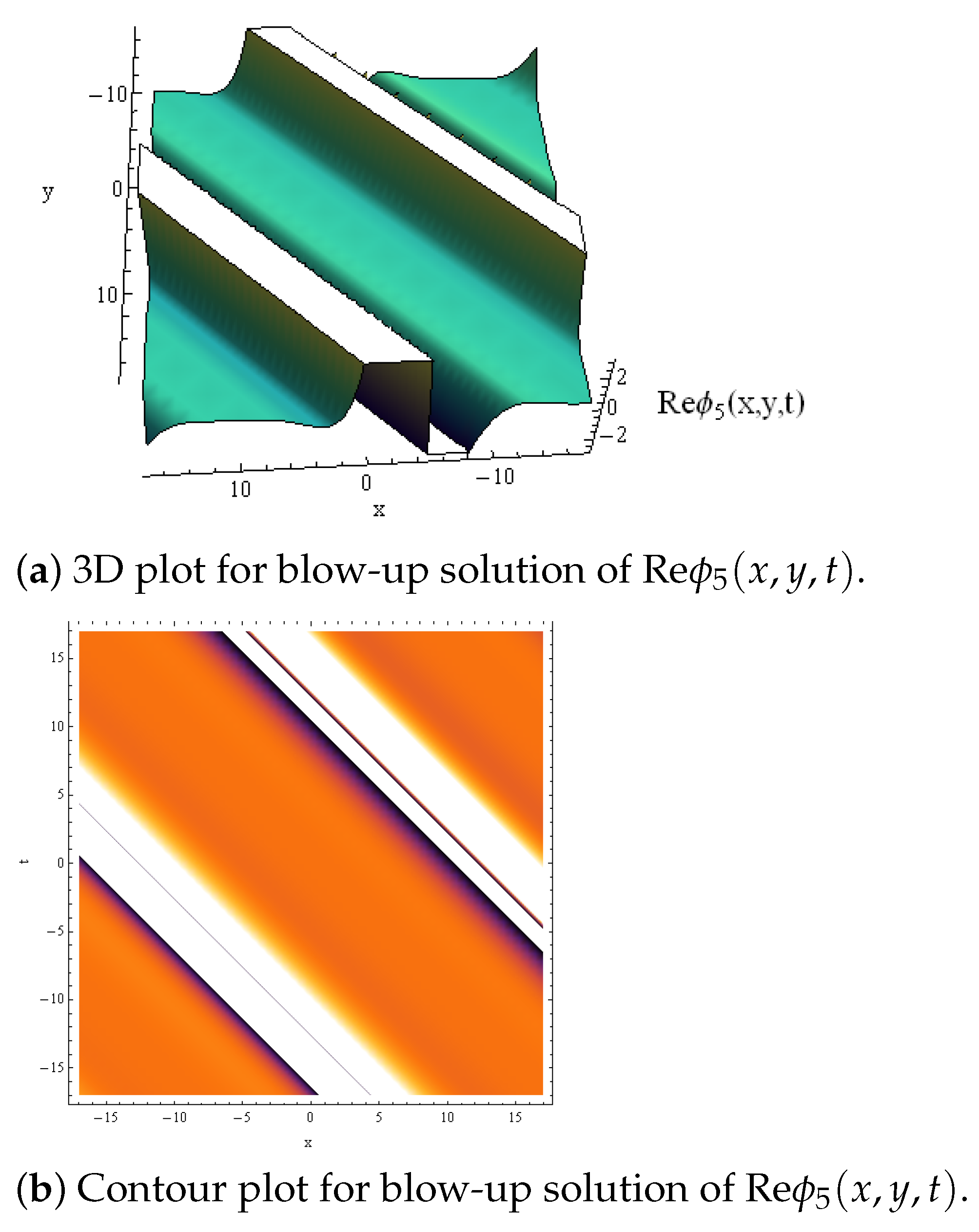
4. Physical Interpretation
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Malik, H.K.; Srivastava, R.; Kumar, S.; Singh, D. Small amplitude dust acoustic solitary wave in magnetized two ion temperature plasma. J. Taibah Univ. Sci. 2020, 14, 417–422. [Google Scholar] [CrossRef]
- Wazwaz, A.M.; Kaur, L. Optical solitons for nonlinear Schrödinger equation in the normal dispersive regimes. Optik 2019, 184, 428–435. [Google Scholar] [CrossRef]
- Younis, M.; Ali, S.; Mahmood, S.A. Solitons for compound KdV Burgers equation with variable coefficients and power law nonlinearity. Nonlinear Dyn. 2015, 81, 1191–1196. [Google Scholar] [CrossRef]
- Abdelwahed, H.G.; Abdelrahman, M.A.E. New nonlinear periodic, solitonic, dissipative waveforms for modified-Kadomstev-Petviashvili-equation in nonthermal positron plasma. Results Phys. 2020, 19, 103393. [Google Scholar] [CrossRef]
- Singh, S.V.; Lakhina, G.S. Ion-acoustic supersolitons in the presence of non-thermal electrons. Commun. Nonlinear Sci. Numer. Simul. 2015, 23, 274–281. [Google Scholar] [CrossRef]
- Abdelwahed, H.G. Nonlinearity contributions on critical MKP equation. J. Taibah Univ. Sci. 2020, 14, 777–782. [Google Scholar] [CrossRef]
- Nakkeeran, K. Bright and dark optical solitons in fiber media with higher-order effects. Chaos Solitons Fractals 2002, 13, 673–679. [Google Scholar] [CrossRef]
- Triki, H.; Bensalem, C.; Biswas, A.; Khan, S.; Zhou, Q.; Adesanya, S.; Moshokoa, S.P.; Belic, M. Self-similar optical solitons with continuous-wave background in a quadratic-cubic non-centrosymmetric waveguide. Opt. Commun. 2019, 437, 392–398. [Google Scholar] [CrossRef]
- Wazwaz, A.M.; Triki, H. Soliton solutions of the cubic-quintic nonlinear Schrödinger equation with variable coefficients. Rom. J. Phys. 2016, 61, 360–366. [Google Scholar]
- Wazwaz, A.M. Bright and dark optical solitons for (2+1)-dimensional Schrödinger (NLS) equations in the anomalous dispersion regimes and the normal dispersive regimes. Optik 2019, 192, 162948. [Google Scholar] [CrossRef]
- ⌀ksendal, B. Stochastic Differential Equations: An Introduction with Applications, 6th ed.; Springer: Berlin/Heidelberg, Germany, 2003. [Google Scholar]
- Macías-Díaz, J.E.; Raza, A.; Ahmed, N.; Rafiq, M. Analysis of a nonstandard computer method to simulate a nonlinear stochastic epidemiological model of coronavirus-like diseases. Comput. Methods Programs Biomed. 2021, 204, 106054. [Google Scholar] [CrossRef] [PubMed]
- Mirzaee, F.; Rezaei, S.; Samadyar, N. Numerical solution of two-dimensional stochastic time-fractional sine-Gordon equation on non-rectangular domains using finite difference and meshfree methods. Eng. Anal. Bound. Elem. 2021, 127, 53–63. [Google Scholar] [CrossRef]
- Rafiq, M.; Ahmadian, A.; Raza, A.; Baleanu, D.; Ahsan, M.S.; Abdul Sathar, M.H. Numerical control measures of stochastic malaria epidemic model. Comput. Mater. Contin. 2020, 65, 33–51. [Google Scholar] [CrossRef]
- Karatzas, I.; Shreve, S.E. Brownian Motion and Stochastic Calculus, 2nd ed.; Springer: Berlin/Heidelberg, Germany, 1991. [Google Scholar]
- Pishro-Nik, H. Introduction to Probability, Statistics and Random Processes; Kappa Research, LLC: Sunderland, MA, USA, 2014. [Google Scholar]
- Raza, A.; Arif, M.S.; Rafiq, M. A reliable numerical analysis for stochastic gonorrhea epidemic model with treatment effect. Int. J. Biomath. 2019, 12, 1950072. [Google Scholar] [CrossRef]
- Raza, A.; Arif, M.S.; Rafiq, M. A reliable numerical analysis for stochastic dengue epidemic model with incubation period of virus. Adv. Differ. Equ. 2019, 2019, 32. [Google Scholar] [CrossRef]
- Kundu, A.; Mukherjee, A.; Naskar, T. Modelling rogue waves through exact dynamical lump soliton controlled by ocean currents. Proc. R. Soc. A 2014, 470, 20130576. [Google Scholar] [CrossRef]
- Wen, X. Higher-order rational solutions for the (2+1)-dimensional KMN equation. Proc. Rom. Acad. Ser. A 2017, 18, 191–198. [Google Scholar]
- Ekici, M.; Sonmezoglua, A.; Biswas, A.; Belic, M.R. Optical solitons in (2+1)-Dimensions with Kundu-Mukherjee-Naskar equation by extended trial function scheme. Chin. J. Phys. 2019, 57, 72–77. [Google Scholar] [CrossRef]
- Biswas, A.; Yildirim, Y.; Yasar, E.; Zhou, Q.; Moshokoa, S.P.; Belic, M. Optical soliton perturbation with quadraticcubic nonlinearity using a couple of strategic algorithms. Chin. J. Phys. 2018, 56, 1990–1998. [Google Scholar] [CrossRef]
- Alkhidhr, H.A.; Abdelwahed, H.G.; Abdelrahman, M.A.E.; Alghanim, S. Some solutions for a stochastic NLSE in the unstable and higher order dispersive environments. Results Phys. 2022, 34, 105242. [Google Scholar] [CrossRef]
- Fedrizzi, E. Partial Differential Equation and Noise. Ph.D. Thesis, Université Paris Diderot, Paris, France, 2012. [Google Scholar]
- Alomair, R.A.; Hassan, S.Z.; Abdelrahman, M.A.E. A new structure of solutions to the coupled nonlinear Maccari’s systems in plasma physics. AIMS Math. 2022, 7, 8588–8606. [Google Scholar] [CrossRef]
- Available online: https://www.mathworks.com/support/search.html?q=plots&page=1 (accessed on 4 November 2023).
- Almutairi, A.; El-Shewy, E.K.; Amin, A.H.; Abdelrahman, M.A.E. New solitary electrostatic structures for Zakharov model in subsonic limit for solar-wind. Results Phys. 2022, 37, 105521. [Google Scholar] [CrossRef]
- Abdelwahed, H.G.; El-Shewy, E.K.; Alghanim, S.; Abdelrahman, M.A.E. On the physical fractional modulations on Langmuir plasma structures. Fractal Fract. 2022, 6, 430. [Google Scholar] [CrossRef]
- Biondini, G.; El, G.A.; Hoefer, M.A.; Miller, P.D. Dispersive hydrodynamics: Preface. Phys. D 2016, 333, 1–5. [Google Scholar] [CrossRef]
- Chang, J.J.; Engels, P.; Hoefer, M.A. Formation of dispersive shock waves by merging and splitting Bose-Einstein condensates. Phys. Rev. Lett. 2008, 101, 170404. [Google Scholar] [CrossRef]
- Guan, W.Y.; Li, B.Q. New observation on the breather for a generalized nonlinear Schrödinger system with two higher-order dispersion operators in inhomogeneous optical fiber. Optik 2019, 181, 853–861. [Google Scholar] [CrossRef]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
El-Shewy, E.K.; Abdo, N.F.; Abdelrahman, M.A.E. Modulations of Stochastic Modeling in the Structural and Energy Aspects of the Kundu–Mukherjee–Naskar System. Mathematics 2023, 11, 4881. https://doi.org/10.3390/math11244881
El-Shewy EK, Abdo NF, Abdelrahman MAE. Modulations of Stochastic Modeling in the Structural and Energy Aspects of the Kundu–Mukherjee–Naskar System. Mathematics. 2023; 11(24):4881. https://doi.org/10.3390/math11244881
Chicago/Turabian StyleEl-Shewy, Emad K., Noura F. Abdo, and Mahmoud A. E. Abdelrahman. 2023. "Modulations of Stochastic Modeling in the Structural and Energy Aspects of the Kundu–Mukherjee–Naskar System" Mathematics 11, no. 24: 4881. https://doi.org/10.3390/math11244881
APA StyleEl-Shewy, E. K., Abdo, N. F., & Abdelrahman, M. A. E. (2023). Modulations of Stochastic Modeling in the Structural and Energy Aspects of the Kundu–Mukherjee–Naskar System. Mathematics, 11(24), 4881. https://doi.org/10.3390/math11244881





