Mathematical Modeling of Structure and Dynamics of Concentrated Tornado-like Vortices: A Review
Abstract
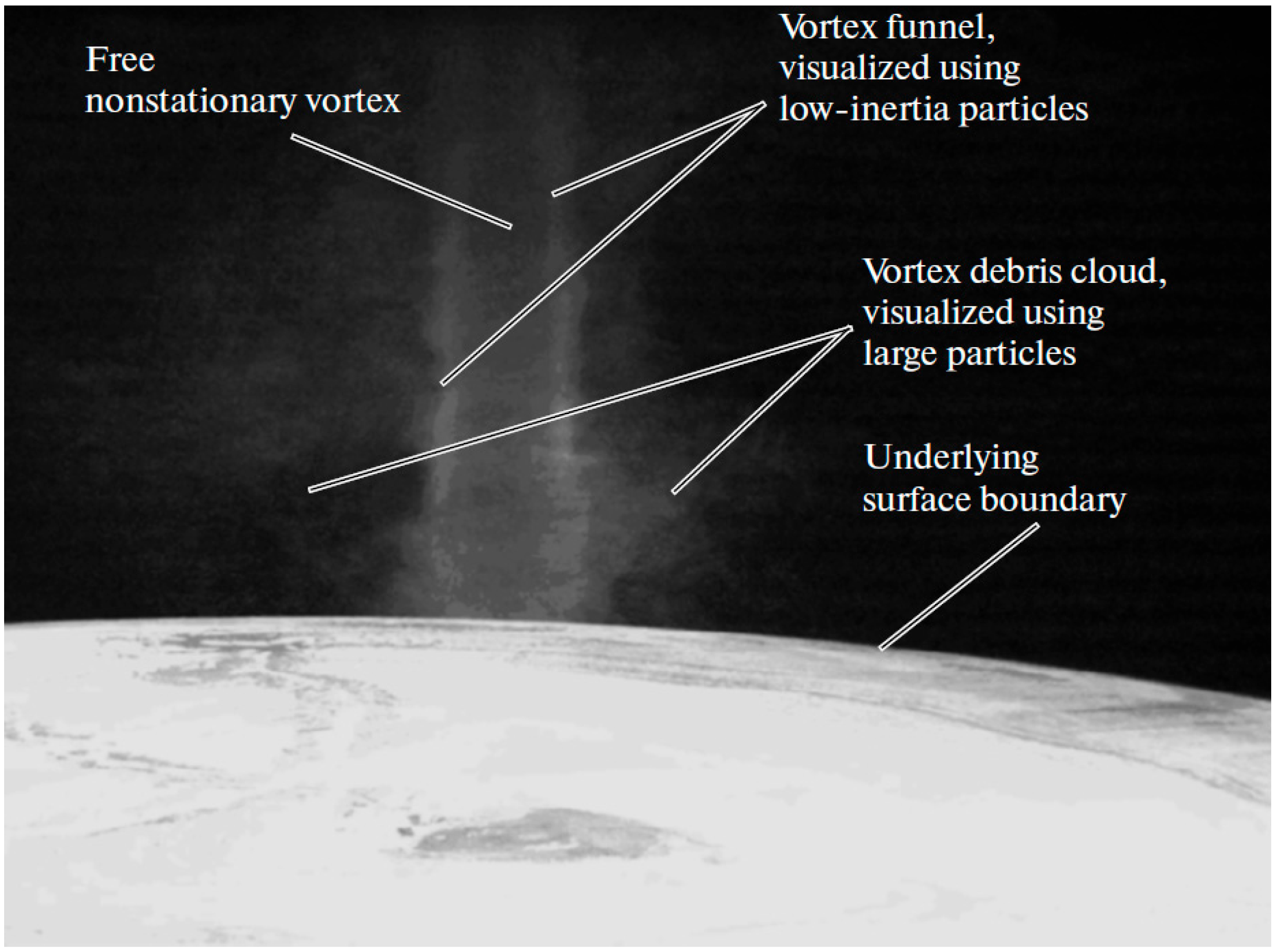
1. Introduction
2. Mathematical Modeling: Analytical Simulation
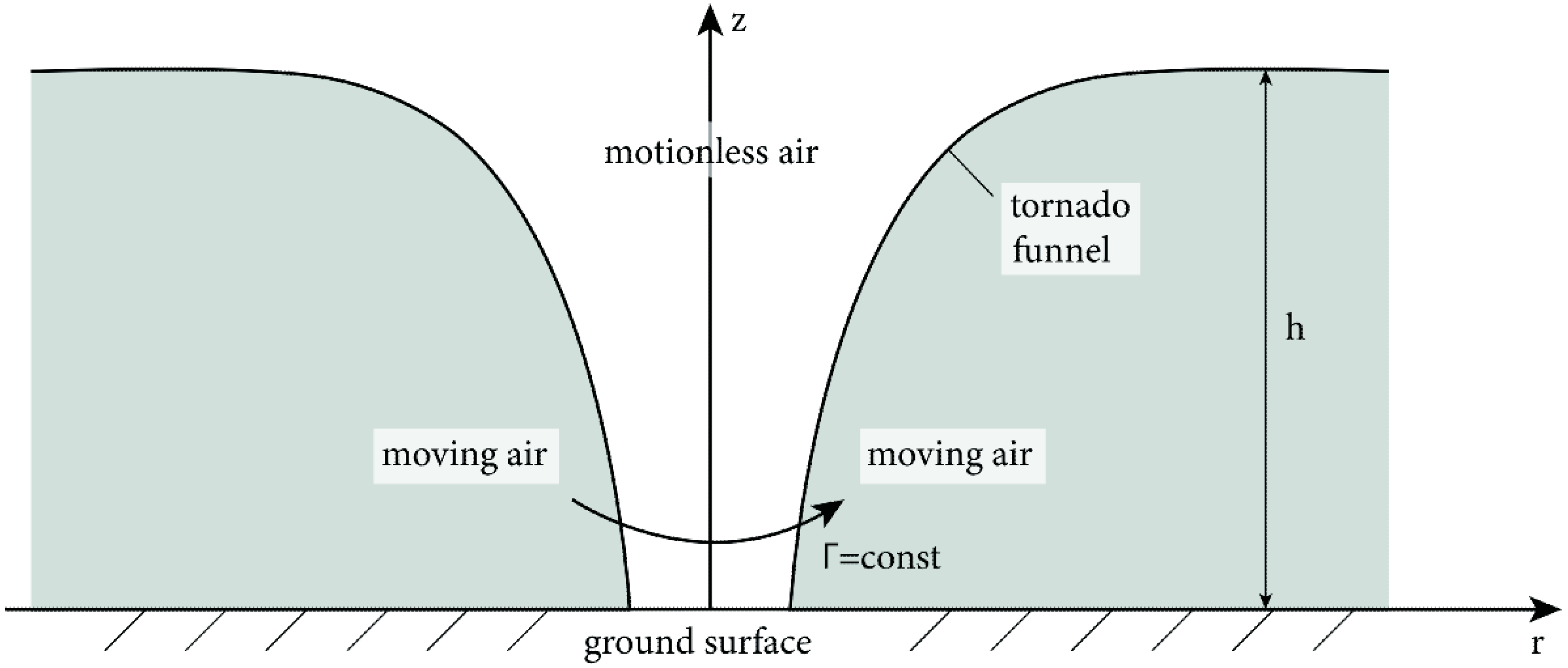
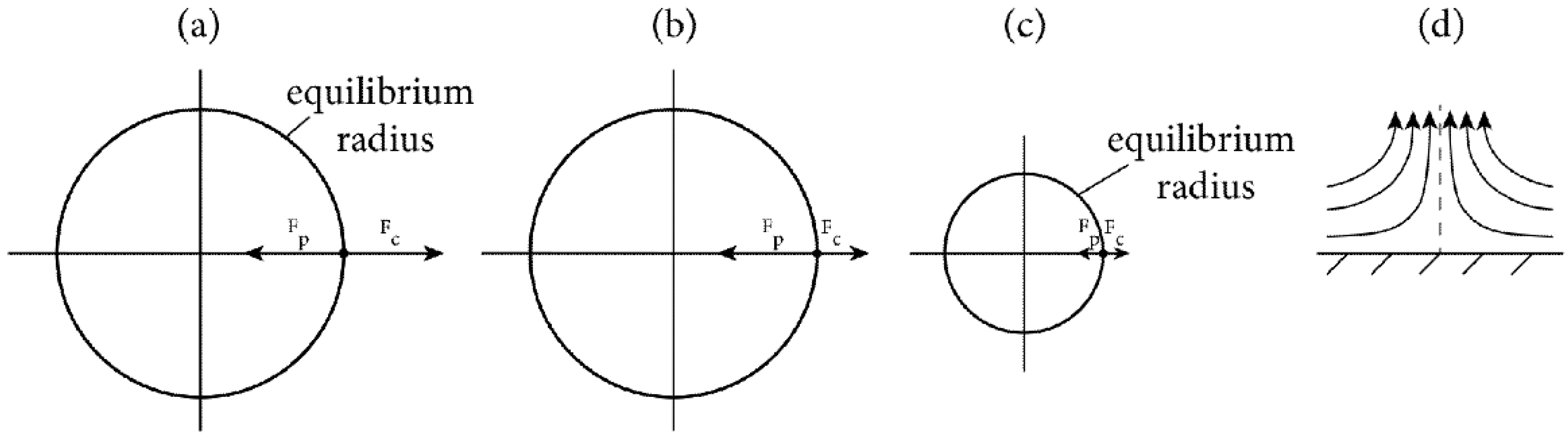
2.1. Simple Analytical Models
2.2. Thermodynamic Models
3. Mathematical Modeling: Numerical Simulation
3.1. Main Trends of Numerical Modeling
3.2. Early Numerical Studies
3.3. Two-Phase Nature of Tornado
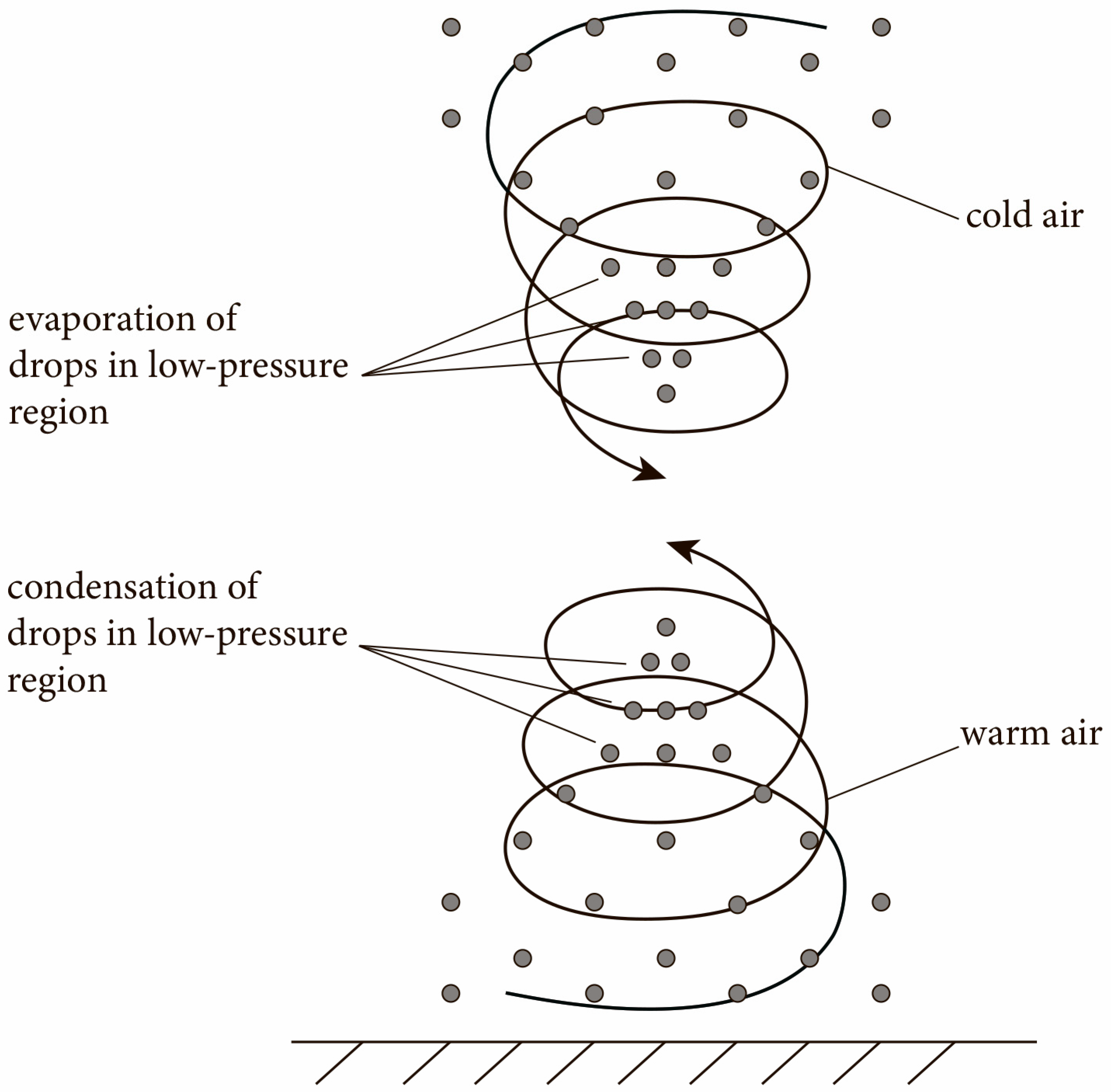
3.4. Processes of Evaporation and Condensation
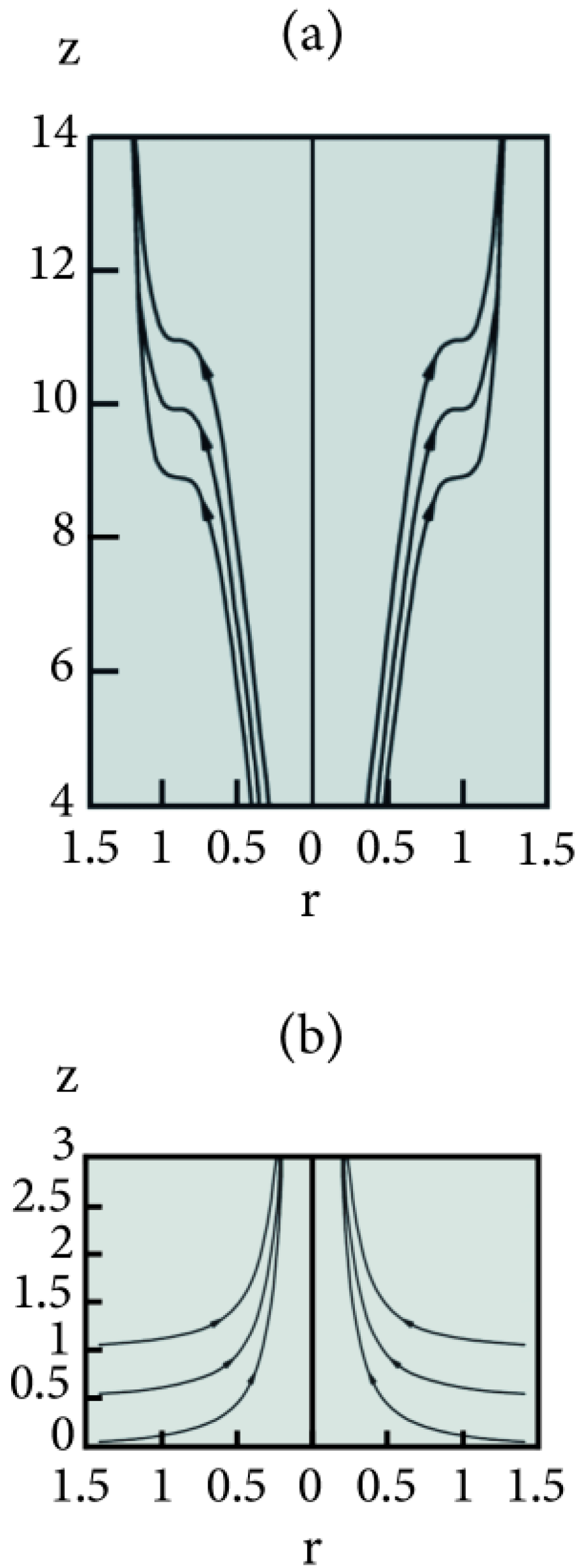
3.5. Disperse Phase Motion in Tornado-like Flows
4. Latest Results
4.1. Dynamics of the Cyclostrophic Balance
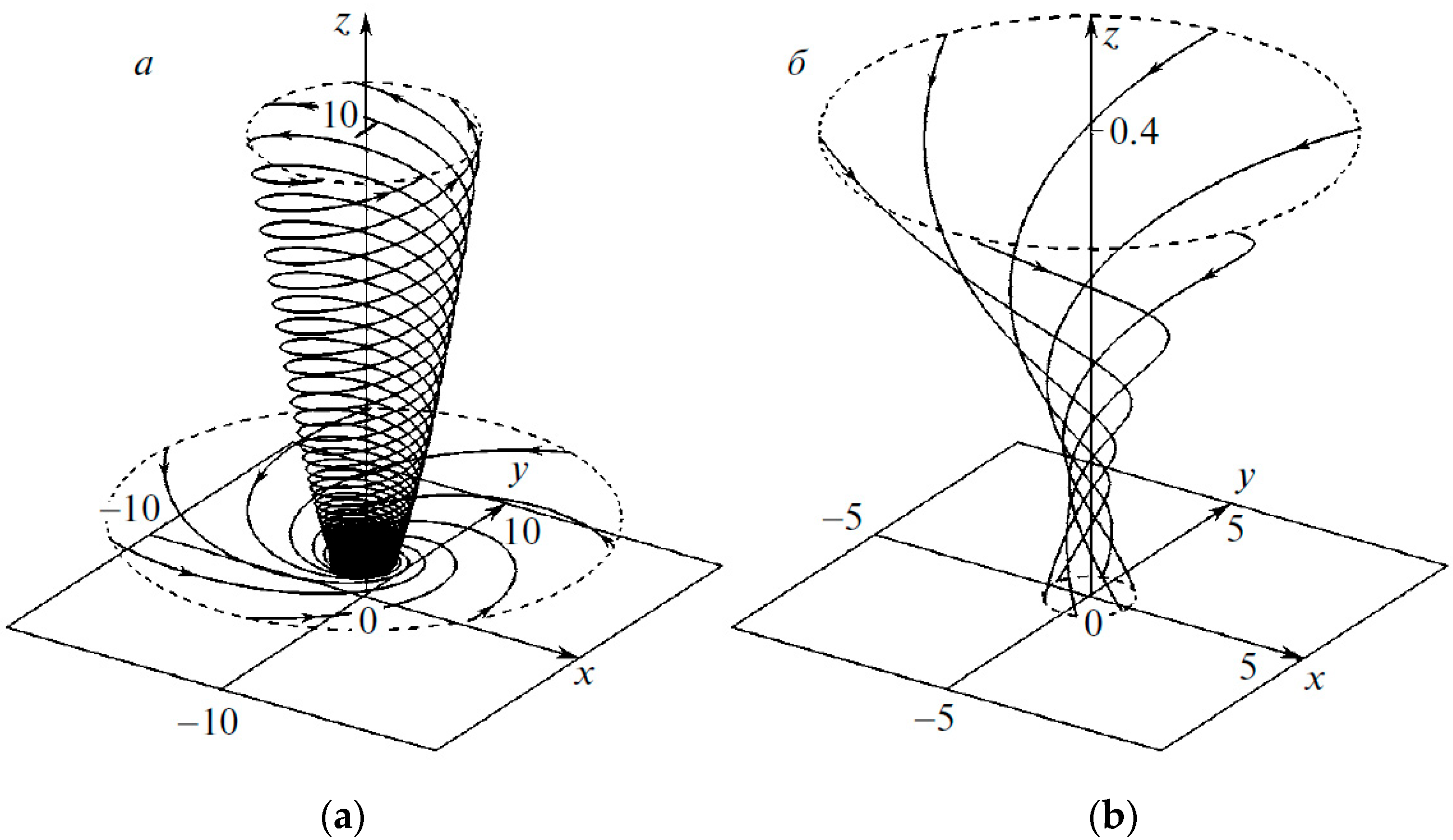
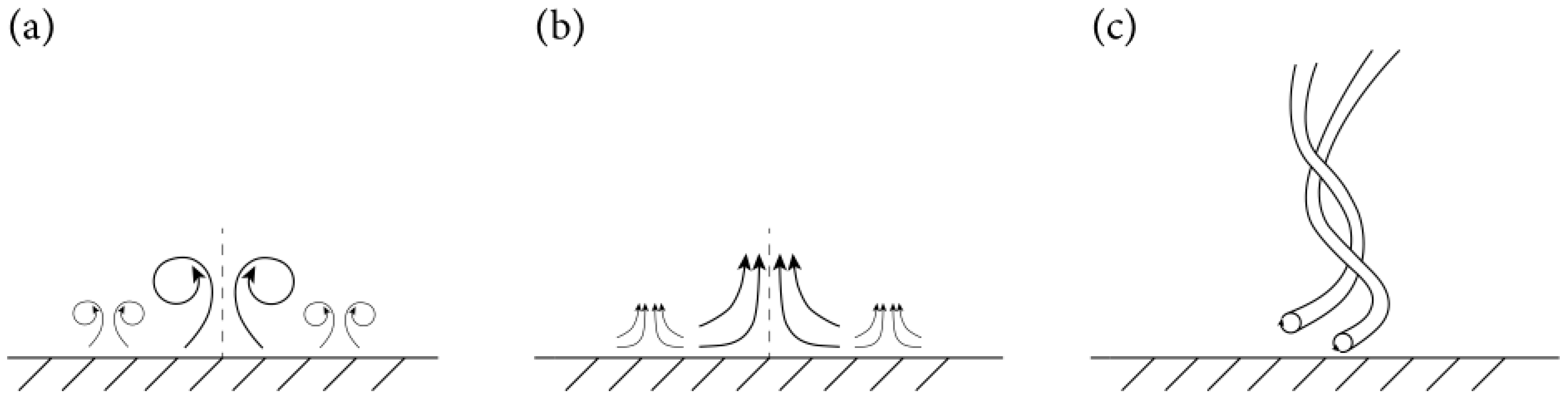
4.2. Main Mechanisms of Tornadogenesis
4.3. Main Factors of Tornadogenesis
5. Conclusions
- (1)
- The ability to plan laboratory studies of the conditions for the generation, stability, and control of significantly non-stationary concentrated vortices;
- (2)
- The ability to improve measurements of the characteristics of real natural vortex structures;
- (3)
- The ability to develop mathematical models that adequately describe the structure and dynamics of both laboratory and natural non-stationary vortices throughout their life cycle (from generation to decay).
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| distance from the axis of symmetry of the vortex, m | |
| universal gas constant, J/(kg K); Earth radius, m | |
| distance from the surface of the Earth, m | |
| , , | velocity components in a cylindrical coordinate system (), m/s |
| , , | velocity components in Cartesian coordinates (), m/s |
| , , | fluctuation velocity components in a cylindrical coordinate system (), m/s |
| particle gravitational settling rate, m/s | |
| debris gravitational settling rate, m/s | |
| pressure of moving air, Pa | |
| pressure of fixed air, Pa | |
| density of moving air, kg/m3 | |
| density of fixed air, kg/m3 | |
| dispersed phase density, kg/m3 | |
| kinematic viscosity, m2/s | |
| turbulent viscosity, m2/s | |
| turbulent thermal diffusivity, m2/s | |
| circulation, m2/s | |
| gravitational acceleration, m/s2 | |
| stream function, m3/s | |
| azimuthal component of vorticity, s−1 | |
| vertical vorticity component, s−1 | |
| first Coriolis parameter, s−1 | |
| second Coriolis parameter, s−1 | |
| temperature, K | |
| averaged (by entropy) temperature of the heat source, K | |
| averaged (by entropy) temperature of heat sink, K | |
| potential temperature, K | |
| wet adiabatic gradient, K/m | |
| mesovortex energy, J | |
| macrovortex energy, J | |
| isobaric heat capacity of air, J/(kg K) | |
| entropy, J/K | |
| volumetric concentration of the dispersed phase | |
| ratio of the densities of the gas and dispersed phases | |
| spin parameter | |
| dimensionless dissipation coefficient | |
| dimensionless dissipation coefficient | |
| thermodynamic efficiency of the “dust devil” | |
| dimensionless current function | |
| radial Reynolds number | |
| vortex Reynolds number | |
| Froude number | |
| Superscripts | |
| deviation from the mean | |
| average (over time) value | |
| Subscripts | |
| air | |
| still air, in the core of the vortex | |
| particles | |
| funnel surface | |
| on the surface of the Earth, in the center, the initial moment of time | |
| at an infinite distance from the center | |
| in the surrounding atmosphere | |
| maximum value | |
References
- Alekseenko, S.; Kuibin, P.; Okulov, V. Theory of Concentrated Vortices: An Introduction; Spinger: Berlin/Heidelberg, Germany, 2007; 506p. [Google Scholar]
- Bluestein, H.B. Severe Convective Storms and Tornadoes: Observations and Dynamics; Spinger Science & Business Media: Berlin/Heidelberg, Germany, 2013; 456p. [Google Scholar]
- Rotunno, R. The fluid dynamics of tornadoes. Annu. Rev. Fluid Mech. 2013, 45, 59–84. [Google Scholar] [CrossRef]
- Varaksin, A.Y.; Romash, M.E.; Kopeitsev, V.N. Tornado; Begell House: New York, NY, USA, 2015; 394p. [Google Scholar]
- Finley, A.J.; Brun, A.S.; Carlsson, M.; Szydlarski, M.; Hansteen, V.; Shoda, M. Stirring the base of the solar wind: On heat transfer and vortex formation. Astron. Astrophys. 2022, 665, A118. [Google Scholar] [CrossRef]
- Barczynski, K.; Schmieder, B.; Peat, A.W.; Labrosse, N.; Mein, P.; Mein, N. Spectro-imagery of an active tornado-like prominence: Formation and evolution. Astron. Astrophys. 2021, 653, A94. [Google Scholar] [CrossRef]
- Vasheghani Farahani, S.; Ghanbari, E.; Ghaffari, G.; Safari, H. Torsional wave propagation in solar tornadoes. Astron. Astrophys. 2017, 599, A19. [Google Scholar] [CrossRef][Green Version]
- Agee, E.; Taylor, L. Historical analysis of U.S. tornado fatalities (1808–2017): Population, science and technology. Weather Clim. Soc. 2019, 11, 355–368. [Google Scholar] [CrossRef]
- Anderson-Frey, A.K. Tornado fatalities: An environmental perspective. Weather Forecast. 2019, 34, 1999–2015. [Google Scholar] [CrossRef]
- Alexander, C.R.; Wurman, J. The 30 May 1998 Spencer, South Dakota, storm. Part I: The structural evolution and environment of the tornadoes. Mon. Weather Rev. 2005, 133, 72–96. [Google Scholar] [CrossRef]
- Wurman, J.; Alexander, C.R. The 30 May 1998 Spencer, South Dakota, storm. Part II: Comparison of observed damage and radar-derived winds in the tornadoes. Mon. Weather Rev. 2005, 133, 97–119. [Google Scholar] [CrossRef]
- Kosiba, K.A.; Wurman, J.; Richardson, Y.; Markowski, P.; Robinson, P.; Marquis, J.N. Genesis of the Goshen County, Wyoming, tornado on 5 June 2009 during VORTEX2. Mon. Weather Rev. 2013, 141, 1157–1182. [Google Scholar] [CrossRef]
- Trapp, R.J.; Kosiba, K.A.; Marquis, J.N.; Kumjian, M.R.; Nesbitt, S.W.; Wurman, J.; Salio, P.; Grover, M.A.; Robinson, P.; Hence, D.A. Multi-platform and multiple-doppler radar observations of a supercell thunderstorm in South America during RELAMPAGO. Mon. Weather Rev. 2020, 148, 3225–3241. [Google Scholar] [CrossRef]
- Ying, S.J.; Chang, C.C. Exploratory model study of tornado-like vortex dynamics. J. Atmos. Sci. 1970, 27, 3–14. [Google Scholar] [CrossRef]
- Wan, C.A.; Chang, C.C. Measurement of the velocity field in a simulated tornado-like vortex using a three-dimensional velocity probe. J. Atmos. Sci. 1972, 29, 116–127. [Google Scholar] [CrossRef]
- Ward, N.B. The exploration of certain features of tornado dynamics using laboratory model. J. Atmos. Sci. 1972, 29, 1194–1204. [Google Scholar] [CrossRef]
- Fizjarrald, D.E. A laboratory simulation of convective vortices. J. Atmos. Sci. 1973, 30, 894–902. [Google Scholar] [CrossRef]
- Church, C.R.; Snow, J.T.; Agee, E.M. Tornado vortex simulation at Purdue University. Bull. Amer. Meteorol. Soc. 1977, 58, 900–908. [Google Scholar] [CrossRef]
- Baker, G.; Church, C.R. Measurements of core radii and peak velocities in modeled atmospheric vortices. J. Atmos. Sci. 1979, 36, 2413–2424. [Google Scholar] [CrossRef]
- Snow, J.T.; Church, C.R.; Barnhart, B.J. An investigation of the surface pressure fields beneath simulated tornado cyclones. J. Atmos. Sci. 1980, 37, 1013–1025. [Google Scholar] [CrossRef]
- Kuai, L.; Haan, F.L.; Gallus, W.A.; Sarkar, P.P. CFD simulations of the flow field of a laboratory-simulated tornado for parameter sensitivity studies and comparison with field measurements. Wind Struct. 2008, 11, 75–96. [Google Scholar] [CrossRef]
- Haan, F.L.; Sarkar, P.P.; Gallus, W.A. Design, construction and performance of a large tornado simulator for wind engineering applications. Eng. Struct. 2008, 30, 1146–1159. [Google Scholar] [CrossRef]
- Varaksin, A.Y.; Romash, M.E.; Kopeitsev, V.N.; Taekin, S.I. The possibility of physical simulation of air tornados under laboratory conditions. High Temp. 2008, 46, 888–891. [Google Scholar] [CrossRef]
- Varaksin, A.Y.; Romash, M.E.; Taekin, S.I.; Kopeitsev, V.N. The generation of free concentrated air vortexes under laboratory conditions. High Temp. 2009, 47, 78–82. [Google Scholar] [CrossRef]
- Varaksin, A.Y.; Romash, M.E.; Kopeitsev, V.N. The parameters of unstable stratification of air leading to generation of free vortexes. High Temp. 2010, 48, 251–255. [Google Scholar] [CrossRef]
- Yih, C.-S. Tornado-like flows. Phys. Fluids 2007, 19, 076601–1–076601-6. [Google Scholar] [CrossRef]
- Shtern, V.; Borissov, A.; Hussain, F. Vortex-sinks with axial flows: Solution and applications. Phys. Fluids 1997, 9, 2941–2959. [Google Scholar] [CrossRef]
- Arsen’ev, S.A.; Nikolaevskii, V.N.; Shelkovnikov, N.K. Eddy instability and origination of whirlwinds and tornadoes. Mosc. Univ. Phys. Bull. 2000, 55, 57–61. [Google Scholar]
- Khrgian, A.K. Atmospheric Physics; Gidrometeoizdat: Leningrad, Russia, 1978; 318p. (In Russian) [Google Scholar]
- Shuleikin, V.V. Calculation of Development, Movement, and Attenuation of Tropical Storms and Major Waves Produced by Hurricanes; Gidrometeoizdat: Leningrad, Russia, 1978; 96p. (In Russian) [Google Scholar]
- Gutman, L.N. Theoretical model of a tornado. Izv. Akad. Nauk SSSR Ser. Geofiz 1957, 79–93. (In Russian) [Google Scholar]
- Lewis, W.; Perkins, P.J. Recorded pressure distribution in the outer portion of a tornado vortex. Mon. Weather Rev. 1953, 81, 379–385. [Google Scholar] [CrossRef]
- Kuo, H.L. On the dynamics of convective atmospheric vortices. J. Atmos. Sci. 1966, 23, 25–42. [Google Scholar] [CrossRef]
- Kuo, H.L. Note on the similarity solutions of the vortex equations in an unstably stratified atmosphere. J. Atmos. Sci. 1967, 24, 95–97. [Google Scholar] [CrossRef]
- Mal’bakhov, V.M.; Gutman, L.N. Nonstationary problem about meso-scale atmospheric vortices with vertical axis. Izv. Akad. Nauk SSSR Fiz. Atmos. Okeana 1968, 4, 586–598. (In Russian) [Google Scholar]
- Mal’bakhov, V.M. Investigation of tornado structure. Izv. Akad. Nauk SSSR Fiz. Atmos. Okeana 1972, 8, 17–28. (In Russian) [Google Scholar]
- Arsen’ev, S.A.; Babkin, V.A.; Gubar’, A.Y.; Nikolaevskiy, V.N. Theory of Mesoscale Turbulence: Vortices of the Atmosphere and Ocean; Inst. Komp’yut. Issled.: Izhevsk, Russia, 2010; 308p. (In Russian) [Google Scholar]
- Kurganskii, M.V. A simple hydrodynamic model of tornado-like vortices. Izv. Atmos. Oceanic Phys. 2015, 51, 292–298. [Google Scholar] [CrossRef]
- Holton, J.R. An Introduction to Dynamic Meteorology; Academic Press: San Diego, CA, USA, 1992; 507p. [Google Scholar]
- Ambaum, M.H.P. Thermal Physics of the Atmosphere; Wiley-Blackwell: Chichester, UK, 2010; 256p. [Google Scholar]
- Davies-Jones, R. A review of supercell and tornado dynamics. Atmos. Res. 2015, 158, 274–291. [Google Scholar] [CrossRef]
- Kurgansky, M.V. A simple model of dry convective helical vortices (with applications to the atmospheric dust devil. Dyn. Atmos. Oceans 2005, 40, 151–162. [Google Scholar] [CrossRef]
- Kurgansky, M.V. Simple models of helical baroclinic vortices. Proc. IUTAM 2013, 7, 193–202. [Google Scholar] [CrossRef]
- Davies-Jones, R.P.; Kessler, E. Tornadoes. In Weather and Climate Modification; Hess, W.N., Ed.; Jon Wiley and Sons: New York, NY, USA, 1974; pp. 552–595. [Google Scholar]
- Lewellen, W.S. Theoretical Models of the Tornado Vortex. In Weather and Climate Modification, Proceedings of the A Simposium on Tornadoes Assessment of Knowledge and Implications for Man, Lubbock, TX, USA, 22–24 June 1976; Hess, W.N., Ed.; Texas Tech. Univ.: Lubbock, TX, USA, 1976; pp. 107–143. [Google Scholar]
- Snow, J.T.; Pauley, R.L. On the thermodynamic method for estimating tornado windspeeds. J. Clim. Appl. Meteorol. 1984, 23, 1465–1468. [Google Scholar] [CrossRef]
- Fiedler, B.H.; Rotunno, R. A theory for the maximum windspeeds in tornado-like vortices. J. Atmos. Sci. 1986, 43, 2328–2340. [Google Scholar] [CrossRef]
- Renno, N.O.; Ingersoll, A.P. Natural convection as a heat engine: A theory for CAPE. J. Atmos. Sci. 1996, 53, 572–585. [Google Scholar] [CrossRef]
- Shuleikin, V.V. Sea Physics; Nauka: Moscow, Russia, 1990. (In Russian) [Google Scholar]
- Renno, N.O.; Burkett, M.L.; Larkin, M.P. A simple thermodynamical theory for dust devils. J. Atmos. Sci. 1998, 55, 3244–3252. [Google Scholar] [CrossRef]
- Renno, N.O. A simple theory for waterspouts. J. Atmos. Sci. 2001, 58, 927–932. [Google Scholar] [CrossRef]
- Sinclair, P.C. General characteristics of dust devils. J. Appl. Meteorol. 1969, 8, 32–45. [Google Scholar] [CrossRef]
- Sinclair, P.C. The lower structure of dust devils. J. Atmos. Sci. 1973, 30, 1599–1619. [Google Scholar] [CrossRef]
- Golden, J.H. The life cycle of Florida Keys’ waterspouts. J. Appl. Meteorol. 1974, 13, 676–692. [Google Scholar] [CrossRef]
- Golden, J.H. Scale-interaction implications for the waterspout life cycle. II. J. Appl. Meteorol. 1974, 13, 693–709. [Google Scholar] [CrossRef]
- Simpson, J.; Morton, B.R.; McCumber, M.C.; Penc, R.S. Observations and mechanisms of GATE waterspouts. J. Atmos. Sci. 1986, 43, 753–782. [Google Scholar] [CrossRef]
- Golden, J.H.; Bluestein, H.B. The NOAA-National Geographic Society waterspout expedition (1993). Bull. Amer. Meteorol. Soc. 1994, 75, 2281–2288. [Google Scholar] [CrossRef]
- Romps, D.M. Clausius-Clapeyron scaling of CAPE from analytical solutions to RCE. J. Atmos. Sci. 2016, 73, 3719–3737. [Google Scholar] [CrossRef]
- Mapes, B.E. Water’s two height scales: The moist adiabat and the radiative troposphere. Quart. J. Roy. Meteorol. Soc. 2001, 127, 2353–2366. [Google Scholar] [CrossRef]
- Singh, M.S.; O’Gorman, P.A. Influence of entrainment on the thermal stratification in simulations of radiative-convective equilibrium. Geophys. Res. Lett. 2013, 40, 4398–4403. [Google Scholar] [CrossRef]
- Arsen’ev, S.A.; Gubar’, A.Y.; Nikolaevskiy, V.N. Self-organization of tornado and hurricanes in atmospheric currents with meso-scale eddies. Dokl. Earth Sci. 2004, 396, 588–593. [Google Scholar]
- Arsen’ev, S.A.; Gubar’, A.Y.; Shelkovnikov, N.K. Generation of typhoons and hurricanes by a mesoscale turbulence. Mosc. Univ. Phys. Bull. 2007, 62, 113–117. [Google Scholar] [CrossRef]
- Gubar’, A.Y.; Avetisyan, A.I.; Babkova, V.V. Tornado rise: 3D numerical model in mesoscale turbulence theory of Nikolaevskiy. Dokl. Earth Sci. 2008, 419, 467–472. [Google Scholar] [CrossRef]
- Nikolaevskiy, V.N.; Gubar’, A.Y. Vortices and tornado in mesoscale turbulence theory: A numerical pattern of 3D tornado rise. Geofiz. Issled. 2014, 15, 65–82. (In Russian) [Google Scholar]
- Koprov, B.M.; Koprov, V.M.; Makarova, T.I.; Golitsyn, G.S. Coherent structures in the atmospheric surface layer under stable and unstable conditions. Bound.-Later Meteorol. 2004, 111, 19–32. [Google Scholar] [CrossRef]
- Golitsyn, G.S.; Gostintsev, Y.A.; Solodovnik, A.S. Turbulent floating jet in a stratified atmosphere. J. Appl. Mech. Tech. Phys. 1989, 30, 566–577. [Google Scholar] [CrossRef]
- Etkin, D.A. Beyond the year 2000, more tornadoes in Western Canada—Implifications from the historical record. Nat. Hazards 1995, 12, 19–27. [Google Scholar] [CrossRef]
- Golitsyn, G.S. Statistics and energetics of tropical cyclones. Dokl. Earth Sci. 1997, 354, 633–636. [Google Scholar]
- Nolan, D.S.; Farrell, B.F. The structure and dynamics of tornado-like vortices. J. Atmos. Sci. 1999, 56, 2908–2936. [Google Scholar] [CrossRef]
- Rotunno, R. Numerical simulation of a laboratory vortex. J. Atmos. Sci. 1977, 34, 1942–1956. [Google Scholar] [CrossRef]
- Rotunno, R. A study in tornado like vortex dynamics. J. Atmos. Sci. 1979, 36, 140–155. [Google Scholar] [CrossRef]
- Rotunno, R. An investigation of a three dimensional asymmetric vortex. J. Atmos. Sci. 1984, 41, 283–298. [Google Scholar] [CrossRef]
- Gall, R.L. Internal dynamics of tornado-like vortices. J. Atmos. Sci. 1982, 39, 2721–2736. [Google Scholar] [CrossRef]
- Walko, R.; Gall, R.L. Some effects of momentum diffusion on axisymmetric vortices. J. Atmos. Sci. 1986, 43, 2137–2148. [Google Scholar] [CrossRef]
- Lewellen, W.S.; Lewellen, D.C.; Sykes, R.I. Large-eddy simulation of a tornado’s interaction with the surface. J. Atmos. Sci. 1997, 54, 581–605. [Google Scholar] [CrossRef]
- Lewellen, D.C.; Lewellen, W.S.; Xia, J. The influence of a local swirl ration on tornado intensification near the surface. J. Atmos. Sci. 2000, 57, 527–544. [Google Scholar] [CrossRef]
- Xia, J.; Lewellen, W.S.; Lewellen, D.C. Influence of Mach number on tornado corner flow dynamics. J. Atmos. Sci. 2003, 60, 2820–2825. [Google Scholar] [CrossRef]
- Lewellen, D.C.; Lewellen, W.S. Near-surface intensification of tornado vortices. J. Atmos. Sci. 2007, 64, 2176–2194. [Google Scholar] [CrossRef]
- Lewellen, D.C.; Lewellen, W.S. Near-surface vortex intensification through corner flow collapse. J. Atmos. Sci. 2007, 64, 2195–2209. [Google Scholar] [CrossRef]
- Ishihara, T.; Oh, S.; Tokuyama, Y.A. Numerical study on flow fields of tornado-like vortices using the LES turbulence model. J. Wind Eng. Ind. Aerodyn. 2011, 99, 239–248. [Google Scholar] [CrossRef]
- Ishihara, T.; Liu, Z.Q. Numerical study on dynamics of a tornado-like vortex with touching down by using the LES turbulence model. Wind Struct. 2014, 19, 89–111. [Google Scholar] [CrossRef][Green Version]
- Liu, Z.Q.; Ishihara, T. Numerical study of turbulent flow fields and the similarity of tornado vortices using large-eddy simulations. J. Wind Eng. Ind. Aerodyn. 2015, 145, 42–60. [Google Scholar] [CrossRef]
- Varaksin, A.Y.; Romash, M.E.; Kopeitsev, V.N. The possibilities of visualization in the case of simulation of air tornados. High Temp. 2010, 48, 588–592. [Google Scholar] [CrossRef]
- Varaksin, A.Y.; Romash, M.E.; Kopeitsev, V.N.; Gorbachev, M.A. Physical simulation of air tornados: Some dimensionless parameters. High Temp. 2011, 49, 310–313. [Google Scholar] [CrossRef]
- Varaksin, A.Y.; Protasov, M.V.; Teplitskii, Y.S. About choice of particle parameters for visualization and diagnostics of free concentrated air vortices. High Temp. 2014, 52, 554–559. [Google Scholar] [CrossRef]
- Varaksin, A.Y.; Romash, M.E.; Kopeitsev, V.N. Effect of net structures on wall-free non-stationary air heat vortices. Int. J. Heat Mass Transf. 2013, 64, 817–828. [Google Scholar] [CrossRef]
- Varaksin, A.Y.; Zaichik, L.I. The effect of a fine divided impurity on the turbulence intensity of a carrier flow in a pipe. High Temp. 1998, 36, 983–986. [Google Scholar]
- Zaichik, L.I.; Varaksin, A.Y. Effect of the wake behind large particles on the turbulence intensity of carrier flow. High Temp. 1999, 37, 655–658. [Google Scholar]
- Varaksin, A.Y.; Ryzhkov, S.V. Vortex flows with particles and droplets (a review). Symmetry 2022, 14, 2016. [Google Scholar] [CrossRef]
- Varaksin, A.Y.; Ryzhkov, S.V. Turbulence in two-phase flows with macro-, micro- and nanoparticles: A review. Symmetry 2022, 14, 2433. [Google Scholar] [CrossRef]
- Klemp, J.B.; Wilhelmson, R.B. The simulation of three-dimensional convective storm dynamics. J. Atmos. Sci. 1978, 35, 1070–1096. [Google Scholar] [CrossRef]
- Wicker, L.J.; Wilhelmson, R.B. Simulation and analysis of tornado development and decay within a three-dimensional supercell thunderstorm. J. Atmos. Sci. 1995, 52, 2675–2703. [Google Scholar] [CrossRef]
- Grasso, L.D.; Cotton, W.R. Numerical simulation of a tornado vortex. J. Atmos. Sci. 1995, 52, 1192–1203. [Google Scholar] [CrossRef]
- Markowski, P.M.; Straka, J.M.; Rasmussen, E.N. Tornadogenesis resulting from the transport of circulation by a downdraft: Idealized numerical simulations. J. Atmos. Sci. 2003, 60, 795–823. [Google Scholar] [CrossRef]
- Sinkevich, O.A. A model of the flow in tornado vortex in a view of phase transitions. High Temp. 1996, 34, 922–927. [Google Scholar]
- Sinkevich, O.A.; Chikunov, S.E. Numerical simulation of two-phase flow in a tornado funnel. High Temp. 2002, 40, 604–612. [Google Scholar] [CrossRef]
- Smirnov, E.P. A numerical study of hydrodynamics and heat and mass transfer of a two-phase flow in an atmospheric tornado-forming cloud and a tornado model. High Temp. 2016, 54, 281–288. [Google Scholar] [CrossRef]
- Kushin, V.V. Smerch (Tornado); Energoatomizdat: Moscow, Russia, 1993. (In Russian) [Google Scholar]
- Gu, Z.; Zhao, Y.; Li, Y.; Yu, Y.; Feng, X. Numerical simulation of dust lifting within dust devils—Simulation of an intense vortex. J. Atmos. Sci. 2006, 63, 2630–2641. [Google Scholar] [CrossRef]
- Lebedeva, N.A.; Osiptsov, A.N. Structure of inertial-admixture accumulation zones in a tornado-like flow. Fluid Dyn. 2009, 44, 68–79. [Google Scholar] [CrossRef]
- Gol’dshtik, M.A. A paradoxical solution of the Navier-Stokes equations. Prikl. Mat. Mekh. 1960, 24, 610–624. (In Russian) [Google Scholar] [CrossRef]
- Varaksin, A.Y. Effect of macro-, micro- and nanoparticles on turbulence in a carrier gas. Dokl. Phys. 2021, 66, 72–75. [Google Scholar] [CrossRef]
- Liu, S.; Wang, Z.; Gong, Z.; Chen, F.; Peng, Q. Physically based modeling and animation of tornado. J. Zhejiang Univ. Sci. A 2006, 7, 1099–1106. [Google Scholar] [CrossRef]
- Liu, S.; Wang, Z.; Gong, Z.; Peng, Q. Real time simulation of a tornado. Vis. Comput. 2007, 23, 559–567. [Google Scholar] [CrossRef]
- Maruyama, T. Simulation of flying debris using a numerically generated tornado-like vortex. J. Wind Eng. Ind. Aerodyn. 2011, 99, 249–256. [Google Scholar] [CrossRef]
- Lewellen, D.C.; Gong, B.; Lewellen, W.S. Effect of finescale debris on near-surface tornado dynamics. J. Atmos. Sci. 2008, 65, 3247–3262. [Google Scholar] [CrossRef]
- Davies-Jones, R.P. Tornado dynamics. In Thunderstorm Morphology and Dynamics, 2nd ed.; Kessler, E., Ed.; University of Oklahoma Press: Norman, OK, USA, 1986; pp. 197–236. [Google Scholar]
- Wakimoto, R.; Wilson, J.W. Non-supercell tornadoes. Mon. Weather Rev. 1989, 117, 1113–1140. [Google Scholar] [CrossRef]
- Brady, R.H.; Szoke, E.J. A case study of non-mesocyclone tornado development in northeast Colorado: Similarities to waterspout formation. Mon. Weather Rev. 1989, 117, 843–856. [Google Scholar] [CrossRef]
- Davies-Jones, R. Can a descending rain curtain in a supercell instigate tornadogenesis barotropically? Atmos. Res. 2008, 65, 2469–2497. [Google Scholar] [CrossRef][Green Version]
- Roberts, B.; Xue, M.; Schenkman, A.D.; Dawson, D.T. The role of surface drag in tornadogenesis within an idealized supercell simulation. J. Atmos. Sci. 2016, 73, 3371–3395. [Google Scholar] [CrossRef]
- Roberts, B.; Xue, M.; Dawson, D.T. The effect of surface drag strength on mesocyclone intensification and tornadogenesis in idealized supercell simulations. J. Atmos. Sci. 2020, 77, 1699–1721. [Google Scholar] [CrossRef]
- Davies-Jones, R. Invented forces in supercell models. J. Atmos. Sci. 2021, 78, 2927–2939. [Google Scholar] [CrossRef]
- Markowski, P.M.; Bryan, G. LES of laminar flow in the PBL: A potential problem for convective storm simulations. Mon. Weather Rev. 2016, 144, 1841–1850. [Google Scholar] [CrossRef]
- Kuzenov, V.V.; Ryzhkov, S.V. Numerical simulation of the interaction of a magneto-inertial fusion target with plasma and laser drivers. High Temp. 2022, 60, S7–S15. [Google Scholar] [CrossRef]
- Kuzenov, V.V.; Ryzhkov, S.V. Thermophysical Parameter Estimation of a Neutron Source Based on the Action of Broadband Radiation on a Cylindrical Target. Fusion Sci. Technol. 2023, 79, 399–406. [Google Scholar] [CrossRef]
- Ryzhkov, S.V. The behavior of a magnetized plasma under the action of laser with high pulse energy. Probl. At. Sci. Technol. 2010, 4, 105–110. [Google Scholar]
- Kuzenov, V.V.; Ryzhkov, S.V. Evaluation of hydrodynamic instabilities in inertial confinement fusion target in a magnetic field. Probl. At. Sci. Technol. 2013, 4, 103–107. [Google Scholar]
- Flournoy, M.D.; Coniglio, M.C. Origins of vorticity in a simulated tornadic mesovortex observed during PECAN on 6 July 2015. Mon. Weather Rev. 2019, 147, 107–134. [Google Scholar] [CrossRef]
- Fischer, J.; Dahl, J.M.L. Transition of near-ground vorticity dynamics during tornadogenesis. J. Atmos. Sci. 2022, 79, 467–483. [Google Scholar] [CrossRef]
- Parker, M.D.; Wicker, L.J. Imported and storm-generated near-ground vertical vorticity in a simulated supercell. J. Atmos. Sci. 2014, 71, 3027–3051. [Google Scholar]
- Dahl, J.M.L. Near-ground rotation in simulated supercells: On the robustness of the baroclinic mechanism. Mon. Weather Rev. 2015, 143, 4929–4942. [Google Scholar] [CrossRef]
- Parker, M.D.; Dahl, J.M.L. Production of near-surface vertical vorticity by idealized downdrafts. Mon. Weather Rev. 2015, 143, 2795–2816. [Google Scholar] [CrossRef]
- Markowski, P.M. An idealized numerical simulation investigation of the effects of surface drag on the development of near-surface vertical vorticity in supercell thunderstorms. J. Atmos. Sci. 2016, 73, 4349–4385. [Google Scholar] [CrossRef]
- Fischer, J.; Dahl, J.M.L. The relative importance of updraft and cold pool characteristics in supercell tornadogenesis using highly idealized simulations. J. Atmos. Sci. 2020, 77, 4089–4107. [Google Scholar] [CrossRef]
- Mashiko, W.; Niino, H.; Kato, T. Numerical simulation of tornadogenesis in an outer-rainband minisupercell of Typhoon Shanshan on 17 September 2006. Mon. Weather Rev. 2009, 137, 4238–4260. [Google Scholar] [CrossRef]
- Mashiko, W. A numerical study of the 6 May 2012 Tsukuba City supercell tornado. Part II: Mechanisms of tornadogenesis. Mon. Weather Rev. 2016, 144, 3077–3098. [Google Scholar] [CrossRef]
- Kuzenov, V.V.; Ryzhkov, S.V.; Yu; Varaksin, A. Simulation of Parameters of Plasma Dynamics of a Magneto Plasma Compressor. Appl. Sci. 2023, 13, 5538. [Google Scholar] [CrossRef]
- Rotunno, R.; Markowski, P.M.; Bryan, G.H. “Near ground” vertical vorticity in supercell thunderstorm models. J. Atmos. Sci. 2017, 74, 1757–1766. [Google Scholar] [CrossRef]
- Yokota, S.; Niino, H.; Seko, H.; Kunii, M.; Yamauchi, H. Important factors for tornadogenesis as revealed by high-resolution ensemble forecasts of the Tsukuba supercell tornado of 6 May 2012 in Japan. Mon. Weather Rev. 2018, 146, 1109–1132. [Google Scholar] [CrossRef]
- Tao, T.; Tamura, T. Numerical study of the 6 May 2012 Tsukuba supercell tornado: Vorticity sources responsible for tornadogenesis. Mon. Weather Rev. 2020, 148, 1205–1228. [Google Scholar] [CrossRef]
- Boyer, C.H.; Dahl, J.M.L. The mechanisms responsible for large near-surface vertical vorticity within simulated supercells and quasi-linear storms. Mon. Weather Rev. 2020, 148, 4281–4297. [Google Scholar] [CrossRef]
- Varaksin, A.Y.; Mochalov, A.A. A double screw spiral as a possible mechanism of wall-free nonstationary air vortex generation. Dokl. Phys. 2019, 64, 301–303. [Google Scholar] [CrossRef]
- Kuzenov, V.V.; Ryzhkov, S.V.; Varaksin, A.Y. The adaptive composite block-structured grid calculation of the gas-dynamic characteristics of an aircraft moving in a gas environment. Mathematics 2022, 10, 2130. [Google Scholar] [CrossRef]
- Kuzenov, V.V.; Ryzhkov, S.V. Approximate calculation of convective heat transfer near hypersonic aircraft surface. J. Enhanc. Heat Transf. 2018, 25, 181–193. [Google Scholar] [CrossRef]
- Kuzenov, V.V.; Ryzhkov, S.V. Numerical simulation of pulsed jets of a high-current pulsed surface discharge. Comput. Therm. Sci. 2021, 13, 45–56. [Google Scholar] [CrossRef]
- Kuzenov, V.V.; Ryzhkov, S.V.; Varaksin, A.Y. Calculation of heat transfer and drag coefficients for aircraft geometric models. Appl. Sci. 2022, 12, 11011. [Google Scholar] [CrossRef]
- Varaksin, A.Y.; Ryzhkov, S.V. Particle-laden and droplet-laden two-phase flows past bodies (a Review). Symmetry 2023, 15, 388. [Google Scholar] [CrossRef]
- Kuzenov, V.V.; Ryzhkov, S.V. The qualitative and quantitative study of radiation sources with a model configuration of the electrode system. Symmetry 2021, 13, 927. [Google Scholar] [CrossRef]
- Kuzenov, V.V.; Ryzhkov, S.V. Estimation of the neutron generation in the combined magneto-inertial fusion scheme. Phys. Scripta. 2021, 96, 125613. [Google Scholar] [CrossRef]
- Kuzenov, V.V.; Ryzhkov, S.V.; Frolko, P.A. Numerical simulation of the coaxial magneto-plasma accelerator and non-axisymmetric radio frequency discharge. J. Phys. Conf. Ser. 2017, 830, 012049. [Google Scholar] [CrossRef]
- Mozgovoy, A.G.; Romadanov, I.V.; Ryzhkov, S.V. Formation of a compact toroid for enhanced efficiency. Phys. Plasmas 2014, 21, 022501. [Google Scholar] [CrossRef]
- Kuzenov, V.V.; Ryzhkov, S.V.; Varaksin, A.Y. Computational and Experimental Modeling in Magnetoplasma Aerodynamics and High-Speed Gas and Plasma Flows (A Review). Aerospace 2023, 10, 662. [Google Scholar]
- Ryzhkov, S.V. Magneto-Inertial Fusion and Powerful Plasma Installations (A Review). Appl. Sci. 2023, 13, 6658. [Google Scholar] [CrossRef]
- Kuzenov, V.V.; Ryzhkov, S.V. Calculation of plasma dynamic parameters of the magneto-inertial fusion target with combined exposure. Phys. Plasmas 2019, 26, 092704. [Google Scholar] [CrossRef]
- Varaksin, A.Y.; Ryzhkov, S.V. Mathematical Modeling of Gas-Solid Two-Phase Flows: Problems, Achievements and Perspectives (A Review). Mathematics 2023, 11, 3290. [Google Scholar] [CrossRef]
- Markowski, P.M.; Richardson, Y.P. The influence of environmental low-level shear and cold pools on tornadogenesis: Insights from idealized simulations. J. Atmos. Sci. 2014, 71, 243–275. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Varaksin, A.Y.; Ryzhkov, S.V. Mathematical Modeling of Structure and Dynamics of Concentrated Tornado-like Vortices: A Review. Mathematics 2023, 11, 3293. https://doi.org/10.3390/math11153293
Varaksin AY, Ryzhkov SV. Mathematical Modeling of Structure and Dynamics of Concentrated Tornado-like Vortices: A Review. Mathematics. 2023; 11(15):3293. https://doi.org/10.3390/math11153293
Chicago/Turabian StyleVaraksin, Aleksey Yu., and Sergei V. Ryzhkov. 2023. "Mathematical Modeling of Structure and Dynamics of Concentrated Tornado-like Vortices: A Review" Mathematics 11, no. 15: 3293. https://doi.org/10.3390/math11153293
APA StyleVaraksin, A. Y., & Ryzhkov, S. V. (2023). Mathematical Modeling of Structure and Dynamics of Concentrated Tornado-like Vortices: A Review. Mathematics, 11(15), 3293. https://doi.org/10.3390/math11153293





