Localized Method of Fundamental Solutions for Two-Dimensional Inhomogeneous Inverse Cauchy Problems
Abstract
:1. Introduction
2. The Inhomogeneous Inverse Cauchy Problem
3. Solution Procedure
3.1. The Recursive Multiple Reciprocity Technique (RC-MRM)
3.2. Introduction of the LMFS
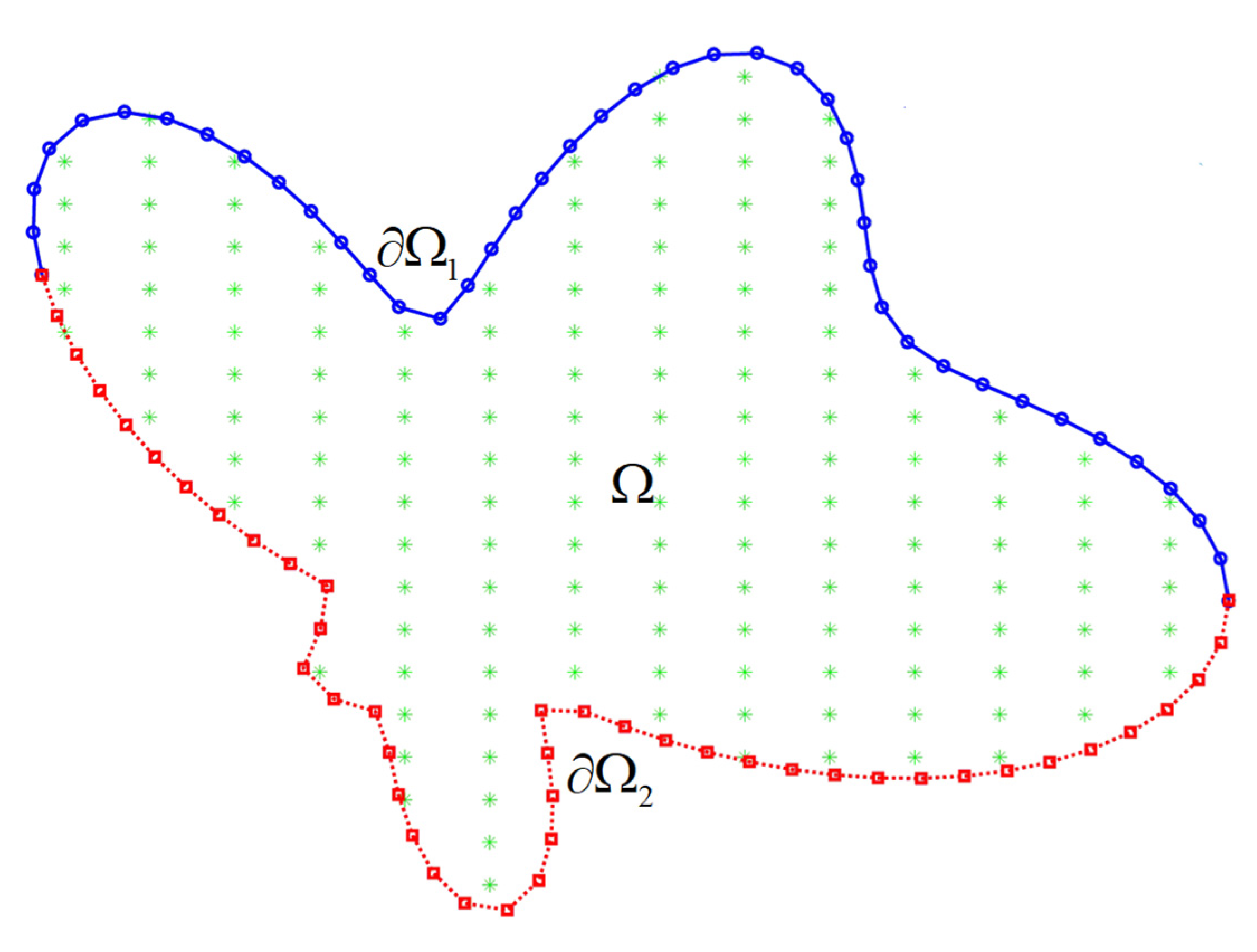
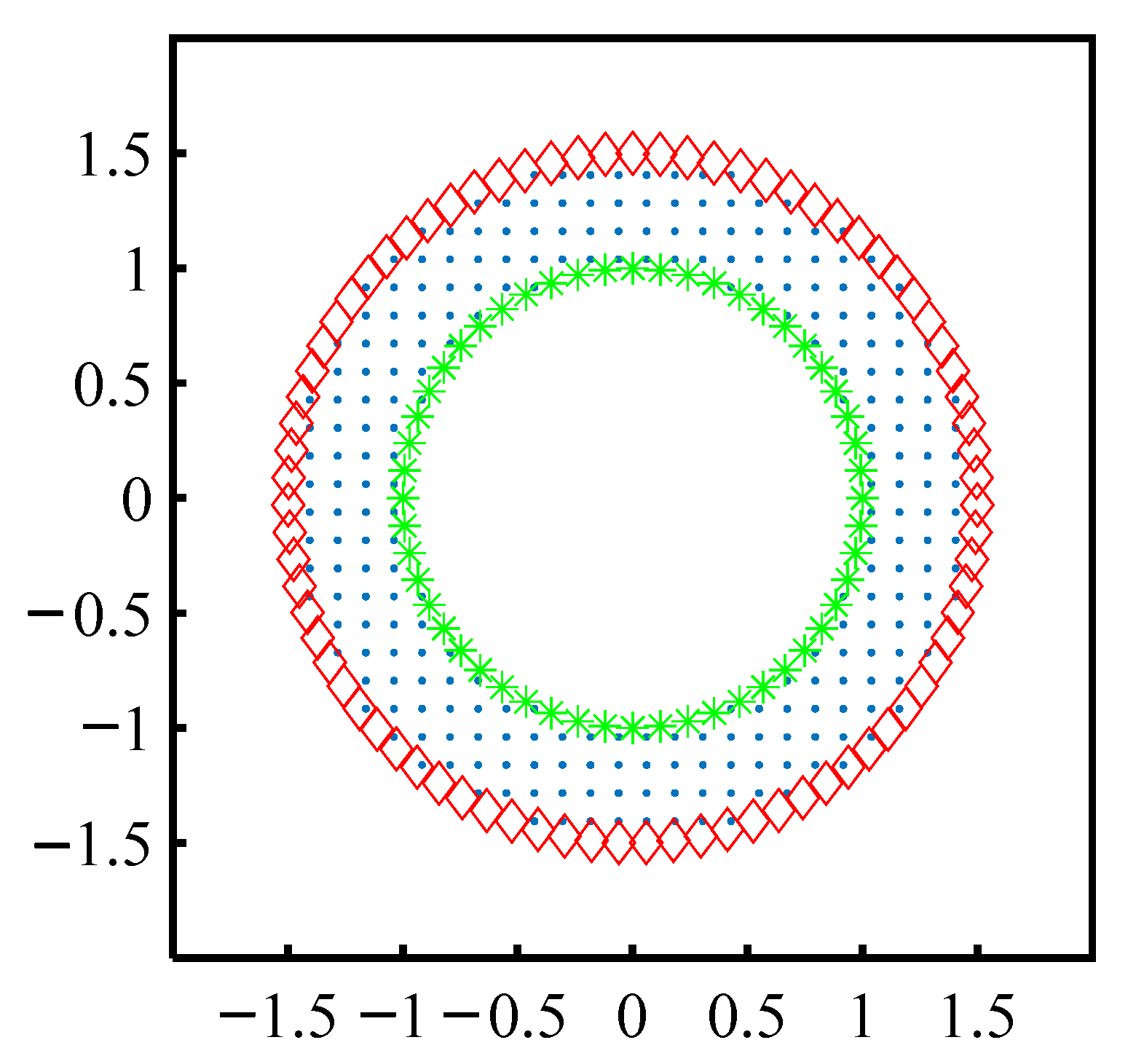
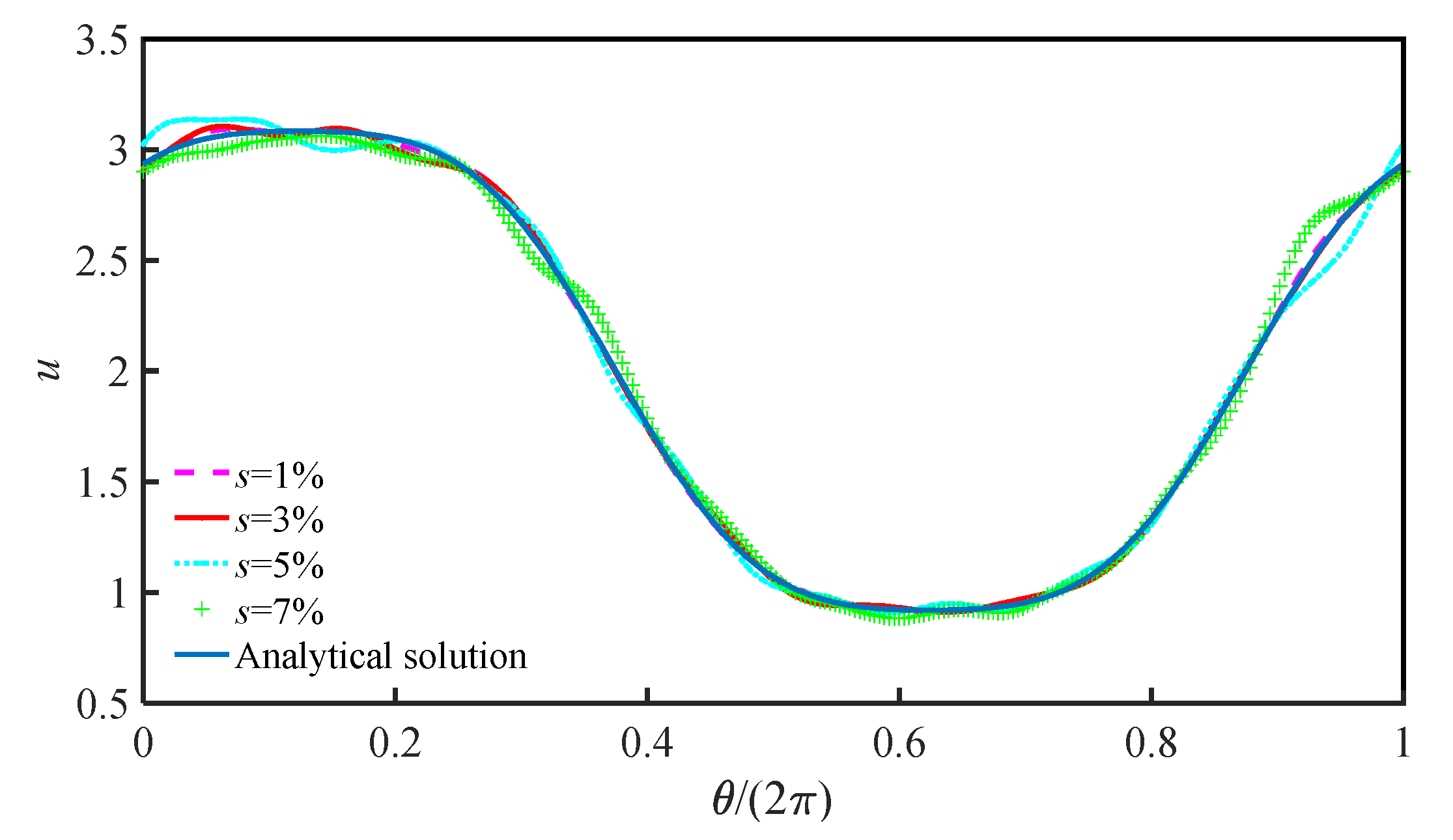
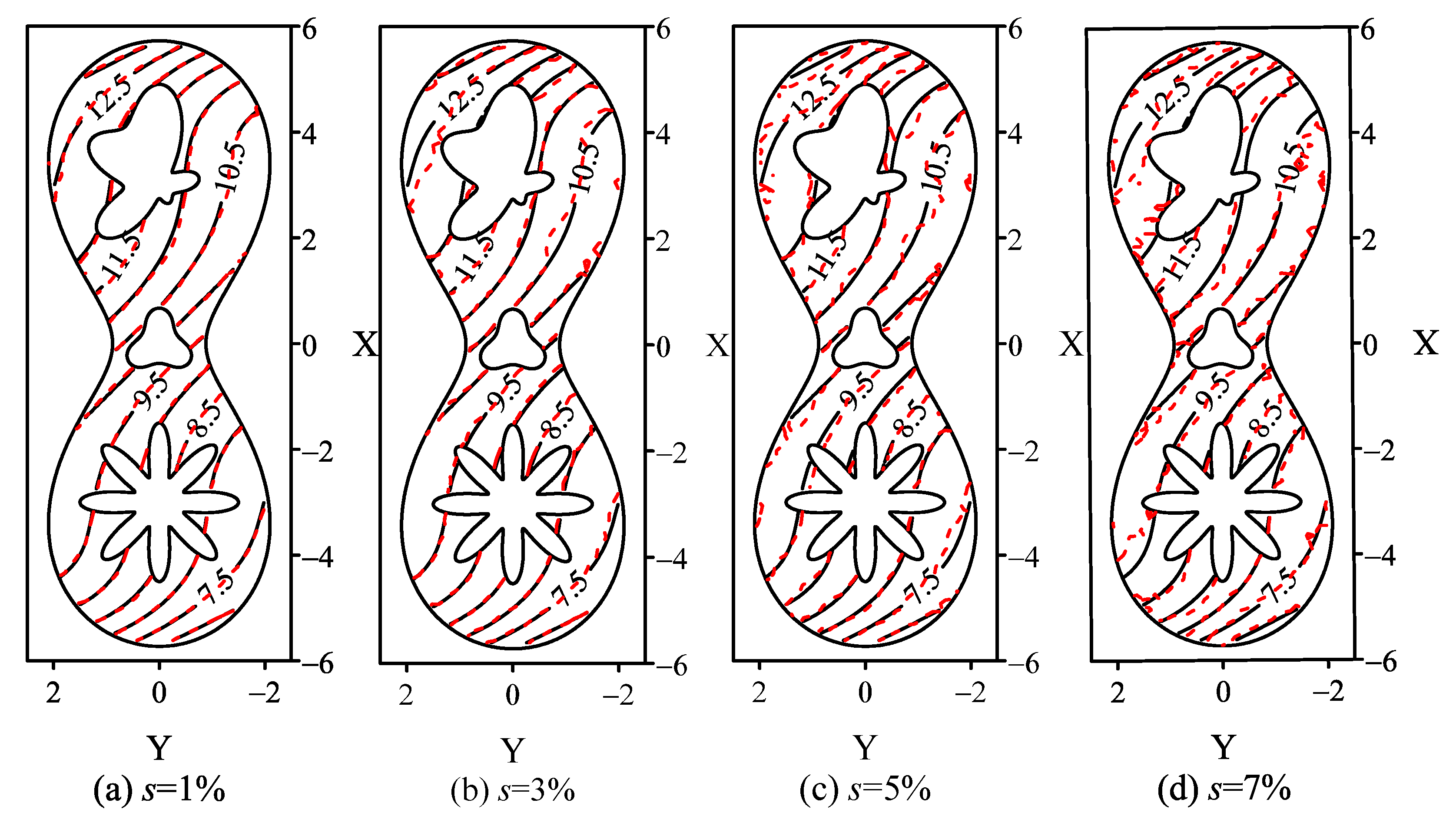
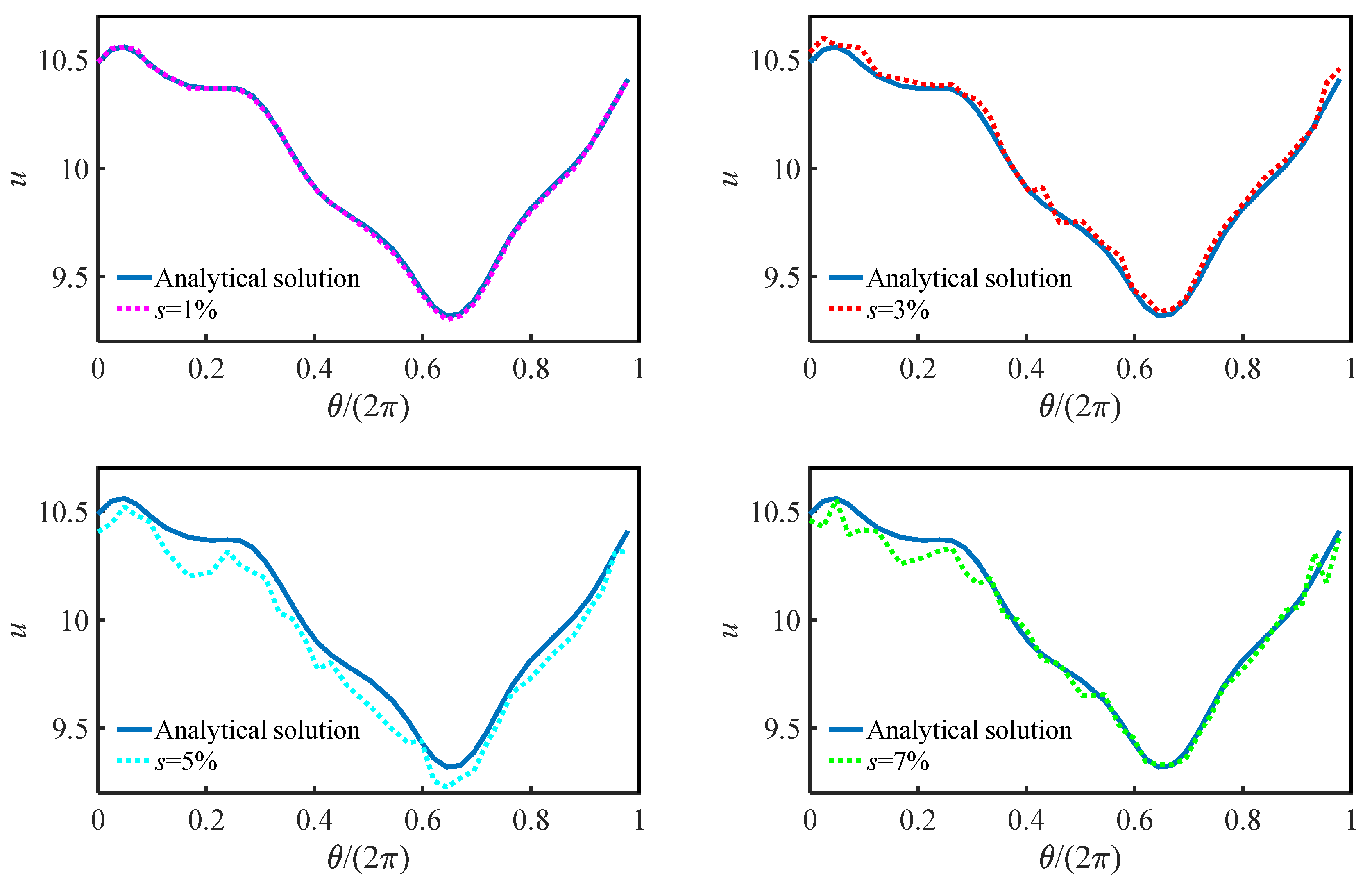
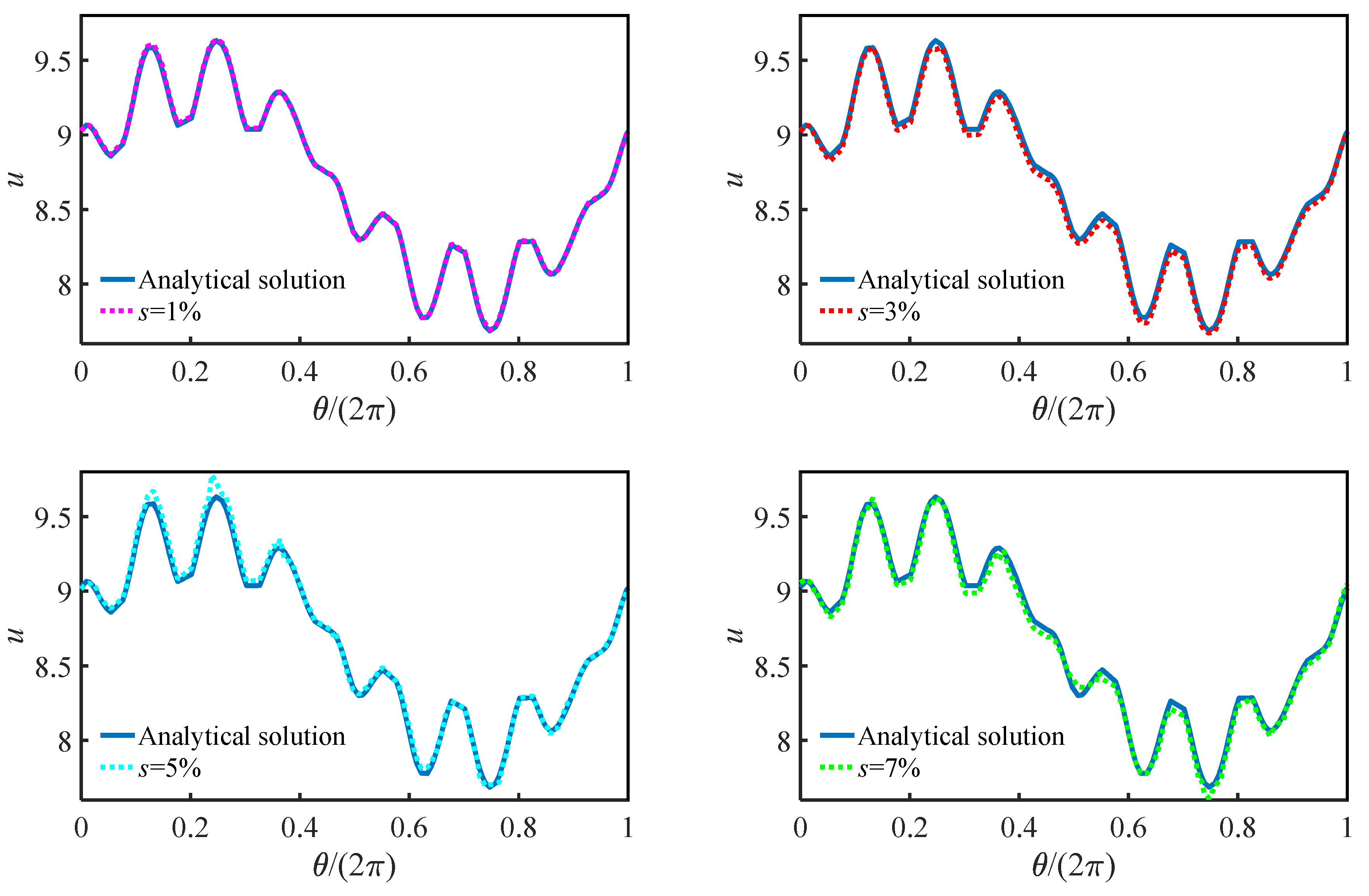
4. Numerical Examples
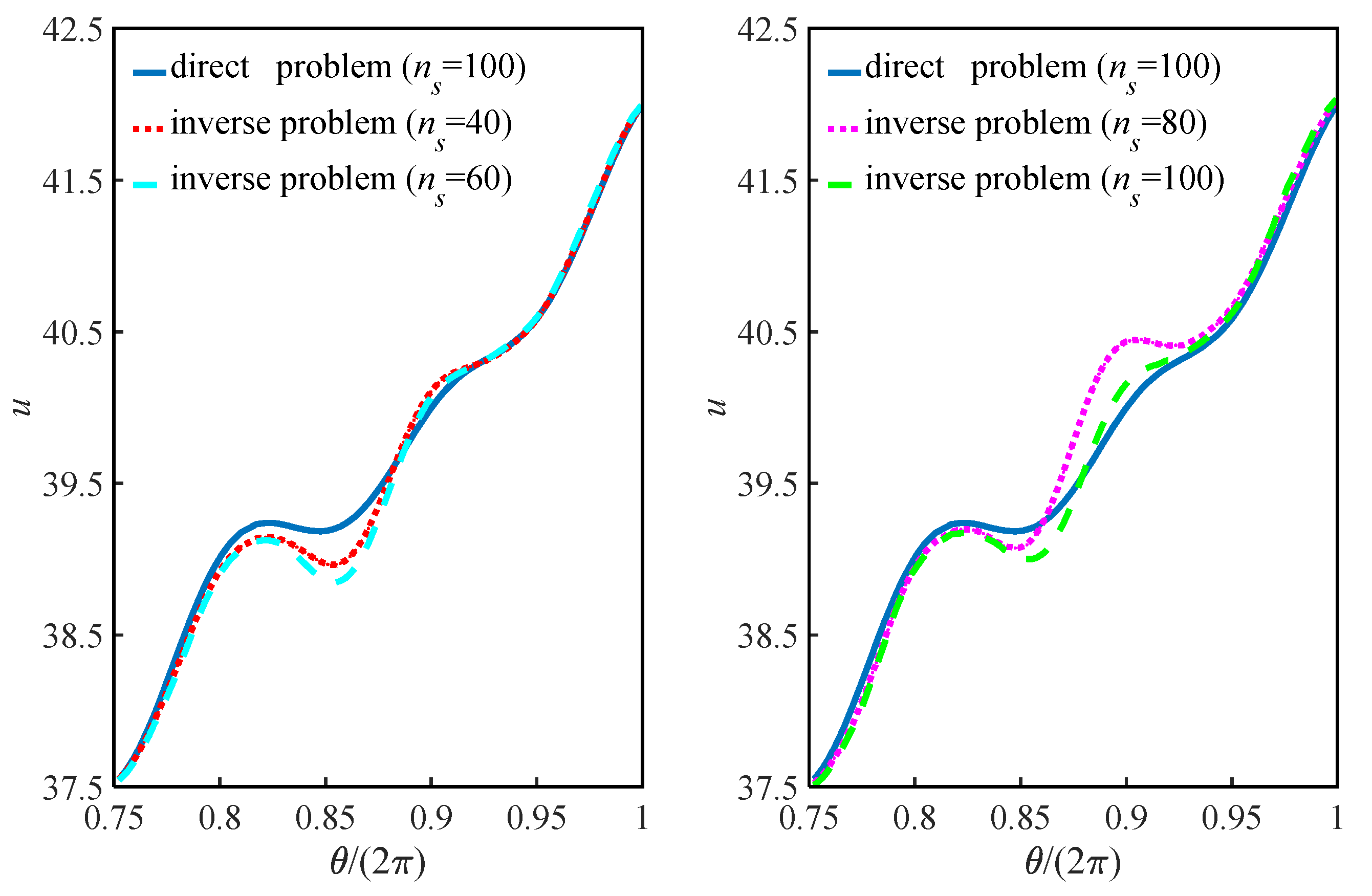
4.1. Example 1
4.2. Example 2
4.3. Example 3
4.4. Example 4
- Step 1. Direct problem:
- Step 2. Inverse problem:
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Chakib, A.; Nachaoui, A. Convergence analysis for finite element approximation to an inverse Cauchy problem. Inverse Probl. 2006, 22, 1191–1206. [Google Scholar] [CrossRef]
- Vanrumste, B.; Van Hoey, G.; Van de Walle, R.; Michel, R.D.; Lemahieu, I.A.; Boon, P.A. The validation of the finite difference method and reciprocity for solving the inverse problem in EEG dipole source analysis. Brain Topogr. 2001, 14, 83–92. [Google Scholar] [CrossRef] [PubMed]
- Lesnic, D.; Elliott, L.; Ingham, D.B. An alternating boundary element method for solving Cauchy problems for the biharmonic equation. Inverse Probl. Eng. 1997, 5, 145–168. [Google Scholar] [CrossRef]
- Marin, L.; Elliott, L.; Ingham, D.B.; Lesnic, D. Boundary element method for the Cauchy problem in linear elasticity. Eng. Anal. Bound. Elem. 2001, 25, 783–793. [Google Scholar] [CrossRef]
- Marin, L.; Hao, D.N.; Lesnic, D. Conjugate gradient-boundary element method for a Cauchy problem in the Lame system. In BETECH XIV; Brebbia, C.A., Kassab, A.J., Eds.; WTT Press: Southampton, UK, 2001; pp. 229–238. [Google Scholar]
- Liu, C.S. A modified collocation Trefftz method for the inverse Cauchy problem of Laplace equation. Eng. Anal. Bound. Elem. 2008, 32, 778–785. [Google Scholar] [CrossRef]
- Liu, C.S.; Atluri, S.N. Numerical solution of the Laplacian Cauchy problem by using a better postconditioning collocation Trefftz method. Eng. Anal. Bound. Elem. 2013, 37, 74–83. [Google Scholar] [CrossRef]
- Yang, J.P.; Hsin, W.C. Weighted reproducing kernel collocation method based on error analysis for solving inverse elasticity problems. Acta Mech. 2019, 230, 3477–3497. [Google Scholar] [CrossRef]
- Zhang, T.; Dong, L.T.; Alotaibi, A.; Atluri, S.N. Application of the MLPG mixed collocation method for solving inverse problems of linear isotropic/anisotropic elasticity with simply/multiply-connected domains. CMES Comput. Model. Eng. Sci. 2013, 94, 1–28. [Google Scholar]
- Zheng, H.; Zhang, C.; Wang, Y.S.; Chen, W.; Sladek, J.; Sladek, V. A local RBF collocation method for band structure computations of 2D solid/fluid and fluid/solid phononic crystals. Int. J. Numer. Methods Eng. 2017, 110, 467–500. [Google Scholar] [CrossRef]
- Zheng, H.; Zhang, C.; Wang, Y.S.; Sladek, J.; Sladek, V. A meshfree local RBF collocation method for anti-plane transverse elastic wave propagation analysis in 2D phononic crystals. J. Comput. Phys. 2016, 305, 997–1014. [Google Scholar] [CrossRef]
- Cheng, H.D.; Hong, Y.X. An overview of the method of fundamental solutions—Solvability, uniqueness, convergence, and stability. Eng. Anal. Bound. Elem. 2020, 120, 118–152. [Google Scholar] [CrossRef]
- Jin, B.T.; Zheng, Y. Boundary knot method for the Cauchy problem associated with the inhomogeneous Helmholtz equation. Eng. Anal. Bound. Elem. 2005, 29, 925–935. [Google Scholar] [CrossRef]
- Gu, Y.; Chen, W.; Fu, Z.J. Singular boundary method for inverse heat conduction problems in general anisotropic media. Inverse Probl. Sci. Eng. 2014, 129, 124–136. [Google Scholar] [CrossRef]
- Chen, W.; Fu, Z.J. Boundary particle method for inverse Cauchy problems of inhomogeneous Helmholtz equations. J. Mar. Sci. Technol. 2009, 17, 157–163. [Google Scholar] [CrossRef]
- Karageorghis, A.; Fairweather, G. The method of fundamental solutions for the numerical solution of the biharmonic equation. J. Comput. Phys. 1987, 69, 433–459. [Google Scholar] [CrossRef]
- Karageorghis, A.; Lesnic, D.; Marin, L. A survey of applications of the MFS to inverse problems. Inverse Probl. Sci. Eng. 2011, 19, 309–336. [Google Scholar] [CrossRef]
- Rek, Z.; Sarler, B. The method of fundamental solutions for the Stokes flow with the subdomain technique. Eng. Anal. Bound. Elem. 2021, 128, 80–89. [Google Scholar] [CrossRef]
- Flyer, N.; Fornberg, B.; Bayona, V.; Barnett, G.A. On the role of polynomials in RBF-FD approximations: I. Interpolation and accuracy. J. Comput. Phys. 2016, 321, 21–38. [Google Scholar] [CrossRef] [Green Version]
- Bayona, V.; Flyer, N.; Fornberg, B.; Barnett, G.A. On the role of polynomials in RBF-FD approximations: II. Numerical solution of elliptic PDEs. J. Comput. Phys. 2017, 332, 257–273. [Google Scholar] [CrossRef] [Green Version]
- Fan, C.M.; Huang, Y.K.; Chen, C.S.; Kuo, S.R. Localized method of fundamental solutions for solving two-dimensional Laplace and biharmonic equations. Eng. Anal. Bound. Elem. 2019, 101, 188–197. [Google Scholar] [CrossRef]
- Qu, W.Z.; Fan, C.M.; Gu, Y.; Wang, F.J. Analysis of three-dimensional interior acoustic fields by using the localized method of fundamental solutions. Appl. Math. 2019, 76, 122–132. [Google Scholar] [CrossRef]
- Gu, Y.; Fan, C.M.; Qu, W.Z.; Wang, F.J. Localized method of fundamental solutions for large-scale modelling of three-dimensional anisotropic heat conduction problems. Comput. Struct. 2019, 220, 144–155. [Google Scholar] [CrossRef]
- Nowak, A.J.; Partridge, P.W. Comparison of the dual reciprocity and the multiple reciprocity methods. Eng. Anal. Bound. Elem. 1992, 10, 155–160. [Google Scholar] [CrossRef]
- Patridge, P.W.; Brebbia, C.A.; Wrobel, L.W. The Dual Reciprocity Boundary Element Method; Computational Mechanics Publication: Southampton, UK, 1992. [Google Scholar]
- Nowak, A.J.; Neves, A.C. (Eds.) The Multiple Reciprocity Boundary Element Method; Computational Mechanics Publication: Southampton, UK, 1994; pp. 1–41. [Google Scholar]
- Wei, X.; Huang, A.; Sun, L.L.; Chen, B. Multiple reciprocity singular boundary method for 3D inhomogeneous problems. Eng. Anal. Bound. Elem. 2020, 117, 212–220. [Google Scholar] [CrossRef]
- Chen, W.; Fu, Z.J.; Jin, B.T. A truly boundary-only meshfree method for inhomogeneous problems based on recursive composite multiple reciprocity technique. Eng. Anal. Bound. Elem. 2010, 34, 196–205. [Google Scholar] [CrossRef]
- Fu, Z.J.; Chen, W. A truly boundary-only meshfree method applied to Kirchhoff plate bending problems. Adv. Appl. Math. Mech. 2009, 1, 341–352. [Google Scholar]
- Fu, Z.; Chen, W.; Yang, W. Winkler plate bending problems by a truly boundary-only boundary particle method. Comput. Mech. 2009, 44, 757–763. [Google Scholar] [CrossRef]
- Cheng, A.H.-D.; Antes, H.; Ortner, N. Fundamental solutions of products of Helmholtz and polyharmonic operators. Eng. Anal. Bound. Elem. 1994, 14, 187–191. [Google Scholar] [CrossRef]
- Wang, F.J.; Fan, C.M.; Hua, Q.S.; Gu, Y. Localized MFS for the inverse Cauchy problems of two-dimensional Laplace and biharmonic equations. Appl. Math. Comput. 2020, 364, 124658. [Google Scholar] [CrossRef]
- Fan, C.M.; Li, P.W.; Yeih, W.C. Generalized finite difference method for solving two-dimensional inverse Cauchy problem. Inverse Probl. Sci. Eng. 2014, 23, 737–759. [Google Scholar] [CrossRef]


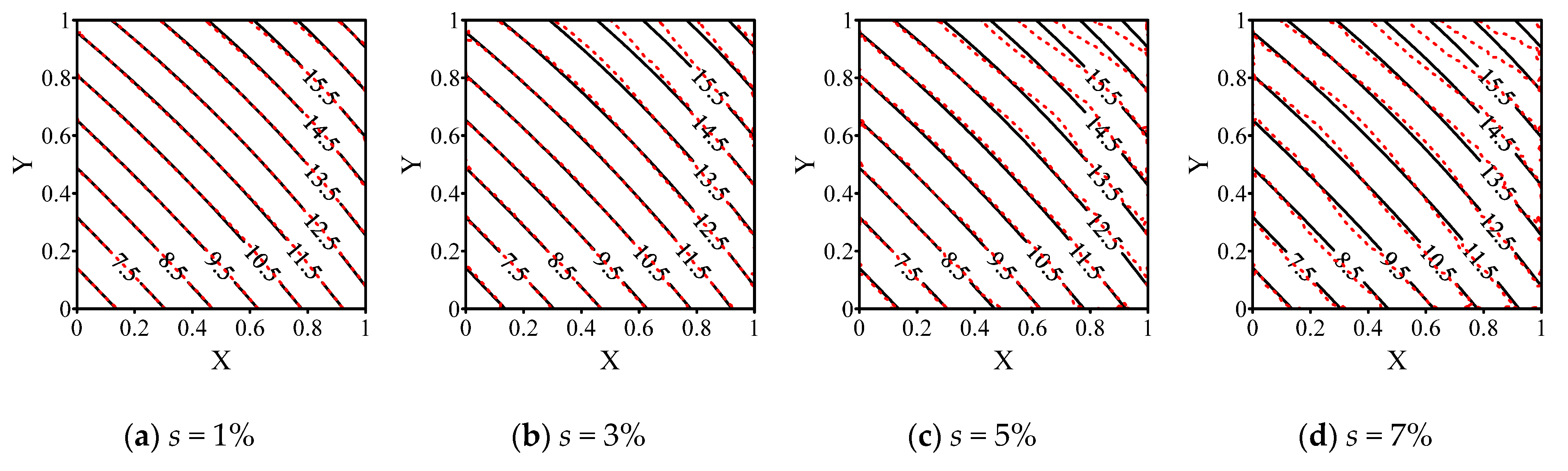
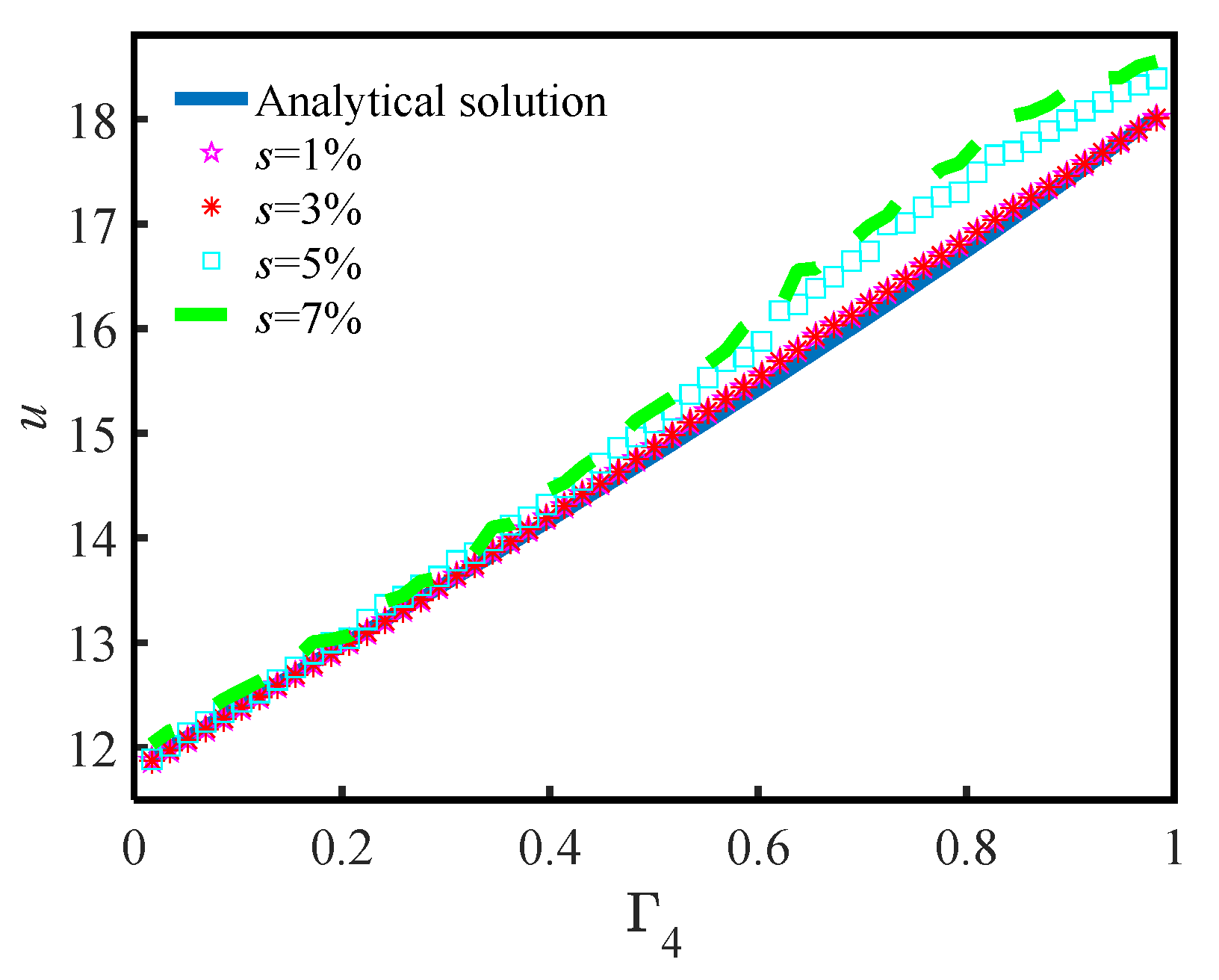


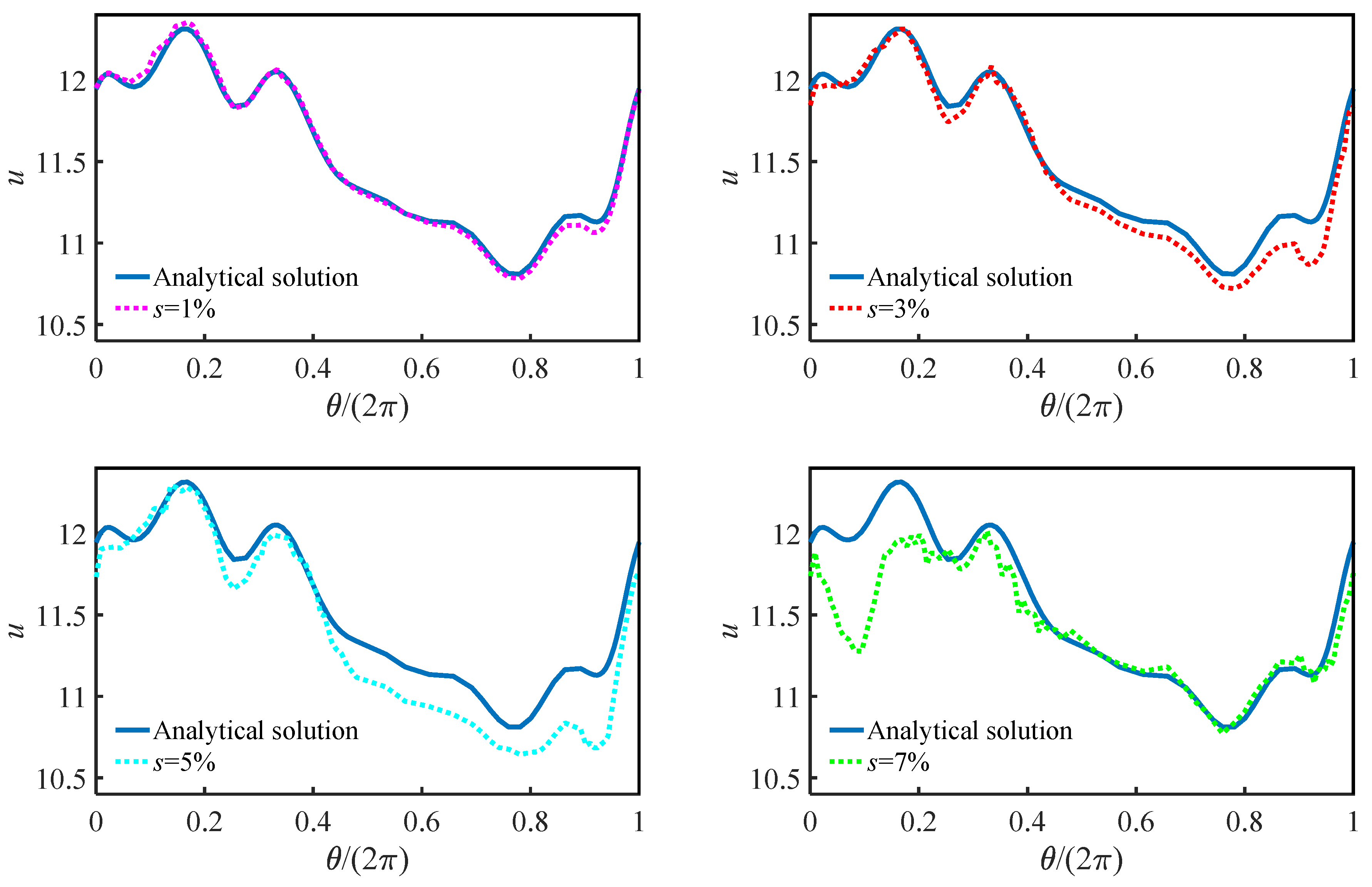

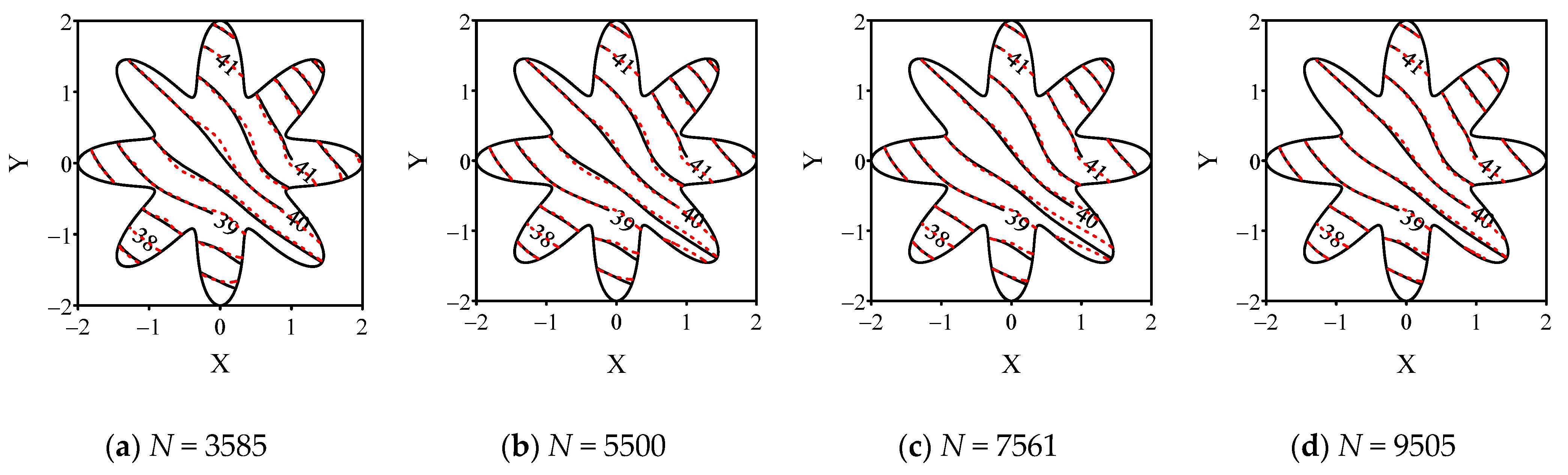
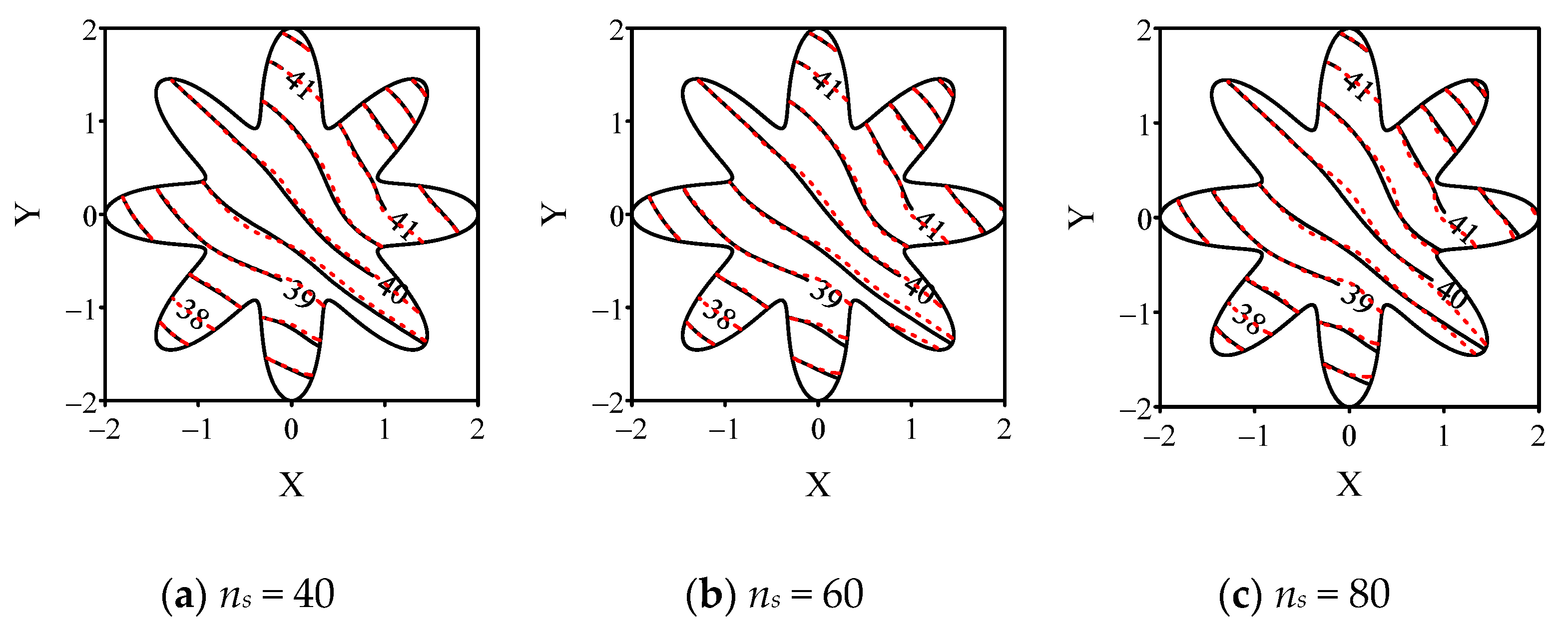
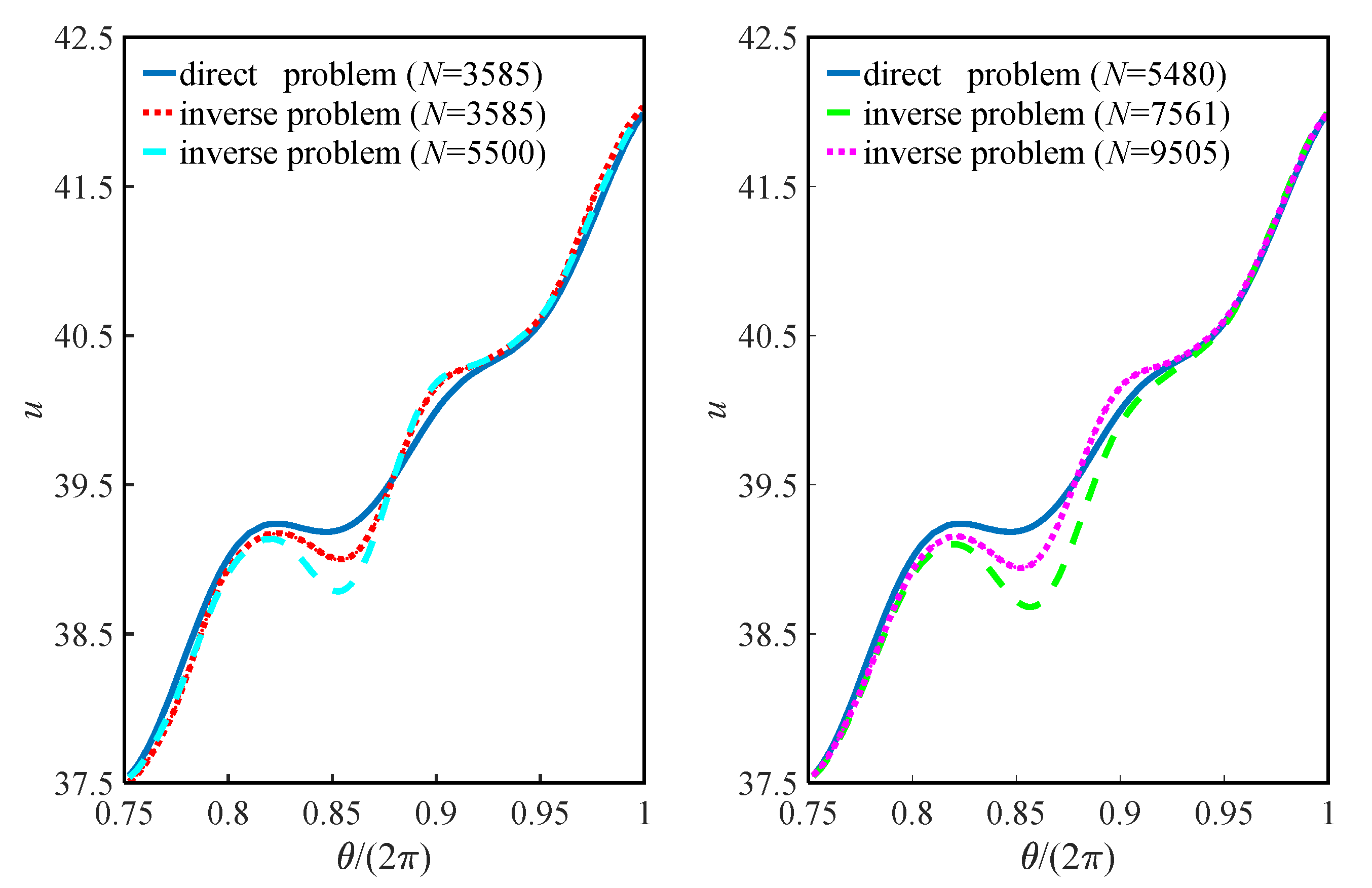
| ns | 200 | 240 | 280 | 320 | 360 | |
|---|---|---|---|---|---|---|
| s = 1% | Emaxr | 1.02 × 10−2 | 8.71 × 10−3 | 8.46 × 10−3 | 9.47 × 10−3 | 9.16 × 10−3 |
| Eglobal | 3.38 × 10−3 | 3.62 × 10−3 | 3.39 × 10−3 | 3.21 × 10−3 | 1.69 × 10−3 | |
| s = 3% | Emaxr | 2.67 × 10−2 | 2.64 × 10−2 | 2.90 × 10−2 | 2.92 × 10−2 | 2.84 × 10−2 |
| Eglobal | 5.81 × 10−3 | 7.64 × 10−3 | 9.94 × 10−3 | 5.80 × 10−3 | 6.28 × 10−3 | |
| s = 5% | Emaxr | 4.95 × 10−2 | 4.70 × 10−2 | 4.40 × 10−2 | 4.77 × 10−2 | 4.18 × 10−2 |
| Eglobal | 2.18 × 10−2 | 1.41 × 10−2 | 1.76 × 10−2 | 8.59 × 10−3 | 9.31 × 10−3 | |
| s = 7% | Emaxr | 6.97 × 10−2 | 6.81 × 10−2 | 6.45 × 10−2 | 6.17 × 10−2 | 6.20 × 10−2 |
| Eglobal | 3.22 × 10−2 | 1.71 × 10−2 | 1.94 × 10−2 | 1.90 × 10−2 | 1.08 × 10−2 | |
| N | 3591 | 4615 | 5616 | 6552 | 7560 | |
|---|---|---|---|---|---|---|
| s = 1% | Emaxr | 9.40 × 10−3 | 9.64 × 10−3 | 9.49 × 10−3 | 1.16 × 10−2 | 1.47 × 10−2 |
| Eglobal | 2.29 × 10−3 | 2.35 × 10−3 | 2.43 × 10−3 | 3.63 × 10−3 | 5.19 × 10−3 | |
| s = 3% | Emaxr | 2.64 × 10−2 | 2.92 × 10−2 | 2.67 × 10−2 | 2.83 × 10−2 | 3.32 × 10−2 |
| Eglobal | 6.96 × 10−3 | 8.47 × 10−3 | 4.35 × 10−3 | 1.00 × 10−2 | 1.16 × 10−2 | |
| s = 5% | Emaxr | 4.71 × 10−2 | 4.57 × 10−2 | 4.64 × 10−2 | 4.61 × 10−2 | 4.90 × 10−2 |
| Eglobal | 1.18 × 10−2 | 1.78 × 10−2 | 1.33 × 10−2 | 1.18 × 10−2 | 1.17 × 10−2 | |
| s = 7% | Emaxr | 5.83 × 10−2 | 6.03 × 10−2 | 6.66 × 10−2 | 6.96 × 10−2 | 6.77 × 10−2 |
| Eglobal | 1.70 × 10−2 | 1.49 × 10−2 | 2.28 × 10−2 | 2.34 × 10−2 | 3.10 × 10−2 | |
| ns | 80 | 100 | 120 | 140 | 160 | |
|---|---|---|---|---|---|---|
| s = 1% | Emaxr | 1.32 × 10−2 | 1.18 × 10−2 | 1.05 × 10−2 | 9.84 × 10−3 | 8.30 × 10−3 |
| Eglobal | 3.66 × 10−3 | 3.02 × 10−3 | 3.51 × 10−3 | 2.56 × 10−3 | 2.27 × 10−3 | |
| s = 3% | Emaxr | 3.02 × 10−2 | 3.15 × 10−2 | 3.07 × 10−2 | 3.00 × 10−2 | 2.84 × 10−2 |
| Eglobal | 9.41 × 10−3 | 7.09 × 10−3 | 6.50 × 10−3 | 6.66 × 10−3 | 7.44 × 10−3 | |
| s = 5% | Emaxr | 5.21 × 10−2 | 5.13 × 10−2 | 5.20 × 10−2 | 4.98 × 10−2 | 4.66 × 10−2 |
| Eglobal | 1.73 × 10−2 | 1.51 × 10−2 | 1.54 × 10−2 | 1.81 × 10−2 | 1.38 × 10−2 | |
| s = 7% | Emaxr | 7.84 × 10−2 | 7.25 × 10−2 | 7.19 × 10−2 | 6.78 × 10−2 | 6.90 × 10−2 |
| Eglobal | 2.26 × 10−2 | 1.77 × 10−2 | 1.87 × 10−2 | 2.12 × 10−2 | 1.69 × 10−2 | |
| N | 3259 | 4661 | 6052 | 7405 | 8834 | |
|---|---|---|---|---|---|---|
| s = 1% | Emaxr | 1.05 × 10−2 | 1.10 × 10−2 | 1.10 × 10−2 | 1.19 × 10−2 | 1.41 × 10−2 |
| Eglobal | 3.15 × 10−3 | 3.01 × 10−3 | 3.00 × 10−3 | 3.67 × 10−3 | 3.54 × 10−3 | |
| s = 3% | Emaxr | 3.07 × 10−2 | 2.99 × 10−2 | 3.38 × 10−2 | 3.47 × 10−2 | 3.78 × 10−2 |
| Eglobal | 6.50 × 10−3 | 9.48 × 10−3 | 1.01 × 10−2 | 1.01 × 10−2 | 1.28 × 10−2 | |
| s = 5% | Emaxr | 5.20 × 10−2 | 5.22 × 10−2 | 5.26 × 10−2 | 5.49 × 10−2 | 5.57 × 10−2 |
| Eglobal | 1.54 × 10−2 | 1.50 × 10−2 | 1.44 × 10−2 | 1.69 × 10−2 | 1.72 × 10−2 | |
| s = 7% | Emaxr | 7.19 × 10−2 | 7.00 × 10−2 | 7.19 × 10−2 | 8.35 × 10−2 | 8.38 × 10−2 |
| Eglobal | 1.87 × 10−2 | 2.17 × 10−2 | 1.64 × 10−2 | 2.25 × 10−2 | 2.88 × 10−2 | |
| ns | 100 | 120 | 160 | 180 | 200 | |
|---|---|---|---|---|---|---|
| s = 1% | Emaxr | 9.62 × 10−3 | 8.05 × 10−3 | 9.04 × 10−3 | 9.95 × 10−3 | 9.70 × 10−3 |
| Eglobal | 2.58 × 10−3 | 2.16 × 10−3 | 2.02 × 10−3 | 2.22 × 10−3 | 1.99 × 10−3 | |
| s = 3% | Emaxr | 2.82 × 10−2 | 2.37 × 10−2 | 2.90 × 10−2 | 2.82 × 10−2 | 2.83 × 10−2 |
| Eglobal | 7.49 × 10−3 | 6.16 × 10−3 | 5.56 × 10−3 | 6.32 × 10−3 | 5.89 × 10−3 | |
| s = 5% | Emaxr | 4.38 × 10−2 | 4.08 × 10−2 | 4.59 × 10−2 | 4.73 × 10−2 | 4.88 × 10−2 |
| Eglobal | 1.35 × 10−2 | 1.09 × 10−2 | 1.08 × 10−2 | 1.00 × 10−2 | 1.12 × 10−2 | |
| s = 7% | Emaxr | 6.98 × 10−2 | 6.11 × 10−2 | 6.17 × 10−2 | 6.32 × 10−2 | 5.85 × 10−2 |
| Eglobal | 1.74 × 10−2 | 1.59 × 10−2 | 1.43 × 10−2 | 1.24 × 10−2 | 1.28 × 10−2 | |
| N | 1629 | 3472 | 4562 | 6047 | 7680 | |
|---|---|---|---|---|---|---|
| s = 1% | Emaxr | 9.95 × 10−3 | 9.75 × 10−3 | 8.09 × 10−3 | 9.27 × 10−3 | 1.16 × 10−2 |
| Eglobal | 1.96 × 10−3 | 2.40 × 10−3 | 1.59 × 10−3 | 2.08 × 10−3 | 2.14 × 10−3 | |
| s = 3% | Emaxr | 2.60 × 10−2 | 2.80 × 10−2 | 2.67 × 10−2 | 2.67 × 10−2 | 2.88 × 10−2 |
| Eglobal | 5.40 × 10−3 | 6.71 × 10−3 | 5.66 × 10−3 | 5.95 × 10−3 | 6.99 × 10−3 | |
| s = 5% | Emaxr | 4.92 × 10−2 | 4.99 × 10−2 | 4.26 × 10−2 | 4.00 × 10−2 | 4.71 × 10−2 |
| Eglobal | 8.94 × 10−3 | 1.04 × 10−2 | 8.82 × 10−3 | 7.97 × 10−3 | 8.75 × 10−3 | |
| s = 7% | Emaxr | 6.91 × 10−2 | 6.72 × 10−2 | 5.73 × 10−2 | 6.89 × 10−2 | 6.46 × 10−2 |
| Eglobal | 1.39 × 10−2 | 1.61 × 10−2 | 1.32 × 10−2 | 1.24 × 10−2 | 1.34 × 10−2 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, J.; Zheng, H.; Fan, C.-M.; Fu, M.-F. Localized Method of Fundamental Solutions for Two-Dimensional Inhomogeneous Inverse Cauchy Problems. Mathematics 2022, 10, 1464. https://doi.org/10.3390/math10091464
Zhang J, Zheng H, Fan C-M, Fu M-F. Localized Method of Fundamental Solutions for Two-Dimensional Inhomogeneous Inverse Cauchy Problems. Mathematics. 2022; 10(9):1464. https://doi.org/10.3390/math10091464
Chicago/Turabian StyleZhang, Junli, Hui Zheng, Chia-Ming Fan, and Ming-Fu Fu. 2022. "Localized Method of Fundamental Solutions for Two-Dimensional Inhomogeneous Inverse Cauchy Problems" Mathematics 10, no. 9: 1464. https://doi.org/10.3390/math10091464
APA StyleZhang, J., Zheng, H., Fan, C.-M., & Fu, M.-F. (2022). Localized Method of Fundamental Solutions for Two-Dimensional Inhomogeneous Inverse Cauchy Problems. Mathematics, 10(9), 1464. https://doi.org/10.3390/math10091464







