Analytical Method for Generalized Nonlinear Schrödinger Equation with Time-Varying Coefficients: Lax Representation, Riemann-Hilbert Problem Solutions
Abstract
:1. Introduction
2. Lax Presentation and RH Problem
3. Solvability of RH Problem and Time Evolution Laws for Scattering Data
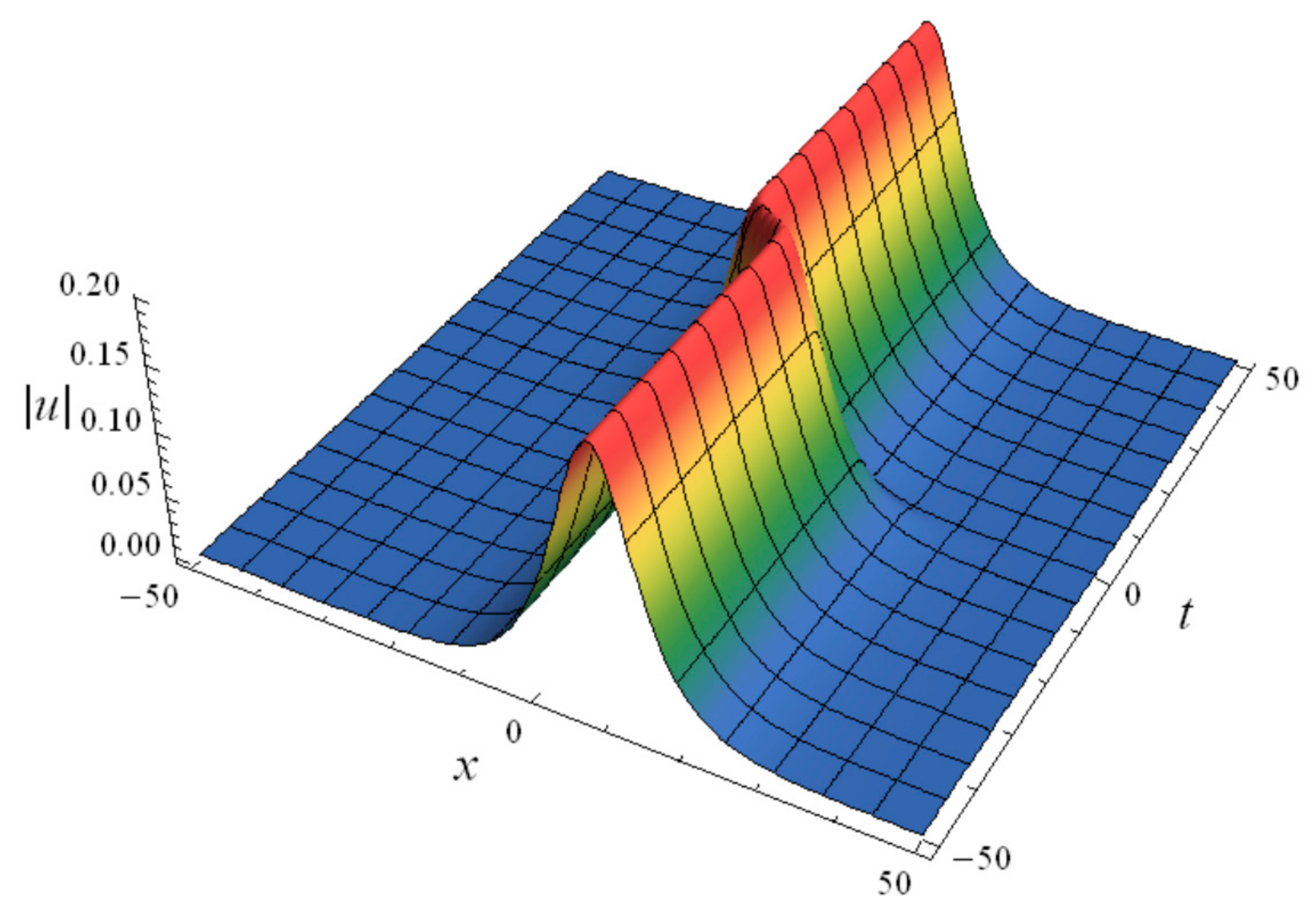
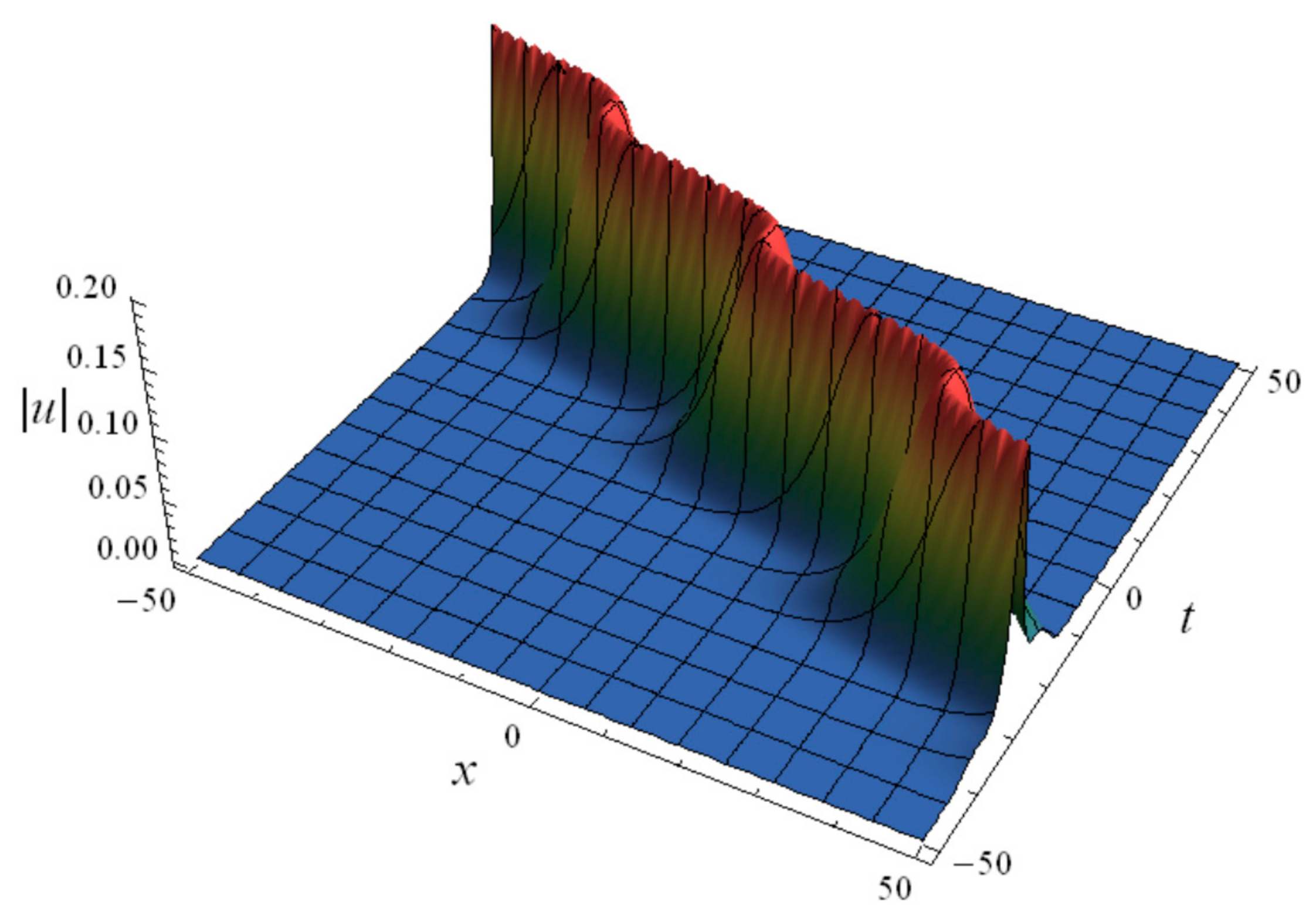
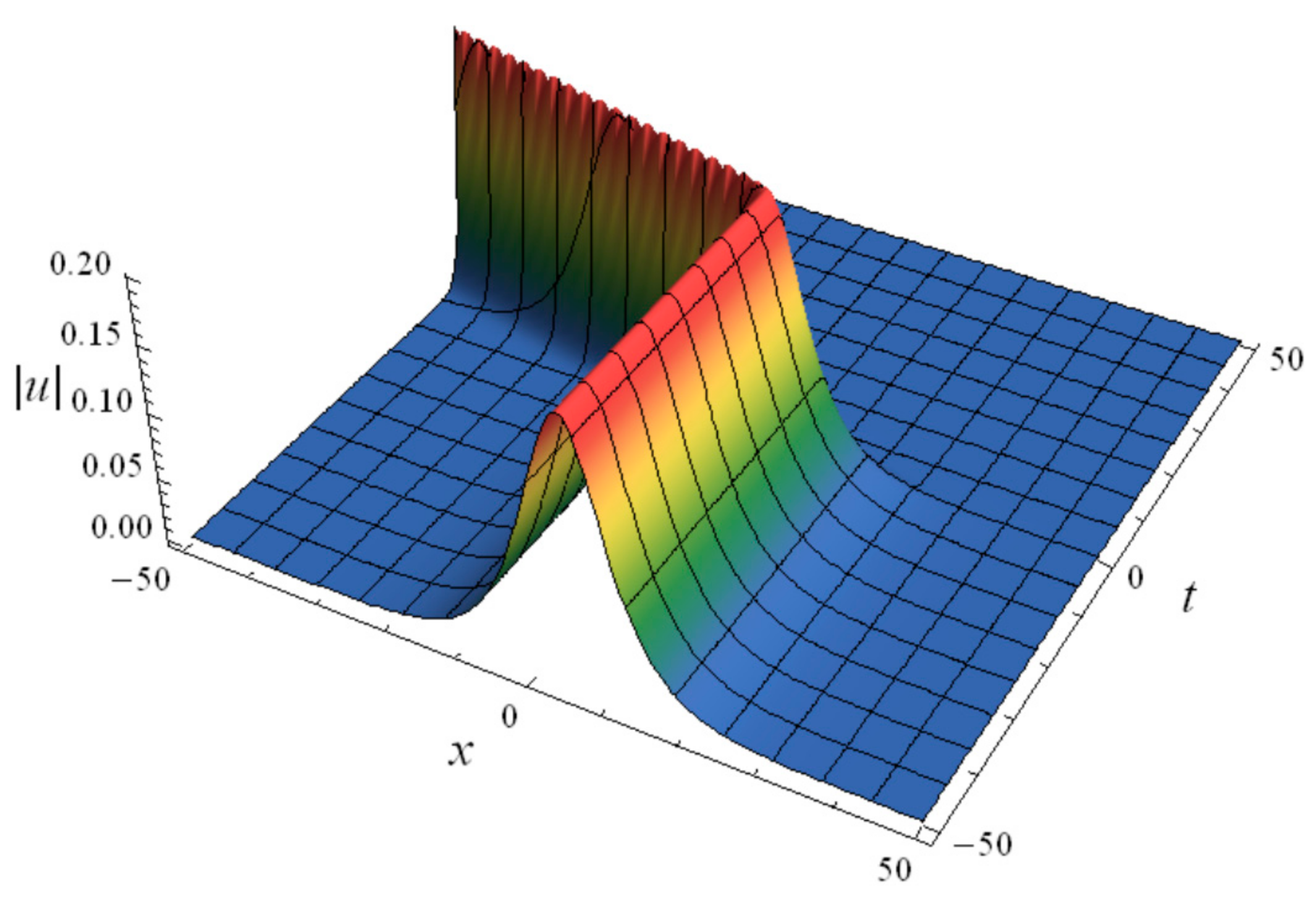
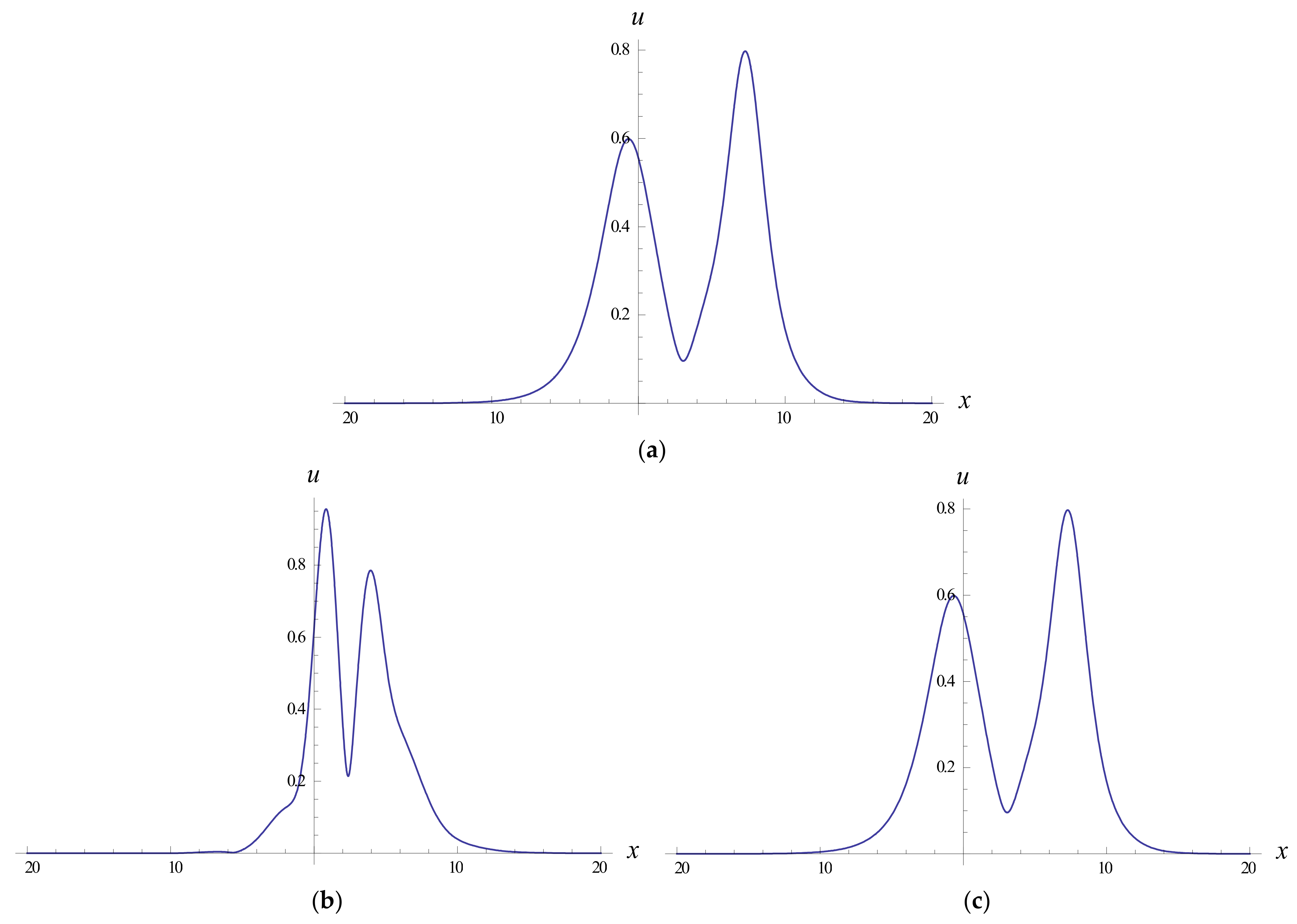
4. Long-Time Asymptotic Solution and N-Soliton Solution
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Liu, W.M.; Kengne, E. Schrödinger Equations in Nonlinear Systems; Springer Nature Singapore Pte Ltd.: Singapore, 2019. [Google Scholar]
- Gardner, C.S.; Greene, J.M.; Kruskal, M.D.; Miura, R.M. Method for solving the Korteweg-de Vries equation. Phys. Rev. Lett. 1967, 19, 1095–1197. [Google Scholar] [CrossRef]
- Matveev, V.B.; Salle, M.A. Darboux Transformation and Soliton; Springer: Berlin, Germany, 1991. [Google Scholar]
- Hirota, R. Exact envelope-soliton solutions of a nonlinear wave equation. J. Math. Phys. 1973, 14, 805–809. [Google Scholar] [CrossRef]
- Wang, M.L. Exact solutions for a compound KdV-Burgers equation. Phys. Lett. A 1996, 213, 279–287. [Google Scholar] [CrossRef]
- Fan, E.G. Travelling wave solutions in terms of special functions for nonlinear coupled evolution systems. Phys. Lett. A 2002, 300, 243–249. [Google Scholar] [CrossRef]
- He, J.H.; Wu, X.H. Exp-function method for nonlinear wave equations. Chaos Soliton. Fract. 2006, 30, 700–708. [Google Scholar] [CrossRef]
- Zhang, S.; Xia, T.C. A generalized auxiliary equation method and its application to (2 + 1)-dimensional asymmetric Nizhnik-Novikov-Vesselov equations. Phys. A Math. Theor. 2007, 40, 227–248. [Google Scholar] [CrossRef]
- Ma, W.X.; Lee, J.H. A transformed rational function method and exact solutions to 3 + 1 dimensional Jimbo-Miwa equation. Chaos Soliton. Fract. 2009, 42, 1356–1363. [Google Scholar] [CrossRef] [Green Version]
- Tian, S.F. Initial-boundary value problems for the general coupled nonlinear Schrödinger equation on the interval via the Fokas method. J. Diff. Equ. 2016, 262, 506–588. [Google Scholar] [CrossRef]
- Xu, B.; Zhang, S. A novel approach to time-dependent-coefficient WBK system: Doubly periodic waves and singular nonlinear dynamics. Complexity 2018, 2018, 3158126. [Google Scholar] [CrossRef] [Green Version]
- Xu, B.; Zhang, S. Exact solutions with arbitrary functions of the (4 + 1)-dimensional Fokas equation. Therm. Sci. 2019, 23, 2403–2411. [Google Scholar] [CrossRef]
- Xu, B.; Zhang, S. Exact solutions of nonlinear equations in mathematical physics via negative power expansion method. J. Math. Phys. Anal. Geo. 2021, 17, 369–387. [Google Scholar] [CrossRef]
- Zhang, S.; Xu, B. Painlevé test and exact solutions for (1 + 1)-dimensional generalized Broer-Kaup equations. Mathematics 2022, 10, 486. [Google Scholar] [CrossRef]
- Serkin, V.N.; Hasegawa, A.; Belyaeva, T.L. Nonautonomous solitons in external potentials. Phys. Rev. Lett. 2007, 98, 074102. [Google Scholar] [CrossRef]
- Kruglov, V.I.; Peacock, A.C.; Harvey, J.D. Exact self-similar solutions of the generalized nonlinear Schrödinger equation with distributed coefficients. Phys. Rev. Lett. 2003, 90, 113902. [Google Scholar] [CrossRef]
- Yang, J.K. Nonlinear Waves in Integrable and Nonintegrable Systems; SIAM: Philadelphia, PA, USA, 2010. [Google Scholar]
- Deift, P.; Zhou, X. A steepest descent method for oscillatory Riemann-Hilbert problems. Ann. Math. 1993, 137, 295–368. [Google Scholar] [CrossRef] [Green Version]
- Xu, J.; Fan, E.G.; Chen, Y. Long-time asymptotic for the derivative nonlinear Schrödinger equation with step-like initial value. Math. Phys. Anal. Geom. 2013, 16, 253–288. [Google Scholar] [CrossRef] [Green Version]
- Ma, W.X. Riemann-Hilbert problems and N-soliton solutions for a coupled mKdV system. J. Geom. Phys. 2018, 132, 45–54. [Google Scholar] [CrossRef]
- Hu, B.B.; Xia, T.C.; Ma, W.X. Riemann-Hilbert approach for an initial-boundary value problem of the two-component modified Korteweg-de Vries equation on the half-line. Appl. Math. Comput. 2018, 332, 148–159. [Google Scholar] [CrossRef]
- Wang, D.S.; Guo, B.; Wang, X.L. Long-time asymptotics of the focusing Kundu-Eckhaus equation with nonzero boundary conditions. J. Differ. Equ. 2019, 266, 5209–5253. [Google Scholar] [CrossRef]
- Chen, S.Y.; Yan, Z.Y.; Guo, B.L. Long-time asymptotics for the focusing Hirota equation with non-zero boundary conditions at infinity via the Deift-Zhou approach. Math. Phys. Anal. Geom. 2021, 24, 17. [Google Scholar] [CrossRef]
- Guo, H.D.; Xia, T.C. Multi-soliton solutions for a higher-order coupled nonlinear Schrödinger system in an optical fiber via Riemann-Hilbert approach. Nonlinear Dyn. 2021, 103, 1805–1816. [Google Scholar] [CrossRef]
- Li, Z.Q.; Tian, S.F.; Zhang, T.T.; Yang, J.J. Riemann-Hilbert approach and multi-soliton solutions of a variable-coefficient fifth-order nonlinear Schrödinger equation with N distinct arbitrary-order poles. Mod. Phys. Lett. B 2021, 35, 2150194. [Google Scholar] [CrossRef]
- Zhang, B.; Fan, E.G. Riemann-Hilbert approach for a Schrödinger-type equation with nonzero boundary conditions. Mod. Phys. Lett. B 2021, 35, 2150208. [Google Scholar] [CrossRef]
- Wei, H.Y.; Fan, E.G.; Guo, H.D. Riemann-Hilbert approach and nonlinear dynamics of the coupled higher-order nonlinear Schrödinger equation in the birefringent or two-mode fiber. Nonlinear Dyn. 2021, 104, 649–660. [Google Scholar] [CrossRef]
- Xu, B.; Zhang, S. Riemann-Hilbert approach for constructing analytical solutions and conservation laws of a local time-fractional nonlinear Schrödinger equation. Symmetry 2021, 13, 13091593. [Google Scholar] [CrossRef]
- Serkin, V.N.; Hasegawa, A. Novel soliton solutions of the nonlinear Schrödinger equation model. Phys. Rev. Lett. 2000, 85, 4502–4505. [Google Scholar] [CrossRef]
- Kruglov, V.I.; Harvey, J.D. Asymptotically exact parabolic solutions of the generalized nonlinear Schrödinger equation with varying parameters. J. Opt. Soc. Am. B 2006, 23, 2541–2550. [Google Scholar] [CrossRef]
- Serkin, V.N.; Belyaeva, T.L. Optimal control of dark solitons. Optik 2018, 168, 827–838. [Google Scholar] [CrossRef]
- Zhang, S.; Zhang, L.J.; Xu, B. Rational waves and complex dynamics: Analytical insights into a generalized nonlinear Schrödinger equation with distributed coefficients. Complexity 2019, 2019, 3206503. [Google Scholar] [CrossRef]
- Plemelj, J. Riemannsche Funktionenscharen mit gegebener Monodromiegruppe. Monatsch. Math. Phys. 1908, 19, 211–246. [Google Scholar] [CrossRef] [Green Version]
- Zakharov, V.E.; Shabat, A.B. Integration of nonlinear equations of mathematical physics by the method of inverse scattering. II. Funct. Anal. Appl. 1979, 13, 166–174. [Google Scholar] [CrossRef]
- Mai, X.L.; Li, W.; Dong, S.H. Exact solution to the nonlinear Schrödinger equation with time-dependent coefficients. Adv. High Energy Phys. 2021, 2021, 6694980. [Google Scholar] [CrossRef]
- Guo, Q.; Liu, J. New exact solution to the nonlinear Schrödinger equation with variable coefficients. Results Phys. 2020, 16, 102857. [Google Scholar] [CrossRef]
- Kosti, A.A.; Colreavy-Donnelly, S.; Caraffini, F.; Anastassi, Z.A. Efficient computation of the nonlinear Schrödinger equation with time-dependent coefficients. Mathematics 2020, 8, 374. [Google Scholar] [CrossRef] [Green Version]






Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, B.; Zhang, S. Analytical Method for Generalized Nonlinear Schrödinger Equation with Time-Varying Coefficients: Lax Representation, Riemann-Hilbert Problem Solutions. Mathematics 2022, 10, 1043. https://doi.org/10.3390/math10071043
Xu B, Zhang S. Analytical Method for Generalized Nonlinear Schrödinger Equation with Time-Varying Coefficients: Lax Representation, Riemann-Hilbert Problem Solutions. Mathematics. 2022; 10(7):1043. https://doi.org/10.3390/math10071043
Chicago/Turabian StyleXu, Bo, and Sheng Zhang. 2022. "Analytical Method for Generalized Nonlinear Schrödinger Equation with Time-Varying Coefficients: Lax Representation, Riemann-Hilbert Problem Solutions" Mathematics 10, no. 7: 1043. https://doi.org/10.3390/math10071043
APA StyleXu, B., & Zhang, S. (2022). Analytical Method for Generalized Nonlinear Schrödinger Equation with Time-Varying Coefficients: Lax Representation, Riemann-Hilbert Problem Solutions. Mathematics, 10(7), 1043. https://doi.org/10.3390/math10071043





