Adaptive Neural Control for an Uncertain 2-DOF Helicopter System with Unknown Control Direction and Actuator Faults
Abstract
:1. Introduction
2. Problem Formulation and Preliminaries
2.1. System Description
2.2. Preliminaries
3. Control Design and Stability Analysis
3.1. Control Design
Stability Analysis
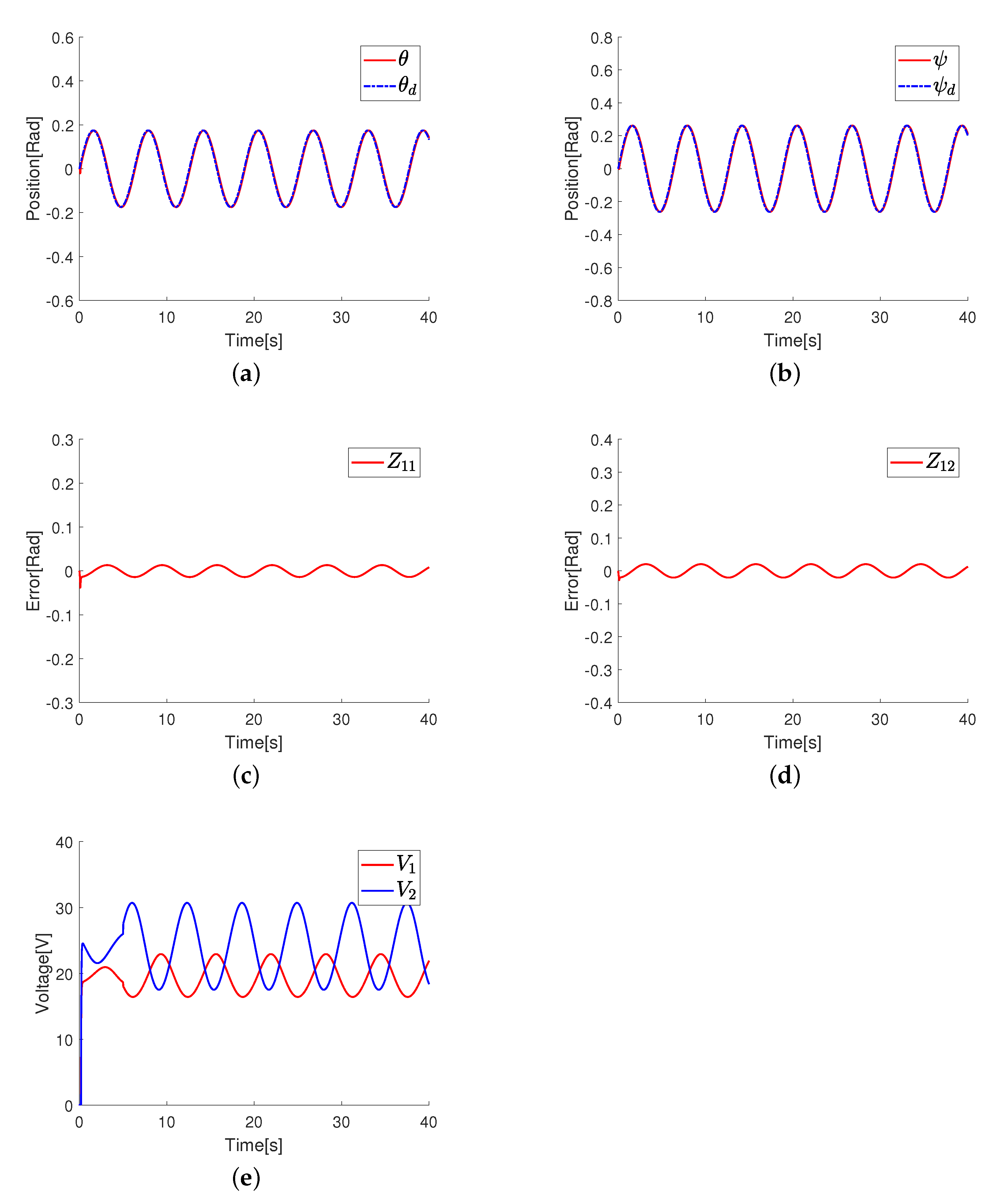
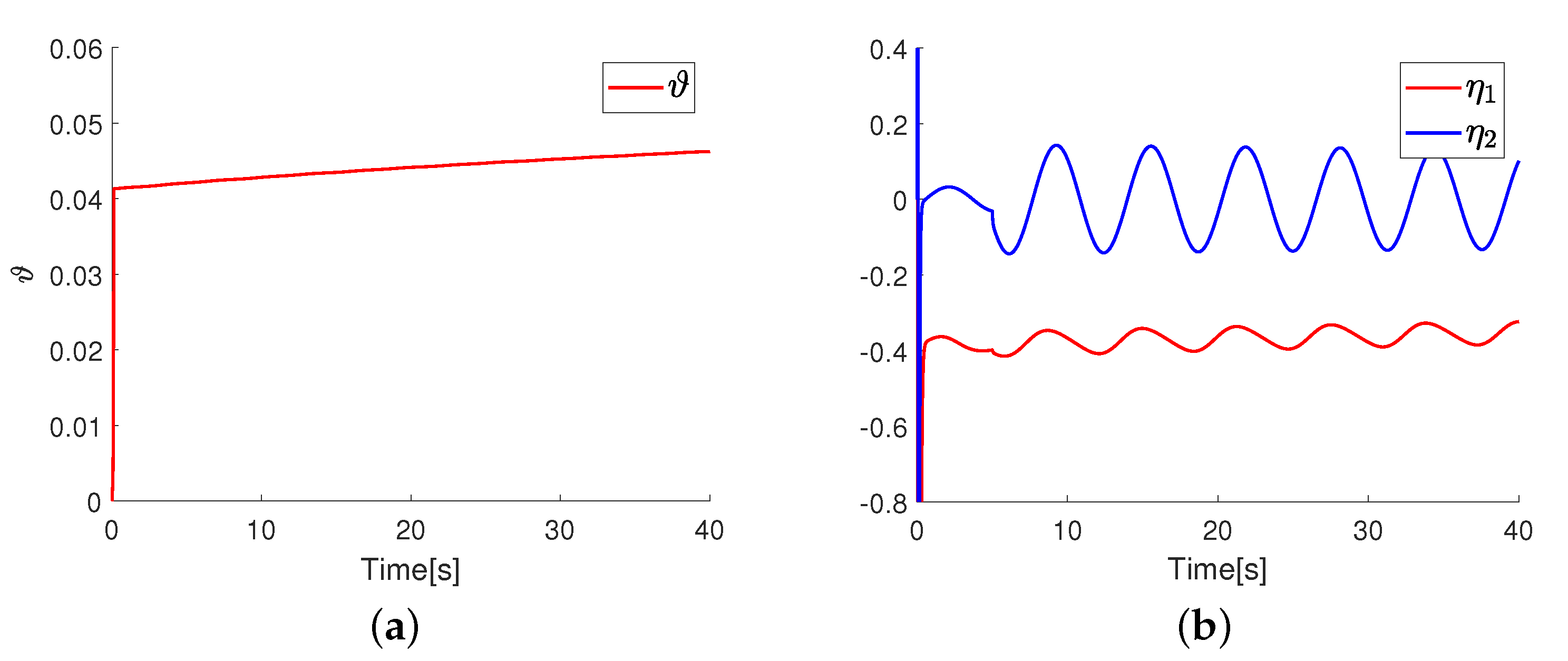
4. Simulations
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Jiang, B.; Li, B.; Zhou, W.; Lo, L.Y.; Chen, C.K.; Wen, C.Y. Neural Network Based Model Predictive Control for a Quadrotor UAV. Aerospace 2022, 9, 460. [Google Scholar] [CrossRef]
- Reyhanoglu, M.; Jafari, M.; Rehan, M. Simple Learning-Based Robust Trajectory Tracking Control of a 2-DOF Helicopter System. Electronics 2022, 11, 2075. [Google Scholar] [CrossRef]
- Mu, C.; Zhang, Y. Learning-Based Robust Tracking Control of Quadrotor with Time-Varying and Coupling Uncertainties. IEEE Trans. Neural Netw. Learn. Syst. 2020, 31, 259–273. [Google Scholar] [CrossRef] [PubMed]
- Hernandez-Gonzalez, M.; Alanis, A.Y.; Hernandez-Vargas, E.A. Decentralized discrete-time neural control for a Quanser 2-DOF helicopter. Appl. Soft Comput. 2012, 12, 2462–2469. [Google Scholar] [CrossRef]
- Kim, S.K.; Ahn, C.K. Performance-Boosting Attitude Control for 2-DOF Helicopter Applications via Surface Stabilization Approach. IEEE Trans. Ind. Electron. 2022, 69, 7234–7243. [Google Scholar] [CrossRef]
- Liu, Z.; Liang, J.; Zhao, Z.; Efe, M.O.; Hong, K.S. Adaptive Fault-Tolerant Control of a Probe-and-Drogue Refueling Hose Under Varying Length and Constrained Output. IEEE Trans. Control Syst. Technol. 2022, 30, 869–876. [Google Scholar] [CrossRef]
- Kaletka, J.; Kurscheid, H.; Butter, U. FHS, the new research helicopter: Ready for service. Aerosp. Sci. Technol. 2005, 9, 456–467. [Google Scholar] [CrossRef]
- Verginis, C.K.; Bechlioulis, C.P.; Soldatos, A.G.; Tsipianitis, D. Robust Trajectory Tracking Control for Uncertain 3-DOF Helicopters With Prescribed Performance. IEEE/ASME Trans. Mechatron. 2022, 27, 3559–3569. [Google Scholar] [CrossRef]
- Subramanian, R.G.; Elumalai, V.K. Robust MRAC augmented baseline LQR for tracking control of 2 DoF helicopter. Robot. Auton. Syst. 2016, 86, 70–77. [Google Scholar] [CrossRef]
- Nuthi, P.; Subbarao, K. Experimental Verification of Linear and Adaptive Control Techniques for a Two Degrees-of-Freedom Helicopter. J. Dyn. Syst. Meas. Control 2016, 137, 064501. [Google Scholar] [CrossRef]
- Maiti, R.; Sharma, K.D.; Sarkar, G. PSO based parameter estimation and PID controller tuning for 2-DOF nonlinear twin rotor MIMO system. Int. J. Autom. Control 2018, 12, 582. [Google Scholar] [CrossRef]
- Chun, T.Y.; Park, J.B.; Choi, Y.H. Reinforcement Q-learning based on Multirate Generalized Policy Iteration and Its Application to a 2-DOF Helicopter. Int. J. Control. Autom. Syst. 2018, 16, 377–386. [Google Scholar] [CrossRef]
- Delaram, J.; Houshamand, M.; Ashtiani, F.; Fatahi Valilai, O. A utility-based matching mechanism for stable and optimal resource allocation in cloud manufacturing platforms using deferred acceptance algorithm. J. Manuf. Syst. 2021, 60, 569–584. [Google Scholar] [CrossRef]
- Delaram, J.; Houshamand, M.; Ashtiani, F.; Valilai, O.F. Development of public cloud manufacturing markets: A mechanism design approach. Int. J. Syst. Sci. Oper. Logist. 2022, 1–27. [Google Scholar] [CrossRef]
- Zhang, F.; Wang, C. Deterministic learning from neural control for uncertain nonlinear pure-feedback systems by output feedback. Int. J. Robust Nonlinear Control 2020, 30, 2701–2718. [Google Scholar] [CrossRef]
- Mohamed, I.S.; Rovetta, S.; Do, T.D.; Dragicević, T.; Diab, A.A.Z. A Neural-Network-Based Model Predictive Control of Three-Phase Inverter With an Output LC Filter. IEEE Access 2019, 7, 124737–124749. [Google Scholar] [CrossRef]
- He, W.; Dong, Y.; Sun, C. Adaptive Neural Impedance Control of a Robotic Manipulator With Input Saturation. IEEE Trans. Syst. Man Cybern. Syst. 2016, 46, 334–344. [Google Scholar] [CrossRef]
- Liu, Y.J.; Zhao, W.; Liu, L.; Li, D.; Tong, S.; Chen, C.L.P. Adaptive Neural Network Control for a Class of Nonlinear Systems With Function Constraints on States. IEEE Trans. Neural Netw. Learn. Syst. 2021, 1–10. [Google Scholar] [CrossRef]
- Chen, L.; Cui, R.; Yang, C.; Yan, W. Adaptive Neural Network Control of Underactuated Surface Vessels With Guaranteed Transient Performance: Theory and Experimental Results. IEEE Trans. Ind. Electron. 2020, 67, 4024–4035. [Google Scholar] [CrossRef] [Green Version]
- Zhao, Z.; He, W.; Ge, S.S. Adaptive Neural Network Control of a Fully Actuated Marine Surface Vessel With Multiple Output Constraints. IEEE Trans. Control Syst. Technol. 2014, 22, 1536–1543. [Google Scholar]
- Zhou, Q.; Zhao, S.; Li, H.; Lu, R.; Wu, C. Adaptive Neural Network Tracking Control for Robotic Manipulators With Dead Zone. IEEE Trans. Neural Netw. Learn. Syst. 2019, 30, 3611–3620. [Google Scholar] [CrossRef] [PubMed]
- Chen, Z.; Huang, F.; Sun, W.; Gu, J.; Yao, B. RBF-Neural-Network-Based Adaptive Robust Control for Nonlinear Bilateral Teleoperation Manipulators With Uncertainty and Time Delay. IEEE/ASME Trans. Mechatron. 2020, 25, 906–918. [Google Scholar] [CrossRef]
- Chen, M.; Shi, P.; Lim, C.C. Adaptive Neural Fault-Tolerant Control of a 3-DOF Model Helicopter System. IEEE Trans. Syst. Man Cybern. Syst. 2016, 46, 260–270. [Google Scholar] [CrossRef] [Green Version]
- Ouyang, Y.; Dong, L.; Xue, L.; Sun, C. Adaptive control based on neural networks for an uncertain 2-DOF helicopter system with input deadzone and output constraints. IEEE/CAA J. Autom. Sin. 2019, 6, 807–815. [Google Scholar] [CrossRef]
- Zhao, Z.; He, W.; Mu, C.; Zou, T.; Hong, K.S.; Li, H.X. Reinforcement Learning Control for a 2-DOF Helicopter With State Constraints: Theory and Experiments. IEEE Trans. Autom. Sci. Eng. 2022, 1–11. [Google Scholar] [CrossRef]
- Zhao, Z.; Zhang, J.; Liu, Z.; Mu, C.; Hong, K.S. Adaptive Neural Network Control of an Uncertain 2-DOF Helicopter With Unknown Backlash-Like Hysteresis and Output Constraints. IEEE Trans. Neural Netw. Learn. Syst. 2022, 1–10. https://ieeexplore.ieee.org/abstract/document/9760116. [CrossRef]
- Zhao, Z.; He, W.; Zhang, F.; Wang, C.; Hong, K.S. Deterministic Learning from Adaptive Neural Network Control for a 2-DOF Helicopter System With Unknown Backlash and Model Uncertainty. IEEE Trans. Ind. Electron. 2022, 1–10. [Google Scholar] [CrossRef]
- Oliveira, T.R.; Peixoto, A.J.; Hsu, L. Sliding Mode Control of Uncertain Multivariable Nonlinear Systems With Unknown Control Direction via Switching and Monitoring Function. IEEE Trans. Autom. Control 2010, 55, 1028–1034. [Google Scholar] [CrossRef]
- Wang, C.; Wen, C.; Lin, Y. Adaptive Actuator Failure Compensation for a Class of Nonlinear Systems With Unknown Control Direction. IEEE Trans. Autom. Control 2017, 62, 385–392. [Google Scholar] [CrossRef]
- Choi, Y.H.; Yoo, S.J. Tracking Control Strategy Using Filter-Based Approximation for the Unknown Control Direction Problem of Uncertain Pure-Feedback Nonlinear Systems. Mathematics 2020, 8, 1341. [Google Scholar] [CrossRef]
- Chen, Z. Nussbaum functions in adaptive control with time-varying unknown control coefficients. Automatica 2019, 102, 72–79. [Google Scholar] [CrossRef]
- Chen, W.; Li, X.; Ren, W.; Wen, C. Adaptive Consensus of Multi-Agent Systems with Unknown Identical Control Directions Based on a Novel Nussbaum-Type Function. IEEE Trans. Autom. Control 2014, 59, 1887–1892. [Google Scholar] [CrossRef]
- Zhang, C.L.; Li, J.M. Adaptive iterative learning control of non-uniform trajectory tracking for strict feedback nonlinear time-varying systems with unknown control direction. Appl. Math. Model. 2015, 39, 2942–2950. [Google Scholar] [CrossRef]
- Liu, Y.J.; Tong, S. Barrier Lyapunov functions for Nussbaum gain adaptive control of full state constrained nonlinear systems. Automatica 2017, 76, 143–152. [Google Scholar] [CrossRef]
- Liang, B.; Zheng, S.; Ahn, C.K.; Liu, F. Adaptive Fuzzy Control for Fractional-Order Interconnected Systems With Unknown Control Directions. IEEE Trans. Fuzzy Syst. 2022, 30, 75–87. [Google Scholar] [CrossRef]
- Lv, M.; Yu, W.; Cao, J.; Baldi, S. Consensus in High-Power Multiagent Systems With Mixed Unknown Control Directions via Hybrid Nussbaum-Based Control. IEEE Trans. Cybern. 2022, 52, 5184–5196. [Google Scholar] [CrossRef] [PubMed]
- Xu, Y.; Wang, C.; Wang, G.; Cai, X.; Xu, L.; Jing, C. Connectivity-preserving-based Distributed Synchronized Tracking of Networked Uncertain Underactuated Surface Vessels with Actuator Failures and Unknown Control Directions. Int. J. Control. Autom. Syst. 2021, 19, 3996–4009. [Google Scholar] [CrossRef]
- Shen, Q.; Jiang, B.; Cocquempot, V. Fault-Tolerant Control for T–S Fuzzy Systems With Application to Near-Space Hypersonic Vehicle with Actuator Faults. IEEE Trans. Fuzzy Syst. 2012, 20, 652–665. [Google Scholar] [CrossRef]
- Jin, X.; Lü, S.; Qin, J.; Zheng, W.X. Auxiliary Constrained Control of a Class of Fault-Tolerant Systems. IEEE Trans. Syst. Man Cybern. Syst. 2021, 51, 2272–2283. [Google Scholar] [CrossRef]
- Zhao, Z.; Liu, Z.; He, W.; Hong, K.S.; Li, H.X. Boundary adaptive fault-tolerant control for a flexible Timoshenko arm with backlash-like hysteresis. Automatica 2021, 130, 109690. [Google Scholar] [CrossRef]
- Guan, X.; Fan, F.; Zhu, Y.; Song, W.; Guirao, J.; Gao, W. Application of RBF neural network optimized globally by genetic algorithm in intelligent color matching of wood dyeing. J. Intell. Fuzzy Syst. 2017, 33, 2895–2901. [Google Scholar] [CrossRef]
- Ma, L.; Xu, N.; Zhao, X.; Zong, G.; Huo, X. Small-Gain Technique-Based Adaptive Neural Output-Feedback Fault-Tolerant Control of Switched Nonlinear Systems With Unmodeled Dynamics. IEEE Trans. Syst. Man Cybern. Syst. 2021, 51, 7051–7062. [Google Scholar] [CrossRef]
- Zhao, Z.; Liu, Y.; Zou, T.; Hong, K.S.; Li, H.X. Robust Adaptive Fault-Tolerant Control for a Riser-Vessel System With Input Hysteresis and Time-Varying Output Constraints. IEEE Trans. Cybern. 2022, 1–12. [Google Scholar] [CrossRef] [PubMed]
- Yin, S.; Wang, G.; Gao, H. Data-Driven Process Monitoring Based on Modified Orthogonal Projections to Latent Structures. IEEE Trans. Control Syst. Technol. 2016, 24, 1480–1487. [Google Scholar] [CrossRef]
- Liu, Z.; Shi, J.; Zhao, X.; Zhao, Z.; Li, H.X. Adaptive Fuzzy Event-Triggered Control of Aerial Refueling Hose System with Actuator Failures. IEEE Trans. Fuzzy Syst. 2022, 30, 2981–2992. [Google Scholar] [CrossRef]
- Mu, C.; Zhang, Y.; Sun, C. Data-Based Feedback Relearning Control for Uncertain Nonlinear Systems with Actuator Faults. IEEE Trans. Cybern. 2022, 1–14. [Google Scholar] [CrossRef]
- Wang, T.; Lu, M.; Zhu, X.; Patton, R.J. Aggressive Maneuver Oriented Robust Actuator Fault Estimation of a 3-DOF Helicopter Prototype Considering Measurement Noises. IEEE/ASME Trans. Mechatron. 2022, 27, 1672–1682. [Google Scholar] [CrossRef]
- Mokhtari, S.; Abbaspour, A.; Kang, K.Y.; Sargolzaei, A. Neural Network-Based Active Fault-Tolerant Control Design for Unmanned Helicopter with Additive Faults. Remote Sens. 2021, 13, 2396. [Google Scholar] [CrossRef]
- Peng, H.; Wei, L.; Zhu, X.; Xu, W.; Zhang, S. Aggressive maneuver oriented integrated fault-tolerant control of a 3-DOF helicopter with experimental validation. Aerosp. Sci. Technol. 2022, 120, 107265. [Google Scholar] [CrossRef]
- Chen, M.; Yan, K.; Wu, Q. Multiapproximator-Based Fault-Tolerant Tracking Control for Unmanned Autonomous Helicopter With Input Saturation. IEEE Trans. Syst. Man Cybern. Syst. 2022, 52, 5710–5722. [Google Scholar] [CrossRef]
- Zhang, F.; Wang, C.; Yang, F. Pattern-based NN control for uncertain pure-feedback nonlinear systems. J. Frankl. Inst. 2019, 356, 2530–2558. [Google Scholar] [CrossRef]
- Zhao, Z.; Ren, Y.; Mu, C.; Zou, T.; Hong, K.S. Adaptive Neural-Network-Based Fault-Tolerant Control for a Flexible String With Composite Disturbance Observer and Input Constraints. IEEE Trans. Cybern. 2021, 1–11. [Google Scholar] [CrossRef] [PubMed]
- Zhang, F.; Wu, W.; Hu, J.; Wang, C. Deterministic learning from neural control for a class of sampled-data nonlinear systems. Inf. Sci. 2022, 595, 159–178. [Google Scholar] [CrossRef]
- Ye, X.; Jiang, J. Adaptive nonlinear design without a priori knowledge of control directions. IEEE Trans. Autom. Control 1998, 43, 1617–1621. [Google Scholar]
- Chen, H.; Haus, B.; Mercorelli, P. Extension of SEIR Compartmental Models for Constructive Lyapunov Control of COVID-19 and Analysis in Terms of Practical Stability. Mathematics 2021, 9, 2076. [Google Scholar] [CrossRef]
- Mu, C.; Wang, K.; Ni, Z. Adaptive Learning and Sampled-Control for Nonlinear Game Systems Using Dynamic Event-Triggering Strategy. IEEE Trans. Neural Netw. Learn. Syst. 2022, 33, 4437–4450. [Google Scholar] [CrossRef]
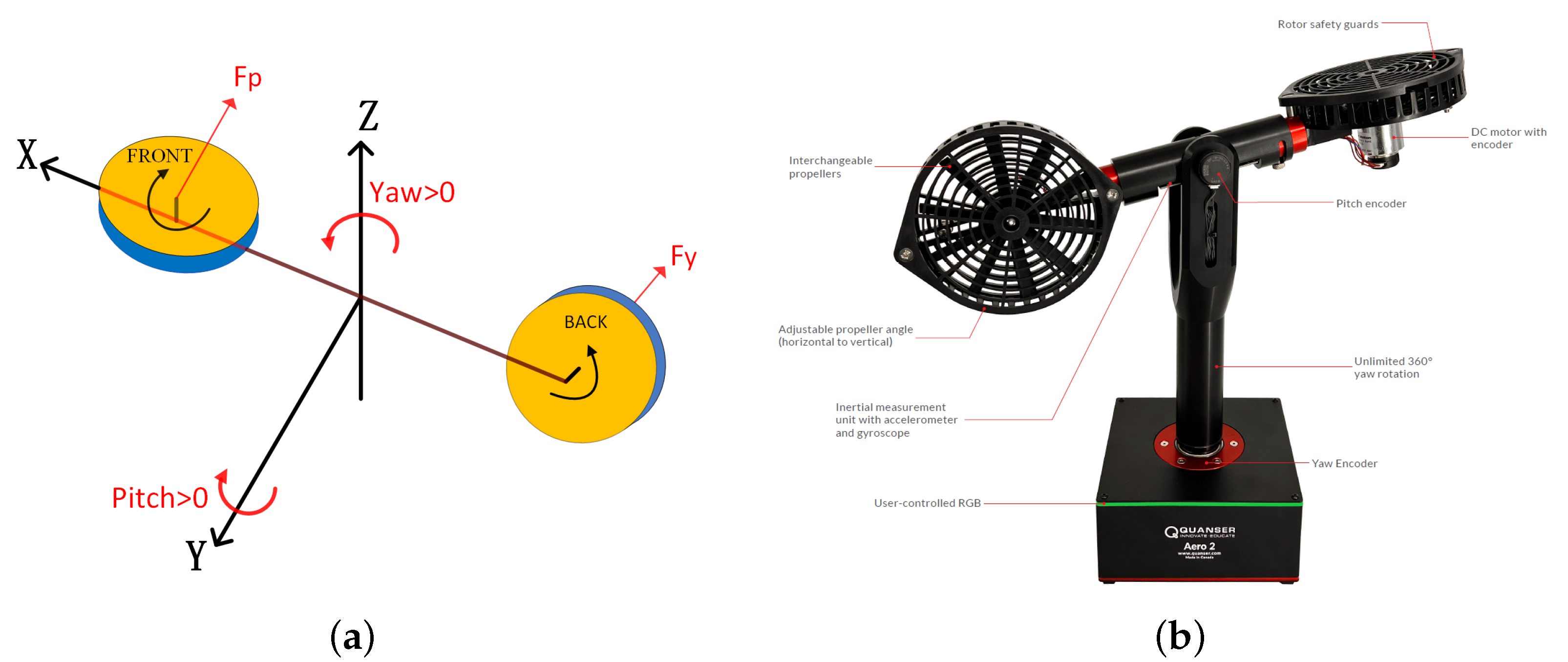

| Symbol | Definition |
|---|---|
| Pitch angle | |
| Yaw angle | |
| Pitch angular velocity | |
| Yaw angular velocity | |
| Pitch angular acceleration | |
| Yaw angular acceleration | |
| The voltage inputs of the front motor | |
| The voltage inputs of the back motor | |
| The viscous friction constants of pitch | |
| The viscous friction constants of yaw | |
| The moments of inertia of the pitch axis | |
| The moments of inertia of the yaw axis | |
| Torque thrust gains acting on pitch axis from pitch propeller | |
| Torque thrust gains acting on pitch axis from yaw propeller | |
| Torque thrust gains acting on yaw axis from pitch propeller | |
| Torque thrust gains acting on yaw axis from yaw propeller | |
| L | The center of mass distance from the body-fixed frame origin |
| M | The mass of the 2-DOF helicopter |
| g | The gravitational acceleration |
| Symbol | Value | Unit | Symbol | Value | Unit |
|---|---|---|---|---|---|
| L | M |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, B.; Wu, J.; He, W.; Tang, G.; Zhao, Z. Adaptive Neural Control for an Uncertain 2-DOF Helicopter System with Unknown Control Direction and Actuator Faults. Mathematics 2022, 10, 4342. https://doi.org/10.3390/math10224342
Wu B, Wu J, He W, Tang G, Zhao Z. Adaptive Neural Control for an Uncertain 2-DOF Helicopter System with Unknown Control Direction and Actuator Faults. Mathematics. 2022; 10(22):4342. https://doi.org/10.3390/math10224342
Chicago/Turabian StyleWu, Bing, Jiale Wu, Weitian He, Guojian Tang, and Zhijia Zhao. 2022. "Adaptive Neural Control for an Uncertain 2-DOF Helicopter System with Unknown Control Direction and Actuator Faults" Mathematics 10, no. 22: 4342. https://doi.org/10.3390/math10224342
APA StyleWu, B., Wu, J., He, W., Tang, G., & Zhao, Z. (2022). Adaptive Neural Control for an Uncertain 2-DOF Helicopter System with Unknown Control Direction and Actuator Faults. Mathematics, 10(22), 4342. https://doi.org/10.3390/math10224342







