1. Introduction
In 1988, the geometric process repair model (GPRM) was first introduced by Lam [
1,
2]. Since then, it has been widely studied, and many extended models have been proposed [
3]. For example, the geometric process is generalized to the extended geometric process [
4], threshold geometric process [
5], doubly geometric process [
6], phase-type geometric process [
7],
α-series process [
8], and so on. The GPRM is suitable for describing the phenomenon whereby “the successive working times of the system after repair become shorter and shorter, while the consecutive repair times of the system after failure become longer and longer” [
1,
2]. However, a system after repair does not always degrade successively in practice [
9]. As Zhang & Wang [
10] say, “a serious failure may lead to the deteriorating of the system, while a slight failure can be eliminated, so that the system is not degenerative”. Zhang & Wang [
4] proposed an extended geometric process repair model (EGPRM) and explicitly expressed the average reward rate. Zhang & Wang [
11] obtained different optimal replacement policies to minimize the average cost rate, maximize the average availability rate, and optimize the tradeoff model of the average cost rate and the average availability rate. Zhang & Wang [
10] proposed an EGPRM considering a repair-replacement problem for a cold standby repairable system with two different components and one repairman, and the optimal replacement policy based on the failure number of Component 2 was given by minimizing the average cost rate of the system. Considering the repairman having multiple vacations, Wang et al. [
12] proposed an EGPRM and explicitly expressed the long-run average cost rate based on the failure number of the component. From the above literature, it can be found that although the EGPRM has more parameters than the GPRM, the EGPRM is closer to the reality than the GPRM, and the parameters can be estimated by the statistical EM (i.e., Expectation-Maximization) algorithm [
11].
The above papers are all focused on the replacement policy
N, i.e., the system is replaced when the number of failures of the system reaches
N. It is much more reasonable to consider bivariate or multi-variant repair and replacement policies from the consideration of practice, and they have also been widely studied. Zhang [
13] generalized Lam’s work by introducing a bivariate replacement policy (
T,
N). Wang & Zhang [
14] proposed a bivariate replacement policy (
L,
N) based on the fixed-length interval of the preventive repair, and the preventive repair number of the system. Wang & Zhang [
15] proposed a bivariate replacement policy based on system reliability and failure number of the system (
R,
N). Chang et al. [
16] considered a bivariate replacement policy (
n,
T) based on the life age and the number of Type-I and Type-II failures. Sheu et al. [
17] extended the bivariate replacement policy (
T,
N) to two trivariate replacement policies (
K,
N,
T) and (
N,
S,
T), in which
K is a tolerance limit of failure and
S is the lower working age.
Since the working time after a lot of maintenance will become shorter and shorter, the maintenance will become more and more frequent, incurring more and more maintenance costs; therefore, when the working time after the last repair is too short, it is not a wise choice to repair the system, and the best option is to replace it. Aiming to resolve this issue, Dong et al. [
18] proposed a bivariate replacement policy in which the system is replaced whenever the working time reaches
T or at the first hitting time of the working time after repair concerning the working time threshold, whichever occurs first. In this paper, we will propose an EGPRM and further consider a new bivariate replacement policy
, i.e., the system is replaced when the working time after the last repair is not longer than the replacement threshold
τ1, or if the system is repaired
N times, whichever occurs first. There are many applications of the EGPRM and the replacement policy
. For example, water filters are devices that remove impurities from water through good physical barriers and chemical or biological processes. The filter cartridge is the core device of water filters, and most of the water filters are made up of multi-stage filter cartridges that are arranged in order of precision from low to high. Interceptions such as rust, sand, colloid, and other impurities are deposited inside the filter cartridges; thus, they need to be regularly manually disassembled and washed, or parts of the filter cartridges need to be replaced (for example, the filter cartridge with low precision) to guarantee the normal operation of the machine. In general, when the speed of filtering water is lower than a certain critical value, which can be viewed as a failure, we wash the filter cartridges or replace part of the filter cartridges, which can be considered an imperfect repair. With the increase in cleaning times or the number of replacements of parts of the filter cartridges, the water filter’s efficiency becomes lower and lower. In other words, the working time after the last repair becomes shorter and shorter; when the working time of a water filter is too short for it to be worth repairing, it is best to replace all the filter cartridges at the same time, which means that a renewal cycle is completed. Moreover, when the number of repairs reaches a fixed threshold, we will also replace all the filter cartridges simultaneously. Thus, considering a tradeoff between the work efficiency and the cost, it is necessary to study the optimal repair and replacement policy to minimize the long run average cost rate.
The delayed repair is common for complex repair systems due to several practical factors [
19]. One reason is that the system failure cannot be detected in time [
20,
21,
22,
23,
24,
25]. Another reason is that the delayed repair time may be due to a maintenance resources mobilization (e.g., maintenance crew, spare parts, tools) [
26,
27], which will be considered in the paper. For example, Zhang [
27] pointed out that it is impossible to repair the system immediately if the repairman is on holiday. The random procurement lead time is considered by Yu et al. [
26], if the
Nth failure of the old system occurs too early, the replacement has to wait until the desired spare part is delivered. There is a threshold for the system to be repaired immediately, which is called the repair threshold in this paper; when the working time after the last repair is longer than the time used for a maintenance resources mobilization, there is no delayed repair time. Otherwise, it needs to wait until the duration after the last repair reaches the repair threshold. Obviously, the delayed repair depends on the working time after the last repair, which is discussed in this paper; however, the delayed repair time and working time are assumed to be independent of each other in most early works [
27].
To the authors’ best knowledge, an extended geometric process repair model with imperfect repair considering replacement threshold and repair threshold has not been found, and
Table 1 summarizes the current results on similar topics.
Remark 1. In Table 1, N,,, andare all symbols of replacement policies, where N stands for the replacement policy under which the system is replaced when the number of failures of the system gets to N;stands for the replacement policy whenever the working age of the system reaches T or at the first hitting time of the working time after repair for the working time thresholdτ, whichever occurs first;stands for the replacement policy under which the system is replaced when the working time is shorter than the replacement thresholdτ1, or if the system is repaired N times, whichever occurs first;stands for a replacement policy under which the system is replaced when the working time is shorter than the replacement thresholdτ1.
The main contribution of this paper to the existing literature is as follows:
A novel model for imperfect delayed repair is built by using extended geometric processes.
Replacement and repair thresholds are involved.
Two kinds of replacement policies and are considered.
The explicit expressions of the long-run average cost rate are obtained.
The existence of optimal policies is proved, and numerical examples are presented to demonstrate the application of the results obtained in the paper.
The remainder of the paper is organized as follows: the problem definition is introduced in
Section 2. In
Section 3, the exact expressions of the long-run average cost rate under the policy
and its special case (policy
) are derived and optimal policies are proved.
Section 4 provides numerical examples to show that optimal replacement policies
and
are existent and unique. Finally, conclusions are given in
Section 5.
2. Problem Definition
In this paper, we study a repairable system based on the extended geometric process, and the basic assumptions about the replacement model are given as follows:
Assumption 1. Initially, the system is new.
Assumption 2. The system degrades geometrically with probability qn and does not degrade with probability pn = 1 − qn at the nth repair for n = 1,2,…
Let
Xn be the working time after the (
n − 1)th maintenance and {
Xn,
n = 1,2,…} be a non-increasing process, where
X1 is a new system’s working time. Thus, {
Xn,
n = 1,2,…} constitutes an extended geometric process with the cumulative distribution function
where
pn +
qn = 1, 0 ≤
pn ≤ 1, (
n = 1,2,…),
a > 1 and
t ≥ 0. Furthermore, we assume that
E[
X1] =
λ > 0.
Let
Yn be the repair time after the
nth failure, and {
Yn,
n = 1,2,…} forms an extended geometric process, which has the cumulative distribution function
where 0 <
b < 1. Assume
EY1 =
η ≥ 0, and
η = 0 implies that it is negligible for the repair time.
Assumption 3. Xn andYn are independent of each other, n = 1,2,….
Assumption 4. The system is subjected to self-announcing failures, i.e., system failures can be detected simultaneously. Assume a replacement thresholdexists, i.e., when the working timeis no longer than, the system will be replaced. Furthermore, we assume the system is replaced immediately, and the replacement takes negligible time.
Assumption 5. There exists the time for a maintenance resources mobilization, which takes time, i.e., the repair threshold.
If , the system can always be repaired immediately when the working time after the last repair is longer than . If , there exist three cases for the working time (): (a) , the system will be replaced immediately; (b) , the system will be repaired, but it needs to wait until the duration after the (n − 1)th repair reaches , i.e., there exists the delayed repair; (c) , the system will be repaired immediately. In the paper, we focus on the case of , because does not work for the case of .
Assumption 6. The cost rate of the repair is; thus,is the cost of a repair when the system is repaired immediately after theth failure. Moreover, we assume that there is a penalty because of the delayed repair, and the cost is in proportion to the length of the delayed repair time; thus, the cost of a repair is, when the system is not repaired at once, whereis the wait time after theth failure,is the penalty cost rate during the wait for repair state, and The fixed replacement cost is.
Assumption 7. The replacement policyis used, i.e., the system is replaced at the first hitting time of the working timeconcerning the replacement thresholdor at the moment of theth failure, whichever occurs first.
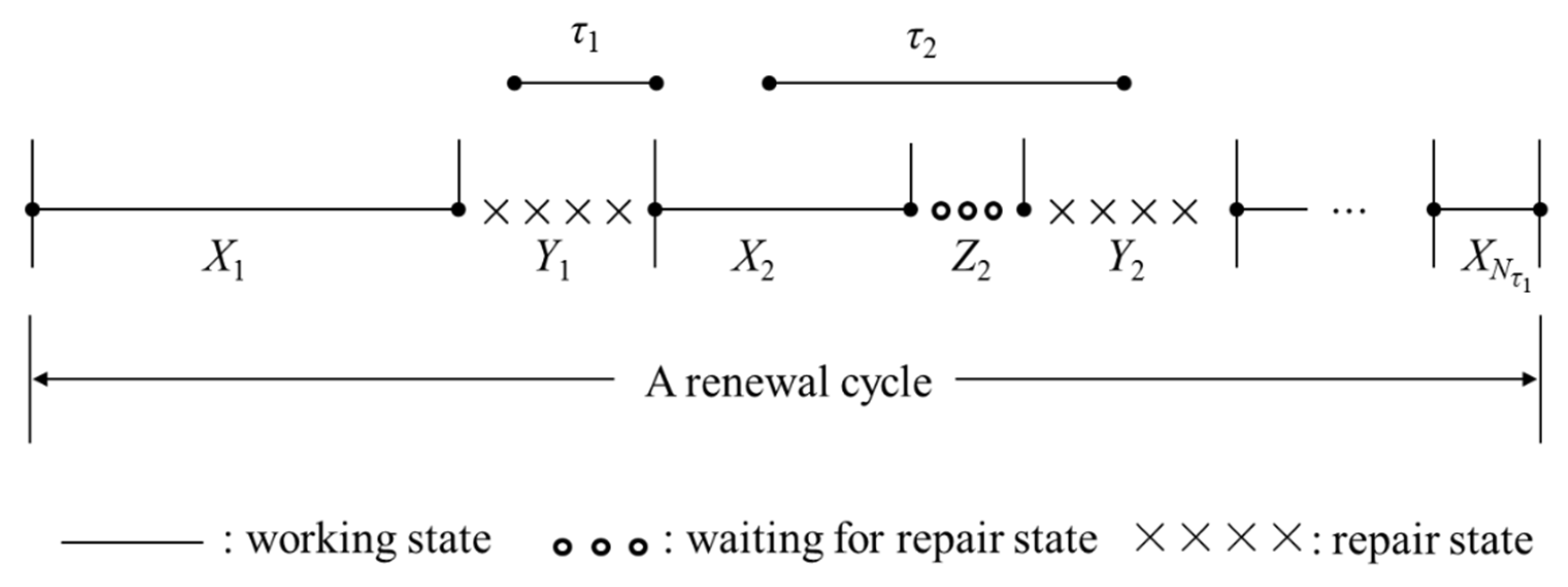
Remark 2. For the replacement policy, if we let N → ∞, i.e., we do not consider the number of repairs, we can obtain the replacement policy , i.e., the system is replaced at the first hitting time of the working time for the replacement threshold , where . In the paper, we also discuss the replacement policy in detail.
Remark 3. For the replacement policy, if we let, i.e., we do not consider the replacement threshold, we can obtain the replacement policy, i.e., the system is replaced when the number of repair reaches, which has been discussed by many authors under different situations, see, for example, Lam [
1,
2]
, Zhang [
27]
, Zhang & Wang [
4,
10,
11]
, and references therein. A renewal cycle is defined as the time interval between the installation of a new system and the first replacement or a time interval between two consecutive replacements. A sample path of the system is illustrated in
Figure 1. Our objective is to find the optimal replacement policies to minimize the long-run average cost rate of the system under two policies,
and
.
3. Optimization Model Development
Let
denote the first replacement time, and let
denote the interval between the (
)th replacement and the
th replacement. Obviously,
forms a renewal process. According to the renewal theorem, the long-run average cost rate is given by
where a renewal cycle is a time between two consecutive replacements. In practice, the repair and replacement policies are mainly accounted for with regard to the cost or the availability consideration, aiming to find the optimal policies which minimize (or maximize) the long-run average cost rate (or availability) [
28,
29,
30,
31,
32,
33,
34,
35,
36]. In the following, we will concentrate on the optimal replacement policies to minimize the long-run average cost rate under two kinds of replacement policies,
and
.
3.1. Long-Run Average Cost Rate under Replacement Policy
Under the replacement policy
, the system is replaced at the time of the
th failure or at the first hitting time (
) of
for
, whichever occurs first; therefore, the length of a renewal cycle
is a random variable, and we have
where
(or
) is the working time during a cycle based on policy
(or
N);
(or
) is the repair time during a cycle based on policy
(or
N);
(or
) is the wait time during a cycle under policy
(or
N); define
;
is an indicator function of event
, i.e.,
, if
occurs; otherwise
.
The expected length of a renewal cycle follows
Computing expectations by conditioning, the first term of Equation (6) becomes
From the results of Zhang & Wang (2017), since
and
are extended geometric processes, we have
where
,
,
and
.
The expression of
follows from Equation (3) that
By substituting Equations (8)–(10) into Equation (7), we have
where, define
;
follows from the fact that
is the event that the working time after the
nth repair is longer than
for
, and it is not longer than
after the (
k − 1)th repair.
Similar to the calculation of the first term of Equation (6), for the second term of Equation (6), we have
Therefore, according to Equations (11) and (12), Equation (6) becomes
Similarly, the expected cost in a renewal cycle is given by
Therefore, according to Equations (13) and (15), the long-run average cost rate
under policy
is given by
Specially, if and , , we can obtain the special case of the EGPRM by substituting and () into Equation (16), i.e., the result is that the system degrades geometrically with constant probability and does not degrade at each maintenance with constant probability . Furthermore, if , , we can get the GPRM by substituting and () into Equation (16), i.e., the result in the case that the system after repair degrades successively.
There exists the minimum long-run average cost rate. Equation (16) is a bivariate function of
and
. When
is fixed, it is a function of
. For example, if
,
will become
Thus, if
is fixed, we can minimize
analytically or numerically to get optimal
, i.e., when
,
are obtained respectively, such that the corresponding
,
, …,
, … is minimized. Because of the finiteness of the total lifetime span for the repairable system, the minimum long-run average cost rate can be confirmed. Therefore, the minimum long-run average cost rate based on
,
, …,
, … can be obtained; thus, we have
Furthermore, we can also obtain the optimal policy from another angle, i.e.,
3.2. Special Cases
Some special cases are summarized as follows.
Case 1. Policy .
If we let
, the policy
becomes the policy
, and it follows from Equation (16) that the long-run average cost rate under the policy
is given by
Especially, if the system degrades geometrically with constant probability and does not degrade at each maintenance with constant probability , i.e., and , , we can obtain the special case of the EGPRM by substituting and , () into Equation (17). Furthermore, if the system after maintenance degrades successively, i.e., , , we can get the GPRM by substituting and , () into Equation (17).
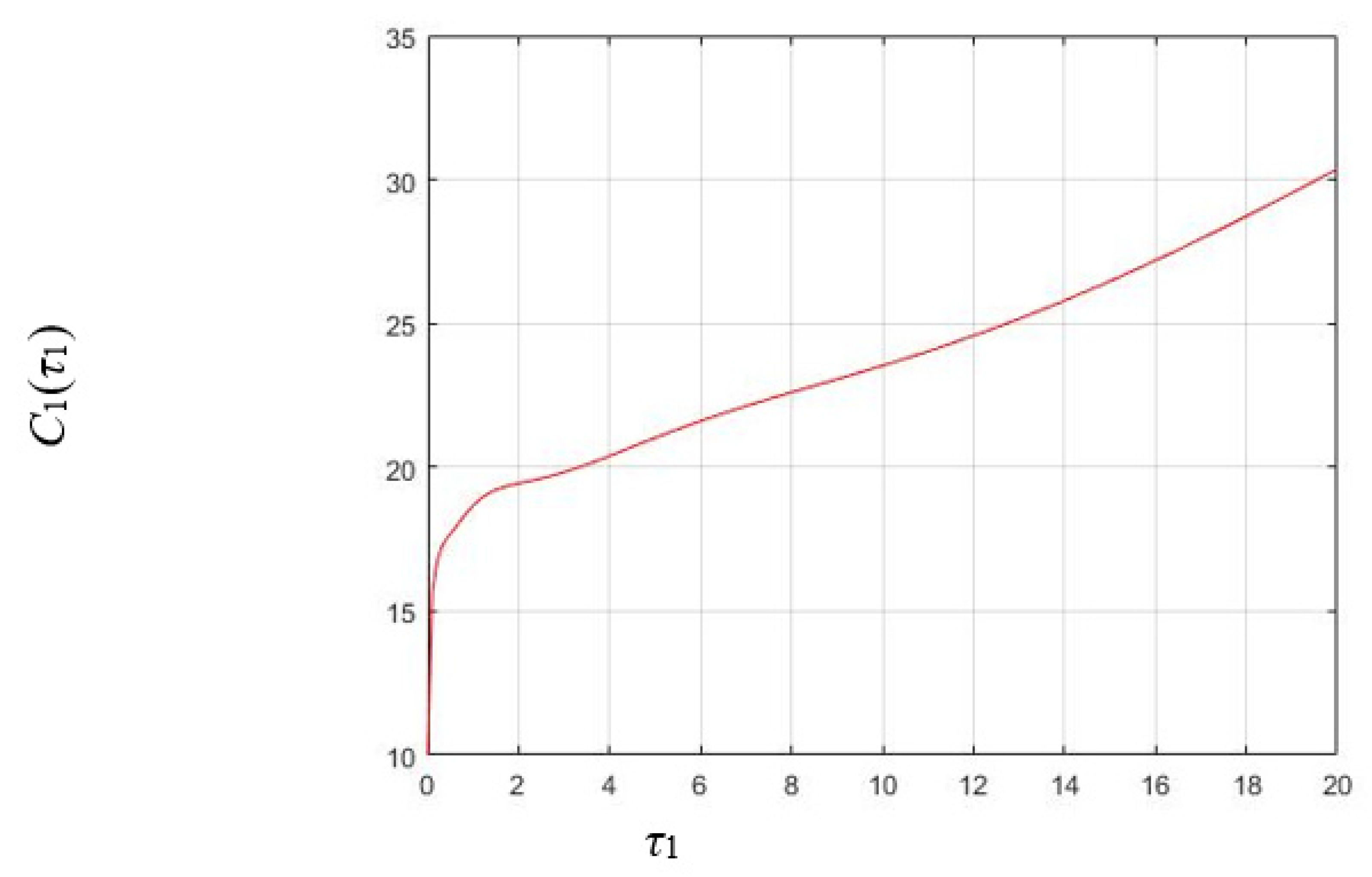
The optimal , which minimizes , exists. Because is a continuous function on the interval , from the extreme value theorem, there must exist an optimal which minimizes . Moreover, the optimal policies can be obtained by numerical methods under some conditions, and the optimal policies under different conditions are unique in the following numerical examples.
Furthermore,
therefore, the optimal policy
is better than the optimal policy
.
Case 2..
Since
and
for any
, the policy
is the case that the system will fail only when the working time reaches 0; therefore, Equation (17) becomes
If
, the policy
will become the policy
, and Equation (16) becomes
Case 3. .
Moreover, if
,
and
,
, Equation (20) becomes Lam’s result [
1,
2], i.e.,
5. Conclusions
The working time becomes shorter and shorter after many repairs are conducted for a repairable degradation system; when the working time of a system is too short for it to be worth repairing, it is best to replace it. Moreover, because of the delayed repair, the system cannot be repaired immediately, and the delayed repair time is dependent on the working time, whereas the delayed repair time is always assumed to be independent of the working time in most early works. In order to describe the above phenomena, a repair replacement model was developed using the extended geometric processes.
To minimize the long-run average cost rate, there are two replacement policies, one is based on the working time after the last repair, and the system is replaced when the working time first hits the replacement threshold; the other is a bivariate policy, and the system is replaced when the working time first hits the replacement threshold or when the Nth failure happens, whichever comes first. The explicit expressions of the long-run average cost rate under these two policies and some special cases can be easily used. The existence of optimal policies is proved, and numerical examples are presented to illustrate the application of the developed approach. Moreover, the optimal policy is proved to be better than the optimal policy .
Furthermore, if we consider the reward, Lam’s model [
1,
2] can be viewed as a special case of ours, in the case that
.
According to the proposed model, the characteristics of the long-run average cost rate curves based on the real applications can be easily obtained, and the optimal replacement policies are suitable in practical applications.
As a generalization and development of the GPRM, EGPRM is more reasonable than GPRM, and there are still many aspects worthy of in-depth study. For example, EGPRM can be applied to maintenance problems for a multi-component repairable system, and different systems, including series systems, parallel systems or k-out-of-n: G systems or multiple failure modes, are also worth considering. In addition, the parameter estimation is very important, which is also of great interest to investigate.