Curriculum Design in Construction Engineering Departments for Colleges in Taiwan
Abstract
:1. Introduction
2. Literature Review
2.1. Core Competency Requirements for CPM Personnel
2.2. Creative Thinking Instruction
- Jones (2011) regarded brainstorming as the most direct and common approach to training creative teaching abilities; it was the sudden appearance of ideas and aesthetic feeling, which would stimulate the partners to induce resonance [19];
- Attribute listing: Attribute listing was to list old thoughts, ideas, and principles for recombination or modification to become novel. The creation of new products was mostly the remodeling of old objects, and the remodeling was to change the attributes;
- Morphological analysis: Attribute listing stressed modification, while morphological analysis paid attention to combinations;
- Synectics: Developed by Gordon, synectics utilized analogy and metaphor for helping thinkers analyze problems so as to form various points of view; and
- Reviews of 6 w: It was intended to review current regulations or products from six angles of (1) Why? (2) What? (3) Who? (4) When? (5) Where? and (6) How?
2.3. Science, Technology, Engineering, and Mathematics Education for CPM Personnel
2.4. Summary of Competencies for CPM Personnel
3. Methodology of Research
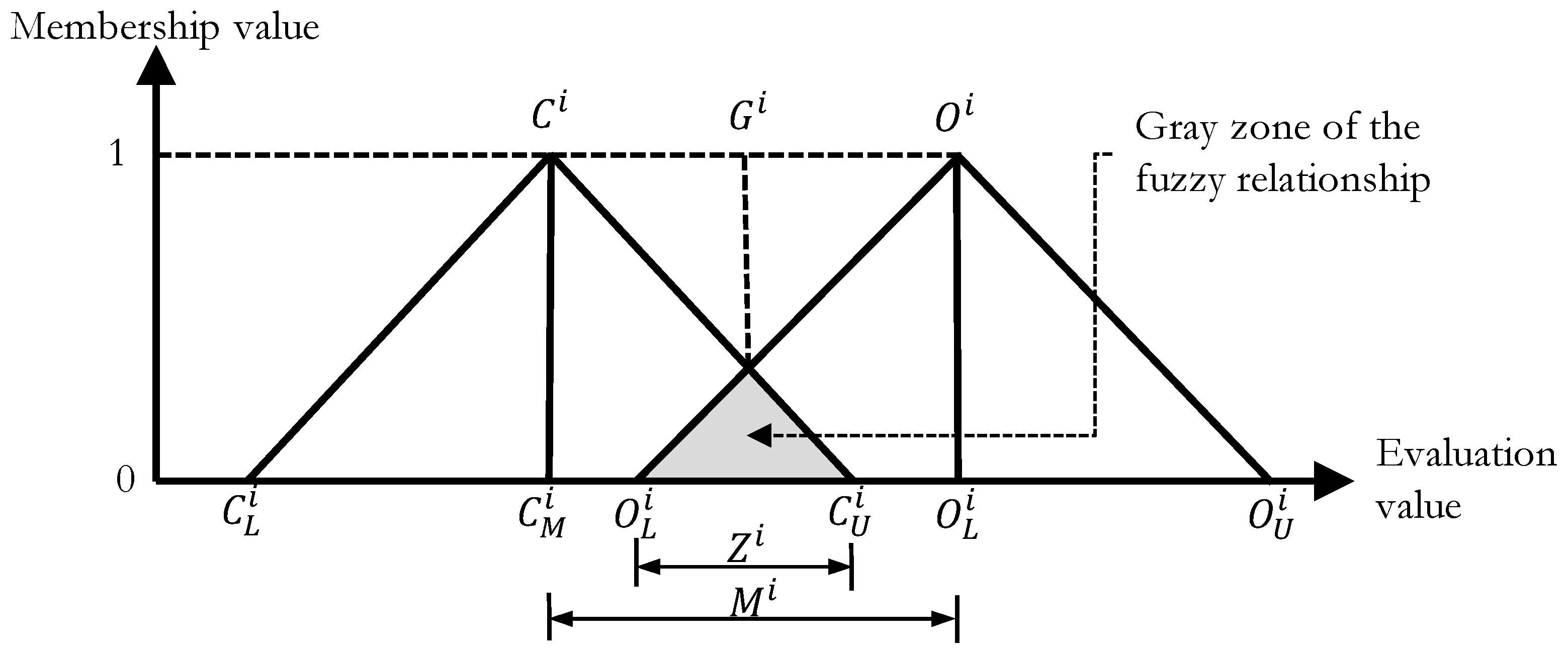
3.1. Fuzzy Delphi Method
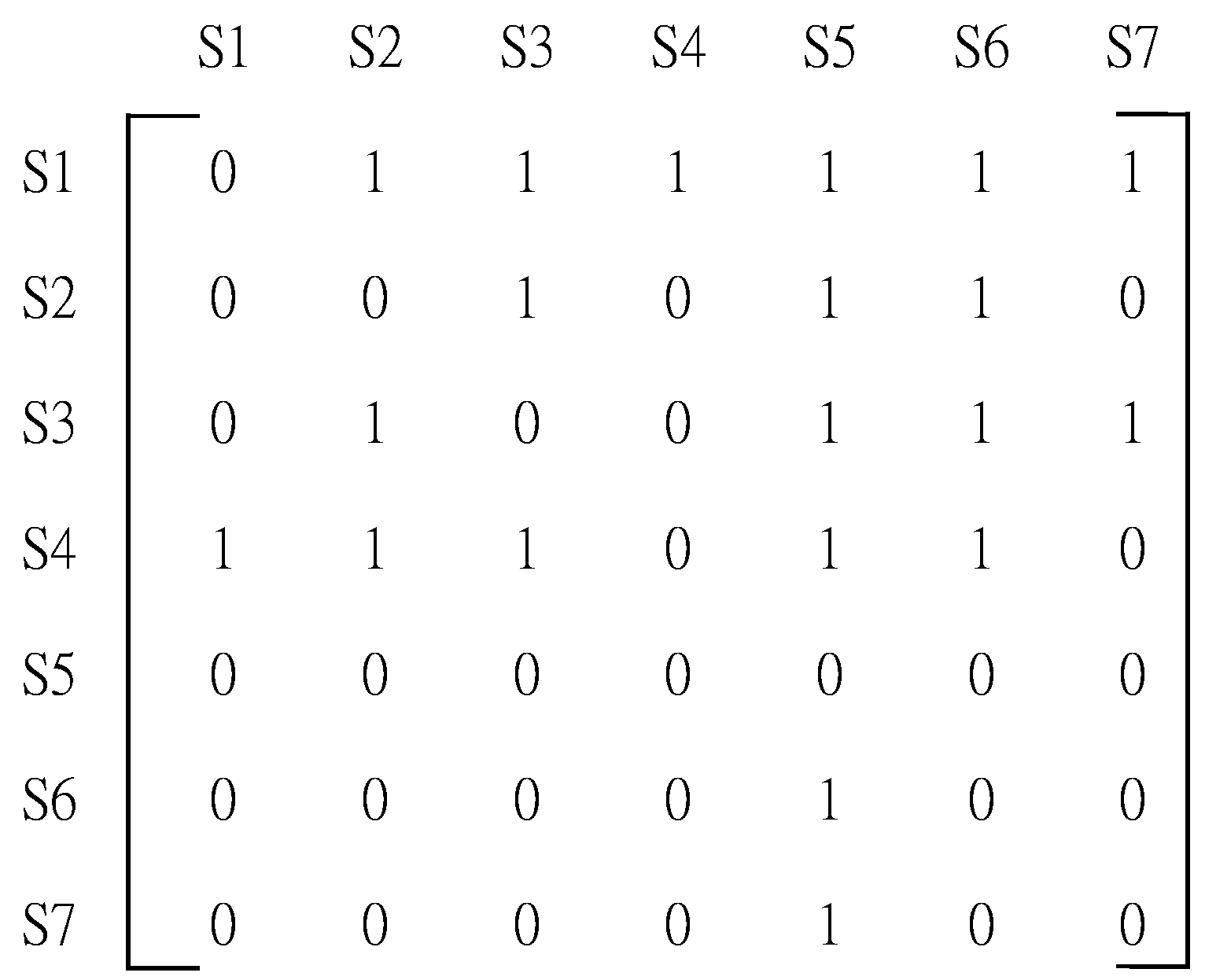
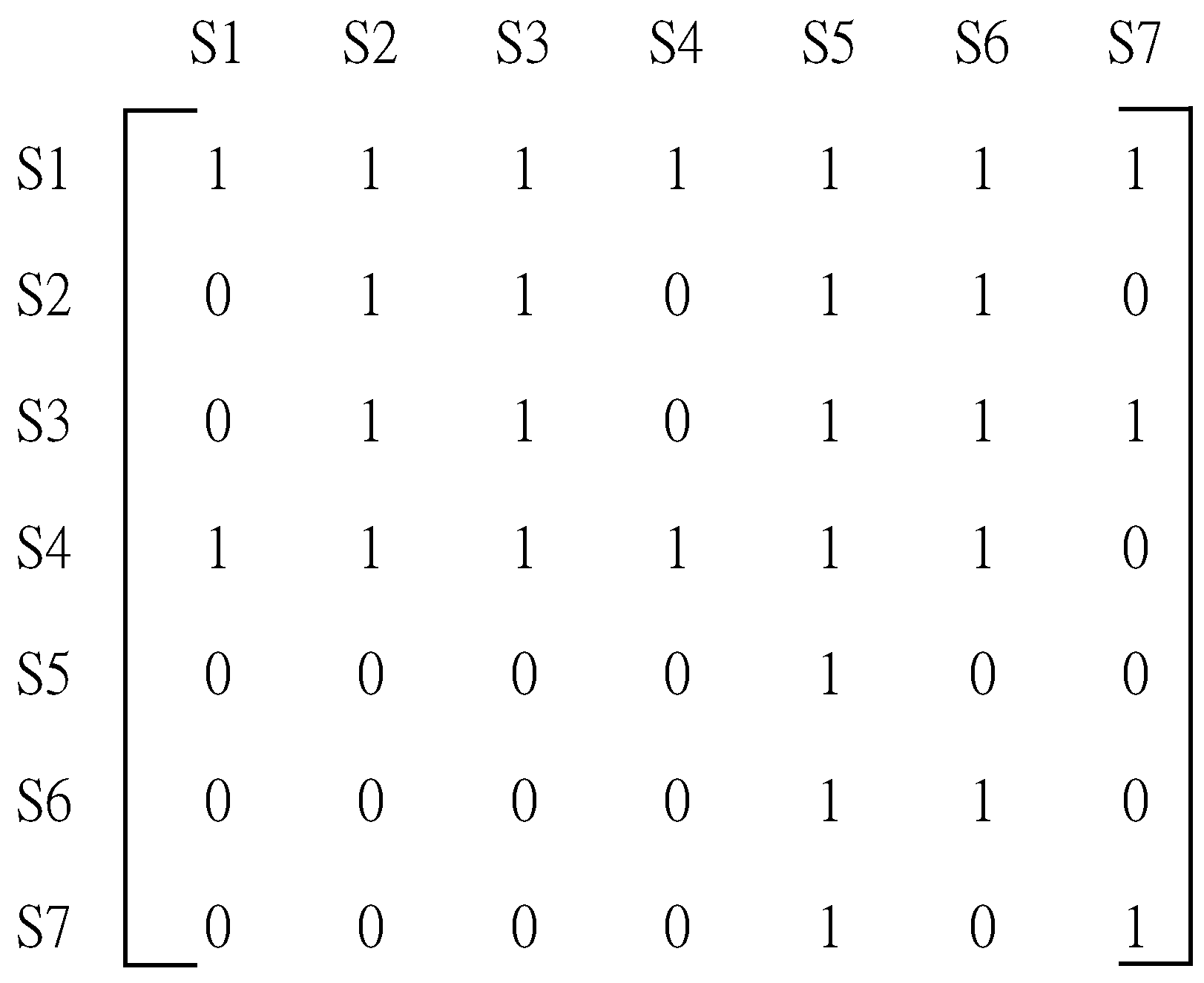
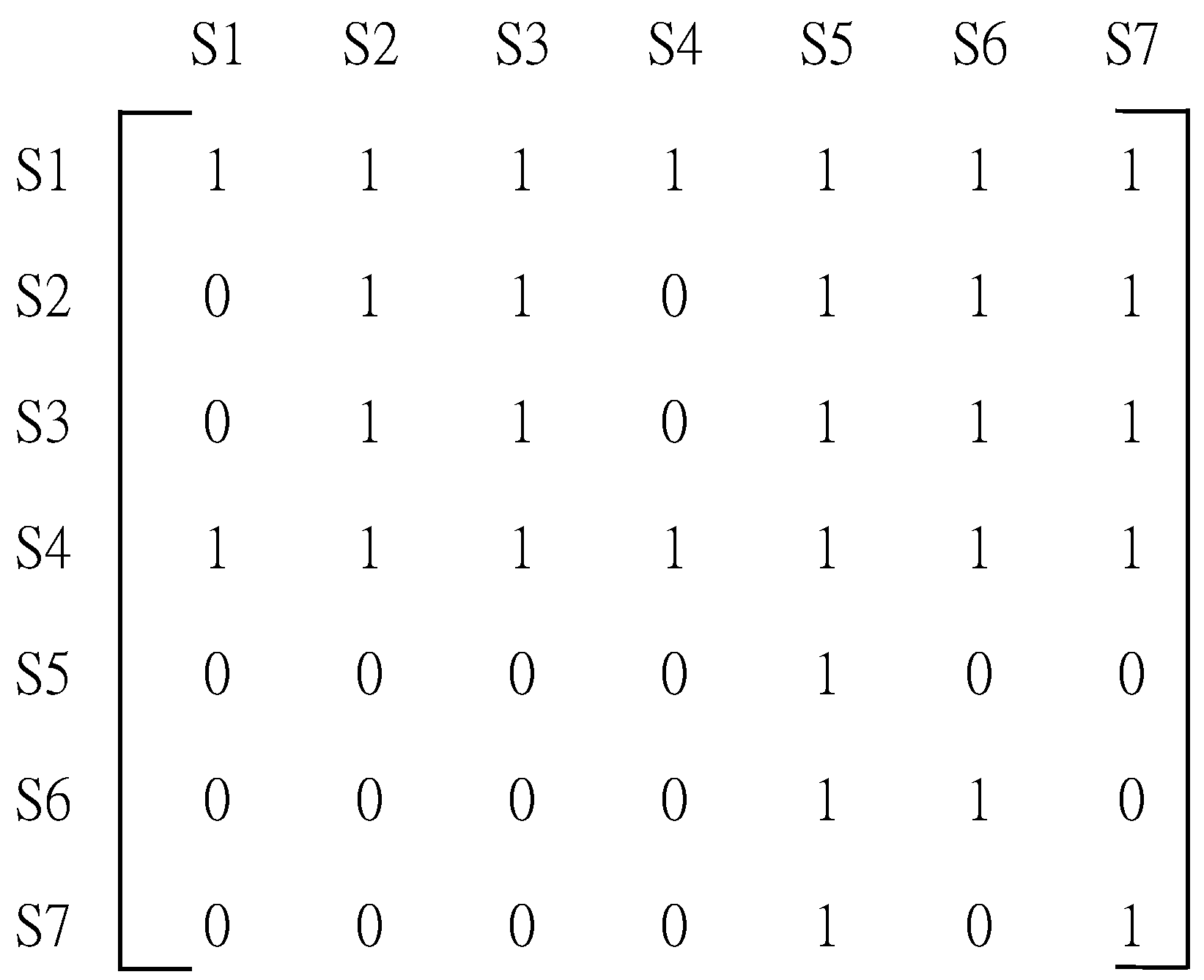
3.2. Interpretive Structural Modelling (ISM) Definition
3.3. Calculation Steps of ISM
4. Discussion
4.1. Statistical Analysis Using the Fuzzy Delphi Method
4.2. Analysis of ISM Statistics
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Grout, I. Remote laboratories as a means to widen participation in stem education. Educ. Sci. 2017, 7, 85. [Google Scholar] [CrossRef]
- Hite, R.; Milbourne, J. A proposed conceptual framework for k–12 stem master teacher (stemmate) development. Educ. Sci. 2018, 8, 218. [Google Scholar] [CrossRef]
- Tsai, H.-Y.; Chung, C.-C.; Lou, S.-J. Construction and development of istem learning model. Eurasia J. Math. Sci. Technol. Educ. 2018, 14, 15–32. [Google Scholar] [CrossRef]
- Yıldırım, B.; Selvi, M. An experimental research on effects of stem applications and mastery learning. Eğitimde Kuram ve Uygulama 2017, 13, 183–210. [Google Scholar] [CrossRef]
- Yıldırım, B.; Sidekli, S. Stem applications in mathematics education: The effect of stem applications on different dependent variables. J. Balt. Sci. Educ. 2018, 17, 200–214. [Google Scholar]
- Asghar, A.; Huang, Y.-S.; Elliott, K.; Skelling, Y. Exploring secondary students’ alternative conceptions about engineering design technology. Educ. Sci. 2019, 9, 45. [Google Scholar] [CrossRef]
- Palmer, T.A.; Burke, P.F.; Aubusson, P. Why school students choose and reject science: A study of the factors that students consider when selecting subjects. Int. J. Sci. Educ. 2017, 39, 645–662. [Google Scholar] [CrossRef]
- Kotkas, T.; Holbrook, J.; Rannikmäe, M. A theory-based instrument to evaluate motivational triggers perceived by students in stem career-related scenarios. J. Balt. Sci. Educ. 2017, 16, 836–854. [Google Scholar]
- Xiang, G.; Yalan, D.; Yu, Y. Discussion on the impact of international evaluation on development of construction management professional education. Eurasia J. Math. Sci. Technol. Educ. 2017, 13, 5655–5662. [Google Scholar] [CrossRef]
- Shi, W.-Z.; Wang, J. Comparison on views of nature of science between math and physics students. J. Balt. Sci. Educ. 2017, 16, 77–85. [Google Scholar]
- Torres, J.; Vasconcelos, C. Nature of science and models: Comparing portuguese prospective teachers’ views. Eurasia J. Math. Sci. Technol. Educ. 2015, 11, 1473–1494. [Google Scholar]
- Siew, N.M.; Chin, M.K. Design, development and evaluation of a problem-based with cooperative module on scientific creativity of pre-schoolers. J. Balt. Sci. Educ. 2018, 17, 215–228. [Google Scholar]
- Hu, W.; Shi, Q.Z.; Han, Q.; Wang, X.; Adey, P. Creative scientific problem finding and its developmental trend. Creat. Res. J. 2010, 22, 46–52. [Google Scholar] [CrossRef]
- Chang, C.-Y.; Lin, P.-L. The relationship between science achievement and self-concept among gifted students from the third international earth science olympiad. Eurasia J. Math. Sci. Technol. Educ. 2017, 13, 3993–4007. [Google Scholar]
- Birinci, C.M. Teacher in reggio emilia approach: Educational needs and views. Eurasia J. Math. Sci. Technol. Educ. 2017, 14, 279–290. [Google Scholar] [CrossRef]
- Canman, D. İnsan Kaynakları Yönetimi; Yargı Yayınevi: Ankara, Turkey, 2000. [Google Scholar]
- Hu, R.; Wu, Y.-Y.; Shieh, C.-J. Effects of virtual reality integrated creative thinking instruction on students’ creative thinking abilities. Eurasia J. Math. Sci. Technol. Educ. 2016, 12, 477–486. [Google Scholar]
- Cao, J.; Nishihara, A. Understand learning style by eye tracking in slide video learning. J. Educ. Multimed. Hypermedia 2012, 21, 335–358. [Google Scholar]
- Jones, R.M. Development of Attitudes of Children toward Coastal Environment Themes Survey: Exploring Attitudes of Louisiana Middle School Students. Master’s Thesis, Louisiana State University, Baton Rouge, LA, USA, 2011. [Google Scholar]
- Flogie, A.; Lakota, A.B.; Aberšek, B. The psychosocial and cognitive influence of ict on competences of stem students. J. Balt. Sci. Educ. 2018, 17, 267–276. [Google Scholar]
- Bílek, M. Intercultural learning: Challenges for current science education. J. Balt. Sci. Educ. 2018, 17, 532–534. [Google Scholar]
- LaForce, M.; Noble, E.; Blackwell, C. Problem-based learning (PBL) and student interest in stem careers: The roles of motivation and ability beliefs. Educ. Sci. 2017, 7, 92. [Google Scholar] [CrossRef]
- UNESCO Office Jakarta; Regional Bureau for Science in Asia and the Pacific. Annual Report 2010. Unesco Office Jakarta. Available online: http://unesdoc.unesco.org/images/0019/001921/192108e.pdf (accessed on 5 December 2018).
- EL-Deghaidy, H.; Mansour, N.; Alzaghibi, M.; Alhammad, K. Context of stem integration in schools: Views from in-service science teachers. Eurasia J. Math. Sci. Technol. Educ. 2017, 13, 2459–2484. [Google Scholar] [CrossRef]
- Augustine, N.R. Rising above the gathering storm: Energizing and employing america for a brighter economic future. Retrieved March 2005, 19, 2008. [Google Scholar]
- Bicer, A.; Capraro, R.M.; Capraro, M.M. Integrated stem assessment model. Eurasia J. Math. Sci. Technol. Educ. 2017, 13, 3959–3968. [Google Scholar]
- Zadeh, L.A. Fuzzy sets. Inf. Control 1965, 8, 338–353. [Google Scholar] [CrossRef]
- Ishikawa, A.; Amagasa, M.; Shiga, T.; Tomizawa, G.; Tatsuta, R.; Mieno, H. The max-min delphi method and fuzzy delphi method via fuzzy integration. Fuzzy Set Syst. 1993, 55, 241–253. [Google Scholar] [CrossRef]
- Hsieh, H.-N.; Hou, C.-Y.; Chou, C.-Y. Smart city strategy refined with public participation in the case of hsinchu city, taiwan. ICIC Express Lett. Part B Appl. 2015, 6, 619–626. [Google Scholar]
- Su, C.-H.; Hsaio, K.-C. Developing and evaluating gamifying learning system by using flow-based model. Eurasia J. Math. Sci. Technol. Educ. 2015, 11, 1283–1306. [Google Scholar]
- Ho, S.-Y.; Chen, W.-T.; Hsu, W.-L. Assessment system for junior high schools in taiwan to select environmental education facilities and sites. Eurasia J. Math. Sci. Technol. Educ. 2017, 13, 1485–1499. [Google Scholar] [CrossRef]
- Shyr, W.-J. Developing the principal technology leadership competency indicators for technical high schools in k-12 in taiwan. Eurasia J. Math. Sci. Technol. Educ. 2017, 13, 2085–2093. [Google Scholar] [CrossRef]
- Chen, Y.-L.; Cheng, A.-C.; Hsueh, S.-L.; Qu, D. Dahp expected utility based evaluation model for management performance on interior environmental decoration–an example in taiwan. Eurasia J. Math. Sci. Technol. Educ. 2017, 13, 8257–8265. [Google Scholar] [CrossRef]
- Hsieh, H.-N.; Chou, C.-Y.; Chen, Y.-Y.; Hou, C.-Y. Applying a fuzzy delphi method to construct intelligent city indicators: A case study of taichung. ICIC Express Lett. Part B Appl. 2014, 5, 1693–1699. [Google Scholar]
- Jeng, T.-B. Fuzzy Assessment Model for Maturity of Software Organization in Improving Its Staff’s Capability. Master’s Thesis, National Taiwan University of Science and Technology, Taipei City, Taiwan, 2001. [Google Scholar]
- Warfield, J.N. Developing interconnection matrices in structural modeling. IEEE Trans. Syst. Man Cybern. 1974, 1, 81–87. [Google Scholar] [CrossRef]
- Liu, H.-L. Planning wetland ecology-based outdoor education courses in taiwanese junior high schools. Eurasia J. Math. Sci. Technol. Educ. 2017, 13, 3261–3281. [Google Scholar] [CrossRef]
- Wu, Y.; Bian, Q. Research on the drivers of cost control in hydropower construction project. ICIC Express Lett. Part B Appl. 2012, 3, 1603–1608. [Google Scholar]
- Lee, Y.-C.; Shiau, Y.-C.; Hsu, W.-L. Applying interpretive structure modeling on the interactive correlations on factor analysis in natural and cultural scenic area at taiwan. Artif. Life Robot. 2015, 21, 37–42. [Google Scholar] [CrossRef]
- Warfield, J.N. Societal Systems: Planning, Policy, and Complexity; John Wiley & Sons: Hoboken, NJ, USA, 1976. [Google Scholar]
- Sage, A.P. Methodology for Large-Scale Systems; McGraw-Hill College: Hightstown, NJ, USA, 1977. [Google Scholar]
- Warfield, J.; Ayiku, M. Sociotechnical modeling for developing nations. SCIMA 1989, 18, 25–40. [Google Scholar]

| Number | Training Course | Competencies to Be Developed |
|---|---|---|
| S1 | Science | General sciences: Physics, chemistry, and engineering economics Professional sciences: Geography, material mechanics, and ecology |
| S2 | Technology | Engineering statistics, engineering valuation, surveying, and geographic information systems Construction drawing interpretation and mapping, information technology, project management, information model construction |
| S3 | Engineering | Experiment design, implementation, analysis, and statistical interpretation Planning and designing construction project systems, components, and processes Skills, modern technologies, and tools required for managing and implementing projects |
| S4 | Mathematics | Calculus and engineering mathematics Geometry and logic |
| S5 | Leadership and execution | Communication, coordination, and teamwork Identifying, analyzing, summarizing, and solving construction problems International project management Policy development, global view, and cross-departmental understanding based on systems thinking |
| S6 | Innovation | Creativity and policy innovation capacity Creative thinking and creative tools Five cognitive forces of creativity: sensitivity, fluency, flexibility, originality, and elaboration Four affective attitudes of creativity: imagination, willingness to face challenges, curiosity, and willingness to be adventurous |
| S7 | Ethics and law | Continual learning and knowledge of sustainable engineering, contemporary topics, and the effects of engineering solutions on ecology, the environment, society, and the global community Knowledge of construction law, abiding by professional ethics, dedication to work, and team spirit required to collectively undertake social responsibilities Establishing a culture of high moral standards, honesty, and integrity in the construction industry |
| Number | Training Course | Value | G1 | G2 | G3 | S1 | S2 | S3 | I1 | I2 | I3 | Min | Max | Geometric Mean | Consensus Gi |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| S1 | Science | Single value | 7 | 7 | 7 | 8 | 6 | 6 | 7 | 6 | 8 | 6 | 8 | 6.85 | 6.68 |
| Minimum value (C) | 5 | 5 | 5 | 5 | 5 | 5 | 6 | 4 | 7 | 4 | 7 | 5.17 | |||
| Maximum value (O) | 8 | 8 | 9 | 9 | 7 | 7 | 8 | 9 | 9 | 7 | 9 | 8.18 | |||
| S2 | Technology | Single value | 8 | 7 | 7 | 6 | 6 | 7 | 8 | 5 | 6 | 5 | 8 | 6.60 | 7.40 |
| Minimum value (C) | 9 | 5 | 6 | 4 | 5 | 6 | 6 | 2 | 3 | 2 | 9 | 4.72 | |||
| Maximum value (O) | 9 | 9 | 9 | 7 | 7 | 8 | 9 | 7 | 8 | 7 | 9 | 8.06 | |||
| S3 | Engineering | Single value | 6 | 6 | 7 | 6 | 7 | 6 | 7 | 6 | 8 | 6 | 8 | 6.52 | 7.29 |
| Minimum value (C) | 4 | 5 | 6 | 4 | 5 | 4 | 5 | 4 | 8 | 4 | 8 | 4.87 | |||
| Maximum value (O) | 7 | 7 | 8 | 9 | 9 | 9 | 8 | 9 | 9 | 7 | 9 | 8.29 | |||
| S4 | Mathematics | Single value | 6 | 6 | 7 | 5 | 7 | 7 | 9 | 5 | 5 | 5 | 9 | 6.22 | 6.52 |
| Minimum value (C) | 4 | 5 | 6 | 4 | 6 | 5 | 7 | 5 | 4 | 4 | 7 | 5.02 | |||
| Maximum value (O) | 8 | 8 | 9 | 8 | 9 | 8 | 10 | 8 | 6 | 6 | 10 | 8.15 | |||
| S5 | Leadership and execution | Single value | 8 | 8 | 9 | 7 | 6 | 7 | 8 | 7 | 7 | 6 | 9 | 7.40 | 8.31 |
| Minimum value (C) | 6 | 7 | 9 | 5 | 5 | 6 | 9 | 5 | 6 | 5 | 9 | 6.29 | |||
| Maximum value (O) | 10 | 9 | 10 | 10 | 8 | 9 | 9 | 9 | 9 | 8 | 10 | 9.20 | |||
| S6 | Innovation | Single value | 8 | 7 | 8 | 8 | 8 | 9 | 7 | 8 | 8 | 7 | 9 | 7.87 | 8.22 |
| Minimum value (C) | 7 | 6 | 9 | 6 | 7 | 9 | 5 | 7 | 7 | 5 | 9 | 6.89 | |||
| Maximum value (O) | 10 | 9 | 9 | 10 | 9 | 10 | 9 | 10 | 10 | 9 | 10 | 9.54 | |||
| S7 | Ethics and law | Single value | 8 | 8 | 9 | 7 | 8 | 7 | 8 | 7 | 7 | 7 | 9 | 7.64 | 7.91 |
| Minimum value (C) | 7 | 7 | 9 | 5 | 5 | 6 | 9 | 5 | 6 | 5 | 9 | 6.39 | |||
| Maximum value (O) | 10 | 9 | 10 | 10 | 10 | 9 | 9 | 9 | 9 | 9 | 10 | 9.43 |
| No. | Competency Training Course | Conservative Value | Optimistic Value | Single Value | Geometric Mean M | Test Value | Consensus Value | Ranking | |||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Min | Max | Min | Max | Min | Max | Ci | Oi | ai | Mi | Zi | Mi-Zi | Gi | |||
| S1 | Science | 4 | 7 | 7 | 9 | 6 | 8 | 5.17 | 8.18 | 6.85 | 3.01 | 0.00 | 3.01 | 6.68 | 6 |
| S2 | Technology | 2 | 9 | 7 | 9 | 5 | 8 | 4.72 | 8.06 | 6.60 | 3.34 | 2.00 | 1.34 | 7.40 | 4 |
| S3 | Engineering | 4 | 8 | 7 | 9 | 6 | 8 | 4.87 | 8.29 | 6.52 | 3.42 | 1.00 | 2.42 | 7.29 | 5 |
| S4 | Mathematics | 4 | 7 | 6 | 10 | 5 | 9 | 5.02 | 8.15 | 6.22 | 3.13 | 1.00 | 2.13 | 6.52 | 7 |
| S5 | Leadership and execution | 5 | 9 | 8 | 10 | 6 | 9 | 6.29 | 9.20 | 7.40 | 2.91 | 1.00 | 1.91 | 8.31 | 1 |
| S6 | Innovation | 5 | 9 | 9 | 10 | 7 | 9 | 6.89 | 9.54 | 7.87 | 2.65 | 0.00 | 2.65 | 8.22 | 2 |
| S7 | Ethics and law | 5 | 9 | 9 | 10 | 7 | 9 | 6.39 | 9.43 | 7.64 | 3.04 | 0.00 | 3.04 | 7.91 | 3 |
| Si | R(Si) | A(Si) | R(si) ∩ A(si) |
|---|---|---|---|
| S1 | 1,2,3,4,5,6,7 | 1,4 | 1,4 |
| S2 | 2,3,5,6,7 | 1,2,3,4 | 2,3 |
| S3 | 2,3,5,6,7 | 1,2,3,4 | 2,3 |
| S4 | 1,2,3,4,5,6,7 | 1,4 | 1,4 |
| S5 | 5 | 1,2,3,4,5,6,7 | 5 |
| S6 | 5,6 | 1,2,3,4,6 | 6 |
| S7 | 5,7 | 1,2,3,4,7 | 7 |
| Si | R(Si) | A(Si) | R(si) ∩ A(si) |
|---|---|---|---|
| S1 | 1,2,3,4,6,7 | 1,4 | 1,4 |
| S2 | 2,3,6,7 | 1,2,3,4 | 2,3 |
| S3 | 2,3,6,7 | 1,2,3,4 | 2,3 |
| S4 | 1,2,3,4,6,7 | 1,4 | 1,4 |
| S6 | 6 | 1,2,3,4,6 | 6 |
| S7 | 7 | 1,2,3,4,7 | 7 |
| Si | R(Si) | A(Si) | R(si) ∩ A(si) |
|---|---|---|---|
| S1 | 1,2,3,4 | 1,4 | 1,4 |
| S2 | 2,3 | 1,2,3,4 | 2,3 |
| S3 | 2,3 | 1,2,3,4 | 2,3 |
| S4 | 1,2,3,4 | 1,4 | 1,4 |
| Si | R(Si) | A(Si) | R(si) ∩ A(si) |
|---|---|---|---|
| S1 | 1,4 | 1,4 | 1,4 |
| S4 | 1,4 | 1,4 | 1,4 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hsu, W.-L.; Chen, Y.-S.; Shiau, Y.-C.; Liu, H.-L.; Chern, T.-Y. Curriculum Design in Construction Engineering Departments for Colleges in Taiwan. Educ. Sci. 2019, 9, 65. https://doi.org/10.3390/educsci9010065
Hsu W-L, Chen Y-S, Shiau Y-C, Liu H-L, Chern T-Y. Curriculum Design in Construction Engineering Departments for Colleges in Taiwan. Education Sciences. 2019; 9(1):65. https://doi.org/10.3390/educsci9010065
Chicago/Turabian StyleHsu, Wei-Ling, Yi-Sian Chen, Yan-Chyuan Shiau, Hsin-Lung Liu, and Tian-Yow Chern. 2019. "Curriculum Design in Construction Engineering Departments for Colleges in Taiwan" Education Sciences 9, no. 1: 65. https://doi.org/10.3390/educsci9010065
APA StyleHsu, W.-L., Chen, Y.-S., Shiau, Y.-C., Liu, H.-L., & Chern, T.-Y. (2019). Curriculum Design in Construction Engineering Departments for Colleges in Taiwan. Education Sciences, 9(1), 65. https://doi.org/10.3390/educsci9010065








