The Impact of a MOOC: Long-Term Analysis of Teachers’ Learning Outcomes and Practices
Abstract
1. Introduction and Literature Review
“[…] TPACK is the basis of effective teaching with technology, requiring an understanding of the representation of concepts using technologies; pedagogical techniques that use technologies in constructive ways to teach content; knowledge of what makes concepts difficult or easy to learn and how technology can help redress some of the problems that students face; knowledge of students’ prior knowledge and theories of epistemology; and knowledge of how technologies can be used to build on existing knowledge to develop new epistemologies or strengthen old ones”.
Professional Development in Outdoor Mathematics
- Level 1 (Recognizing): Acknowledgement of the usefulness of technology, but no actual use in assessment.
- Level 2 (Accepting): Desire to integrate technology, but challenges exist when connecting it to the contents.
- Level 3 (Adapting): Noticeable adjustments in the pedagogy to test technology, also in assessment.
- Level 4 (Exploring): Seeking more ways to integrate technology throughout the curriculum.
- Level 5 (Advancing): Incorporation of technology as an essential and regular part of teaching and assessment tool.
2. Theoretical Framework
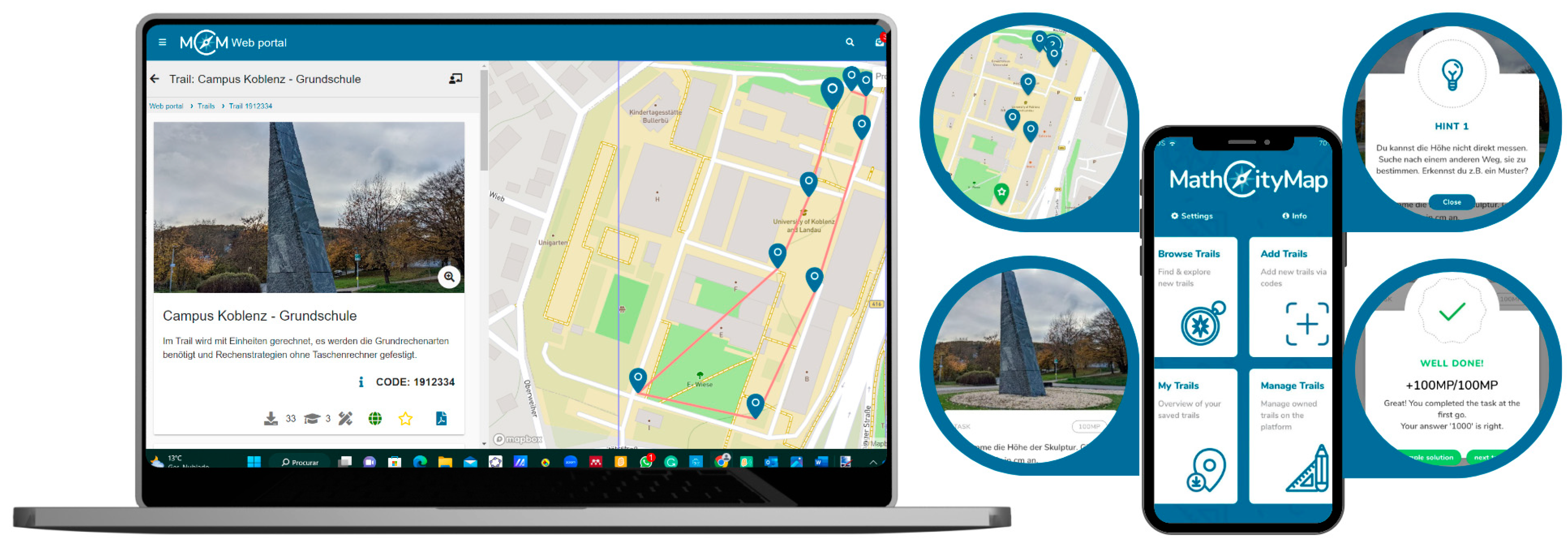
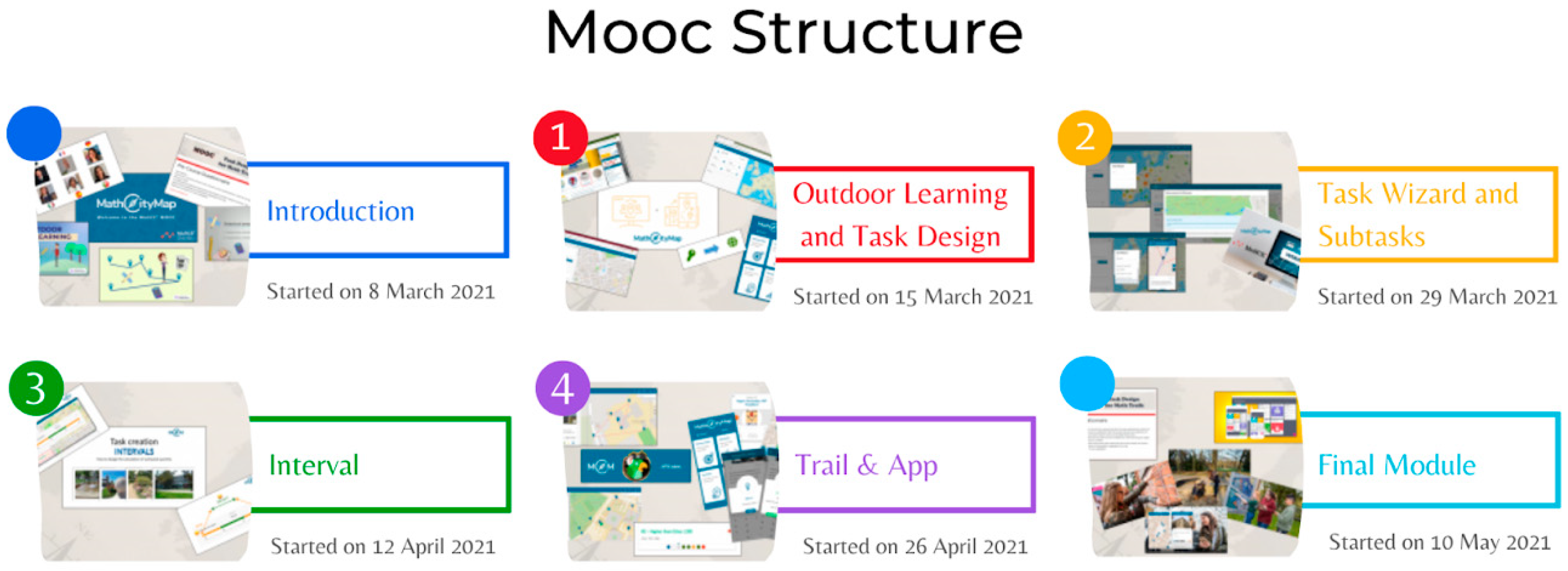
3. The MaSCE3 MOOC
4. Research Design
4.1. Initial Learning Outcomes Related to the MaSCE3 MOOC
- The changes in the attitude towards outdoor mathematics;
- The learning progress and usage concerning the digital features of MCM;
- The MOOC ratings.
4.2. Research Question
During the year following the end of the MOOC, to what extent did the internalisation process take place in the teachers’ community regarding the pragmatic and theoretical components of praxeology concerning the incorporation of the MathCityMap system into their teaching practices?
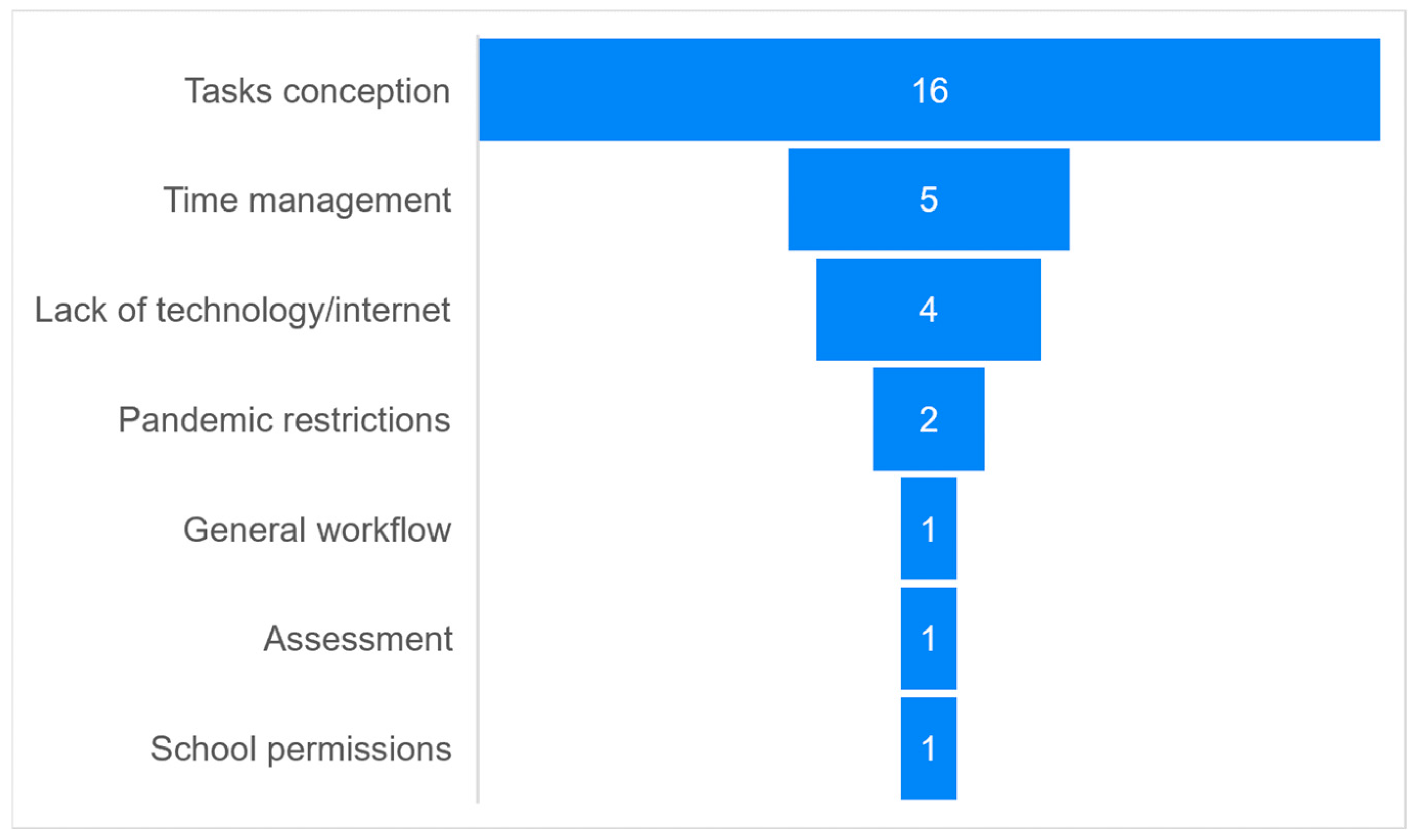
5. Methodology and Data Analysis
- Task: propose an outdoor math trail to the students;
- Technique: designing the math trail with MCM; encouraging students to use the MCM App to enjoy the trail; monitoring students with the Digital Classroom during the use of the trail;
- Justification: using MCM simplifies the teacher’s design load and the Digital Classroom allows the class to be in control even in an external environment;
- Theory: practising mathematics outdoors with one’s students.
5.1. Perceptions About the Usability of MCM Web Portal and Skills’ Awareness
- Factor Dimension 1 (factor_usage): Standardised loadings for u1, u2, u3, and u4 (the corresponding usage items, stated in Table 4) were 1.000, 0.819, 1.150, and 0.941, respectively (p < 0.001).
- Factor Dimension 2 (factor_learnability): Loadings of 1.000, 0.850, and 1.252 for items l1, l2, and l3 (the corresponding learnability items, stated in Table 4), respectively (p < 0.001).
5.2. Reliability
6. Results
6.1. Usability of the MCM Web Portal
6.2. Teachers’ Awareness of Skills
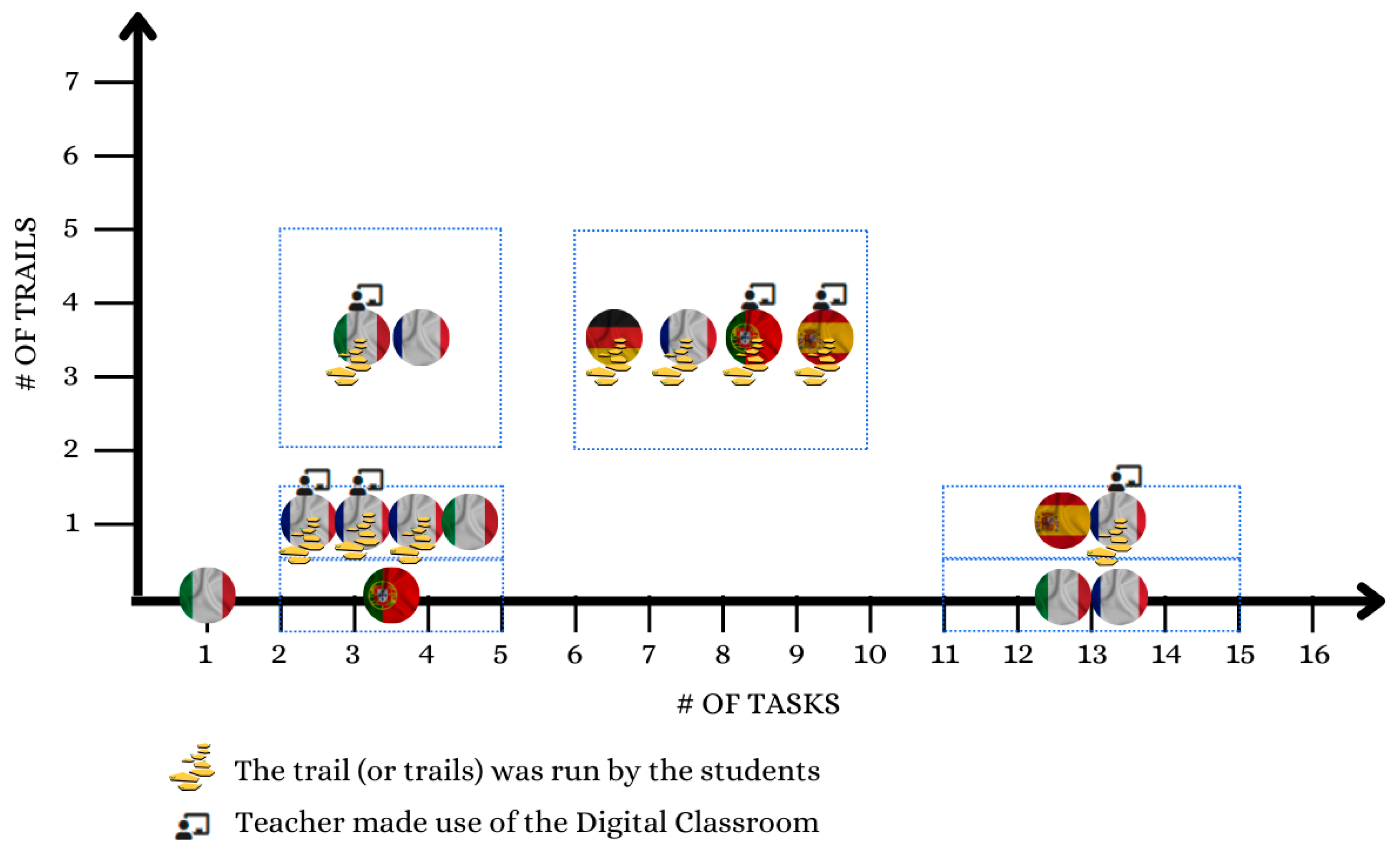
6.3. Activities During the Year After the MOOC
7. Discussion
- Task: learning to design math trail tasks in order to create a math trail;
- Technique: solving the task using MCM;
- Justification: making use of MCM simplifies the teacher’s design load and the Digital Classroom allows the class to be in control even in an external environment;
- Theory: practicing mathematics outdoors with your own students.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
| 1. | To allow the reader to have an understanding of the data, we report here the categories and the data analyzed (taken from Taranto et al., 2021) relating to the initial phases of the MOOC (pre) and after completing the MOOC (post). Designing math tasks for outdoor mathematics: Pre: 8/19 and Post: 17/19; Designing math trails for outdoor mathematics: Pre: 8/19 and Post: 16/19; Using MCM web portal: Pre: 8/19 and Post: 19/19; Using MCM app: Pre: 8/19 and Post: 18/19. |
| 2. | Note that this meta-didactical praxeology is similar to that in Table 1, but more detailed, in light of the clarifications on MCM made in the previous paragraphs. |
References
- Anderson, R. K. (2020). Social media facilitated collaboration: An analysis of in-the-moment support in a mathematics education Facebook group. In H. Borko, & D. Potari (Eds.), Proceedings of the twenty-fifth ICMI study (Lisbon, Portugal) (pp. 581–588). National and Kapodistrian University of Athens. [Google Scholar]
- Arzarello, F., Robutti, O., Sabena, C., Cusi, A., Garuti, R., Malara, N., & Martignone, F. (2014). Meta-didactical transposition: A theoretical model for teacher education programmes. In A. Clark-Wilson, O. Robutti, & N. Sinclair (Eds.), The mathematics teacher in the digital era: An international perspective on technology focused professional development, vol. 2 of the series: Mathematics education in the digital era (pp. 347–372). Springer. [Google Scholar] [CrossRef]
- Bujang, M. A., Omar, E. D., & Baharum, N. (2018). A review on sample size determination for Cronbach’s alpha test: A simple guide for researchers. The Malaysian Journal of Medical Sciences: MJMS, 25, 85–99. [Google Scholar] [CrossRef]
- Cahyono, A. N., Masrukan, Mulyono, Ludwig, M., Jablonski, S., & Oehler, D.-X. K. (2023). Indonesia-Germany MathCityMap training: Shifting mobile math trails teacher training to a hybrid environment. Journal on Mathematics Education, 14(1), 55–68. [Google Scholar] [CrossRef]
- Cai, J., & Howson, G. (2013). Toward an international mathematics curriculum. In M. A. (Ken) Clements, A. J. Bishop, C. Keitel, J. Kilpatrick, & F. K. S. Leung (Eds.), Third international handbook of mathematics education (pp. 949–974). Springer. [Google Scholar] [CrossRef]
- Chevallard, Y. (1999). L’analyse des pratiques enseignantes en théorie anthropologique du didactique. Recherches en Didactique des Mathématiques, 19(2), 221–265. [Google Scholar]
- Clarke, D. (2007). Ten key principles from research for the professional development of mathematics teachers. In G. C. Leder, & H. Forgasz (Eds.), Stepping stones for the 21st Century (pp. 27–39). Brill. [Google Scholar] [CrossRef]
- Cusi, A., Robutti, O., Panero, M., Taranto, E., & Aldon, G. (2022). Meta-Didactical Transposition. 2: The evolution of a framework to analyse teachers’ collaborative work with researchers in technological settings. In A. Clark-Wilson, N. Sinclair, & O. Robutti (Eds.), The mathematics teacher in the digital era. Mathematics education in the digital era (Vol. 16, pp. 365–389). Springer. [Google Scholar] [CrossRef]
- Dunn, T. J., Baguley, T., & Brunsden, V. (2014). From alpha to omega: A practical solution to the pervasive problem of internal consistency estimation. British Journal of Psychology (London, England: 1953), 105(3), 399–412. [Google Scholar] [CrossRef]
- Edwards, A. A., Joyner, K. J., & Schatschneider, C. (2021). A simulation study on the performance of different reliability estimation methods. Educational and Psychological Measurement, 81(6), 1089–1117. [Google Scholar] [CrossRef] [PubMed]
- Goodboy, A. K., & Martin, M. M. (2020). Omega over alpha for reliability estimation of unidimensional communication measures. Annals of the International Communication Association, 44(4), 422–439. [Google Scholar] [CrossRef]
- Gurjanow, I., Ludwig, M., & Zender, J. (2018). Aids and obstacles in the use of ICT-two surveys amongst MCM users. In F. J. Hsieh (Ed.), Proceedings of the 8th ICMI east Asia regional conference on mathematics education (Vol. 1, pp. 463–471). EARCOME. [Google Scholar]
- Hollebrands, K. F., & Lee, H. S. (2020). Effective design of massive open online courses for mathematics teachers to support their professional learning. ZDM Mathematics Education, 52(5), 859–875. [Google Scholar] [CrossRef]
- Jablonski, S. (2023). Is it all about the setting?—A comparison of mathematical modelling with real objects and their representation. Educational Studies in Mathematics, 113, 307–330. [Google Scholar] [CrossRef]
- Jablonski, S., & Ludwig, M. (2020). Development of an intensive study programme on outdoor mathematics teaching with digital tools. In M. Ludwig, S. Jablonski, A. Caldeira, & A. Moura (Eds.), Research on outdoor STEM education in the digiTal age. Proceedings of the ROSETA online conference in June 2020 (pp. 111–118). WTM. [Google Scholar] [CrossRef]
- Koehler, M. J., & Mishra, P. (2005). What happens when teachers design educational technology? The development of technological pedagogical content knowledge. Journal of Educational Computing Research, 32(2), 131–152. [Google Scholar] [CrossRef]
- Koehler, M. J., & Mishra, P. (2009). What is technological pedagogical content knowledge (TPACK)? Contemporary Issues in Technology and Teacher Education, 9(1), 60–70. [Google Scholar] [CrossRef]
- Ludwig, M., & Jesberg, J. (2015). Using mobile technology to provide outdoor modelling tasks—The MathCityMap-project. In Procedia proceedings of WCES (pp. 2776–2781). Elsevier. [Google Scholar]
- Macià, M., & García, I. (2016). Informal online communities and networks as a source of teacher professional development: A review. Teaching and Teacher Education, 55, 291–307. [Google Scholar] [CrossRef]
- Milicic, G., Jablonski, S., & Ludwig, M. (2020, November 9–10). Teacher training for outdoor education—Curricula development for the MathCityMap system. ICERI2020 Proceedings (pp. 3514–3522), Online. [Google Scholar]
- OECD. (2009). Creating effective teaching and learning environments: First results from TALIS. Available online: https://www.oecd.org/en/publications/creating-effective-teaching-and-learning-environments_9789264068780-en.html (accessed on 3 March 2025).
- Orçan, F. (2023). Comparison of Cronbach’s alpha and McDonald’s omega for ordinal data: Are they different? International Journal of Assessment Tools in Education, 10(4), 709–722. [Google Scholar] [CrossRef]
- Panero, M., Aldon, G., Trgalová, J., & Trouche, L. (2017, February 1–5). Analysing MOOCs in terms of teacher collaboration potential and issues: The French experience. Congress of European Research in Mathematics Education (pp. 2446–2453), Dublin, Ireland. [Google Scholar]
- Paulsen, J., & BrckaLorenz, A. (2017). Internal consistency. FSSE psychometric portfolio. Available online: https://nsse.indiana.edu/fsse/ (accessed on 3 March 2025).
- Pimenta, P., Domingo, A., & Costa, M. C. (2023, July 10–14). Sustainability of professional development programmes to teach mathematics using technology. 13th Congress of the European Society for Research in Mathematics Education (CERME), Budapest, Hungary. [Google Scholar]
- Rakes, C. R., Stites, M. L., Ronau, R. N., Bush, S. B., Fisher, M. H., Safi, F., Desai, S., Schmidt, A., Andreasen, J. B., Saderholm, J., Amick, L., Mohr-Schroeder, M. J., & Viera, J. (2022). Teaching mathematics with technology: TPACK and effective teaching practices. Education Sciences, 12(2), 133. [Google Scholar] [CrossRef]
- Ratnayake, I., Thomas, M., & Kensington-Miller, B. (2020). Professional development for digital technology task design by secondary mathematics teachers. ZDM Mathematics Education, 52, 1423–1437. [Google Scholar] [CrossRef]
- Ricart, M., Estrada, A., & Fortuny, J. M. (2022). Analysis of tutoring in the professional development of STEM teachers. Mathematics, 10(18), 3331. [Google Scholar] [CrossRef]
- Robutti, O. (2018). Meta-didactical transposition. In S. Lerman (Ed.), Encyclopedia of mathematics education. Springer. [Google Scholar] [CrossRef]
- Rogers, M. P., Abell, S., Lannin, J., Wang, C.-Y., Musikul, K., & Barker, D. (2007). Effective professional development in science and mathematics education: Teachers’ and facilitators’ views. International Journal of Science and Mathematics Education, 5, 507–532. [Google Scholar] [CrossRef]
- Taber, K. S. (2017). The use of Cronbach’s alpha when developing and reporting research instruments in science education. Research in Science Education, 48, 6. [Google Scholar] [CrossRef]
- Taranto, E., Aldon, G., Robutti, O., & Cusi, A. (2023). Design of resources for and by mathematics teachers: The process of internalization in MOOCs. In B. Pepin, G. Gueudet, & J. Choppin (Eds.), Handbook of digital resources in mathematics education. Springer international handbooks of education. Springer. [Google Scholar] [CrossRef]
- Taranto, E., & Arzarello, F. (2020). Math MOOC UniTo: An Italian project on MOOCs for mathematics teacher education, and the development of a new theoretical framework. ZDM—The International Journal on Mathematics Education, 52(5), 843–858. [Google Scholar] [CrossRef]
- Taranto, E., Jablonski, S., Recio, T., Mercat, C., Cunha, E., Lázaro, C., Ludwig, M., & Mammana, M. F. (2021). Professional development in mathematics education—Evaluation of a MOOC on outdoor mathematics. Mathematics, 9(22), 2975. [Google Scholar] [CrossRef]
- Taranto, E., Robutti, O., & Arzarello, F. (2020). Learning within MOOCs for mathematics teacher education. ZDM—The International Journal on Mathematics Education, 52(7), 1439–1453. [Google Scholar] [CrossRef]
- Thomas, M. O. J., & Chinnappan, M. (2008). Teaching and learning with technology: Realising the potential. In H. Forgasz, A. Barkatsas, A. Bishop, B. Clarke, S. Keast, W.-T. Seah, P. Sullivan, & S. Willis (Eds.), Research in mathematics education in Australasia 2004–2007 (pp. 167–194). Sense Publishers. [Google Scholar]
- Viladrich, C., Angulo-Brunet, A., & Doval, E. (2017). A journey around alpha and omega to estimate internal consistency reliability. Anales de Psicología/Annals of Psychology, 33(3), 755–782. [Google Scholar] [CrossRef]
- Zehetmeier, S., & Krainer, K. (2011). Ways of promoting the sustainability of mathematics teachers’ professional development. ZDM—International Journal on Mathematics Education, 43(6), 875–887. [Google Scholar] [CrossRef]
- Zender, J., Gurjanow, I., Cahyono, A. N., & Ludwig, M. (2020). New studies in mathematics trails. International Journal of Studies in Education and Science (IJSES), 1(1), 1–14. [Google Scholar]

| Praxeology | Didactical praxeology (of a teacher in a classroom) | Meta-didactical praxeology (of a teacher in professional development) | ||
| Pragmatic Components | Task | Introducing students to the type of task (e.g., solving a second-degree equation) | Solving an assigned didactical task (e.g., learning to design math trail tasks in order to create a math trail) | |
| Technique | How to organise such an approach (e.g., second-degree equation solution formulas) | How to solve it (e.g., solving the task using the MathCityMap (MCM) system) | ||
| Theoretical Components | Justification | Why one has to organise it like that (e.g., the justification of the formula for second-degree equations through the completion of squares or even zero-product property) | Why one has to solve in such a way (e.g., making use of MCM simplifies the teacher’s design and monitoring load) | |
| Theory | Why one knows that one has to organise it like that (e.g., the theory of algebraic equations) | Why one knows that one has to solve it like that (e.g., practising mathematics outdoors with own students) |
| Country | Number of Participants Who Finished the MOOC |
|---|---|
| France | 8 |
| Italy | 11 |
| Uruguay | 1 |
| Greece | 1 |
| Portugal | 6 |
| Spain | 3 |
| United Kingdom | 1 |
| Germany | 1 |
| Slovakia | 1 |
| Total | 33 |
| #. Question Block | Example of Items | Number of Questions/Items |
|---|---|---|
| 1. General Information | What country do you currently live in? | 8 |
| 2. MOOC Completion | Have you completed the MOOC? | 3 |
| 3. Experiences with MCM one year after the end of the MOOC (tasks and trails) | During this year, how did you make use of MCM? | 8 |
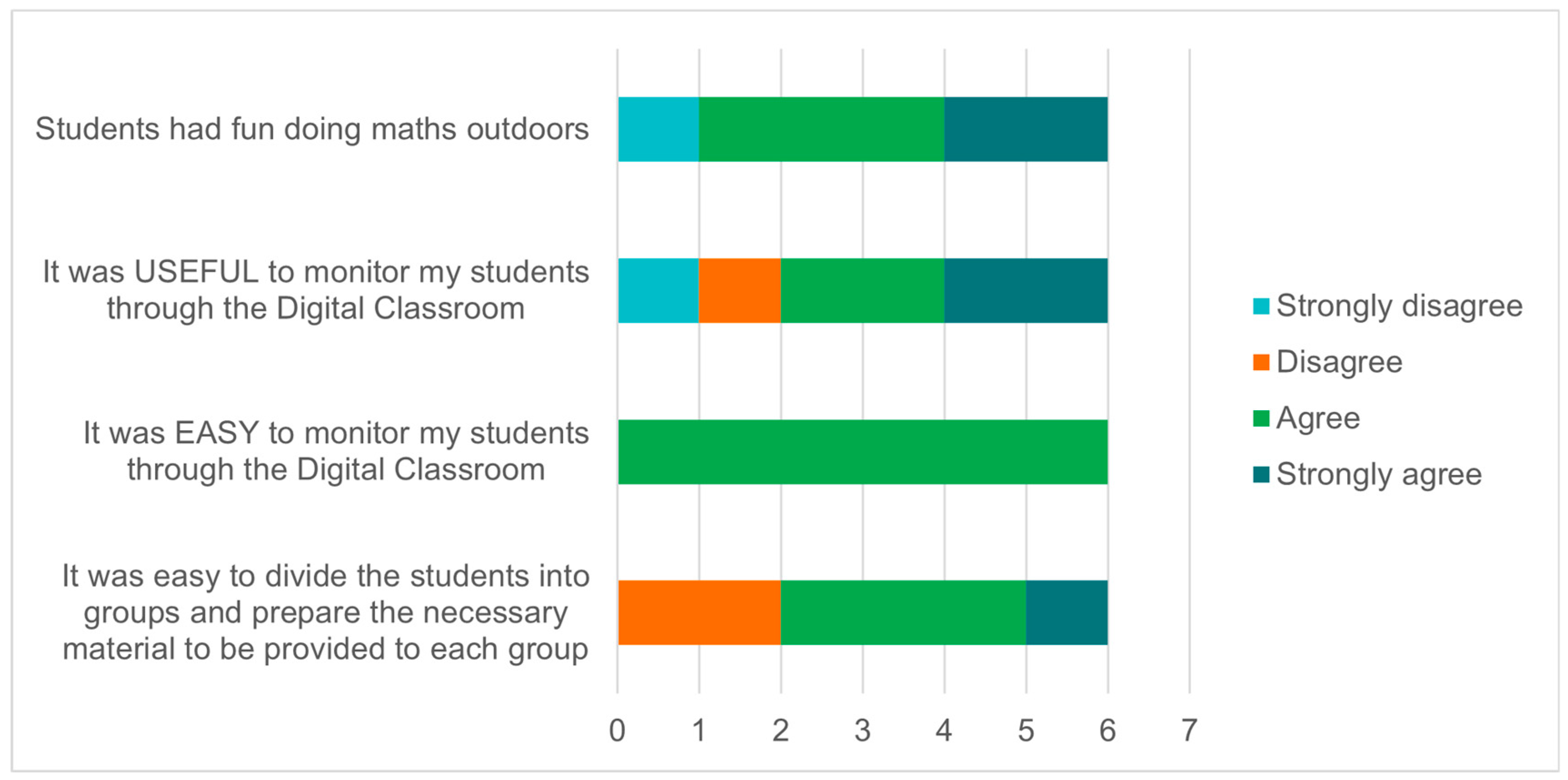
| 4. Digital Classroom | Please indicate your level of agreement with the following statements, remembering how you used to work: It was easy to monitor my students through the Digital Classroom (from 1 = Strongly disagree to 4 = Strongly agree). | 24 |
| 5. Opinions on the MCM web portal and app | Which of the following answer formats do you feel most comfortable with when designing MCM tasks? | 23 |
| 6. Review system | The review times have been carried out in a short time. | 6 |
| 7. A year ago (directly after the MOOC): awareness of own skills | Rate your awareness of your skills in designing math tasks to practice maths outdoors | 4 |
| 8. Today, one year after the end of the MOOC: awareness of own skills and future intentions | Are you planning to use MathCityMap in the future? | 8 |
| Construct | Dimensions | Items |
|---|---|---|
| Teachers’ perceptions about the usability of the MCM web portal | Usage | u1—I can orientate myself in the web portal |
| u2—Creating tasks is intuitive | ||
| u3—Creating trails is intuitive | ||
| u4—With MCM, it became easier for me to create outdoor tasks | ||
| Learnability | l1—It was easy for me to learn how the MCM web portal works | |
| l2—The interaction between the web portal and app was easy to understand | ||
| l3—It was easy for me to learn how Digital Classroom works |
| Estimate | Std.Err | z-Value | p (>|z|) | |
|---|---|---|---|---|
| factor_usage = ~ | ||||
| u1 | 1.000 | |||
| u2 | 0.819 | 0.178 | 4.597 | 0.000 |
| u3 | 1.150 | 0.210 | 5.481 | 0.000 |
| u4 | 0.941 | 0.224 | 4.198 | 0.000 |
| factor_learnability = ~ | ||||
| l1 | 1.000 | |||
| l2 | 0.850 | 0.161 | 5.268 | 0.000 |
| l3 | 1.252 | 0.211 | 5.939 | 0.000 |
| Construct: Teachers’ Perceptions | Dimensions | # Items | Omega | Cronbach’s Alpha | Inter-Item Correlation | Average Inter-Item Correlations | |
|---|---|---|---|---|---|---|---|
| Minimum | Maximum | ||||||
| The usability of the MCM web portal | Usage | 4 | 0.817 | 0.821 | 0.376 | 0.766 | 0.541 |
| Learnability | 3 | 0.853 | 0.829 | 0.573 | 0.736 | 0.635 | |
| Awareness of own skills—A year ago, when the teachers finished the MOOC | 4 | 0.957 | 0.958 | 0.801 | 0.964 | 0.854 | |
| Awareness of own skills—One year after the MOOC | 4 | 0.957 | 0.958 | 0.8 | 0.92 | 0.852 | |
| Dimensions | Variables | Response | Frequency | Percent | Valid Percent |
|---|---|---|---|---|---|
| Usage | I can orientate myself in the web portal | 3-Agree | 20 | 51.3 | 51.3 |
| 4-Strongly Agree | 19 | 48.7 | 48.7 | ||
| Total | 39 | 100 | 100 | ||
| Creating tasks is intuitive | 3-Agree | 25 | 64.1 | 64.1 | |
| 4-Strongly Agree | 14 | 35.9 | 35.9 | ||
| Total | 39 | 100 | 100 | ||
| Creating trails is intuitive | 2-Disagree | 3 | 7.7 | 7.7 | |
| 3-Agree | 23 | 59 | 59 | ||
| 4-Strongly Agree | 13 | 33.3 | 33.3 | ||
| Total | 39 | 100 | 100 | ||
| With MCM, it became easier for me to create outdoor tasks | 2-Disagree | 2 | 5.1 | 5.1 | |
| 3-Agree | 16 | 41 | 41 | ||
| 4-Strongly Agree | 21 | 53.8 | 53.8 | ||
| Total | 39 | 100 | 100 | ||
| Learnability | It was easy for me to learn how the MCM web portal works | 2-Disagree | 2 | 5.1 | 5.1 |
| 3-Agree | 23 | 59 | 59 | ||
| 4-Strongly Agree | 14 | 35.9 | 35.9 | ||
| Total | 39 | 100 | 100 | ||
| The interaction between the web portal and app was easy to understand | 2-Disagree | 3 | 7.7 | 7.7 | |
| 3-Agree | 24 | 61.5 | 61.5 | ||
| 4-Strongly Agree | 12 | 30.8 | 30.8 | ||
| Total | 39 | 100 | 100 | ||
| It was easy for me to learn how Digital Classroom works | 1-Strongly Disagree | 1 | 2.6 | 2.6 | |
| 2-Disagree | 10 | 25.6 | 25.6 | ||
| 3-Agree | 18 | 46.2 | 46.2 | ||
| 4-Strongly Agree | 10 | 25.6 | 25.6 | ||
| Total | 39 | 100 | 100 |
| Test Statistics a | ||||
|---|---|---|---|---|
| Q1_Present—Q1_Retro | Q2_Present—Q2_Retro | Q3_Present—Q3_Retro | Q4_Present—Q4_Retro | |
| Z | −0.577 b | −0.500 b | −0.258 c | 0.000 d |
| Asymp. Sig. (2-tailed) | 0.564 | 0.617 | 0.796 | 1.000 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Taranto, E.; Jablonski, S.; Recio, T.; Cunha, E.; Ludwig, M.; Mammana, M.F. The Impact of a MOOC: Long-Term Analysis of Teachers’ Learning Outcomes and Practices. Educ. Sci. 2025, 15, 336. https://doi.org/10.3390/educsci15030336
Taranto E, Jablonski S, Recio T, Cunha E, Ludwig M, Mammana MF. The Impact of a MOOC: Long-Term Analysis of Teachers’ Learning Outcomes and Practices. Education Sciences. 2025; 15(3):336. https://doi.org/10.3390/educsci15030336
Chicago/Turabian StyleTaranto, Eugenia, Simone Jablonski, Tomas Recio, Elisabete Cunha, Matthias Ludwig, and Maria Flavia Mammana. 2025. "The Impact of a MOOC: Long-Term Analysis of Teachers’ Learning Outcomes and Practices" Education Sciences 15, no. 3: 336. https://doi.org/10.3390/educsci15030336
APA StyleTaranto, E., Jablonski, S., Recio, T., Cunha, E., Ludwig, M., & Mammana, M. F. (2025). The Impact of a MOOC: Long-Term Analysis of Teachers’ Learning Outcomes and Practices. Education Sciences, 15(3), 336. https://doi.org/10.3390/educsci15030336








